Long-Term Changes in Water and Ion Flows of the Pechora River, the Longest Full-Water European Arctic River
Abstract
:1. Introduction
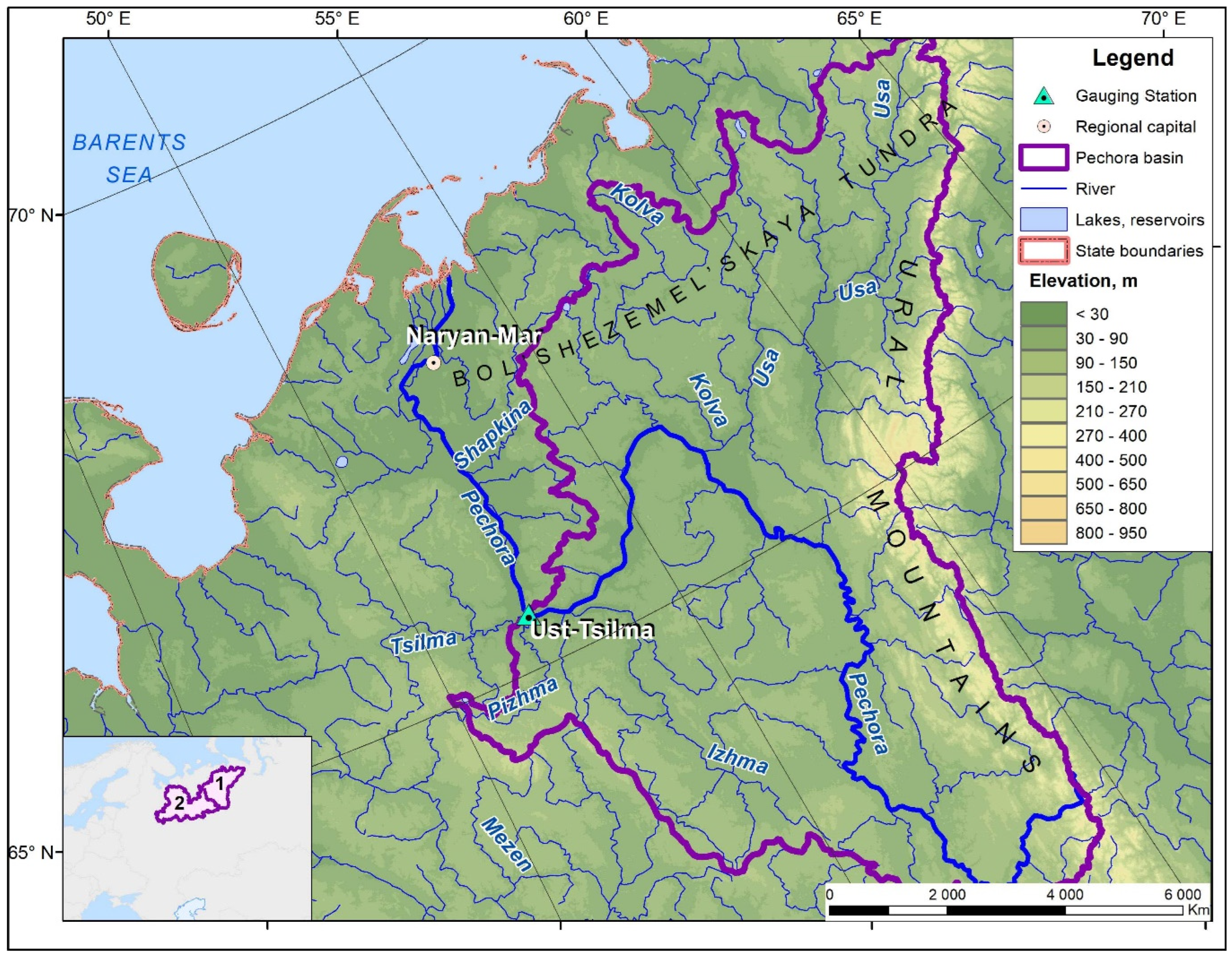
1.1. Characteristics of the Pechora River Basin
1.2. Hydrochemical Characteristics of the Pechora River at Ust’-Tsil’ma Village
2. Materials and Methods
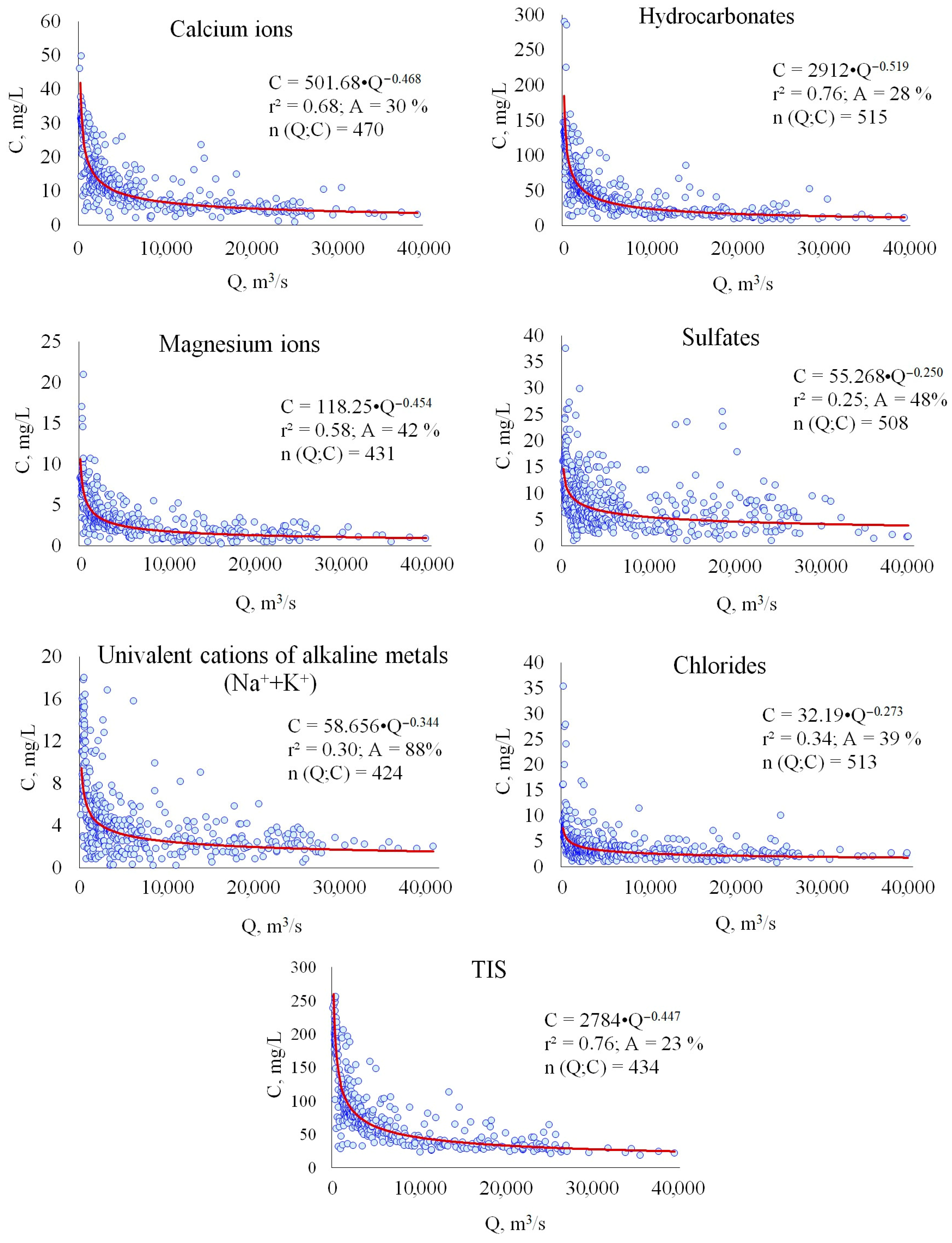
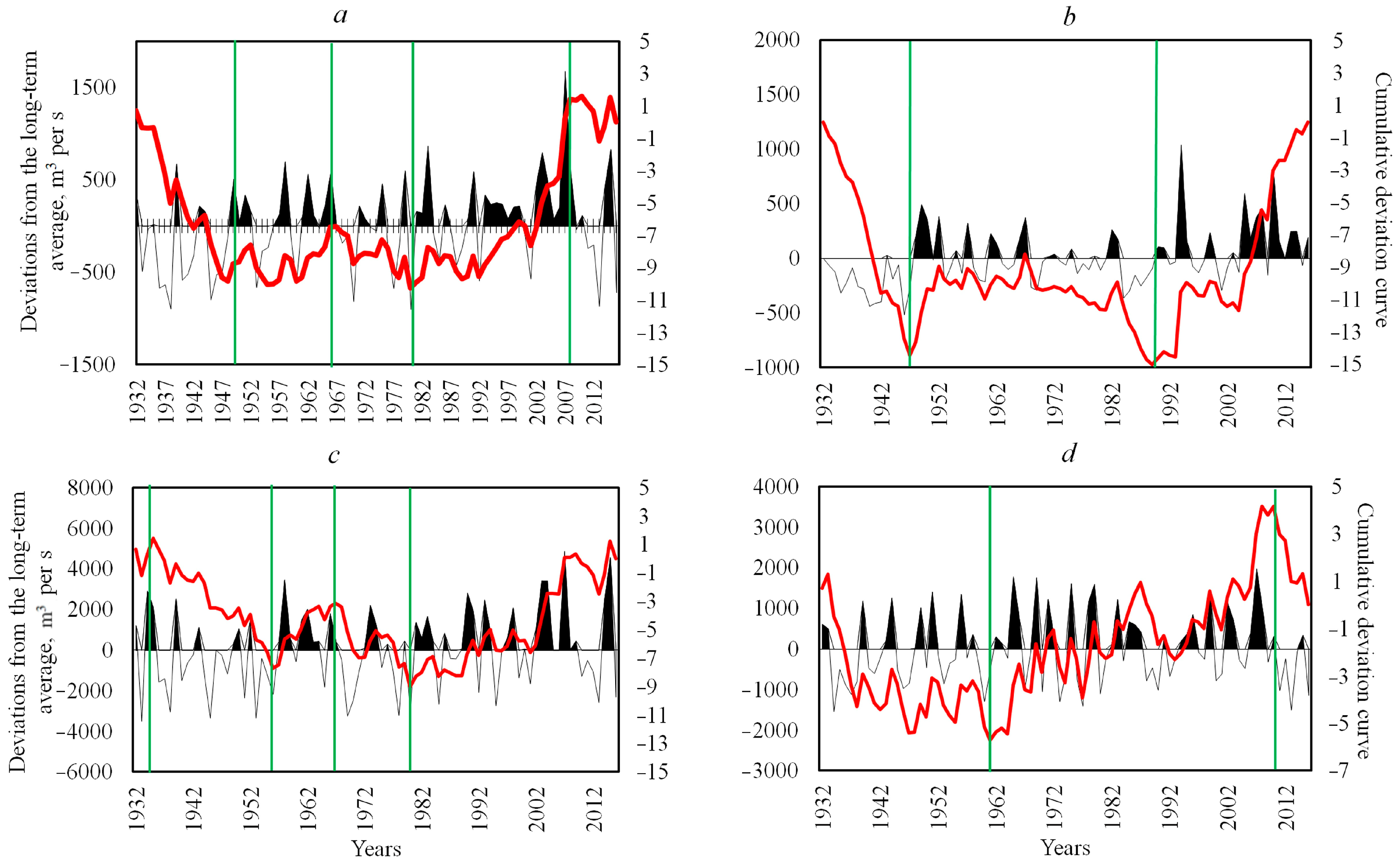
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Second Assessment Report of Climate Change and Its Impacts on the Territory of the Russian Federation; Technical Summary; Roshydromet: Moscow, Russia, 2014; p. 94. (In Russian)
- Borzenkova, I.I.; Vinnikov, K.Y.; Spirina, L.P.; Stekhnovsky, D.I. Changes of the Surface Air Temperature of the Northern Hemisphere during the 1881–1975 Period. Sov. Meteorol. Hydrol. 1976, 7, 21–29. [Google Scholar]
- Budyko, M.I.; Vinnikov, K.Y. Global Warming. Sov. Meteorol. Hydrol. 1976, 7, 12–20. [Google Scholar]
- Reid, P.C.; Hari, R.E.; Beaugrand, G.; Livingstone, D.M.; Marty, C.; Straile, D.; Barichivich, J.; Goberville, E.; Adrian, R.; Aono, Y.; et al. Global Impacts of the 1980s Regime Shift. Glob. Change Biol. 2016, 22, 682–703. [Google Scholar] [CrossRef] [PubMed]
- Core Writing Team; Lee, H.; Romero, J. (Eds.) Climate Change 2023: Synthesis Report; Intergovernmental Panel on Climate Change: Geneva, Switzerland, 2023; pp. 1–34. [Google Scholar]
- National Snow and Ice Data Center (NSIDC) 2024: Arctic Sea Ice News and Analysis|Sea Ice Data Updated Daily with One-Day Lag (nsidc.org). Available online: https://nsidc.org/arcticseaicenews (accessed on 13 March 2024).
- Kacimi, S.; Kwok, R. Arctic Snow Depth, Ice Thickness, and Volume from ICESat-2 and CryoSat-2: 2018–2021. Geophys. Res. Lett. 2022, 49, e2021GL097448. [Google Scholar] [CrossRef]
- Zhang, P.; Chen, G.; Ting, M.; Leung, L.R.; Guan, B.; Li, L. More Frequent Atmospheric Rivers Slow the Seasonal Recovery of Arctic Sea Ice. Nat. Clim. Change 2023, 13, 266–273. [Google Scholar] [CrossRef]
- Ma, W.; Chen, G.; Peings, Y.; Alviz, N. Atmospheric River Response to Arctic Sea Ice Loss in the Polar Amplification Model Intercomparison Project. Geophys. Res. Lett. 2021, 48, e2021GL094883. [Google Scholar] [CrossRef]
- Ford, J.D.; Pearce, T.; Canosa, I.V.; Harper, S. The Rapidly Changing Arctic and Its Societal Implications. Wiley Interdiscip. Rev. Clim. Change 2021, 12, e735. [Google Scholar] [CrossRef]
- Muraveisky, S.D. Rivers and Lakes, Hydrobiology, Runoff; Geografgiz: Moscow, Russia, 1960; p. 387. (In Russian) [Google Scholar]
- Shiklomanov, I.A. Water Resources of Russia and Their Use (WRRU); State Hydrological Institute: St. Petersburg, Russia, 2008; p. 600. (In Russian) [Google Scholar]
- Goldman, C.R.; Kumagai, M.; Robarts, R.D. Climatic Change and Global Warming of Inland Waters: Impacts and Mitigation for Ecosystems and Societies; Wiley-Blackwell: Malden, MA, USA, 2013; p. 496. [Google Scholar]
- Smirnov, M.P. Organic Substances and Mineralization of River Waters in Russia, the CIS Countries, the Baltic Countries; NOK: Rostov-on-Don, Russia, 2015; p. 358. (In Russian) [Google Scholar]
- Magritsky, D.V.; Frolova, N.L.; Evstigneev, V.M.; Povalishnikova, E.S.; Kireeva, M.B.; Pakhomova, O.M. Long-Term Changes of River Water Inflow into the Seas of the Russian Arctic Sector. Polarforschung 2018, 87, 177–194. [Google Scholar]
- Georgiadi, A.G.; Kashutina, E.A.; Milyukova, I.P. Long-Term Changes of Water Flow, Water Temperature and Heat Flux of the Largest Siberian Rivers. Polarforschung 2018, 87, 167–176. [Google Scholar]
- Groisman, P.Y.; Gutman, G. Regional Environmental Changes in Siberia and Their Global Consequences; Springer Science & Business Media: Berlin, Germany, 2013; p. 369. [Google Scholar]
- Yang, D.; Kane, D. Arctic Hydrology, Permafrost and Ecosystems; Springer International Publishing: New York, NY, USA, 2021; p. 914. [Google Scholar]
- Andreyanov, V.G. Cyclical fluctuations of annual runoff and their accounting in hydrological calculations problems of runoff calculations. Proc. State Hydrol. Inst. 1959, 68, 3–50. (In Russian) [Google Scholar]
- North, R.P.; Livingstone, D.M.; Hari, R.E.; Köster, O.; Niederhauser, P.; Kipfer, R. The physical impact of the late 1980s climate regime shift on Swiss rivers and lakes. Inland Waters 2013, 3, 341–350. [Google Scholar] [CrossRef]
- Rodionov, S.N.; James, E. Overland application of a sequential regime shift detection method to the Bering Sea ecosystem. ICES J. Mar. Sci. 2005, 62, 328–332. [Google Scholar] [CrossRef]
- Shi, X.; Qin, T.; Nie, H.; Weng, B.; He, S. Changes in Major Global River Discharges Directed into the Ocean. Int. J. Environ. Res. Public Health 2019, 16, 1469. [Google Scholar] [CrossRef] [PubMed]
- Markov, M.L. Scientific and Applied Handbook: Long-Term Variability of Water Resources and the Main Characteristics of the Flow of Rivers in the Russian Federation; OOO “RIAL”: St. Petersburg, Russia, 2021; p. 190. (In Russian) [Google Scholar]
- Georgiadi, A.G.; Koronkevich, N.I.; Barabanova, E.A.; Kashutina, E.A.; Milyukova, I.P. Contribution of climatic and anthropogenic factors to changes in the flow of large rivers of the Russian Plain and Siberia. Dokl. Earth Sci. 2019, 488, 1211–1216. [Google Scholar] [CrossRef]
- Sharma, S.; Singh, P.K. Long term spatiotemporal variability in rainfall trends over the state of Jharkhand, India. Climate 2017, 5, 18. [Google Scholar] [CrossRef]
- Georgiadi, A.G.; Danilenko, A.O. Northern Dvina River: Long Periods of Increased and Decreased Water and Ionic Runoff in the 19th–21st Centuries. Geogr. Nat. Resour. 2022, 43, 149–155. [Google Scholar] [CrossRef]
- Holmes, R.M.; Peterson, B.J.; Gordeev, V.V.; Zhulidov, A.V.; Meybeck, M.; Lammers, R.B.; Vorosmarty, C.J. Flux of nutrients from Russian rivers to the Arctic Ocean: Can we establish a baseline against which to judge future changes. Water Resour. Res. 2000, 36, 2309–2320. [Google Scholar] [CrossRef]
- Dittmar, T.; Kattner, G. The biogeochemistry of the river and shelf ecosystem of the Arctic Ocean: A review. Mar. Chem. 2003, 83, 103–120. [Google Scholar] [CrossRef]
- Gogina, M. Total Runoff of Dissolved Substances from the European Part of Russia into the Arctic Seas. Master’s Thesis, Department of Geography of the Moscow State University, Moscow, Russia, 2004. (In Russian). [Google Scholar] [CrossRef]
- Emmerton, C.A.; Lesack, L.F.W.; Vincent, W.F. Mackenzie River nutrient delivery to the Arctic Ocean and effects of the Mackenzie Delta during open water conditions. Glob. Biogeochem. Cycl. 2008, 22, GB1024. [Google Scholar] [CrossRef]
- Nikanorov, A.M.; Smirnov, M.P.; Klimenko, O.A. Long Term Trends in Total and Anthropogenic Discharge of Organic and Biogenic Substances by Russian Rivers. Water Resour. 2010, 37, 361–371. [Google Scholar] [CrossRef]
- Alekseevsky, N.I. Geoecological State of the Arctic Sea Shore of Russia and Security the Geoecological State of the Arctic Coast of Russia and the Safety of Environmental Management; GEOS: Moscow, Russia, 2007; p. 585. (In Russian) [Google Scholar]
- Zhang, S.-M.; Mu, C.-C.; Li, Z.-L.; Dong, W.-W.; Wang, X.Y.; Streletskaya, I.; Grebenets, V.; Sokratov, S.; Kizyakov, A.; Wu, X.-D. Export of nutrients and suspended solids from major Arctic rivers and their response to permafrost degradation. Adv. Clim. Change Res. 2021, 12, 466–474. [Google Scholar] [CrossRef]
- Chupakov, A.V.; Pokrovsky, O.S.; Moreva, O.Y.; Kotova, E.I.; Vorobyeva, T.Y.; Shirokova, L.S. Export of organic carbon, nutrients and metals by the mid-sized Pechora River to the Arctic Ocean. Chem. Geol. 2023, 632, 121524. [Google Scholar] [CrossRef]
- Gordeev, V.V.; Pokrovsky, O.S.; Zhulidov, A.V.; Filippov, A.S.; Gurtovaya, T.Y.; Holmes, R.M.; Kosmenko, L.S.; McClelland, J.W.; Peterson, B.J.; Tank, S.E. Dissolved Major and Trace Elements in the Largest Eurasian Arctic Rivers: Ob, Yenisey, Lena, and Kolyma. Water 2024, 16, 316. [Google Scholar] [CrossRef]
- Frey, K.E.; McClelland, J.W. Impacts of permafrost degradation on arctic river biogeochemistry. Hydrol. Process. Int. J. 2009, 23, 169–182. [Google Scholar] [CrossRef]
- Frey, K.E.; Siegel, D.I.; Smith, L.C. Geochemistry of west Siberian streams and their potential response to permafrost degradation. Water Resour. Res. 2007, 43, W03406. [Google Scholar] [CrossRef]
- Zhila, I.M.; Alyushinskaya, N.M. Resources of Surface Waters of the USSR. T.3: Northern Territory; Gidrometeoizdat: Leningrad, Russia, 1965; p. 610. (In Russian) [Google Scholar]
- Dobrovolsky, G.V.; Taskaev, A.I.; Zaboeva, I.V. Soil Atlas of the Komi Republic; OOO “Komi Republican Publishing House”: Syktyvkar, Russia, 2010; p. 356. (In Russian) [Google Scholar]
- Alekin, A.O.; Brazhnikova, L.V. Dissolved Substances Flow from the USSR Territory; Nauka: Moscow, Russia, 1964; p. 143. (In Russian) [Google Scholar]
- Nikanorov, A.M. (Ed.) Hydrochemical Atlas; GUGK at the USSR Council of Ministers: Moscow, Russia, 1990; p. 110. [Google Scholar]
- Nikanorov, A.M.; Bryzgalo, V.A. Rivers of Russia. Part II. Rivers of the European North and Siberia (Hydrochemistry and Hydroecology); NOK: Rostov-on-Don, Russia, 2010; p. 200. (In Russian) [Google Scholar]
- Danilenko, A.O.; Reshetnyak, O.S.; Kosmenko, L.S.; Kondakova, M.Y. Changes in the Chemical Denudation Intensity in the River Pechora Catchment under the Influence of Non-Stationary Climate and Economic Activities. Water Ecol. 2020, 4, 38–49. [Google Scholar] [CrossRef]
- Reshetnyak, O.S. Long-Term Variability of the Ion Runoff of Large Rivers in the Arctic Zone of Russia. Bull. High. Educat. Institut. North Caucasus Reg. Nat. Sci. 2021, 3, 80–86. [Google Scholar]
- Savenko, A.V.; Savenko, V.S. Trace Element Composition of the Dissolved Matter Runoff of the Russian Arctic Rivers. Water 2024, 16, 565. [Google Scholar] [CrossRef]
- Alekin, O.A. Fundamentals of Hydrochemistry; Gidrometeoizdat: Leningrad, Russia, 1970; p. 444. (In Russian) [Google Scholar]
- Durov, S.A. Geometric Method in Hydrochemistry; Rostov Book Publishing House: Rostov-on-Don, Russia, 1959; p. 196. (In Russian) [Google Scholar]
- Datsenko, Y.S.; Efimova, L.E.; Zaslavskaya, O.M.; Pakhomova, O.M. Ion Runoff into the Arctic Seas of Russia. Rus. Polar Res. 2016, 1, 12–15. [Google Scholar]
- Boeva, L.V. The Guidelines for the Chemical Analysis of Land Surface Waters, Part 1; NOK: Rostov-on-Don, Russia, 2009; p. 1044. (In Russian) [Google Scholar]
- Kundzewicz, Z.W.; Robson, A.J. Change detection in hydrological records—A review of the methodology/Revue méthodologique de la détection de changements dans les chroniques hydrologiques. Hydrol. Sci. J. 2004, 49, 7–19. [Google Scholar] [CrossRef]
- Garcia, N.O.; Mechoso, C.R. Variability in the discharge of South American rivers and in climate/Variabilité des débits de rivières d’Amérique du Sud et du climat. Hydrol. Sci. J. 2005, 50, 478. [Google Scholar] [CrossRef]
- Fadeev, V.V.; Tarasov, M.N.; Pavelko, V.L. Dependence of Mineralization and Ionic Composition of River Water on Their Water Regime; Gidrometeoizdat: Leningrad, Russia, 1989; p. 173. (In Russian) [Google Scholar]
| Period/Months | River Flow | Dominant Source of Runoff |
|---|---|---|
| November–April | Winter low flow | Groundwater |
| May–June | Spring–summer high flow | Snowmelt water, overland flow |
| July–October | Summer–autumn low flow | Soil–subsoil water and overland flow during rainfall |
| River Water Flow Phases | Snowmelt Flood Flow | Summer–Autumn Flow | Winter Flow | Annual Flow |
|---|---|---|---|---|
| Decreased flow | 1936–1956 (21) 10,767 m3/s | 1933–1961 (29) 3094 m3/s | 1933–1949 (17) 736 m3/s | 1932–1948 (17) 3204 m3/s |
| 1969–1980 (12) 10,708 m3/s | – | – | 1967–1980 (24) 3349 m3/s | |
| Increased flow | 1957–1967 (11) 12,271 m3/s | 1962–2010 (49) 3460 m3/s | 1989–2016 (28) 1036 m3/s | 1949–1966 (18) 3564 m3/s |
| 1981–2015 (25) 12,148 m3/s | – | – | 1981–2008 (28) 3673 m3/s | |
| Phases of water flow close to the long-term mean | – | – | 1950–1988 (39) 885 m3/s | – |
| River Water Flow Phases | Snowmelt Flood Flow | Summer–Autumn Flow | Winter Flow | Annual Flow |
|---|---|---|---|---|
| I | 12,179 | 3460 | 1036 | 3631 |
| D | 10,745 | 3094 | 736 | 3270 |
| I − D, m3/s | 1434 | 367 | 300 | 361 |
| (I − D)/D × 100, % | 13 | 12 | 41 | 11 |
| Water Flow Phase | Ion Runoff (Gaverage ± σ), Million ton Per Year | ||||||
|---|---|---|---|---|---|---|---|
| Ca2+ | Mg2+ | Na+ + K+ | HCO3− | SO42− | Cl− | TIS | |
| Year as a whole | |||||||
| I | 1.11 ± 0.08 | 0.29 ± 0.02 | 0.44 ± 0.04 | 4.17 ± 0.29 | 0.76 ± 0.08 | 0.34 ± 0.03 | 7.33 ± 0.58 |
| D | 0.99 ± 0.10 | 0.25 ± 0.03 | 0.38 ± 0.04 | 3.73 ± 0.39 | 0.66 ± 0.08 | 0.29 ± 0.03 | 6.51 ± 0.69 |
| I − D | 0.12 | 0.04 | 0.06 | 0.44 | 0.1 | 0.05 | 0.82 |
| (I − D)/D × 100, % | 12 | 13 | 14 | 12 | 15 | 15 | 13 |
| Snowmelt flood period | |||||||
| I | 0.38 ± 0.03 | 0.10 ± 0.01 | 0.17 ± 0.02 | 1.36 ± 0.10 | 0.34 ± 0.04 | 0.15 ± 0.02 | 2.59 ± 0.22 |
| D | 0.34 ± 0.04 | 0.09 ± 0.01 | 0.15 ± 0.02 | 1.23 ± 0.13 | 0.29 ± 0.04 | 0.13 ± 0.02 | 2.32 ± 0.27 |
| I − D | 0.04 | 0.01 | 0.02 | 0.13 | 0.05 | 0.02 | 0.27 |
| (I − D)/D × 100, % | 11 | 12 | 13 | 11 | 15 | 15 | 12 |
| Summer–autumn period | |||||||
| I | 0.42 ± 0.06 | 0.11 ± 0.02 | 0.16 ± 0.03 | 1.57 ± 0.21 | 0.27 ± 0.06 | 0.12 ± 0.03 | 2.75 ± 0.42 |
| D | 0.38 ± 0.05 | 0.10 ± 0.01 | 0.14 ± 0.02 | 1.44 ± 0.19 | 0.24 ± 0.05 | 0.11 ± 0.02 | 2.49 ± 0.37 |
| I − D | 0.04 | 0.01 | 0.02 | 0.13 | 0.03 | 0.01 | 0.26 |
| (I − D)/D × 100, % | 10 | 11 | 12 | 9 | 14 | 14 | 10 |
| Winter low-water season | |||||||
| I | 0.33 ± 0.02 | 0.08 ± 0.01 | 0.11 ± 0.01 | 1.29 ± 0.08 | 0.16 ± 0.02 | 0.07 ± 0.01 | 2.08 ± 0.16 |
| D | 0.26 ± 0.03 | 0.06 ± 0.01 | 0.08 ± 0.01 | 1.06 ± 0.11 | 0.12 ± 0.02 | 0.05 ± 0.01 | 1.66 ± 0.20 |
| I − D | 0.07 | 0.02 | 0.03 | 0.23 | 0.04 | 0.02 | 0.42 |
| (I − D)/D × 100, % | 24 | 27 | 30 | 22 | 36 | 35 | 25 |
| The phase of water flow close to its long-term mean value | 0.30 ± 0.02 | 0.07 ± 0.01 | 0.10 ± 0.01 | 1.20 ± 0.09 | 0.14 ± 0.02 | 0.07 ± 0.01 | 1.92 ± 0.16 |
| Water Flow Phase | Concentrations (Caverage ± σ), mg/L | ||||||
|---|---|---|---|---|---|---|---|
| Ca2+ | Mg2+ | Na+ + K+ | HCO3− | SO42− | Cl− | TIS | |
| Year as a whole | |||||||
| I | 16.14 ± 7.17 | 4.19 ± 1.81 | 4.60 ± 1.56 | 65.03 ± 31.59 | 8.55 ± 2.17 | 4.23 ± 1.16 | 103.82 ± 44.33 |
| D | 17.95 ± 8.29 | 4.64 ± 2.09 | 4.96 ± 1.75 | 73.25 ± 37.03 | 9.03 ± 2.38 | 4.49 ± 1.28 | 114.88 ± 51.03 |
| I − D | −1.81 | −0.45 | −0.37 | −8.21 | −0.48 | −0.26 | −11.06 |
| (I − D)/D × 100, % | −10 | −10 | −7 | −11 | −5 | −6 | −10 |
| Snowmelt Spring-Summer flood period | |||||||
| I | 7.87 ± 4.89 | 2.08 ± 1.25 | 2.69 ± 1.13 | 29.46 ± 21.04 | 5.78 ± 1.65 | 2.76 ± 0.87 | 52.15 ± 30.56 |
| D | 9.02 ± 6.00 | 2.38 ± 1.52 | 2.96 ± 1.35 | 34.39 ± 26.08 | 6.18 ± 1.94 | 2.97 ± 1.03 | 59.35 ± 37.34 |
| I − D | −1.15 | −0.29 | −0.27 | −4.93 | −0.40 | −0.21 | −7.21 |
| (I − D)/D×100, % | −13 | −12 | −9 | −14 | −6 | −7 | −12 |
| Summer–autumn period | |||||||
| I | 11.89 ± 2.87 | 3.12 ± 0.73 | 3.73 ± 0.66 | 45.90 ± 12.30 | 7.40 ± 0.97 | 3.61 ± 0.51 | 77.75 ± 17.95 |
| D | 13.05 ± 3.53 | 3.41 ± 0.90 | 3.99 ± 0.79 | 50.93 ± 15.35 | 7.77 ± 1.11 | 3.80 ± 0.59 | 84.95 ± 21.95 |
| I − D | −1.16 | −0.29 | −0.26 | −5.03 | −0.37 | −0.20 | −7.20 |
| (I − D)/D×100, % | −9 | −9 | −6 | −10 | −5 | −5 | −8 |
| Winter low-water season | |||||||
| I | 21.02 ± 4.19 | 5.43 ± 1.05 | 5.68 ± 0.86 | 86.34 ± 18.86 | 10.06 ± 1.14 | 5.04 ± 0.62 | 134.11 ± 25.68 |
| D | 26.13 ± 6.25 | 6.70 ± 1.56 | 6.65 ± 1.20 | 110.01 ± 28.90 | 11.28 ± 1.52 | 5.71 ± 0.83 | 165.00 ± 37.90 |
| I − D | −5.10 | −1.27 | −0.97 | −23.67 | −1.22 | −0.67 | −30.89 |
| (I − D)/D×100, % | −20 | −19 | −15 | −22 | −11 | −12 | −19 |
| The phase of water flow close to its long-term mean value | 22.49 ± 4.66 | 5.79 ± 1.17 | 5.96 ± 0.93 | 93.07 ± 21.21 | 10.43 ± 1.21 | 5.24 ± 0.66 | 143.04 ± 28.43 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Georgiadi, A.G.; Danilenko, A.O.; Groisman, P.Y. Long-Term Changes in Water and Ion Flows of the Pechora River, the Longest Full-Water European Arctic River. Water 2024, 16, 1264. https://doi.org/10.3390/w16091264
Georgiadi AG, Danilenko AO, Groisman PY. Long-Term Changes in Water and Ion Flows of the Pechora River, the Longest Full-Water European Arctic River. Water. 2024; 16(9):1264. https://doi.org/10.3390/w16091264
Chicago/Turabian StyleGeorgiadi, Aleksandr G., Alesya O. Danilenko, and Pavel Y. Groisman. 2024. "Long-Term Changes in Water and Ion Flows of the Pechora River, the Longest Full-Water European Arctic River" Water 16, no. 9: 1264. https://doi.org/10.3390/w16091264
APA StyleGeorgiadi, A. G., Danilenko, A. O., & Groisman, P. Y. (2024). Long-Term Changes in Water and Ion Flows of the Pechora River, the Longest Full-Water European Arctic River. Water, 16(9), 1264. https://doi.org/10.3390/w16091264







