Experimental Study on Nonlinear Vibrations of Flexible Monopile-Foundation Offshore Wind Turbines in Regular Waves
Abstract
1. Introduction
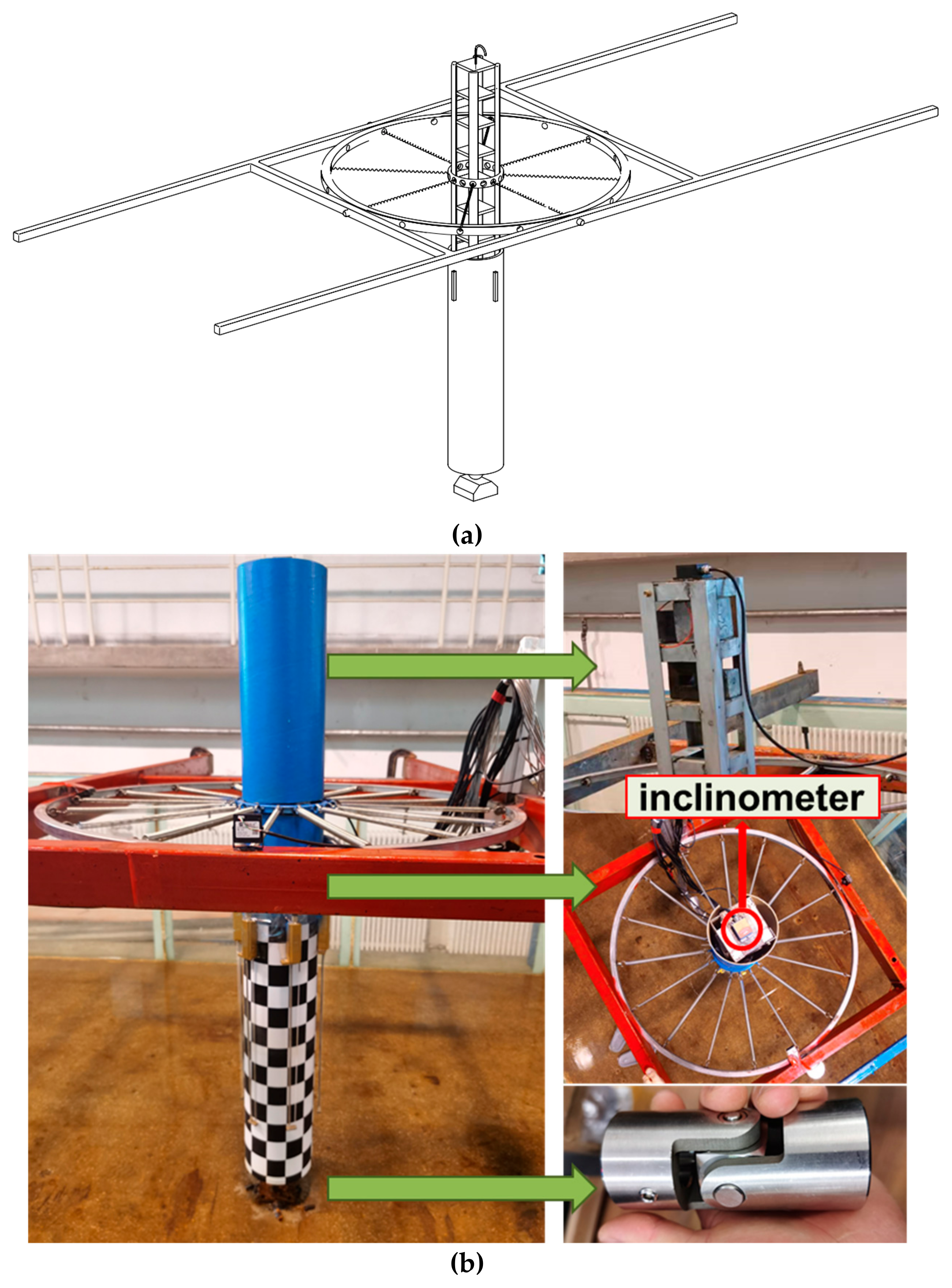
2. Experimental Set-Ups
2.1. Model Description
2.2. Sensor Arrangement and Data Acquisition
2.3. Test Conditions
3. Results and Discussion
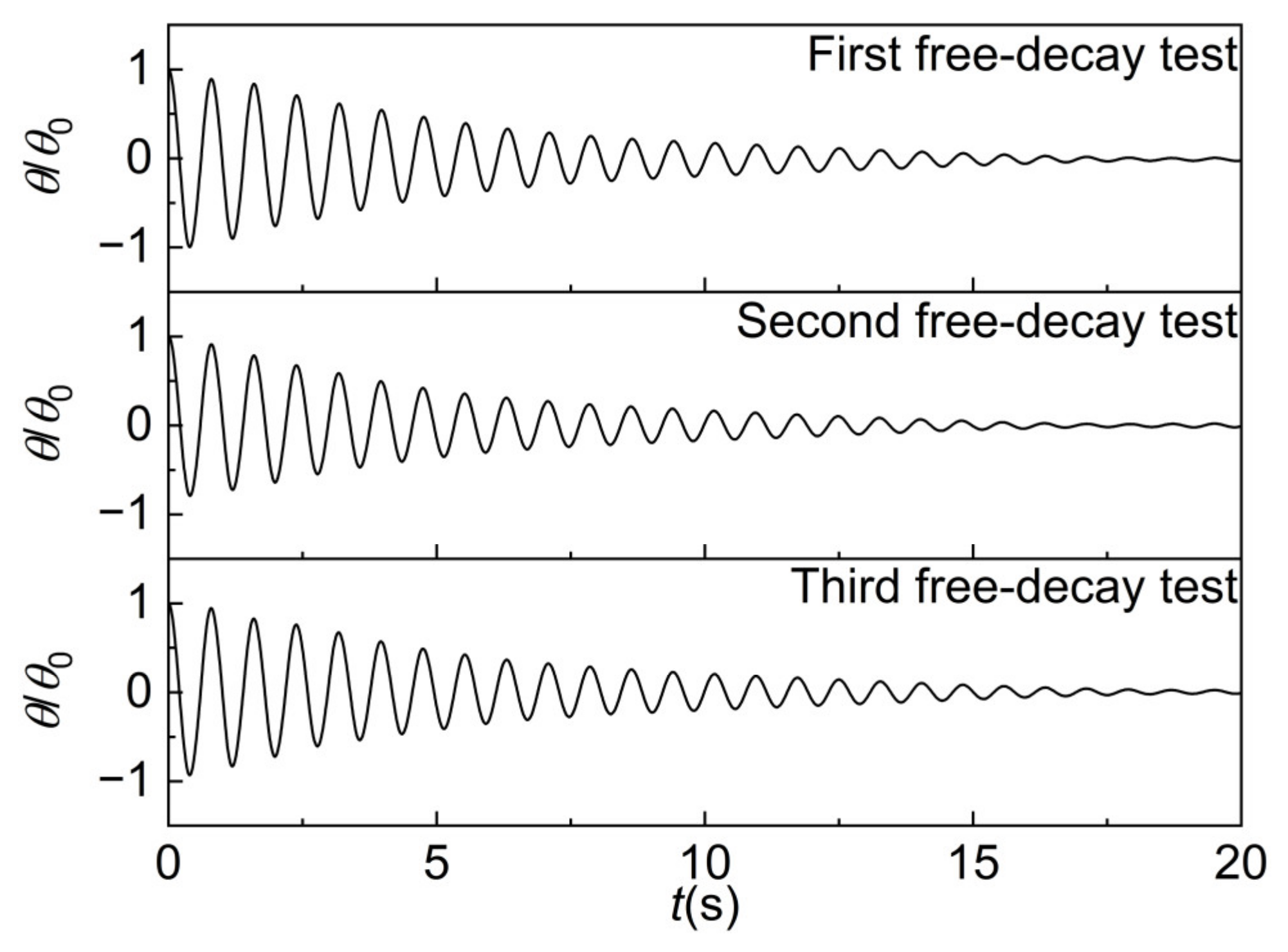
3.1. Free-Decay Text
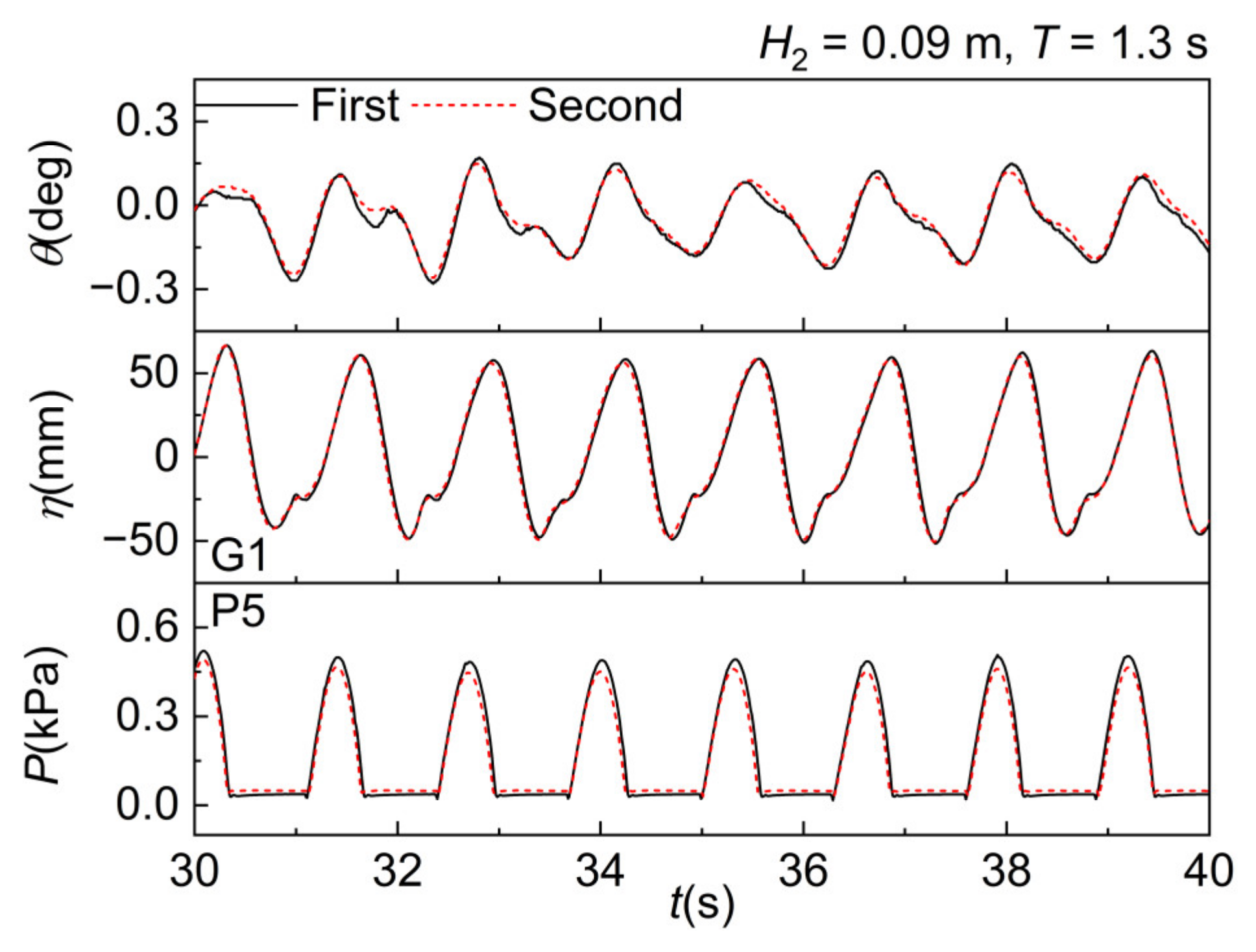
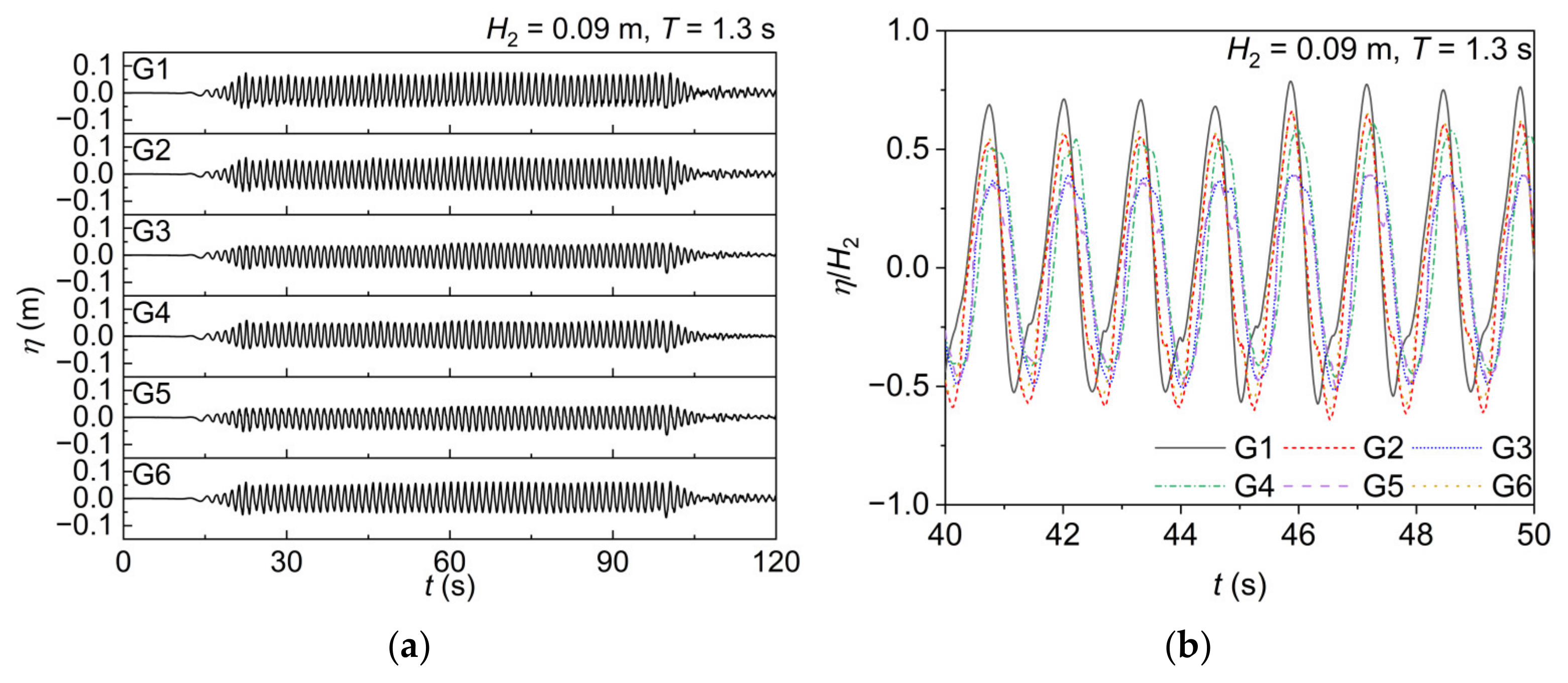
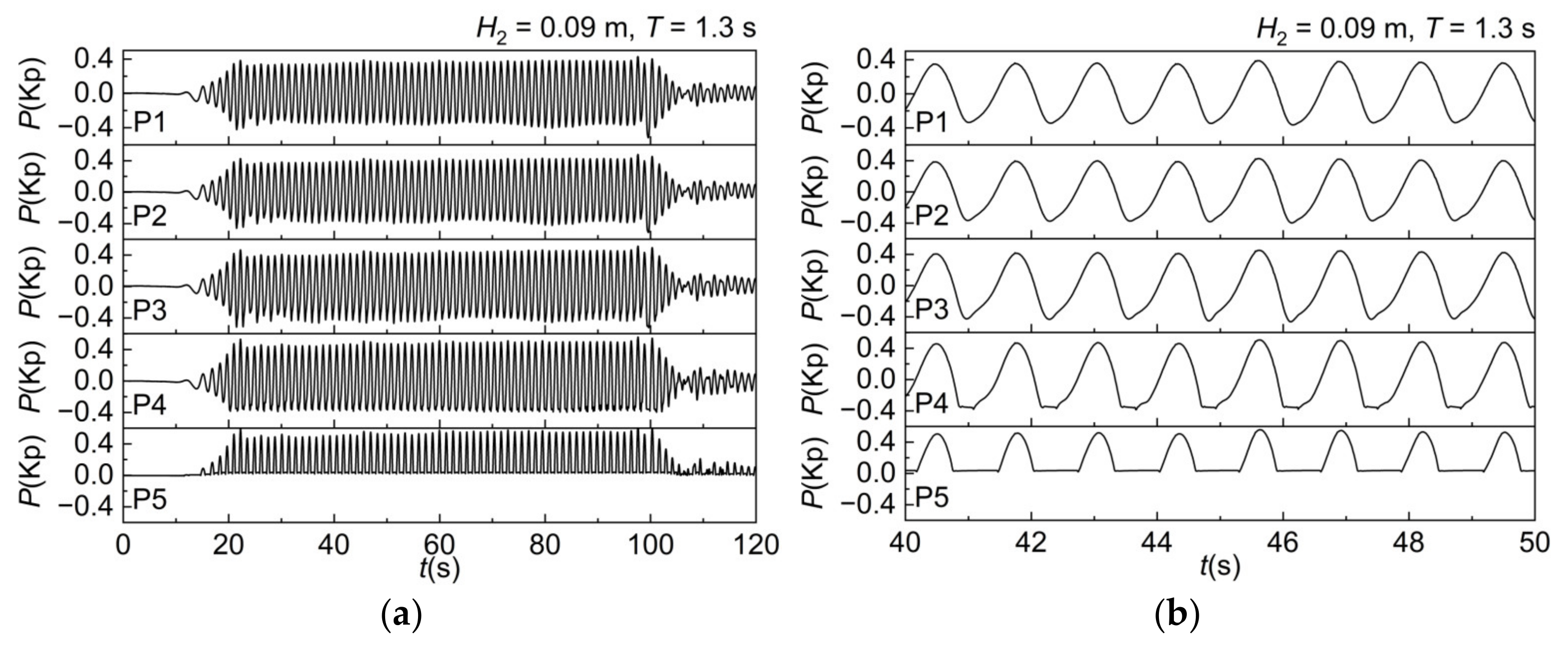
3.2. Repeatability and Data Consistency
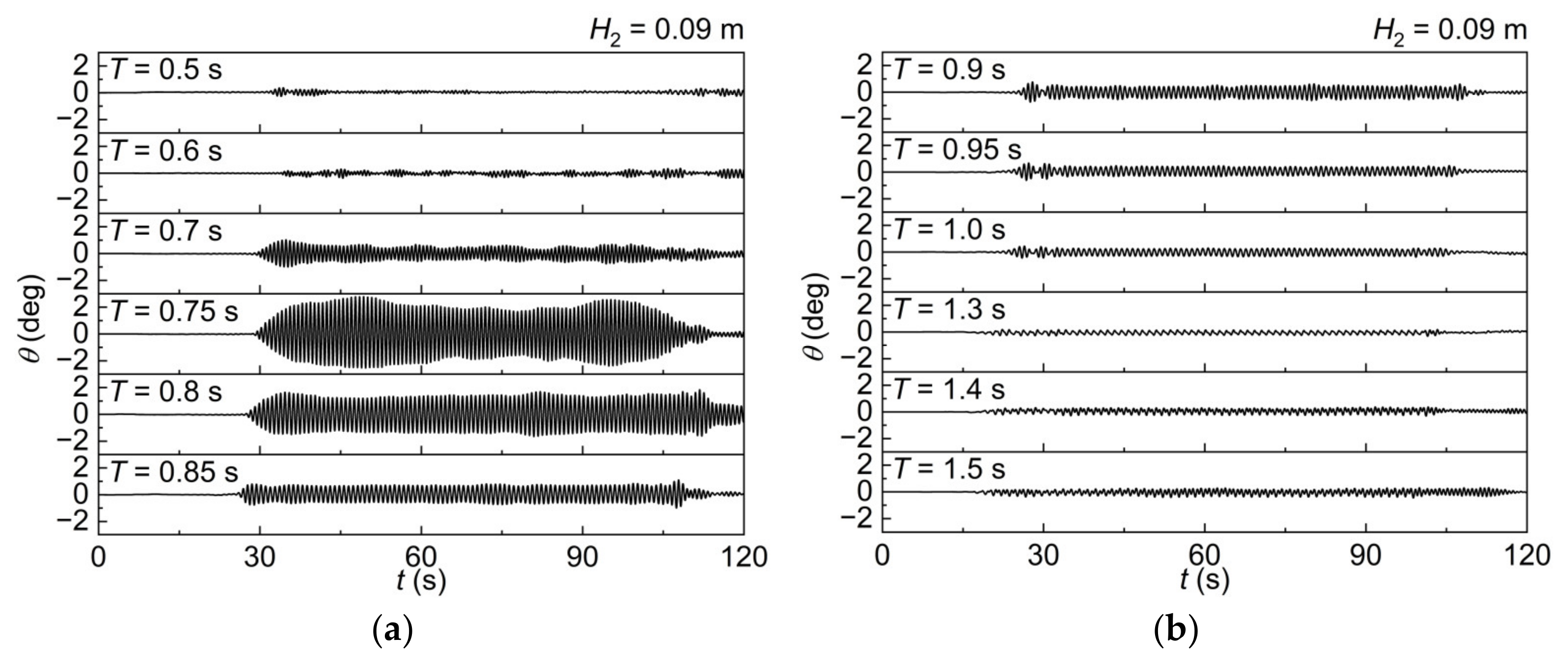
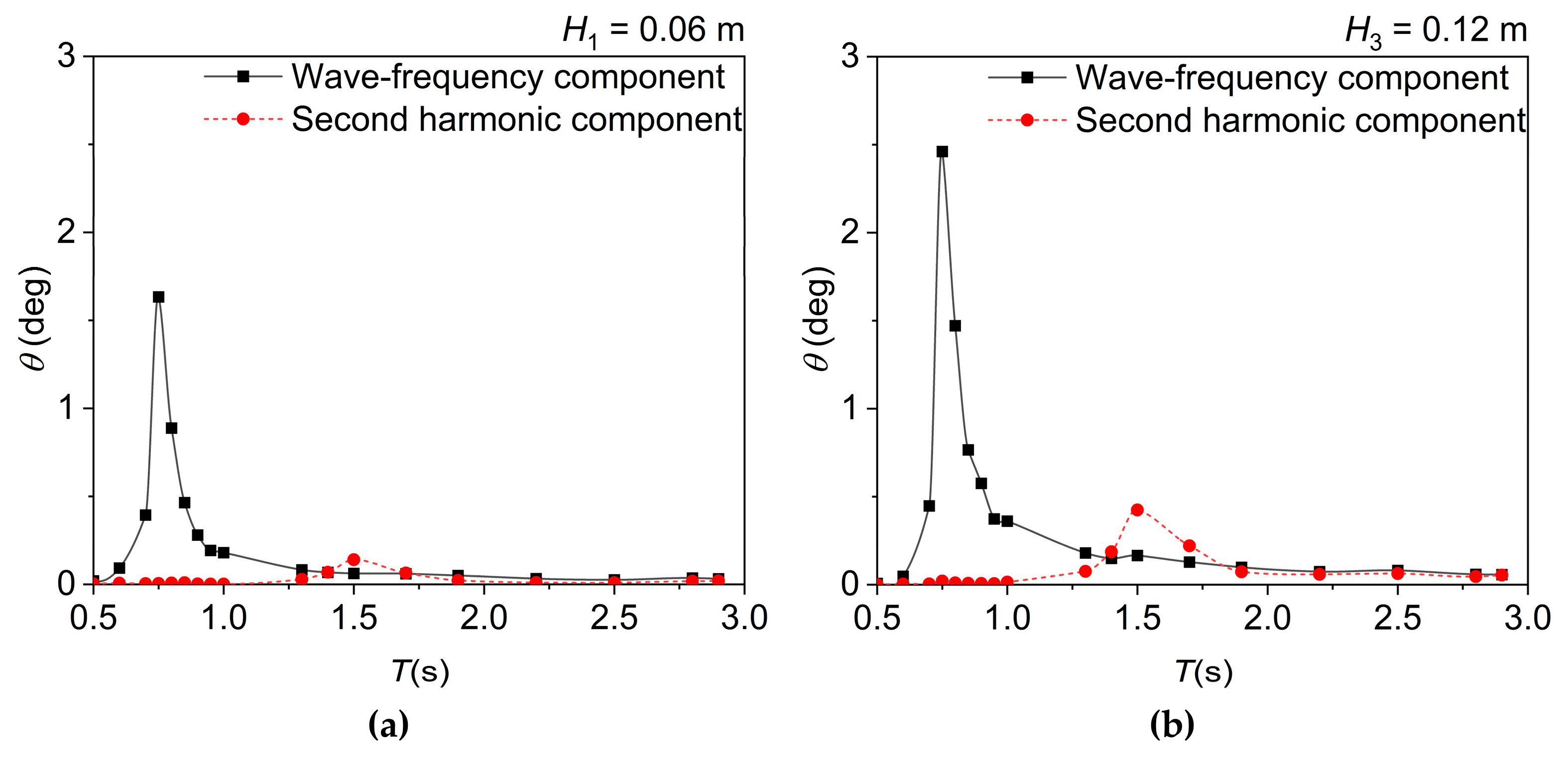
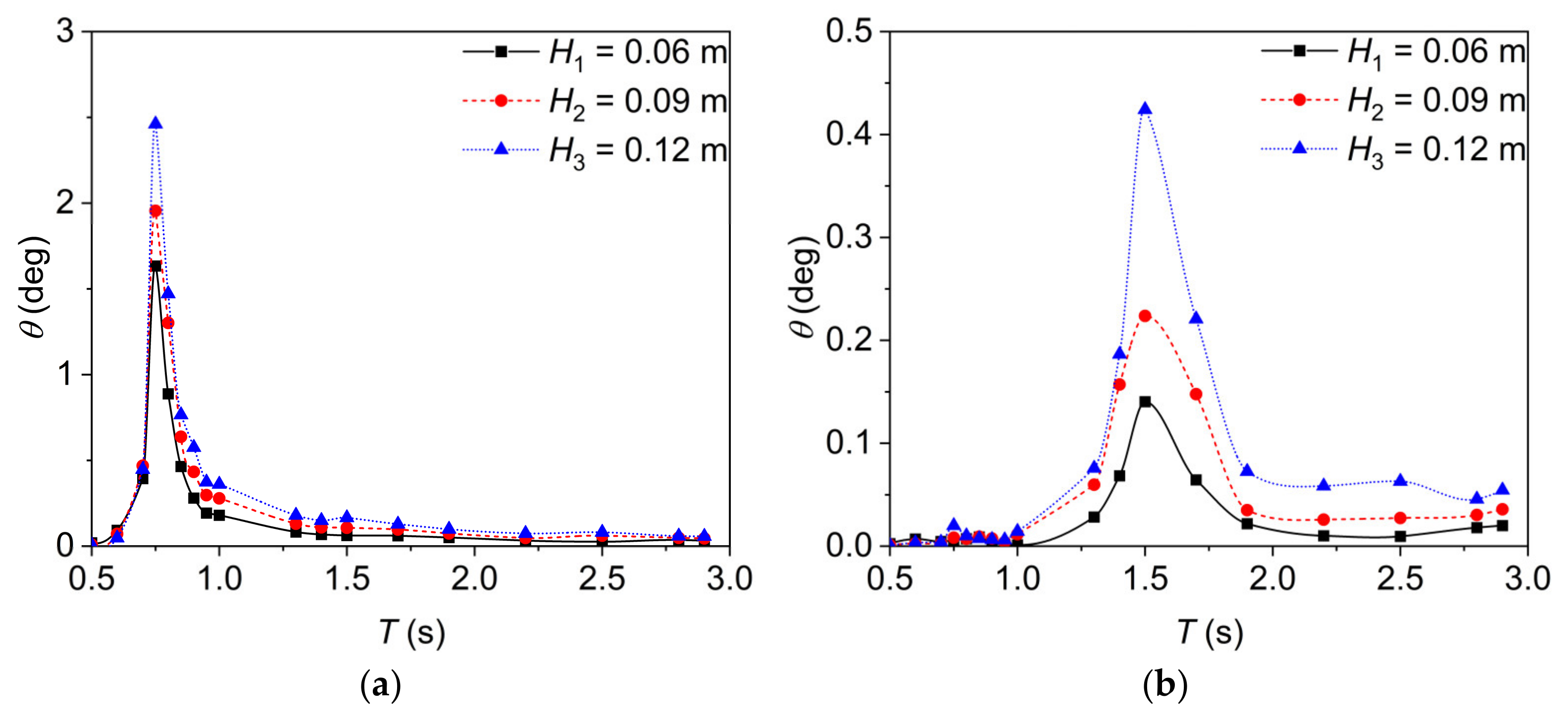
3.3. Analysis of Nonlinear Vibration Characteristics
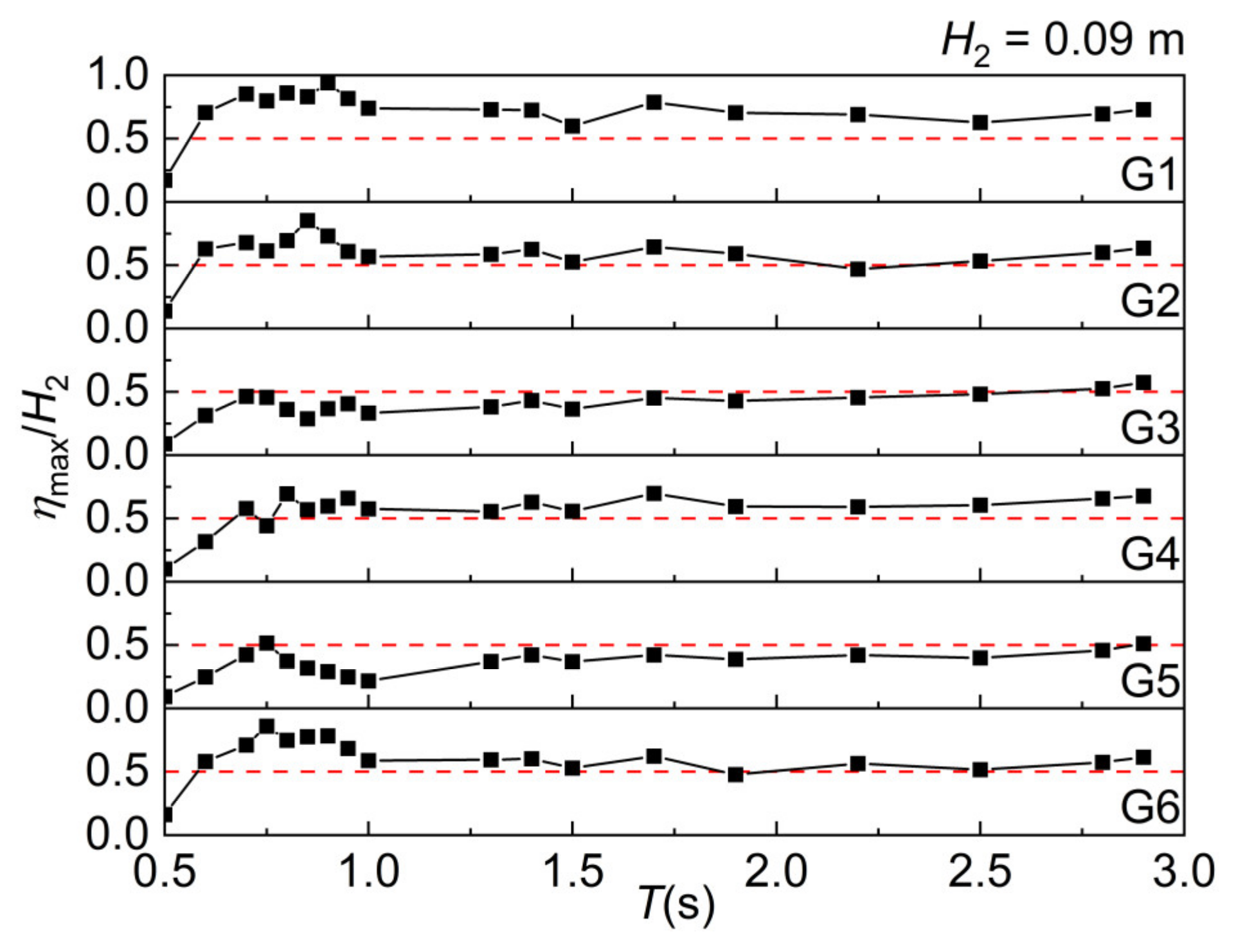
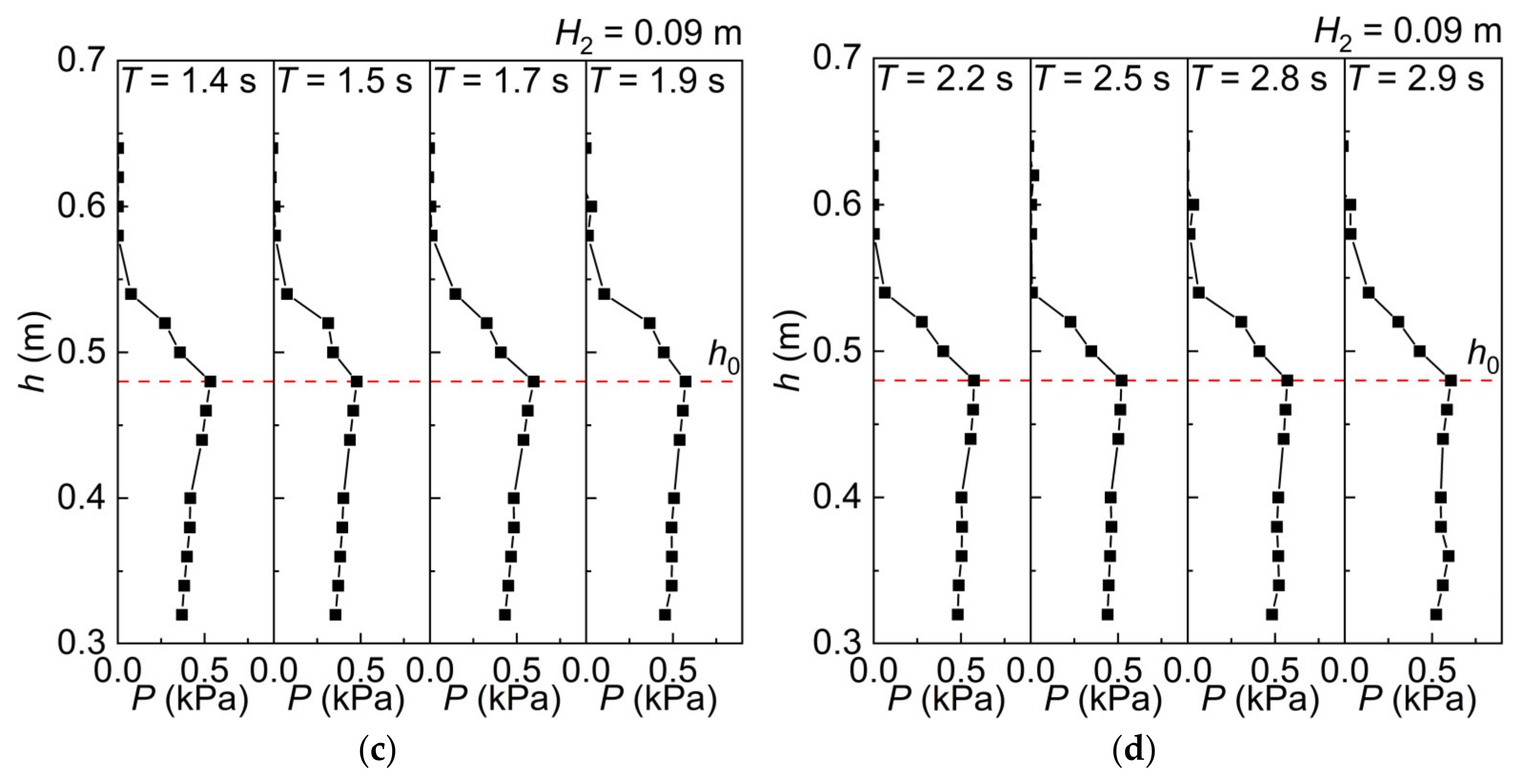
3.4. Run-Up on the Model
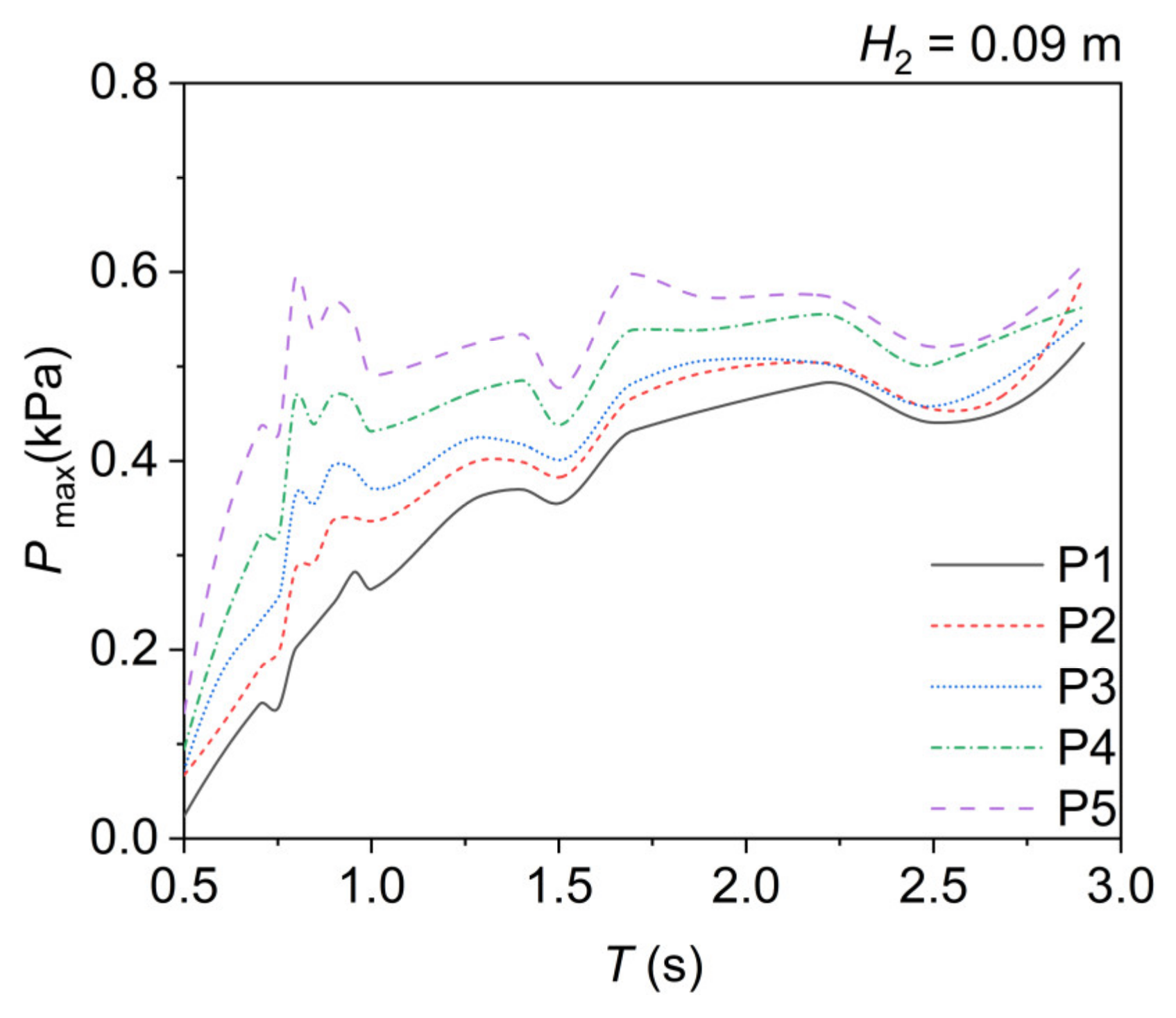
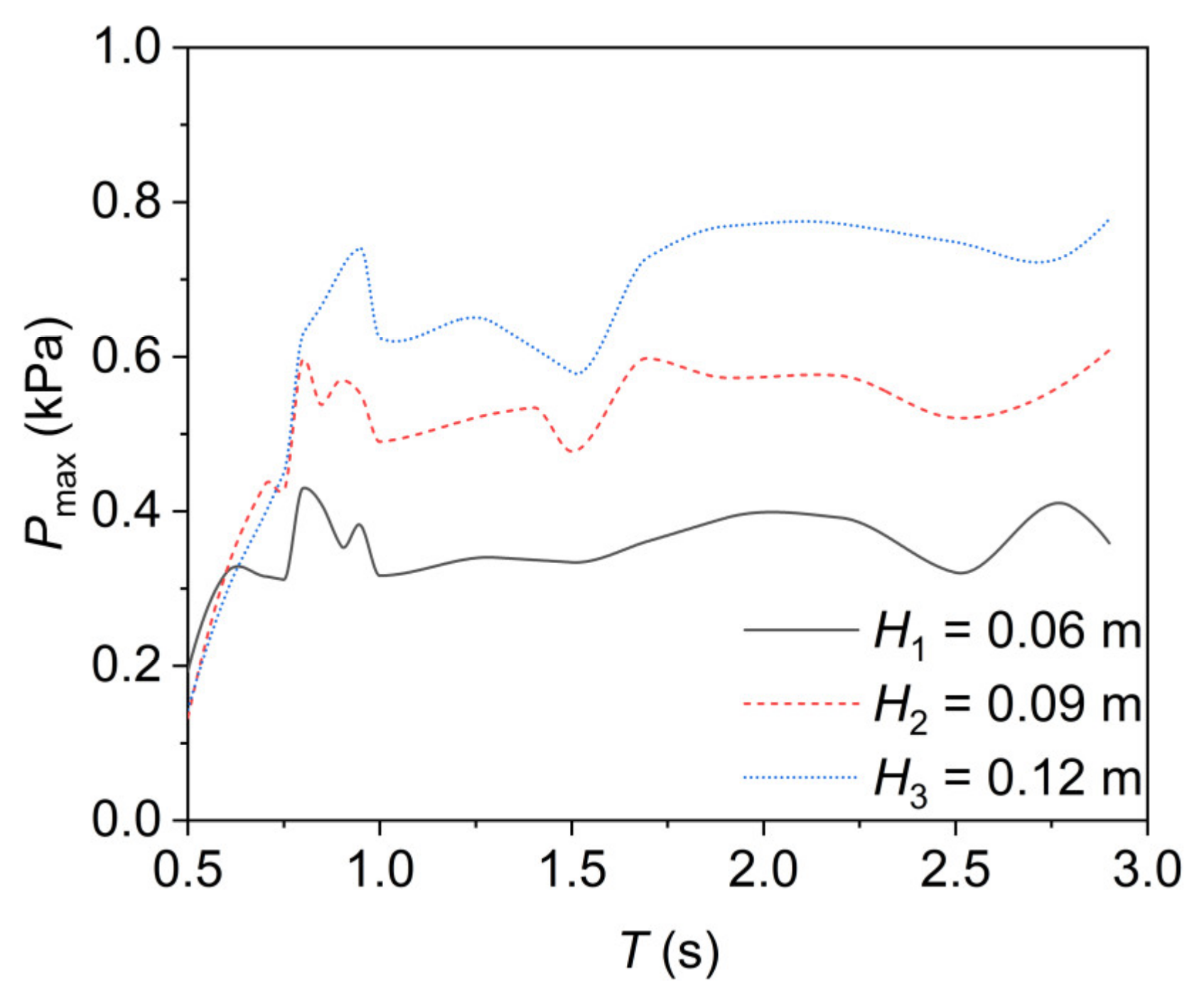
3.5. Maximum Pressure on the Model
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sun, X.; Huang, D.; Wu, G. The Current State of Offshore Wind Energy Technology Development. Energy 2012, 41, 298–312. [Google Scholar] [CrossRef]
- Arshad, M.; O’Kelly, B.C. Analysis and Design of Monopile Foundations for Offshore Wind-Turbine Structures. Mar. Georesources Geotechnol. 2016, 34, 503–525. [Google Scholar] [CrossRef]
- Bošnjaković, M.; Katinić, M.; Santa, R.; Marić, D. Wind Turbine Technology Trends. Appl. Sci. 2022, 12, 8653. [Google Scholar] [CrossRef]
- Wu, X.; Hu, Y.; Li, Y.; Yang, J.; Duan, L.; Wang, T.; Adcock, T.; Jiang, Z.; Gao, Z.; Lin, Z.; et al. Foundations of Offshore Wind Turbines: A Review. Renew. Sustain. Energy Rev. 2019, 104, 379–393. [Google Scholar] [CrossRef]
- Schløer, S.; Bredmose, H.; Bingham, H.B. The Influence of Fully Nonlinear Wave Forces on Aero-Hydro-Elastic Calculations of Monopile Wind Turbines. Mar. Struct. 2016, 50, 162–188. [Google Scholar] [CrossRef]
- Chou, J.-S.; Tu, W.-T. Failure Analysis and Risk Management of a Collapsed Large Wind Turbine Tower. Eng. Fail. Anal. 2011, 18, 295–313. [Google Scholar] [CrossRef]
- Chen, X.; Xu, J.Z. Structural Failure Analysis of Wind Turbines Impacted by Super Typhoon Usagi. Eng. Fail. Anal. 2016, 60, 391–404. [Google Scholar] [CrossRef]
- Morris-Thomas, M.T.; Thiagarajan, K.P. The Run-up on a Cylinder in Progressive Surface Gravity Waves: Harmonic Components. Appl. Ocean Res. 2004, 26, 98–113. [Google Scholar] [CrossRef]
- Niedzwecki, J.M.; Duggal, A.S. Wave Runup and Forces on Cylinders in Regular and Random Waves. J. Waterw. Port Coast. Ocean Eng. 1992, 118, 615–634. [Google Scholar] [CrossRef]
- Marino, E.; Lugni, C.; Borri, C. A Novel Numerical Strategy for the Simulation of Irregular Nonlinear Waves and Their Effects on the Dynamic Response of Offshore Wind Turbines. Comput. Methods Appl. Mech. Eng. 2013, 255, 275–288. [Google Scholar] [CrossRef]
- Marino, E.; Lugni, C.; Borri, C. The Role of the Nonlinear Wave Kinematics on the Global Responses of an OWT in Parked and Operating Conditions. J. Wind Eng. Ind. Aerodyn. 2013, 123, 363–376. [Google Scholar] [CrossRef]
- Paulsen, B.T.; Bredmose, H.; Bingham, H.B.; Schløer, S. Steep Wave Loads From Irregular Waves on an Offshore Wind Turbine Foundation: Computation and Experiment. In Proceedings of the ASME 2013 32nd International Conference on Ocean, Offshore and Arctic Engineering, Nantes, France, 9–14 June 2013; American Society of Mechanical Engineers: New York, NY, USA, 2013; Volume 9, p. V009T12A028. [Google Scholar]
- Deng, S.; Qin, M.; Ning, D.; Lin, L.; Wu, S.; Zhang, C. Numerical and Experimental Investigations on Non-Linear Wave Action on Offshore Wind Turbine Monopile Foundation. J. Mar. Sci. Eng. 2023, 11, 883. [Google Scholar] [CrossRef]
- Bredmose, H.; Jacobsen, N.G. Breaking Wave Impacts on Offshore Wind Turbine Foundations: Focused Wave Groups and CFD. In Proceedings of the 29th International Conference on Ocean, Offshore and Arctic Engineering, Shanghai, China, 6–11 June 2010; ASMEDC: New York, NY, USA, 2010; Volume 3, pp. 397–404. [Google Scholar]
- Ding, H.; Zhao, G.; Tang, T.; Taylor, P.H.; Adcock, T.A.; Dai, S.; Ning, D.; Chen, L.; Li, J.; Wang, R.; et al. Experimental investigation of nonlinear forces on a monopile offshore wind turbine foundation under directionally spread waves. J. Offshore Mech. Arct. Eng. 2025, 147, 042002. [Google Scholar] [CrossRef]
- Sclavounos, P.D.; Zhang, Y.; Ma, Y.; Larson, D.F. Offshore Wind Turbine Nonlinear Wave Loads and Their Statistics. J. Offshore Mech. Arct. Eng. 2019, 141, 031904. [Google Scholar] [CrossRef]
- Mockutė, A.; Marino, E.; Lugni, C.; Borri, C. Comparison of Nonlinear Wave-Loading Models on Rigid Cylinders in Regular Waves. Energies 2019, 12, 4022. [Google Scholar] [CrossRef]
- De Ridder, E.J.; Aalberts, P.; Van Den Berg, J.; Buchner, B.; Peeringa, J. The Dynamic Response of an Offshore Wind Turbine With Realistic Flexibility to Breaking Wave Impact. In Proceedings of the ASME 2011 30th International Conference on Ocean, Offshore and Arctic Engineering, Rotterdam, The Netherlands, 19–24 June 2011; ASMEDC: New York, NY, USA, 2011; Volume 5, pp. 543–552. [Google Scholar]
- Bachynski, E.E.; Ormberg, H. Hydrodynamic Modeling of Large-Diameter Bottom-Fixed Offshore Wind Turbines. In Proceedings of the ASME 2015 34th International Conference on Ocean, Offshore and Arctic Engineering, St. John’s, NL, Canada, 31 May–5 June 2015; American Society of Mechanical Engineers: New York, NY, USA, 2015; Volume 9, p. V009T09A051. [Google Scholar]
- Ryan, G.V.; Tang, T.; McAdam, R.A.; Adcock, T.A. Influence of non-linear wave load models on monopile supported offshore wind turbines for extreme conditions. Ocean Eng. 2025, 323, 120510. [Google Scholar] [CrossRef]
- Jiang, C.; el Moctar, O. Experimental decomposition of nonlinear hydrodynamic loads and hydroelastic responses in wave–structure interactions of monopile wind turbines. Phys. Fluids 2024, 36, 117112. [Google Scholar] [CrossRef]
- Wang, S.; Larsen, T.J.; Bredmose, H. Ultimate load analysis of a 10 MW offshore monopile wind turbine incorporating fully nonlinear irregular wave kinematics. Mar. Struct. 2021, 76, 102922. [Google Scholar] [CrossRef]
- Bachynski, E.; Thys, M.; Delhaye, V. Dynamic Response of a Monopile Wind Turbine in Waves: Experimental Uncertainty Analysis for Validation of Numerical Tools. Appl. Ocean Res. 2019, 89, 96–114. [Google Scholar] [CrossRef]
- Bachynski, E.E.; Thys, M.; Dadmarzi, F.H. Observations from Hydrodynamic Testing of a Flexible, Large-Diameter Monopile in Irregular Waves. J. Phys. Conf. Ser. 2020, 1669, 012028. [Google Scholar] [CrossRef]
- Suja-Thauvin, L.; Krokstad, J.R.; Bachynski, E.E.; De Ridder, E.-J. Experimental Results of a Multimode Monopile Offshore Wind Turbine Support Structure Subjected to Steep and Breaking Irregular Waves. Ocean Eng. 2017, 146, 339–351. [Google Scholar] [CrossRef]
- Bredmose, H.; Dixen, M.; Ghadirian, A.; Larsen, T.J.; Schløer, S.; Andersen, S.J.; Wang, S.; Bingham, H.B.; Lindberg, O.; Christensen, E.D.; et al. DeRisk—Accurate Prediction of ULS Wave Loads. Outlook and First Results. Energy Procedia 2016, 94, 379–387. [Google Scholar] [CrossRef]
- Nielsen, A.W.; Schlütter, F.; Sørensen, J.V.T.; Bredmose, H. Wave Loads on a Monopile in 3D Waves. In Proceedings of the ASME 2012 31st International Conference on Ocean, Offshore and Arctic Engineering, Rio de Janeiro, Brazil, 1–6 June 2012; American Society of Mechanical Engineers: New York, NY, USA, 2012; Volume 7, pp. 403–411. [Google Scholar]
- Paulsen, B.T.; Bredmose, H.; Bingham, H.B. An Efficient Domain Decomposition Strategy for Wave Loads on Surface Piercing Circular Cylinders. Coast. Eng. 2014, 86, 57–76. [Google Scholar] [CrossRef]
- Bredmose, H.; Slabiak, P.; Sahlberg-Nielsen, L.; Schlütter, F. Dynamic Excitation of Monopiles by Steep and Breaking Waves: Experimental and Numerical Study. In Proceedings of the ASME 2013 32nd International Conference on Ocean, Offshore and Arctic Engineering, Nantes, France, 9–14 June 2013; American Society of Mechanical Engineers: New York, NY, USA, 2013; Volume 8, p. V008T09A062. [Google Scholar]
- Suja-Thauvin, L.; Krokstad, J.R.; Bachynski, E.E. Critical Assessment of Non-Linear Hydrodynamic Load Models for a Fully Flexible Monopile Offshore Wind Turbine. Ocean Eng. 2018, 164, 87–104. [Google Scholar] [CrossRef]
- Bachynski, E.E.; Kristiansen, T.; Thys, M. Experimental and Numerical Investigations of Monopile Ringing in Irregular Finite-Depth Water Waves. Appl. Ocean Res. 2017, 68, 154–170. [Google Scholar] [CrossRef]





| Model Scale | Full Scale | |
|---|---|---|
| Diameter | 0.18 m | 9 m |
| Model height | 1.5 m | 75 m |
| Depth of water | 0.48 m | 24 m |
| Resonance frequency | 1.30 Hz | 0.18 Hz |
| Wave height | 0.06 m, 0.09 m, 0.12 m | 3 m, 4.5 m, 6 m |
| Wave period | 0.5–2.9 s | 3.5–20.5 s |
| T (s) | ε = H/L | ||
|---|---|---|---|
| H1 = 0.06 m | H2 = 0.09 m | H3 = 0.12 m | |
| 0.5 | 0.154 | 0.231 | 0.307 |
| 0.6 | 0.107 | 0.160 | 0.214 |
| 0.7 | 0.078 | 0.118 | 0.157 |
| 0.75 | 0.068 | 0.103 | 0.137 |
| 0.8 | 0.060 | 0.090 | 0.121 |
| 0.85 | 0.054 | 0.080 | 0.107 |
| 0.9 | 0.048 | 0.072 | 0.096 |
| 0.95 | 0.044 | 0.065 | 0.087 |
| 1 | 0.040 | 0.060 | 0.080 |
| 1.3 | 0.026 | 0.039 | 0.052 |
| 1.4 | 0.024 | 0.035 | 0.047 |
| 1.5 | 0.021 | 0.032 | 0.043 |
| 1.7 | 0.018 | 0.027 | 0.036 |
| 1.9 | 0.016 | 0.024 | 0.032 |
| 2.2 | 0.013 | 0.020 | 0.027 |
| 2.5 | 0.012 | 0.017 | 0.023 |
| 2.8 | 0.010 | 0.015 | 0.020 |
| 2.9 | 0.010 | 0.015 | 0.020 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, S.; Zhang, H.; Chen, Z.; Liu, X.; Zheng, L.; Du, M.; Li, R.; Li, D. Experimental Study on Nonlinear Vibrations of Flexible Monopile-Foundation Offshore Wind Turbines in Regular Waves. Water 2025, 17, 1176. https://doi.org/10.3390/w17081176
Wu S, Zhang H, Chen Z, Liu X, Zheng L, Du M, Li R, Li D. Experimental Study on Nonlinear Vibrations of Flexible Monopile-Foundation Offshore Wind Turbines in Regular Waves. Water. 2025; 17(8):1176. https://doi.org/10.3390/w17081176
Chicago/Turabian StyleWu, Songxiong, Hao Zhang, Ziwen Chen, Xiaoting Liu, Long Zheng, Mengjiao Du, Rongfu Li, and Donghai Li. 2025. "Experimental Study on Nonlinear Vibrations of Flexible Monopile-Foundation Offshore Wind Turbines in Regular Waves" Water 17, no. 8: 1176. https://doi.org/10.3390/w17081176
APA StyleWu, S., Zhang, H., Chen, Z., Liu, X., Zheng, L., Du, M., Li, R., & Li, D. (2025). Experimental Study on Nonlinear Vibrations of Flexible Monopile-Foundation Offshore Wind Turbines in Regular Waves. Water, 17(8), 1176. https://doi.org/10.3390/w17081176






