Demarcation Technique and Geometric Analysis of Village Boundaries Resulting from Delineation of Land Areas (ujazd) in the Middle Ages in the Wielkopolska Region (Poland)
Abstract
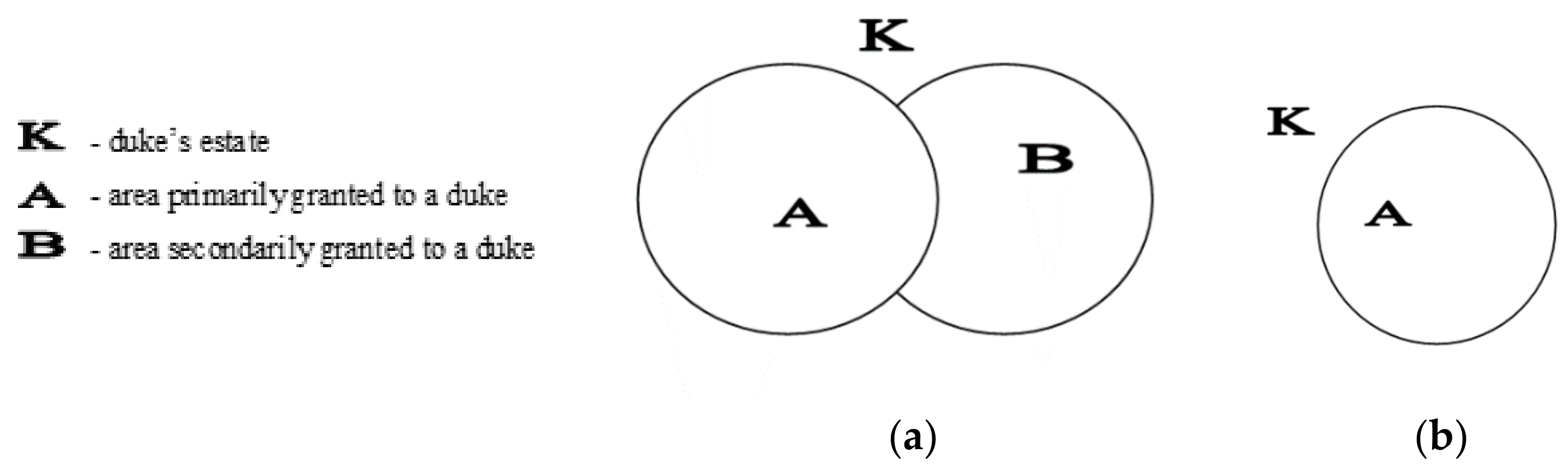
:1. Introduction
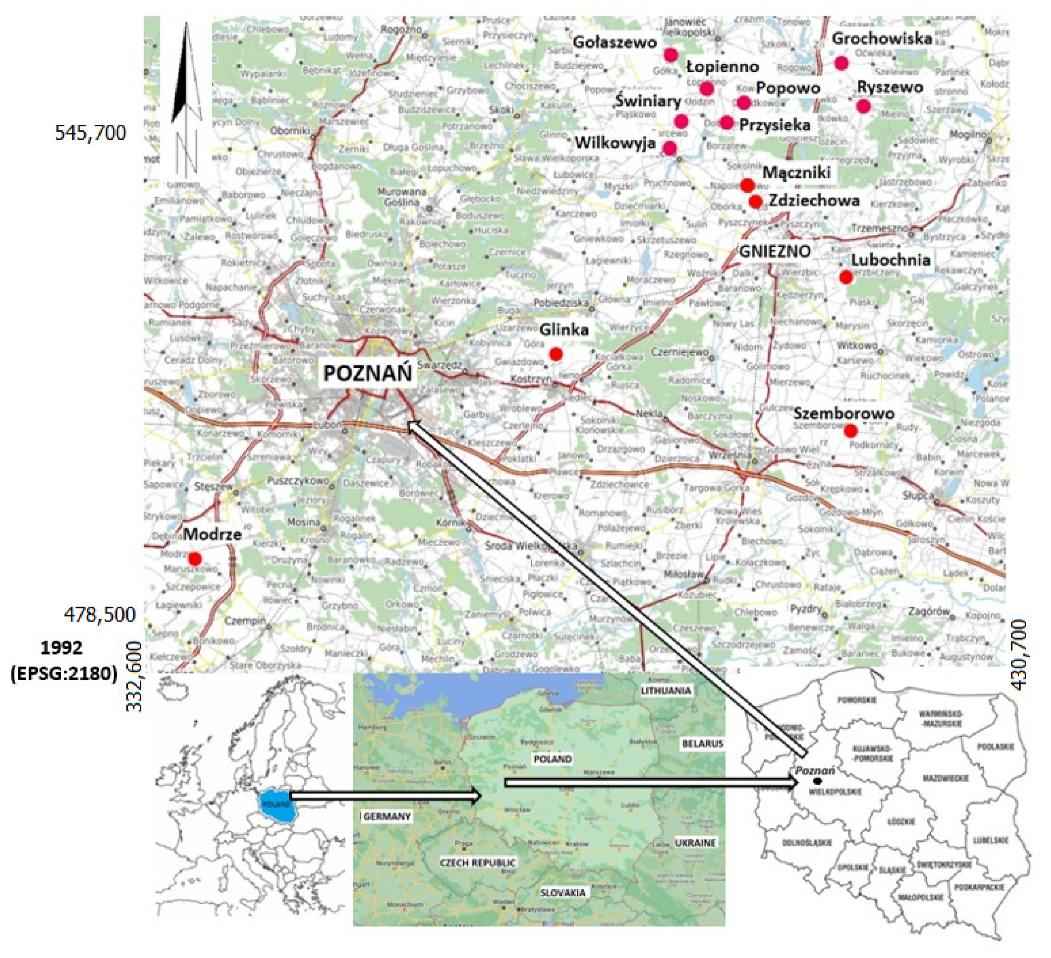
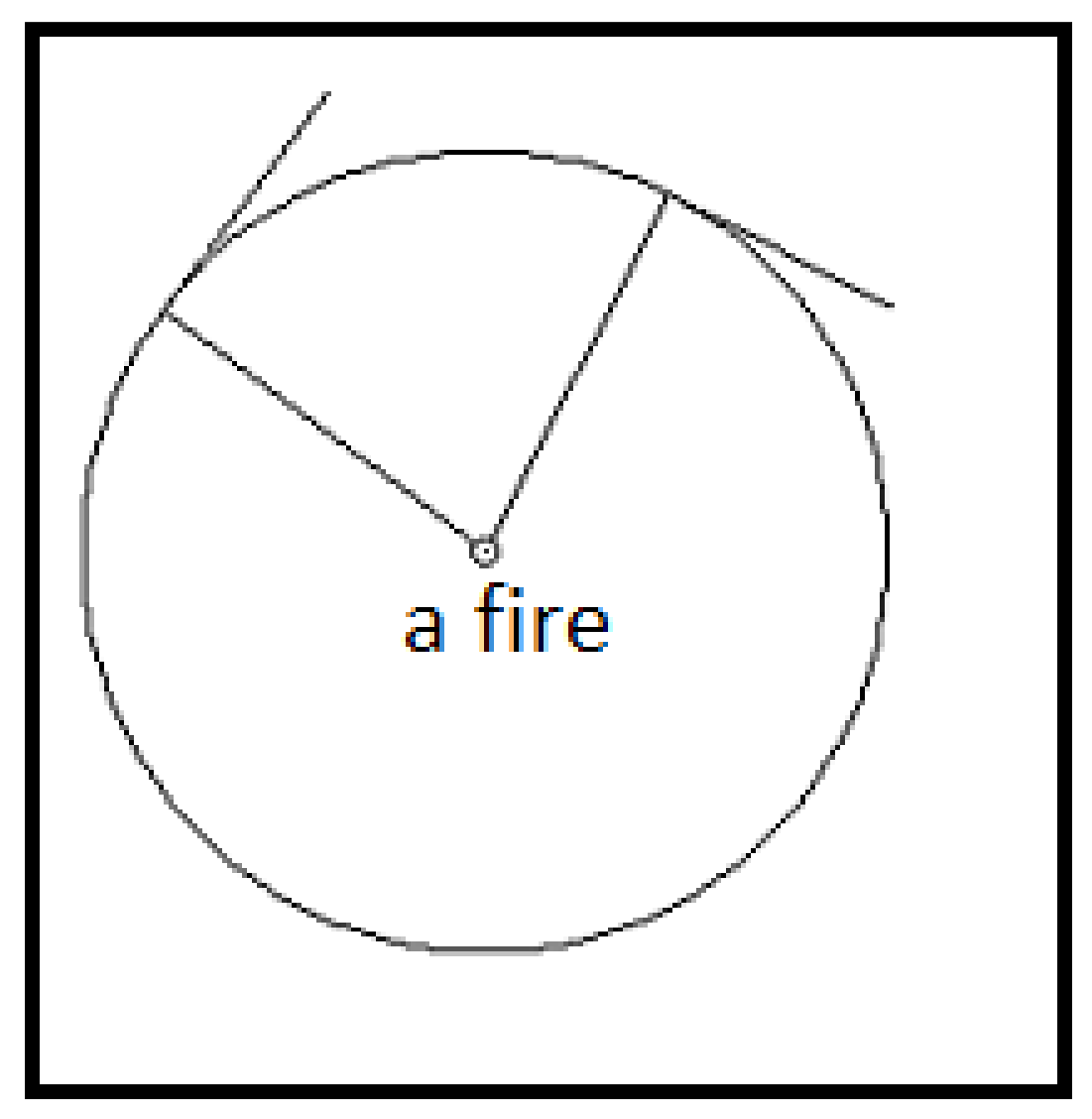
2. Materials and Methods
- scanning error 0.0001 m–1:25,000 scale = 2.5 m;
- reading error 0.0003 m–1:25,000 scale = 7.5 m;
- point position error m;
- segment length error m.
3. Results and Discussions
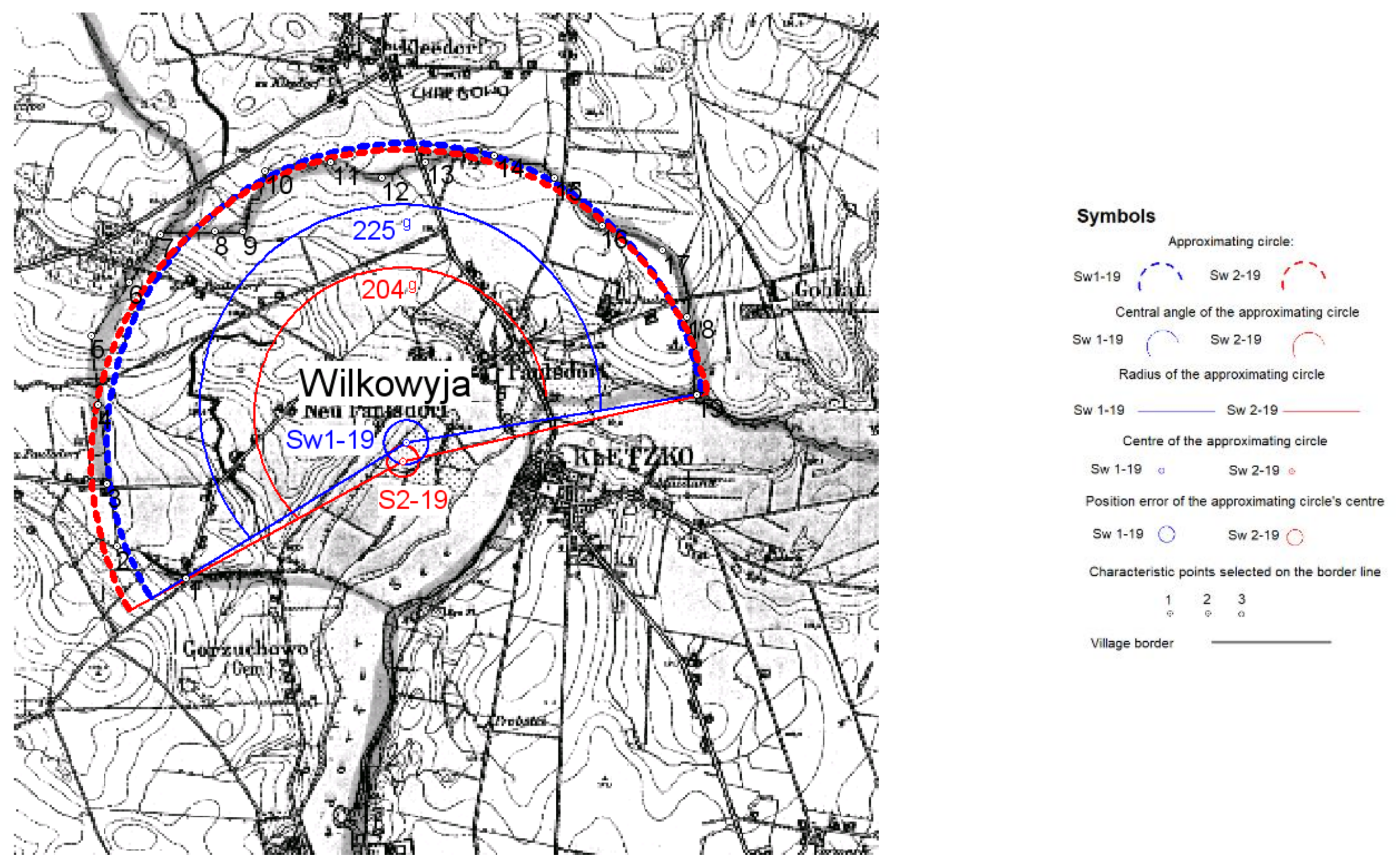
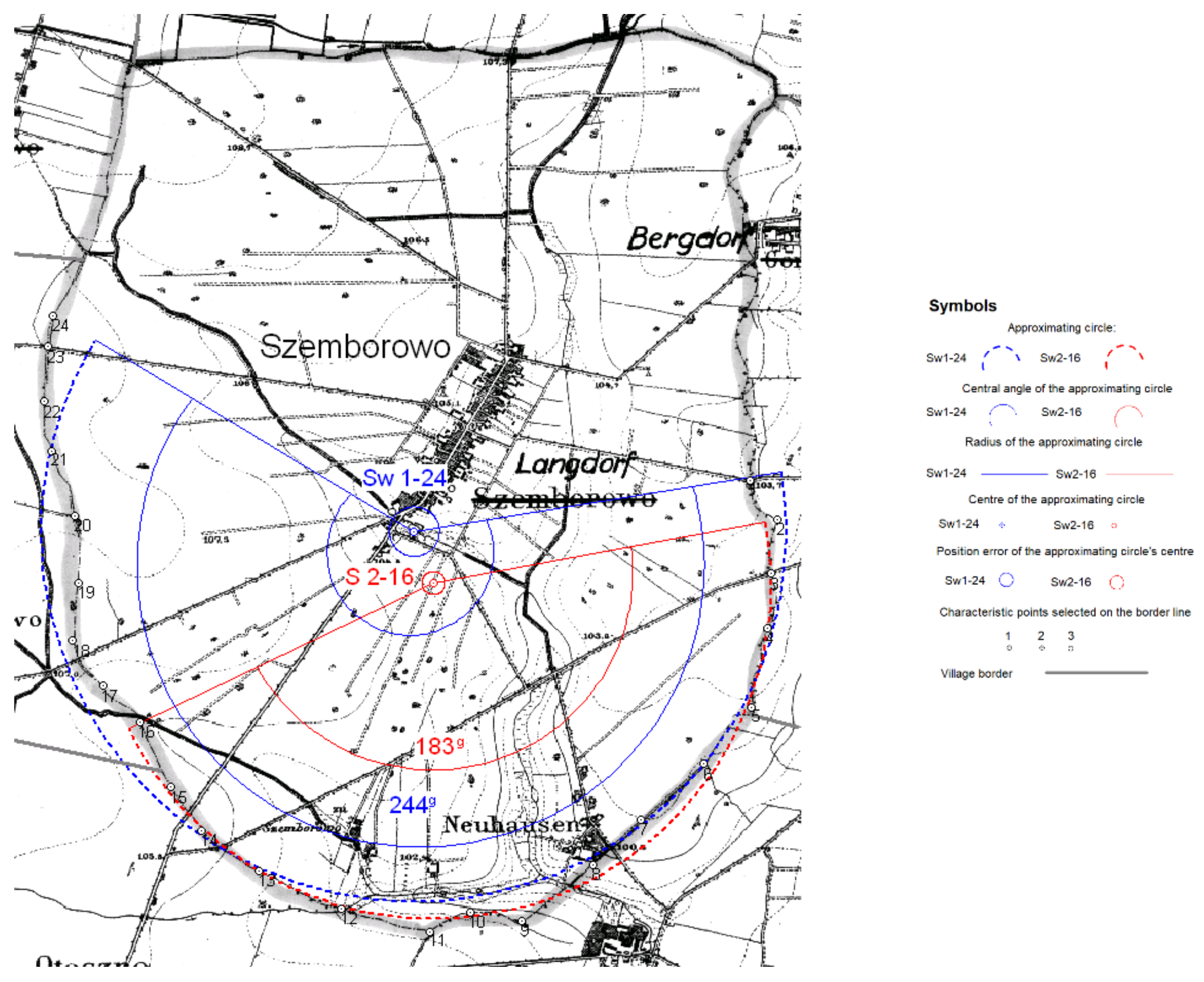
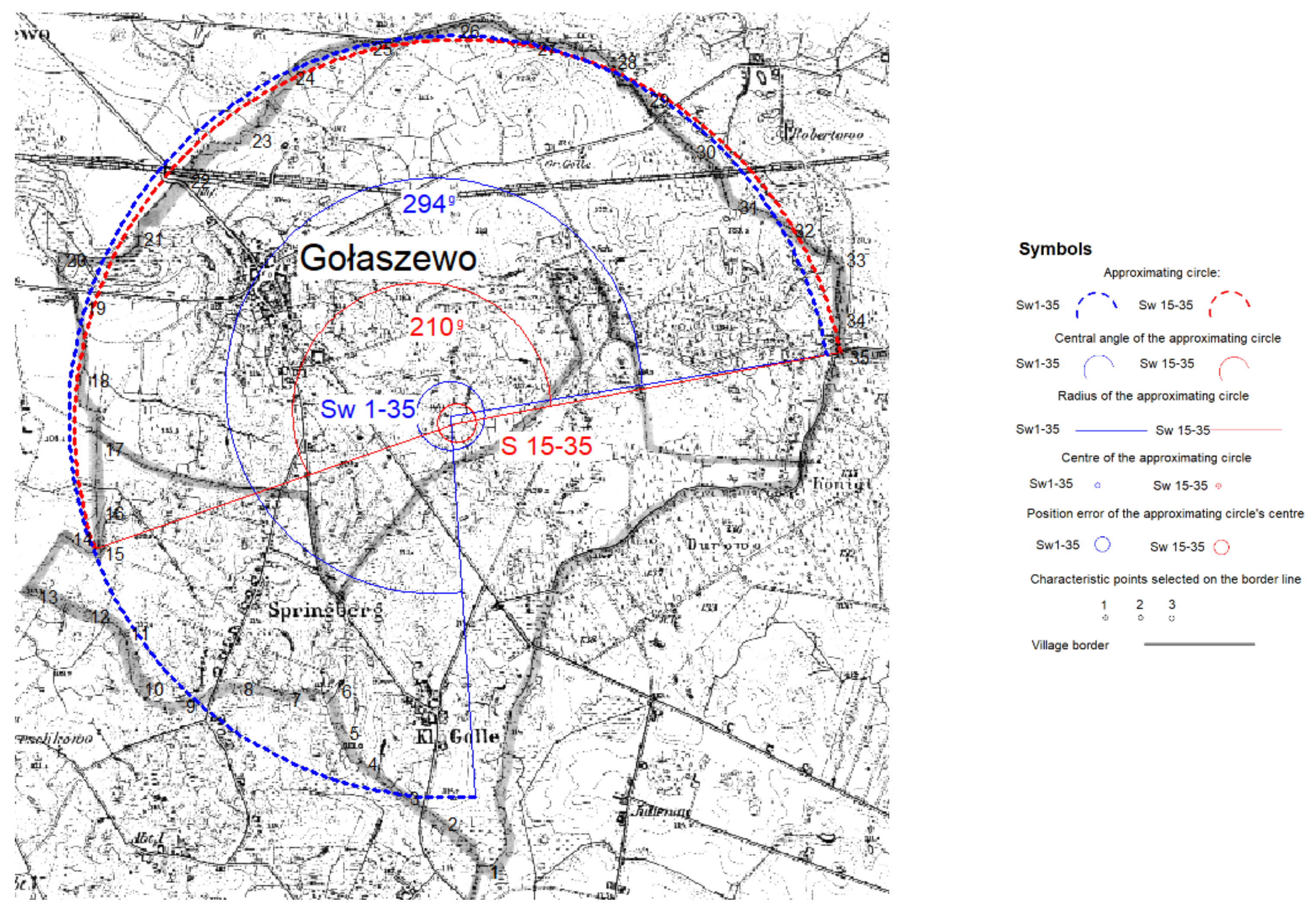
- Segments of a somewhat different curvature at the endpoints of the boundaries studied were omitted in the following villages: Glinka (see Table 3, no. 1 and Figure 9), Gołaszewo (see Table 3, no. 2 and Figure 7), Szemborowo (see Table 3, no. 3 and Figure 6), Wilkowyja (see Table 3, no. 4 and Figure 4), Zdziechowa (see Table 3, no. 5, 6 and Figure 5).
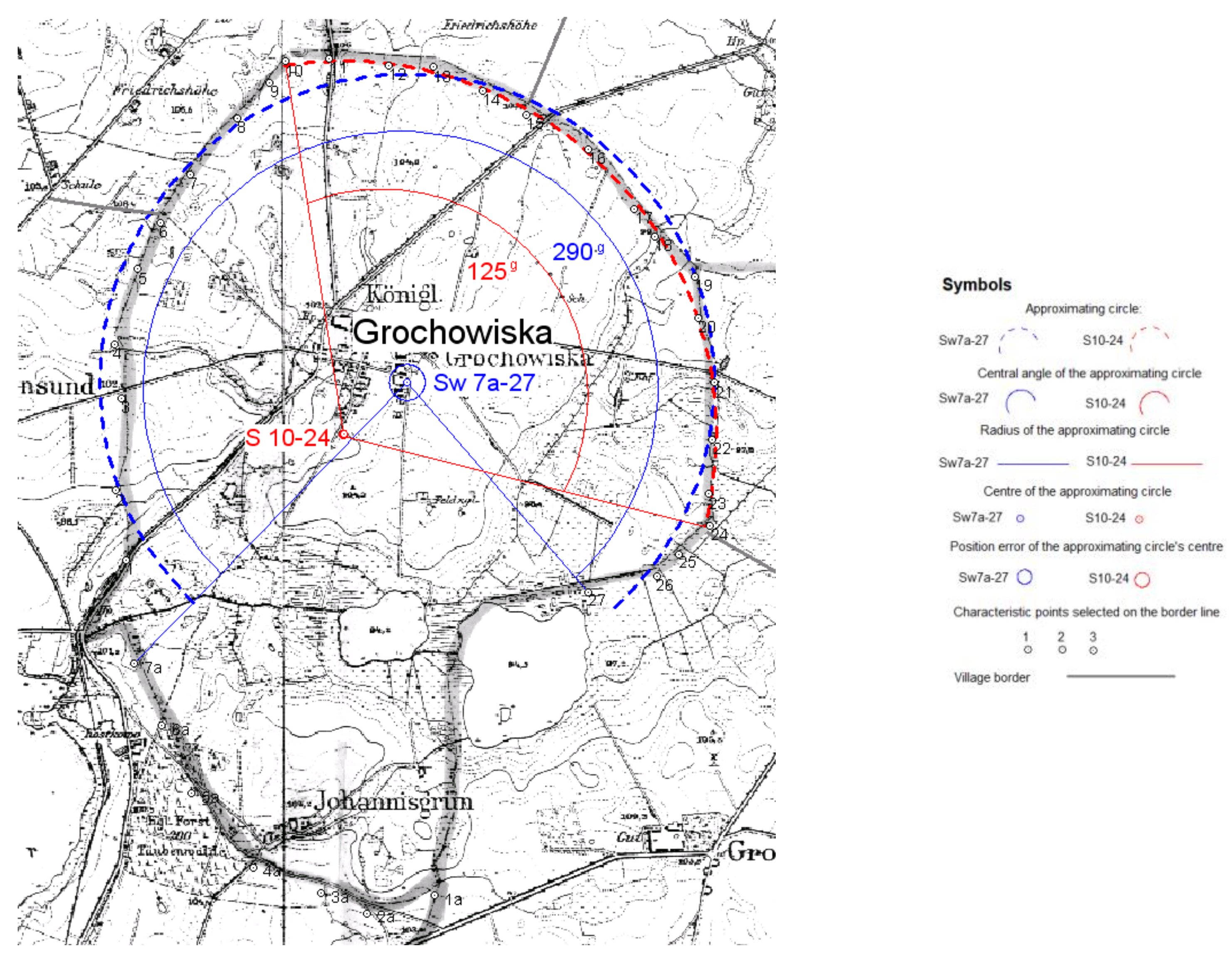
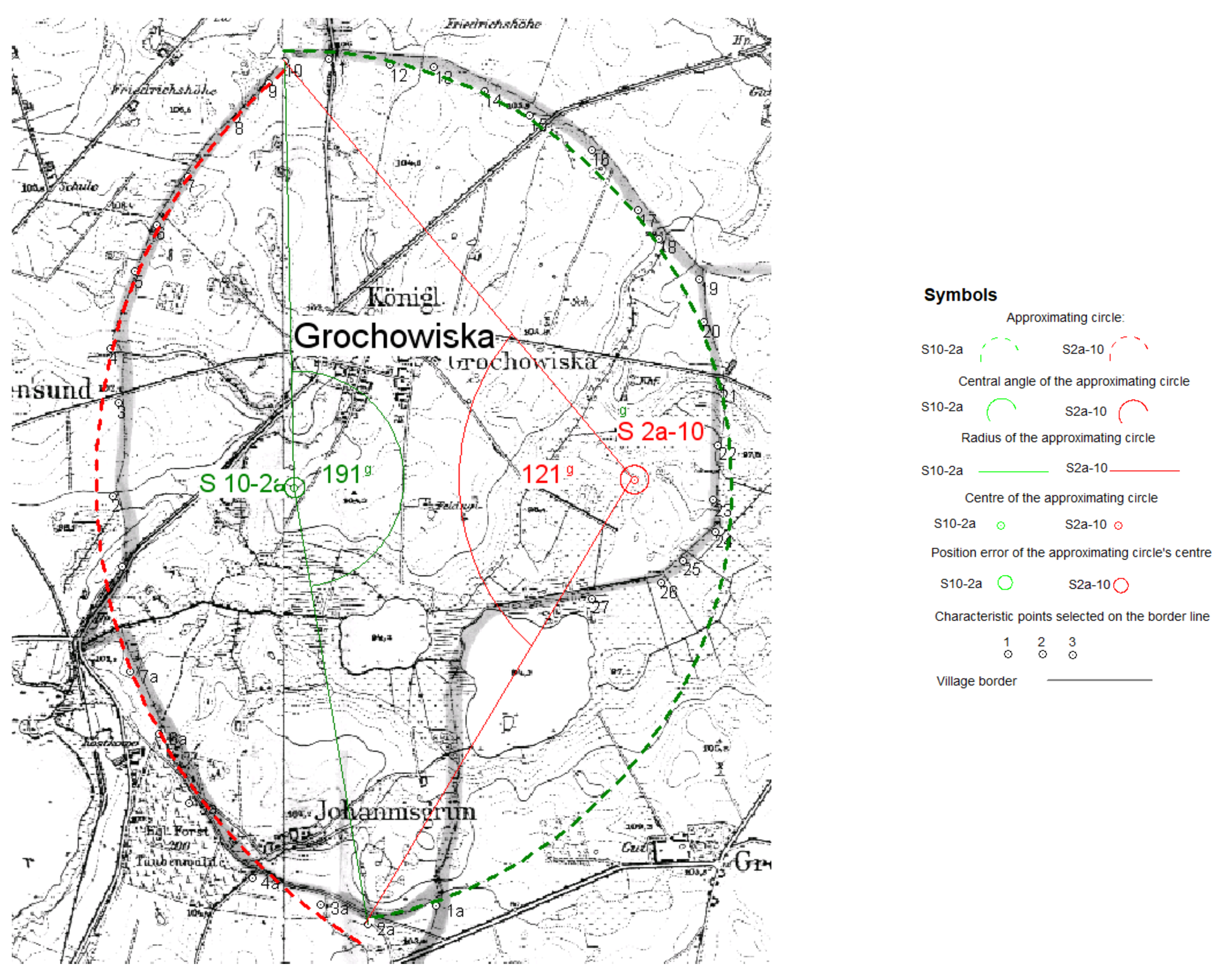
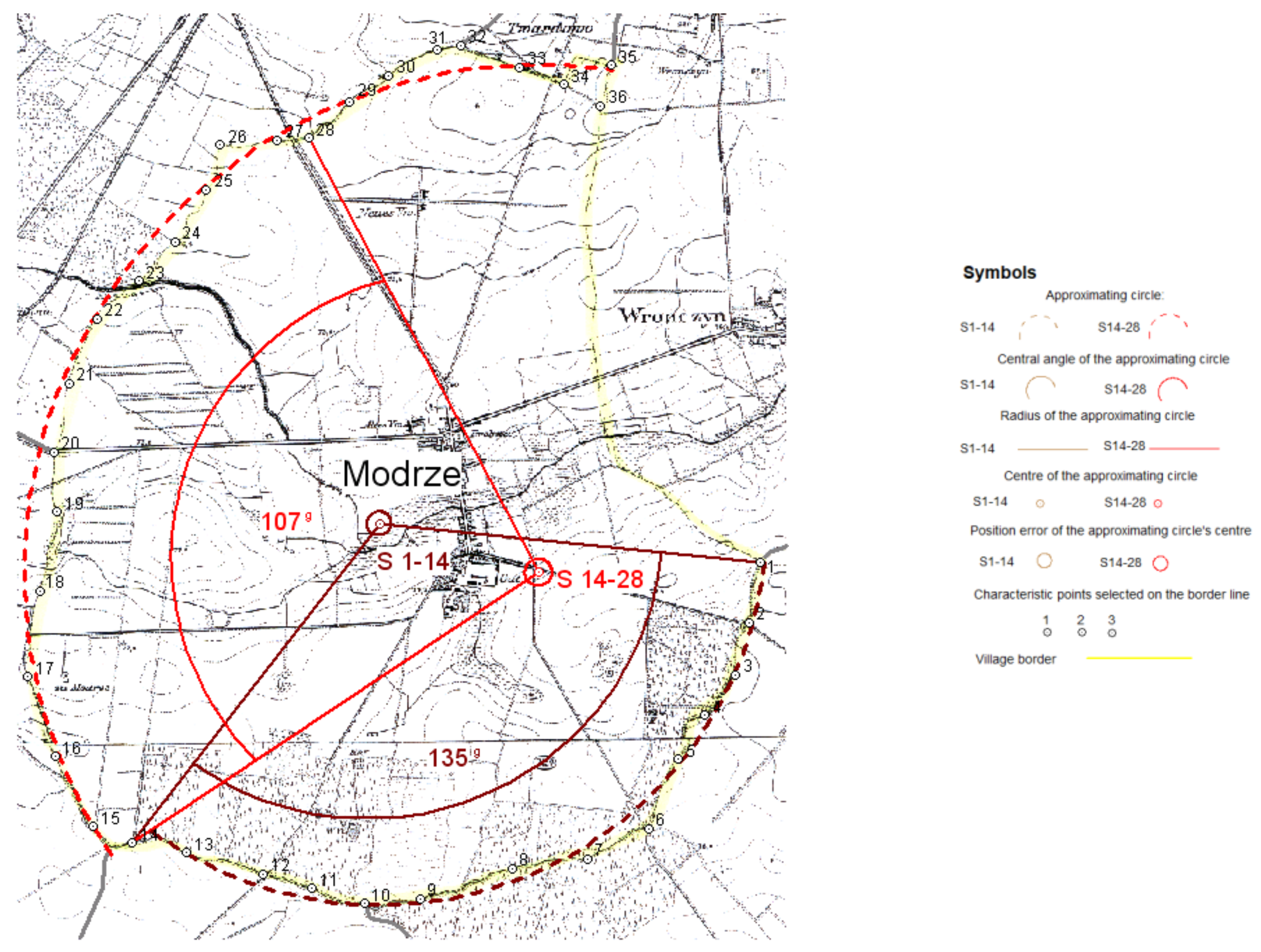
- Segments of the border were divided into parts with different curvature by approximating a circle to each part separately in the villages: Grochowiska (see Table 3, no. 7, 8, 9 and Figure 12 and Figure 16), Modrze (see Table 3, no. 10–11 and Figure 17). In both cases, the arcs’ centers were symbolized as the S point no.–the point where the number marked, respectively, the arcs’ endpoints, for example, S 3–15.
- 3.
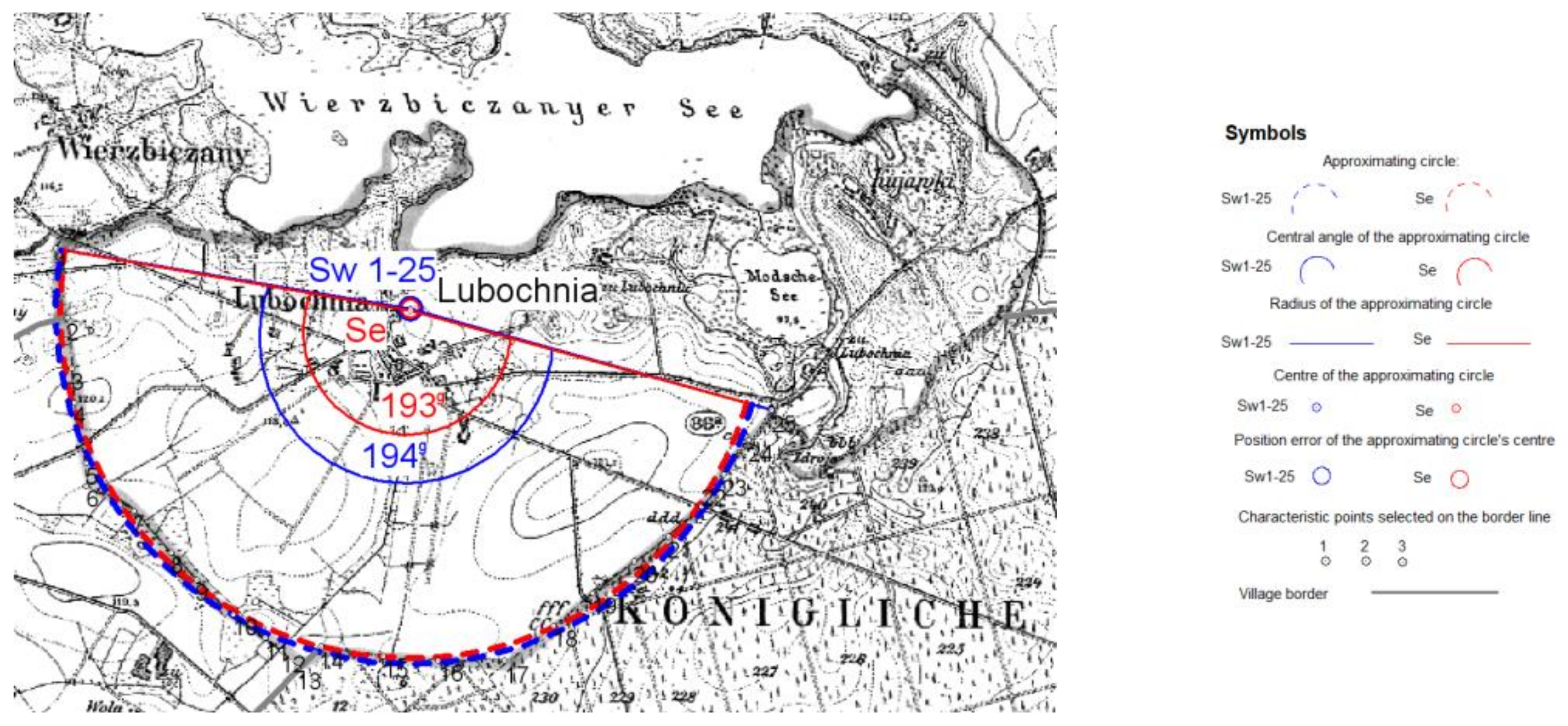
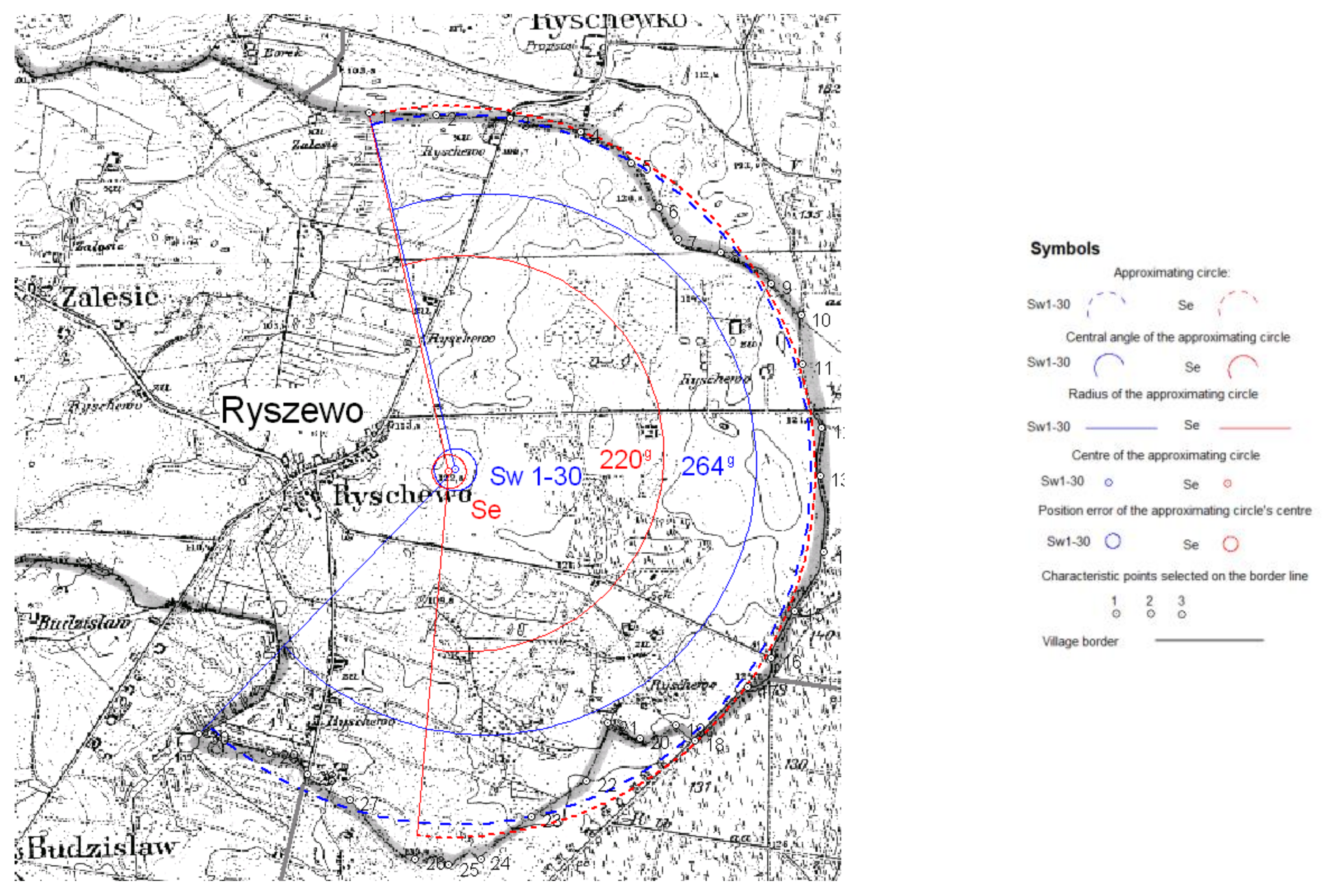
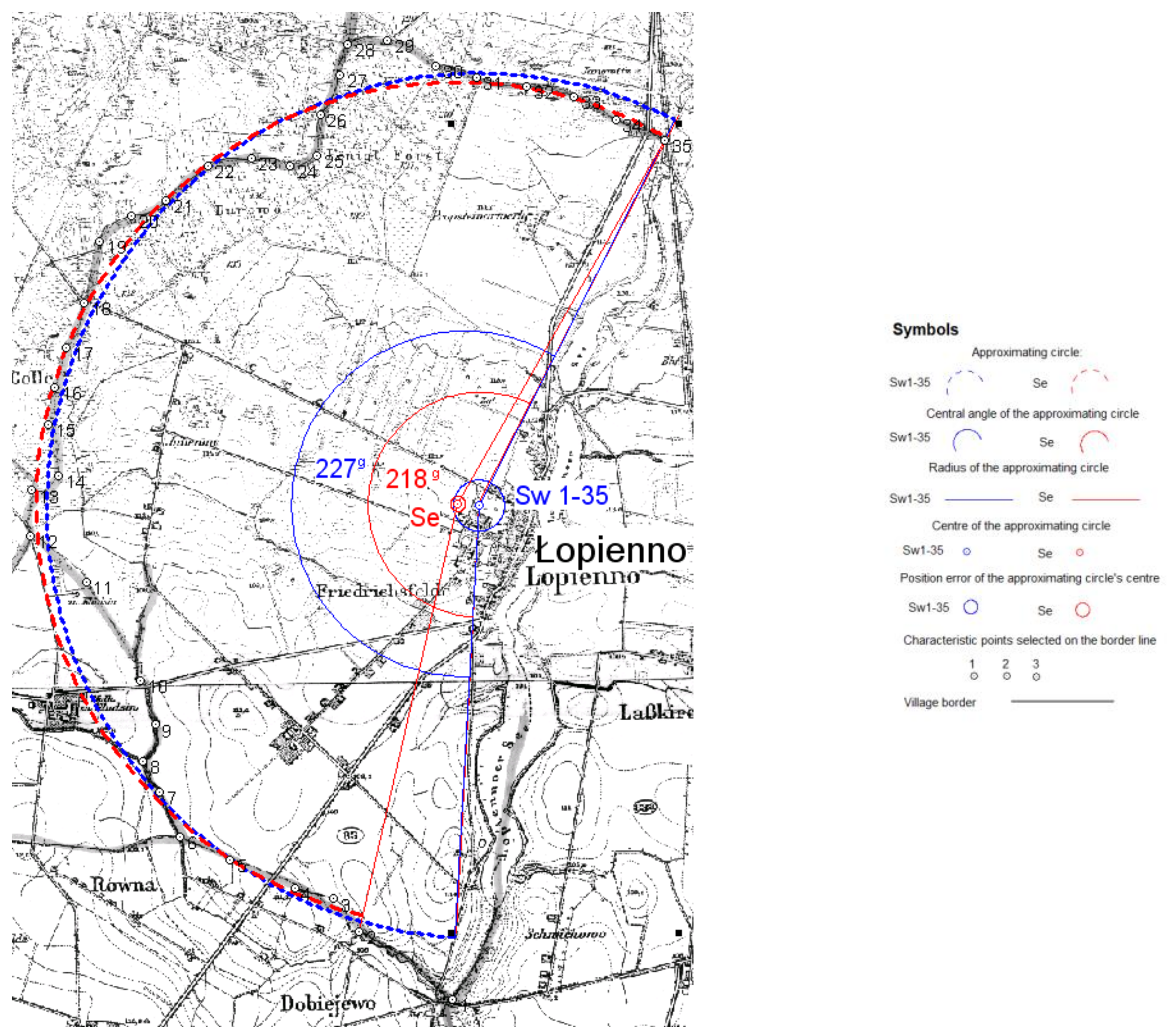
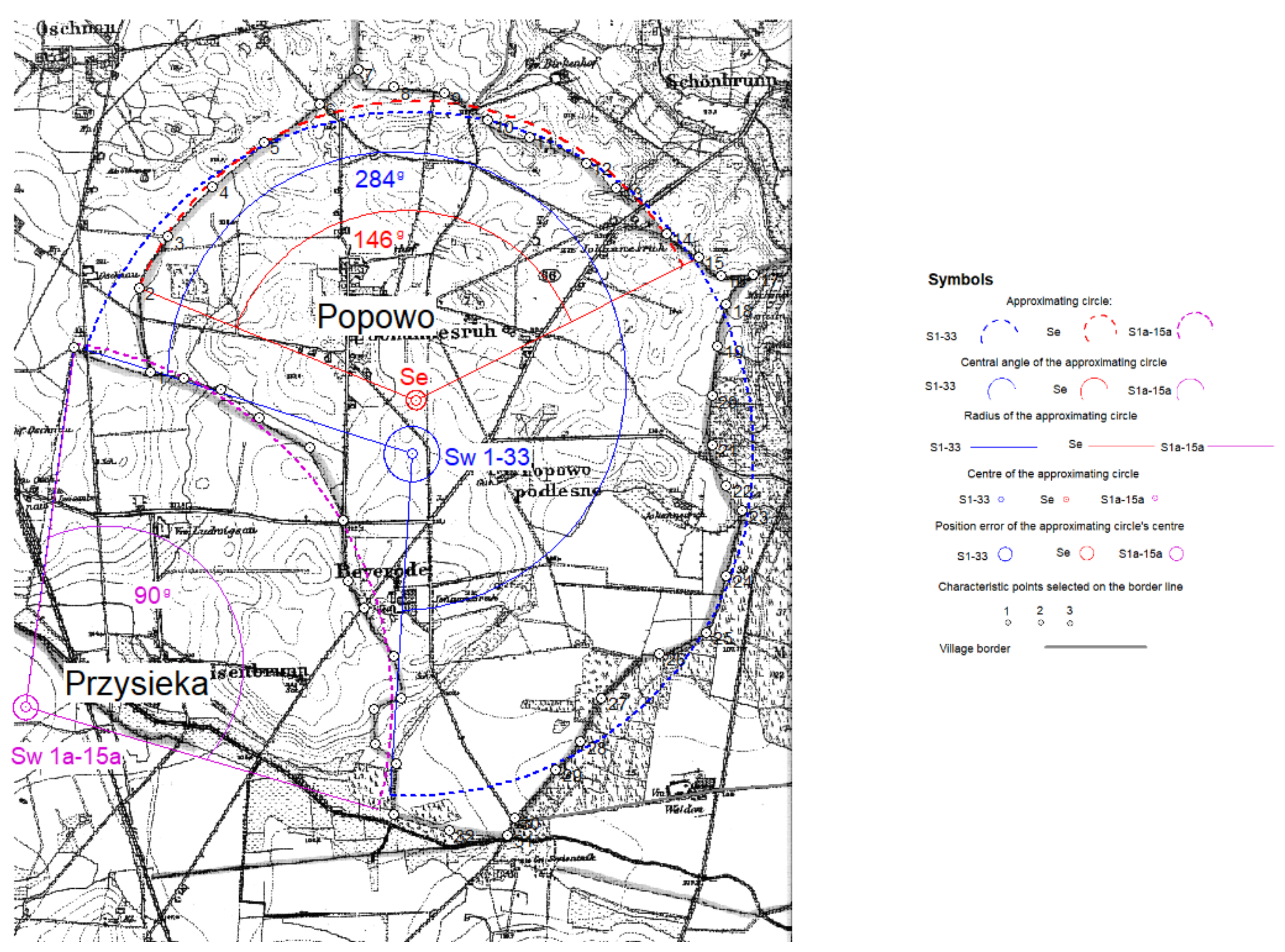
- Single points diverging from the border’s arc and points for which the difference between the actual radius at a given point and the radius of the approximating circle was very large were eliminated (over 100 m); Lubochnia (see Table 3, No. 12 and Figure 8), Łopienno (see Table 3, No. 13 and Figure 11), Popowo, (see Table 3, No. 14 and Figure 13), Ryszewo (see Table 3, No. 15 and Figure 10), Świniary (see Table 3, No. 16 and Figure 14). In this case, the arcs’ centers were indicated with Se.
4. Conclusions
- In seven cases, next to village buildings or in its center (Figure 11—Łopienno, Figure 6—Szemborowo, Figure 12—Grochowiska, Figure 8—Lubochnia, Figure 4—Wilkowyja, Figure 5—Zdziechowa, Figure 15 and Figure 17—Modrze). A comparison of the location of village buildings in 1:25,000 maps from the late nineteenth century and David Gilly’s map proves that it has not changed since the end of the eighteenth century. It can thus be assumed that it corresponds to their position from the demarcation times.
- In two cases (Figure 14–Świniary, Figure 13–Popowo and Przysieka), the arcs’ centers were outside the present village buildings–they might have been related to settlements that have not survived to our times. As for the boundaries of Popowo, whose name indicates an old origin of the village, we can assume that they arose as a result of more than just one ujazd.
Funding
Conflicts of Interest
References
- Scazzosi, L. Rural Landscape as Heritage: Reasons for and Implications of Principles Concerning Rural Landscapes as Heritage ICOMOS-IFLA 2017. Built Herit. 2018, 2, 39–52. [Google Scholar] [CrossRef]
- Picuno, P.; Cillis, G.; Statuto, D. Investigating the time evolution of a rural landscape: How historical maps may provide environmental information when processed using a GIS. Ecol. Eng. 2019, 139, 105580. [Google Scholar] [CrossRef]
- Zhang, L.; Guang, T.Z. Approach of Landscape Imagery Based-on Archetype Theory. Chin. Landsc. Archit. 2014, 5, 40–43. [Google Scholar]
- Tieskens, K.F.; Schulp, C.J.E.; Levers, C.; Lieskovský, J.; Kuemmerle, T.; Plieninger, T.; Verburg, P.H. Characterizing European cultural landscapes: Accounting for structure, management intensity and value of agricultural and forest landscapes. Land Use Policy 2017, 62, 29–39. [Google Scholar] [CrossRef] [Green Version]
- Lekakis, S.; Dragouni, M. Heritage in the making: Rural heritage and its mnemeiosis at Naxos Island, Greece. J. Rural Stud. 2020, 77, 84–92. [Google Scholar] [CrossRef]
- Wang, F.; Prominski, M. Landscapes with locality in urban or rural areas. Indoor Built Environ. 2020, 29, 1047–1052. [Google Scholar] [CrossRef]
- Żemła-Siesicka, A.; Myga-Piątek, U. A Landscape Persistence Assessment of Częstochowa Upland: A Case Study of Ogrodzieniec, Poland. Sustainability 2021, 13, 6408. [Google Scholar] [CrossRef]
- Myga-Piątek, U. Pamięć krajobrazu—Zapis dziejów w przestrzeni. Stud. Geohist. 2015, 3, 31–47. [Google Scholar] [CrossRef]
- Rippon, S. Understanding the Medieval Landscape. In Reflections: 50 Years of Medieval Archaeology, 1957–2007; Routledge: London, UK, 2018. [Google Scholar] [CrossRef]
- Simms, A. Historical landscape studies in Ireland. Belgeo 2004, 2–3, 301–320. [Google Scholar] [CrossRef] [Green Version]
- Cazzani, A.; Boriani, M. The Role of Historic Roads to Preserve and Valorize the Landscape. In Cycling & Walking for Regional Development; Springer: Cham, Switzerland, 2021. [Google Scholar] [CrossRef]
- Van Lanen, R.J.; Groenewoudt, B.J.; Spek, T.; Jansma, E. Route persistence. Modelling and quantifying historical route-network stability from the Roman period to early-modern times (AD 100–1600): A case study from the Netherlands. Archaeol. Anthropol. Sci. 2018, 10, 1037–1052. [Google Scholar] [CrossRef] [Green Version]
- Blanco, A.; De Bustamante, I.; Juan, P.-A. Using old cartography for the inventory of a forgotten heritage: The hydraulic heritage of the Community of Madrid. Sci. Total Environ. 2019, 665, 314–328. [Google Scholar] [CrossRef]
- He, F.; Li, S.; Zhang, X. A spatially explicit reconstruction of forest cover in China over 1700–2000. Glob. Planet. Chang. 2015, 131, 73–81. [Google Scholar] [CrossRef]
- Gimmi, U.; Lachat, T.; Bürgi, M. Reconstructing the Collapse of Wetland Networks in the Swiss Lowlands 1850–2000. Landsc. Ecol. 2011, 26, 1071. [Google Scholar] [CrossRef]
- Deng, X.; Li, Z. A review on historical trajectories and spatially explicit scenarios of land-use and land-cover changes in China. J. Land Use Sci. 2016, 11, 709–724. [Google Scholar] [CrossRef]
- Yuanyuan, Y.; Shuwen, Z.; Jiuchun, Y.; Liping, C.; Kun, B.; Xiaoshi, X. A review of historical reconstruction methods of land use/land cover. J. Geogr. Sci. 2014, 24, 746–766. [Google Scholar] [CrossRef]
- Turner, S.; Bolòs, J.; Kinnaird, T. Changes and continuities in a Mediterranean landscape: A new interdisciplinary approach to understanding historic character in western Catalonia. Landsc. Res. 2018, 43, 922–938. [Google Scholar] [CrossRef] [Green Version]
- Dolejš, M.; Nádvorník, J.; Raška, P.; Riezner, J. Frozen Histories or Narratives of Change? Contextualizing Land-Use Dynamics for Conservation of Historical Rural Landscapes. Environ. Manag. 2019, 63, 352–365. [Google Scholar] [CrossRef]
- Forejt, M.; Dolejš, M.; Raška, P. How reliable is my historical land-use reconstruction? Assessing uncertainties in old cadastral maps. Ecol. Indic. 2018, 94, 237–245. [Google Scholar] [CrossRef]
- Olsson, G.; Rogers, S.; Ballantyne, B.A. Surveys, Parcels and Tenure on Canada Lands; Surveyor General Branch, Natural Resources Canada: Edmonton, AB, Canada, 2010. [Google Scholar]
- Baker, A.R.H. Howard Levi Gray and English Field Systems: An Evaluation. Agric. Hist. 1965, 39, 86–91. [Google Scholar]
- Renes, H. Grainlands. The landscape of open fields in a European perspective. Landsc. Hist. 2010, 31, 37–70. [Google Scholar] [CrossRef]
- Libecap, G.D.; Lueck, D.; O’Grady, T. Large Scale Institutional Changes: Land Demarcation within the British Empire. National Bureau of Economic Research. 2010. Available online: https://www.researchgate.net/publication/46467501_Large_Scale_Institutional_Changes_Land_Demarcation_Within_the_British_Empire (accessed on 9 June 2021).
- Lueck, D.; Libecap, G.; Lopes, A. Land Demarcation Systems in California: A Legacy of History. SSRN Electron. J. 2012, 7. Available online: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=2093684 (accessed on 9 June 2021). [CrossRef]
- Lai, L.W.; Davies, S.N. Surveying was a kind of writing on the land: The economics of land division as town planning. Plan. Theory 2020, 19, 421–444. [Google Scholar] [CrossRef]
- Laschi, A.; Neri, F.; Montorselli, N.; Marchi, E. A Methodological Approach Exploiting Modern Techniques for Forest Road Network Planning. Croat. J. For. Eng. 2016, 37, 319–331. [Google Scholar]
- Angel, S.; Parent, J.; Civco, D.L. Ten compactness properties of circles: Measuring shape in geography. Can. Geogr. Géogr. Can. 2010, 54, 441–461. [Google Scholar] [CrossRef]
- Demetriou, D.; See, L.; Stillwell, J. A Parcel Shape Index for Use in Land Consolidation Planning. Trans. GIS 2012, 17, 861–882. [Google Scholar] [CrossRef]
- Foski, M. Using the parcel shape index to determine arable land division types. Acta Geogr. Slov. 2019, 59, 83–101. [Google Scholar] [CrossRef] [Green Version]
- Field Systems Introductions to Heritage Assets Published 31 October 2018. Available online: https://historicengland.org.uk/images-books/publications/iha-field-systems/heag204-field-systems/ (accessed on 1 June 2021).
- Lee, D.; Sallee, G. Theoretical Patterns of Farm Shape and Central Place Location. J. Reg. Sci. 1974, 14, 423–430. [Google Scholar] [CrossRef]
- Gorbenkova, E.; Shcherbina, E. Historical-Genetic Features in Rural Settlement System: A Case Study from Mogilev District (Mogilev Oblast, Belarus). Land 2020, 9, 165. [Google Scholar] [CrossRef]
- Nxumalo, C.; Whittal, J. Municipal Boundary Demarcation in South Africa: Processes and Effects on Governance in Traditional Rural Areas. S. Afr. J. Geomat. 2013, 2, 325–341. [Google Scholar]
- Verspay, J.P.W.; Londen, H.; Symonds, J.; Renes, J.; Huijbers, A.M.J.H. Village Formation in The Netherlands during the Middle Ages (AD 800–1600) an Assessment of Recent Excavations and a Path to Progress; Agency of the Netherlands Cultural Heritage: Paris, France, 2018. [Google Scholar] [CrossRef]
- Demidowicz, G. Planned landscapes in north-east Poland: The Suraż estate, 1550–1760. J. Hist. Geogr. 1985, 11, 21–47. [Google Scholar] [CrossRef]
- Libecap, G.; Lueck, D. The Demarcation of Land and the Role of Coordinating Property Institutions. J. Political Econ. 2011, 119, 426. [Google Scholar] [CrossRef] [Green Version]
- Ingvild, Ø. Settlement patterns and field systems in medieval Norway. Landsc. Hist. 2012, 30, 37–54. [Google Scholar] [CrossRef]
- Hall, D. The Open Fields of Northamptonshire. Northamptonshire Record Society. 1995. Available online: http://www.northamptonshirerecordsociety.org.uk/nrseBksOpenFields.html (accessed on 1 June 2021).
- Widgren, M. Can Landscapes be Read. In European Rural Landscapes: Persistence and Change in a Globalising Environment; Palang, H., Sooväli, H., Antrop, M., Setten, G., Eds.; Springer: Berlin, Germany, 2004; pp. 455–465. [Google Scholar] [CrossRef]
- Hansen Roeck, B. Early agrarian landscapes in Finland. Geogr. Ann. Ser. B Hum. Geogr. 1998, 80, 187–201. [Google Scholar] [CrossRef]
- Kerekes, A.; Mircea, A. Evaluating urban sprawl and land-use change using remote sensing, gis techniques and historical maps. Case study: The city of dej, Romania. An. Univ. Oradea Ser. Geogr. 2019, 29, 52–63. [Google Scholar] [CrossRef]
- Stojković, S. GIS analysis of land use changes: Case study: The Stara Pazova municipality, Serbia. Zb. Rad. Geogr. Fak. Univ. Beogr. 2017, 65, 295–306. [Google Scholar] [CrossRef]
- Statuto, D.; Cillis, G.; Picuno, P. Historical cartography and gis tools for the analysis of land use and landscape changes. In Proceedings of the 43rd Symposium “Actual Tasks on Agricultural Engineering”, Opatija, Croatia, 24–27 February 2015. [Google Scholar]
- Statuto, D.; Cillis, G.; Picuno, P. Analysis of the effects of agricultural land use change on rural environment and landscape through historical cartography and GIS tools. J. Agric. Eng. 2016, 47, 28–39. [Google Scholar] [CrossRef] [Green Version]
- Biger, G. Historical Geography and International Boundaries. J. Geogr. Politics Soc. 2012, 29, 69–77. [Google Scholar] [CrossRef]
- Yang, Y.; Zhang, S.; Liu, Y. Analyzing historical land use changes using a Historical Land Use Reconstruction Model: A case study in Zhenlai County, northeastern China. Sci. Rep. 2017, 7, 41275. [Google Scholar] [CrossRef]
- Oliskiewicz-Krzywicka, A. The beginnings of the formation of rural cadastral boundaries in Poland. Geod. Rev. 1998, 71, 14–17. [Google Scholar]
- Bujak, F. Studies on Settlements Małopolska; Academy of Learning: Kraków, Poland, 1905; Volume I. [Google Scholar]
- Łaguna, S. On Polish Border Law; Library of Legal Skills: Warsaw, Poland, 1875. [Google Scholar]
- Szulc, H. On morphological typologies of rural settlements in Poland. Geogr. Rev. 1975, 47, 627–636. [Google Scholar]
- Urbańczyk, S. Dictionary of Old Polish; Tom, I.X., Ed.; The Ossolińskis National Institute im. Ossolińskich: Kraków, Poland, 1982–1987.
- Smolka, S. Mieszko Stary and His Age; State Scientific Publishers: Warszawa, Poland, 1929. [Google Scholar]
- Burszta, J. From Slavic Settlement to Modern Village; The Ossolińskis National Institute im. Ossolińskich: Wrocław, Poland, 1959. [Google Scholar]
- Sulimierski, F.; Chlebowski, B.; Walewski, W.; Krzywicki, J. Geographical Dictionary of the Polish Kingdom and Other Slavic Countries; Print Age: Warsaw, Poland, 1880–1914; Volume 1–15, Available online: http://dir.icm.edu.pl/pl/Slownik_geograficzny/ (accessed on 1 May 2020).
- Jurek, T. Historical Geographical Dictionary of the Polish Lands in the Middle Ages (Poznań Region); Institute of History of the Polish Academy of Sciences Since 1982: Poznań, Poland, 1987–2019; Available online: http://www.slownik.ihpan.edu.pl/ (accessed on 1 May 2020).
- Hładyłowicz, K.J. Landscape changes and settlement development in Wielkopolska from the 15th to the 19th century. In Studies in Social and Economic History; Wiśniewski, L., Ed.; Printing House: Lwów, Ukraine, 1932; Volume 12. [Google Scholar]
- Gil, J. Approximation of Measurement Results by Circle Equation, Version 1.0; University of Zielona Góra: Zielona Góra, Poland, 2002.
- Fernández, J.F.; Fernández Mier, M. The Archaeology of Medieval Villages Currently Inhabited in Europe; Archaeopress Publishing: Oxford, UK, 2019. [Google Scholar] [CrossRef]
- Delimitation and Demarcation of Boundaries in Africa: General Issues and Case Studies. Available online: https://www.peaceau.org/uploads/au-2-en-2013-delim-a-demar-user-guide.pdf (accessed on 1 May 2021).
- Stojan, S. The establishment and demarcation of borders in europe, in the early Modern Age. Res. Sci. Today Suppl. 2014, 2, 6–14. Available online: https://www.rstjournal.com/mdocs-posts/01-silviu-stoian-the-establishment-and-demarcation-of-borders-in-europe-in-the-early-modern-age/ (accessed on 1 May 2021).
- Di Fazio, S.; Modica, G. Historic Rural Landscapes: Sustainable Planning Strategies and Action Criteria. The Italian Experience in the Global and European Context. Sustainability 2018, 10, 3834. [Google Scholar] [CrossRef] [Green Version]
- Lipský, Z. Historical Development of czecH Rural Landscape: Implications for Present Landscape Planning. Landscape Ecology: Theory and Applications for Practical Purposes. 2000. Available online: https://www.researchgate.net/publication/242556546_Historical_development_of_czecH_rural_landscape_implications_for_present_landscape_planni (accessed on 1 May 2021).



| Distances between Points on the Objects [m]: | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Points | Glinka | Gołaszewo | Grochowiska | Lubochnia | Łopienno | Zdziechowa | Modrze | Popowo | Przysieka | Ryszewo | Świniary | Szemborowo | Wilkowyja |
| No. | |||||||||||||
| 1–2 | 230 | 300 | 350 | 300 | 770 | 310 | 420 | 620 | 360 | 500 | 130 | 250 | 240 |
| 2–3 | 310 | 220 | 460 | 220 | 280 | 320 | 360 | 430 | 210 | 320 | 270 | 280 | 200 |
| 3–4 | 210 | 250 | 270 | 150 | 270 | 300 | 340 | 480 | 250 | 320 | 200 | 290 | 250 |
| 4–5 | 150 | 170 | 400 | 270 | 470 | 290 | 350 | 490 | 210 | 350 | 170 | 420 | 220 |
| 5–6 | 130 | 200 | 260 | 100 | 360 | 420 | 510 | 490 | 300 | 340 | 250 | 390 | 210 |
| 6–7 | 260 | 240 | 280 | 240 | 330 | 180 | 460 | 380 | 410 | 280 | 280 | 440 | 180 |
| 7–8 | 150 | 230 | 370 | 240 | 240 | 220 | 510 | 290 | 230 | 250 | 190 | 350 | 170 |
| 8–9 | 160 | 280 | 240 | 160 | 250 | 200 | 640 | 370 | 440 | 180 | 310 | 470 | 90 |
| 9–10 | 180 | 220 | 140 | 220 | 310 | 260 | 370 | 370 | 580 | 210 | 290 | 270 | 200 |
| 10–11 | 160 | 270 | 220 | 170 | 750 | 280 | 360 | 320 | 420 | 280 | 190 | 230 | 210 |
| 11–12 | 240 | 210 | 300 | 30 | 480 | 280 | 340 | 450 | 350 | 210 | 200 | 480 | 170 |
| 12–13 | 170 | 260 | 220 | 100 | 300 | 230 | 530 | 280 | 280 | 230 | 290 | 470 | 150 |
| 13–14 | 80 | 310 | 280 | 140 | 210 | 180 | 370 | 490 | 240 | 310 | 350 | 370 | 220 |
| 14–15 | 220 | 170 | 250 | 280 | 350 | 390 | 280 | 290 | 580 | 230 | 290 | 280 | 200 |
| 15–16 | 350 | 190 | 350 | 240 | 250 | 310 | 530 | 210 | 360 | 350 | 380 | 210 | |
| 16–17 | 150 | 300 | 380 | 290 | 270 | 180 | 570 | 240 | 310 | 310 | 270 | 210 | |
| 17–18 | 290 | 330 | 170 | 260 | 320 | 220 | 580 | 300 | 250 | 300 | 290 | 230 | |
| 18–19 | 340 | 340 | 280 | 220 | 420 | 300 | 550 | 310 | 180 | 310 | 300 | 250 | |
| 19–20 | 150 | 238 | 210 | 230 | 270 | 340 | 400 | 360 | 350 | 310 | 350 | ||
| 20–21 | 90 | 380 | 330 | 190 | 250 | 260 | 470 | 350 | 110 | 250 | 360 | ||
| 21–22 | 330 | 350 | 290 | 200 | 360 | 210 | 480 | 320 | 180 | 260 | |||
| 22–23 | 200 | 350 | 270 | 170 | 290 | 120 | 380 | 220 | 170 | 290 | |||
| 23–24 | 210 | 350 | 160 | 190 | 270 | 230 | 350 | 490 | 290 | 160 | |||
| 24–25 | 310 | 390 | 210 | 170 | 190 | 310 | 410 | 430 | 310 | ||||
| 25–26 | 210 | 420 | 160 | 270 | 250 | 310 | 370 | 310 | |||||
| 26–27 | 290 | 370 | 350 | 300 | 300 | 380 | 530 | 150 | |||||
| 27–28 | 350 | 380 | 210 | 290 | 210 | 350 | 160 | ||||||
| 28–29 | 370 | 240 | 270 | 430 | 360 | 270 | 420 | ||||||
| 29–30 | 300 | 330 | 360 | 340 | 320 | 460 | 230 | ||||||
| 30–31 | 150 | 330 | 280 | 350 | 360 | 130 | 210 | ||||||
| 31–32 | 130 | 280 | 350 | 360 | 160 | 420 | 340 | ||||||
| 32–33 | 280 | 320 | 160 | 420 | 420 | 350 | |||||||
| 33–34 | 280 | 320 | 280 | 320 | 270 | ||||||||
| 34–35 | 170 | 360 | 250 | 340 | 240 | ||||||||
| 35–36 | 280 | 290 | 300 | ||||||||||
| 36–37 | 340 | 370 | |||||||||||
| 37–38 | 280 | 470 | |||||||||||
| 38–39 | 330 | ||||||||||||
| 39–40 | 280 | ||||||||||||
| 40–41 | 300 | ||||||||||||
| 41–42 | 370 | ||||||||||||
| 42–43 | 180 | ||||||||||||
| 43–44 | 360 | ||||||||||||
| Mean | |||||||||||||
| distance | 220 | 280 | 280 | 200 | 330 | 280 | 400 | 370 | 350 | 280 | 260 | 330 | 200 |
| No. | Village | α [g] | τ [m] | Number of Points | R [m] | ±mo [m] | P [ha] | Approximated Points | Figure Number |
|---|---|---|---|---|---|---|---|---|---|
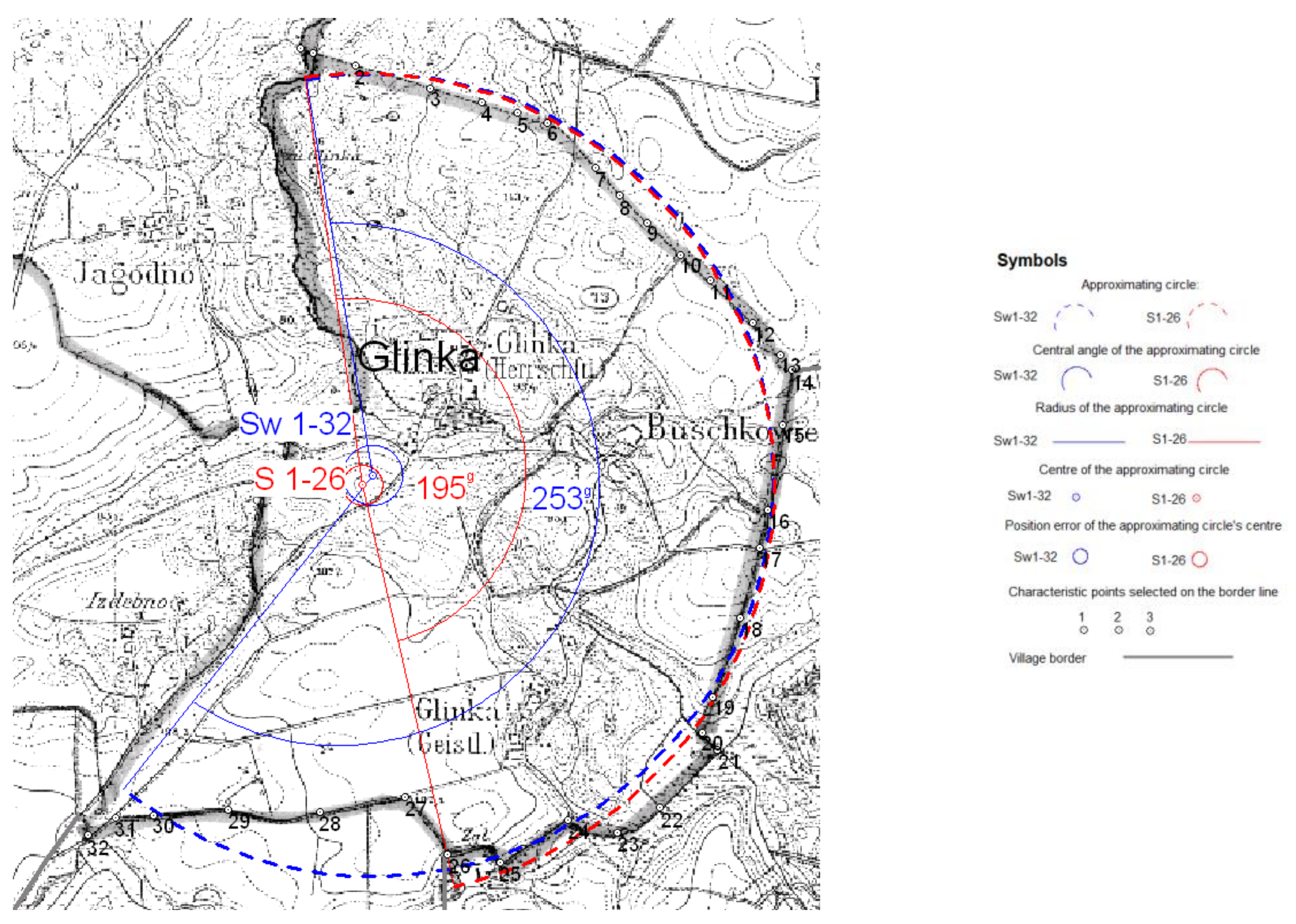
| 1 | Glinka Sw 1–32 | 253 | 6400 | 33 | 1610 | 120 | 611 | all points | 9 |
| 2 | Gołaszewo Sw 1–35 | 294 | 8210 | 35 | 1780 | 160 | 909 | all points | 7 |
| 3 | Grochowiska Sw 7a–27 | 290 | 7010 | 28 | 1540 | 90 | 647 | all points | 12 |
| 4 | Lubochnia Sw 1–25 | 194 | 4690 | 25 | 1540 | 50 | 339 | all points | 8 |
| 5 | Łopienno Sw 1–35 | 227 | 10,260 | 35 | 2880 | 170 | 1641 | all points | 11 |
| 6 | Modrze Sw 1–36 | 315 | 17,000 | 36 | 2690 | 250 | 1935 | points 1–36 | 15 |
| 7 | Popowo Sw 1–33 | 284 | 10,630 | 33 | 2470 | 200 | 1580 | all points | 13 |
| 8 | Przysieka Sw 1a–15a | 90 | 3740 | 15 | 2650 | 90 | 241 | all points | 13 |
| 9 | Ryszewo Sw 1–30 | 264 | 6960 | 30 | 1680 | 100 | 941 | all points | 10 |
| 10 | Szemborowo Sw 1–24 | 244 | 7430 | 24 | 1940 | 130 | 837 | all points | 6 |
| 11 | Świniary Sw 1–21 | 205 | 4890 | 21 | 1520 | 80 | 378 | all points | 14 |
| 12 | Wilkowyja Sw 1–19 | 225 | 3360 | 19 | 950 | 70 | 174 | all points | 4 |
| 13 | Zdziechowa Sw 1–28, 37–44 | 334 | 11,540 | 36 | 2200 | 120 | 1457 | all without 29-36 | 5 |
| mean | 248 | 7860 | 28 | 1960 | 130 | 899 |
| No. | Village | α [g] | τ [m] | Number of Points | R [m] | ±mo [m] | Approximated Points | Figure Number |
|---|---|---|---|---|---|---|---|---|
| 1. Elimination of segments of different curvature. | ||||||||
| 1 | Glinka S 1–26 | 195 | 5050 | 27 | 1650 | 80 | points 1–26 | 9 |
| 2 | Gołaszewo S 15–35 | 210 | 5900 | 21 | 1790 | 90 | points 15–35 | 7 |
| 3 | Szemborowo S 2–16 | 183 | 5060 | 15 | 1760 | 60 | points 2–16 | 6 |
| 4 | Wilkowyja S 2–19 | 204 | 3170 | 18 | 990 | 50 | points 2–19 | 4 |
| 5 | Zdziechowa S 1–23 | 136 | 5640 | 23 | 2640 | 60 | points 1–23 | 5 |
| 6 | Zdziechowa S 1–17 | 100 | 4150 | 17 | 2640 | 60 | points 1–17 | 5 |
| mean | 171 | 4830 | 20 | 1910 | 70 | |||
| 2. Parts of the borders of different curvature, approximated separately | ||||||||
| 7 | Grochowiska S 2a–10 | 121 | 5050 | 16 | 2660 | 70 | points 2a–10 (2a–7a and 1–10) | 16 |
| 8 | Grochowiska S 10–2a | 191 | 6480 | 17 | 2160 | 50 | points 10–2a | 16 |
| 9 | Grochowiska S 10–24 | 125 | 3670 | 15 | 1870 | 20 | points 10–24 | 12 |
| 10 | Modrze S 1–14 | 135 | 5450 | 14 | 2570 | 80 | points 1–14 | 17 |
| 11 | Modrze S 14–28 | 107 | 5750 | 15 | 3420 | 90 | points 14–28 | 17 |
| mean | 140 | 5280 | 15 | 2540 | 60 | |||
| 3. Elimination of single points on the boundary line | ||||||||
| 12 | Lubochnia Se | 193 | 4610 | 23 | 1520 | 40 | All points without 13, 25 | 8 |
| 13 | Łopienno Se | 227 | 9620 | 19 | 2810 | 50 | points 2–35, without 9–14, 19, 23–25, 27–30 | 11 |
| 14 | Popowo Se | 146 | 4950 | 13 | 2160 | 70 | points 2–15, without 7 | 13 |
| 15 | Ryszewo Se | 220 | 5980 | 23 | 1730 | 80 | points 3–28, without 8, 9, 23 | 10 |
| 16 | Świniary Se | 205 | 4890 | 19 | 1520 | 50 | all points without 9, 12 | 14 |
| mean | 198 | 6010 | 19 | 1950 | 60 | |||
| Mean for all | 169 | 5340 | 18 | 2120 | 60 | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oliskiewicz-Krzywicka, A. Demarcation Technique and Geometric Analysis of Village Boundaries Resulting from Delineation of Land Areas (ujazd) in the Middle Ages in the Wielkopolska Region (Poland). Land 2021, 10, 623. https://doi.org/10.3390/land10060623
Oliskiewicz-Krzywicka A. Demarcation Technique and Geometric Analysis of Village Boundaries Resulting from Delineation of Land Areas (ujazd) in the Middle Ages in the Wielkopolska Region (Poland). Land. 2021; 10(6):623. https://doi.org/10.3390/land10060623
Chicago/Turabian StyleOliskiewicz-Krzywicka, Anna. 2021. "Demarcation Technique and Geometric Analysis of Village Boundaries Resulting from Delineation of Land Areas (ujazd) in the Middle Ages in the Wielkopolska Region (Poland)" Land 10, no. 6: 623. https://doi.org/10.3390/land10060623
APA StyleOliskiewicz-Krzywicka, A. (2021). Demarcation Technique and Geometric Analysis of Village Boundaries Resulting from Delineation of Land Areas (ujazd) in the Middle Ages in the Wielkopolska Region (Poland). Land, 10(6), 623. https://doi.org/10.3390/land10060623





