Neutrosophic Triplet Non-Associative Semihypergroups with Application
Abstract
:1. Introduction
2. Preliminaries
3. Neutrosophic Triplet -Semihypergroups
- left neutrosophic triplet set if for every , there exist and such that
- right neutrosophic triplet set if for every , there exist and such that
- neutrosophic triplet set if for every , there exist and such that
- pure left neutrosophic triplet set if for every , there exist and such that
- pure right neutrosophic triplet set if for every , there exist and such that
- pure neutrosophic triplet set if for every , there exist and such that
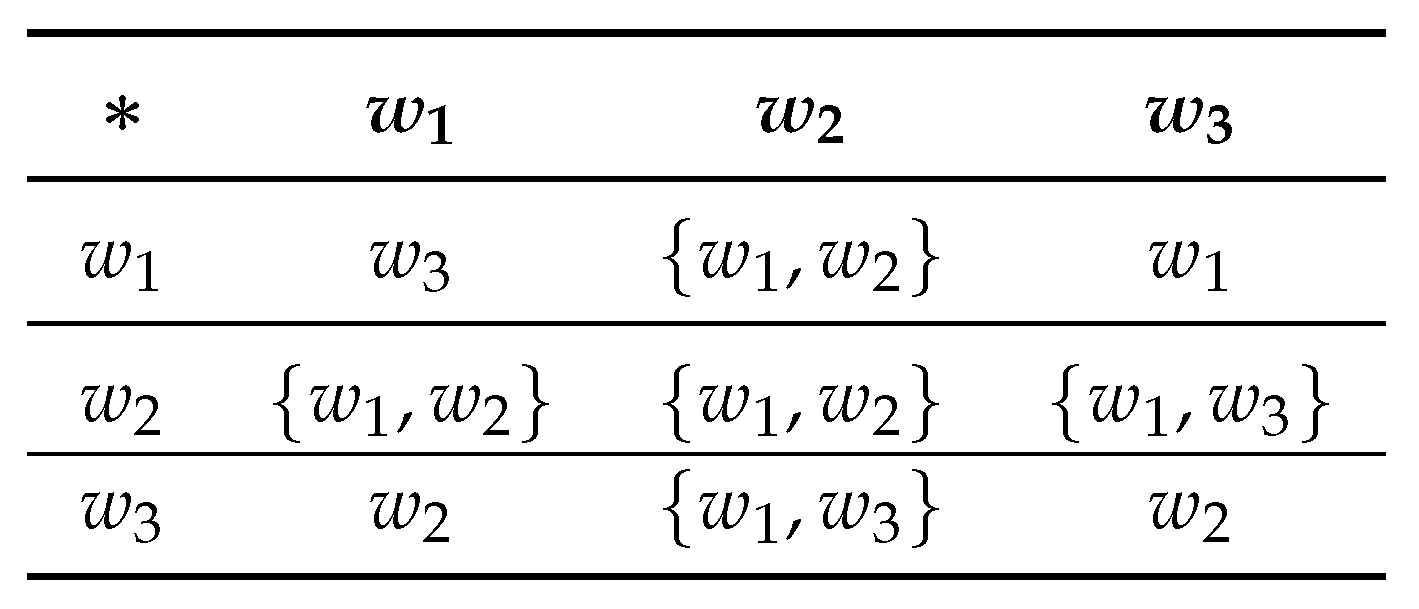
- is well defined.
- satisfies the left invertive law.

- is a well defined.
- satisfies the left invertive law.

- for all
- for all

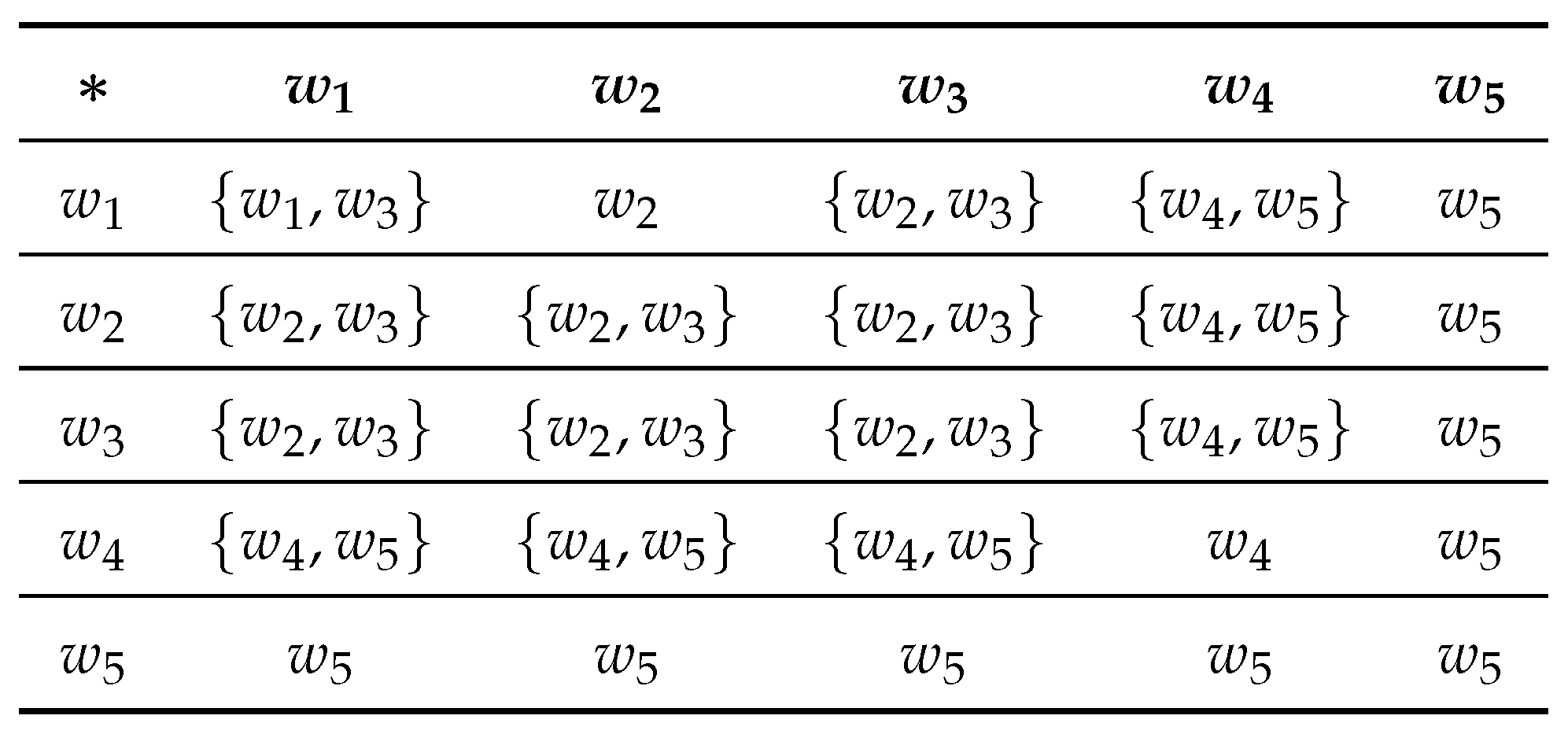
- K is a neutrosophic triplet -semihypergroup.
- For all
- The image of f is a neutrosophic triplet -subsemihypergroup of
- The inverse image of f is a neutrosophic -subsemihypergroup of .
- Every neutrosophic triplet -semihypergroup is an -semihypergroup, but the reverse may or may not true.
- In neutrosophic triplet -semihypergroup, every element must have a left but in an -semihypergroup the left of an element may or may not exist.
- In neutrosophic -semihypergroup, every element must have left but in an -semihypergroup the element may or may not have semihypergroup.
- In neutrosophic -semihypergroup pure left is not equal to pure left Identity.
4. Application
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Smarandache, F. A Unifying Field in Logics. Neutrosophy, Neutrosophic Probability, Set and Logic; Rehoboth American Research Press: Rehoboth, NM, USA, 1999. [Google Scholar]
- Kandasamy, W.B.V.; Smarandache, F. Some Neutrosophic Algebraic Structures and Neutrosophic N-Algebraic Structures; Hexis: Phoenix, AZ, USA, 2006; p. 219. [Google Scholar]
- Kandasamy, W.B.V.; Smarandache, F. N-Algebraic Structures And S-N-Algebraic Structures; Hexis: Phoenix, AZ, USA, 2006; p. 209. [Google Scholar]
- Kandasamy, W.B.V.; Smarandache, F. Basic Neutrosophic Algebraic Structures and Their Applications to Fuzzy and Neutrosophic Models; Hexis: Phoenix, AZ, USA, 2004; p. 149. [Google Scholar]
- Al-Quran, A.; Hassan, N. The complex neutrosophic soft expert set and its application in decision making. J. Intell. Fuzzy Syst. 2018, 34, 569–582. [Google Scholar] [CrossRef]
- Al-Quran, A.; Hassan, N. The complex neutrosophic soft expert relation and its multiple attribute decision-making method. Entropy 2018, 20, 101. [Google Scholar] [CrossRef]
- Abu Qamar, M.; Hassan, N. Q-neutrosophic soft relation and its application in decision making. Entropy 2018, 20, 172. [Google Scholar]
- Abu Qamar, M.; Hassan, N. Entropy, measures of distance and similarity of Q-neutrosophic soft sets and some applications. Entropy 2018, 20, 672. [Google Scholar]
- Uluçay, V.; Sahin, M.; Hassan, N. Generalized neutrosophic soft expert set for multiple-criteria decision-making. Symmetry 2018, 10, 437. [Google Scholar] [CrossRef]
- Smarandache, F. Neutrosophic Perspectives: Triplets, Duplets, Multisets, Hybrid Operators, Modal Logic, Hedge Algebras. And Applications; Pons Publishing House: Brussels, Belgium, 2017. [Google Scholar]
- Zhang, X.H.; Smarandache, F.; Liang, X.L. Neutrosophic duplet semi-group and cancellable neutrosophic triplet groups. Symmetry 2017, 9, 275. [Google Scholar] [CrossRef]
- Bal, M.; Shalla, M.M.; Olgun, N. Neutrosophic triplet cosets and quotient groups. Symmetry 2018, 10, 126. [Google Scholar] [CrossRef]
- Jaiyeola, T.G.; Smarandache, F. Some results on neutrosophic triplet group and their applications. Symmetry 2018, 10, 202. [Google Scholar] [CrossRef]
- Smarandache, F.; Ali, M. Neutrosophic triplet group. Neural Comput. Appl. 2018, 29, 595–601. [Google Scholar] [CrossRef]
- Marty, F. Sur une generalization de la notion de groupe. In Proceedings of the 8th Congres des Mathematicians Scandinaves, Stockholm, Sweden; 1934; pp. 45–49. [Google Scholar]
- Corsini, P. Prolegomena of Hypergroup Theory; Aviani Editore: Udine, Italy, 1993. [Google Scholar]
- Vougiouklis, T. Hyperstructures and Their Representations; Hadronic Press: Palm Harbor, FL, USA, 1994. [Google Scholar]
- Corsini, P.; Leoreanu, V. Applications of Hyperstructure Theory; Kluwer Academic: Dordrecht, The Netherlands, 2003. [Google Scholar]
- Vougiouklis, T. A new class of hyperstructures. J. Comb. Inf. Syst. Sci. 1995, 20, 229–235. [Google Scholar]
- Vougiouklis, T. ∂-operations and Hv-fields. Acta Math. Sin. (Engl. Ser.) 2008, 24, 1067–1078. [Google Scholar] [CrossRef]
- Vougiouklis, T. The h/v-Structures, Algebraic Hyperstructures and Applications; Taru Publications: New Delhi, India, 2004; pp. 115–123. [Google Scholar]
- Spartalis, S. On Hv-semigroups. Ital. J. Pure Appl. Math. 2002, 11, 165–174. [Google Scholar]
- Spartalis, S. On reversible Hv-group. In Proceedings of the 5th International Congress on Algebraic Hyperstructures and Applications, Jasi, Romania, 4–10 July 1993; pp. 163–170. [Google Scholar]
- Vougiouklis, T. The fundamental relation in hyperrings: The general hyperfield. In Proceedings of the Algebraic Hyperstructures and Applications, Xanthi, Greece, April 1991; pp. 203–211. [Google Scholar]
- Spartalis, S. Quoitients of P-Hv-rings. In New Frontiers in Hyperstructures; Hadronic Press: Palm Harbor, FL, USA, 1996; pp. 167–176. [Google Scholar]
- Spartalis, S.; Vougiouklis, T. The fundamental relations on Hv-rings. Riv. Mat. Pura Appl. 1994, 7, 7–20. [Google Scholar]
- Spartalis, S. On the number of Hv-rings with P-hyperoperations. Discret. Math. 1996, 155, 225–231. [Google Scholar] [CrossRef]
- Davvaz, B.; Fotea, V.L. Hyperring Theory and Applications; International Academic Press: New York, NY, USA, 2007. [Google Scholar]
- Kazim, M.A.; Naseerudin, N. On almost semigroups. Aligarh Bull. Math. 1972, 2, 41–47. [Google Scholar]
- Kamran, M.S. Conditions for LA-Semigroups to Resemble Associative Structures. Ph.D. Thesis, Quaid-i-Azam University, Islamabad, Pakistan, 1993. [Google Scholar]
- Protic, P.V.; Stevanovic, N. AG-test and some general properties of Abel-Grassmann’s groupoids. Pure Math. Appl. 1995, 6, 371–383. [Google Scholar]
- Protic, P.V.; Stevanovic, N. The structural theorem for AG*-groupoids. Ser. Math. Inform. 1995, 10, 25–33. [Google Scholar]
- Yusuf, S.M. On Left Almost Ring. In Proceedings of the 7th International Pure Mathematics Conference, Islamabad, Pakistan, 5–7 August 2006. [Google Scholar]
- Hila, K.; Dine, J. On hyperideals in left almost semihypergroups. ISRN Algebra 2011, 2011, 953124. [Google Scholar] [CrossRef]
- Yaqoob, N.; Corsini, P.; Yousafzai, F. On intra-regular left almost semihypergroups with pure left identity. J. Math. 2013, 2013, 510790. [Google Scholar] [CrossRef]
- Yousafzai, F.; Hila, K.; Corsini, P.; Zeb, A. Existence of non-associative algebraic hyperstructures and related problems. Afr. Mat. 2015, 26, 981–995. [Google Scholar] [CrossRef]
- Amjad, V.; Hila, K.; Yousafzai, F. Generalized hyperideals in locally associative left almost semihypergroups. N. Y. J. Math. 2014, 20, 1063–1076. [Google Scholar]
- Gulistan, M.; Yaqoob, N.; Shahzad, M. A Note On Hv-LA-semigroup. UPB Sci. Bull. Ser. A 2015, 77, 93–106. [Google Scholar]
- Yaqoob, N.; Gulistan, M. Partially ordered left almost semihypergroups. J. Egypt. Math. Soc. 2015, 23, 231–235. [Google Scholar] [CrossRef]
- Rehman, I.; Yaqoob, N.; Nawaz, S. Hyperideals and hypersystems in LA-hyperrings. Songklanakarin J. Sci. Technol. 2017, 39, 651–657. [Google Scholar]
- Nawaz, S.; Rehman, I.; Gulistan, M. On left almost semihyperrings. Int. J. Anal. Appl. 2018, 16, 528–541. [Google Scholar]
- Yaqoob, N.; Cristea, I.; Gulistan, M.; Nawaz, S. Left almost polygroups. Ital. J. Pure Appl. Math. 2018, 39, 465–474. [Google Scholar]
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gulistan, M.; Nawaz, S.; Hassan, N. Neutrosophic Triplet Non-Associative Semihypergroups with Application. Symmetry 2018, 10, 613. https://doi.org/10.3390/sym10110613
Gulistan M, Nawaz S, Hassan N. Neutrosophic Triplet Non-Associative Semihypergroups with Application. Symmetry. 2018; 10(11):613. https://doi.org/10.3390/sym10110613
Chicago/Turabian StyleGulistan, Muhammad, Shah Nawaz, and Nasruddin Hassan. 2018. "Neutrosophic Triplet Non-Associative Semihypergroups with Application" Symmetry 10, no. 11: 613. https://doi.org/10.3390/sym10110613
APA StyleGulistan, M., Nawaz, S., & Hassan, N. (2018). Neutrosophic Triplet Non-Associative Semihypergroups with Application. Symmetry, 10(11), 613. https://doi.org/10.3390/sym10110613





