Tsallis, Rényi and Sharma-Mittal Holographic Dark Energy Models in Loop Quantum Cosmology
Abstract
:1. Introduction
2. Basic Equations
- 0 shows the decelerated phase of the universe.
- , corresponds to the dust dominated era.
- , leads to the radiation dominated era.
- , shows the accelerated phase of the universe.
2.1. Tsallis Holographic Dark Energy (HDE) Model
2.2. Rényi HDE Model
2.3. Sharma-Mittal HDE Model
3. Cosmological Parameters
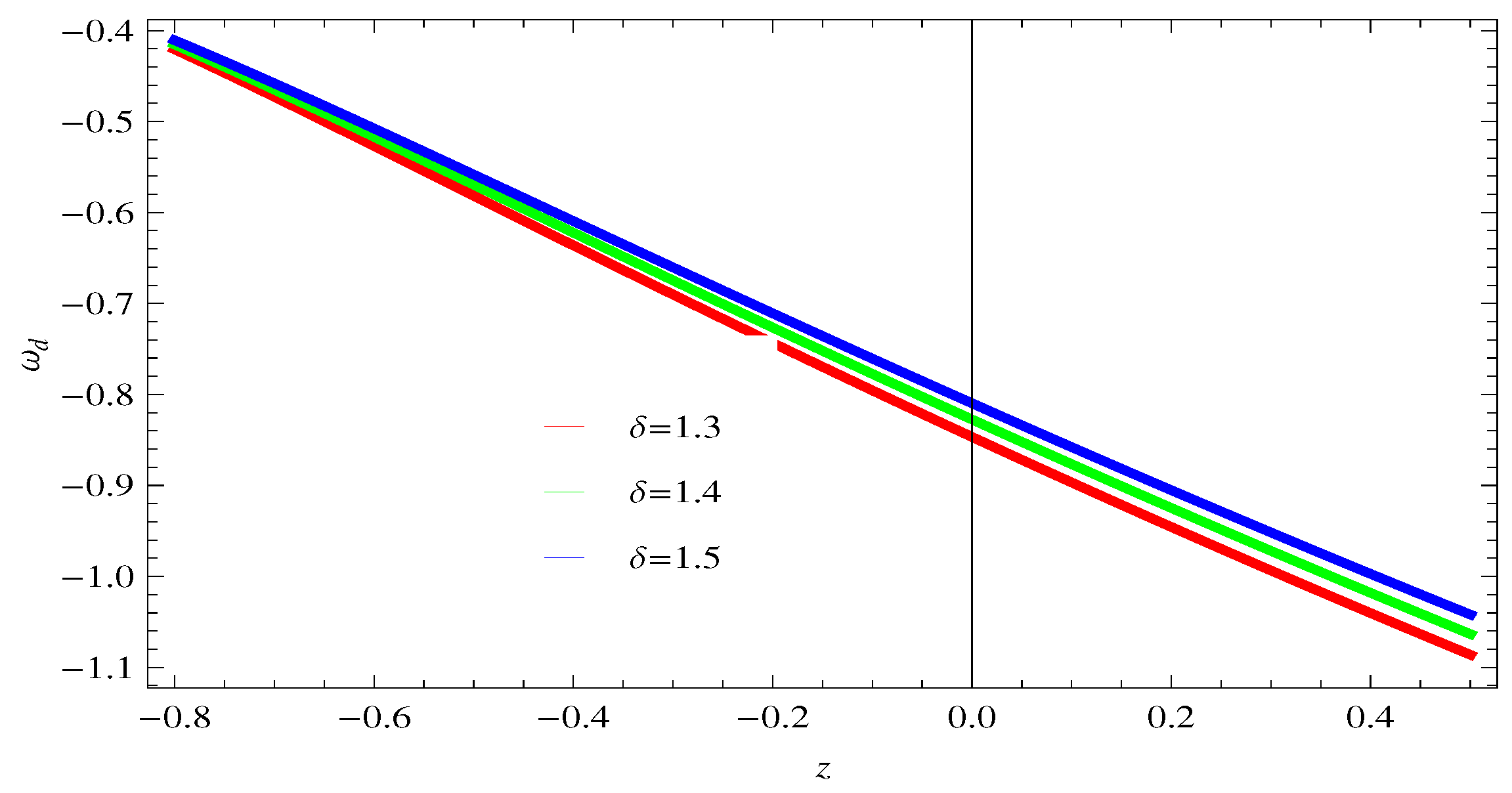
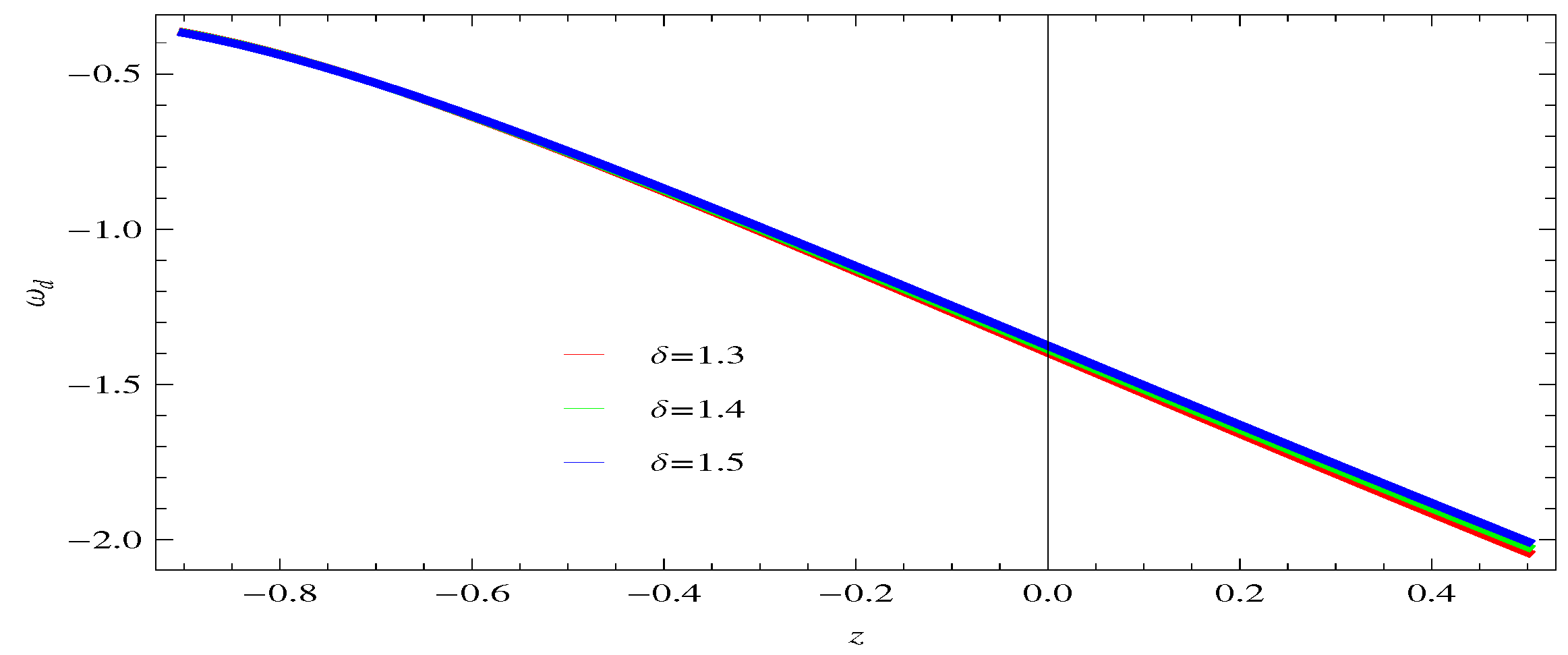
3.1. EoS Parameter
- corresponds to non-relativistic matter.
- quintessence.
- cosmological constant.
- phantom.
- In this case , evolve across the boundary of cosmological constant shows the quintom behavior.
3.1.1. For Tsallis HDE
3.1.2. For Rényi HDE
3.1.3. For Sharma-Mittal HDE
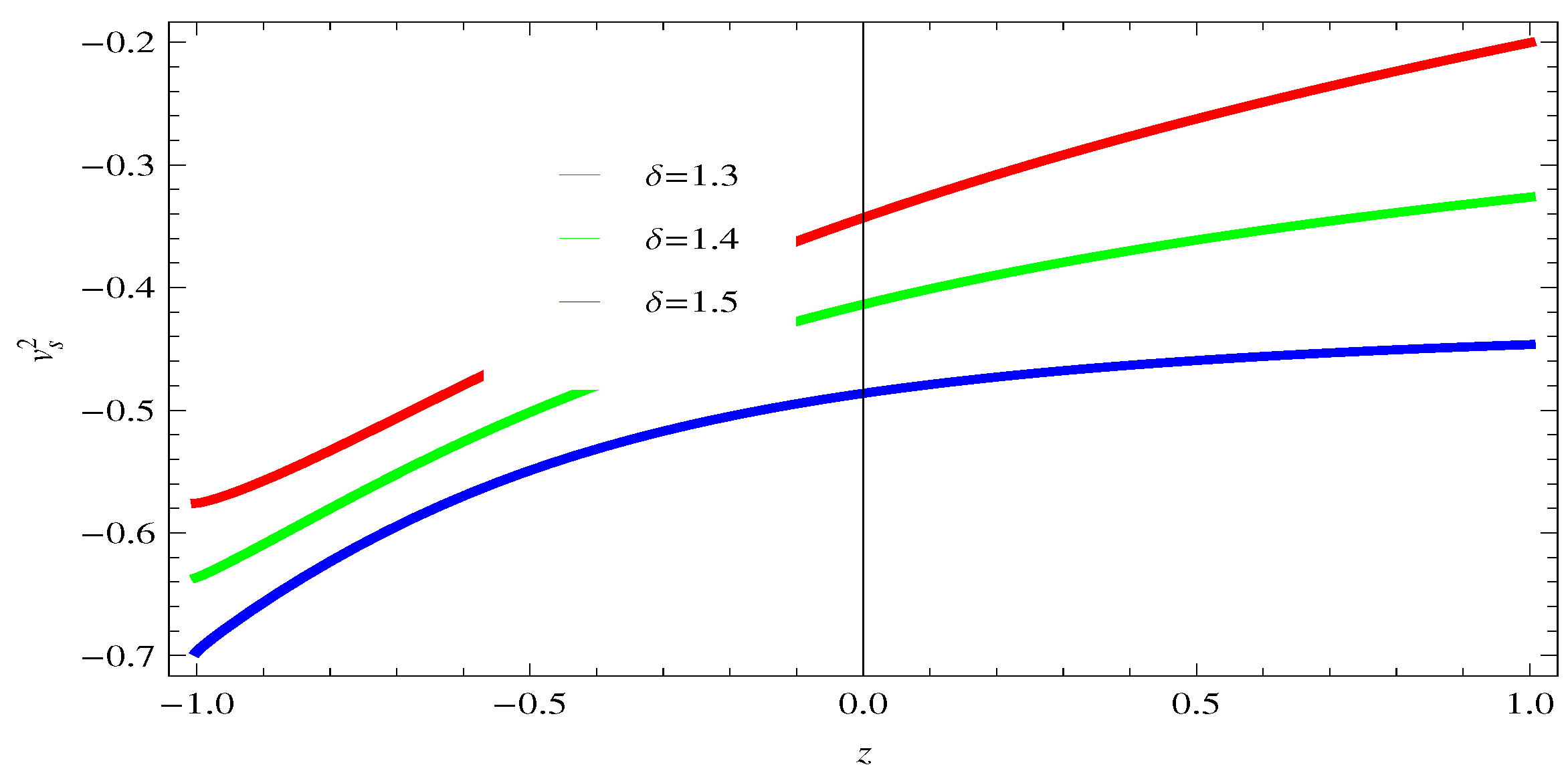
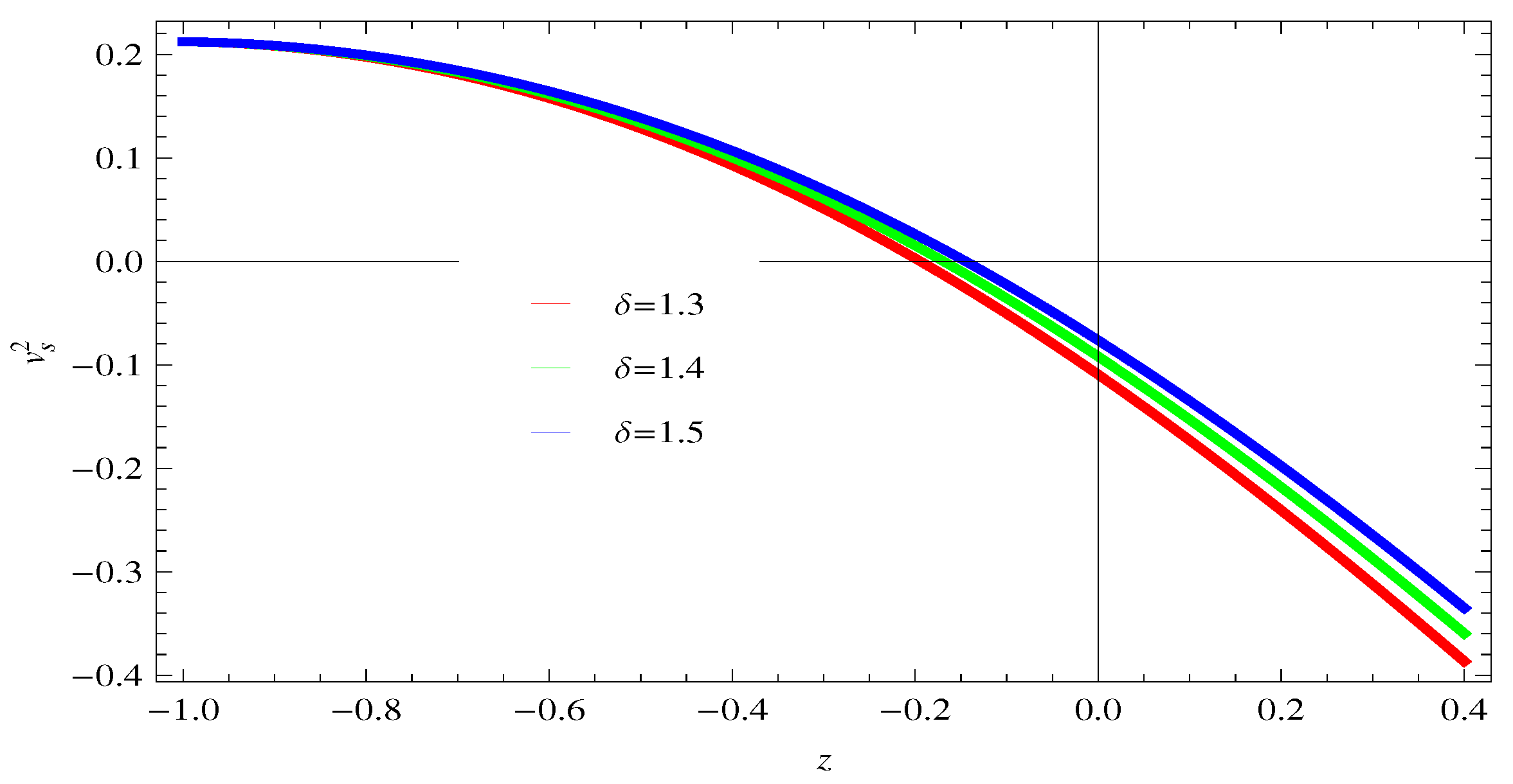
3.2. Stability Analysis
3.2.1. For Tsallis HDE
3.2.2. For Rényi HDE
3.2.3. For Sharma-Mittal HDE
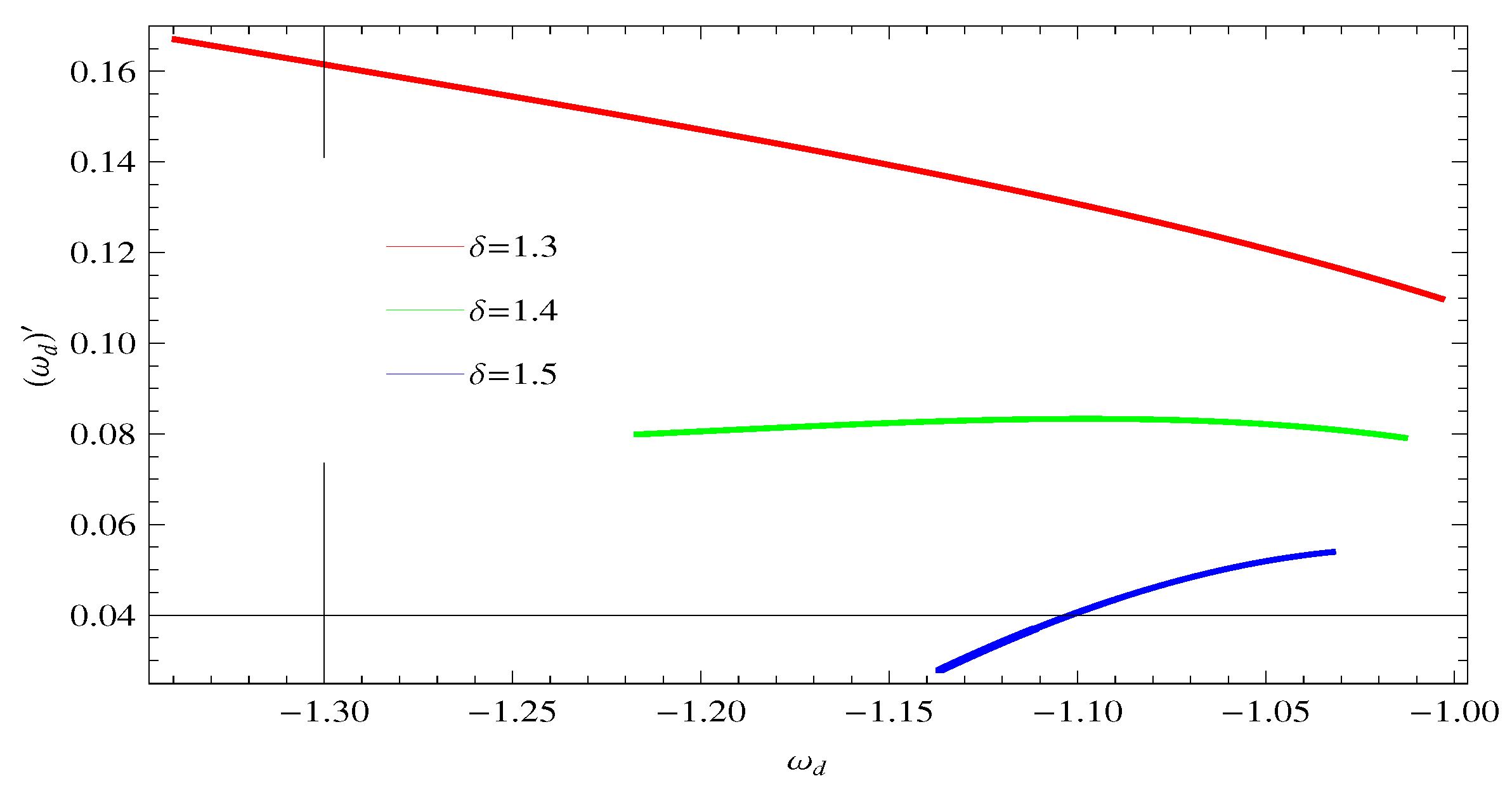
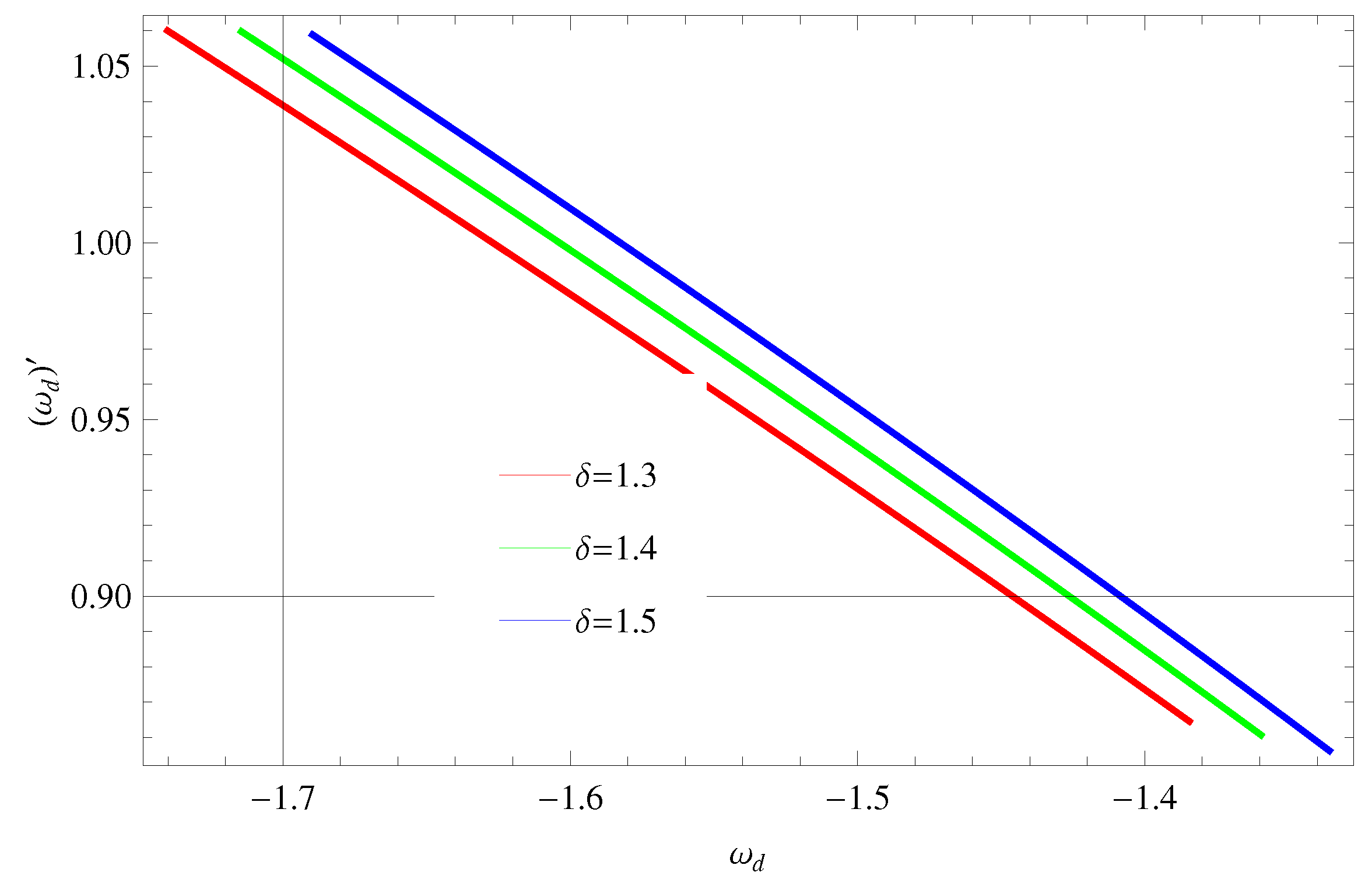
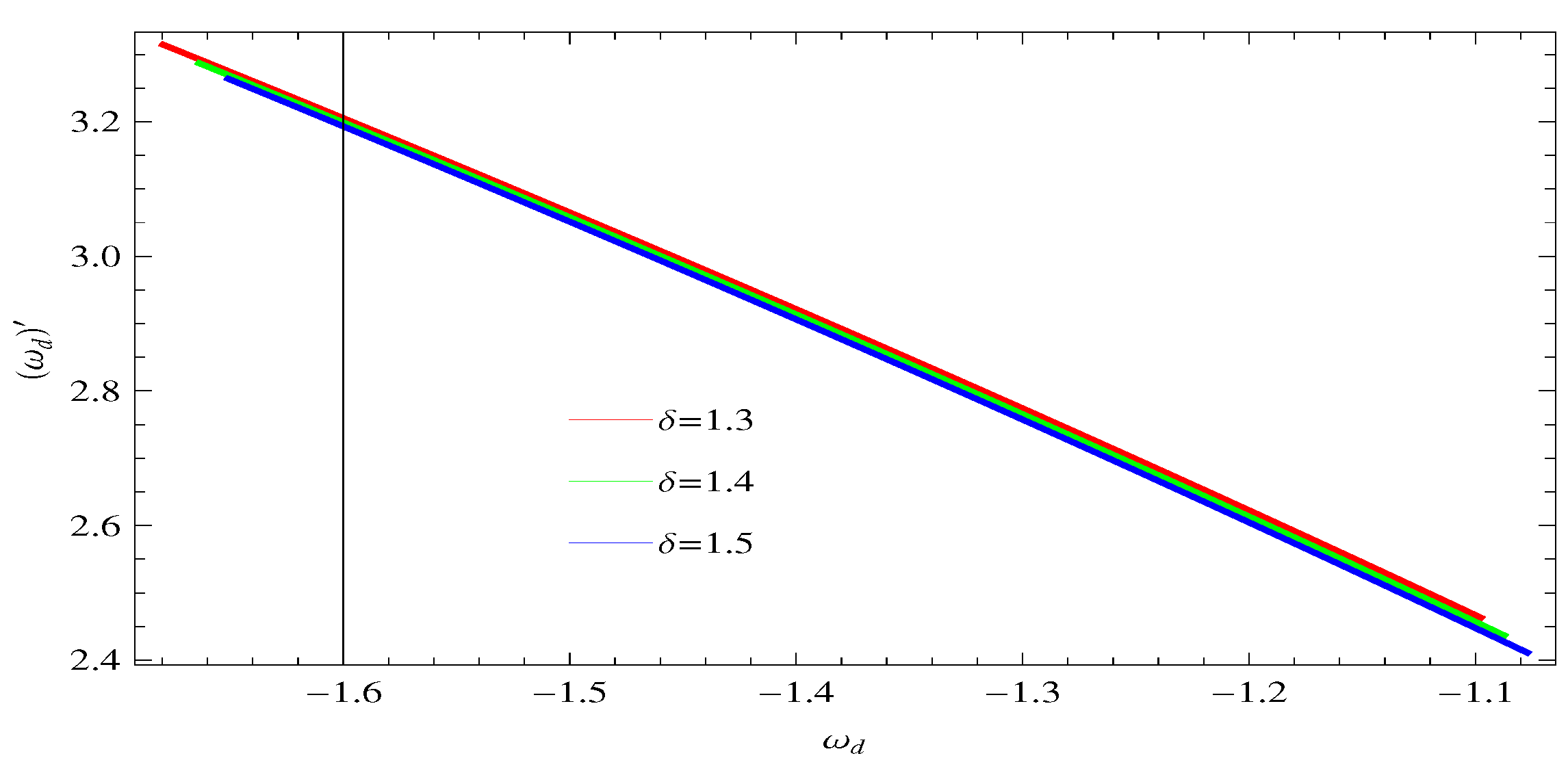
3.3. - Plane
3.3.1. For Tsallis HDE
3.3.2. For Rényi HDE
3.3.3. For Sharma-Mittal HDE
4. Concluding Remarks
- The trajectories of EoS parameter in all three models HDE exhibit the quintom-like nature of the universe as it shows transition of the universe from phantom era (at early and present) towards quintessence era (latter epoch) by evolving phantom barrier.
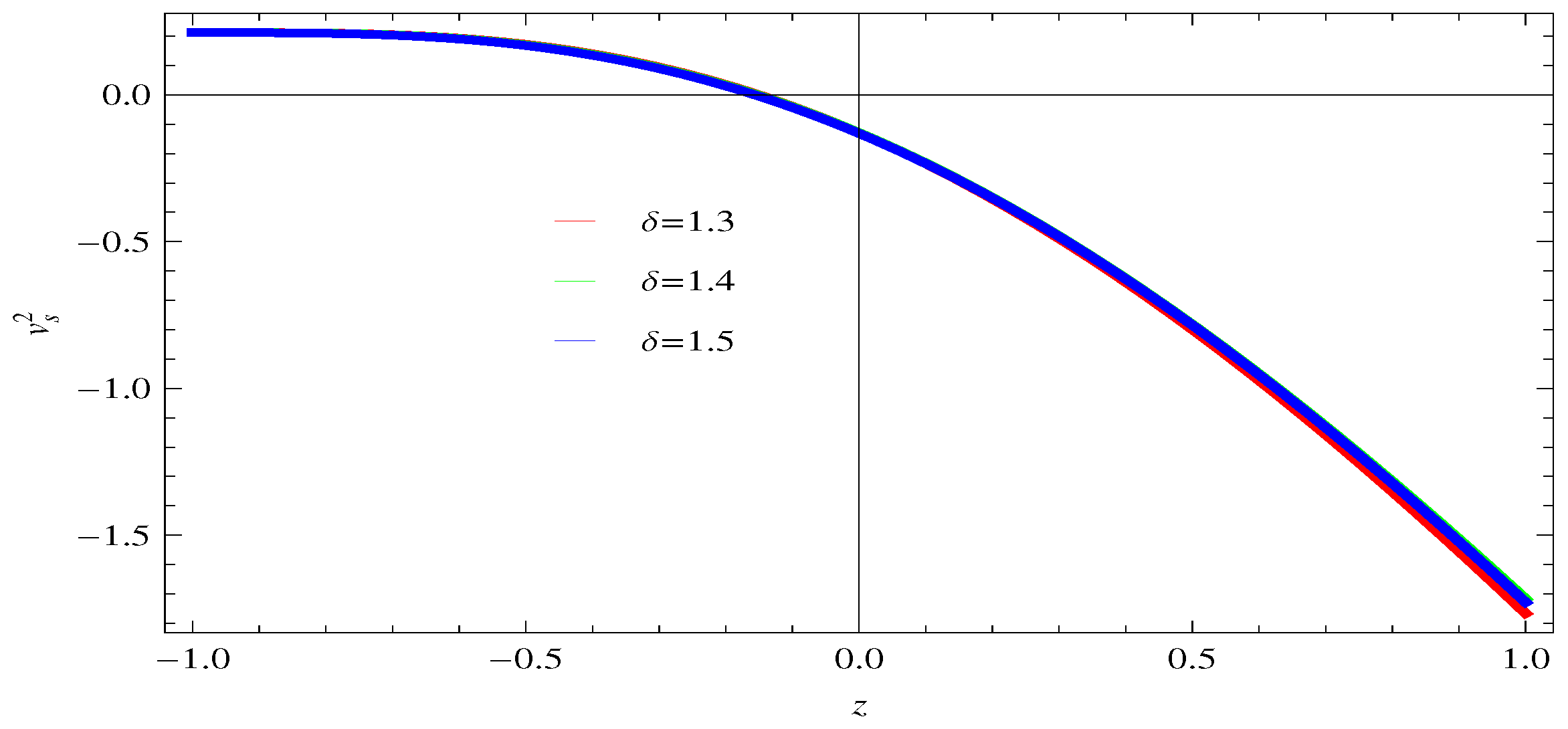
- To analyze the stability of the Tsallis HDE, Rényi HDE and Sharma-Mittal HDE models we check the graphical behavior of squared sound speed. For Tsallis HDE model, it is observed that for all values of z which leads to the instability of this model. On the other hand, for Rényi HDE, the squared speed of sound shows unstable behavior at the early and present epoch while leads to the stability at the latter epoch. The same behavior of the squared speed of sound has been observed in case of Sharma-Mittal HDE model.
- Also, corresponds to thawing region ( and ) for all three models of HDE.
Author Contributions
Funding
Conflicts of Interest
References
- Riess, A.G.; Filippenko, A.V.; Challis, P.; Clocchiattia, A.; Diercks, A.; Garnavich, P.M.; Gilliland, R.L.; Hogan, C.J.; Jha, S.; Kirshner, R.P.; et al. Observational evidence from supernovae for an accelerating Universe and a cosmological constant. Astron. J. 1998, 116, 1009–1038. [Google Scholar] [CrossRef]
- Perlmutter, S.; Aldering, G.; Goldhaber, G.; Knop, R.A.; Nugent, P.; Castro, P.G.; Deustua, S.; Fabbro, S.; Goobar, A.; Groom, D.E.; et al. Measurements of Omega and Lambda from 42 High-Redshift Supernovae. Astrophys. J. 1999, 517, 565–586. [Google Scholar] [CrossRef]
- Bernardis, P.; Ade, P.A.R.; Bock, J.J.; Bond, J.R.; Borrill, J.; Boscaleri, A.; Coble, K.; Crill, B.P.; DeGasperis, G.; Farese, P.C.; et al. A flat Universe from high-resolution maps of the cosmic microwave background radiation. Nature 2000, 404, 955–959. [Google Scholar] [CrossRef] [PubMed]
- Perlmutter, S.; Aldering, G.; Amanullah, R.; Astier, P.; Blanc, G.; Burns, M.S.; Conley, A.; Deustua, S.E.; Doi, M.; Ellis, R.; et al. New Constraints on and w from an Independent Set of 11 High-Redshift Supernovae Observed with the Hubble Space Telescope. Astrophys. J. 2003, 598, 102–137. [Google Scholar]
- Colless, M.; Dalton, G.; Maddox, S.; Sutherland, W.; Norberg, P.; Cole, S.; Hawthrone, J.B.; Bridges, T.; Cannon, R.; Collins, C.; et al. The 2dF Glaxy Redshift Survey: Spectra and redshift. Mon. Not. R. Astron. Soc. 2001, 328, 1039–1063. [Google Scholar] [CrossRef]
- Tegmark, M.; Strauss, M.A.; Blanton, M.R.; Abazajian, K.; Dodelson, S.; Sandvik, H.; Wang, X.; Weinberg, D.H.; Zehavi, I.; Bahcall, N.A.; et al. Cosmological parameters from SDSS and WMAP. Phys. Rev. D 2004, 69, 103501–103526. [Google Scholar] [CrossRef]
- Cole, S.; Percival, W.J.; Peacock, R.A.; Norberg, P.; Baugh, C.M.; Frenk, C.S.; Baldry, I.; Bland-Hawthorn, J.; Bridges, T.; Cannon, R.; et al. The 2dF Galaxy Redshift Survey: Power-spectrum analysis of the final dataset and cosmological implications. Mon. Not. R. Astron. Soc. 2005, 362, 505–534. [Google Scholar] [CrossRef] [Green Version]
- Springel, V.; Frenk, C.S.; White, S.M.D. The large-scale structure of the Universe. Nature 2006, 440, 1137–1144. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hanany, S.; Ade, P.; Balbi, A.; Bock, J.; Borrill, J.; Boscaleri, A.; Bernardis, P.; Ferreira, P.G.; Hristov, V.V.; Jaffe, A.H.; et al. MAXIMA-1: A Measurement of the Cosmic Microwave Background Anisotropy on angular scales of 10 arcminutes to 5 degrees. Astrophys. J. Lett. 2000, 545, L5–L9. [Google Scholar] [CrossRef]
- Netterfield, C.B.; Ade, P.A.R.; Bock, J.J.; Bond, J.R.; Borrill, J.; Boscaleri, A.; Coble, K.; Contaldi, C.R.; Crill, B.P.; Bernardis, P.; et al. A measurement by BOOMERANG of multiple peaks in the angular power spectrum of the cosmic microwave background. Astrophys. J. 2002, 571, 604–614. [Google Scholar] [CrossRef]
- Spergel, D.N.; Verde, L.; Peiris, H.V.; Komatsu, E.; Nolta, M.R.; Bennett, C.L.; Halpern, M.; Hinshaw, G.; Jarosik, N.; Kogut, A.; et al. First Year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Determination of Cosmological Parameters. Astrophys. J. Suppl. 2003, 148, 175–194. [Google Scholar] [CrossRef]
- Roos, M. Introduction to Cosmology; John Wiley and Sons: Chichester, UK, 2003. [Google Scholar]
- Nojiri, S.; Odintsov, S.D. The new form of the equation of state for dark energy fluid and accelerating universe. Phys. Lett. B 2006, 639, 144–150. [Google Scholar] [CrossRef]
- Bamba, K.; Capozziello, S.; Nojiri, S.; Odintsov, S.D. Dark energy cosmology: The equivalent description via different theoretical models and cosmography tests. Astrophys. Space Sci. 2012, 342, 155–228. [Google Scholar] [CrossRef]
- Zimdahl, W.; Pavon, D. Scaling Cosmology. Gen. Rel. Grav. 2003, 35, 413–422. [Google Scholar] [CrossRef] [Green Version]
- Del Campo, S.; Herrera, R.; Olivares, G.; Pavon, D. Interacting models of soft coincidence. Phys. Rev. D 2006, 74, 023501-9. [Google Scholar] [CrossRef]
- Sadjadi, H.M.; Alimohammadi, M. Cosmological coincidence problem in interacting dark energy models. Phys. Rev. D 2006, 74, 103007-7. [Google Scholar] [CrossRef]
- Setare, M.R. The holographic dark energy in non-flat Brans-Dicke cosmology. Phys. Lett. B 2007, 644, 99–103. [Google Scholar] [CrossRef]
- Malekjani, M.; Naderi, T.; Pace, F. Effects of ghost dark energy perturbations on the evolution of spherical overdensities. MNRAS B 2015, 453, 4148–4158. [Google Scholar] [CrossRef]
- Chiba, T.; Okabe, T.; Yamaguchi, M. Kinetically driven quintessence. Phys. Rev. D 2000, 62, 023511–023518. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. Quantum de Sitter cosmology and phantom matter. Phys. Lett. B 2003, 562, 147–152. [Google Scholar] [CrossRef] [Green Version]
- Pasquier, V.; Moschella, U.; Kamenshchick, A.Y.; Moschella, U.; Pasquier, V. An alternative to quintessence. Phys. Lett. B 2001, 511, 265–268. [Google Scholar] [Green Version]
- Kleidis, K.; Spyrou, N.K. Polytropic dark matter flows illuminate dark energy and accelerated expansion. Astron. Astrographys. 2015, 576, A23. [Google Scholar] [CrossRef]
- Kleidis, K.; Spyrou, N.K. Dark Energy: The Shadowy Reflection of Dark Matter. Entropy 2016, 18, 94. [Google Scholar] [CrossRef]
- Weinberg, S. The cosmological constant problem. Rev. Mod. Phys. 1998, 61, 1–20. [Google Scholar] [CrossRef]
- Copeland, E.J.; Sami, M.; Tsujikawa, S. Dynamics of dark energy. Int. J. Mod. Phys. D 2006, 15, 1753–1935. [Google Scholar] [CrossRef]
- Padmanabhan, T. Cosmological Constant—The Weight of the Vacuum. Phys. Rep. 2003, 380, 235–320. [Google Scholar] [CrossRef]
- Felice, A.D.; Tsujikawa, S. f(R) Theories. Living Rev. Relativ. 2010, 13, 3–163. [Google Scholar] [CrossRef] [PubMed]
- Sahni, V. The Cosmological Constant Problem and Quintessence. Class. Quant. Grav. 2002, 19, 3435–3448. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. Unified cosmic history in modified gravity: From F(R) theory to Lorentz non-invariant models. Phys. Rep. 2011, 505, 59–144. [Google Scholar] [CrossRef]
- Linder, E.V. Einstein’s other gravity and the acceleration of the Universe. Phys. Rev. D 2010, 81, 127301–127303. [Google Scholar] [CrossRef]
- Ferraro, R.; Fiorini, F. Non-trivial frames for f(T) theories of gravity and beyond. Phys. Lett. B 2011, 702, 75–80. [Google Scholar] [CrossRef]
- Sharif, M.; Rani, S. Wormhole solutions in f(T) gravity with noncommutative geometry. Phys. Rev. D 2013, 88, 123501–123510. [Google Scholar] [CrossRef]
- Brans, C.H.; Dicke, R.H. Mach’s Principle and a Relativistic Theory of Gravitation. Phys. Rev. D 1961, 124, 925–935. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. Modified Gauss-Bonnet theory as gravitational alternative for dark energy. Phys. Lett. B 2005, 631, 1–6. [Google Scholar] [CrossRef]
- Cognola, G.; Elizalde, E.; Nojiri, S.; Odintsov, S.D.; Zerbini, S. Dark energy in modified Gauss-Bonnet gravity: Late-time acceleration and the hierarchy problem. Phys. Rev. D 2006, 73, 084007–084022. [Google Scholar] [CrossRef]
- Harko, T.; Francisco, S.N.; Lobo, F.S.N.; Nojiri, S.; Odintsov, S.D. f(R,T) gravity. Phys. Rev. D 2011, 84, 024020–024031. [Google Scholar] [CrossRef]
- Capozziello, S.; De Laurentis, M. Extended Theories of Gravity. Phys. Rep. 2011, 509, 167–321. [Google Scholar] [CrossRef] [Green Version]
- Capozziello, S.; Faraoni, V. Beyond Einstein Gravity: A Survey of Gravitational Theories for Cosmology and Astrophysics, 1st ed.; Springer: Dordrecht, The Netherlands, 2010; Volume 170. [Google Scholar]
- Bamba, K.; Odintsov, S. Inflationary cosmology in modified gravity theories. Symmetry 2015, 7, 220–240. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D.; Oikonomou, V.K. Modified Gravity Theories on a Nutshell: Inflation, Bounce and Late-time Evolution. Phys. Rep. 2017, 692, 1–104. [Google Scholar] [CrossRef]
- Susskind, L. The World as a Hologram. J. Math. Phys. 1995, 36, 6377–6396. [Google Scholar] [CrossRef]
- Nojiri, S.; Odintsov, S.D. Unifying phantom inflation with late-time acceleration: Scalar phantom non-phantom transition model and generalized holographic dark energy. Gen. Rel. Grav. 2006, 38, 1285–1304. [Google Scholar] [CrossRef]
- Cohen, A.G.; Kaplan, D.B.; Nelson, A.E. Effective Field Theory, Black Holes, and the Cosmological Constant. Phys. Rev. Lett. 1999, 82, 4971–4974. [Google Scholar] [CrossRef] [Green Version]
- Li, M. A Model of Holographic Dark Energy. Phys. Lett. B 2004, 603, 1–5. [Google Scholar] [CrossRef]
- Sheykhi, A. Holographic scalar field models of dark energy. Phys. Rev. D 2011, 84, 107302–107306. [Google Scholar] [CrossRef]
- Hu, B.; Ling, Y. Interacting dark energy, holographic principle, and coincidence problem. Phys. Rev. D 2006, 73, 123510–123518. [Google Scholar] [CrossRef]
- Ma, Y.Z.; Gong, Y.; Chen, X. Features of holographic dark energy under combined cosmological constraints. Eur. Phys. J. C 2009, 60, 303–315. [Google Scholar] [CrossRef] [Green Version]
- Tsallis, C. Possible generalization of Boltzmann-Gibbs statistics. J. Stat. Phys. 1988, 52, 479–487. [Google Scholar] [CrossRef]
- Rényi, A. On measures of entropy and Information. In Proceedings of the 4th Berkely Symposium on Mathematics, Statistics and Probability; University California Press: Berkeley, CA, USA, 1961; Volume 1, pp. 547–561. [Google Scholar]
- Sharma, B.D.; Mittal, D.P. New nonadditive measures of entropy for discrete probability distributions. J. Math. Sci. 1975, 10, 28–40. [Google Scholar]
- Tavayef, M.; Sheykhi, A.; Bamba, K.; Moradpour, H. Tsallis holographic dark energy. Phys. Lett. B 2018, 781, 195–200. [Google Scholar] [CrossRef]
- Moradpour, H.; Moosavi, S.A.; Lobo, I.P.; Graca, J.P.M.; Jawad, A.; Salako, I.G. Thermodynamic approach to holographic dark energy and the Renyi entropy. Eur. Phys. J. C 2018, 829, 78–83. [Google Scholar] [CrossRef]
- Jahromi, A.S.; Moosavi, S.A.; Moradpour, H.; Grac, J.P.M.; Lobo, I.P.; Salako, I.G.; Jawad, A. Generalized entropy formalism and a new holographic dark energy model. Phys. Lett. B 2018, 780, 21–24. [Google Scholar] [CrossRef]
- Bojowald, M. Loop Quantum Cosmology. Living Rev. Relativ. 2008, 11, 4–134. [Google Scholar] [CrossRef] [PubMed]
- Rovelli, C. Loop Quantum Gravity. Living Rev. Relativ. 2008, 11, 5–73. [Google Scholar] [CrossRef] [PubMed]
- Oliveros, A. Slow-roll inflation from massive vector fields non-minimally coupled to gravity. Astrphys. Space Sci. 2017, 362, 1–6. [Google Scholar] [CrossRef]
- Jamil, M.; Debnath, U. Interacting modified Chaplygin gas in loop quantum cosmology. Astrophys. Space Sci. 2011, 333, 3–8. [Google Scholar] [CrossRef]
- Chakraborty, S.; Debnath, U.; Ranjit, C. Observational constants of modified Chaplygin gas in loop quantum cosmology. Eur. Phys. J. C 2012, 72, 2101–2108. [Google Scholar] [CrossRef]
- Ashtekar, A. Albert Einstein Century International conference. AIP Conf. Proc. 2006, 861, 3–14. [Google Scholar]
- Ashtekar, A.; Pawlowski, T.; Singh, P. Quantum Nature of the Big Bang: Improved dynamics. Phys. Rev. D 2006, 74, 084003–084042. [Google Scholar] [CrossRef]
- Singh, P. Loop cosmological dynamics and dualities with Randall-Sundrum braneworlds. Phys. Rev. D 2006, 73, 063508–063516. [Google Scholar] [CrossRef]
- Sami, M.; Singh, P.; Tsujikawa, S. Avoidance of future singularities in loop quantum cosmology. Phys. Rev. D 2006, 74, 043514–043519. [Google Scholar] [CrossRef]
- Zimdahl, W.; Pavon, D.; Chimento, L.P. Interacting Quintessence. Phys. Lett. B 2001, 521, 133–138. [Google Scholar] [CrossRef]
- Li, M.; Li, X.; Wang, S.; Wang, Y. Dark Energy. Commun. Theor. Phys. 2011, 56, 525–604. [Google Scholar] [CrossRef] [Green Version]
- Arevalo, F.; Bacalhau, A.P.R.; Zimdahl, W. Cosmological dynamics with non-linear interactions. Class. Quant. Grav. 2012, 29, 235001–235023. [Google Scholar] [CrossRef]
- Sharif, M.; Zubair, M. Energy Conditions Constraints and Stability of Power Law Solutions in f(R,T) Gravity. J. Phys. Soc. Jpn. 2013, 82, 014002–014010. [Google Scholar] [CrossRef]
- Sharif, M.; Zubair, M. Cosmological reconstruction and stability in f(R,T) gravity. Gen. Relativ. Gravit. 2014, 46, 1723–1752. [Google Scholar] [CrossRef]
- Tsallis, C.; Citro, L.J.L. Black hole thermodynamical entropy. Eur. Phys. J. C 2013, 73, 2487–2493. [Google Scholar] [CrossRef]
- Caldwell, R.R.; Linder, E.V. Limits of Quintessence. Phys. Rev. Lett. 2005, 95, 141301–141304. [Google Scholar] [CrossRef] [PubMed]

© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jawad, A.; Bamba, K.; Younas, M.; Qummer, S.; Rani, S. Tsallis, Rényi and Sharma-Mittal Holographic Dark Energy Models in Loop Quantum Cosmology. Symmetry 2018, 10, 635. https://doi.org/10.3390/sym10110635
Jawad A, Bamba K, Younas M, Qummer S, Rani S. Tsallis, Rényi and Sharma-Mittal Holographic Dark Energy Models in Loop Quantum Cosmology. Symmetry. 2018; 10(11):635. https://doi.org/10.3390/sym10110635
Chicago/Turabian StyleJawad, Abdul, Kazuharu Bamba, Muhammad Younas, Saba Qummer, and Shamaila Rani. 2018. "Tsallis, Rényi and Sharma-Mittal Holographic Dark Energy Models in Loop Quantum Cosmology" Symmetry 10, no. 11: 635. https://doi.org/10.3390/sym10110635
APA StyleJawad, A., Bamba, K., Younas, M., Qummer, S., & Rani, S. (2018). Tsallis, Rényi and Sharma-Mittal Holographic Dark Energy Models in Loop Quantum Cosmology. Symmetry, 10(11), 635. https://doi.org/10.3390/sym10110635





