Precanonical Structure of the Schrödinger Wave Functional of a Quantum Scalar Field in Curved Space-Time

Abstract

The functional Schrödinger representation of a nonlinear scalar quantum field theory in curved space-time is shown to emerge as a singular limit from the formulation based on precanonical quantization. The previously established relationship between the functional Schrödinger representation and precanonical quantization is extended to arbitrary curved space-times. In the limiting case when the inverse of the ultraviolet parameter $\varkappa$ introduced by precanonical quantization is mapped to the infinitesimal invariant spatial volume element, the canonical functional derivative Schrödinger equation is derived from the manifestly covariant partial derivative precanonical Schrödinger equation. The Schrödinger wave functional is expressed as the trace of the multidimensional spatial product integral of Clifford-algebra-valued precanonical wave function or the product integral of a scalar function obtained from the precanonical wave function by a sequence of transformations. In non-static space-times, the transformations include a nonlocal transformation given by the time-ordered exponential of the zero-th component of spin-connection.

1. Introduction

Quantum field theory in curved space-time [1,2,3,4,5] is often considered as an opportunity to study an interplay between gravitation, space-time and quantum theory in order to gain insights and intuitions into the quantum geometry of space-time and gravitation. The study of various formulations of quantum fields on curved backgrounds should allow us to identify the concepts and mathematical structures of quantum field theory which are important beyond the simplifying framework of the Poincaré-invariant Minkowski spacetime.

An approach to quantization of fields called precanonical quantization [6,7,8,9] is based on mathematical structures of the De Donder-Weyl (DW) Hamiltonian theory known in the calculus of variations [10,11,12,13]. In this theory, a space-time decomposition is not required and all space-time variables are treated on equal footing. In this sense the DW Hamiltonian formulation is an intermediate description of classical fields between the Lagrangian and the canonical Hamiltonian level (hence the name “precanonical”). By treating all space-time variables on equal footing as a multidimensional analogue of the evolution parameter in mechanics this formulation allows us to avoid the necessity of treating fields as infinite-dimensional Hamiltonian systems at least on the level of formulating an appropriate Hamilton-like form of field dynamics, its quantization, and then formulating the corresponding quantum theory of fields. This way, the approach may help to circumvent technical difficulties the usual canonical quantization of fields brings in.

In the canonical Hamiltonian field theory, it is the infinite-dimensional symplectic structure on the phase space which yields the Poisson bracket of observables represented by functionals which underlies the canonical quantization. There are many geometrical structures in classical field theory which have been put forward as analogues of the symplectic structure in the DW Hamiltonian formalism: multisymplectic [14,15,16,17,18,19], n-plectic [20,21], polysymplectic [22,23,24,25], k-plectic [26] and others [27,28,29,30]. The book [31] gives a good introductory comparison of some of the relevant geometries underlying classical field theory. We have proposed a different polysymplectic structure [9,32,33,34,35] represented by a certain equivalence of class of forms, which naturally emerges from the geometrical structures of the calculus of variations (the Poincaré-Cartan form) and leads to a definition of the analogue of Poisson brackets suitable for geometric quantization and a generalization of canonical quantization. There are also several different proposals of a generalization of Poisson brackets to the DW Hamiltonian formalism [23,24,25,27,30,36,37,38] (often referred to, somewhat misleadingly, as the “covariant Hamiltonina formalism”, which is the term already reserved in theoretical physics for the covariant version of the canonical Hamiltonian formalism) and more general multisymplectic geometries [39,40,41,42,43,44] whose study has been inspired by the multi/poly-symplectic approaches in classical field theory. Note that some of those “Poisson brackets” often are not actually Poisson in any generalized sense as they do not possess either an analogue of the Leibniz property or a suitable generalization of the Jacobi property. Both of those properties of Poisson brackets are, however, crucial for quantization relevant for physics. That is why, this is the bracket operation on differential forms, which is derived from the polysymplectic structure and leads to the Poisson–Gerstenhaber algebra [9,34,35] as a generalization of Poisson algebra, which has led us to a generalization of the canonical quantization [6,7,8] and geometrical quantization [9,45] in the context of the DW Hamiltonian formulation of fields. Further discussion of this bracket or its different treatments and generalizations can be found in [37,46,47,48,49]. A very similar bracket was put forward recently also in [50,51,52] using a reasoning different from ours and that of [46,47]. Besides, the polysymplectic structure and the Poisson–Gerstenhaber brackets which underlie precanonical quantization have been used not so long ago in the considerations of vielbein gravity [53], classical BRST symmetry [54], topologically massive YM field [55], MacDowell–Mansouri gravity [56] and BF gravity [57].

As a truly Hamiltonian theory, the DW Hamiltonian formulation also leads to a generalization of the Hamilton–Jacobi (HJ) theory [11,12,13] (see also [58,59,60] for a relevant recent geometric treatment). The corresponding DW-HJ equation is a partial derivative equation rather than a functional derivative equation one derives within the canonical Hamiltonian formalism. This leads to the question: which formulation of the quantum theory of fields reproduces the DW-HJ equation in the classical limit? Although the question is not yet clarified in general case, in [7] it is shown that the DW-HJ equation emerges in the classical limit from our scheme of precanonical quantization of scalar field theory.

Several approaches to quantization of field theories based on, or motivated by the DW Hamiltonian formulation have been attempted in the literature using geometric quantization [24,25,61], canonical-like quantization [62,63,64,65], path-integral quantization [66] and deformation quantization [67,68]. Some of the proposals [62,63] bear resemblance to the results of precanonical quantization in [6,7,8], although they lack the foundation of the proper generalization of Poisson brackets to the DW Hamiltonian formulation [34,35] and their quantization according to the Dirac rule. Unfortunately, none of those proposals has been developed so far to the extent that a comparison with the results of precanonical quantization or standard quantum field theory would be possible.

One can also find a claim in [69,70] that their “manifestly covariant approach” to quantization of gravity is “consistent with” the DW Hamiltonian formulation of gravity on the classical level. However, the analogues of Hamiltonian, Hamilton–Jacobi and Schrödinger equations for gravity proposed in [69,70] are essentially using a field theoretic generalization of the treatment of hydrodynamics in the Lagrangian variables and the proper time parameter of the Lagrangian paths in order to accomplish the desired manifest covariance, and this is very different from what the DW formulation suggests, where, instead of introducing a proper time as an additional element (parameter) of the theory, one treats all space-time variables on equal footing as a multidimensional analogue of the time parameter in mechanics. It still remains to be seen how the approach of [69,70] can be applied to simpler and better understood field-theoretic systems than the Einstein gravity and if the results of such application are compatible with the standard QFT. However, there is also a similarity between [69], the precanonical quantization approach of this paper, and the precanonical quantum gravity [71,72,73,74,75,76,77,78,79]: in both approaches, the fundamental Hamiltonian, Hamilton–Jacobi and Schrödinger equations are manifestly covariant partial differential equations, and the wave functions live on a finite-dimensional space of field components. One may expect, therefore, that the methods of the present paper establishing a connection between the precanonical quantization and the functional Schrödinger representation in QFT could be helpful also for the study of possible relations between the ideas of [69] and the canonical quantum gravity in metric variables [80]. Moreover, the recent progress [81] in understanding the relation between the DW Hamilton–Jacobi equation for gravity [82] and the timeless canonical HJ equation for general relativity derived by Peres [83] may be helpful for understanding the relations between the approach of [69,70] and/or the precanonical quantum gravity of [71,72,73,74,75,76,77,78,79] with the current approaches to quantum gravity based on canonical quantization [80,84,85].

Unlike the proposals in [24,25,61,62,63,64,65,66], the precanonical quantization is based on a firm foundation of a proper generalization of the symplectic structure and the Poisson algebra to the DW Hamiltonian formulation [34,35] and its quantization according to the Dirac rule [6,7,8], a generalization of the Dirac rule in the context of curved space-time [86] and quantum gravity [71,72,76], and geometric prequantization [9,45]. We found that quantization of a small Heisenberg-like subalgebra of the aforementioned Poisson–Gerstenhaber algebra leads to a hypercomplex generalization of the formalism of quantum theory where both operators and wave functions are Clifford-algebra-valued. The precanonical analogue of the Schrödinger equation is formulated using the Dirac operator on the space-time which appears as a multidimensional generalization of the time derivative in the left side of the standard Schrödinger equation [6,7,8,9,45].

It should be noted that our hypercomplex generalization of quantum theory derived from precanonical quantization is different from other proposals of such a generalization in the literature [87,88,89,90,91,92,93,94]. In those generalizations, typically, the time dimension still retains its distinguished status, and the modifications concern only the mathematical nature of the Hilbert space assumed to be different from the Hilbert space of complex-valued functions. Moreover, unlike those approaches, within the approach of precanonical quantization the hypercomplex wave functions appear as a consequence of quantization itself. An experiment proposed in [95] will hopefully establish the limits of validity of all of those proposals, including ours.

One of the features of precanonical formulation of quantized fields is that it allows us to reproduce the classical field equations in DW Hamiltonian form as the equations for expectation values of operators defined by precanonical quantization and evolving according to the precanonical Schrödinger equation [71,86]. By treating the space-time variables on equal footing it also leads to a formulation of quantum theory of fields on a finite-dimensional space of field and space-time variables thus providing a new framework for the quantum gauge theory [96,97,98] and the theory of quantum gravity [72,76] which looks more promising than the canonical quantization both conceptually and from the point of view of a possibility of a rigorous mathematical treatment.

In order to apply the potential of precanonical quantization it is important to understand how it can be related to more familiar and already working concepts of standard QFT. In this paper, we extend our previous results about the relationship between precanonical quantization and the functional Schrödinger picture in QFT [96,98,99,100,101,102] to nonlinear scalar field theory in arbitrary curved space-times.

Below, we proceed as follows: in Section 2, we first recall the results of canonical quantization in the functional Schrödinger representation and the precanonical quantization of scalar field theory in curved space-time, and then we discuss drastic differences between them. Section 2 also serves to introduce the notations used throughout the paper. The connection between the functional Schrödinger representation and the results of precanonical quantization in curved space-time is established in Section 3 which consists of several subsections reflecting the multi-step nature of the argumentation. Namely, we first outline a general idea which allows us to anticipate a connection between the Schrödinger wave functional and precanonical wave function based on the respective probabilistic interpretation of both objects. Second, in Section 3.1, we present a restriction of precanonical Schrödinger equation to the section of the bundle of field coordinates over space-time, which represents a field configuration $\varphi \left(\mathbf{x}\right)$ that the Schrödinger wave functional is a functional of. The restriction of precanonical Schrödinger equation is formulated in terms of the total covariant derivative introduced in Section 3.1.1. It allows us, in Section 3.2, to write the equation for the time evolution of the wave functional composed from the precanonical wave function restricted to a field configuration $\varphi \left(\mathbf{x}\right)$. To proceed with the derivation of the Schödinger equation for the wave functional from the restriction of the precanonical Schrödinger equation to a field configuration, in Section 3.3, we evaluate the functional derivatives of the functional composed from precanonical wave function with respect to the field configurations $\varphi \left(\mathbf{x}\right)$. Further, in Section 3, we analyse different terms in the equation presented in Section 3.2 and show how they can be either expressed in terms of the functional derivatives of the composed functional or cancelled in a certain limiting case. The result of Section 3 is the derivation of the functional derivative Schrödinger equation from the restricted precanonical Schrödinger equation up to an additional term which involves the commutator of the zero-th component of the spin connection matrix with the precanonical wave function, see Equation (39). In Section 4, we consider static space-times with the vanishing zero-th component of the spin connection and obtain the expression of the Schrödinger wave functional as the trace of the continual product or the product integral of precanonical wave functions restricted to a field configuration. A more general case of non-static space-times with non-vanishing zero-th component of the spin connection is considered in Section 5 where we show that the extraneous term in (39), which contains the commutator of the zero-th component of spin connection with precanonical wave function, disappears if the wave functional is expressed in terms of transformed precanonical wave functions with the transformation given by the time-ordered exponential of the zero-th component of spin connection. This observation allows us to extend the results from static space-times to nonstatic ones. In Section 6, we present our conclusions and highlight the main steps of the derivation of the functional Schrödinger equation from precanonical Schödinger equation and the expression of the Schrödinger wave functional as a product integral of precanonical wave functions or their transforms defined in Section 4 and Section 5. We also discuss the physical meaning of the ultra-violet parameter $\varkappa$ whose infinite value corresponds to the limiting case in which it is shown to be possible to derive from precanonical quantization the standard functional Schrödinger representation of QFT.

2. Quantum Scalar Field on a Curved Space-Time: The Canonical and Precanonical Descriptions

Let us recall that the conventional canonical quantization of scalar field theory in curved space-time can be formulated in the functional Schrödinger representation of QFT [103,104]. It leads to the description of the corresponding quantum field in terms of the Schrödinger wave functional $\mathbf{\Psi }\left(\right[\varphi \left(\mathbf{x}\right)],t)$ satisfying the Schrödinger equation [105,106,107,108,109,110,111]

$$\mathrm{i}ħ{\partial }_t\mathbf{\Psi }=\int d\mathbf{x}\sqrt{g}\left(\frac{{ħ}^2}{2}\frac{g_{00}}{g}\frac{{\delta }^2}{\delta \varphi {\left(\mathbf{x}\right)}^2}-\frac{1}{2}g{}^{ij}{\partial }_i\varphi \left(\mathbf{x}\right){\partial }_j\varphi \left(\mathbf{x}\right)+V\left(\varphi \right)\right)\mathbf{\Psi },$$

(1)

where the right hand side is the canonical Hamiltonian operator formulated in terms of functional derivative operators, which acts on the wave functional Ψ, $x^{\mu }=(t,\mathbf{x})=(t,x^i)$ are space-time coordinates, $g_{\mu \nu }$ is the space-time metric tensor whose components depend on $x^{\mu }$, $g=|det(g_{\mu \nu }\left)\right|$. In this equation, one uses the space-time coordinates adapted to the space-like foliation such as the induced metric on the space-like leaves of the foliation is $g_{ij}$, the lapse $N=\sqrt{g_{00}}$ and the shift functions $N_i=g_{0i}=0$.

The precanonical quantization of a scalar field $\varphi \left(x\right)$ on a curved space-time background given by the metric tensor $g_{\mu \nu }\left(x\right)$ (cf. [71,86]) gives rise to the description in terms of a wave function $\Psi (\varphi ,x^{\mu })$ on the finite-dimensional bundle with the local coordinates $(\varphi ,x^{\mu })$ which takes values in the complexified space-time Clifford algebra, i.e., in n-dimensional space-time,

$$\Psi =\psi +{\psi }_{\mu }{\gamma }^{\mu }+\frac{1}{2!}{\psi }_{{\mu }_1{\mu }_2}{\gamma }^{{\mu }_1{\mu }_2}+\dots +\frac{1}{n!}{\psi }_{{\mu }_1\dots {\mu }_n}{\gamma }^{{\mu }_1\dots {\mu }_n}.$$

The precanonical wave function $\Psi$ satisfies the partial derivative precanonical Schrödinger equation (pSE)

$$\mathrm{i}ħ{\gamma }^{\mu }\left(x\right){\nabla }_{\mu }\Psi =\left(-\frac{1}{2}{ħ}^2\varkappa \frac{{\partial }^2}{\partial {\varphi }^2}+\frac{1}{\varkappa }V\left(\varphi \right)\right)\Psi =:\frac{1}{\varkappa }\widehat{H}\Psi ,$$

(2)

where ${\gamma }^{\mu }\left(x\right)$ are the curved space-time Dirac matrices such that

$${\gamma }^{\mu }\left(x\right){\gamma }^{\nu }\left(x\right)+{\gamma }^{\nu }\left(x\right){\gamma }^{\mu }\left(x\right)=2g^{\mu \nu }\left(x\right),$$

(3)

${\gamma }^{{\mu }_1\dots {\mu }_p}$ are the antisymmetrized products of p Dirac matrices,

$${\nabla }_{\mu }:={\partial }_{\mu }+{\omega }_{\mu }\left(x\right)$$

(4)

is the covariant derivative with the spin-connection matrices ${\omega }_{\mu }\left(x\right)=\frac{1}{4}{\omega }_{\mu }{}_{AB}\left(x\right)\underline{\gamma }{}^{AB}$ (see, e.g., [112]) acting on Clifford-algebra-valued wave functions by the commutator product [102], and $\underline{\gamma }{}^A$ are the constant Dirac matrices which factorize the Minkowski metric ${\eta }^{AB}$ of the tangent space

$$\underline{\gamma }{}^A\underline{\gamma }{}^B+\underline{\gamma }{}^B\underline{\gamma }{}^A=2{\eta }^{AB}.$$

(5)

Throughout the paper the metric signature is $+---\dots$ and we mostly follow the notation and conventions used in [71,86,100,101]. In particular, the plane capital Greek letters like $\Psi$ and $\Phi$ denote wave functions on a finite dimensional space of $\varphi$ and $x^{\mu }$ and the boldface capital Greek letters like $\mathbf{\Psi }$ and $\mathbf{\Phi }$ denote functionals of field configurations $\varphi \left(\mathbf{x}\right)$. From now on we also set $ħ=1$.

The operator $\widehat{H}$ in (2) is the De Donder-Weyl (DW) Hamiltonian operator constructed according to the procedure of precanonical quantization [7,8,71,86]. In the expression of $\widehat{H}$ there appears an ultraviolet parameter $\varkappa$ of the dimension of the inverse spatial volume. This parameter typically appears in the representations of precanonical quantum operators [6,7,8,86]. For the scalar fields on curved background the DW Hamiltonian operator $\widehat{H}$ coincides with its counterpart in flat space-time (cf. [6,7,8,86]). Correspondingly, the curved space-time manifests itself only through the curved space-time Dirac matrices (3) and the spin-connection in the left-hand side of (2).

As we have seen, the description of quantum fields obtained from precanonical quantization is very different from a familiar description of quantum fields derived from the canonical quantization. In particular, while in the description using the functional Schrödinger picture the role of space variables $\mathbf{x}$ is different from the role the time variable t, the precanonical description is entirely space-time symmetric, manifestly covariant and independent of the assumption of global hyperbolicity of space-time. One can also wonder how the description in terms of precanonical wave function on a finite-dimensional space and the corresponding partial derivative precanonical Schrödinger equation can match the description in terms of functionals on an infinite-dimensional space of field configurations at a fixed time and the corresponding functional derivative Schrödinger equation, or how the multiparticle states and multi-point correlation functions of standard QFT could be related to the natural objects within the precanonical description such as the Green function of the precanonical Schrödinger equation (2).

However, one can reduce the perceived gap between those two descriptions by noticing that already on the classical level the solutions of field equations can be equally well treated using both the language of partial derivative equation on a finite dimensional space (in the Lagrangian, DW Hamiltonian and DW–HJ descriptions) and the language of functional derivative equations (in the canonical Hamiltonian and Hamilton–Jacobi description). Moreover, one can derive the canonical Hamiltonian and HJ equations from the DW Hamiltonian and DWHJ equations, respectively (see, e.g., [99,101]). In the next section, we will show how those relationships between the canonical and precanonical are extended to the quantum level in curved space-times.

3. Relating the Precanonical Wave Function and the Schrödinger Wave Functional

Our preceding work has already established a relationship between the functional Schrödinger representation and precanonical quantization of scalar and Yang–Mills fields in flat space-time [98,100,101]. The familiar QFT in functional Schrödinger representation was derived from the precanonical quantization as the limiting case when the combination $\underline{\gamma }{}_0\varkappa$ is replaced by $\delta \left(\mathbf{0}\right)$, a regularized value of Dirac delta-function $\delta (\mathbf{x}-{\mathbf{x}}^{\prime })$ at coinciding spatial points, which can be understood as the cutoff of the momentum space volume introduced by a regularization. Here we intend to extend this relationship to curved space-time using the example of a quantum scalar field.

The Schrödinger wave functional $\mathbf{\Psi }\left(\right[\varphi \left(\mathbf{x}\right)],t)$ is interpreted as the probability amplitude of finding a field configuration $\varphi \left(\mathbf{x}\right)$ at some moment of time t. The precanonical wave function $\Psi (\varphi ,x)$ is the probability amplitude of observing the field value $\varphi$ at the space-time point x. Then the time-dependent complex functional probability amplitude $\mathbf{\Psi }\left(\right[\varphi \left(\mathbf{x}\right)],t)$ can be expected to be a composition of space-time dependent Clifford-algebra-valued probability amplitudes given by the precanonical wave function $\Psi (\varphi ,x)$. It means that the Schrödinger wave functional $\mathbf{\Psi }\left(\right[\varphi \left(\mathbf{x}\right)],t)$ is a functional of precanonical wave functions $\Psi (\varphi ,x)$ restricted to a specific field configuration which is represented by a section $\Sigma$ in the total space of the bundle with the local coordinates $(\varphi ,x)$, which is defined by the equation $\Sigma :\varphi =\varphi \left(\mathbf{x}\right)$ at time t. Thus by denoting the restriction of precanonical wave function $\Psi (\varphi ,x)$ to $\Sigma$ as

$${\Psi }_{\Sigma }(\mathbf{x},t):=\Psi (\varphi =\varphi \left(\mathbf{x}\right),\mathbf{x},t)$$

we assume that

$$\mathbf{\Psi }(\left[\varphi \left(\mathbf{x}\right)\right],t)=\mathbf{\Psi }\left([{\Psi }_{\Sigma }(\mathbf{x},t),\varphi \left(\mathbf{x}\right)]\right),$$

(6)

so that the time dependence of the wave functional $\mathbf{\Psi }$ is totally controlled by the time dependence of precanonical wave function restricted to $\Sigma$. Then the chain rule differentiation yields the time derivative of $\mathbf{\Psi }$

$$\mathrm{i}{\partial }_t\mathbf{\Psi }=\mathrm{Tr}\int d\mathbf{x}\left\{\frac{\delta \mathbf{\Psi }}{\delta {\Psi }_{\Sigma }^T(\mathbf{x},t)}\mathrm{i}{\partial }_t{\Psi }_{\Sigma }(\mathbf{x},t)\right\},$$

(7)

where ${\Psi }^T$ denotes the transpose of the matrix $\Psi$. In the following we will be avoiding unnecessarily cumbersome notation by denoting ${\Psi }_{\Sigma }(\mathbf{x},t)$ also as ${\Psi }_{\Sigma }\left(\mathbf{x}\right)$ or even ${\Psi }_{\Sigma }$.

3.1. The Restriction of Precanonical Schrödinger Equation to $\Sigma$

The time derivative of ${\Psi }_{\Sigma }$ is determined by the restriction of pSE (2) rewritten in space+time split form to $\Sigma$:

$$\mathrm{i}{\partial }_t{\Psi }_{\Sigma }=-\mathrm{i}{\gamma }_0{\gamma }^i\left(\frac{d}{d{x}^i}-{\partial }_i\varphi \left(\mathbf{x}\right)\frac{\partial }{\partial \varphi }\right){\Psi }_{\Sigma }-\mathrm{i}{\gamma }_0{\gamma }^i[{\omega }_i,{\Psi }_{\Sigma }]-\mathrm{i}[{\omega }_0,{\Psi }_{\Sigma }]+\frac{{\gamma }_0}{\varkappa }{\widehat{H}}_{\Sigma }{\Psi }_{\Sigma },$$

(8)

where $\frac{d}{d{x}^i}$ is the total derivative along $\Sigma$,

$$\frac{d}{d{x}^i}:={\partial }_i+{\partial }_i\varphi \left(\mathbf{x}\right)\frac{\partial }{\partial \varphi }+{\partial }_i{\varphi }_{,k}\left(\mathbf{x}\right)\frac{\partial }{\partial {\varphi }_{,k}}+\dots .$$

(9)

In (9) ${\varphi }_{,k}$ denote the fiber coordinates of the first-jet bundle of the bundle of field variables $\varphi$ over space-time (cf. [113,114]) and ${\widehat{H}}_{\Sigma }$ in (8) is the restriction of the DW Hamiltonian operator $\widehat{H}$ to $\Sigma$. Since $\widehat{H}$ contains no space-time (horizontal) derivatives, ${\widehat{H}}_{\Sigma }=\widehat{H}$ and

$$\frac{1}{\varkappa }\widehat{H}=-\frac{\varkappa }{2}\frac{{\partial }^2}{\partial {\varphi }^2}+\frac{1}{\varkappa }V\left(\varphi \right).$$

(10)

3.1.1. The Total Covariant Derivative

Let us introduce the notion of the total covariant derivative acting on Clifford-algebra-valued tensors, particularly on those restricted to $\Sigma$. The derivative will be called “total” in the sense that (i) when acting on a Clifford-valued tensor function $T_{{\nu }_1{\nu }_2\dots }^{{\mu }_1{\mu }_2\dots }$ it includes both the spin-connection matrix ${\omega }_{\mu }{}^{}$ and the Christoffel symbols ${\Gamma }_{\beta \gamma }^{\alpha }$ (c.f. [115]) and (ii) when a tensor quantity with the components depending both on x and $\varphi$ is restricted to $\Sigma$, its derivative with respect to x-s is understood in the sense of the total derivative (9):

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill {\nabla }_{\alpha }^{\mathrm{tot}}{T}_{{\nu }_1{\nu }_2\dots }^{{\mu }_1{\mu }_2\dots }:=& \frac{d}{d{x}^{\alpha }}{T}_{{\nu }_1{\nu }_2\dots }^{{\mu }_1{\mu }_2\dots }+[{\omega }_{\alpha },T_{{\nu }_1{\nu }_2\dots }^{{\mu }_1{\mu }_2\dots }]\hfill \\ & +{\Gamma }_{\alpha \beta }^{{\mu }_1}{T}_{{\nu }_1{\nu }_2\dots }^{\beta {\mu }_2\dots }+{\Gamma }_{\alpha \beta }^{{\mu }_2}{T}_{{\nu }_1{\nu }_2\dots }^{{\mu }_1\beta \dots }+\dots .\hfill \\ & -{\Gamma }_{\alpha {\nu }_1}^{\beta }{T}_{\beta {\nu }_2\dots }^{{\mu }_1{\mu }_2\dots }-{\Gamma }_{\alpha {\nu }_2}^{\beta }{T}_{{\nu }_1\beta \dots }^{{\mu }_1{\mu }_2\dots }-\dots \hfill \end{array}\end{array}$$

(11)

The commutator in the second term guarantees that the total covariant derivative of the Clifford product of two Clifford-valued tensor quantities fulfils the Leibniz rule. The Christoffel symbols appear in the covariant derivative of non-scalar Clifford quantities, e.g., in the condition of metric compatibility

$${\nabla }_{\alpha }^{\mathrm{tot}}{\gamma }^{\mu }=0,$$

(12)

where only the first partial derivative term in (9) is non-vanishing when acting on x-dependent $\gamma$-matrices.

Now, in terms of the total covariant derivative ${\nabla }^{tot}$ acting on ${\Psi }_{\Sigma }$, Equation (8) takes the form

$$\mathrm{i}{\partial }_t{\Psi }_{\Sigma }=-\mathrm{i}{\gamma }_0{\gamma }^i{\nabla }_i^{\mathrm{tot}}{\Psi }_{\Sigma }-\mathrm{i}[{\omega }_0,{\Psi }_{\Sigma }]+\mathrm{i}{\gamma }_0{\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right){\partial }_{\varphi }{\Psi }_{\Sigma }+\frac{1}{\varkappa }{\gamma }_0{\widehat{H}}_{\Sigma }{\Psi }_{\Sigma }.$$

(13)

3.2. The Time Evolution of the Schrödinger Wave Functional from pSE

From (7), (8) and (10) the equation of the time evolution of the wave functional (6) constructed from precanonical wave functions takes the form

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill i{\partial }_t\mathbf{\Psi }=& \mathrm{Tr}\int \mathrm{d}\mathbf{x}\left\{\frac{\delta \mathbf{\Psi }}{\delta {\Psi }_{\Sigma }^T(\mathbf{x},t)}\right[\underset{I}{\underbrace{-\mathrm{i}{\gamma }_0{\gamma }^i\frac{d}{d{x}^i}{\Psi }_{\Sigma }\left(\mathbf{x}\right)}}+\underset{II}{\underbrace{\mathrm{i}{\gamma }_0{\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right){\partial }_{\varphi }{\Psi }_{\Sigma }\left(\mathbf{x}\right)\phantom{\frac{C}{D}}}}\hfill \\ & \hfill \underset{IIIa}{\underbrace{\phantom{(}-\frac{\mathrm{i}}{4}{\gamma }_0{\gamma }^i[{\omega }_i,{\Psi }_{\Sigma }\left(\mathbf{x}\right)]\phantom{\frac{A}{B}}}}\underset{IIIb}{\underbrace{\phantom{(}-\frac{\mathrm{i}}{4}[{\omega }_0,{\Psi }_{\Sigma }]}}\underset{IV}{\underbrace{-\frac{\varkappa }{2}{\gamma }_0{\partial }_{\varphi \varphi }{\Psi }_{\Sigma }\left(\mathbf{x}\right)}}+\underset{V}{\underbrace{\frac{1}{\varkappa }{\gamma }_{0}V\left(\varphi \left(\mathbf{x}\right)\right){\Psi }_{\Sigma }\left(\mathbf{x}\right)}}\left]\right\}.\end{array}\end{array}$$

(14)

In order to derive from this equation the functional derivative Schrödinger equation (1), we need to try to express the terms in the right hand side of (14) in terms of the functional derivatives of the composite functional $\mathbf{\Psi }$ in (6) with respect to $\varphi \left(\mathbf{x}\right)$. Those are calculated in the following section.

3.3. The Functional Derivatives of  $\mathbf{\Psi }$

By using the chain rule of the functional differentiation and introducing the notations

$${\mathbf{\Phi }}^{}\left(\mathbf{x}\right):=\frac{\delta \mathbf{\Psi }}{\delta {\Psi }_{\Sigma }^T\left(\mathbf{x}\right)}$$

(15)

and

$${\partial }_{\varphi }{\Psi }_{\Sigma }\left(\mathbf{x}\right):=(\partial \Psi /\partial \varphi ){{|}_{\Sigma }\left(\mathbf{x}\right),{\partial }_{\varphi \varphi }{\Psi }_{\Sigma }\left(\mathbf{x}\right):=({\partial }^2\Psi /\partial {\varphi }^2)|}_{\Sigma }\left(\mathbf{x}\right),$$

(16)

we obtain

$$\begin{array}{c}\hfill \frac{\delta \mathbf{\Psi }}{\delta \varphi \left(\mathbf{x}\right)}=\mathrm{Tr}\left\{{\mathbf{\Phi }}^{}\left(\mathbf{x}\right){\partial }_{\varphi }{\Psi }_{\Sigma }\left(\mathbf{x}\right)\right\}+\frac{\overline{\delta }\mathbf{\Psi }}{\overline{\delta }\varphi \left(\mathbf{x}\right)},\end{array}$$

(17)

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill \frac{{\delta }^2\mathbf{\Psi }}{\delta \varphi {\left(\mathbf{x}\right)}^2}=& \mathrm{Tr}\left\{\delta \left(\mathbf{0}\right){\mathbf{\Phi }}^{}\left(\mathbf{x}\right){\partial }_{\varphi \varphi }{\Psi }_{\Sigma }\left(\mathbf{x}\right)+2\frac{\overline{\delta }\mathbf{\Phi }\left(\mathbf{x}\right)}{\overline{\delta }\varphi \left(\mathbf{x}\right)}{\partial }_{\varphi }{\Psi }_{\Sigma }\left(\mathbf{x}\right)\right\}\hfill \\ \hfill & +\mathrm{Tr}\mathrm{Tr}\left\{\frac{{\delta }^2\mathbf{\Psi }}{\delta {\Psi }_{\Sigma }^T\left(\mathbf{x}\right)\otimes \delta {\Psi }_{\Sigma }^T\left(\mathbf{x}\right)}{\partial }_{\varphi }{\Psi }_{\Sigma }\left(\mathbf{x}\right)\otimes {\partial }_{\varphi }{\Psi }_{\Sigma }\left(\mathbf{x}\right)\right\}+\frac{{\overline{\delta }}^2\mathbf{\Psi }}{\overline{\delta }\varphi {\left(\mathbf{x}\right)}^2}.\hfill \end{array}\end{array}$$

(18)

where $\overline{\delta }$ denotes the partial functional derivative with respect to $\varphi \left(\mathbf{x}\right)$, as opposite to the total functional derivative $\delta$, and $\delta \left(\mathbf{0}\right)$ is a regularized value of $\delta {\Psi }_{\Sigma }\left(\mathbf{x}\right)/\delta {\Psi }_{\Sigma }^T\left({\mathbf{x}}^{\prime }\right)$ at $\mathbf{x}={\mathbf{x}}^{\prime }$, which can be defined using a point splitting or lattice regularization to make sense of $(n-1)$-dimensional delta-function $\delta (\mathbf{x}-{\mathbf{x}}^{\prime })$ at coinciding points. This is the simplest regularization one may use to make sense of the second functional derivative at coinciding points which appears in the functional derivative Schrödinger equation (1).

3.4. The Correspondence between Terms I–V in Equation (14) and the Canonical Hamiltonian Operator in (1)

3.4.1. The Potential Term V

Our starting observation will be that the term V with $V\left(\varphi \right)$ in (14) has to reproduce the last term in the functional derivative Schrödinger equation (1). This means that there exists a mapping ↦ such that

$$\int \mathrm{d}\mathbf{x}\mathrm{Tr}\left\{\mathbf{\Phi }\left(\mathbf{x}\right)\frac{1}{\varkappa }{\gamma }_{0}V\left(\varphi \left(\mathbf{x}\right)\right){\Psi }_{\Sigma }\left(\mathbf{x}\right))\right\}\mapsto \int \mathrm{d}\mathbf{x}\sqrt{g}V\left(\varphi \left(\mathbf{x}\right)\right)\mathbf{\Psi }.$$

(19)

The existence of the map in (19) implies that the following relation should be fulfilled at any spatial point $\mathbf{x}$:

$$\mathrm{Tr}\left\{\mathbf{\Phi }\left(\mathbf{x}\right)\frac{1}{\varkappa }{\gamma }_0{\Psi }_{\Sigma }\left(\mathbf{x}\right)\right\}\mapsto \sqrt{g}\mathbf{\Psi }.$$

(20)

Then the functional differentiation of both sides of (20) with respect to ${\Psi }_{\Sigma }^T\left(\mathbf{x}\right)$ yields

$$\mathrm{Tr}\left\{\frac{{\delta }^2\mathbf{\Psi }}{\delta {\Psi }_{\Sigma }^T\left(\mathbf{x}\right)\otimes \delta {\Psi }_{\Sigma }^T\left(\mathbf{x}\right)}\frac{1}{\varkappa }{\gamma }_0{\Psi }_{\Sigma }\left(\mathbf{x}\right)\right\}+\mathbf{\Phi }\left(\mathbf{x}\right)\frac{1}{\varkappa }{\gamma }_0\delta \left(\mathbf{0}\right)\mapsto \sqrt{g}\mathbf{\Phi }\left(\mathbf{x}\right),$$

(21)

where again, $\delta \left(\mathbf{0}\right)=\delta {\Psi }_{\Sigma }\left(\mathbf{x}\right)/\delta {\Psi }_{\Sigma }^T\left(\mathbf{x}\right).$ This type of relation is possible if the following two conditions are fulfilled:

$$\frac{{\delta }^2\mathbf{\Psi }}{\delta {\Psi }_{\Sigma }^T\left(\mathbf{x}\right)\otimes \delta {\Psi }_{\Sigma }^T\left(\mathbf{x}\right)}\mapsto 0$$

(22)

and

$$\frac{1}{\varkappa }{\gamma }_0\left(x\right)\delta \left(\mathbf{0}\right)-\sqrt{g}\left(x\right)\mapsto 0.$$

(23)

The latter can be understood as the condition

$${\gamma }^0\sqrt{g}\varkappa \mapsto \delta \left(\mathbf{0}\right).$$

(24)

By taking into account that $\sqrt{g}=\sqrt{g_{00}h}$, where $h:=|det(g_{ij}\left)\right|$, and ${\gamma }^0\sqrt{g_{00}}=\underline{\gamma }{}_0$ is the time-like tangent Minkowski space Dirac matrix (usually denoted as $\beta$), the condition (24) can be rewritten as

$$\underline{\gamma }{}_0\varkappa \mapsto \delta \left(\mathbf{0}\right)/\sqrt{h}={\delta }^{\mathrm{inv}}\left(\mathbf{0}\right),$$

(25)

where ${\delta }^{\mathrm{inv}}\left(\mathbf{x}\right)$ is the invariant $(n-1)$-dimensional delta-function defined by the property

$$\int d\mathbf{x}\sqrt{h}{\delta }^{\mathrm{inv}}\left(\mathbf{x}\right)=1.$$

(26)

This formula generalizes to curved space-times the limiting map $\underline{\gamma }{}_0\varkappa \mapsto \delta \left(\mathbf{0}\right)$ already found in flat space-time [100,101], with the $(n-1)$-dimensional delta-function replaced by the invariant one. Moreover, the definition (26) may be viewed as a statement that ${\delta }^{\mathrm{inv}}\left(\mathbf{0}\right)$ is the inverse of the invariant volume element $\sqrt{h}d\mathbf{x}$. This allows us to interpret (25) as

$$\frac{1}{\varkappa }\mapsto \underline{\gamma }{}_0\sqrt{h}d\mathbf{x}.$$

(27)

We will use this interpretation below in Section 4 and Section 5 when writing the expressions of the wave functional in terms of precanonical wave function using the product integral.

3.4.2. The Second Functional Derivative Term

Our next observation is that, in the limiting case (24), the term $IV$ in (14) is able to reproduce the first term in the right-hand side of (18)

$$IV:-\frac{\varkappa }{2}{\gamma }_0{\partial }_{\varphi \varphi }{\Psi }_{\Sigma }\mapsto -\frac{1}{\sqrt{g}}{g}_{00}\delta \left(\mathbf{0}\right){\partial }_{\varphi \varphi }{\Psi }_{\Sigma }.$$

(28)

A comparison with (18) shows that the term $IV$ in (14) leads to the following expression in functional derivatives of $\mathbf{\Psi }$:

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill IV:\mathrm{Tr}\left\{\frac{1}{2}\mathbf{\Phi }\left(\mathbf{x}\right)\varkappa {\gamma }_0{\partial }_{\varphi \varphi }{\Psi }_{\Sigma }\left(\mathbf{x}\right)\right\}\mapsto \frac{1}{2}\frac{g_{00}}{\sqrt{g}}\left(\frac{{\delta }^2\mathbf{\Psi }}{\delta \varphi {\left(\mathbf{x}\right)}^2}-2\mathrm{Tr}\left\{\frac{\overline{\delta }\mathbf{\Phi }\left(\mathbf{x}\right)}{\overline{\delta }\varphi \left(\mathbf{x}\right)}{\partial }_{\varphi }{\Psi }_{\Sigma }\left(\mathbf{x}\right)\right\}-\frac{{\overline{\delta }}^2\mathbf{\Psi }}{\overline{\delta }\varphi {\left(\mathbf{x}\right)}^2}\right)& .\hfill \end{array}\end{array}$$

(29)

The first term in the right-hand side of (29) correctly reproduces the first term in the functional derivative Schrödinger equation (1). However, the second and the third term need further consideration.

3.4.3. The Non-Ultralocality Term and the Wave Functional $\mathbf{\Psi }$ in Terms of Precanonical ${\Psi }_{\Sigma }$

Since the right hand side of (14) is expected to lead to a functional derivative operator acting on the wave functional $\mathbf{\Psi }$, as in the right hand side of the functional derivative Schrödinger equation (1), the second term in (29) with ${\partial }_{\varphi }{\Psi }_{\Sigma }$ has to be cancelled by the term $II$ in (14) which also contains ${\partial }_{\varphi }{\Psi }_{\Sigma }$. Therefore, it is required that

$$II+2\mathrm{nd}\mathrm{term}\mathrm{of}\left(29\right):\mathrm{i}{\mathbf{\Phi }}^{}\left(\mathbf{x}\right){\gamma }_0{\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right)+\frac{g_{00}}{\sqrt{g}}\frac{\overline{\delta }\mathbf{\Phi }\left(\mathbf{x}\right)}{\overline{\delta }\varphi \left(\mathbf{x}\right)}\mapsto 0,$$

(30)

where the sign ↦ stresses the fact that it is sufficient that the left hand side vanishes under the condition (24) rather than as an equality. In fact, by functionally differentiating both sides of (30) with respect to $\varphi \left({\mathbf{x}}^{\prime }\right)$ we can see that (30) with ↦ replaced by the equality sign is not an integrable equation in functional derivatives. Nevertheless, by bearing in mind that (30) has to be valid only under the condition (24), the solution $\mathbf{\Phi }\left(\mathbf{x}\right)$ can be written in the form

$${\mathbf{\Phi }}^{}\left(\mathbf{x}\right)=\Xi (\left[{\Psi }_{\Sigma }\right];\check{\mathbf{x}})e^{-\mathrm{i}\varphi \left(\mathbf{x}\right){\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right)/\varkappa },$$

(31)

where the “integration constant” $\Xi (\left[{\Psi }_{\Sigma }\right];\check{\mathbf{x}})$ is a functional of ${\Psi }_{\Sigma }\left({\mathbf{x}}^{\prime }\right)$ on the punctured space with the removed point $\mathbf{x}$ such that ${\mathbf{x}}^{\prime }\ne \mathbf{x}$. By construction, this functional satisfies the identity

$$\frac{\overline{\delta }\Xi (\left[{\Psi }_{\Sigma }\right];\check{\mathbf{x}})}{\overline{\delta }\varphi \left(\mathbf{x}\right)}\equiv 0.$$

Indeed, by differentiating (31) with respect to $\varphi \left(\mathbf{x}\right)$, replacing $\varkappa$ according to the limiting map (24), and taking into account that ${\gamma }_0\left(x\right){\gamma }_0\left(x\right)=:g_{00}\left(x\right)$ and ${\partial }_i\delta \left(\mathbf{0}\right)=0$ (that restricts the admissible class of regularizations of delta-function $\delta (\mathbf{x}$)), we conclude that (31) solves (30) under the condition (24). Note also that the functional (31) by construction fulfils

$$\frac{\delta \mathbf{\Phi }\left(\mathbf{x}\right)}{\delta {\Psi }_{\Sigma }^T\left(\mathbf{x}\right)}=\frac{{\delta }^2\mathbf{\Psi }}{\delta {\Psi }_{\Sigma }^T\left(\mathbf{x}\right)\otimes \delta {\Psi }_{\Sigma }^T\left(\mathbf{x}\right)}\equiv 0,$$

(32)

which is consistent with the condition (22). Thus the required cancellation of the terms with ${\partial }_{\varphi }{\Psi }_{\Sigma }\left(\mathbf{x}\right)$ (under the condition (24)) fixes the form of the functional $\mathbf{\Phi }\left(\mathbf{x}\right)$ introduced in (15). This allows us to express the wave functional $\mathbf{\Psi }$ in the form

$$\mathbf{\Psi }\sim \mathrm{Tr}{\left\{\Xi (\left[{\Psi }_{\Sigma }\right];\check{\mathbf{x}})e^{-\mathrm{i}\varphi \left(\mathbf{x}\right){\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right)/\varkappa }\frac{{\gamma }_0}{\sqrt{g}\varkappa }{\Psi }_{\Sigma }\left(\mathbf{x}\right)\right\}}_{|\varkappa \stackrel{}{⟼}{\gamma }_0\delta \left(\mathbf{0}\right)/\sqrt{g}},$$

(33)

which is valid at any point $\mathbf{x}$. Here the equality up to a normalization factor which will depend on $\varkappa$ and $\sqrt{g}$ is denoted as ∼. The notation ${\{\dots \}}_{|\varkappa ⟼{\gamma }_0\delta \left(\mathbf{0}\right)/\sqrt{g}}$ indicates that every appearance of $\varkappa$ in the expression inside braces is replaced by ${\gamma }_0\delta \left(\mathbf{0}\right)/\sqrt{g}$ as prescribed by the limiting map (24).

Using (33) we can now evaluate the last term in (29) in the limit (24):

$$\text{3-rd}\mathrm{term}\mathrm{of}\left(29\right):\frac{1}{2}\frac{g_{00}}{\sqrt{g}}\frac{{\overline{\delta }}^2\mathbf{\Psi }}{\overline{\delta }\varphi {\left(\mathbf{x}\right)}^2}\mapsto -\frac{1}{2}\sqrt{g}{g}^{ij}{\partial }_i\varphi \left(\mathbf{x}\right){\partial }_j\varphi \left(\mathbf{x}\right)\mathbf{\Psi }.$$

(34)

The right hand side of (34) correctly reproduces the second term in the functional derivative Schrödinger equation (1), thus correctly accounting for the inherent non-ultralocality of relativistic quantum scalar field theory (cf. [116,117]) in curved space-time.

Thus, all terms in the functional derivative Schrödinger equation (1) are now derived from pSE restricted to $\Sigma$, Equation (8). However, there are still unaccounted for terms I, $IIIa$ and $IIIb$ in (14)

$$I+IIIa+IIIb:-\mathrm{i}\int \mathrm{d}\mathbf{x}\mathrm{Tr}\left\{\mathbf{\Phi }\left(\mathbf{x}\right){\gamma }_0{\gamma }^i{\nabla }_i^{tot}{\Psi }_{\Sigma }+\mathbf{\Phi }\left(\mathbf{x}\right)[{\omega }_0,{\Psi }_{\Sigma }]\right\}.$$

(35)

Let us recall that in flat space-time [98,100,101], those terms are reduced to the term I with the total derivative $d{\Psi }_{\Sigma }\left(\mathbf{x}\right)/d{x}^i$ which does not contribute to the equation for the functional $\mathbf{\Psi }$ if ${\Psi }_{\Sigma }\left(\mathbf{x}\right)$ vanishes at the spatial infinity. Let us see now if this property extends to curved space-times.

3.4.4. The Vanishing Contribution from the Terms I and $IIIa$

At first we consider the first term in (35). Using the covariant Stokes theorem we obtain

$$\begin{array}{c}I+IIIa:-\mathrm{i}\int \mathrm{d}\mathbf{x}\mathrm{Tr}\left\{\mathbf{\Phi }\left(\mathbf{x}\right){\gamma }_0{\gamma }^i{\nabla }_i^{tot}{\Psi }_{\Sigma }\right\}=-\mathrm{i}\int \mathrm{d}\mathbf{x}\sqrt{h}\left(\mathrm{Tr}\left\{\frac{1}{\sqrt{h}}\mathbf{\Phi }\left(\mathbf{x}\right){\gamma }_0{\gamma }^i{\nabla }_i^{tot}{\Psi }_{\Sigma }\right\}\right)\hfill \\ =-\mathrm{i}\int \mathrm{d}\mathbf{x}\sqrt{h}\mathrm{Tr}\left\{{\nabla }_i^{tot}\left(\frac{1}{\sqrt{h}}\mathbf{\Phi }\left(\mathbf{x}\right){\gamma }_0{\gamma }^i{\Psi }_{\Sigma }\right)\right\}+\mathrm{i}\int \mathrm{d}\mathbf{x}\left(\sqrt{h}\mathrm{Tr}\left\{{\nabla }_i^{tot}\left(\frac{1}{\sqrt{h}}\mathbf{\Phi }\left(\mathbf{x}\right){\gamma }_0{\gamma }^i\right){\Psi }_{\Sigma }\right\}\right)\hfill \\ =-\mathrm{i}{\oint }_{\partial \Sigma }\mathrm{d}{\mathbf{x}}_i\mathrm{Tr}\left\{\mathbf{\Phi }{\gamma }_0{\gamma }^i{\Psi }_{\Sigma }\right\}+\mathrm{i}\int \mathrm{d}\mathbf{x}\mathrm{Tr}\left\{\mathbf{\Phi }\left({\nabla }_i^{tot}\left({\gamma }_0{\gamma }^i\right)\right){\Psi }_{\Sigma }\right\}\hfill \\ +\mathrm{i}\int \mathrm{d}\mathbf{x}\left(\frac{-{\nabla }_i\sqrt{h}}{\sqrt{h}}\mathrm{Tr}\left\{\mathbf{\Phi }{\gamma }_0{\gamma }^i{\Psi }_{\Sigma }\right\}+\mathrm{Tr}\left\{\left({\nabla }_i^{tot}\mathbf{\Phi }\left(\mathbf{x}\right)\right){\gamma }_0{\gamma }^i{\Psi }_{\Sigma }\right\}\right),\hfill \end{array}$$

(36)

where $\mathrm{d}{\mathbf{x}}_i=d^{n-2}{\mathbf{x}|}_{\partial \Sigma }{n}_i\left(\mathbf{x}\right)$ is the measure of $(n-2)$-dimensional integration over the boundary $\partial \Sigma$ with the normal vector $n_i\left(\mathbf{x}\right)$ tangent to $\Sigma$. In the right hand side of (36),

(i)

the first boundary term is the result of the covariant Stokes theorem and it vanishes if ${\Psi }_{\Sigma }$ vanishes on the boundary $\partial \Sigma$, i.e., the spatial infinity;

(ii)

the following three terms follow from the Leibniz rule for the total covariant derivative ${\nabla }_i^{tot}$ with respect to the Clifford products of tensor Clifford-algebra-valued functions;

(iii)

in the second term, ${\nabla }_i^{tot}\left({\gamma }_0{\gamma }^i\right)=0$ due to the covariant constancy of Dirac matrices (12);

(iv)

in the third term, the metric compatibility yields ${\nabla }_i\sqrt{h}=0$;

(v)

in the fourth term, the explicit formula for $\mathbf{\Phi }\left(\mathbf{x}\right)$ in (31) yields

$${\nabla }_i^{tot}\mathbf{\Phi }\left(\mathbf{x}\right)=\frac{-\mathrm{i}}{\varkappa }\mathbf{\Phi }\left(\mathbf{x}\right)\left({\partial }_i\varphi {\gamma }^l{\partial }_l\varphi +\varphi {\gamma }^l{\partial }_{il}\varphi +\varphi \left({\nabla }_i^{tot}{\gamma }^l\right){\partial }_l\varphi \right).$$

(37)

By noticing that the last term in (37) vanishes due to (12) and substituting (37) into the last term in (36), using the covariant Stokes theorem and the assumption that the field configurations $\varphi \left(\mathbf{x}\right)$ vanish at the spatial infinity, we obtain

$$\begin{array}{c}\hfill \begin{array}{cc}\hfill \int \mathrm{d}\mathbf{x}\mathrm{Tr}\left\{\mathbf{\Phi }\left(\mathbf{x}\right)\frac{1}{\varkappa }{\gamma }_0{\Psi }_{\Sigma }\left(\mathbf{x}\right)\left(g^{il}{\partial }_i\varphi {\partial }_l\varphi +\varphi g^{il}{\partial }_{il}\varphi \right)\right\}& =\mathbf{\Psi }\int \mathrm{d}\mathbf{x}\sqrt{g}\left(g^{il}{\partial }_i\varphi {\partial }_l\varphi +\varphi g^{il}{\partial }_{il}\varphi \right)\hfill \\ \hfill & =-\mathbf{\Psi }\int \mathrm{d}\mathbf{x}\sqrt{h}{\nabla }_i^{}\left(\sqrt{g_{00}}{g}^{il}\right)\frac{1}{2}{\partial }_l{\varphi }^2=0,\hfill \end{array}\end{array}$$

(38)

where, in the first equality, we use the fact that $\mathrm{Tr}\left\{\mathbf{\Phi }\left(\mathbf{x}\right)\frac{1}{\varkappa \sqrt{g}}{\gamma }_0{\Psi }_{\Sigma }\left(\mathbf{x}\right)\right\}=\mathbf{\Psi }$ (c.f. (20)) and the covariant Stokes theorem in the second equality. The result is that the right-hand side of (38) vanishes because of the metricity of space-time: ${\nabla }_{\alpha }^{}{g}^{\mu \nu }=0$.

Therefore, it is demonstrated that in the limiting case (24) all four terms in the right-hand side of (36) vanish, so that the terms I and $IIIa$ in (14) do not contribute to the equation for the functional $\mathbf{\Psi }$.

By combining the above considerations we obtain from (14) the following equation for the functional $\mathbf{\Psi }$:

$$\mathrm{i}ħ{\partial }_t\mathbf{\Psi }=\int d\mathbf{x}\sqrt{g}\left(\frac{{ħ}^2}{2}\frac{g_{00}}{g}\frac{{\delta }^2}{\delta \varphi {\left(\mathbf{x}\right)}^2}-\frac{1}{2}g{}^{ij}{\partial }_i\varphi \left(\mathbf{x}\right){\partial }_j\varphi \left(\mathbf{x}\right)+V\left(\varphi \right)\right)\mathbf{\Psi }-\frac{\mathrm{i}}{4}\mathrm{Tr}\left\{\mathbf{\Phi }\left(\mathbf{x}\right)[{\omega }_0,{\Psi }_{\Sigma }]\right\}.$$

(39)

We see that the first three terms in the right hand side reproduce the canonical Hamiltonian operator in the functional derivative Schrödinger equation (1). However, the last term, which does not vanish in arbitrary non-static space-times where ${\omega }_0\ne 0$, still cannot be expressed in terms of $\mathbf{\Psi }$ alone. For this reason, we will treat static space-times with ${\omega }_0=0$ and non-static ones with ${\omega }_0\ne 0$ separately.

4. Static Space-Times with ${\mathbf{\omega }}_{\mathbf{0}}=\mathbf{0}$

In static space-times, when ${\omega }_0=0$, Equation (39) coincides with the canonical functional derivative Schrödinger equation (1). Thus the latter is derived from the precanonical Schrödinger equation as the limiting case corresponding to (24). In this case, we can also specify the functional $\Xi (\left[{\Psi }_{\Sigma }\left(\mathbf{x}\right)\right],\check{\mathbf{x}})$ in (33) by combining the observations presented above together and noticing that the relation (33) is valid at any given point $\mathbf{x}$. This is possible only if the functional $\mathbf{\Psi }$ is the continual product of identical terms at all points $\mathbf{x}$, namely,

$$\mathbf{\Psi }\sim \mathrm{Tr}\left\{\prod _{\mathbf{x}}{e}^{-i\varphi \left(\mathbf{x}\right){\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right)/\varkappa }\underline{\gamma }{}_0{\Psi }_{\Sigma }(\varphi \left(\mathbf{x}\right),\mathbf{x},t)\right\}{}_{|\varkappa \mapsto {\gamma }_0\delta \left(\mathbf{0}\right)/\sqrt{g}},$$

(40)

where ∼ means an equality up to a normalization factor which includes $\varkappa$ and $\sqrt{h}$.

The formal continual product expression in (40) can be understood as the multidimensional product integral [118,119]

(41)

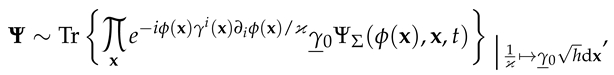
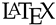where the notation of the product integral of matrix-valued functions $F\left(\mathbf{x}\right)$ as proposed by R. Gill [120] (and implemented in the package prodint) is used

(42)

The expression in (41) generalizes a similar result obtained in flat space-time earlier [101]. The only difference is that in curved space-time the spatial integration measure $\mathrm{d}\mathbf{x}$ is replaced by the invariant one $\sqrt{h}\mathrm{d}\mathbf{x}$ and the Dirac matrices in static space-times are $\mathbf{x}$-dependent.

In $(1+1)$-dimensional space-time, the product integral above is given by the well known path-ordered exponential, or the Peano–Baker series (also known as the Dyson series in the context of perturbative QFT and the path-ordered phase related to the Wilson loop in gauge theory), cf. Equation (55) below. A multidimensional generalization is briefly discussed in the books [118,119] and probably needs further refinement. However, in our case, instead of defining the product integral of arbitrary non-commutative matrices, we need only the trace of the product integral of Clifford-algebra valued functions. This significantly simplifies the task of defining the expression (41) mathematically. For example, in the one-dimensional case, the taking of the trace of each of the terms in the series expansion of the ordered exponential in (55)) implies that the matrices under the integrals in the series expansion of the trace of product integral are multiplied in the cycling permuted way, which can be generalized to the multidimensional case, rather than a time-ordered one, which implies a one-parameter ordering whose multidimensional generalization is problematic. Then, if the corresponding limit exists,

(43)

where $P\left(N\right)$ denotes all permutations of $(1,2,\dots ,N)$, the volume of integration $V\ni \mathbf{x}$ is partitioned into N small sub-volumes $\Delta {\mathbf{x}}_1,\dots ,\Delta {\mathbf{x}}_N$ whose volumes are taken to zero as $N\to \infty$, and $F\left({\mathbf{x}}_i\right)$ denotes the matrix F at a point ${\mathbf{x}}_i\in \Delta {\mathbf{x}}_i$. The existence of the limit in (43) and its independence on the partitioning of V into $N\to \infty$ sub-volumes $\Delta {\mathbf{x}}_i$ and the choice of points ${\mathbf{x}}_i$ within the subvolumes $\Delta {\mathbf{x}}_i$ imply a certain continuity of the dependence of the matrix elements of F of $\mathbf{x}$, similarly to the definition of the Riemann integral of functions.

Now, by taking into account the fact that some of the terms in (36) are proven to not contribute to the time evolution of $\mathbf{\Psi }$ we can write the effective equation for the time evolution of ${\Psi }_{\Sigma }$ which contains only the terms which do contribute to the time evolution of the wave functional $\mathbf{\Psi }$:

$$\begin{array}{cc}\hfill \mathrm{i}{\partial }_t{\Psi }_{\Sigma }& ={\gamma }_0\left(-\frac{\varkappa }{2}{\partial }_{\varphi \varphi }+\mathrm{i}{\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right){\partial }_{\varphi }+\frac{1}{\varkappa }V\left(\varphi \right)\right){\Psi }_{\Sigma }.\end{array}$$

(44)

By substituting ${\Psi }_{\Sigma }$ in the form

$${\Psi }_{\Sigma }=e^{+\frac{\mathrm{i}}{\varkappa }\varphi \left(\mathbf{x}\right){\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right)}{\Phi }_{\Sigma },$$

(45)

we obtain

$$\mathrm{i}{\partial }_t{\Psi }_{\Sigma }=e^{+\frac{\mathrm{i}}{\varkappa }\varphi \left(\mathbf{x}\right){\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right)}\mathrm{i}{\partial }_t{\Phi }_{\Sigma }$$

(46)

in the left hand side of (44) and

$${\gamma }_0{e}^{+\frac{\mathrm{i}}{\varkappa }\varphi \left(\mathbf{x}\right){\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right)}\left(-\frac{\varkappa }{2}{\partial }_{\varphi \varphi }-\frac{1}{2\varkappa }{g}^{ij}\left(\mathbf{x}\right){\partial }_i\varphi \left(\mathbf{x}\right){\partial }_j\varphi \left(\mathbf{x}\right)+\frac{1}{\varkappa }V\left(\varphi \right)\right){\Phi }_{\Sigma }$$

(47)

in the right hand side. Hence, ${\Phi }_{\Sigma }$ obeys

$$\mathrm{i}{\partial }_t{\Phi }_{\Sigma }=\tilde{\gamma }{}_0\left(\mathbf{x}\right)\left(-\frac{\varkappa }{2}{\partial }_{\varphi \varphi }-\frac{1}{2\varkappa }{g}^{ij}\left(\mathbf{x}\right){\partial }_i\varphi \left(\mathbf{x}\right){\partial }_j\varphi \left(\mathbf{x}\right)+\frac{1}{\varkappa }V\left(\varphi \right)\right){\Phi }_{\Sigma },$$

(48)

where

$$\tilde{\gamma }{}_{\mu }\left(\mathbf{x}\right):=e^{-\frac{\mathrm{i}}{\varkappa }\varphi \left(\mathbf{x}\right){\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right)}{\gamma }_{\mu }\left(\mathbf{x}\right)e^{+\frac{\mathrm{i}}{\varkappa }\varphi \left(\mathbf{x}\right){\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right)}.$$

(49)

Obviously, ${\left\{\tilde{\gamma }{}_{\mu }\left(\mathbf{x}\right)\tilde{\gamma }{}_{\nu }\left(\mathbf{x}\right)\right\}}_{+}={\left\{{\gamma }_{\mu }\left(\mathbf{x}\right){\gamma }_{\nu }\left(\mathbf{x}\right)\right\}}_{+}=2g_{\mu \nu }\left(\mathbf{x}\right)$, hence the transformation in (49) is a local Clifford algebra automorphism.

From Equation (48) one can conclude that ${\Phi }_{\Sigma }$ can be written in the form

$${\Phi }_{\Sigma }=(1+\underline{\gamma }{}^0){\Phi }_{\Sigma }^{\times },$$

(50)

where ${\Phi }_{\Sigma }^{\times }$ is a scalar function such that

$$\mathrm{i}{\partial }_t{\Phi }_{\Sigma }^{\times }=\sqrt{g_{00}}\left(-\frac{\varkappa }{2}{\partial }_{\varphi \varphi }-\frac{1}{2\varkappa }{g}^{ij}\left(\mathbf{x}\right){\partial }_i\varphi \left(\mathbf{x}\right){\partial }_j\varphi \left(\mathbf{x}\right)+\frac{1}{\varkappa }V\left(\varphi \right)\right){\Phi }_{\Sigma }^{\times }.$$

(51)

In terms of the scalar function ${\Phi }_{\Sigma }^{\times }$ Equation (41) takes the form

(52)

where we use the projector property of the matrix $\frac{1}{2}\left(1+\underline{\gamma }{}_0\right)$. Obviously, the multidimensional product integral of the scalar function ${\Phi }_{\Sigma }^{\times }$ is defined without any complications related to the definition of the product integral of non-commutative matrix functions.

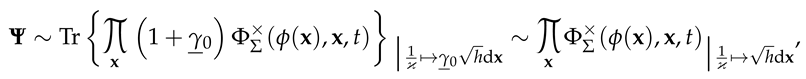
5. Non-Static Space-Times with ${\mathbf{\omega }}_{\mathbf{0}}\ne \mathbf{0}$

In non-static space-times, when ${\omega }_0\ne 0$, the last term in (39) does not allow us to obtain a close equation for the functional $\mathbf{\Psi }$. In order to find a way out, let us write the effective equation similar to (44) which governs the time evolution of ${\Psi }_{\Sigma }$, with the term I and the spatial part of the term $IIIa$ in (14) removed, as they are proven in (36) to have no contribution to the time evolution of the functional $\mathbf{\Psi }$:

$$\begin{array}{c}\hfill \mathrm{i}{\partial }_t{\Psi }_{\Sigma }={\gamma }_0\left(-\frac{\varkappa }{2}{\partial }_{\varphi \varphi }+\mathrm{i}{\gamma }^i{\partial }_i\varphi \left(\mathbf{x}\right){\partial }_{\varphi }+\frac{1}{\varkappa }V\left(\varphi \right))\right){\Psi }_{\Sigma }-\mathrm{i}[{\omega }_0,{\Psi }_{\Sigma }]=:\widehat{H}{}_0-\mathrm{i}[{\omega }_0,{\Psi }_{\Sigma }].\end{array}$$

(53)

We first note that by transforming ${\Psi }_{\Sigma }$ as follows:

$${\Psi }_{\Sigma }:=U{\Psi }_{\Sigma }^{\prime }{U}^{-1},$$

(54)

where

(55)

is the tranformation determined by the time-ordered exponential, we obtain

$$\mathrm{i}{\partial }_t\Psi =-\mathrm{i}[{\omega }_0,\Psi ]+U\mathrm{i}{\partial }_t{\Psi }_{\Sigma }^{\prime }{U}^{-1}.$$

(56)

Then

$$\mathrm{i}{\partial }_t{\Psi }_{\Sigma }^{\prime }=U^{-1}{\widehat{H}}_0{\Psi }_{\Sigma }U={\widehat{H}}_0^{\prime }{\Psi }^{\prime },$$

(57)

where

$${\Psi }^{\prime }:=U^{-1}{\Psi }_{\Sigma }U,{\widehat{H}}_0^{\prime }:=U^{-1}{\widehat{H}}_{0}U.$$

(58)

As the transformation U affects only the terms with ${\gamma }^{\mu }$-s,

$${\widehat{H}}_0{}^{\prime }={\gamma }_0^{\prime }\left(-\frac{\varkappa }{2}{\partial }_{\varphi \varphi }+\mathrm{i}{\gamma }^{\prime }{}^i{\partial }_i\varphi \left(\mathbf{x}\right){\partial }_{\varphi }+\frac{1}{\varkappa }V\left(\varphi \right)\right),$$

(59)

where

$${\gamma }^{\prime }{}^{\mu }(\mathbf{x},t):=U^{-1}(\mathbf{x},t){\gamma }^{\mu }\left(x\right)U(\mathbf{x},t).$$

(60)

It is easy to check that

$${\gamma }^{\prime }{}^{\mu }{\gamma }^{\prime }{}^{\nu }+{\gamma }^{\prime }{}^{\nu }{\gamma }^{\prime }{}^{\mu }=2U^{-1}{g}^{\mu \nu }U=2g^{\mu \nu }.$$

(61)

Hence the U-transformation is just an automorphism of the Clifford algebra of space-time given by the nonlocal transformation defined by the time-ordered exponential (55).

Using (57) one can write

$$\begin{array}{cc}\hfill \mathrm{i}{\partial }_t\mathbf{\Psi }& =\mathrm{Tr}\int \mathrm{d}\mathbf{x}\frac{\delta \mathbf{\Psi }}{\delta {\Psi }^{\prime }{}_{\Sigma }^T\left(\mathbf{x}\right)}\mathrm{i}{\partial }_t{\Psi }_{\Sigma }^{\prime }\hfill \end{array}$$

(62)

$$\begin{array}{c}=\mathrm{Tr}\int \mathrm{d}\mathbf{x}\frac{\delta \mathbf{\Psi }}{\delta {\Psi }^{\prime }{}_{\Sigma }^T\left(\mathbf{x}\right)}{\widehat{H}}_0^{\prime }{\Psi }_{\Sigma }^{\prime }.\hfill \end{array}$$

(63)

By comparing it with (7) and (8) we conclude that the results in static space-times with ${\omega }_0=0$ are generalized to non-static space-times with ${\omega }_0\ne 0$ using the U-transformed (primed) quantities:

$$\begin{array}{ccc}\hfill {\gamma }^{\mu }& \to {\gamma }^{\prime \mu }& =U^{-1}{\gamma }^{\mu }U,\hfill \end{array}$$

(64)

$$\begin{array}{ccc}\hfill {\Psi }_{\Sigma }& \to {\Psi }_{\Sigma }^{\prime }& =U^{-1}{\Psi }_{\Sigma }U,\hfill \end{array}$$

(65)

$$\begin{array}{ccc}\hfill {\widehat{H}}_{0\Sigma }& \to \widehat{H}{}_{0\Sigma }^{\prime }& =U^{-1}{H}_{0\Sigma }U,\hfill \end{array}$$

(66)

with U given by the time-ordered exponential in (55). Then, the wave functional (41) rewritten in terms of the primed objects,

(67)

represents, up to a normalization factor, the Schrödinger wave functional in terms of precanonical wave functions in an arbitrary curved space-time, and it satisfies (39) without the last term, i.e., the functional derivative Schrödinger equation (1).

In summary, we have demonstrated that in curved space-times the canonical functional derivative Schrödinger equation (1) and the explicit product integral Formula (41) relating the Schrödinger wave functional with the Clifford-valued precanonical wave function can be derived from the precanonical Schrödinger equation (2) in the singular limiting case when $\underline{\gamma }{}_0\varkappa$ is replaced by $\delta \left(\mathbf{0}\right)/\sqrt{h}$, a regularized invariant delta-function at coinciding spatial points. A natural interpretation of the latter is that it represents the UV cutoff of the total volume of the momentum space which one has to introduce in order to make sense of the second variational derivative at coinciding points in Equation (1). As in the previously considered case of quantum fields in flat space-time [98,100,101], the standard unregularized formulation of QFT in curved space-time in functional Schrödinger representation thus emerges from the precanonical description as a singular limiting case.

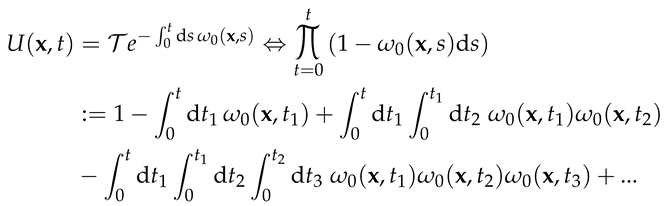
6. Conclusions

We explored a connection between the description of an interacting quantum scalar field in curved space-time derived from precanonical quantization and the standard description in the functional Schrödinger picture resulting from the canonical quantization.

We have demonstrated that the functional derivative Schrödinger equation (1) can be derived from the partial derivative precanonical Schrödinger equation (2) in the limiting case (24). Namely, the restriction of the precanonical Schrödinger equation to the subspace $\Sigma$ representing a field configuration at time t, Equation (8), governs the time evolution of the wave functional according to (7) and (14). Then, in the limiting case (24),

(i)

the potential term in (1) is reproduced by the term V in (14);

(ii)

The second functional derivative term in (1) is reproduced by the term $IV$ in (14) up to two additional terms which have no obvious counterpart in (1);

(iii)

The required cancellation of one of those additional terms with a similar term $II$ in (14), which also has no obvious counterpart in (1), leads to the expression of the Schrödinger wave functional as the trace of the continuous product of restricted precanonical wave functions (40) over all spatial points, which we later interpret as a multidimensional product integral, Equation (41);

(iv)

The expression of the wave functional in terms of precanonical wave functions substituted into the second additional term mentioned in (ii) reproduces the second term in the right-hand side of (1), which is responsible for non-ultralocality;

(v)

The expression of the wave functional in terms of precanonical wave functions obtained in (iii) also implies that under the boundary conditions of vanishing fields $\varphi \left(\mathbf{x}\right)$ and ${\Psi }_{\Sigma }(\varphi \left(\mathbf{x}\right),\mathbf{x},t)$ at the spatial infinity the terms I and $IIIa$ in (14) do not contribute to the canonical Schrödinger equation (1);

(vi)

As a consequence of (i)–(v), in static space-times with ${\omega }_0=0$, the functional Schrödinger equation (1) is thus derived from the precanonical Schrödinger equation (2) and the Schrödinger wave functional is expressed as the trace of the product integral of precanonical wave functions;

(vii)

In non-static space-times with ${\omega }_0\ne 0$, the transformation (55) absorbs the contribution of the term $IIIb$ in (14) thus allowing us again to obtain the functional Schrödinger equation (1) from the precanonical Schrödinger equation (2) and to express the Schrödinger wave functional in terms of transformed precanonical wave functions;

(viii)

Both in static and non-static space-times, the Schrödinger wave functional can be represented as the product integral of transforms of the restricted precanonical wave function, which are derived from the precanonical wave function by a series of transformations in Section 4 and Section 5.

The result of this paper generalizes to arbitrary space-times (with $g_{0i}=0$) the statement of our previous papers [98,100,101] that the standard functional Schrödinger representation of QFT is a certain singular limiting case of the theory of quantum fields obtained by precanonical quantization.

The symbolic or singular nature of the limiting transition from precanonical quantization to the standard formulation of QFT in functional Schrödinger representation is related to the fact that the latter, due to the presence of the second functional derivative at coinciding points, is not a well-defined theory unless a regularization is introduced. The regularization typically introduces a UV cutoff scale as an additional element of the theory removed by a subsequent renormalization. In precanonical quantization, the ultraviolet scale $\varkappa$ appears as an inherent element quantization, which, unlike other theories introducing an ultraviolet fundamental length or cutoff, does not alter the relativistic space-time at smaller scales.

This rises a question: is $\varkappa$ a fundamental scale or an auxiliary element of precanonical quantization of fields. On the one hand, in free scalar theory, $\varkappa$ disappears from the observable characteristics of a quantum field because the spectrum of DW Hamiltonian operator is proportional to $\varkappa$. However, in interacting scalar theory, powers of $\varkappa$ enter in the perturbative corrections to the spectrum of DW Hamiltonian $\widehat{H}$ thus suggesting that $\varkappa$ can be renormalized away by absorbing the expressions with the bare mass, bare coupling constant and $\varkappa$ in the “observed mass”. On the other hand, an estimation of the mass gap in $(3+1)$-dimensional quantum pure SU(2) gauge theory derived by precanonical quantization: $\Delta m\gtrsim 0.86{(g^2\varkappa )}^{1/3}$ [97] (g is the bare gauge coupling constant) and a naive estimation of the cosmological constant based on the precanonically quantized pure Einstein gravity [76] seem to consistently point to the very rough estimation of the scale of $\varkappa$ at ∼${10}^2$ MeV, i.e., well below the Planck scale. We hope to clarify this surprising fact and the nature of $\varkappa$ in our future work.