Full-Scale Experimental and Numerical Investigations on the Modal Parameters of a Single-Span Steel-Frame Footbridge
Abstract
:1. Introduction
2. Related Works
3. Materials and Methods
3.1. Concepts and Methods of the Investigation
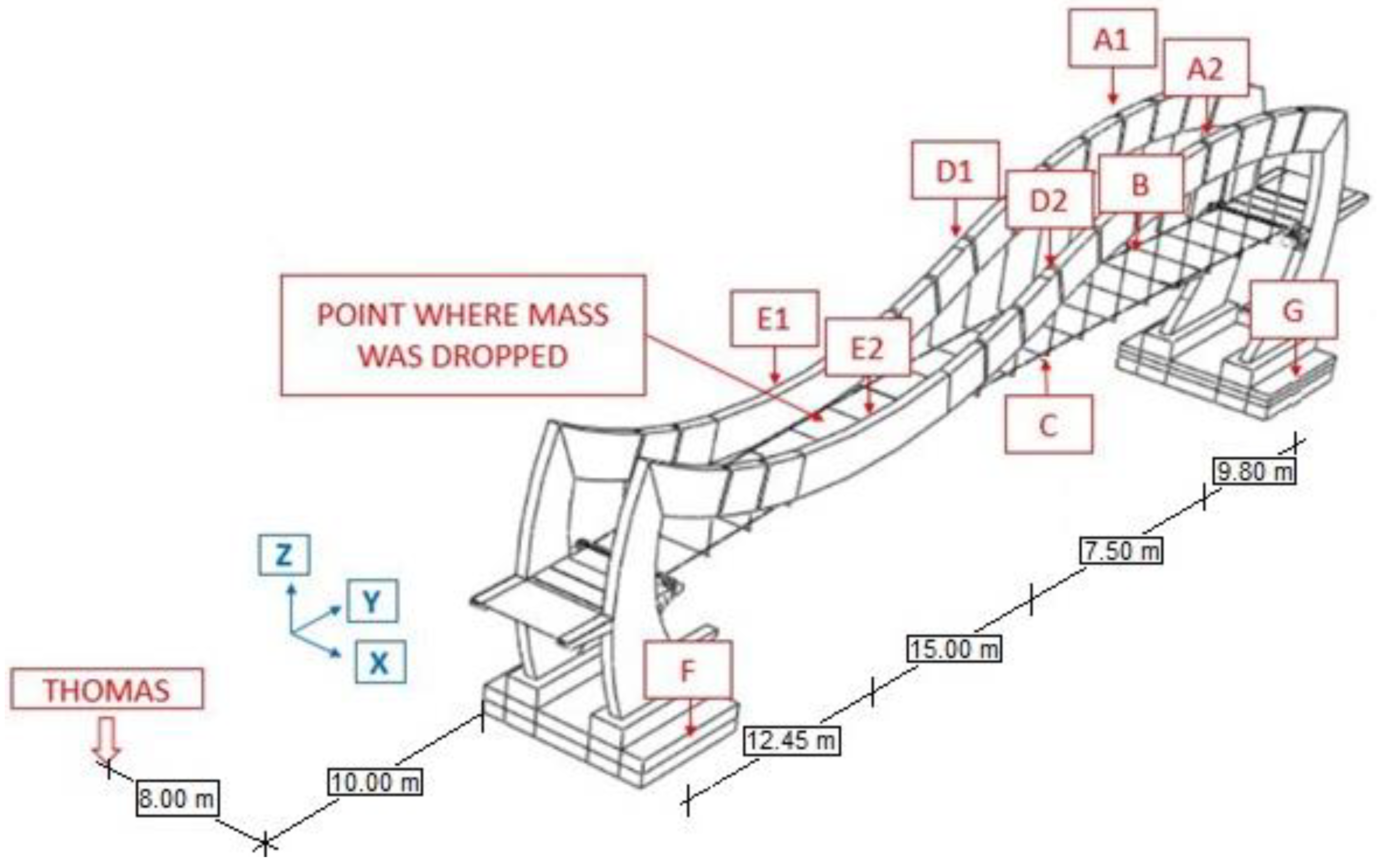
3.2. Structural Layout of the Footbridge and Experimental Setup
3.3. Preliminaries
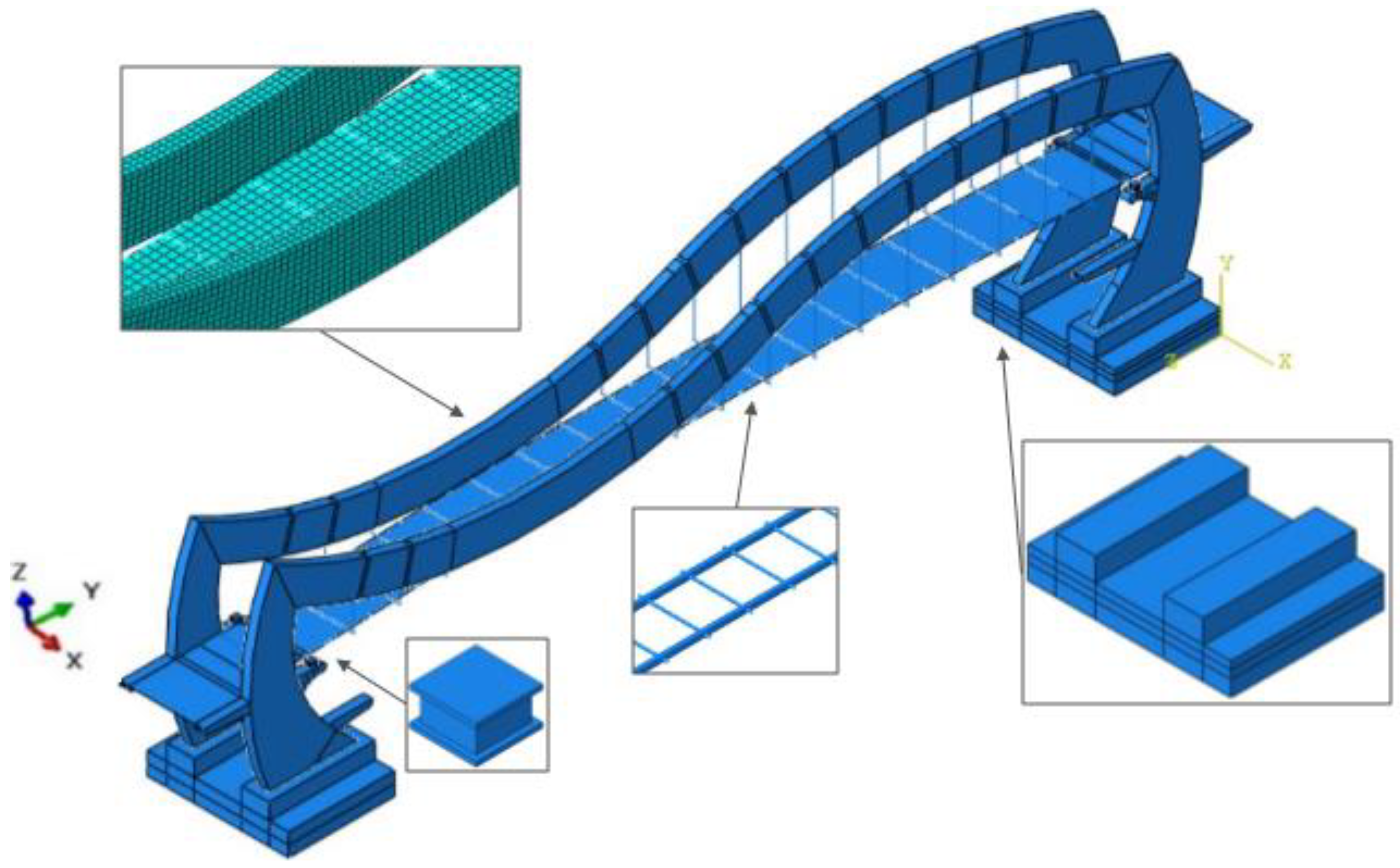
3.4. Finite Element (FE) Model of the Footbridge
4. Results
4.1. Numerical Calculation of Natural Frequencies and Modes of Vibration of the Footbridge
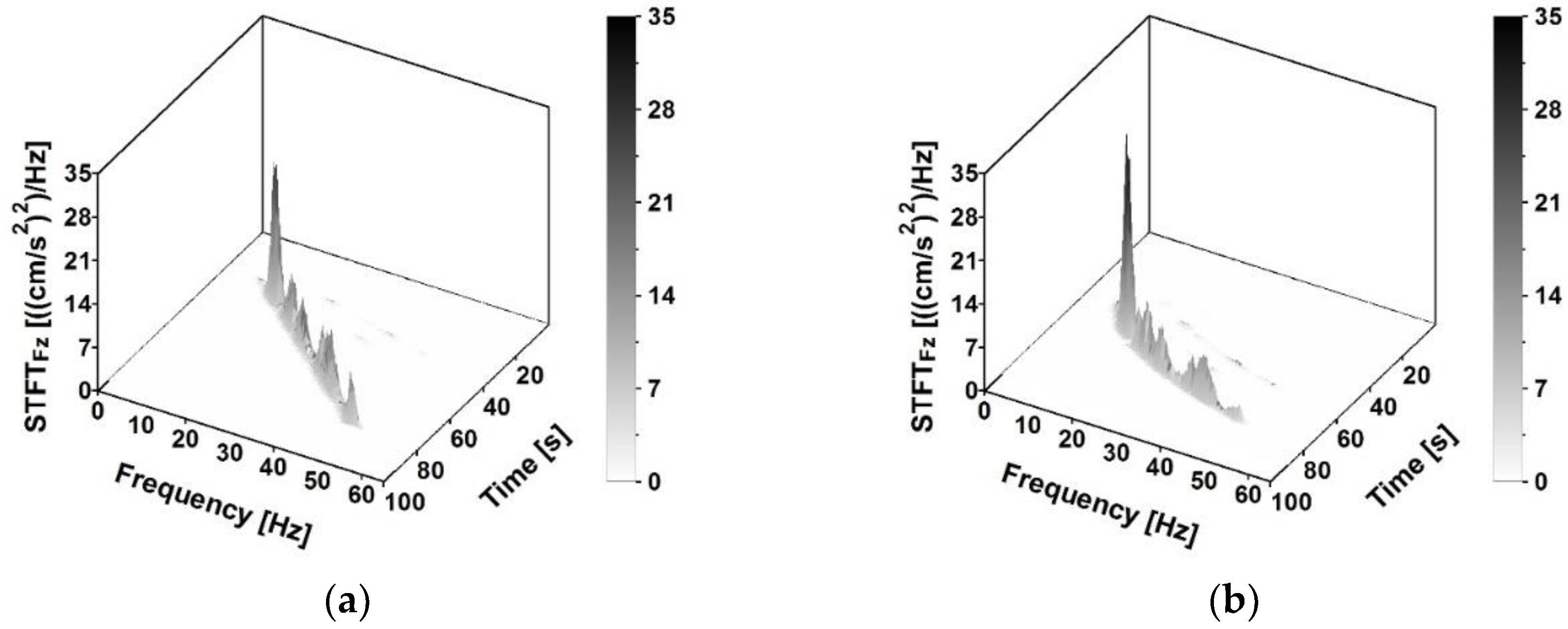
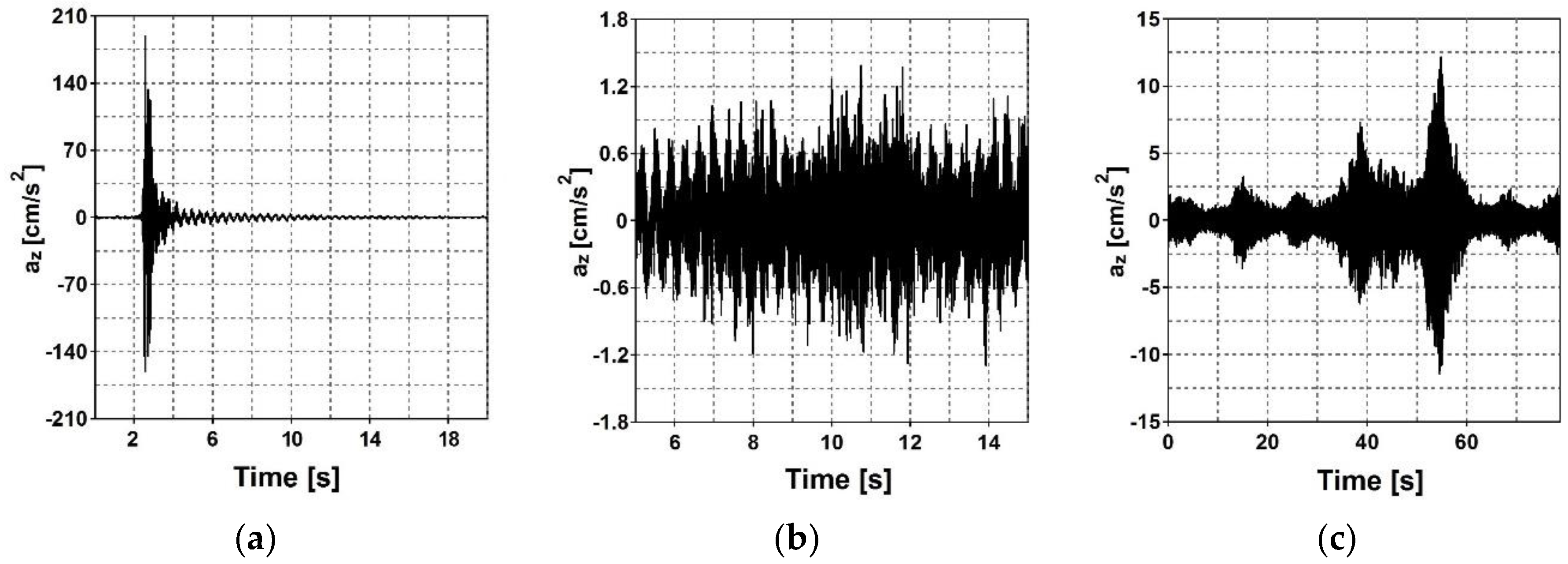
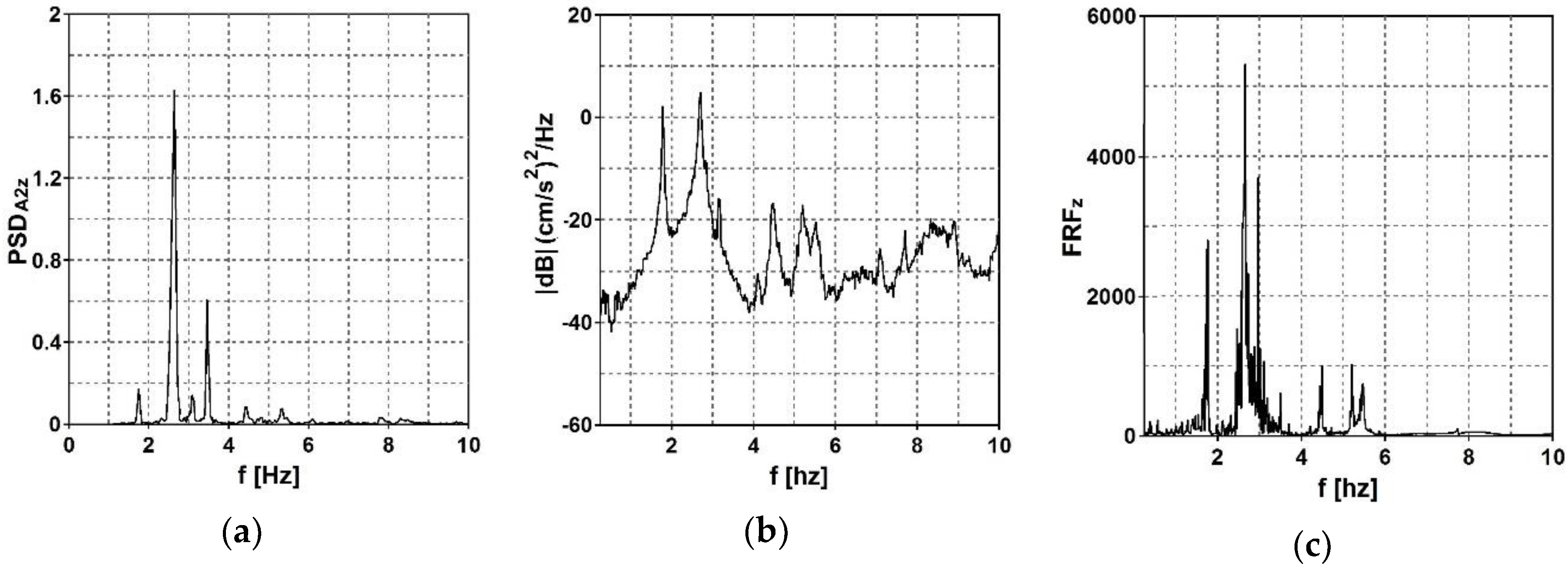
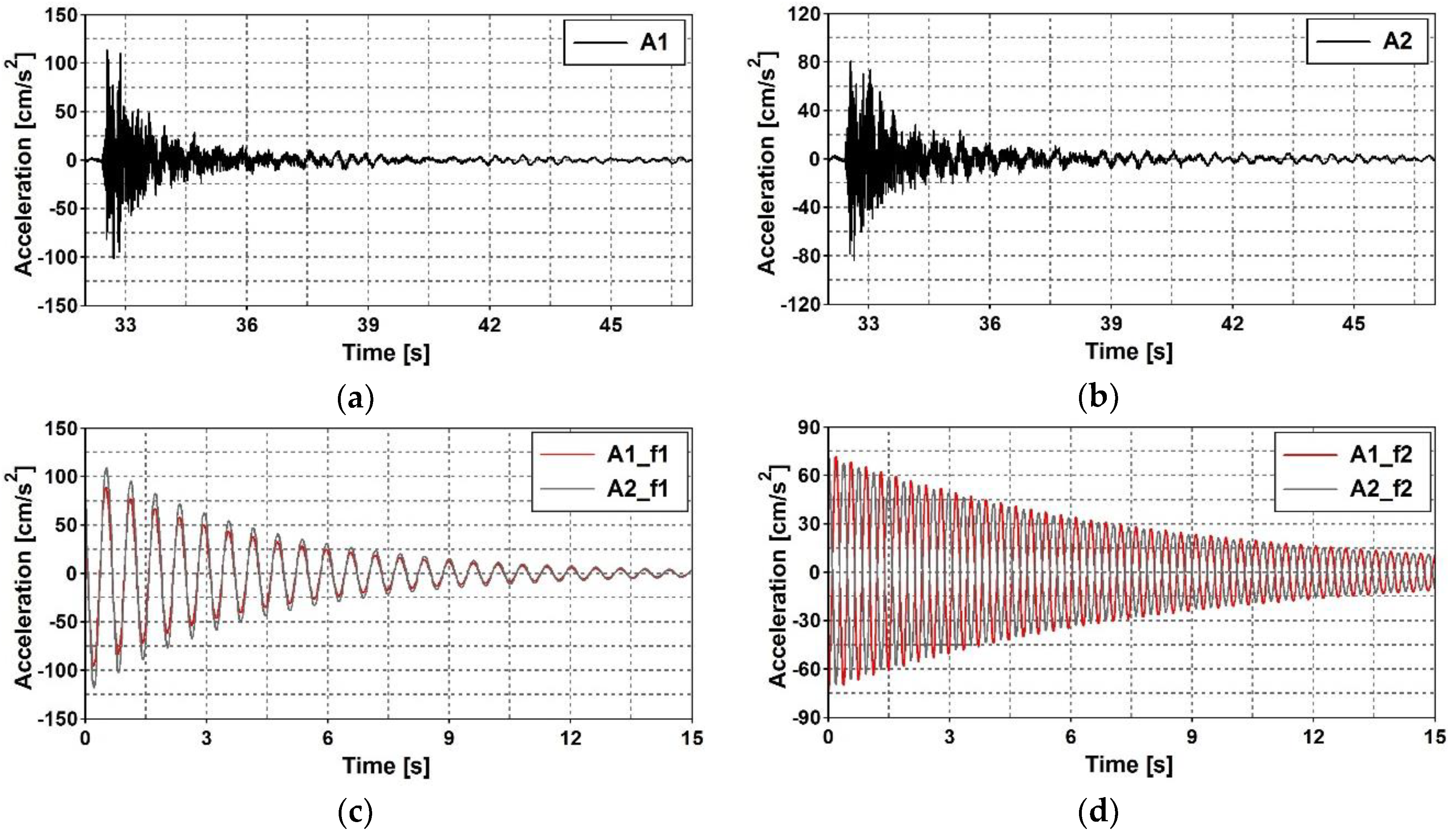
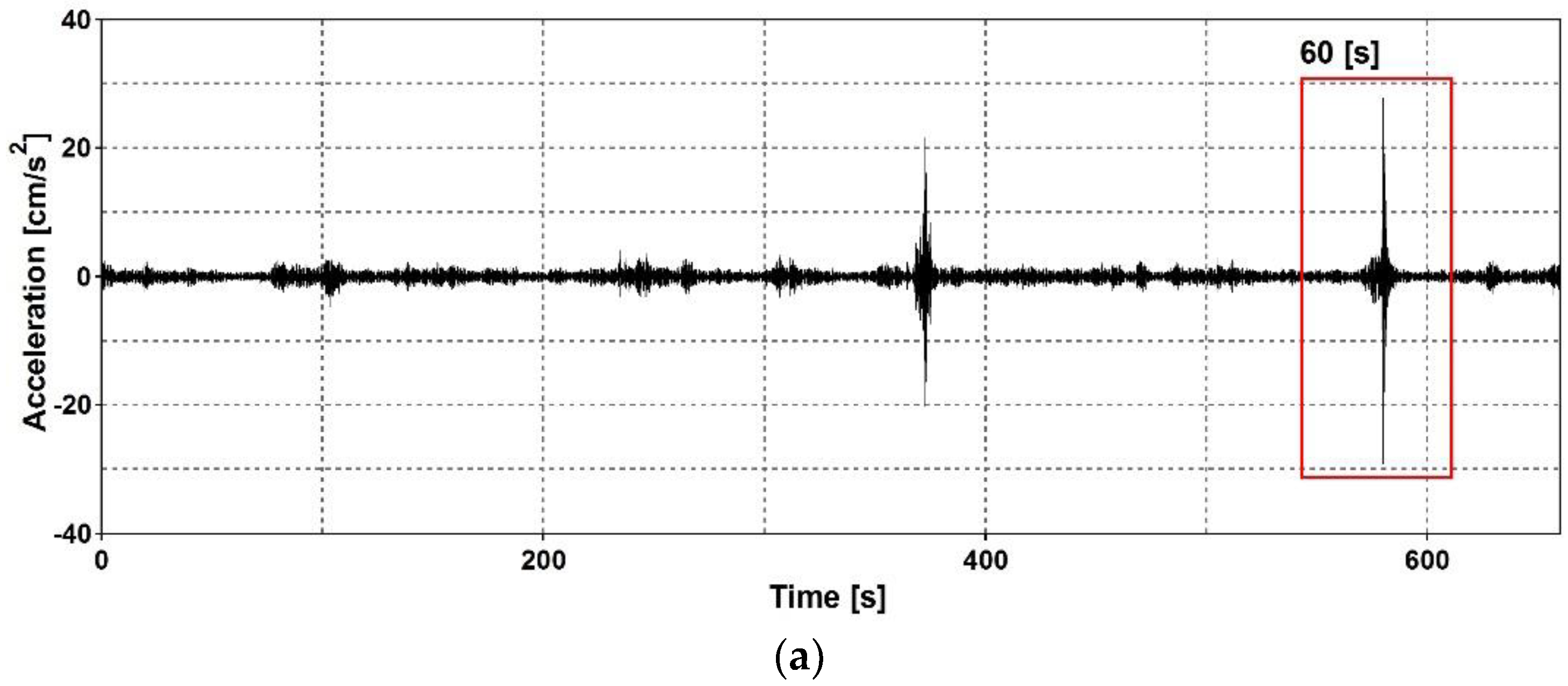
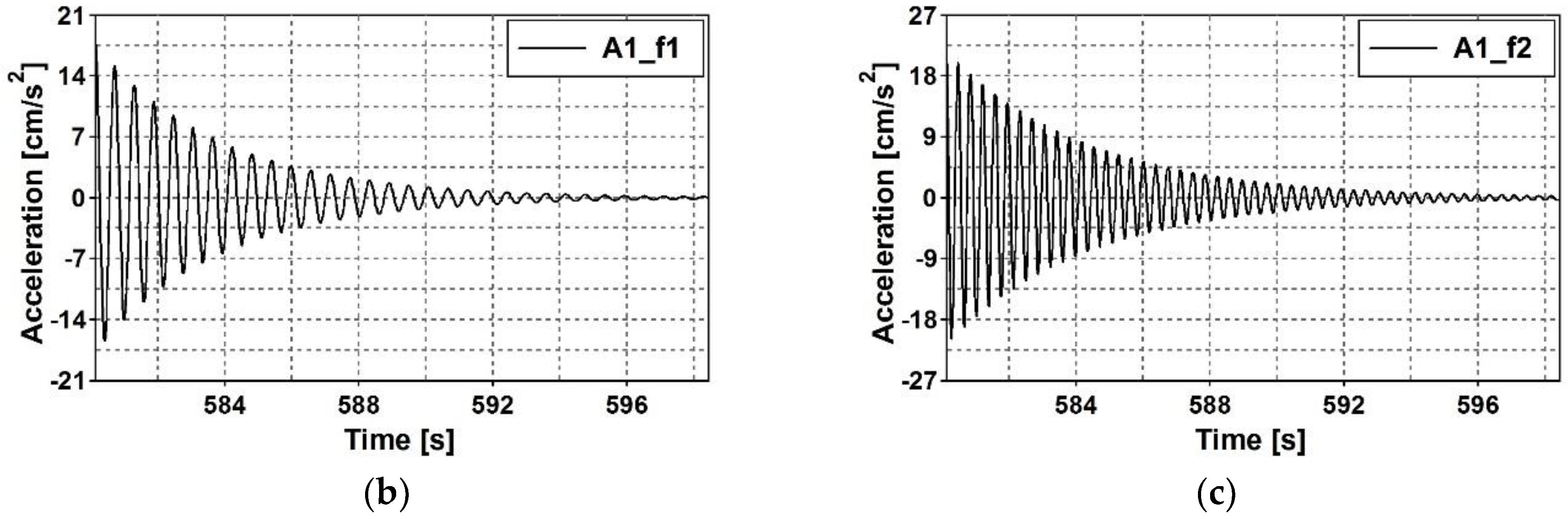
4.2. Experimental Evaluation of Dynamic Properties of the Footbridge
5. Discussion
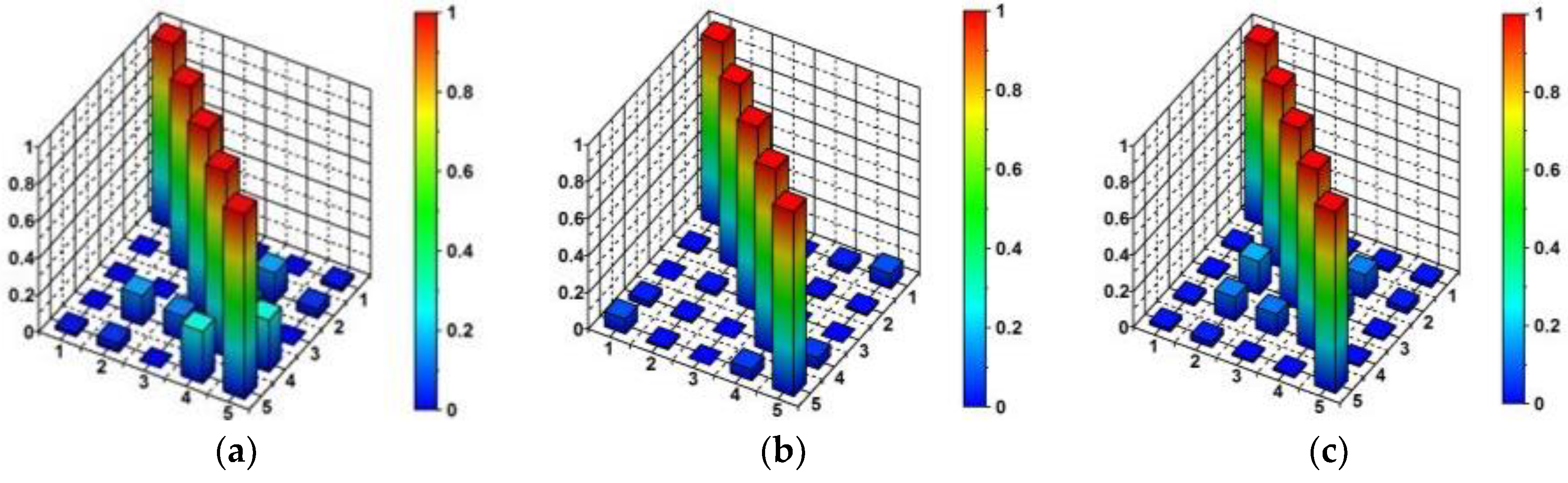
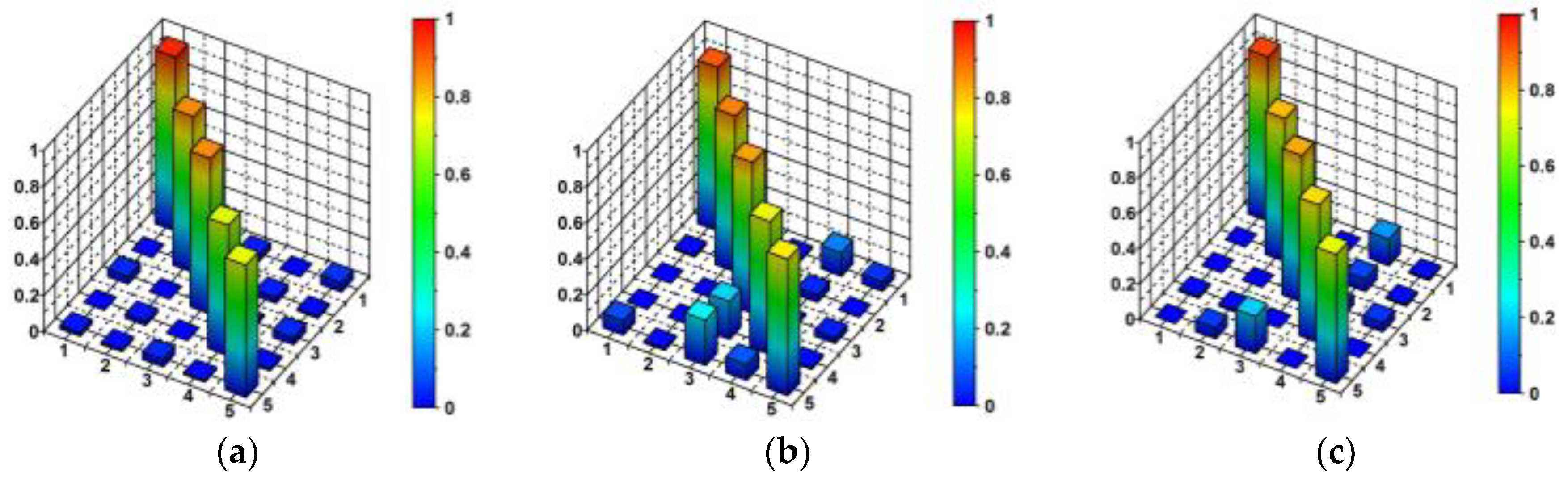
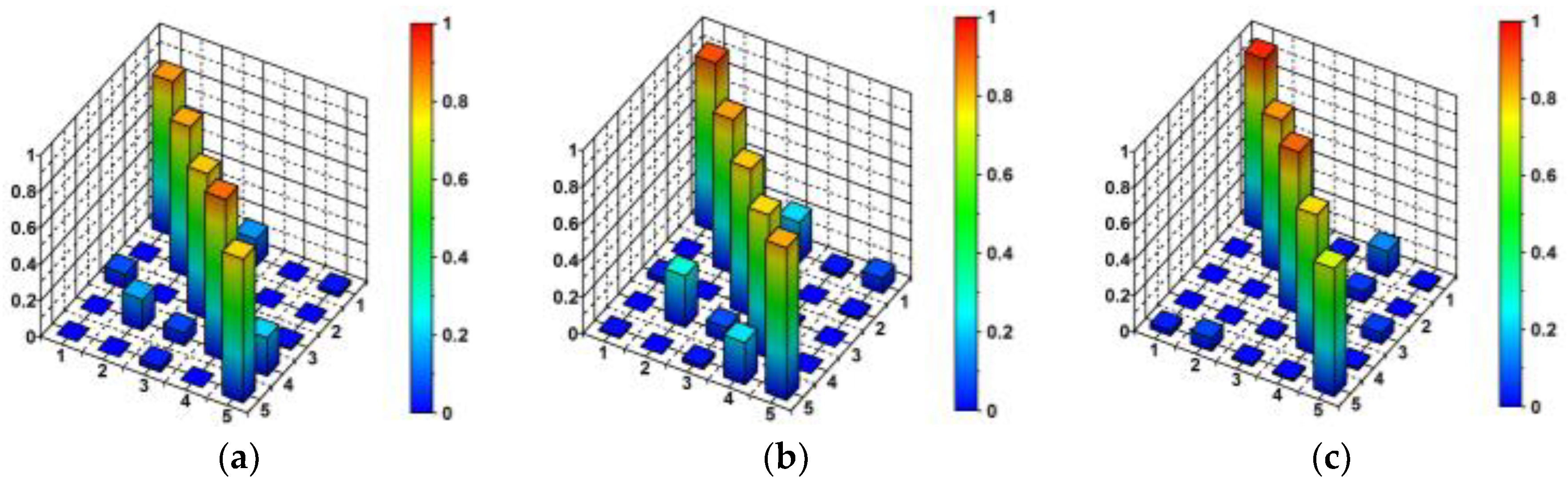
5.1. Investigation of Self-Correlation of the Experimental Mode Shapes
5.2. Experimental and Numerical Modal Models Validation
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bachmann, H. ‘Lively’ footbridges—Real challenge. In Proceedings of the International Conference on the Design and Dynamic Behaviour of Footbridges, Footbridge 2002, Paris, France, 20–22 November 2002. [Google Scholar]
- Flaga, A. Footbridges; WKŁ: Warsaw, Poland, 2011. (In Polish) [Google Scholar]
- Caetano, E.; Cunha, A. Dynamic Design of Slender Footbridges. Structures and Architecture: Concepts, Applications and Challenges. In Proceedings of the 2nd International Conference on Structures and Architecture (ICSA 2013), Guimarães, Portugal, 24–26 July 2013. [Google Scholar]
- Oviedo-Trespalacios, O.; Scott-Parker, B. Footbridge usage in high-traffic flow highways: The intersection of safety and security in pedestrian decision-making. Transp. Res. Part F Traffic Psychol. Behav. 2017, 49, 177–187. [Google Scholar] [CrossRef]
- Drygala, I.J.; Polak, M.A.; Dulinska, J.M. The dynamic evaluation of composite materials footbridges. In Proceedings of the 39th IABSE Symposium, Vancouver, IABSE c/o ETH Hönggerberg, Zürich, Switzerland, 21–23 September 2017; pp. 2285–2292. [Google Scholar]
- Russell, J.; Wei, X.; Živanović, S.; Kruger, C. Dynamic Response of an FRP Footbridge due to Pedestrians and Train Buffeting. Procedia Eng. 2017, 199, 3059–3064. [Google Scholar] [CrossRef]
- Dallard, P.; Fitzpatrick, A.J.; Flint, A.; Le Bourva, S.; Low, A.; Ridsdill Smith, R.M.; Willford, M. The London Millennium Footbridge. Struct. Eng. 2001, 79, 17–33. [Google Scholar]
- Piccardo, G.; Tubino, F. Equivalent spectral model and maximum dynamic response for the serviceability analysis of footbridges. Eng. Struct. 2012, 40, 445–456. [Google Scholar] [CrossRef]
- Ali, N.B.H.; Rhode-Barbarigos, L.; Albi, A.A.P.; Smith, I.F.C. Design optimization and dynamic analysis of a tensegrity-based footbridge. Eng. Struct. 2010, 32, 3650–3659. [Google Scholar] [CrossRef]
- Živanović, S.; Pavic, A.; Reynolds, P. Vibration serviceability of footbridges under human-induced excitation: A literature review. J. Sound Vib. 2005, 279, 1–74. [Google Scholar] [CrossRef]
- Matsumoto, Y.; Nishioka, T.; Shiojiri, H.; Matsuzaki, K. Dynamic design of footbridges. IABSE Proc. 1978, 17, 1–15. [Google Scholar]
- Van Nimmen, K.; Lombaert, G.; De Roeck, G.; Van den Broeck, P. Vibration serviceability of footbridges: Evaluation of the current codes of practice. Eng. Struct. 2014, 59, 448–461. [Google Scholar] [CrossRef]
- Association Française de Génie Civil (AFGC). SÉTRA 2006: Assessment of Vibrational Behaviour of Footbridges under Pedestrian Loading; Technical Guide; Technical Department for Transport, Roads and Bridges Engineering and Road Safety: Paris, France, 2006. [Google Scholar]
- EN 1990:2002/Al (2005) Eurocode—Basis of Structural Design; CEN: Brussels, Belgium, 2005.
- Pańtak, M. Elaboration of the vibration comfort criteria for footbridges during vibrations induced by pedestrians. In Bridge Maintenance, Safety, Management, Resilience and Sustainability, Proceedings of the Sixth International Conference on Bridge Maintenance, Safety and Management, Lake Maggiore, Italy, 8–12 July 2012; CRC Press: Boca Raton, FL, USA, 2012; pp. 2334–2339. [Google Scholar]
- Živanović, S.; Pavic, A.; Reynolds, P. Finite element modelling and updating of a lively footbridge: The complete process. J. Sound Vib. 2007, 301, 126–145. [Google Scholar] [CrossRef]
- Lai, E.; Gentile, C.; Mulas, M.G. Experimental and numerical serviceability assessment of a steel suspension footbridge. J. Constr. Steel Res. 2017, 132, 16–28. [Google Scholar] [CrossRef]
- Murzyn, I.J. Dynamic Response Analysis of Footbridges under Seismic and Paraseismic Loading. Ph.D. Thesis, Cracow University of Technology (CUT), Kraków, Poland, 2016. (In Polish). [Google Scholar]
- Tubino, F. Probabilistic assessment of the dynamic interaction between multiple pedestrians and vertical vibrations of footbridges. J. Sound Vib. 2018, 417, 80–96. [Google Scholar] [CrossRef]
- Drygala, I.J.; Dulinska, J.M. A theoretical and experimental evaluation of the modal properties of a cable-stayed footbridge. Procedia Eng. 2017, 199, 2937–2942. [Google Scholar] [CrossRef]
- Marecik, K.; Pańtak, M. A comparative analysis of selected models of pedestrian-generated dynamic loads on footbridges-vertical loads. MATEC Web Conf. 2018, 222. [Google Scholar] [CrossRef]
- Wootton, A.J.; Butcher, J.B.; Kyriacou, T.; Day, C.R.; Haycock, P.W. Structural health monitoring of a footbridge using Echo State Networks and NARMAX. Engineering Applications of Artificial Intelligence. Eng. Appl. Artif. Intell. 2017, 64, 152–163. [Google Scholar] [CrossRef]
- Occhiuzzi, A.; Spizzuoco, M.; Ricciardelli, F. Loading models and response control of footbridges excited by running pedestrians. Struct. Control Health Monit. 2008, 15, 349–368. [Google Scholar] [CrossRef]
- Salgado, R.; Brancob, J.M.; Cruz, P.J.S.; Ayala, G. Serviceability assessment of the Góis footbridge using vibration monitoring. Case Stud. Nondestruct. Test. Eval. 2014, 2, 71–76. [Google Scholar] [CrossRef]
- Taylor, I.J.; Vezza, M. A numerical investigation into the aerodynamic characteristics and aeroelastic stability of a footbridge. J. Fluid Struct. 2009, 25, 155–177. [Google Scholar] [CrossRef]
- Drygala, I.J.; Dulinska, J.M.; Wazowski, M. Seismic performance of a cable-stayed footbridge using a concrete damage plasticity model. Procedia Eng. 2017, 193, 525–532. [Google Scholar] [CrossRef]
- Wang, D.; Wu, C.; Zhang, Y.; Li, S. Study on vertical vibration control of long-span steel footbridge with tuned mass dampers under pedestrian excitation. J. Constr. Steel Res. 2019, 154, 84–98. [Google Scholar] [CrossRef]
- Gheitasi, A.; Ozbulut, O.E.; Usmani, S.; Alipour, M.; Harris, D.K. Experimental and analytical vibration serviceability assessment of an in-service footbridge. Case Stud. Nondestruct. Test. Eval. 2016, 6, 79–88. [Google Scholar] [CrossRef]
- Simulia Corp. ABAQUS Users’ Manual v. 6.13; Dassault Systemes Simulia Corp.: Providence, RI, USA, 2013. [Google Scholar]
- Ewins, D.J. Modal Testing: Theory, Practise and Application, 2nd ed.; Research Studies Press Ltd.: Philadelphia, PA, USA, 2000. [Google Scholar]
- Lalanne, C. Mechanical Vibration and Shock Analysis, 2nd ed.; ISTE Ltd.: London, UK, 2009; Volume 2. [Google Scholar]
- Uhl, T.; Lisowski, W.; Kurowski, P. In-Operation Modal Analysis and Its Application; Wydawnictwo Katedry Robotyki i Dynamiki Maszyn, University of Science and Technology: Krakow, Poland, 2001. [Google Scholar]
- Ting, T.; Chen, T.L.C.; Twomey, W. Correlating mode shapes based on the modal assurance criterion. Finite Elem. Anal. Des. 1993, 14, 353–360. [Google Scholar] [CrossRef]
- Guo, N.; Yang, Z.; Wang, L.; Ouyang, Y.; Zhang, X. Dynamic model updating based on strain mode shape and natural frequency using hybrid pattern search technique. J. Sound Vib. 2018, 422, 112–130. [Google Scholar] [CrossRef]
- Au, S.K.; Zhang, F.L. On assessing the posterior mode shape uncertainty in ambient modal identification. Probabilist Eng. Mech. 2011, 26, 427–434. [Google Scholar] [CrossRef]
- Buckle, I.; Nagarajaiah, S.; Ferrell, K. Stability of Elastomeric Isolation Bearings: Experimental Study. J. Struct. Eng. 2002, 128, 3–11. [Google Scholar] [CrossRef]







| Modal Model | Natural Frequency (Hz) Corresponding to: | ||||
|---|---|---|---|---|---|
| 1st Mode | 2nd Mode | 3rd Mode | 4th Mode | 5th Mode | |
| Numerical | 1.71 | 2.45 | 3.33 | 3.41 | 4.40 |
| Experimental Modal Model | Natural Frequency (Hz) Corresponding to: | ||||
|---|---|---|---|---|---|
| 1st Mode | 2nd Mode | 3rd Mode | 4th Mode | 5th Mode | |
| Shock excitation | 1.72 | 2.61 | 3.04 | 3.49 | 4.44 |
| Ambient vibration | 1.76 | 2.68 | 3.15 | 4.19 | 4.47 |
| Sine sweep testing | 1.77 | 2.61 | 2.93 | 3.45 | 4.44 |
| Experimental Modal Model | Logarithmic Decrement [-] Corresponding to: | ||||
|---|---|---|---|---|---|
| 1st Mode | 2nd Mode | 3rd Mode | 4th Mode | 5th Mode | |
| Shock excitation | 0.145 | 0.108 | 0.075 | 0.046 | 0.029 |
| Ambient vibration | 0.131 | 0.101 | 0.056 | 0.035 | 0.025 |
| Sine sweep testing | 0.139 | 0.105 | 0.063 | 0.042 | 0.026 |
| Mode | Numerical Analysis [Hz] | Shock Excitation | Ambient Vibration | Slow Sine Sweep Testing | |||
|---|---|---|---|---|---|---|---|
| fi [Hz] | Error [%] | fi [Hz] | Error [%] | fi [Hz] | Error [%] | ||
| 1 | 1.71 | 1.72 | 0.58 | 1.76 | 2.84 | 1.77 | 3.39 |
| 2 | 2.45 | 2.61 | 6.13 | 2.68 | 8.58 | 2.61 | 6.13 |
| 3 | 3.33 | 3.04 | 9.54 | 3.15 | 5.71 | 2.93 | 13.65 |
| 4 | 3.41 | 3.49 | 2.29 | 4.19 | 18.62 | 3.45 | 1.16 |
| 5 | 4.40 | 4.44 | 0.90 | 4.47 | 1.57 | 4.44 | 0.90 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Drygala, I.J.; Dulinska, J.M. Full-Scale Experimental and Numerical Investigations on the Modal Parameters of a Single-Span Steel-Frame Footbridge. Symmetry 2019, 11, 404. https://doi.org/10.3390/sym11030404
Drygala IJ, Dulinska JM. Full-Scale Experimental and Numerical Investigations on the Modal Parameters of a Single-Span Steel-Frame Footbridge. Symmetry. 2019; 11(3):404. https://doi.org/10.3390/sym11030404
Chicago/Turabian StyleDrygala, Izabela Joanna, and Joanna Maria Dulinska. 2019. "Full-Scale Experimental and Numerical Investigations on the Modal Parameters of a Single-Span Steel-Frame Footbridge" Symmetry 11, no. 3: 404. https://doi.org/10.3390/sym11030404





