Periodic Solution of the Strongly Nonlinear Asymmetry System with the Dynamic Frequency Method
Abstract
:1. Introduction
2. The Basic Idea of the Dynamic Frequency Method
- order 1:
- order 2:
- order :
3. Examples
3.1. Duffing Oscillators
3.2. Oscillator with Coordinate-Dependent Mass
4. Strategy to Improve the Accuracy of Computation
4.1. Modified Energy Balance Method
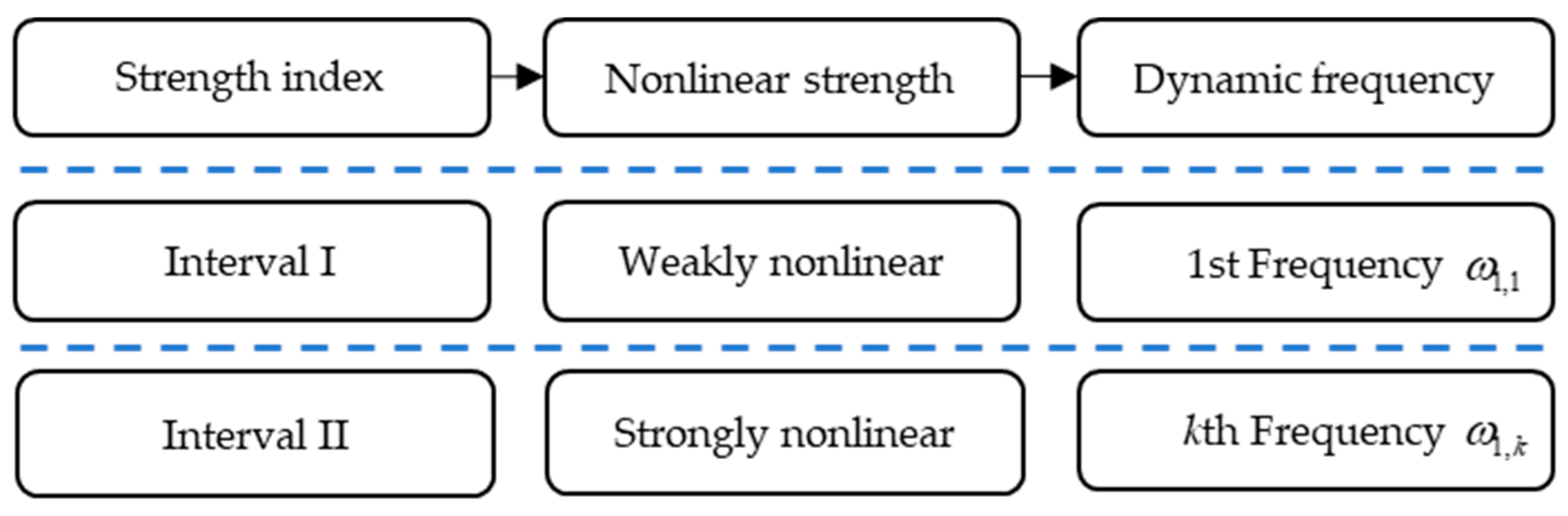
4.2. The Nonlinear Strength Index
5. Conclusions
Author Contributions
Funding
Acknowledgement
Conflicts of Interest
References
- Rontó, A.; Rontó, M. Periodic successive approximations and interval halving. Miskolc Math. Notes 2012, 13, 459–482. [Google Scholar] [CrossRef]
- Rontó, A.; Rontó, M.; Jana, V. A new approach to non-local boundary value problems for ordinary differential systems. Appl. Math. Comput. 2015, 250, 689–700. [Google Scholar] [CrossRef]
- Rontó, M. Numerical-analytic successive approximation method for nonlinear boundary value problems. Nonlinear Anal. Theor. 1997, 30, 3179–3188. [Google Scholar] [CrossRef]
- Rontó, M. On two numerical-analytic methods for the investigation of periodic solutions. Period. Math. Hung. 2008, 56, 121–135. [Google Scholar] [CrossRef]
- Mitropolskii, Y.A.; Moseekov, B.I. Asymptotic Solutions of Partial Differential Equations; Vyshcha Shkola: Kiev, Ukraine, 1976. [Google Scholar]
- Mickens, R.E. Oscillations in Planar Dynamic Systems; World Scientific: Singapore, 1996. [Google Scholar]
- Bogolubov, N.N.; Mitropolski, Y.A. Asymptotic Methods in the Theory of Nonlinear Oscillations; CRC Press: New York, NY, USA, 1961. [Google Scholar]
- Krylov, N.M.; Bogoliubov, N.N. Introduction to Nonlinear Mechanics; Princeton University Press: Princeton, NJ, USA, 1947. [Google Scholar]
- Mahmoud, G.M. Approximate solutions of a class of complex nonlinear dynamical systems. Phys. A 1998, 253, 211–222. [Google Scholar] [CrossRef]
- Sandri, G. A new method of expansion in mathematical physics. Il Nuovo Cimento 1965, 36, 67–93. [Google Scholar] [CrossRef]
- Lindstedt, A. Ueder die integration einer fur die storungstheorie wichtigen differential gleichung. Astron. Nach. 1882, 103, 211–222. [Google Scholar] [CrossRef]
- Nayfeh, A.H.; Mook, D.T. Nonlinear Oscillations; John Wiley & Sons: New York, NY, USA, 1995. [Google Scholar]
- Belendez, A.; Pascual, C.; Mendez, D.I.; Neipp, C. Solution of the relativistic (an) harmonic oscillator using the harmonic balance method. J. Sound Vib. 2007, 311, 1447–1456. [Google Scholar] [CrossRef]
- Garcia-Margallo, J.; Bejarano, J.D. A generalization of the method of harmonic balance. J. Sound Vib. 1987, 116, 591–595. [Google Scholar] [CrossRef]
- Stokes, A. On the approximation of nonlinear oscillations. J. Differ. Equ. 1972, 12, 535–538. [Google Scholar] [CrossRef]
- Garcia-Saldana, J.D.; Gasull, A. A theoretical basis for the harmonic balance method. J. Differ. Equ. 2013, 254, 67–80. [Google Scholar] [CrossRef]
- Alam, M.S. A modified and compact form of Krylov-Bogoliubov-Mitropolskii unified method for solving an nth order nonlinear differential equation. Int. J. Nonlinear Mech. 2004, 39, 1343–1357. [Google Scholar] [CrossRef]
- Sun, W.P.; Wu, B.S.; Lim, C.W. A modified lindstedt-poincare method for strongly mixed-parity nonlinear oscillators. Trans. ASME. J. Comput. Nonlinear Dyn. 2007, 2, 141–145. [Google Scholar] [CrossRef]
- Chung, K.W.; Chan, C.L.; Xu, Z.; Mahmoud, G.M. A perturbation-incremental method for strongly nonlinear autonomous oscillators with many degrees of freedom. Nonlinear Dyn. 2002, 28, 243–259. [Google Scholar] [CrossRef]
- Li, S.; Liao, S.J. An analytic approach to solve multiple solutions of a strongly nonlinear problem. Appl. Math. Comput. 2005, 169, 854–865. [Google Scholar] [CrossRef]
- Chen, S.H.; Cheung, Y.K. A modified Lindstedt-Poincare method for a strongly non-linear two degree-of-freedom system. J. Sound Vib. 1996, 193, 751–762. [Google Scholar] [CrossRef]
- Senator, M.; Bapat, C.N. A perturbation technique that works even when the nonlinearity is not small. J. Sound Vib. 1993, 164, 1–27. [Google Scholar] [CrossRef]
- Amore, P.; Aranda, A. Improved Lindstedt-Poincare method for the solution of the nonlinear problems. J. Sound Vib. 2005, 283, 1115–1136. [Google Scholar] [CrossRef]
- Belhaq, M.; Lakrad, F. Prediction of homoclinic bifurcation: The elliptic averaging method. Chaos Solitons Fractals. 2000, 11, 2251–2258. [Google Scholar] [CrossRef]
- Wang, W.; Zhang, Q.C.; Tian, R.L. Asymptotic solutions and bifurcation analysis of the strongly nonlinear oscillation system with two degrees of freedom. J. Vib. Shock 2008, 27, 130–133. [Google Scholar]
- He, J.H. Preliminary report on the energy balance for nonlinear oscillations. Mech. Res. Commun. 2002, 29, 107–111. [Google Scholar] [CrossRef]
- Davod, A.G.; Ganji, D.D.; Azami, R.; Babazadeh, H. Application of improved amplitude frequency formulation to nonlinear differential equation of motion equations. Mod. Phys. Lett. B 2009, 23, 3423–3436. [Google Scholar] [CrossRef]
- Khan, Y.; Mirzabeigy, A. Improved accuracy of He’s energy balance method for analysis of conservative nonlinear oscillator. Neural Comput. Appl. 2014, 25, 889–895. [Google Scholar] [CrossRef]
- Younesian, D.; Askari, H.; Saadatnia, Z.; Kalami, Y.M. Frequency analysis of strongly nonlinear generalized Duffing oscillators using He’s frequency-amplitude formulation and He’s energy balance method. Comput. Math. Appl. 2010, 59, 3222–3228. [Google Scholar] [CrossRef]
- Elias-Zuniga, A.; Martinez-Romero, O. Energy method to obtain approximate solutions of strongly nonlinear oscillators. Math. Probl. Eng. 2013, 2012, 620591. [Google Scholar]
- Chen, Y.Y.; Chen, S.H. Homoclinic and heteroclinic solutions of cubic strongly nonlinear autonomous oscillators by the hyperbolic perturbation method. Nonlinear Dyn. 2009, 58, 417–429. [Google Scholar] [CrossRef]
- Stoker, J.J. Nonlinear Vibrations in Mechanical and Electrical Systems; Interscience Publishers: New York, NY, USA, 1950. [Google Scholar]
- Mickens, R.E. Iteration procedure for determining approximate solutions to nonlinear oscillator equations. J. Vib. Shock 1987, 116, 185–187. [Google Scholar]
- Li, P.S.; Wu, B.S. An iteration approach to nonlinear oscillations of conservative single-degree-of-freedom systems. Acta Mech. 2004, 170, 69–75. [Google Scholar] [CrossRef]
- Garcia-Saldana, J.D.; Gasull, A. The period function and the harmonic balance method. Bull. Sci. Math. 2015, 139, 33–60. [Google Scholar] [CrossRef]
- Mickens, R.E. Turly Nonlinear Oscillations; World Scientific: Singapore, 1996. [Google Scholar]
- Lau, S.L.; Cheung, Y.K. Amplitude incremental variational principle for nonlinear vibration of elastic system. ASME J. Appl. Mech. 1981, 48, 959–964. [Google Scholar] [CrossRef]
- Wu, B.S.; Li, P.S. A method for obtaining approximate analytic periods for a class of nonlinear oscillators. Meccanica 2001, 36, 167–176. [Google Scholar] [CrossRef]
- Wu, B.S.; Li, P.S. A new approach to nonlinear oscillations. ASME J. Appl. Mech. 2001, 68, 951–952. [Google Scholar] [CrossRef]
- Yamgoué, S.B. On the harmonic balance with linearization for asymmetric single degree of freedom non-linear oscillators. Nonlinear Dyn. 2012, 69, 1051–1062. [Google Scholar] [CrossRef]
- Li, L. Energy method for computing periodic solutions of strongly nonlinear systems-autonomous systems. Nonlinear Dyn. 1996, 9, 223–247. [Google Scholar] [CrossRef]
- Lev, B.I.; Tymchyshyn, V.B.; Zagorodny, A.G. On certain properties of nonlinear oscillator with coordinate-dependent mass. Phys. Lett. A. 2017, 381, 3417–3423. [Google Scholar] [CrossRef]
- Wu, Y.; He, J.H. Homotopy perturbation method for nonlinear oscillators with coordinatedependent mass. Results Phys. 2018, 10, 270–271. [Google Scholar] [CrossRef]
- Ganji, D.D.; Esmaeilpour, M.; Soleimani, S. Approximate solutions to Van der Pol damped nonlinear oscillators by means of He’s energy balance method. Int. J. Comput. Math. 2010, 9, 2014–2023. [Google Scholar] [CrossRef]
- Ismail, G.M. An analytical technique for solving nonlinear oscillators of the motion of a rigid rod rocking bock and tapered beams. J. Appl. Comput. Mech. 2016, 2, 29–34. [Google Scholar]
- Ding, H.; Chen, L.Q. Galerkin methods for natural frequencies of high-speed axially moving beams. J. Sound Vib. 2010, 329, 3484–3494. [Google Scholar] [CrossRef]
- Ding, H.; Chen, L.Q.; Yang, S.P. Convergence of galerkin truncation for dynamic response of nite beams on nonlinear foundations under a moving load. J. Sound Vib. 2012, 331, 2426–2442. [Google Scholar] [CrossRef]
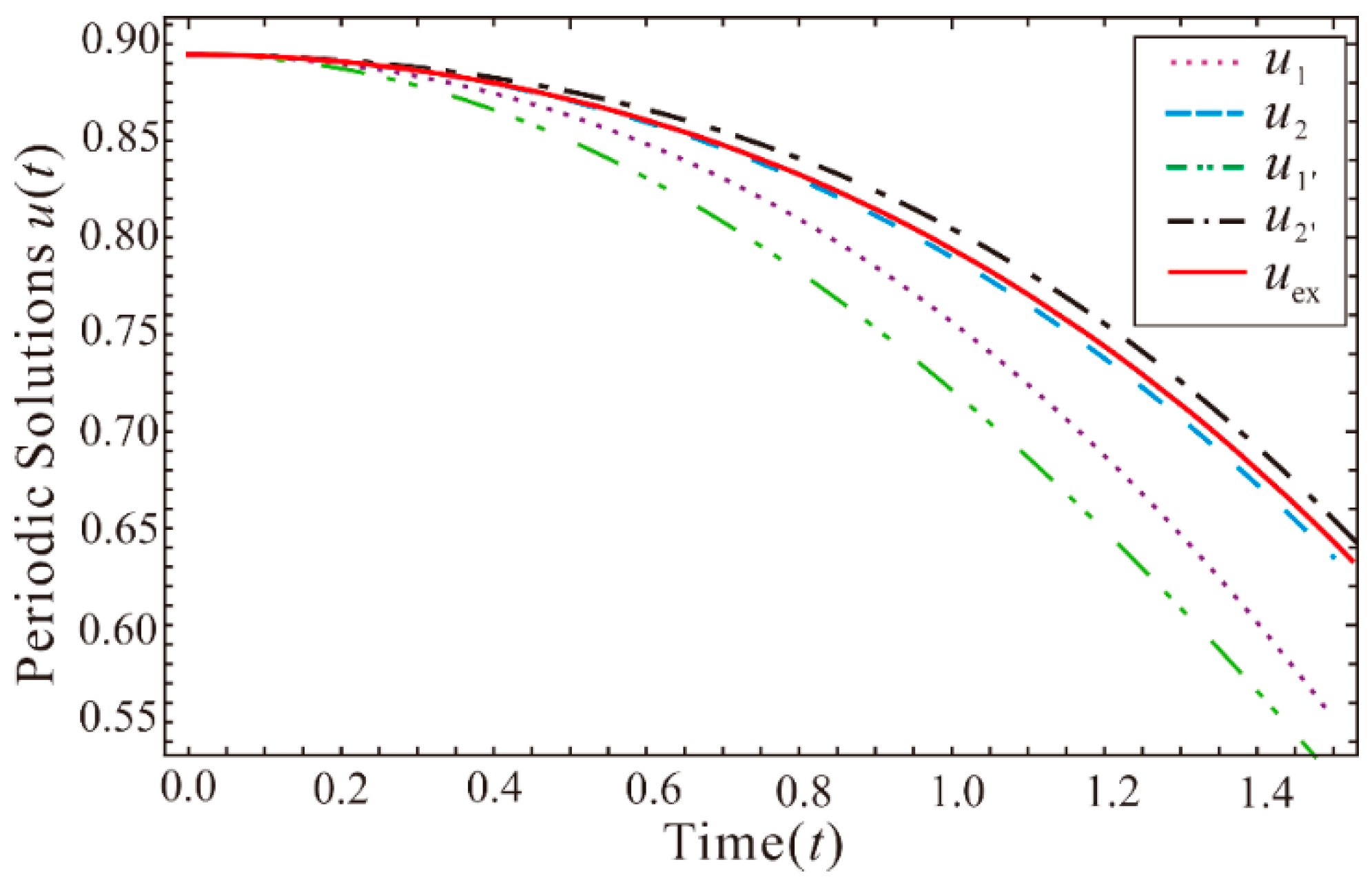
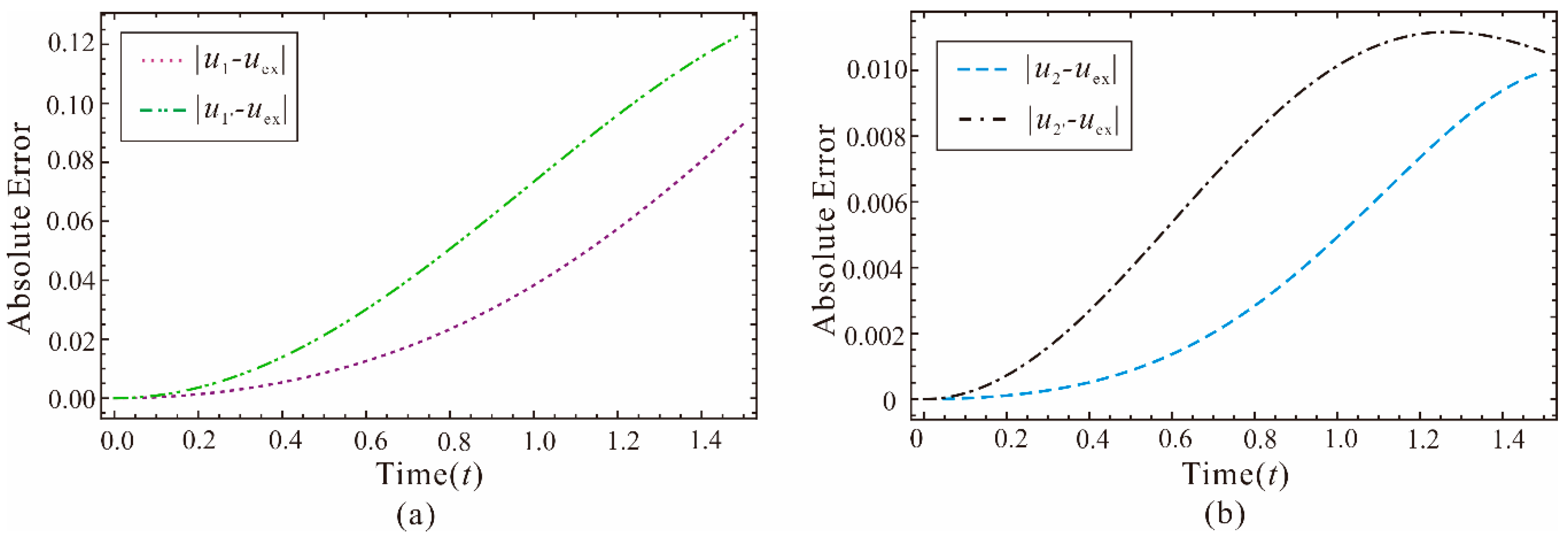



| −0.8 | 0.8939 | 1.0216 |
| −0.7 | 0.9481 | 0.9973 |
| −0.5 | 0.9862 | 0.9966 |
| −0.1 | 0.9997 | 0.9999 |
| 0.1 | 0.9998 | 0.9999 |
| 0.5 | 0.9975 | 0.9988 |
| 0.7 | 0.9960 | 0.9981 |
| 0.8 | 0.9953 | 0.9977 |
| 1 | 0.9939 | 0.9970 |
| 10 | 0.9751 | 0.9859 |
| T1 | T | |
|---|---|---|
| 0.1 | 0.7885 | 0.7861 |
| 0.2 | 0.7300 | 0.7491 |
| 0.3 | 0.6841 | 0.7169 |
| 0.4 | 0.6468 | 0.6886 |
| 0.5 | 0.6160 | 0.6633 |
| 0.6 | 0.5898 | 0.6406 |
| 0.7 | 0.5674 | 0.6201 |
| 0.8 | 0.5479 | 0.6015 |
| Groups | ||||||||
|---|---|---|---|---|---|---|---|---|
| G1 | 2 | −2 | −4 | 2 | −2 | 1 | −3 | |
| G2 | 2 | −2 | −4 | 2 | −2 | 2 | −3 | |
| G3 | 2 | −2 | −6 | 2 | −2 | 2 | −3 | |
| G4 | 2 | −2 | −6 | 2 | −2 | 4 | −3 |
| Groups | G1 | G2 | G3 | G4 |
|---|---|---|---|---|
| Index δ | 0.22 | 0.28 | 0.43 | 0.62 |
| Interval | weakly nonlinear | strongly nonlinear | ||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Z.; Wang, Y.; Wang, W.; Tian, R. Periodic Solution of the Strongly Nonlinear Asymmetry System with the Dynamic Frequency Method. Symmetry 2019, 11, 676. https://doi.org/10.3390/sym11050676
Zhang Z, Wang Y, Wang W, Tian R. Periodic Solution of the Strongly Nonlinear Asymmetry System with the Dynamic Frequency Method. Symmetry. 2019; 11(5):676. https://doi.org/10.3390/sym11050676
Chicago/Turabian StyleZhang, Zhiwei, Yingjie Wang, Wei Wang, and Ruilan Tian. 2019. "Periodic Solution of the Strongly Nonlinear Asymmetry System with the Dynamic Frequency Method" Symmetry 11, no. 5: 676. https://doi.org/10.3390/sym11050676
APA StyleZhang, Z., Wang, Y., Wang, W., & Tian, R. (2019). Periodic Solution of the Strongly Nonlinear Asymmetry System with the Dynamic Frequency Method. Symmetry, 11(5), 676. https://doi.org/10.3390/sym11050676





