Exact Solutions of the (2+1)-Dimensional Stochastic Chiral Nonlinear Schrödinger Equation
Abstract
:1. Introduction
2. The Exact Solutions of the 2D-CNLSE
2.1. Riccati–Bernoulli Sub-ODE Method
2.2. He’s Variational Principle Method
2.3. Sine–Cosine Method
3. Physical Interpretation
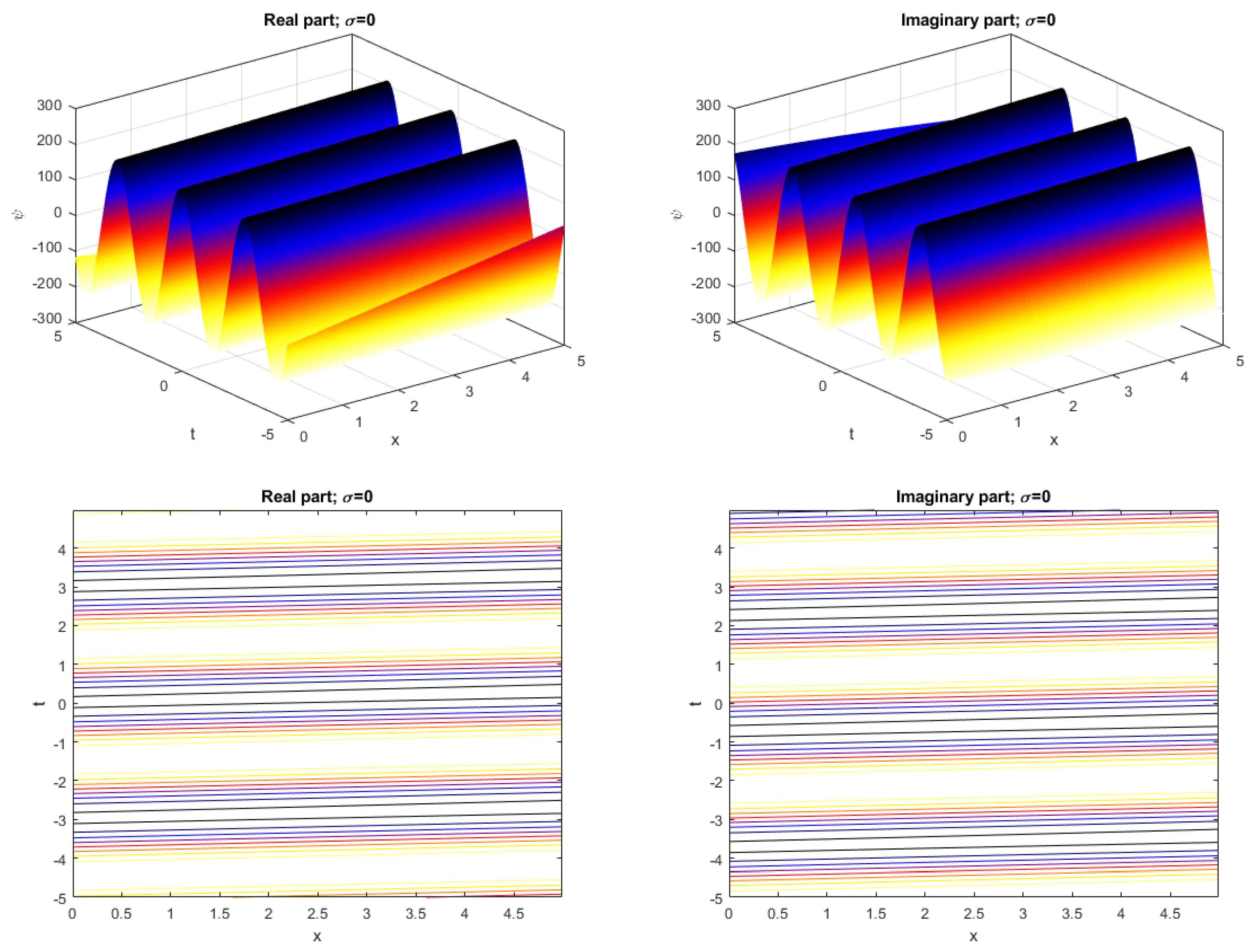
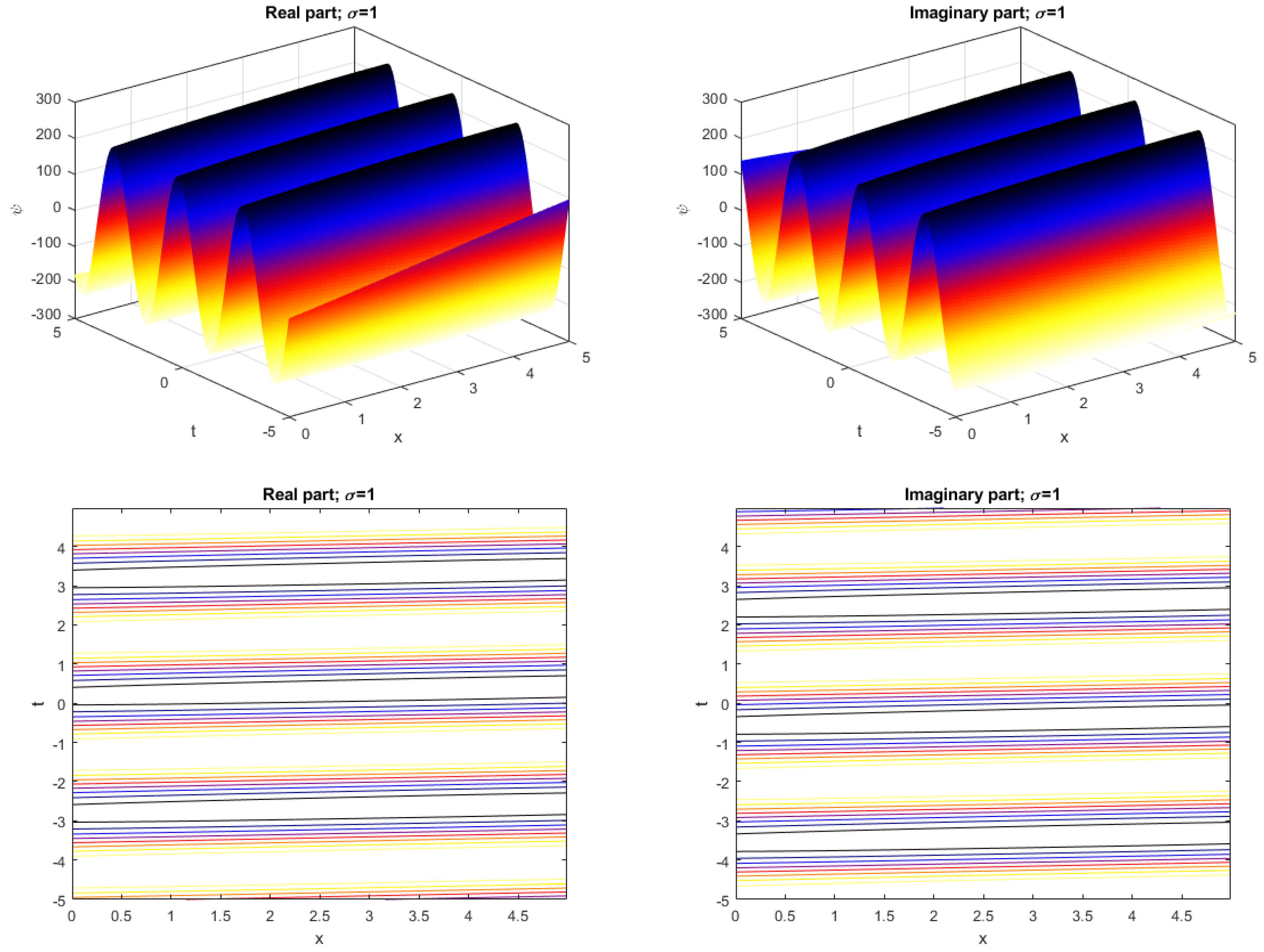
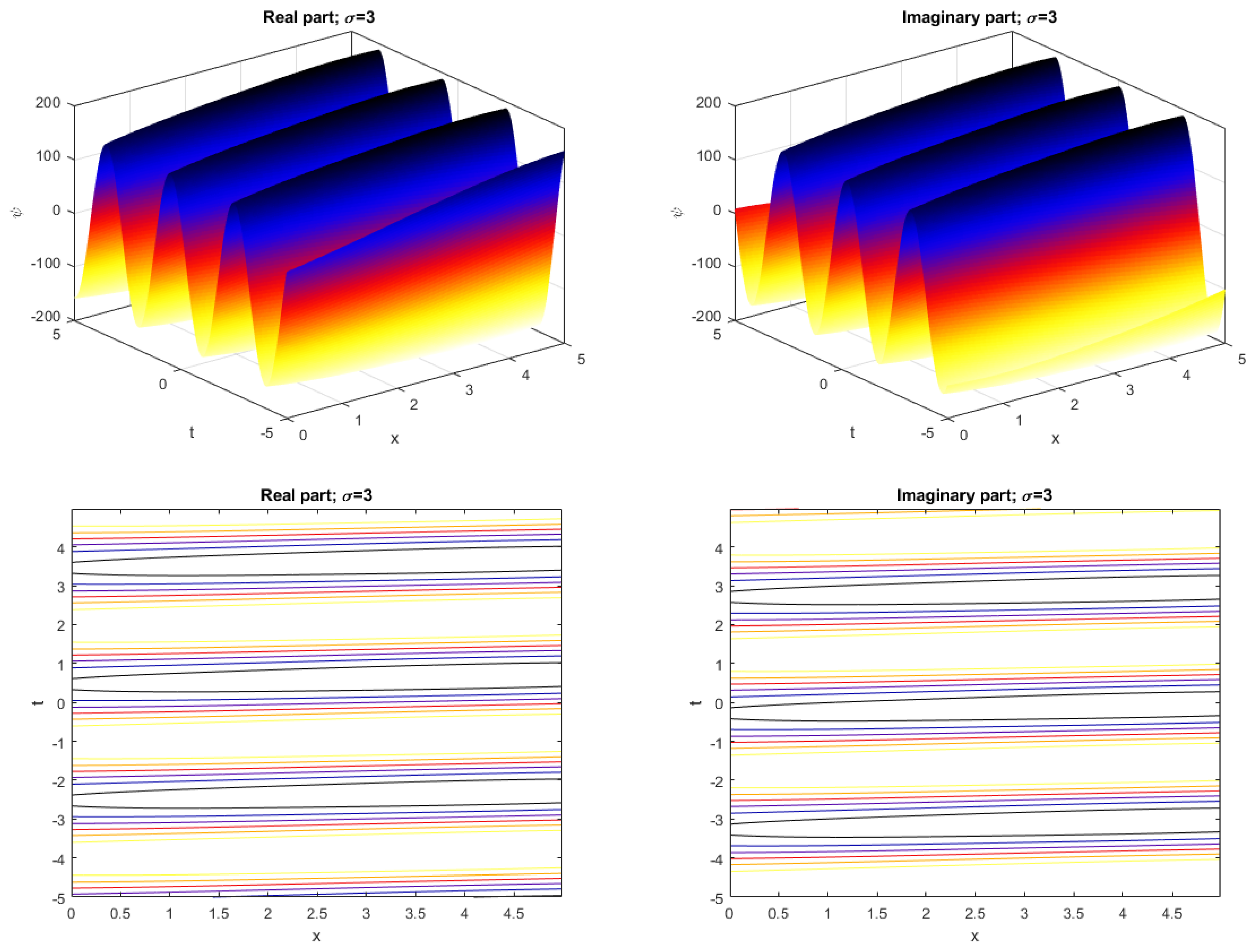
4. The Effect of Multiplicative Noise on the Solutions of the 2D-CNLSE
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Eslami, M. Soliton-like solutions for the coupled Schrödinger’s-Boussinesq equation. Opt. Int. J. Light Electron Opt. 2016, 126, 3987–3991. [Google Scholar] [CrossRef]
- Abdelrahman, M.A.E.; Hassan, S.Z.; Inc, M. The coupled nonlinear Schrödinger-type equations. Mod. Phys. Lett. 2020, 34, 2050078. [Google Scholar] [CrossRef]
- Abdelrahman, M.A.E.; Mohammed, W.W. The impact of multiplicative noise on the solution of the Chiral nonlinear Schrödinger equation. Phys. Scr. 2020, 95, 085222. [Google Scholar] [CrossRef]
- Malik, H.K.; Srivastava, R.; Kumar, S.; Singh, D. Small amplitude dust acoustic solitary wave in magnetized two ion temperature plasma. J. Taibah Univ. Sci. 2020, 14, 417–422. [Google Scholar] [CrossRef] [Green Version]
- Abdelwahed, H.G. Nonlinearity contributions on critical MKP equation. J. Taibah Univ. Sci. 2020, 14, 777–782. [Google Scholar] [CrossRef]
- Abouelregal, A.E. A novel model of non-local thermoelasticity with time derivatives of higher order. Math. Methods Appl. Sci. 2020, 43, 6746–6760. [Google Scholar] [CrossRef]
- Liu, S.; Zhou, Q.; Biswas, A.; Liu, W. Phase-shift controlling of three solitons in dispersion-decreasing fibers. Nonlinear Dyn. 2019, 98, 395–401. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Abdelrahman, M.A.E.; Inc, M.; Hamza, A.E.; Akinlar, M.A. Soliton solutions for system of ion sound and Langmuir waves. Opt. Quant. Elect. 2020, 52, 460. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Mohammed, W.W. Effects of nonlocal thermoelasticity on nanoscale beams based on couple stress theory. Math. Methods Appl. Sci. 2020, 1–17. [Google Scholar] [CrossRef]
- Wang, M.L.; Li, X.Z.; Zhang, J.L. The ()-expansion method and travelling wave solutions of nonlinear evolution equations in mathematical physics. Phys. Lett. A 2008, 372, 417–423. [Google Scholar] [CrossRef]
- Zhang, H. New application of the ()-expansion method. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 3220–3225. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Exact solutions to the double sinh-Gordon equation by the tanh method and a variable separated ODE. method. Comput. Math. Appl. 2005, 50, 1685–1696. [Google Scholar] [CrossRef] [Green Version]
- Wazwaz, A.M. A sine-cosine method for handling nonlinear wave equations. Math. Comput. Model. 2004, 40, 499–508. [Google Scholar] [CrossRef]
- He, J.H. An approximate solution technique depending on an artificial parameter: A special example. Commun. Nonlinear Sci. Numer. Simul. 1998, 3, 92–97. [Google Scholar] [CrossRef]
- Hirota, R. Exact solution of the Korteweg-de Vries equation for multiple collisions of solitons. Phys. Rev. Lett. 1971, 27, 1192–1194. [Google Scholar] [CrossRef]
- Ieda, J.; Uchiyama, M.; Wadati, M. Inverse scattering method for square matrix nonlinear Schrödinger equation under nonvanishing boundary conditions. J. Math. Phys. 2007, 48, 013507. [Google Scholar] [CrossRef] [Green Version]
- Petrov, E.Y.; Kudrin, A.V. Exact Axisymmetric Solutions of the Maxwell Equations in a Nonlinear Nondispersive Medium. Phys. Rev. Lett. 2010, 104, 190404. [Google Scholar] [CrossRef] [Green Version]
- Xiong, H.; Si, L.; Ding, C.; Lü, X.; Yang, X.; Wu, Y. Solutions of the cylindrical nonlinear Maxwell equations. Phys. Rev. E 2012, 85, 016602. [Google Scholar] [CrossRef] [Green Version]
- Mohammed, W.W. Approximate solution of the Kuramoto-Shivashinsky equation on an unbounded domain. Chin. Ann. Math. Ser. B 2018, 39, 145–162. [Google Scholar] [CrossRef]
- He, J.H. An new approach to nonlinear partial differential equations. Commun. Nonlinear Sci. Numer. Simul. 1997, 2, 230–235. [Google Scholar] [CrossRef]
- Yusufoglu, E. The variational iteration method for studying the Klein-Gordon equation. Appl. Math. Lett. 2008, 21, 669–674. [Google Scholar] [CrossRef] [Green Version]
- Yang, X.F.; Deng, Z.C.; Wei, Y. A Riccati-Bernoulli sub-ODE method for nonlinear partial differential equations and its application. Adv. Diff. Equ. 2015, 1, 117–133. [Google Scholar] [CrossRef] [Green Version]
- Abdelrahman, M.A.E.; Sohaly, M.A. Solitary waves for the nonlinear Schrödinger problem with the probability distribution function in stochastic input case. Eur. Phys. J. Plus 2017, 132, 339. [Google Scholar] [CrossRef]
- Wazwaz, A.M. The tanh method: Exact solutions of the Sine–Gordon and Sinh–Gordon equations. Appl. Math. Comput. 2005, 167, 1196–1210. [Google Scholar] [CrossRef]
- Yan, Z.L. Abunbant families of Jacobi elliptic function solutions of the-dimensional integrable Davey-Stewartson-type equation via a new method. Chaos Solitons Fractals 2003, 18, 299–309. [Google Scholar] [CrossRef]
- Khan, K.; Akbar, M.A. The exp(-(ξ))-expansion method for finding travelling wave solutions of Vakhnenko-Parkes equation. Int. J. Dyn. Syst. Differ. Equ. 2014, 5, 72–83. [Google Scholar] [CrossRef]
- Triki, H.; Bensalem, C.; Biswas, A.; Khan, S.; Zhou, Q.; Adesanya, S.; Moshokoa, S.P.; Belic, M. Self-similar optical solitons with continuous-wave background in a quadratic-cubic non-centrosymmetric waveguide. Opt. Commun. 2019, 437, 392–398. [Google Scholar] [CrossRef]
- Biswas, A. Conservation laws for optical solitons with anti-cubic and generalized anti-cubic nonlinearities. Optik 2019, 176, 198–201. [Google Scholar] [CrossRef]
- Wazwaz, A.M. The integrable time-dependent sine-Gordon with multiple optical kink solutions. Optik 2019, 182, 605–610. [Google Scholar] [CrossRef]
- Xiong, H.; Gan, J.; Wu, Y. Kuznetsov-Ma Soliton Dynamics Based on the Mechanical Effect of Light. Phys. Rev. Lett. 2017, 201, 153901. [Google Scholar] [CrossRef]
- Prévôt, C.; Röckner, M. A Concise Course on Stochastic Partial Differential Equations; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Shi, Y.; Pana, M.; Peng, D. Replicator dynamics and evolutionary game of social tolerance: The role of neutral agents. Econ. Lett. 2017, 159, 10–14. [Google Scholar] [CrossRef]
- Mohammed, W.W. Modulation Equation for the Stochastic Swift—Hohenberg Equation with Cubic and Quintic Nonlinearities on the Real Line. Mathematics 2019, 7, 1217. [Google Scholar] [CrossRef] [Green Version]
- Eslami, M. Trial solution technique to chiral nonlinear Schrödinger’s equation in (1+2)-dimensions. Nonlinear Dyn. 2016, 85, 813–816. [Google Scholar] [CrossRef]
- ⌀Ksendal, B. Stochastic Differential Equations: An Introduction with Applications, 6th ed.; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Raza, N.; Javid, A. Optical dark and dark-singular soliton solutions of (1 + 2)-dimensional chiral nonlinear Schrodinger’s equation. Waves Random Complex Media 2019, 29, 496. [Google Scholar] [CrossRef]
- Javid, A.; Raza, N. Chiral solitons of the (1+2)-dimensional nonlinear Schrodinger’s equation. Mod. Phys. Lett. 2019, 33, 1950401. [Google Scholar] [CrossRef]
- He, J.H. Variational principles for some nonlinear partial dikerential equations with variable coencients. Chaos Solitons Fractals 2004, 19, 847–851. [Google Scholar] [CrossRef]
- He, J.H. Some asymptotic methods for strongly nonlinear equations. Int. J. Mod. Phys. B 2006, 20, 1141–1199. [Google Scholar] [CrossRef] [Green Version]
- Ye, Y.H.; Mo, L.F. He’s variational method for the Benjamin–Bona–Mahony equation and the Kawahara equation. Comput. Math. Appl. 2009, 58, 2420–2422. [Google Scholar] [CrossRef] [Green Version]
- Scott, A.C. Encyclopedia of Nonlinear Science; Routledge: New York, NY, USA, 2005. [Google Scholar]
- Taylor, J.R. Optical Solitons: Theory and Experiment; Cambridge University Press: Cambridge, UK, 1992. [Google Scholar]
- Bailung, H.; Sharma, S.K.; Nakamura, Y. Observation of Peregrine Solitons in a Multicomponent Plasma with Negative Ions. Phys. Rev. Lett. 2011, 107, 255005. [Google Scholar] [CrossRef]
- Kibler, B.; Fatome, J.; Finot, C.; Millot, G.; Dias, F.; Genty, G.; Akhmediev, N.; Dudley, J.M. The Peregrine soliton in nonlinear fibre optics. Nat. Phys. 2010, 6, 790–795. [Google Scholar] [CrossRef]
- Chabchoub, A.; Hoffmann, N.P.; Akhmediev, N. Rogue wave observation in a water wave tank. Phys. Rev. Lett. 2011, 106, 204502. [Google Scholar] [CrossRef] [PubMed]
- Pathak, P.; Sharma, S.K.; Akamura, Y.N.; Bailung, H. Observation of ion acoustic multi-Peregrine solitons in multicomponent plasma with negative ions. Phys. Lett. A 2017, 381, 4011–4018. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Albosaily, S.; Mohammed, W.W.; Aiyashi, M.A.; Abdelrahman, M.A.E. Exact Solutions of the (2+1)-Dimensional Stochastic Chiral Nonlinear Schrödinger Equation. Symmetry 2020, 12, 1874. https://doi.org/10.3390/sym12111874
Albosaily S, Mohammed WW, Aiyashi MA, Abdelrahman MAE. Exact Solutions of the (2+1)-Dimensional Stochastic Chiral Nonlinear Schrödinger Equation. Symmetry. 2020; 12(11):1874. https://doi.org/10.3390/sym12111874
Chicago/Turabian StyleAlbosaily, Sahar, Wael W. Mohammed, Mohammed A. Aiyashi, and Mahmoud A. E. Abdelrahman. 2020. "Exact Solutions of the (2+1)-Dimensional Stochastic Chiral Nonlinear Schrödinger Equation" Symmetry 12, no. 11: 1874. https://doi.org/10.3390/sym12111874
APA StyleAlbosaily, S., Mohammed, W. W., Aiyashi, M. A., & Abdelrahman, M. A. E. (2020). Exact Solutions of the (2+1)-Dimensional Stochastic Chiral Nonlinear Schrödinger Equation. Symmetry, 12(11), 1874. https://doi.org/10.3390/sym12111874




