The Linguistic Interval-Valued Intuitionistic Fuzzy Aggregation Operators Based on Extended Hamacher T-Norm and S-Norm and Their Application
Abstract
:1. Introduction
2. Preliminaries
- (1)
- if, then ;
- (2)
- if, then ;
- (3)
- the negation ofis defined as .
- (1)
- if, then ;
- (2)
- if , , then .
- (1)
- ;
- (2)
- ;
- (3)
- ;
- (4)
- .
- (1)
- the linguistic interval-valued intuitionistic fuzzy weighted average (LIVIFWA) operator is a mapping given by
- (2)
- the linguistic interval-valued intuitionistic fuzzy weighted geometric (LIVIFWG) operator is a mappinggiven bywhereis the weight vector, and .
Extended Hamacher T-Norm and S-Norm
- (1)
- ifsatisfies: (1),; (2),,, thenis called an extended aggregation function.
- (2)
- ifsatisfies, thenandare dual aggregation function with.
- (1)
- Commutativity:;
- (2)
- Associativity:;
- (3)
- Monotonicity:;
- (4)
- Neutral element:.
- (1)
- Commutativity:;
- (2)
- Associativity:;
- (3)
- Monotonicity:;
- (4)
- Neutral element:.
- (1)
- ;
- (2)
3. Linguistic Interval-Valued Intuitionistic Fuzzy Hamacher Aggregation Operators
3.1. Linguistic Interval-Valued Intuitionistic Fuzzy Hamacher Operational Laws
- (1)
- ;
- (2)
- ;
- (3)
- ;
- (4)
- .
- (1)
- ;
- (2)
- ;
- (3)
- ;
- (4)
- ;
- (5)
- ;
- (6)
- .
3.2. Linguistic Interval-Valued Intuitionistic Fuzzy Hamacher Aggregation Operators
3.2.1. LIVIFHWA Operator and LIVIFHWG Operator
3.2.2. Some Properties of Two LIVIFH Operators
3.3. Relationship between Operator and Parameter
3.3.1. Limiting Cases of LIVIFH Operators
- (1)
- ;
- (2)
- ;
- (3)
- ;
- (4)
- ;
- (5)
- ;
- (6)
- ;
- (7)
- ;
- (8)
- .
- (1)
- According to Definition 17 and L’Hospital’s rule,
- (2)
- According to Theorem 6,
- (3)
- According to Definition 17,
- (4)
- According to Definition 17,□
- (1)
- When, the LIVIFHWA operator degenerates into the Harmonic weighted average (LIVIFHarWA) operator:
- (2)
- When, the LIVIFHWG operator degenerates into the Harmonic weighted geometric (LIVIFHarWG) operator:
- (3)
- When, the LIVIFHWA operator degenerates into the Algebraic weighted average (LIVIFAWA) operator:
- (4)
- When, the LIVIFHWG operator degenerates into the Algebraic weighted geometric (LIVIFAWG) operator:
- (5)
- When, the LIVIFHWA operator degenerates into the Einstein weighted average (LIVIFEWA) operator:
- (6)
- When, the LIVIFHWG operator degenerates into the Einstein weighted geometric (LIVIFEWG) operator:
- (7)
- When, the LIVIFHWA operator degenerates into the Symmetric weighted average (LIVIFSWA) operator:
- (8)
- When, the LIVIFHWG operator degenerates into the Symmetric weighted geometric (LIVIFSWG) operator:
3.3.2. Monotonicity of Operators with Respect to Their Parameters
- (1)
- The LIVIFHWA operator decreases with increasing parameter;
- (2)
- The LIVIFHWG operator increases with increasing parameter.
4. Multiple Attributes Decision-Making Approach Based on the LIVIFH Operators and Its Application
4.1. Supplier Selection Problem
- (1)
- Supplier alternatives: Let be the set of supplier alternatives, where denotes the th supplier alternative, . The best supplier will be selected from the set of alternatives.
- (2)
- Evaluation attributes: is the set of attributes for the supplier alternatives, where is the th attribute, .
- (3)
- Group of experts: Let be the experts from different research areas, where is the th expert,. is the weight vector of experts, where is the weight of the expert , and satisfies and for . The decision maker aims to coordinate the insights of different experts and select the best one out of supplier alternatives measured on attributes.
- (4)
- Decision-making information matrixes: The experts are requested to express their preferences by using LIVIFNs generated by a discrete linguistic set , which described in Definition 1. For an alternative with respect to an attribute , an expert provides his/her assessments by using LIVIFNs . By collecting each attribute’s evaluation information from expert , the decision-making information matrix is obtained. is continuous virtual linguistic term set with respect to , and ,, , , .
4.2. Decision-Making Method for Solving Supplier Selection Problem
4.3. Case Study
4.3.1. Illustrative Example
4.3.2. Parameter Analysis
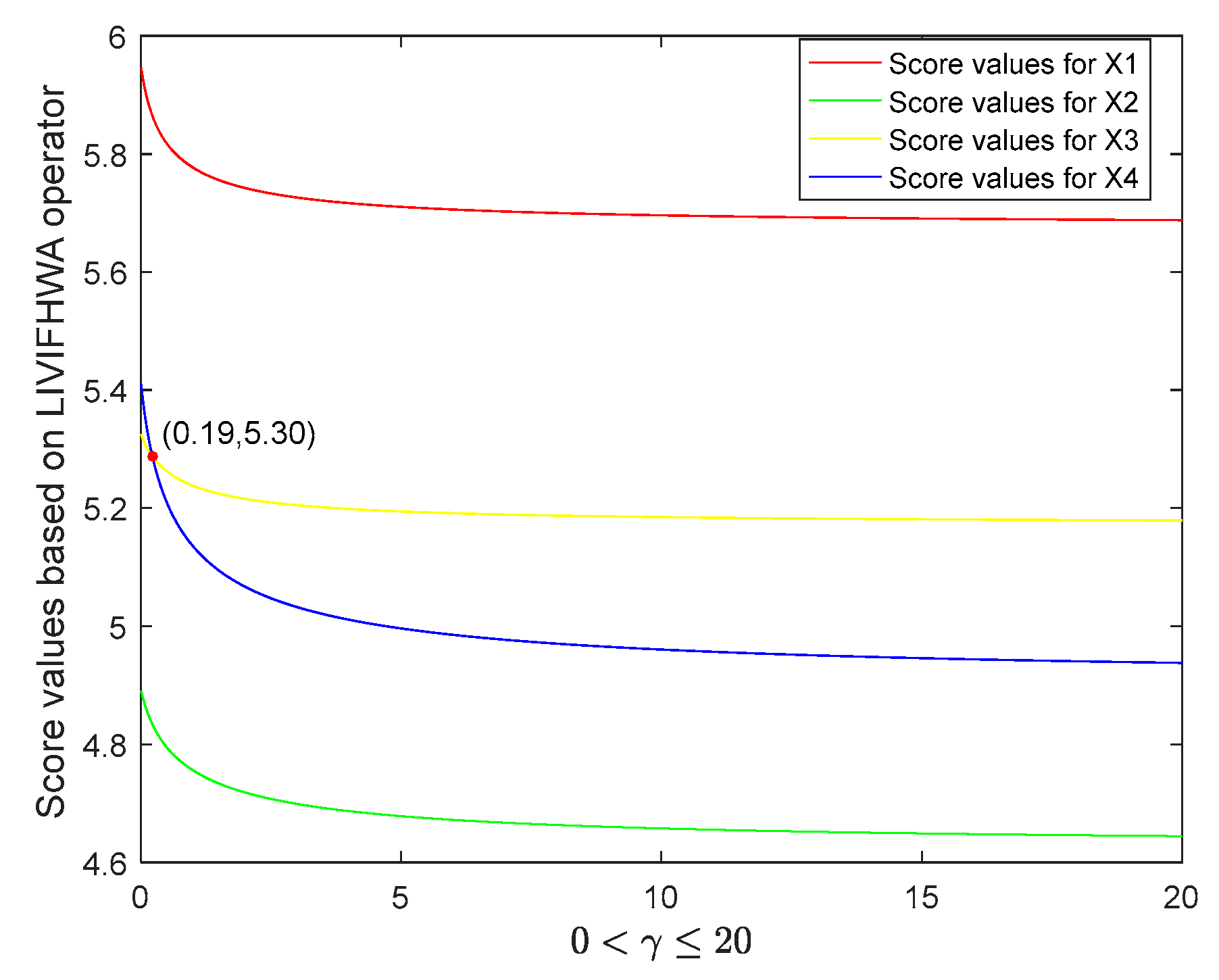
- (1)
- decreased with increasing parameter. This is consistent with the Theorem 10 (1);
- (2)
- When , the rank of alternatives was ; when , the rank of alternatives was , which means the rank of alternatives and switched. This is because the decreasing in score value of was relatively smaller compared with that of . Alternative was always the optimal alternative.
- (1)
- increased with increasing parameter . This is consistent with the Theorem 10 (2);
- (2)
- The rank of alternative was always .
- (1)
- The difference between score values of LIVIFHWA operator and LIVIFHarWA operator increased with increasing parameter, while the difference between score values of LIVIFHWA operator and the LIVIFSWA operator decreased.
- (2)
- The difference between score values of LIVIFHWG operator and LIVIFHarWG operator increased with increasing parameter, while the difference between score values of LIVIFHWG operator and LIVIFSWA operator decreased.To summarize, the relationships between the score values of these five operators were:
4.3.3. Comparative Analysis
- (1)
- The adjustable parameter could reflect the DM’s attitude.The parameter analysis has manifested that the LIVIFH aggregation operators were capable of reflecting the DM’s preferences by determining the appropriate values of the adjustable parameter .
- (2)
- The expansion of the domain for evaluation.The WIVLIFMSM operator could deal with MADM problems with LIVIF inputs, but not MAGDM problems. While the LIVAIFWA/LIVAIFWG operators are capable of dealing with MAGDM problems with LIVIF inputs, but they were merely degenerate cases of the proposed LIVIFHWA and LIVIFHWG operators when the adjustable parameter .
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zadeh, L. The concept of a linguistic variable and its application to approximate reasoning—I. Inf. Sci. 1975, 8, 199–249. [Google Scholar] [CrossRef]
- Martinez, L.; Herrera, F. A 2-tuple fuzzy linguistic representation model for computing with words. IEEE Trans. Fuzzy Syst. 2000, 8, 746–752. [Google Scholar] [CrossRef] [Green Version]
- Xu, Z. Deviation measures of linguistic preference relations in group decision making. Omega 2005, 33, 249–254. [Google Scholar] [CrossRef]
- Wang, J.-H.; Hao, J. A new version of 2-tuple fuzzy linguistic representation model for computing with words. IEEE Trans. Fuzzy Syst. 2006, 14, 435–445. [Google Scholar] [CrossRef]
- Torra, V. Hesitant fuzzy sets. Int. J. Intell. Syst. 2010, 25, 529–539. [Google Scholar] [CrossRef]
- Rodríguez, R.M.; Martinez, L.; Herrera, F. Hesitant Fuzzy Linguistic Term Sets for Decision Making. IEEE Trans. Fuzzy Syst. 2011, 20, 109–119. [Google Scholar] [CrossRef]
- Zhang, G.; Dong, Y.; Xu, Y. Consistency and consensus measures for linguistic preference relations based on distribution assessments. Inf. Fusion 2014, 17, 46–55. [Google Scholar] [CrossRef]
- Wu, Z.; Xu, J. Possibility Distribution-Based Approach for MAGDM with Hesitant Fuzzy Linguistic Information. IEEE Trans. Cybern. 2015, 46, 694–705. [Google Scholar] [CrossRef]
- Chen, Z.-S.; Chin, K.-S.; Li, Y.-L.; Yang, Y. Proportional hesitant fuzzy linguistic term set for multiple criteria group decision making. Inf. Sci. 2016, 357, 61–87. [Google Scholar] [CrossRef]
- Pang, Q.; Wang, H.; Xu, Z. Probabilistic linguistic term sets in multi-attribute group decision making. Inf. Sci. 2016, 369, 128–143. [Google Scholar] [CrossRef]
- Zhang, Z.; Guo, C.H.; Martínez, L. Managing multi-granular linguistic distribution assessments in large-scale multi-attribute group decision-making. IEEE Trans. Syst. Man Cybern. Syst. 2017, 47, 3063–3076. [Google Scholar] [CrossRef] [Green Version]
- Zhang, S.T.; Liu, X.D.; Zhu, J.J.; Wang, Z.Y. Adaptive consensus model with hesitant fuzzy linguistic information considering individual cumulative consensus contribution. Control Decis. 2019. [Google Scholar] [CrossRef]
- Huang, X.J.; Peng, W.S. Correlation coefficient for linguistic hesitant fuzzy sets and its application in decision-making. Control Decis. 2020, 35, 1211–1216. [Google Scholar]
- Zhou, J.; Xiao, F.; Du, N.; Yan, X.Y.; Sun, L.J. Linguistic multi-criteria decision-making method based on emotion perception. Control Decis. 2019. [Google Scholar] [CrossRef]
- Liao, H.C.; Yang, Z.; Xu, Z.S.; Gu, X. A hesitant fuzzy linguistic PROMETHEE method and its application in Sichuan liquor brand evaluation. Control Decis. 2019, 34, 2727–2736. [Google Scholar] [CrossRef]
- Wei, C.P.; Ma, J. Consensus model for hesitant fuzzy linguistic group decision-making. Control Decis. 2018, 33, 275–281. [Google Scholar]
- Wu, H.; Ren, P.; Xu, Z. Hesitant Fuzzy Linguistic Consensus Model Based on Trust-Recommendation Mechanism for Hospital Expert Consultation. IEEE Trans. Fuzzy Syst. 2019, 27, 2227–2241. [Google Scholar] [CrossRef]
- Yin, N.H.; Wang, Z.Q.; Pu, Y. Linguistic information-based decision-making method for responding public construction emergency. China Saf. Sci. J. 2013, 23, 161–165. [Google Scholar]
- Chang, J.P.; Chen, Z.S.; Zhou, G.H. Emergency decision-making considering group conflict and evaluation indexes correlation under the uncertain environment. Comput. Integr. Manuf. Syst. 2018, 24, 228–240. [Google Scholar]
- Atanassov, K.T. Intuitionistic fuzzy sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Zhang, H. Linguistic Intuitionistic Fuzzy Sets and Application in MAGDM. J. Appl. Math. 2014, 2014, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Chen, Z.; Liu, P.; Pei, Z. An approach to multiple attribute group decision making based on linguistic intuitionistic fuzzy numbers. Int. J. Comput. Intell. Syst. 2015, 8, 747–760. [Google Scholar] [CrossRef] [Green Version]
- Liu, P.; Qin, X. Power average operators of linguistic intuitionistic fuzzy numbers and their application to multiple-attribute decision making. J. Intell. Fuzzy Syst. 2017, 32, 1029–1043. [Google Scholar] [CrossRef]
- Peng, H.-G.; Wang, J.-Q.; Cheng, P. A linguistic intuitionistic multi-criteria decision-making method based on the Frank Heronian mean operator and its application in evaluating coal mine safety. Int. J. Mach. Learn. Cybern. 2017, 9, 1053–1068. [Google Scholar] [CrossRef]
- Liu, P.; Liu, J.; Merigó, J.M. Partitioned Heronian means based on linguistic intuitionistic fuzzy numbers for dealing with multi-attribute group decision making. Appl. Soft Comput. 2018, 62, 395–422. [Google Scholar] [CrossRef]
- Liu, P.; Liu, J. A Multiple Attribute Group Decision-making Method Based on the Partitioned Bonferroni Mean of Linguistic Intuitionistic Fuzzy Numbers. Cogn. Comput. 2019, 12, 49–70. [Google Scholar] [CrossRef]
- Liu, P.; Liu, X. Linguistic Intuitionistic Fuzzy Hamy Mean Operators and Their Application to Multiple-Attribute Group Decision Making. IEEE Access 2019, 7, 127728–127744. [Google Scholar] [CrossRef]
- Yuan, R.; Tang, J.; Meng, F. Linguistic Intuitionistic Fuzzy Group Decision Making Based on Aggregation Operators. Int. J. Fuzzy Syst. 2018, 21, 407–420. [Google Scholar] [CrossRef]
- Xu, Z. Uncertain linguistic aggregation operators based approach to multiple attribute group decision making under uncertain linguistic environment. Inf. Sci. 2004, 168, 171–184. [Google Scholar] [CrossRef]
- Liu, P.; Qin, X. A New Decision-Making Method Based on Interval-Valued Linguistic Intuitionistic Fuzzy Information. Cogn. Comput. 2018, 11, 125–144. [Google Scholar] [CrossRef]
- Garg, H.; Kumar, K. Linguistic Interval-Valued Atanassov Intuitionistic Fuzzy Sets and Their Applications to Group Decision Making Problems. IEEE Trans. Fuzzy Syst. 2019, 27, 2302–2311. [Google Scholar] [CrossRef]
- Hamacher, H. Über Logische Verknüpfungen Unscharfer Aussagen und deren Zugehörige Bewertungsfunktionen; working paper No. 75/14, Lehrstuhl fiir Unternehmensforschung; RWTH Aachen University: Aachen, Germany, 1975. [Google Scholar]
- Xia, M.; Xu, Z.; Zhu, B. Some issues on intuitionistic fuzzy aggregation operators based on Archimedean t-conorm and t-norm. Knowl. Based Syst. 2012, 31, 78–88. [Google Scholar] [CrossRef]
- Tan, C.; Yi, W.; Chen, X. Hesitant fuzzy Hamacher aggregation operators for multicriteria decision making. Appl. Soft Comput. 2015, 26, 325–349. [Google Scholar] [CrossRef]
- Wei, G.; Lu, M.; Tang, X.; Wei, Y. Pythagorean hesitant fuzzy Hamacher aggregation operators and their application to multiple attribute decision making. Int. J. Intell. Syst. 2018, 33, 1197–1233. [Google Scholar] [CrossRef]
- Tao, Z.; Liu, X.; Chen, H.; Zhou, L. Using new version of extended t-norms and s-norms for aggregating interval linguistic labels. IEEE Trans. Syst. Man Cybern. Syst. 2017, 47, 3284–3298. [Google Scholar] [CrossRef]
- Xu, Z. A method based on linguistic aggregation operators for group decision making with linguistic preference relations. Inf. Sci. 2004, 166, 19–30. [Google Scholar] [CrossRef]
- Klir, G.; Yuan, B. Fuzzy Sets and Fuzzy Logic: Theory and Applications; Prentice-Hall: Upper Saddle River, NJ, USA, 1995. [Google Scholar]
- Ayhan, M.; Kilic, H.S. A two stage approach for supplier selection problem in multi-item/multi-supplier environment with quantity discounts. Comput. Ind. Eng. 2015, 85, 1–12. [Google Scholar] [CrossRef]



| Expert | Alternative | Attribute | |||
|---|---|---|---|---|---|
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, W.-B.; Shuai, B.; Zhang, S.-H. The Linguistic Interval-Valued Intuitionistic Fuzzy Aggregation Operators Based on Extended Hamacher T-Norm and S-Norm and Their Application. Symmetry 2020, 12, 668. https://doi.org/10.3390/sym12040668
Zhu W-B, Shuai B, Zhang S-H. The Linguistic Interval-Valued Intuitionistic Fuzzy Aggregation Operators Based on Extended Hamacher T-Norm and S-Norm and Their Application. Symmetry. 2020; 12(4):668. https://doi.org/10.3390/sym12040668
Chicago/Turabian StyleZhu, Wei-Bo, Bin Shuai, and Shi-Hang Zhang. 2020. "The Linguistic Interval-Valued Intuitionistic Fuzzy Aggregation Operators Based on Extended Hamacher T-Norm and S-Norm and Their Application" Symmetry 12, no. 4: 668. https://doi.org/10.3390/sym12040668
APA StyleZhu, W.-B., Shuai, B., & Zhang, S.-H. (2020). The Linguistic Interval-Valued Intuitionistic Fuzzy Aggregation Operators Based on Extended Hamacher T-Norm and S-Norm and Their Application. Symmetry, 12(4), 668. https://doi.org/10.3390/sym12040668





