First-Principles Calculations of High-Pressure Physical Properties of Ti0.5Ta0.5 Alloy
Abstract
1. Introduction
2. Theoretical Methodology
3. Analysis and Discussions
3.1. Structure and Stability
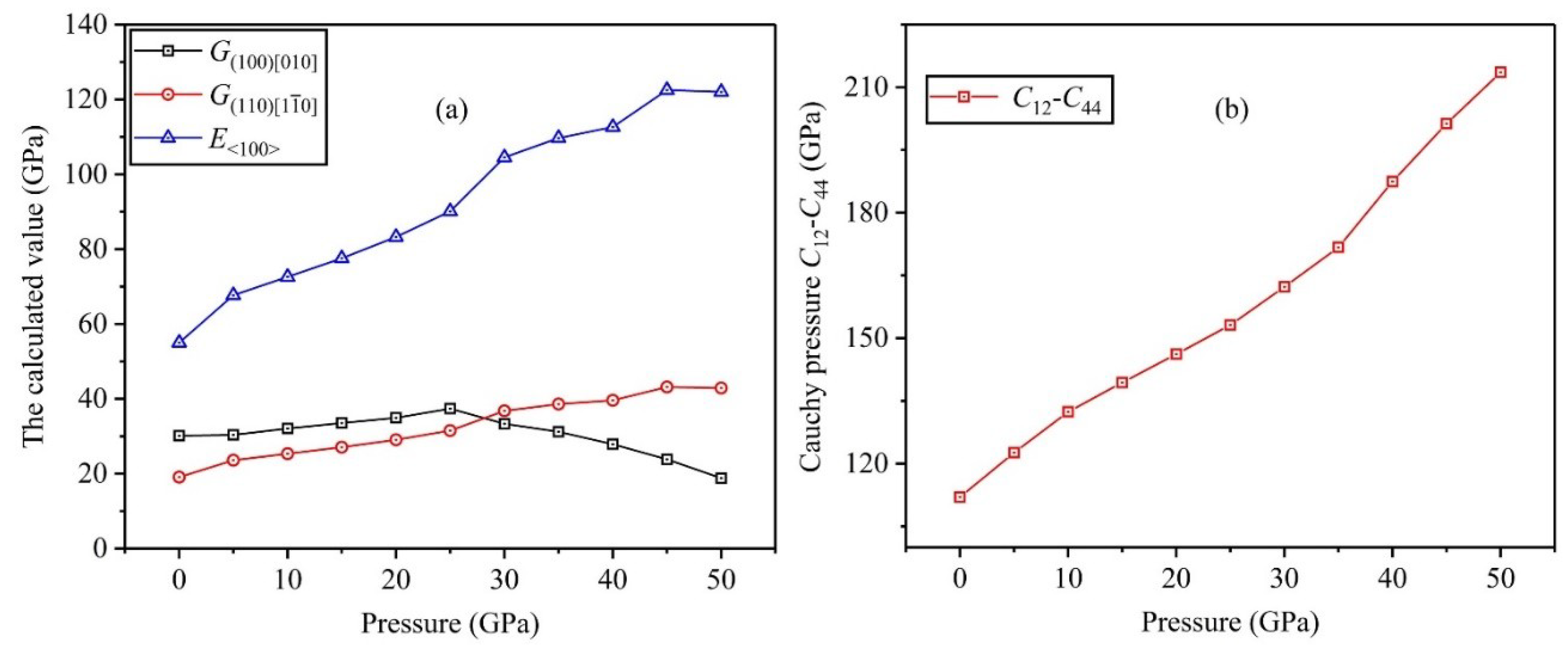
3.2. Mechanical Properties
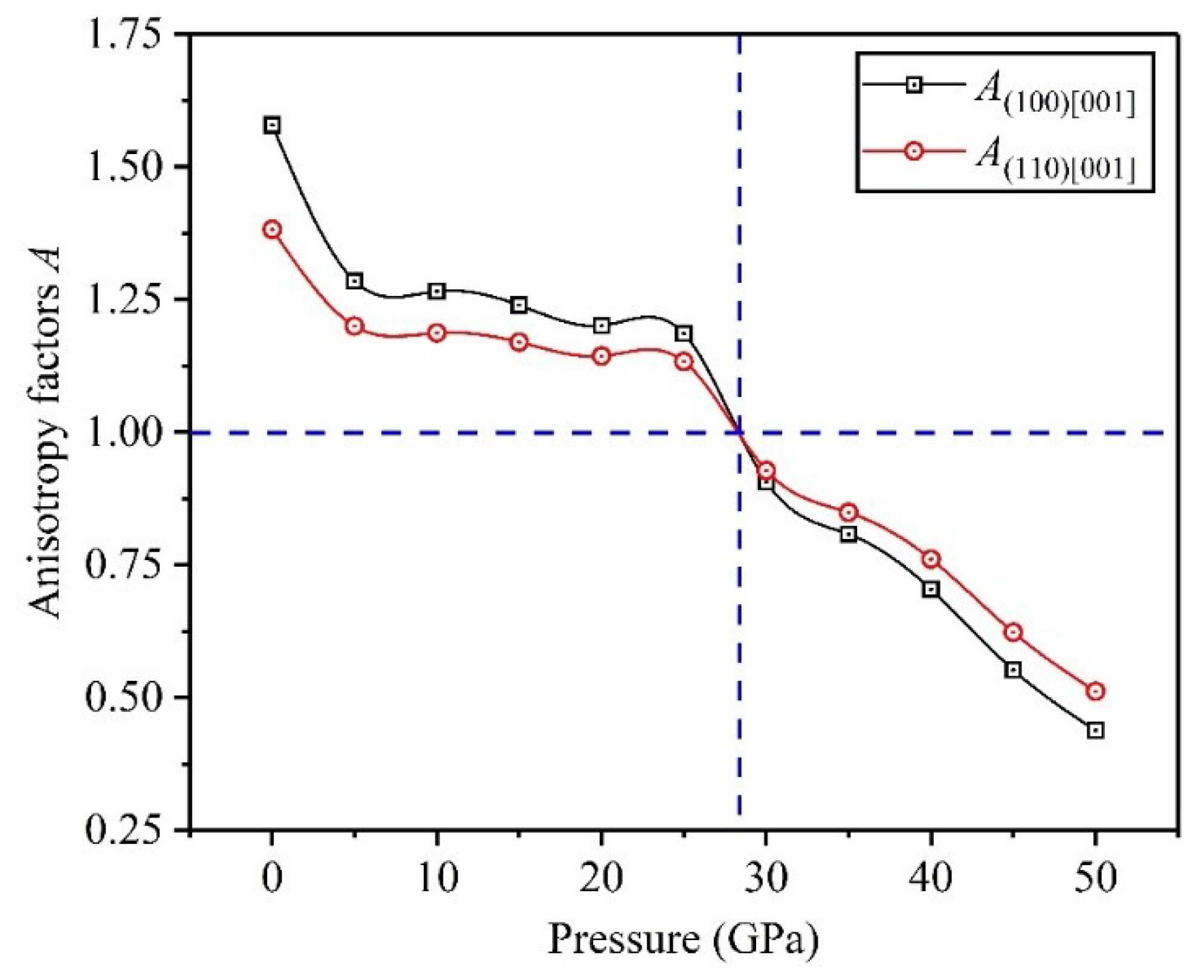
3.3. Anisotropy
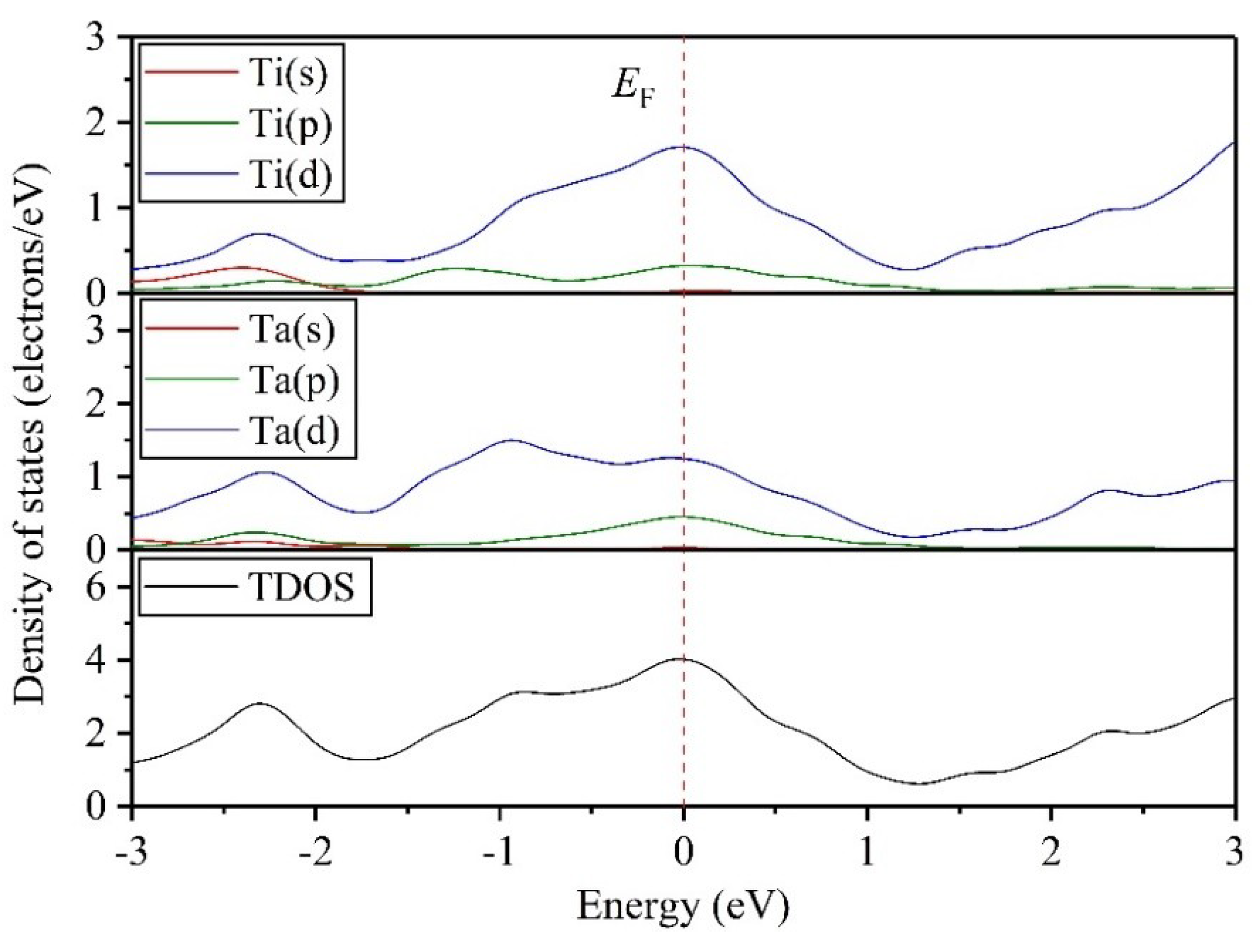
3.4. Electronic Properties
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Chen, H.C.; Pinkerton, A.J.; Li, L. Fibre laser welding of dissimilar alloys of Ti-6Al-4V and inconel 718 for aerospace applications. Int. J. Adv. Manuf. Technol. 2011, 52, 977–987. [Google Scholar] [CrossRef]
- Pang, J.J.; Blackwood, D.J. Corrosion of titanium alloys in high temperature near anaerobic seawater. Corros. Sci. 2016, 105, 17–24. [Google Scholar] [CrossRef]
- Massicot, B.; Latroche, M.; Joubert, J.M. Hydrogenation properties of Fe-Ti-V bcc alloys. J. Alloys Compd. 2011, 509, 372–379. [Google Scholar] [CrossRef]
- Atapour, M.; Pilchak, A.L.; Frankel, G.S.; Williams, J.C. Corrosion behavior of β titanium alloys for biomedical applications. Mat. Sci. Eng. C 2011, 31, 885–891. [Google Scholar] [CrossRef]
- Banerjee, D.; Williams, J.C. Perspective on titanium science and technology. Acta Mater. 2013, 61, 844–879. [Google Scholar] [CrossRef]
- Souza, K.A.D.; Robin, A. Preparation and characterization of Ti-Ta alloys for application in corrosive media. Mater. Lett. 2003, 57, 3010–3016. [Google Scholar] [CrossRef]
- Liu, Y.; Li, K.Y.; Wu, H.; Song, M.; Wang, W.; Li, N.F.; Tang, H.P. Synthesis of Ti-Ta alloys with dual structure by incomplete diffusion between elemental powders. J. Mech. Behav. Biomed. Mater. 2015, 51, 302–312. [Google Scholar] [CrossRef]
- Yan, L.M.; Yuan, Y.W.; Ouyang, L.J.; Li, H.; Mirzasadeghi, A.; Li, L. Improved mechanical properties of the new Ti-15Ta-xZr alloys fabricated by selective laser melting for biomedical application. J. Alloys Compd. 2016, 688, 156–162. [Google Scholar] [CrossRef]
- Zhou, Y.L.; Niinomi, M.; Akahori, T. Effects of Ta content on Young’s modulus and tensile properties of binary Ti-Ta alloys for biomedical applications. Mater. Sci. Eng. A 2004, 371, 283–290. [Google Scholar] [CrossRef]
- Yin, J.O.; Chen, G.; Zhao, S.Y.; Ge, Y.; Li, Z.F.; Yang, P.J.; Han, W.Z.; Wang, J.; Tang, H.P.; Cao, P. Microstructural characterization and properties of Ti-28Ta at.% powders produced by plasma rotating electrode process. J. Alloys Compd. 2017, 713, 222–228. [Google Scholar] [CrossRef]
- Dercz, G.; Matula, I.; Zubko, M.; Kesik, A.K.; Maszybrocka, J.; Simka, W.; Dercz, J.; Swiec, P.; Jendrzejewska, I. Synthesis of porous Ti-50Ta alloy by powder metallurgy. Mater. Charact. 2018, 142, 124–136. [Google Scholar] [CrossRef]
- Sing, S.L.; Yeong, W.Y.; Wiria, F.E. Selective laser melting of titanium alloy with 50 wt% tantalum: Microstructure and mechanical properties. J. Alloys Compd. 2016, 660, 461–470. [Google Scholar] [CrossRef]
- Behera, M.; Raju, S.; Panneerselvam, G.; Rangachari, M.; Saibaba, S. High temperature drop calorimetry measurements of enthalpy increment in Ti-xTa (x = 5, 10, 15, 20 mass%) alloys. J. Phys. Chem. Solids 2014, 75, 283–295. [Google Scholar] [CrossRef]
- Kadletz, P.M.; Motemani, Y.; Iannotta, J.; Salomon, S.; Khare, C.; Grossmann, L.; Maier, H.J.; Ludwig, A.; Schmahl, W.W. Crystallographic structure analysis of a Ti-Ta thin film materials library fabricated by combinatorial magnetron sputtering. ACS Comb. Sci. 2018, 20, 137–150. [Google Scholar] [CrossRef]
- Ojha, A.; Sehitoglu, H. Critical stress for the bcc-hcp martensite nucleation in Ti-6.25at.% Ta and Ti-6.25at.%Nb alloys. Comp. Mater. Sci. 2016, 111, 157–162. [Google Scholar] [CrossRef]
- Jha, H.; Hahn, R.; Schmuki, P. Ultrafast oxide nanotube formation on TiNb, TiZr and TiTa alloys by rapid breakdown anodization. Electrochim. Acta 2010, 55, 8883–8887. [Google Scholar] [CrossRef]
- Ferrari, A.; Sangiovanni, D.G.; Rogal, J.; Drautz, R. First-principles characterization of reversible martensitic transformations. Phys. Rev. B 2019, 99, 1–6. [Google Scholar] [CrossRef]
- Milman, V.; Winkler, B.; White, J.A.; Pickard, C.J.; Payne, M.C.; Akhmatskaya, E.V.; Nobes, R.H. Electronic structure, properties, and phase stability of inorganic crystals: A pseudopotential plane-wave study. Int. J. Quantum Chem. 2000, 77, 895–910. [Google Scholar] [CrossRef]
- Segall, M.D.; Lindan, P.J.D.; Probert, M.J.; Pickard, C.J.; Hasnip, P.J.; Clark, S.J.; Payne, M.C. First-principles simulation: Ideas, illustrations and the CASTEP code. J. Phys. Condens. Mat. 2002, 14, 2717–2744. [Google Scholar] [CrossRef]
- Clark, S.J.; Segall, M.D.; Pickard, C.J.; Hasnip, P.J.; Probert, M.I.J.; Refson, K.; Payne, M.C. First principles methods using CASTEP. Z. Krist. Cryst. Mater. 2005, 220, 567–570. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed]
- Vanderbilt, D. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism. Phys. Rev. B 1990, 41, 7892–7895. [Google Scholar] [CrossRef] [PubMed]
- Fischer, T.H.; Almlof, J. General methods for geometry and wave function optimization. J. Phys. Chem. 1992, 96, 9768–9774. [Google Scholar] [CrossRef]
- Ikehata, H.; Nagasako, N.; Furuta, T.; Fukumoto, A.; Miwa, K.; Saito, T. First-principles calculations for development of low elastic modulus Ti alloys. Phys. Rev. B 2004, 70, 1–8. [Google Scholar] [CrossRef]
- Wu, C.Y.; Xin, Y.H.; Wang, X.F.; Lin, J.G. Effects of Ta content on the phase stability and elastic properties of β Ti-Ta alloys from first-principles calculations. Solid State Sci. 2010, 12, 2120–2124. [Google Scholar] [CrossRef]
- Nye, J.F. Physical Properties of Crystals: Their Representation by Tensors and Matrices; Oxford University Press: Oxford, UK, 1985. [Google Scholar]
- Pugh, S.F. XCII. Relations between the elastic moduli and the plastic properties of polycrystalline pure metals. Philos. Mag. 1954, 45, 823–843. [Google Scholar] [CrossRef]
- Iotova, D.; Kioussis, N.; Lim, S.P. Electronic structure and elastic properties of the Ni3X(X = Mn, Al, Ga, Si, Ge) intermetallics. Phys. Rev. B 1996, 54, 14413–14422. [Google Scholar] [CrossRef]
- Huang, J.H.; Huang, S.P.; Ho, C.S. The ductile-brittle transition of a zirconium alloy due to hydrogen. Scr. Metall. Mater. 1993, 28, 1537–1542. [Google Scholar] [CrossRef]
- Samal, M.K.; Seidenfuss, M.; Roos, E.; Dutta, B.K.; Kushwaha, H.S. Experimental and numerical investigation of ductile-to-brittle transition in a pressure vessel steel. Mater. Sci. Eng. A 2008, 496, 25–35. [Google Scholar] [CrossRef]
- Mattesini, M.; Ahuja, R.; Johansson, B. Cubic Hf3N4 and Zr3N4: A class of hard materials. Phys. Rev. B 2003, 68, 1–5. [Google Scholar] [CrossRef]
- Fu, H.Z.; Zhao, Z.G.; Liu, W.F.; Peng, F.; Gao, T.; Cheng, X.L. Ab initio calculations of elastic constants and thermodynamic properties of TiAl under high pressures. Intermetallics 2010, 18, 761–766. [Google Scholar] [CrossRef]
- Yoo, M.H. On the theory of anomalous yield behavior of Ni3Al—Effect of elastic anisotropy. Scr. Metall. 1986, 20, 915–920. [Google Scholar] [CrossRef]
- Lau, K.; Mccurdy, A.K. Elastic anisotropy factors for orthorhombic, tetragonal, and hexagonal crystals. Phys. Rev. B 1998, 58, 8980–8984. [Google Scholar] [CrossRef]
- Fu, H.Z.; Li, X.F.; Liu, W.F.; Ma, Y.M.; Gao, T.; Hong, X.H. Electronic and dynamical properties of NiAl studied from first principles. Intermetallics 2011, 19, 1959–1967. [Google Scholar] [CrossRef]
- Reed, R.P.; Clark, A.F. American Society of Metals; Metals Park: Geauga County, OH, USA, 1983. [Google Scholar]
- Friák, M.; Šob, M.; Vitek, V. Ab initio calculation of tensile strength in iron. Philos. Mag. 2003, 83, 3529–3537. [Google Scholar] [CrossRef]
- Fu, H.Z.; Peng, W.M.; Gao, T. Structural and elastic properties of ZrC under high pressure. Mater. Chem. Phys. 2009, 115, 789–794. [Google Scholar] [CrossRef]
- Johnson, R.A. Analytic nearest-neighbour model for fcc metals. Phys. Rev. B 1988, 37, 3924–3931. [Google Scholar] [CrossRef]
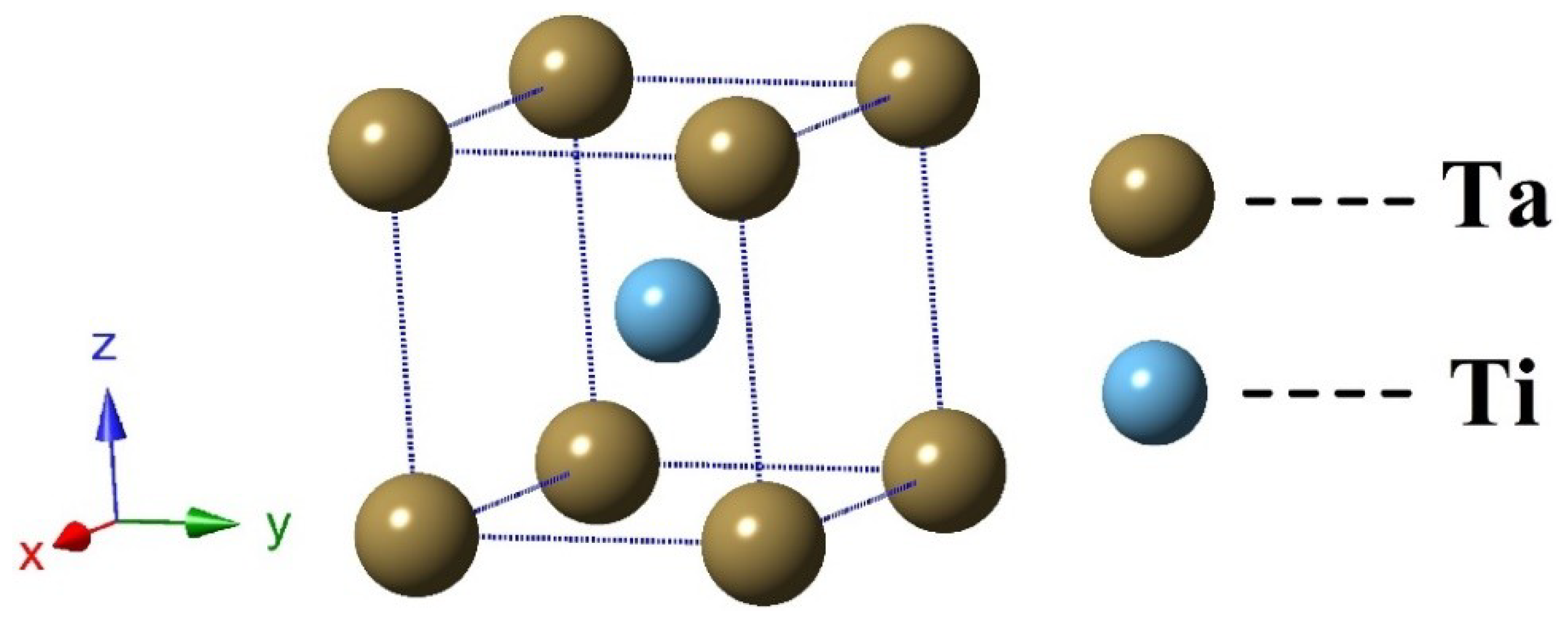
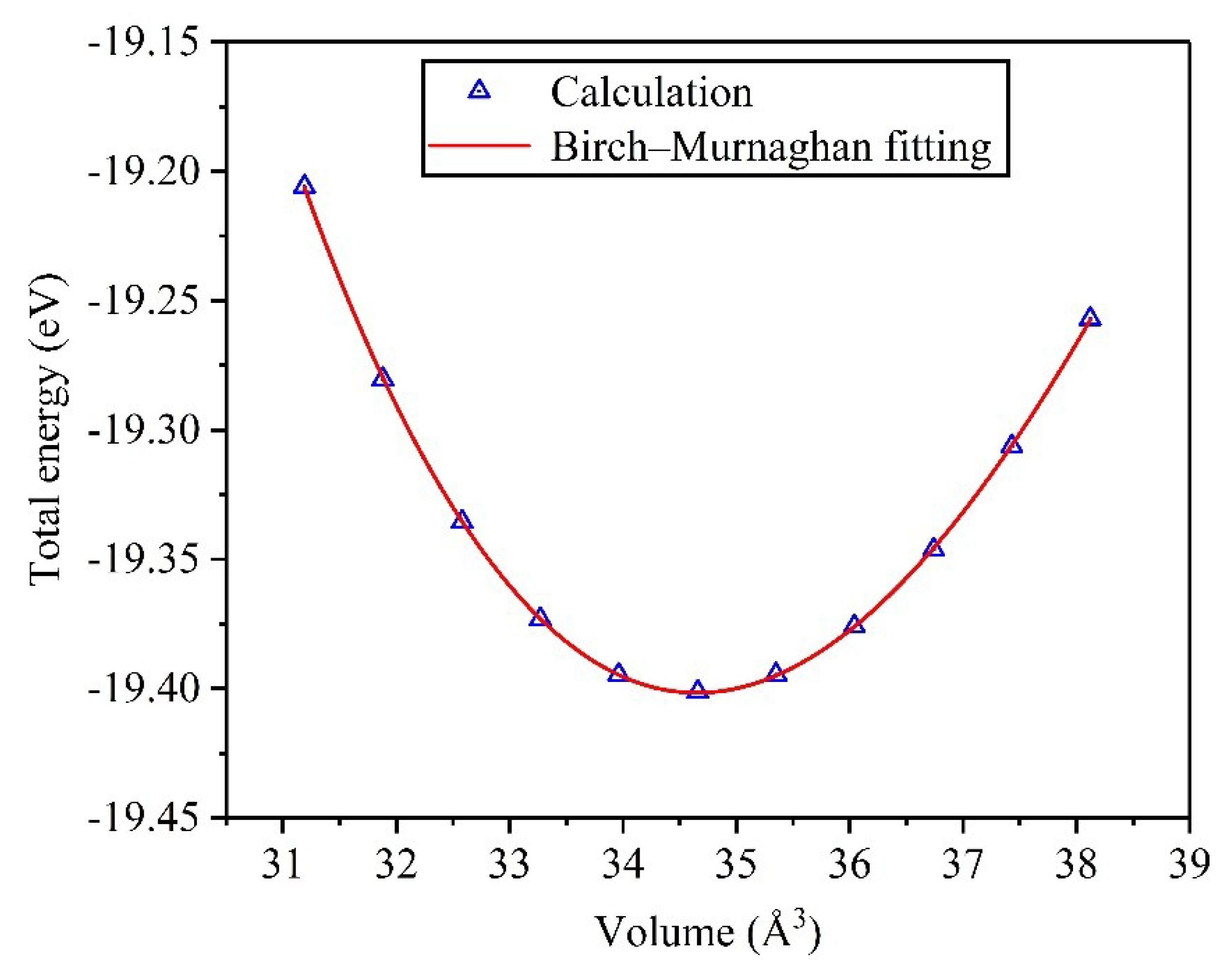

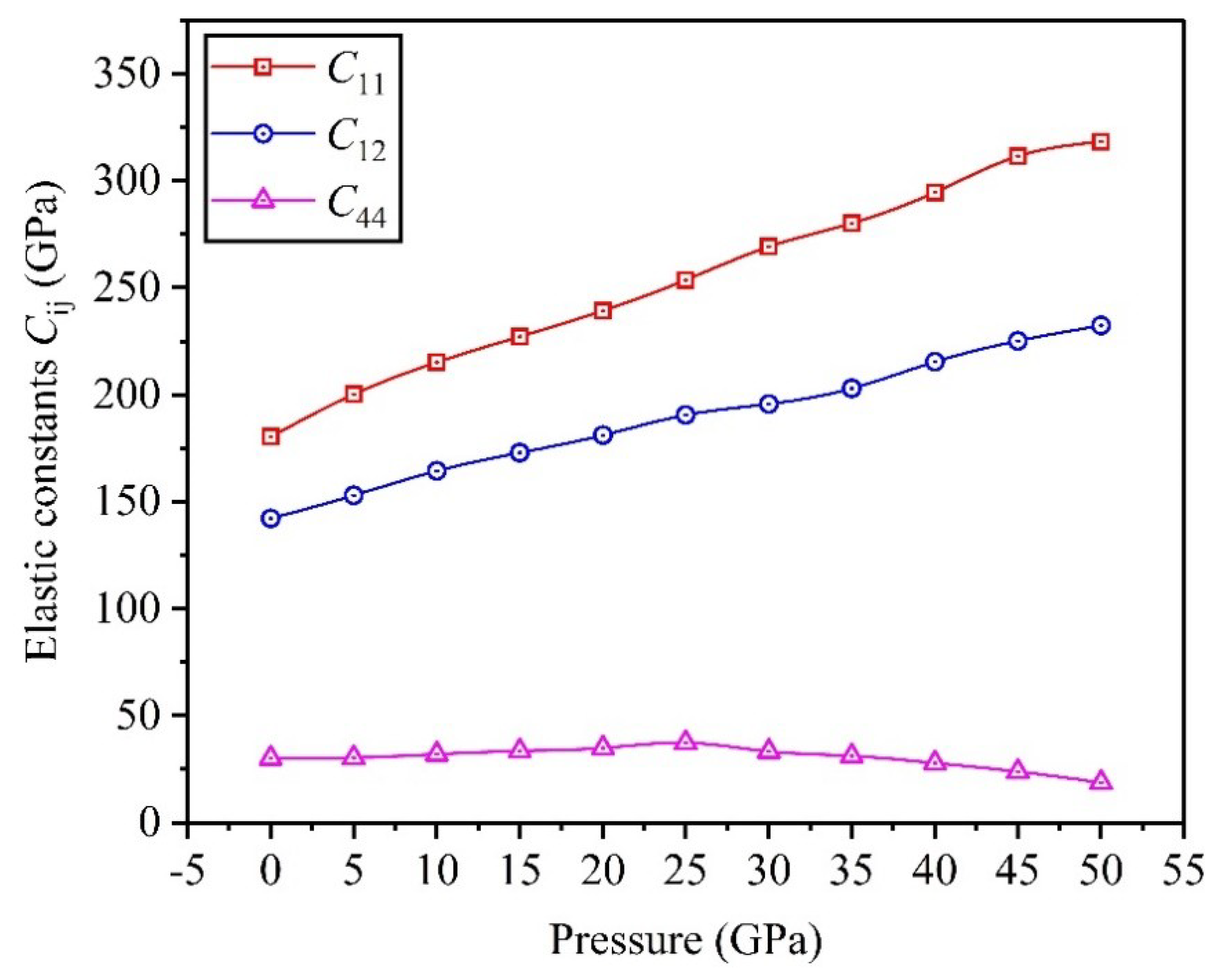
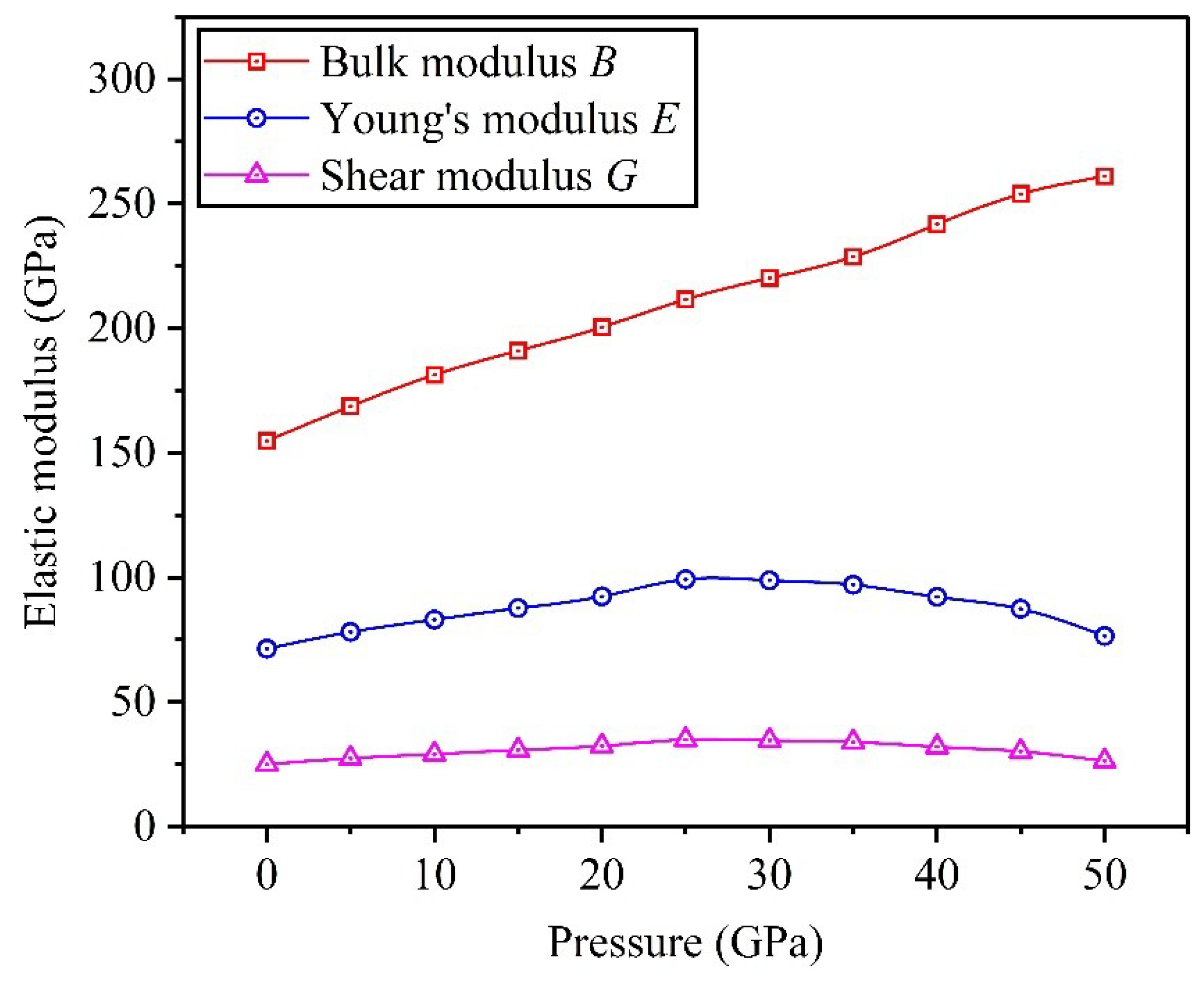




| Ti0.5Ta0.5 Alloy | Present | Experimental Data | Theoretical Data |
|---|---|---|---|
| Lattice constant a0 (Å) | 3.260 | 3.295 [11], 3.286 [14] | 3.278 [24], 3.274 [25] |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, F.; Liu, Y. First-Principles Calculations of High-Pressure Physical Properties of Ti0.5Ta0.5 Alloy. Symmetry 2020, 12, 796. https://doi.org/10.3390/sym12050796
Yu F, Liu Y. First-Principles Calculations of High-Pressure Physical Properties of Ti0.5Ta0.5 Alloy. Symmetry. 2020; 12(5):796. https://doi.org/10.3390/sym12050796
Chicago/Turabian StyleYu, Fang, and Yu Liu. 2020. "First-Principles Calculations of High-Pressure Physical Properties of Ti0.5Ta0.5 Alloy" Symmetry 12, no. 5: 796. https://doi.org/10.3390/sym12050796
APA StyleYu, F., & Liu, Y. (2020). First-Principles Calculations of High-Pressure Physical Properties of Ti0.5Ta0.5 Alloy. Symmetry, 12(5), 796. https://doi.org/10.3390/sym12050796




