Sources of Asymmetry and the Concept of Nonregularity of n-Dimensional Density Matrices

Abstract

The information contained in an n-dimensional (nD) density matrix ρ is parametrized and interpreted in terms of its asymmetry properties through the introduction of a family of components of purity that are invariant with respect to arbitrary rotations of the nD Cartesian reference frame and that are composed of two categories of meaningful parameters of different physical nature: the indices of population asymmetry and the intrinsic coherences. It is found that the components of purity coincide, up to respective simple coefficients, with the intrinsic Stokes parameters, which are also introduced in this work, and that determine two complementary sources of purity, namely the population asymmetry and the correlation asymmetry, whose weighted square average equals the overall degree of purity of ρ. A discriminating decomposition of ρ as a convex sum of three density matrices, viz. the pure, the fully random (maximally mixed) and the discriminating component, is introduced, which allows for the definition of the degree of nonregularity of ρ as the distance from ρ to a density matrix of a system composed of a pure component and a set of 2D, 3D,… and nD maximally mixed components. The chiral properties of a state ρ are analyzed and characterized from its intimate link to the degree of correlation asymmetry. The results presented constitute a generalization to nD systems of those established and exploited for polarization density matrices in a series of previous works.

1. Introduction

Density matrices play a key role in both quantum mechanics and classical treatment of mixed states [1], as for instance in the characterization of the second-order polarization properties of electromagnetic waves [2,3]. In this paper, the concepts of discriminating decomposition, intrinsic density matrix, sources of purity (population and correlation asymmetry), intrinsic Stokes parameters, degree of randomness and nonregularity are introduced and analyzed in terms of certain types of asymmetry exhibited by density matrices representing n-dimensional systems. The fact that these notions have proven to be very fruitful for the study and interpretation of polarization density matrices (three-dimensional systems), supports their generalization to n-dimensional (nD) density matrices. The sections of the paper are organized in the following manner. Main notations and the framework for the decompositions of a density matrix are introduced below, within the present introductory section. The concepts of n-dimensional Stokes parameters and Bloch vector are formulated in Section 2, as required for some results presented in further sections. Section 3 is devoted to the discriminating decomposition of a density matrix into a convex sum of three density matrices, namely the pure, the fully random (maximally mixed), and the discriminating components; the asymmetry features of the last one determining critical aspects of the physical properties of the whole density matrix. Appropriate parameters characterizing the degree of purity (statistical asymmetry) and randomness (statistical symmetry) are considered in Section 4, which together with the notion of the intrinsic density matrix introduced in Section 5, lead to the definition in Section 6 of two complementary sets of invariant descriptors of asymmetry, namely the indices of population asymmetry and the intrinsic coherences, whose contributions to the overall purity of the state are studied in Section 7. By taking advantage of the results of the previous sections, the concept of nonregularity of a density matrix is introduced in Section 8, where its intimate link to the correlation asymmetry and to the chiral properties of the discriminating component is shown. Finally, Section 9 includes a summary and discussion on the main results presented.

Let us consider an n-dimensional system whose state is characterized by a set of n random variables $v_i$ $\left(i=1,\dots ,n\right)$ that can be arranged into a vector $v={\left(v_1,\dots ,v_n\right)}^{\mathrm{T}}$ of the n-dimensional complex vector space ${ℂ}^n$, where the superscript “T” indicates transpose. In the special case that $v_i$ do not fluctuate (i.e., the vector state v is fixed), such a state is pure. Nevertheless, in general, uncertainties or fluctuations on the components of v should be considered, and the corresponding mixed state, which necessarily involves a certain increase of symmetry, is represented by the associated density matrix ρ whose elements ${\rho }_{ij}$ are the ensemble averages ${\rho }_{ij}=\langle v_i^{*}{v}_j\rangle$ over the realizations of the components of v.

From a mathematical point of view, a given n×n matrix ρ can be considered a density matrix if and only if ρ is a trace-normalized positive semidefinite Hermitian matrix, i.e., $\mathrm{tr}\rho =1$, $\rho ={\rho }^{†}$ (where the dagger indicates conjugate transpose and “tr” stands for the trace), while the real n eigenvalues of ρ are nonnegative. Therefore, ρ can always be expressed as $\rho =U \Lambda U^{†}$, where U is a unitary matrix and $\Lambda \equiv \mathrm{diag}\left({\lambda }_1,\dots ,{\lambda }_n\right)$ is the diagonal matrix whose diagonal elements are the eigenvalues of ρ taken in decreasing order $\left({\lambda }_1\ge {\lambda }_2\ge \dots \ge {\lambda }_n\right)$. The diagonalization of ρ leads to its spectral decomposition

$$\rho ={\displaystyle {\displaystyle \sum _{k=1}^n}{\lambda }_k U L_k{U}^{†}}={\displaystyle {\displaystyle \sum _{k=1}^n}{\lambda }_k\left(u_k\otimes u_k^{†}\right)}, L_k\equiv \mathrm{diag}\left(\underset{k}{\underbracket{0,\dots ,0,1}},\underset{n-k}{\underbracket{0,\dots ,0}}\right),$$

(1)

where ⊗ stands for the Kronecker product, $u_k$ are the unit eigenvectors of ρ (which in turn are the vector-columns of U, and represent the pure eigenstates of ρ) and $L_k$ are diagonal matrices whose only nonzero component is $l_k=1$. By denoting $r\equiv \mathrm{rank}\rho$ and considering an arbitrary generalized basis of ${ℂ}^n$, being composed of (a) a set of r independent unit vector states $w_i$ generating $\mathrm{range}\rho$ ($\mathrm{range}\rho$ being the subspace of ${ℂ}^n$ generated by the r eigenvectors $u_1,\dots ,u_r$ with corresponding nonzero eigenvalues ${\lambda }_{ 1},\dots ,{\lambda }_{ r}$), and (b) a set of $n-r$ independent unit vector states $w_j$ generating $\ker \rho$ ($\ker \rho$ being the subspace of ${ℂ}^n$ generated by the $n-r$ eigenvectors $u_{r+1},\dots ,u_n$ with corresponding zero eigenvalues ${\lambda }_{ r+1}=\dots ={\lambda }_n=0$), the density matrix ρ can be expressed as a convex sum of the r pure density matrices $\rho =w_i\otimes w_i^{†}$ $\left(i=1,\dots ,r\right)$, through the arbitrary decomposition of ρ [4,5,6,7,8].

$$\rho ={\displaystyle {\displaystyle \sum _{i=1}^r}{p}_i\left(w_i\otimes w_i^{†}\right)}, p_i=\frac{1}{{\displaystyle {\displaystyle \sum _{q = 1}^r}\frac{1}{{\lambda }_q}{\left|{\left(U^{†}{\widehat{w}}_i\right)}_q\right|}^2}}, \left[{\displaystyle {\displaystyle \sum _{i=1}^r}{p}_i}=1\right].$$

(2)

From a statistical point of view, the most “symmetric” state is represented by ${\rho }_u=I_n/n$ ($I_n$ being the n×n identity matrix, so that, the n eigenvalues of ${\rho }_u$ are equal to $1/n$). Conversely, the most “asymmetric” situation corresponds to a pure density matrix $\rho =u_1\otimes u_1^{†}$, which is characterized by the fact that it has a single nonzero eigenvalue ${\lambda }_{ 1}=1$.

2. nD Stokes Parameters and Bloch Vector

Certain results to be considered in further sections concerning invariant descriptors of the asymmetry properties of a density matrix ρ are closely linked to the concept of nD Stokes parameters, which are defined as the (real) coefficients $s_k$ $\left(i=1,\dots ,n-1\right)$ of the expansion of ρ in terms of the basis of Hermitian matrices composed of the identity matrix $I_n$ together with the $n^2-1$ generalized Pauli matrices ${\Lambda }_k$ (also called generalized Gell-Mann matrices) [9]

$$\rho =\frac{1}{n}\left(I_n+\sqrt{\frac{n\left(n-1\right)}{2}} {\displaystyle {\displaystyle \sum _{k=1}^{n-1}}{s}_k {\Lambda }_k}\right),$$

(3)

where the set ${\Lambda }_k$ $\left(k=1,\dots ,n-1\right)$ of the elements of the Lie algebra of SU(n) is composed of the union of (a) $n\left(n-1\right)/2$ symmetric (nondiagonal) matrices ${\mathrm{W}}_{kl}$ $\left(1\le k<l\le n\right)$ whose elements are zero except for ${\left({\mathrm{W}}_{kl}\right)}_{kl}={\left({\mathrm{W}}_{kl}\right)}_{lk}=1$; (b) $n\left(n-1\right)/2$ antisymmetric matrices ${\Omega }_{kl}$ $\left(1\le k<l\le n\right)$ whose elements are zero except for ${\left({\Omega }_{kl}\right)}_{kl}=-{\left({\Omega }_{kl}\right)}_{lk}=-i$, and (c) the $n-1$ diagonal matrices.

$$\begin{array}{c}{\Lambda }_1=\mathrm{diag}\left(1,-1,\underset{n-2}{\underbrace{0,\dots ,0}}\right), {\Lambda }_2=\sqrt{\frac{1}{3}}\mathrm{diag}\left(1,1,-2,\underset{n-3}{\underbrace{0,\dots ,0}}\right),\\ {\Lambda }_k=\sqrt{\frac{2}{k\left(k+1\right)}}\mathrm{diag}\left(\underset{k}{\underbrace{1,\dots ,1}},-k,\underset{n-k-1}{\underbrace{0,\dots ,0}}\right) ,\\ {\Lambda }_{n-1}=\sqrt{\frac{2}{\left(n-1\right)n}}\mathrm{diag}\left(\underset{n-1}{\underbrace{1,\dots ,1}},-\left(n-1\right)\right).\end{array}$$

(4)

The nD Stokes parameters $s_k=\sqrt{n/\left[2\left(n-1\right)\right]}\mathrm{tr}\left(\rho {\Lambda }_k\right)$, together with $s_0=1$ are the components of the nD normalized Stokes vector ${\left(1,s_1,\dots ,s_{n-1}\right)}^{\mathrm{T}}$. Note that the non-normalized version Φ (coherency matrix) of ρ is $\Phi =s_0 \rho$, with $s_0=\mathrm{tr}\Phi$, which is equivalent to using the non-normalized form of the Stokes vector. The nD Bloch vector (or coherence vector [9]) associated with ρ is $s={\left(s_1,\dots ,s_{n-1}\right)}^{\mathrm{T}}$ [9,10,11,12]. Here we have followed the criterion of Byrd and Khaneja [9] for the definition of parameters $s_k$, in such a manner that the absolute value $\left|s\right|$ of the Bloch vector equals 1 for pure states and, as shown in Section 4, in general coincides with the degree of purity of the state represented by ρ.

3. Discriminating Decomposition of a Density Matrix

Given an nD (n-dimensional) density matrix ρ, its spectral decomposition (1) can be rearranged into the characteristic decomposition (also called trivial decomposition) [4]

$$\rho ={\displaystyle {\displaystyle \sum _{k=1}^n}\left(P_k-P_{k-1}\right)U D_k{U}^{†}}, D_k\equiv \frac{1}{k}\mathrm{diag}\left(\underset{k}{\underbracket{1,1,\dots ,1}},\underset{n-k}{\underbracket{0,\dots ,0}}\right),$$

(5)

where the coefficients are determined by the indices of purity $P_k$ (hereafter IPP) [13], defined as follows in terms of the ordered eigenvalues of ρ.

$$P_k={\displaystyle {\displaystyle \sum _{j=1}^k}{\lambda }_j-k{\lambda }_{k+1}}, \left[1\le k\le n-1, {\lambda }_1\ge {\lambda }_2\ge \dots \ge {\lambda }_n, {\displaystyle {\displaystyle \sum _{j=1}^n}{\lambda }_j}=1\right].$$

(6)

By grouping the addends of (5) involving $U D_k{U}^{†}$ for $k=2,3,\dots ,n-1$ into an aggregate component ${\rho }_d$, the characteristic decomposition becomes the discriminating decomposition

$$\begin{array}{c}\rho =P_1 {\rho }_p+\left(P_{n-1}-P_1\right){\rho }_d+\left(1-P_{n-1}\right){\rho }_u,\\ {\rho }_p\equiv \mathrm{diag}\left(1,0,\dots ,0\right), {\rho }_u\equiv \frac{1}{n}{I}_n, \\ {\rho }_d\equiv \frac{{\displaystyle {\displaystyle \sum _{k=2}^{n-1}}\left(P_k-P_{k-1}\right)U D_k}{U}^{†}}{P_{n-1}-P_1}=\frac{\rho -P_1 {\rho }_p-\left(1-P_{n-1}\right){\rho }_u}{P_{n-1}-P_1},\end{array}$$

(7)

which provides an expansion of ρ into a convex composition of the density matrices ${\rho }_p$, ${\rho }_d$ and ${\rho }_u$, which are associated, respectively, with the pure component ${\rho }_p$ (which has a single nonzero eigenvalue and is generated by the single eigenstate $u_1$), the discriminating component ${\rho }_d$ (whose peculiar features will be analyzed in Section 9), and the fully random (or unpolarized) component ${\rho }_u=I_n/n$, where $I_n$ is the identity matrix, which corresponds to a maximally mixed state, i.e., to an equiprobable mixture of the n orthonormal eigenstates of ρ. The IPP, which determine the coefficients of the characteristic and discriminating decompositions of ρ is a set of $n-1$ invariant parameters that provide complete quantitative information on the structure of purity of ρ and satisfy the nested inequalities $0\le P_1\le \dots \le P_k\le \dots \le P_{n-1}\le 1$ [13]. The 3D and 4D formulations of the IPP have proven to be useful to address certain problems in polarization optics [7,14,15].

As the larger IPP take consecutively their maximal values equal to 1 ($1=P_{n-1}=P_{n-2}=\dots P_{n-1-r}$), the number of nonzero eigenvalues is reduced correspondingly from n to r (with $r\equiv \mathrm{rank}\rho$), that is to say, the $n-r$ upper dimensions collapse because the number of nonvanishing components of the arbitrary and characteristic decompositions becomes r, and the system can be treated mathematically as having r effective dimensions. Nevertheless, as shown in Section 4, the $n-r$ extra dimensions (corresponding to $\ker \rho$, also called the null space of ρ) have a non-neutral effect on the overall purity of ρ.

4. Degrees of Purity and Randomness of a Density Matrix

A proper overall measure of the degree of purity (or degree of asymmetry) of a state ρ is given by [16,17]

$$P_{nD}=\sqrt{\frac{1}{n-1}\left[n\mathrm{tr}{\mathsf{\rho }}^2-1\right]}=\sqrt{\frac{1}{n-1}\left(n{\displaystyle \sum _{k=1}^n}{\lambda }_k^2-1\right)}=\left|\mathbf{s}\right|,$$

(8)

where $\left|s\right|$ stands for the magnitude of the associated nD Bloch vector (see Section 2). $P_{nD}$ gives a measure of the distance from a state ρ to a maximally mixed one (fully random) and can also be expressed as follows in terms of the IPP [13],

$$P_{nD}=\sqrt{\frac{n}{n-1}\left({\displaystyle \sum _{k=1}^{n-1}}\frac{P_k^2}{k\left(k+1\right)}\right)}.$$

(9)

As a counterpart of $P_{nD}$, the distance from the system to a pure one is given by the degree of randomness (or degree of statistical symmetry), which we define as:

$$R_{nD}=\sqrt{1-P_{nD}^2}=\sqrt{\frac{n-\mathrm{tr}{\mathsf{\rho }}^2}{n-1}}=\sqrt{\frac{1}{n-1}\left(n-{\displaystyle \sum _{k=1}^n}{\lambda }_k^2\right)}=\sqrt{1-\frac{n}{n-1}\left({\displaystyle \sum _{k=1}^{n-1}}\frac{P_k^2}{k\left(k+1\right)}\right)}.$$

(10)

Pure systems (i.e., $r=1$), are characterized by $P_{nD}=1$, or equivalently $R_{nD}=0$, which correspond to the case where all the IPP take their maximal values $P_k=1$. Intermediate values $1>P_{nD}>0$ are taken by $P_{nD}$ for mixed states, while the minimal value $P_{nD}=0$ corresponds to the limiting case of maximally mixed states (equiprobable mixtures of the eigenstates), which are also characterized by $R_{nD}=1$ and $P_k=0$ $\left(k=1,\dots ,n-1\right)$. Therefore, the expressions of $P_{nD}$ and $R_{nD}$ in terms of the IPP make explicit the role played by $P_k$ in the quantitative structure of purity of ρ; that is to say, each $P_k$ leads to a particular contribution $n{P}_k^2$/[(n − 1)k(k + 1)] to $P_{nD}^2$, and the lower and upper values of each $P_k$ are limited by $P_{k-1}$ and $P_{k+1}$ respectively $\left(0\le P_1\le \dots \le P_k\le \dots \le P_{n-1}\le 1\right)$. Observe also that, although the number $n-r$ of IPP equal to 1 ($P_r=P_{r+1}=\dots =P_{n-1}=1$) entails that the apparent effective dimensions of the system are r instead of n (i.e., the last $n-r$ eigenvalues of ρ are zero), the apparent excess of dimensions results in a significant contribution to purity. This interesting feature has been studied in Ref. [18] for the particular case of 3D polarization matrices.

The fact that purity is realized if and only if $\mathrm{tr}{\rho }^2=1$ motivated use of the term purity of ρ for the quantity $\mathrm{tr}{\rho }^2$ [19]. Maximally mixed states satisfy $\mathrm{tr}{\rho }^2=1/n$, so that $1/n\le \mathrm{tr}{\rho }^2\le 1$. Nevertheless, an interesting feature of using $P_{nD}$ instead of $\mathrm{tr}{\rho }^2$ as a measure of the degree of purity is that it takes the more natural limiting value $P_{nD}=0$ for maximally mixed states (maximal statistical symmetry). In addition, the overall measure of purity provided by $P_{nD}$ can be expressed, as in (9), as a weighted square average of the IPP, which in turn provides detailed and complete quantitative information on the structure of purity of ρ. The parameter $P_{nD}$ was first introduced by Samson under the scope of ultra low-frequency magnetic fields [16], and later by Barakat [17] (with a different, but equivalent mathematical expression). $P_{nD}$ was also considered implicitly by Byrd and Khaneja [9] as the magnitude of the coherence vector (or Bloch vector) associated with an nD density matrix. The ability of $P_{3D}$ to represent the degree of polarization (or degree of polarimetric purity) for electromagnetic waves, as well as some important features, have been studied by Setälä et al. [18,20,21], Luis [22] and by Gil et al. [4,23,24]. Furthermore, $P_{4D}$ was independently introduced by Gil and Bernabéu as the depolarization index [25] associated with Mueller matrices representing the transformation of polarization states by the action of a material medium.

Another well-known measure of purity is the von Neumann entropy,

$$S_{nD}=S_{3D}=-\mathrm{tr}\left(\mathsf{\rho }{log}_n\mathsf{\rho }\right)=-{\displaystyle \sum _{i=1}^n}\left({\lambda }_i{log}_n{\lambda }_i\right).$$

(11)

Note that, as suggested by Cloude for polarization density matrices [26], although the Napierian logarithm (ln) is commonly used for the definition of the von Neumann entropy, the use of the base n logarithm for the definition of the entropy of nD density matrices has the peculiarity of restricting the values of $S_{nD}$ to the range $0\le S_{nD}\le 1$. Observe also that, while $S_{nD}$ does not have an analytic expression as a function of $P_{nD}$ (or $R_{nD}$), the fact that ${\lambda }_{ i}$ can be expressed in terms of the IPP [13] implies that $S_{nD}$ admits an analytic expression in terms of the IPP $\left(P_k\right)$. Maximal entropy $S_{nD}=1$ corresponds to a maximally mixed state ($P_1=P_2=\dots =P_{n-1}=0$, i.e., $P_{3D}=0$), while the minimum achievable value $S_{3D}=0$ is reached for pure states ($P_1=P_2=\dots =P_{n-1}=1$, i.e., $P_{3D}=1$). The concept of von Neumann entropy has been considered under the context of polarization optics for 2D, 3D and 4D density matrices in a number of works, like in Refs. [2,27,28,29,30,31,32,33,34,35], as well as its comparison to the degree of polarization [2] and to the depolarization index [32,33,35].

5. The Intrinsic Density Matrix

Given a density matrix ρ, it has $n-1$ quantities that are invariant with respect to unitary similarity transformations $V \rho V^{†}$(with V unitary). An interesting set of such invariant quantities is that constituted by the IPP [13] because they determine the quantitative (but not qualitative) structure of purity in a hierarchical and meaningful manner. To get a more qualitative view of the information contained in ρ, it is also interesting to explore its invariants with respect to changes of the n-dimensional Cartesian reference frame taken for its representation. Such changes correspond to orthogonal similarity transformations $Q^{\mathrm{T}}\rho Q$, so that the density matrix of a given physical system adopts a particular form for each Cartesian coordinate system $X_1,X_2,\dots X_n$ considered. Among them, the intrinsic reference frame $X_{1O},X_{2O},\dots X_{nO}$ is defined as the one for which the real part $\mathrm{Re}\left({\rho }_O\right)$ of the corresponding form ${\rho }_O$ of the same state that is represented by ρ with respect to $X_1,X_2,\dots X_n$ becomes diagonal, $\mathrm{Re}\left({\rho }_O\right)=\mathrm{diag}\left(a_1,\dots ,a_n\right)$, with $a_1\ge a_2\ge \dots \ge a_n$. Observe that, from the very definition of the density matrix, the equalities $\mathrm{tr}\rho =\mathrm{tr}{\rho }_O=\mathrm{tr}\mathrm{Re}\left({\rho }_O\right)={\displaystyle {\sum }_{k=1}^n{a}_k}=1$ are satisfied. The transformation from ρ to ${\rho }_O$ through the rotation of the coordinate system from $X_1,X_2,\dots X_n$ to $X_{1O},X_{2O},\dots X_{nO}$ is performed by means of the corresponding orthogonal similarity transformation ${\rho }_O=Q_O^{\mathrm{T}} \rho Q_O$, where $Q_O$ is a proper orthogonal matrix ($Q_O^{\mathrm{T}}=Q_O^{-1}$,$\det Q_O=+1$). Therefore, the intrinsic density matrix ${\rho }_O$ has the peculiar form

$$\begin{array}{c}{\mathsf{\rho }}_O={\mathbf{Q}}_O^{\mathrm{T}}\mathsf{\rho }{\mathbf{Q}}_O=\mathbf{A}+i\frac{1}{2}\mathbf{N},\\ \left[\mathbf{A}\equiv diag\left(a_1,a_2,\dots ,a_n\right),a_1\ge a_2\ge \dots \ge a_n,{\mathbf{N}}^{\mathrm{T}}=-\mathbf{N}\right],\end{array}$$

(12)

where N, whose components are denoted by $\epsilon n_{ij}$ (with $\epsilon =\pm 1$ depending on whether $i+j$ is an odd or even number respectively), is an antisymmetric matrix that encompasses all the imaginary part of ${\rho }_O$. The coefficient $1/2$ in the definition of N and the sign coefficient ε in its components have been introduced for the sake of consistency with the components of the spin vector of polarization density matrices [36,37,38].

The number l of scalar real invariants involved in ρ with respect to arbitrary n-dimensional rotations of the Cartesian coordinate system is equal to the number $n^2-1$ of real variables of ρ (recall that it is Hermitian and trace-normalized) minus the number $n\left(n-1\right)/2$ of parameters (angles) associated with an n×n orthogonal matrix, which results in $l=-1+n\left(n+1\right)/2$, i.e., the n intrinsic populations $a_i$ $\left(i=1,\dots ,n\right)$, with the restriction ${\displaystyle {\sum }_{i=1}^n{a}_i}=1$, plus the $n\left(n-1\right)/2$ intrinsic coherences $n_{ij}$ $\left(i=1,\dots ,n ; j>i\right)$ (hereafter denoted as IC). Note that, as usual when dealing with density matrices of quantum mechanical systems, the terms populations and coherences are used in this work to refer to the diagonal and off-diagonal elements of ρ respectively [39], while the adjective intrinsic is used for quantities derived from the intrinsic density matrix. It is remarkable that in polarization optics $\left(n=3\right)$ the pseudovector ${\left(-n_{23},n_{13},-n_{12}\right)}^T$ defined from the elements of N is precisely the spin density vector of the state represented by the corresponding 3×3 polarization density matrix ρ [36,37]. Nevertheless, the fact that the number $n\left(n-1\right)/2$ of intrinsic coherences equals the dimensions n is not a general property but it is a genuine feature of three-dimensional systems. This is the reason why the generalization of the concept of spin density vector, defined for the case $n=3$, to density matrices with dimensions $n>3$ is not possible in a consistent manner.

The intrinsic representation ${\rho }_O$ of a given ρ has the peculiar feature that the Stokes parameters corresponding to the symmetric (nondiagonal) generators ${\mathrm{W}}_{kl}$ vanish, and therefore the intrinsic Bloch vector contains no more than $l=-1+n\left(n+1\right)/2$ nonzero Stokes parameters.

Since ρ is positive semidefinite, and despite the fact that it is characterized by the nonnegativity of its four eigenvalues, the nonnegativity of its principal minors implies certain restrictions on the values of $a_i$ and $n_{ij}$, among which we can mention $4 a_i a_j\ge n_{ij}^2$ and $4 a_i a_j a_k\ge a_i n_i^2+a_j n_j^2+a_k n_k^2$, which will be useful in further analyses.

6. Population and Correlation Asymmetries. Intrinsic Stokes Parameters

As with the $n-1$ IPP, $P_k$, defined from the n eigenvalues of ρ, we introduce the $n-1$ indices of population asymmetry (hereafter IP) defined from the n intrinsic populations of ρ in the following manner.

$$M_k={\displaystyle \sum _{j=1}^k}{a}_j-k{a}_{k+1},\left(k=1,\dots ,n-1\right).$$

(13)

The IP satisfy the nested inequalities $0\le M_1\le M_2,\dots ,\le M_{n-1}\le 1$, and provide complete information on the structure of the population asymmetry of the system represented by ρ. A state for which all the intrinsic populations are equal $a_1=a_2=\dots =a_n$ (full population symmetry, i.e., $\mathrm{Re}\rho =I_n/n$) is characterized by $M_1=M_2=\dots$$=M_{n-1}=0$, while the maximal population asymmetry is reached when $a_1=1$, $a_2=\dots =a_n=0$, i.e., $M_1=M_2=\dots$$=M_{n-1}=1$.

In analogy to the names used in polarization optics, we call the set composed of the IP plus the IC, the components of purity (CP) of ρ. Therefore, the CP constitutes a proper complete set of l rotational invariants of ρ. To go deeper into the physical significance of the CP, let us consider the expansion of the intrinsic density matrix ${\rho }_O$ in terms of the SU(n) generators ${\Lambda }_k$ introduced in Section 2, which adopts the form

$${\mathsf{\rho }}_O=\frac{1}{n}\left\{{\mathbf{I}}_n+\sqrt{\frac{n\left(n-1\right)}{2}}\left[{\displaystyle \sum _{k=1}^{n-1}}\left(\sqrt{\frac{n}{\left(n-1\right)k\left(k+1\right)}}{M}_k\right){\Lambda }_k+{\displaystyle \sum _{\begin{array}{c}i,j=1\\ i<j\end{array}}^n}\left(\sqrt{\frac{n}{2\left(n-1\right)}}{n}_{ij}\right){\Omega }_{ij}\right]\right\},$$

(14)

where the intrinsic Stokes parameters (i.e., the set of Stokes parameters corresponding to the intrinsic representation ${\rho }_O$ of ρ) are given by the set composed of the $n-1$ intrinsic population-Stokes parameters

$$t_k=c_k{M}_k,\left[c_k\equiv \sqrt{\frac{n}{\left(n-1\right)k\left(k+1\right)}},k=1,\dots ,n-1\right],$$

(15)

together with the $n\left(n-1\right)/2$ intrinsic correlation-Stokes parameters

$$t_{ij}=c{n}_{ij},\left[c\equiv \sqrt{\frac{n}{2\left(n-1\right)}},1\le i<j\le n\right],$$

(16)

showing the remarkable result that, up to respective coefficients $c_k$, $M_k$ are not other than the intrinsic Stokes parameters $t_k$ corresponding to the diagonal generators ${\Lambda }_k$, while the intrinsic correlation-Stokes parameters $t_{ij}$ (associated with the antisymmetric generators ${\Omega }_{ij}$) are directly linked to the intrinsic coherences $t_{ij}=c n_{ij}$. The difference between the physical nature of $M_k$ and $n_{ij}$, together with the fact that a number of $n\left(n-1\right)/2$ Stokes parameters become zero in the intrinsic representation, suggests the appropriateness of the arrangement of the $l=-1+n\left(n+1\right)/2$ nonzero intrinsic Stokes parameters in the form of the following upper triangular Stokes parameters matrix

$$\begin{array}{c}\mathbf{S}\equiv c_n\left(\begin{array}{cccccc}1/c_n& n_{12}& \dots & n_{1k}& \dots & n_{1n}\\ 0& M_1& \dots & n_{2k}& \dots & n_{2n}\\ ⋮& ⋮& \ddots & ⋮& ⋮& ⋮\\ 0& 0& \dots & \sqrt{2/\left[k\left(k+1\right)\right]}{M}_k& \dots & n_{kn}\\ ⋮& ⋮& ⋮& ⋮& \ddots & ⋮\\ 0& 0& \dots & 0& \dots & \sqrt{2/\left[\left(n-1\right)n\right]}{M}_k\end{array}\right),\\ c_n\equiv \sqrt{\frac{n}{2\left(n-1\right)}},\end{array}$$

(17)

whose elements are denoted as $s_{kl}$ $\left(k,l=0,1,\dots ,n-1\right)$ with $s_{00}=1$, $s_{kk}=c_k\sqrt{2/\left[k\left(k+1\right)\right]} M_k$ $\left(k=1,\dots ,n-1\right)$, and $s_{kl}=c_n n_{kl}$ $\left(1\le k<l\le n-1\right)$; in such a manner that the intrinsic population-Stokes parameters are arranged along the diagonal of matrix S, while the intrinsic correlation-Stokes parameters are arranged in its upper off-diagonal part. Obviously, when the non-normalized version $\Phi =\left(\mathrm{tr}\Phi \right) \rho$ of the coherency matrix is considered, then the corresponding (non-normalized) Stokes parameters matrix is given by $\left(\mathrm{tr}\Phi \right) T$. Observe also that $P_{nD}={\Vert S\Vert }_2^2-1$.

In summary, the information contained in the density matrix ρ can be grouped into three kinds of parameters of different nature, (a) the $n\left(n-1\right)/2$ angles determining the orthogonal matrix $Q_O$ that performs the rotation transformation from the intrinsic reference frame to the actual one; (b) the $n-1$ IP, $M_k$, or equivalently, the intrinsic population-Stokes parameters, and (c) the $n\left(n-1\right)/2$ intrinsic coherences, $n_{ij}$, or equivalently, the intrinsic correlation-Stokes parameters.

7. Structure of Purity of a Density Matrix

The Frobenius norm ${\Vert \rho \Vert }_2=\sqrt{\mathrm{tr}{\rho }^2}$ of ρ is invariant under unitary similarity transformations (hence it is also invariant under orthogonal similarity transformations), and can be expressed as follows in terms of the CP

$${∥\mathsf{\rho }∥}_2^2=\mathrm{tr}{\mathsf{\rho }}^2={\displaystyle \sum _{i,j=1}^n}{\left|{\mathsf{\rho }}_{ij}\right|}^2={\displaystyle \sum _{i=1}^n}{a}_i^2+\frac{1}{2}{\displaystyle \sum _{\begin{array}{c}i,j=1\\ I<J\end{array}}^n}{\left|n_{ij}\right|}^2=\frac{1}{n}+{\displaystyle \sum _{k=1}^{n-1}}\frac{M_k^2}{k\left(k+1\right)}+\frac{1}{2}{\displaystyle \sum _{\begin{array}{c}i,j=1\\ i<j\end{array}}^n}{\left|n_{ij}\right|}^2,$$

(18)

and therefore the degree of purity $P_{nD}$ adopts the following expression

$$\begin{array}{cc}\hfill P_{nD}^2& =\frac{1}{n-1}\left[n\mathrm{tr}{\mathsf{\rho }}^2-1\right]=\frac{1}{n-1}\left(n{\displaystyle \sum _{i=1}^n}{a}_i^2-1+\frac{n}{2}{\displaystyle \sum _{\begin{array}{c}i,j=1\\ i<j\end{array}}^n}{\left|n_{ij}\right|}^2\right)\hfill \\ \hfill & =\frac{n}{n-1}\left({\displaystyle \sum _{k=1}^{n-1}}\frac{M_k^2}{k\left(k+1\right)}+\frac{1}{2}{\displaystyle \sum _{\begin{array}{c}i,j=1\\ i<j\end{array}}^n}{\left|n_{ij}\right|}^2\right),\hfill \end{array}$$

(19)

that is,

$$\begin{array}{c}{P}_{nD}^2=P_p^2+\frac{n}{2\left(n-1\right)}{P}_c^2,\\ P_p^2\equiv \frac{n}{n-1}\left({\displaystyle \sum _{k=1}^{n-1}}\frac{M_k^2}{k\left(k+1\right)}\right)={\displaystyle \sum _{k=1}^{n-1}}{t}_k^2,P_c^2\equiv \frac{1}{2}{∥\mathbf{N}∥}_2^2={\displaystyle \sum _{\begin{array}{c}i,j=1\\ i<j\end{array}}^n}{\left|n_{ij}\right|}^2=\frac{2\left(n-1\right)}{n}{\displaystyle \sum _{\begin{array}{c}i,j=1\\ i<j\end{array}}^n}{t_{ij}}^2,\end{array}$$

(20)

where two separate sources of purity are identified, namely the degree of population asymmetry $P_p$ and the degree of correlation asymmetry $P_c$. Both descriptors are restricted to the intervals $0\le P_p\le 1$ and $0\le P_c\le 1$, whose limits correspond to the following kinds of states,

• $P_p=0$ when the state has full population symmetry, $a_1=a_2=\dots =a_n=1/n$, so that the intrinsic density matrix takes the form ${\rho }_O=I_n/n+\left(i/2\right) N$. Note that $P_p=0$ does not necessarily imply that $P_{nD}=0$, i.e., full population symmetry is compatible with a certain degree of correlation asymmetry $P_c\le \sqrt{2\left(n-1\right)/n}$.
• $P_p=1$ when the state has full population asymmetry $a_1=1$, $a_2=\dots =a_n=0$, i.e., ${\rho }_O=\mathrm{diag}\left(1,0,\dots ,0\right)$, which in turn implies $P_{nD}=1$ and $P_c=0$.
• $P_c=0$ when the state lacks correlation asymmetry, in which case ${\rho }_O=\mathrm{diag}\left(a_1,a_2,\dots ,a_n\right)$ and all asymmetry is originated by $P_p$. The complete interval $0\le P_{nD}\le 1$ of values for $P_{nD}$ are achievable, $P_{nD}$ depending on the relative values of the intrinsic populations.
• $P_c=1$ corresponds to pure states $\left(P_{nD}=1\right)$ with maximal correlation asymmetry, in which case ${\rho }_O$ necessarily adopts the form,

  $${\mathsf{\rho }}_{Oc}=\frac{1}{2}\left(\begin{array}{ccccc}1& \mp i& 0& \dots & 0\\ \pm i& 1& 0& \dots & 0\\ 0& 0& 0& \dots & 0\\ ⋮& ⋮& ⋮& \ddots & ⋮\\ 0& 0& 0& 0& 0\end{array}\right),$$

  (21)

  with $P_p=\sqrt{\left(n/2-1\right)/\left(n-1\right)}$. It is remarkable that, when dealing with polarization density matrices $n=2,3$, $P_c=1$ corresponds to a circularly polarized pure state [6], characterized by the fact that its spin density vector takes its maximum achievable magnitude (maximal degree of circular polarization) and lies along the intrinsic axis $X_{3O}$, normal to the plane $X_{1O}{X}_{2O}$ containing the polarization ellipse of the state.
• Pure states are characterized by $1=P_{nD}^2=P_p^2+n{P}_c^2/\left[2\left(n-1\right)\right]$, where the full purity is reached through the balanced contributions of the degrees of population and correlation asymmetry, showing that the concept of purity of a state is identified with such a composition of asymmetries, while, as expected, the symmetry appears as a result of the randomness. An analysis of these features for the case $n=3$ can be found in [38]. For a pure state ($P_{nD}=1$, i.e., $P_1=P_2=\cdots =P_{n-1}=1$), ${\rho }_O$ has the generic form

  $${\mathsf{\rho }}_{Op}=\frac{1}{2}\left(\begin{array}{ccccc}1+cos2\chi & -isin2\chi & 0& \dots & 0\\ isin2\chi & 1-cos2\chi & 0& \dots & 0\\ 0& 0& 0& \dots & 0\\ ⋮& ⋮& ⋮& \ddots & ⋮\\ 0& 0& 0& 0& 0\end{array}\right),\left[\begin{array}{c}-\pi /4\le \chi \le \pi /4\\ M_1=cos2\chi ,P_c=sin2\chi \end{array}\right],$$

  (22)

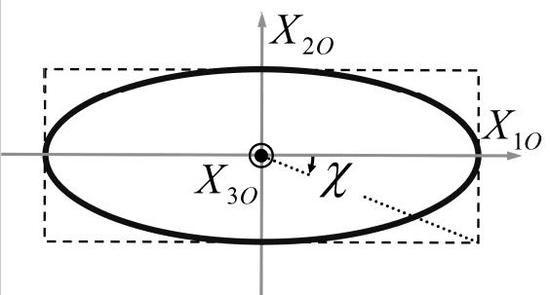
  in such a manner that all the physical information is concentrated into the 2×2 upper-left corner of the intrinsic density matrix, with $M_k=1$ $\left(k=2,3,\dots ,n-1\right)$ and $1=M_1^2+P_c^2$. Thus, a pure state admits a simple representation with respect to its effective intrinsic reference frame $X_{1O}{X}_{2O}{X}_{3O}$ through the intrinsic polarization ellipse (where the term polarization is used in the generic sense of asymmetry and not specifically for polarized light) (Figure 1). The only free parameter of a pure state in its intrinsic representation is the ellipticity angle χ, which determines the shape of the polarization ellipse. In particular, $\chi =0$ for linearly polarized states $\left(M_1=1, P_c=0\right)$, $\chi =\mp \pi /4$ for left-handed/right-handed (anticlockwise/clockwise handedness from the point of view of the receiver) circularly polarized states $\left(M_1=0, P_c=1\right)$ respectively.

Thus, any pure state can be represented with respect to its effective intrinsic reference frame $X_{1O}{X}_{2O}{X}_{3O}$ and exhibits a well-defined handedness H, given by $H\equiv \chi /\left|\chi \right|=\pm 1$ (with the convention $H=0$ for $\chi =0$), $H=+1$ for right-handed states and $H=-1$ for left-handed ones. Observe that H is an intrinsic property that involves chirality. In fact, by considering the spectral decomposition (1) of a density matrix ρ, all the eigenstates $u_k$ have well-defined and intrinsic respective handedness, so that it can properly be said that ρ involves intrinsic chiral properties associated with those of its eigenstates. For a given pure state $u_k$ its chirality vanishes when $\chi =0$, while from an overall point of view, ρ carries non-vanishing chirality as long as at least one of the eigenstates $u_k$ has nonzero spin (i.e., ${\chi }_k\ne 0$, or, equivalently $P_c\left(u_k\right)\ne 0$). The features of the ellipticity angles and the associated spin vectors of generic sets $\left(u_1,u_2,u_3\right)$ of orthonormal states for 3D polarization density matrices have been studied in [40].

As the larger IP, $M_{n-1}\ge M_{n-2}\ge \cdots$, take consecutively their maximal values equal to 1, the effective dimensions of the intrinsic density matrix are reduced correspondingly from n to r (with $r\equiv \mathrm{rank}\rho$), $n-r$ is the number of IPP equal to 1 ($1=P_{n-1}=P_{n-2}=\dots =P_r$), that is to say, the last $n-r$ intrinsic populations $a_n,a_{n-1},\dots a_{r+1}$ become zero, while the elements of the last $n-r$ rows and columns of ρ also become zero.

8. The Concept of Nonregularity of a Density Matrix

The discriminating decomposition (7) of a density matrix ρ is formulated as a convex composition of three density matrices, namely (1) the pure component ${\rho }_p$, whose intrinsic form ${\rho }_{pO}$ has been described in Equation (22); (2) the symmetric component ${\rho }_u=I_n/n$ (also called fully random, or unpolarized component), and (3) the discriminating component ${\rho }_d$. In general, ${\rho }_d$ has a complicated structure, and it can have different interesting forms. When ${\rho }_d$ is a real matrix, then it can be expressed as a weighted sum of density matrices, each one corresponding to a respective equiprobable mixture of a number of, 2, 3,… and n-1 mutually orthogonal pure states with zero spin

$$\begin{array}{c}{\mathsf{\rho }}_d={\displaystyle \sum _{k=2}^{n-1}}\left(P_k-P_{k-1}\right)\mathbf{Q}{\mathbf{D}}_k{\mathbf{Q}}^{†}=\mathbf{Q}\left[{\displaystyle \sum _{k=2}^{n-1}}\left(P_k-P_{k-1}\right){\mathbf{D}}_k\right]{\mathbf{Q}}^{†},\\ {\mathbf{D}}_k\equiv \frac{1}{k}diag\left(\underset{k}{\underbracket{1,1,\dots ,1}},\underset{n-k}{\underbracket{0,\dots ,0}}\right),\end{array}$$

(23)

where the diagonalization matrix Q is orthogonal. Observe that the fact that ${\rho }_d$ is a real matrix, (hence it is symmetric and can be diagonalized through the orthogonal matrix Q) does not imply that the matrix U that diagonalizes ρ is necessarily a real matrix; that is, there are cases for which there is degeneracy for certain eigenvalues of ${\rho }_d$ so that it can be diagonalized either by means of U (in general, complex-valued) or Q (real-valued). In summary, from Equation (7), ${\rho }_d$ is in general complex-valued (in which case U is not real-valued), so that we can distinguish between regular states, defined as those where either $P_1=P_{n-1}$ (in which case the coefficient of ${\rho }_d$ in the discriminating decomposition (7) vanishes), or ${\rho }_d$ is a real matrix, and nonregular states, for which $P_1\ne P_{n-1}$ and $\mathrm{Im}{\rho }_d\ne 0$ [7,41]. Thus, nonregularity occurs when the correlation asymmetry of ${\rho }_d$ is nonzero, $P_c\left({\rho }_d\right)>0$, while regularity appears as the limiting situation where $P_c\left({\rho }_d\right)=0$ (or, alternatively, where the equality $P_1=P_{n-1}$ is satisfied). Consequently, the maximal achievable value for $P_c\left({\rho }_d\right)$ should be inspected in order to define a generalized degree of nonregularity (note that the version for $n=3$ was already defined for density polarization matrices in previous work [41]).

The discriminating density matrix ${\rho }_d$ can be represented with respect to its own intrinsic reference frame $X_{1d},X_{2d},\dots X_{nd}$ (observe that, in general, $X_{d1},X_{d2},\dots X_{dn}$ is different from the intrinsic reference frame $X_{1O},X_{2O},\dots X_{nO}$ of ρ). The off-diagonal elements of the intrinsic form ${\rho }_{dO}=\mathrm{diag}\left(a_{d1},\dots ,a_{dn}\right)+\left(i/2\right) N_{dO}$ are purely imaginary and their absolute values, together with the populations $a_{dk}$, are limited by the constraints of nonnegativity of all principal minors of ${\rho }_{dO}$, in such a manner that the maximization of $P_c\left({\rho }_{dO}\right)=\left(1/\sqrt{2}\right){\Vert N_{dO}\Vert }_2$ requires that ${\rho }_{dO}$ has the form

$${\mathsf{\rho }}_{dO}=\frac{1}{2}\left(\begin{array}{cccccc}1& 0& 0& 0& \cdots & 0\\ 0& 1/2& -i/2& 0& \cdots & 0\\ 0& i/2& 1/2& 0& \cdots & 0\\ 0& 0& 0& 0& \cdots & 0\\ ⋮& ⋮& ⋮& ⋮& \ddots & ⋮\\ 0& 0& 0& 0& \cdots & 0\end{array}\right)$$

(24)

and therefore, $P_c\left({\rho }_{dO}\right)\le 1/2$. Since $P_c\left({\rho }_{dO}\right)$ is invariant under orthogonal similarity transformations of ${\rho }_{dO}$ it follows that $P_c\left({\rho }_{dO}\right)=P_c\left({\rho }_d\right)$ and we deduce that the degree of correlation asymmetry of the discriminating component is limited by $0\le P_c\left({\rho }_d\right)\le 1/2$, where the minimum $P_c\left({\rho }_d\right)=0$ corresponds to regular states, and otherwise, ρ is a nonregular state, with a maximum $P_c\left({\rho }_d\right)=1/2$ for states with maximal nonregularity, hereafter called perfect nonregular states.

The above results support the definition of the degree of nonregularity $P_N\left({\rho }_d\right)$ of a discriminating state ${\rho }_d$ as $P_N\left({\rho }_d\right)\equiv 2 P_c\left({\rho }_d\right)$, with $P_N\left({\rho }_d\right)=0$ for regular discriminating states and $P_N\left({\rho }_d\right)=1$ for perfect nonregular discriminating states, and by considering the weight $\left(P_{n-1}-P_1\right)$ of the component ${\rho }_d$ in the discriminating decomposition (7), we define the degree of nonregularity of a density matrix ρ as

$$P_N\left(\mathsf{\rho }\right)\equiv 2\left(P_{n-1}-P_1\right)P_c\left({\mathsf{\rho }}_d\right),$$

(25)

so that regular states are those that either satisfy $P_{n-1}=P_1$ or whose discriminating component is regular (i.e., ${\rho }_d$ lacks correlation asymmetry), while the degree of nonregularity of ρ is that of ${\rho }_d$ but scaled by the coefficient $P_{n-1}-P_1$, and therefore ρ represents a perfect nonregular state $\left(P_N\left(\rho \right)=1\right)$ when ρ itself has the form of a discriminating density matrix $\rho ={\rho }_d$ with $P_N\left({\rho }_d\right)=1$.

As discussed in Section 7, nonzero correlation asymmetry of a given density matrix ρ involves necessarily certain chirality associated with the eigenstates of ρ, and by noting that the existence of nonzero correlation asymmetry of the discriminating component ${\rho }_d$ of ρ implies nonregularity, we find an interesting and subtle link between the concepts of chirality and nonregularity of a given ρ. Observe also that the complete interval of values $0\le P_c\left(\rho \right)\le 1$ is achievable for regular states; in particular $P_c\left(\rho \right)=0$ when ρ is a real matrix (hence regular) and $P_c\left(\rho \right)=1$ when ρ represents a circularly polarized pure state. Thus, $P_c\left(\rho \right)$ may be interpreted as a measure of the degree of chirality of the state ρ, while the degree of chirality $P_c\left({\rho }_d\right)$ of the discriminating component determines the degree of nonregularity of ρ.

The definition of $P_N$ generalizes the results already obtained for the case $n=3$ in previous works dealing with polarization density matrices [7,41], which have been successfully applied to the characterization of polarization of evanescent waves [42] and tightly focused fields [43].

9. Discussion and Conclusions

There are many problems in physics whose formulation becomes strongly simplified when a specific reference frame is used. A well-known case is the definition of the tangential and normal components of the acceleration associated with the classical motion of a particle by means of the choice of an intrinsic coordinate system for each point of the trajectory. In this work, the definition of an intrinsic coordinate system for each given n-dimensional density matrix ρ is exploited in order to define a set of quantities (the components of purity -CP- of the state) that provide complete information on the rotational invariant properties associated with ρ in a hierarchical and meaningful manner. In fact, it is found that the CP coincide, up to respective simple coefficients, with the n-dimensional Stokes parameters of the state in its intrinsic representation. These results generalize the obtainment of the six intrinsic Stokes parameters for 3D polarization states, whose physical interpretation is as simple as the intensity, the degree of linear polarization, the degree of directionality and the three intrinsic components of the spin density vector of the state [6,37]. Thus, any polarization density matrix can be conceived as the intrinsic one (linked in a very simple way to the intrinsic Stokes parameters) and a spatial rotation of the Cartesian reference frame (three angular parameters).

In the general case of a density matrix ρ representing an n-dimensional mixed state, which depends on up to $n^2-1$ free parameters, the situation is more involved than for 2D or 3D density matrices and the generalization is not straightforward; in fact, as described in Section 5, when $n>3$ the imaginary parts of the off-diagonal elements of ρ cannot be longer interpreted as the components of the spin density vector.

Regarding the invariants of ρ with respect to unitary similarity transformations $U \rho U^{†}$, a parametrization based on the $n-1$ indices of purity (IPP) was defined in previous work [13]. The IPP, $P_k$ $\left(k=1,\dots ,n-1\right)$ are constrained by the nested inequalities $0\le P_1\le \dots \le P_{n-1}$ and provide, in an optimal and hierarchical manner, quantitative (but not qualitative) information on the structure of purity of ρ. In fact, the degree of purity $P_{nD}$ (which represents the degree of statistical asymmetry) can be obtained as a weighted square average of the IPP.

Nevertheless, more detailed and qualitative information can be obtained from ρ through the introduction of the concept of intrinsic density matrix, which leads to the definition of a number of l (with $l=\left[n\left(n+1\right)/2\right]-1$) invariants of ρ with respect to arbitrary rotations of the n-dimensional Cartesian reference frame, i.e., with respect to orthogonal similarity transformations $Q \rho Q^{\mathrm{T}}$. In this case, the total number l of invariants defined in this work and called the components of purity (CP) of ρ, can be grouped into two meaningful sets, namely (a) the $n-1$ indices of population asymmetry (IP), $M_k$ $\left(k=1,\dots ,n-1\right)$, (or, equivalently, the intrinsic population-Stokes parameters) which are constrained by the nested inequalities $0\le M_1\le \dots \le M_{n-1}\le 1$ and provide, in an optimal and hierarchical manner, complete information on the structure of population asymmetry of ρ, and (b) the $n\left(n-1\right)/2$ intrinsic coherences (IC), $n_{ij}$ $\left(i,j=1,\dots ,n; i<j\right)$, (or, equivalently, the intrinsic correlation-Stokes parameters) that provide complete information on the correlation asymmetry of ρ.

From these sets of parameters, two complementary sources of purity, namely the degree of population asymmetry, $P_p$, and the degree of correlation asymmetry, $P_c$, are defined as respective square averages, in such a manner that $P_{nD}$ is, in turn, a weighted square average of $P_p$ and $P_c$. All the above descriptors are used to analyze the peculiar features of the discriminating decomposition of a given density matrix ρ into a convex sum (or incoherent superposition) of three density matrices, namely (1) a pure one, (2) a maximally mixed one, and (3) a discriminating one that holds certain critical properties of ρ and leads to the definition of the degree of nonregularity, which is determined by the degree of correlation asymmetry of the discriminating component, scaled by the difference $P_{n-1}-P_1$ between the maximum and minimum IPP of ρ.

It should be stressed that, through the diagonalization of the intrinsic density matrix, the $n-1$ IPP can be calculated from the $l=\left[n\left(n+1\right)/2\right]-1$ components of purity, which agrees with the obvious fact that orthogonal matrices constitute a type of unitary matrices (those that are real-valued), i.e., all invariants under transformations $U \rho U^{†}$ (with U unitary), are invariants under $Q \rho Q^{\mathrm{T}}$ (with Q orthogonal). Therefore, the set of CP is complete, because parametrizes all the l indicated rotational invariants of ρ. Furthermore, the CP (hence, the intrinsic Stokes parameters) are physically meaningful because they satisfy the following properties

(a)

As shown in Equation (20), the degree of purity $P_{nD}$ is given by a weighted square average of the CP. The degrees of population and correlation asymmetry constitute two complementary sources of purity. Full population asymmetry $P_P=1$ entails full purity $\left(P_{nD}=1\right)$ and zero correlation asymmetry $\left(P_c=0\right)$, while full correlation asymmetry $P_c=1$ implies full purity together with a certain amount of population asymmetry $P_p=\sqrt{\left(n/2-1\right)/\left(n-1\right)}$, in which case the state can be represented by a circularly polarized state embedded into an n-dimensional space.

(b)

The $n-1$ indices of population asymmetry $M_k$ are defined in a hierarchical manner $\left(0\le M_1\le \dots \le M_{n-1}\le 1\right)$, so that $M_{n-1}=0$ implies that the state is maximally mixed, while $M_1=1$ implies full population asymmetry (or population purity) $P_p=1$ and full overall purity $P_{nD}=1$, in which case the state can be represented by a linearly polarized state embedded into an n-dimensional space.

(c)

The $n\left(n-1\right)/2$ intrinsic coherences $n_{ij}$ hold all information on the correlations among the random variables that describe the system. Their values are constrained by those of $M_k$ because of the nonnegativity of the principal minors of ρ. Moreover, the inherent chirality associated with the degree of correlation asymmetry, which has its origin in the handedness of the eigenstates of ρ, has been analyzed and characterized.

(d)

All the information contained in ρ ($n^2-1$ free parameters) can be parametrized by an n-dimensional rotation (non-invariant $n\left(n-1\right)/2$ angular parameters) together with the $n-1$ indices of population asymmetry and the $n\left(n-1\right)/2$ intrinsic coherences of ρ.

The general framework introduced provides invariant descriptors for n-dimensional density matrices that, in turn, have been proven to be fruitful for the study of three-dimensional states of polarization [4,6,7,13,23,24,27,28,30,36,37,38,40,41,42,43,44,45,46,47,48] and of the polarimetric properties of material media [4,5,8,13,23,24,25,32,33,34,35,49,50,51], which supports a well-founded expectation for its useful application to n-dimensional density matrices representing quantum or classical systems.