Analysis of Fractal Structures in Dehydrated Films of Protein Solutions
Abstract
:1. Introduction
2. Materials and Methods
3. Experiments
3.1. Formation of Dehydrated Films of Proteins
3.2. Box-Counting Dimension
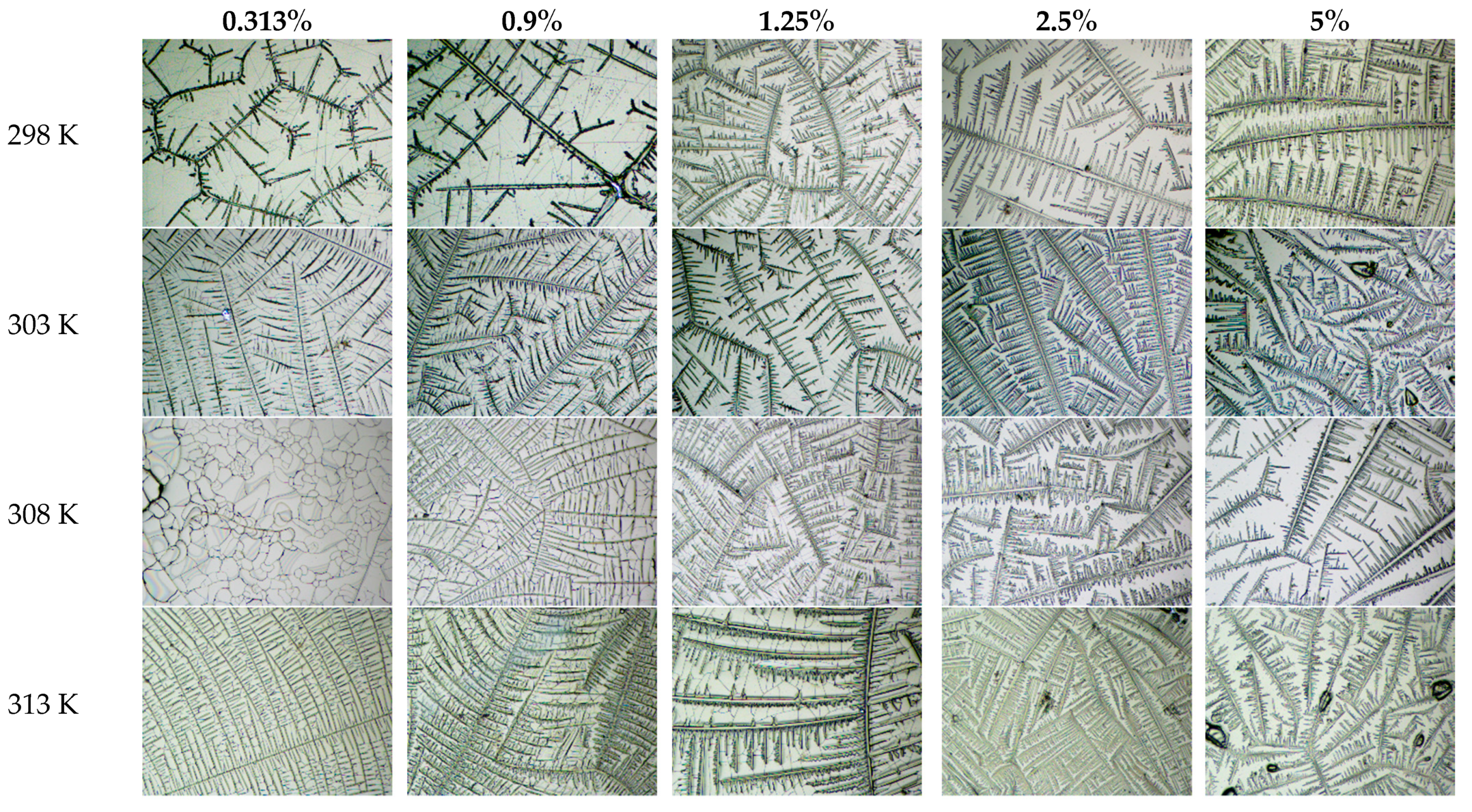
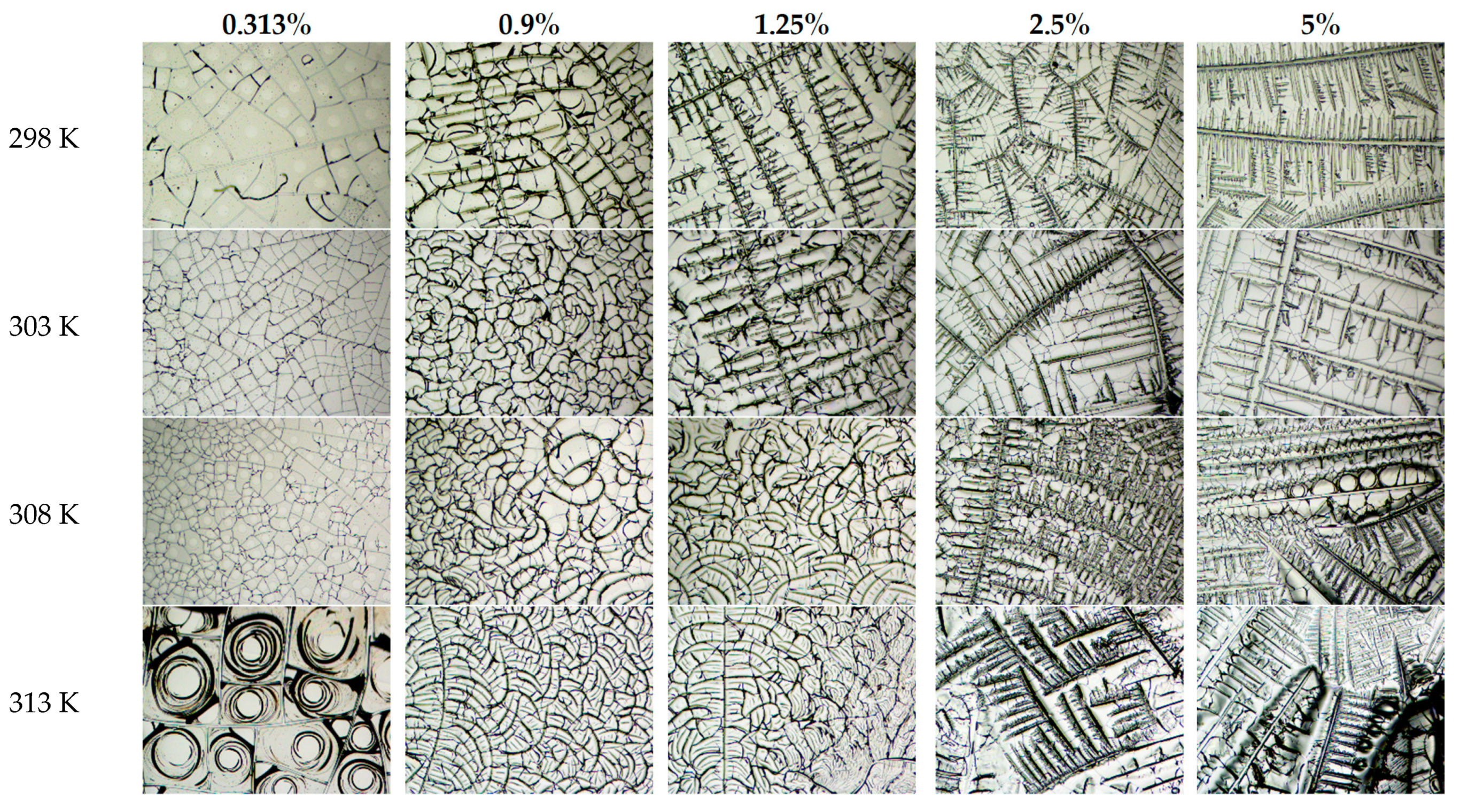
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lotnyk, A.; Behrens, M. Phase change thin films for non-volatile memory applications. Nanoscale Adv. 2019, 1, 3836–3857. [Google Scholar] [CrossRef] [Green Version]
- Liu, J. Surface-supported metal–organic framework thin films: Fabrication methods, applications, and challenges. Chem. Soc. Rev. 2017, 46, 5730–5770. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Fan, Z.; Xiao, D.; Zhu, J. Multiferroic bismuth ferrite-based materials for multifunctional applications: Ceramic bulks, thin films and nanostructures. Prog. Mater. Sci. 2016, 84, 335–402. [Google Scholar] [CrossRef] [Green Version]
- Fu, Y.; Luo, J.; Nguyen, N. Advances in piezoelectric thin films for acoustic biosensors, acoustofluidics and lab-on-chip applications. Prog. Mater. Sci. 2017, 89, 31–91. [Google Scholar] [CrossRef] [Green Version]
- Tang, Y.; Li, P.; Liao, W.; Shi, P. Multiaxial molecular ferroelectric thin films bring light to practical applications. J. Am. Chem. Soc. 2018, 140, 8051–8059. [Google Scholar] [CrossRef] [PubMed]
- Yokota, T.; Kajitani, T.; Shidachi, R. A few-layer molecular film on polymer substrates to enhance the performance of organic devices. Nat. Nanotechnol. 2018, 13, 139–144. [Google Scholar] [CrossRef] [PubMed]
- Maltesen, M. Drying methods for protein pharmaceuticals. Drug Discov. Today: Technol. 2008, 5, e81–e88. [Google Scholar] [CrossRef]
- Bellich, B.; Elisei, E.; Heyd, R.; Saboungi, M.L.; Cesàro, A. Isothermal dehydration of thin films: Calorimetric assessment of model parameters. J. Therm. Anal. Calorim. 2015, 121, 963–973. [Google Scholar] [CrossRef]
- Alessandrini, A.; Gerunda, M.; Facci, P. Tuning molecular orientation in protein films. Surf. Sci. 2003, 542, 64–71. [Google Scholar] [CrossRef]
- Yang, A.; Li, Y.; Yang, C.; Fu, Y.; Wang, N.; Li, L.; Yan, F. Fabric organic electrochemical transistors for biosensors. Adv. Mater. 2019, 30, 1800051. [Google Scholar] [CrossRef]
- Wang, N.; Yang, A.; Fu, Y.; Li, Y.; Yan, F. Functionalized organic thin film transistors for biosensing. Acc. Chem. Res. 2019, 52, 277–287. [Google Scholar] [CrossRef]
- Jia, X.; Fuentes-Hernandez, C.; Wang, C.Y.; Park, Y.; Kippelen, B. Stable organic thin-film transistors. Sci. Adv. 2018, 4, eaao1705. [Google Scholar] [CrossRef] [Green Version]
- He, Z.; Chen, J.; Li, D. Polymer additive controlled morphology for high performance organic thin film transistors. Soft Matter 2019, 15, 5790–5803. [Google Scholar] [CrossRef] [PubMed]
- Velichko, E.N.; Baranov, M.A.; Mostepanenko, V.M. Change of sign in the Casimir interaction of peptide films deposited on a dielectric substrate. Mod. Phys. Lett. A 2020, 35. [Google Scholar] [CrossRef]
- Velichko, E.; Nepomnyashchaya, E.; Baranov, M. Study of Self-assembled Molecular Films as a Method of Search for Promising Materials in Nanoelectronics and Nanocommunications. In Internet of Things, Smart Spaces, and Next Generation Networks and Systems, Proceedings of the Lecture Notes in Computer Science (Including Subseries Lecture Notes in Artificial Intelligence and Lecture Notes in Bioinformatics); Springer: Berlin/Heidelberg, Germany, 2018; Volume 11118, pp. 691–701. [Google Scholar]
- Baranov, M.A.; Dudina, A.I.; Nepomnyaschaya, E.K. Optical analysis of protein-metal interactions. J. Phys. Conf. Ser. 2019, 1226. [Google Scholar] [CrossRef]
- Baranov, M.; Velichko, E.; Andryakov, A. Image Processing for Analysis of Bio-Liquid Films. Opt. Mem. Neural Netw. 2020, 29, 1–6. [Google Scholar] [CrossRef]
- Baranov, M.; Velichko, E.; Shariaty, F. Determination of Geometrical Parameters in Blood Serum Films Using an Image Segmentation Algorithm. Opt. Mem. Neural Netw. 2020, 29, 330–335. [Google Scholar] [CrossRef]
- Baranov, M.A. Image Processing of Biological Liquids Films for Medical Diagnostics. J. Electron. Sci. Technol. 2020, 18, 100027. [Google Scholar] [CrossRef]
- Madison, A. Symmetry of quasicrystals. Phys. Solid State 2013, 55, 855–867. [Google Scholar] [CrossRef]
- Shabalin, V.; Shatokhina, S. Diagnostic markers in the structures of human biological liquids. Singap. Med. J. 2007, 48, 440–446. [Google Scholar]
- Shatokhina, S.N.; Shabalin, V.N.; Buzoverya, M.E.; Punin, V.T. Bio-liquid morphological analysis. Sci. World J. 2004, 4, 657–661. [Google Scholar] [CrossRef] [Green Version]
- Shatokhina, S.N.; Zakharova, N.M.; Dedova, M.G.; Sambulov, V.I.; Shabalin, V.N. Morphological marker of tumor progression in laryngeal cancer. Vopr. Onkol. 2013, 59, 66–70. [Google Scholar] [PubMed]
- Hernández, N.; Hansen, W.; Zhu, D.; Shea, M. Stimulus-responsive self-assembly of protein-based fractals by computational design. Nat. Chem. 2019, 11, 605–614. [Google Scholar] [CrossRef] [PubMed]
- Andoyo, R.; Lestari, V.D.; Mardawati, E. Fractal dimension analysis of texture formation of whey protein-based foods. Int. J. Food Sci. 2018, 1–17. [Google Scholar] [CrossRef] [PubMed]
- MacHado, C.A.; Bentz, K.C.; Tran, R.; Jenkins, T.A.; Barnes, B.E.; Diodati, L.E.; Savin, D.A. Hierarchical Fractal Assemblies from Poly(ethylene oxide- b-lysine- b-leucine). Biomacromolecules 2019, 20, 2557–2566. [Google Scholar] [CrossRef] [PubMed]
- Kumar Rout, R.; Pal Choudhury, P.; Prasad Maity, S.; Daya Sagar, B.S.; Sarif Hassan, S. Fractal and mathematical morphology in intricate comparison between tertiary protein structures. Comput. Methods Biomech. Biomed. Eng. Imaging Vis. 2018, 6, 192–203. [Google Scholar] [CrossRef] [Green Version]
- Bhatia, S. Nanoparticles types, classification, characterization, fabrication methods and drug delivery applications. In Natural Polymer Drug Delivery Systems; Springer: Cham, Switzerland, 2016; pp. 33–93. ISBN 978-3-319-41129-3. [Google Scholar]
- Malekzad, H.; Mirshekari, H.; Sahandi Zangabad, P.; Moosavi Basri, S.M.; Baniasadi, F.; Sharifi Aghdam, M.; Hamblin, M.R. Plant protein-based hydrophobic fine and ultrafine carrier particles in drug delivery systems. Crit. Rev. Biotechnol. 2018, 38, 47–67. [Google Scholar] [CrossRef] [PubMed]
- Piacentini, E.; Dragosavac, M.; Giorno, L. Pharmaceutical particles design by membrane emulsification: Preparation methods and applications in drug delivery. Curr. Pharm. Des. 2017, 23, 302–318. [Google Scholar] [CrossRef] [Green Version]
- Lazzari, S.; Nicoud, L.; Jaquet, B. Fractal-like structures in colloid science. Adv. Colloid Interface Sci. 2016, 235, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Kumar, S.; Yadav, I.; Aswal, V.K.; Kohlbrecher, J. Structure and Interaction of Nanoparticle-Protein Complexes. Langmuir 2018, 34, 5679–5695. [Google Scholar] [CrossRef]
- Inthavong, W.; Kharlamova, A.; Chassenieux, C. Matter Structure and flow of dense suspensions of protein fractal aggregates in comparison with microgels. Soft Matter 2016, 12, 2785–2793. [Google Scholar] [CrossRef] [PubMed]
- Alexandrov, D.V.; Galenko, P.K. Dendrite growth under forced convection: Analysis methods and experimental tests. Physics-Uspekhi 2014, 57, 771–786. [Google Scholar] [CrossRef]
- Adrianov, V.E.; Maslov, V.G.; Baranov, A.V.; Fedorov, A.V.; Artem’ev, M.V. Spectral study of the self-organization of quantum dots during the evaporation of colloidal solutions. J. Opt. Technol. 2011, 78, 699. [Google Scholar] [CrossRef]
- Madaan, K.; Kumar, S.; Poonia, N.; Lather, V.; Pandita, D. Dendrimers in drug delivery and targeting: Drug-dendrimer interactions and toxicity issues. J. Pharm. Bioallied Sci. 2014, 6, 139–150. [Google Scholar] [CrossRef]
- Ruzhitskaya, D.D.; Ryzhikov, S.B.; Ryzhikova, Y.V. The Optical Properties of Fractal Nanodendrites in the Processes of Their Self-Organization. Moscow Univ. Phys. Bull. 2018, 73, 306–309. [Google Scholar] [CrossRef]
- Burkovets, D.; Optics, O.M. Modelling of light scattering by fractal clusters. In Proceedings of the Eighth International Conference on Correlation Optics, Chernivsti, Ukraine, 11–14 September 2007; Volume 7008, p. 700812. [Google Scholar] [CrossRef]
- Gridchina, V.; Korolenko, P.; Russian, Y.R. Scaling in the optical characteristics of nanocluster structures. Bull. Russ. Acad. Sci. Phys. 2015, 79, 1480–1483. [Google Scholar] [CrossRef]
- Khlyustova, A.; Cheng, Y.; Yang, R. Vapor-deposited functional polymer thin films in biological applications. J. Mater. Chem. B 2020, 8, 6588–6609. [Google Scholar] [CrossRef]
- Eisele, N.B.; Andersson, F.I.; Frey, S.; Richter, R.P. Viscoelasticity of thin biomolecular films: A case study on nucleoporin phenylalanine-glycine repeats grafted to a histidine-tag capturing QCM-D sensor. Biomacromolecules 2012, 13, 2322–2332. [Google Scholar] [CrossRef]
- Baranov, M.A.; Velichko, E.N.; Aksenov, E.T. Self-assembled biomacromolecular films as a basis for nonlinear optical devices. In Proceedings of the International Conference Laser Optics, Saint Petersburg, Russia, 4–8 June 2018; p. 356. [Google Scholar]







Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baranov, M.; Velichko, E.; Greshnevikov, K. Analysis of Fractal Structures in Dehydrated Films of Protein Solutions. Symmetry 2021, 13, 123. https://doi.org/10.3390/sym13010123
Baranov M, Velichko E, Greshnevikov K. Analysis of Fractal Structures in Dehydrated Films of Protein Solutions. Symmetry. 2021; 13(1):123. https://doi.org/10.3390/sym13010123
Chicago/Turabian StyleBaranov, Maksim, Elena Velichko, and Konstantin Greshnevikov. 2021. "Analysis of Fractal Structures in Dehydrated Films of Protein Solutions" Symmetry 13, no. 1: 123. https://doi.org/10.3390/sym13010123
APA StyleBaranov, M., Velichko, E., & Greshnevikov, K. (2021). Analysis of Fractal Structures in Dehydrated Films of Protein Solutions. Symmetry, 13(1), 123. https://doi.org/10.3390/sym13010123





