Total Effective Vascular Compliance of a Global Mathematical Model for the Cardiovascular System
Abstract
:1. Introduction
2. Materials and Methods
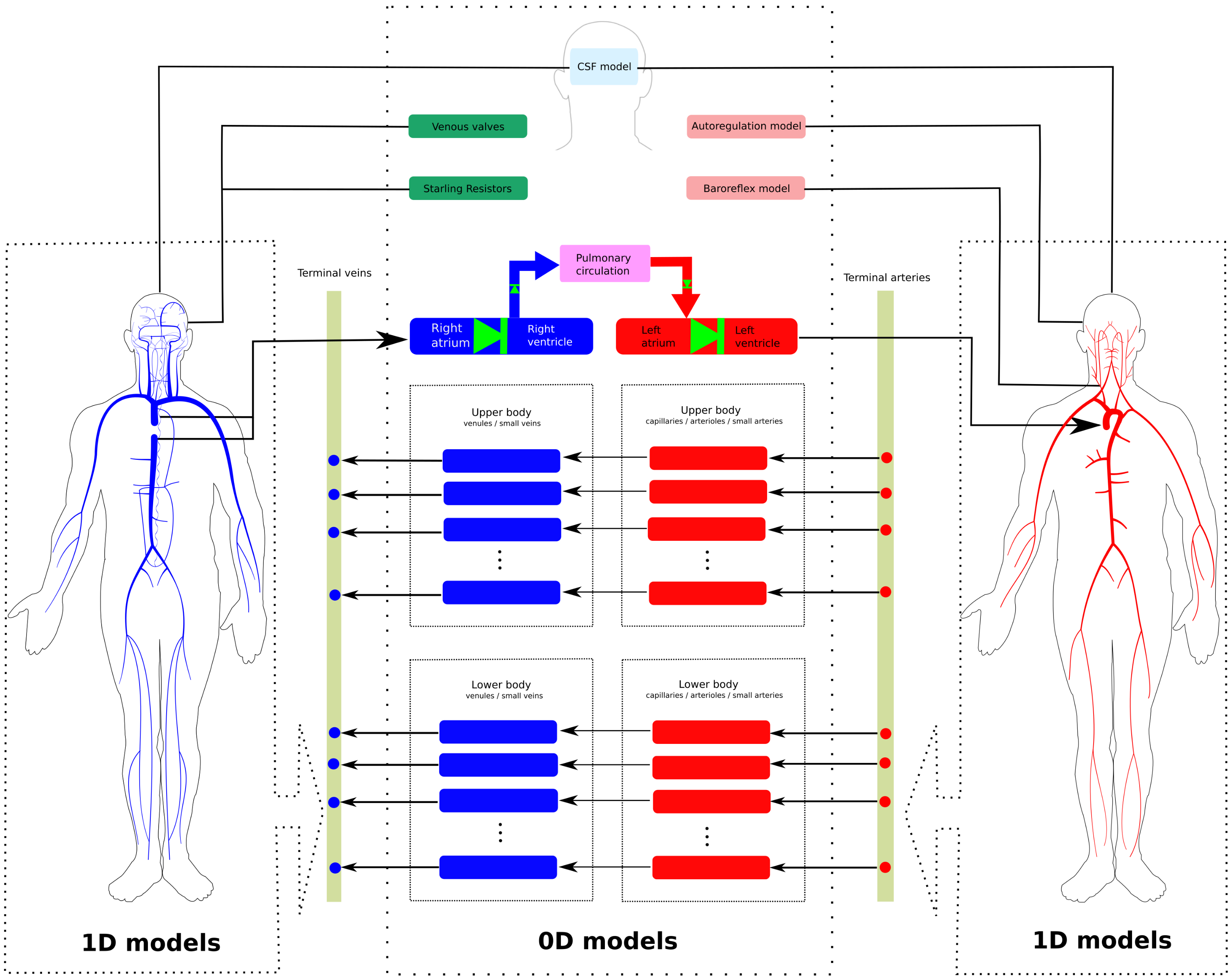
2.1. A Global Closed-Loop Model for the Human Circulation
- characteristic impedances that couple any number of connecting 1D arteries/veins to lumped-parameter models for the microvasculature ( or ) and regulate the pressure drop between 1D domains and vascular beds,
- peripheral resistances and compliances divided between arterioles and capillaries , and
- venous compartments with related compliances (), which represent venules and distal veins not included in the 1D network.
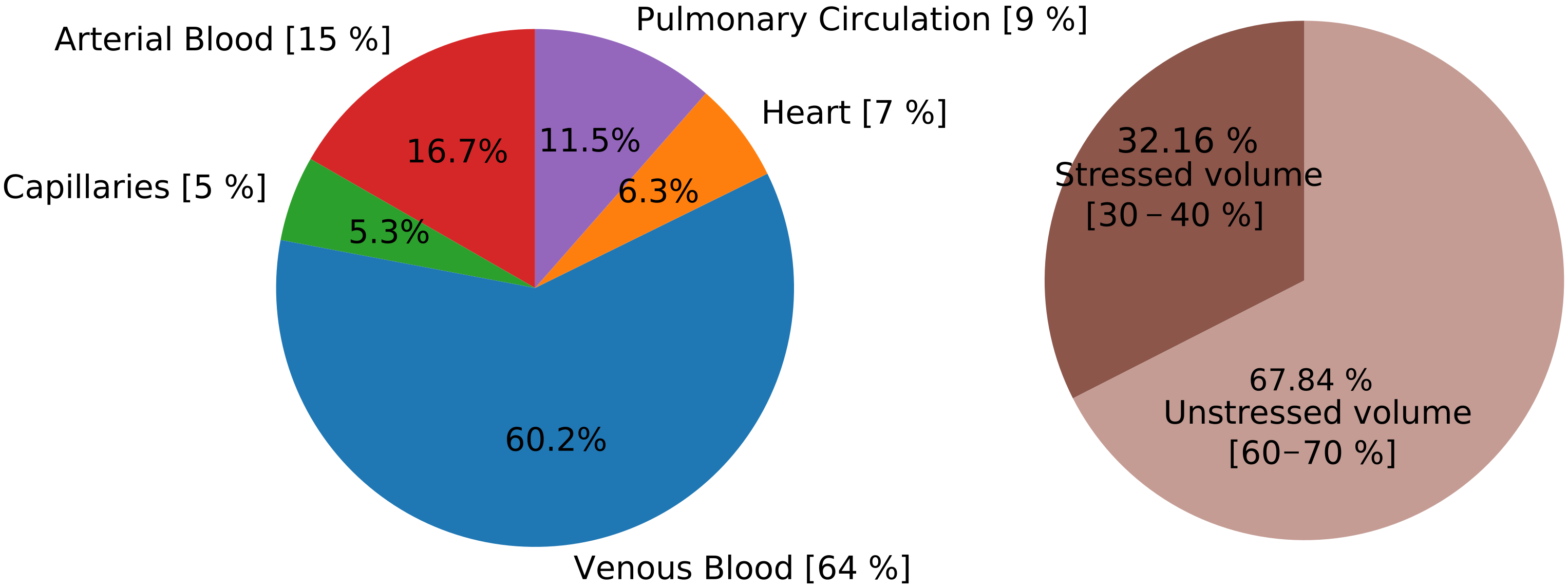
2.2. Compliances, Unstressed Volumes, and Total Blood Volume Distribution
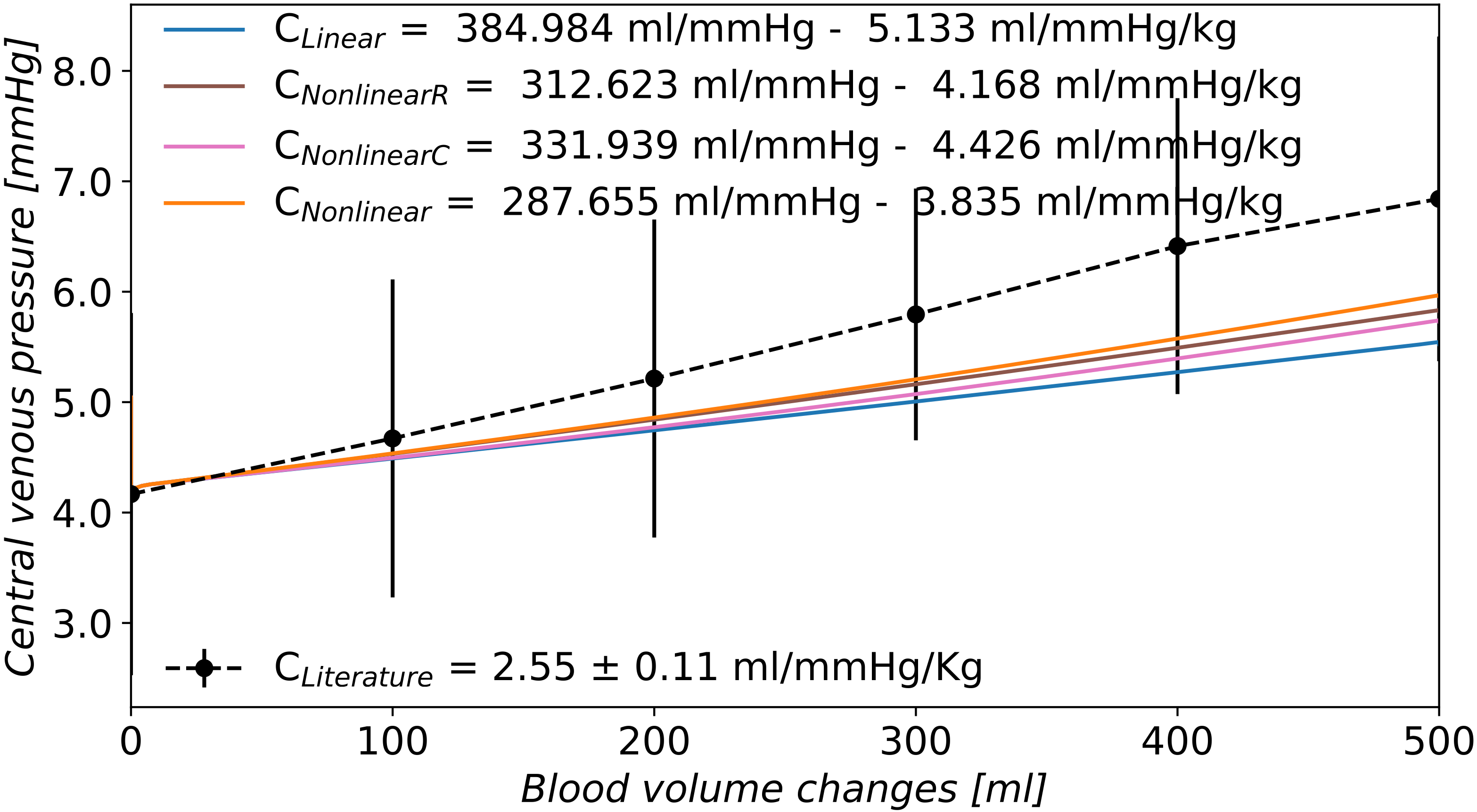
2.3. Nonlinear Venous Resistances and Compliances
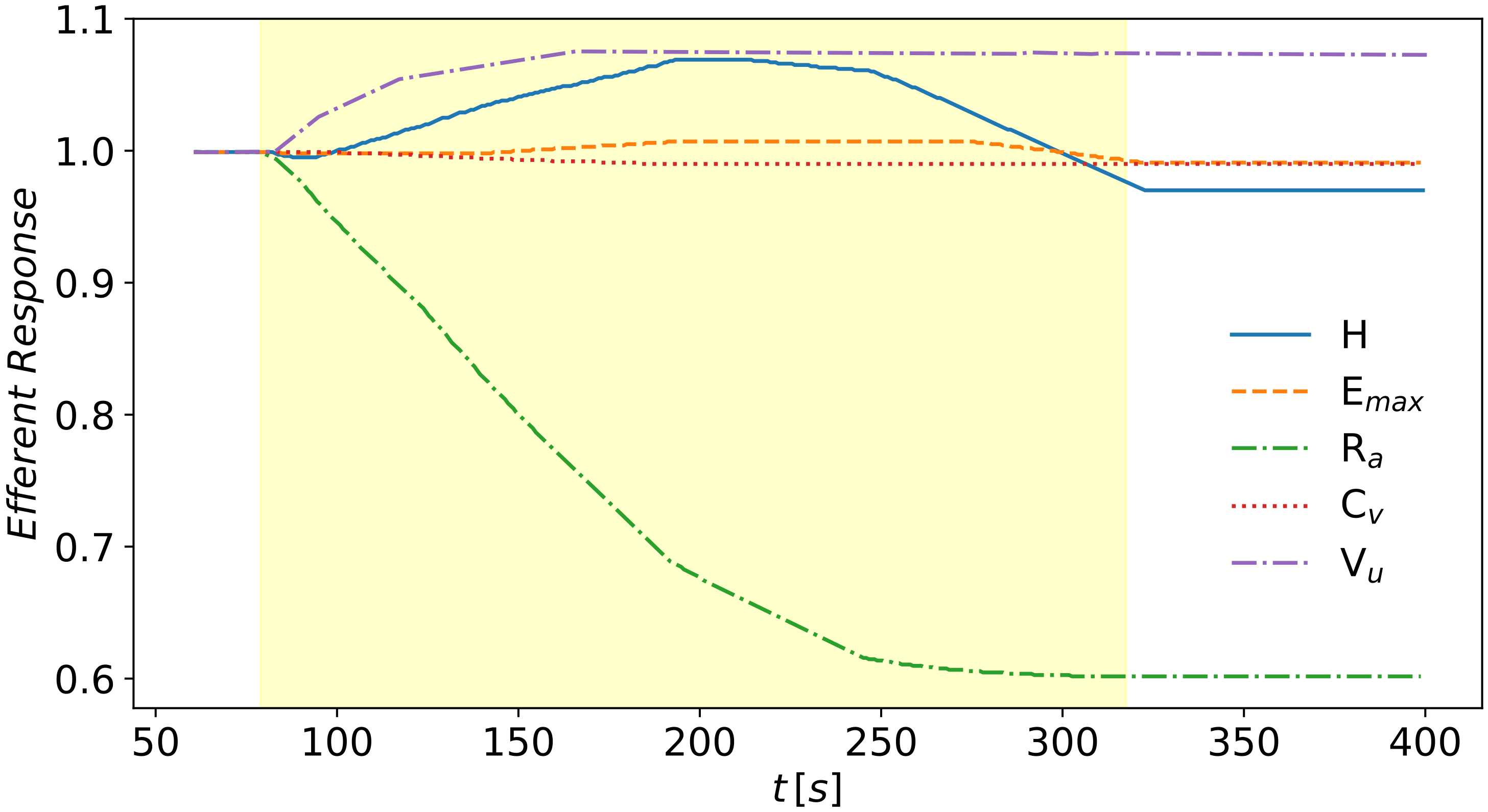
2.4. The Baroreflex Regulation
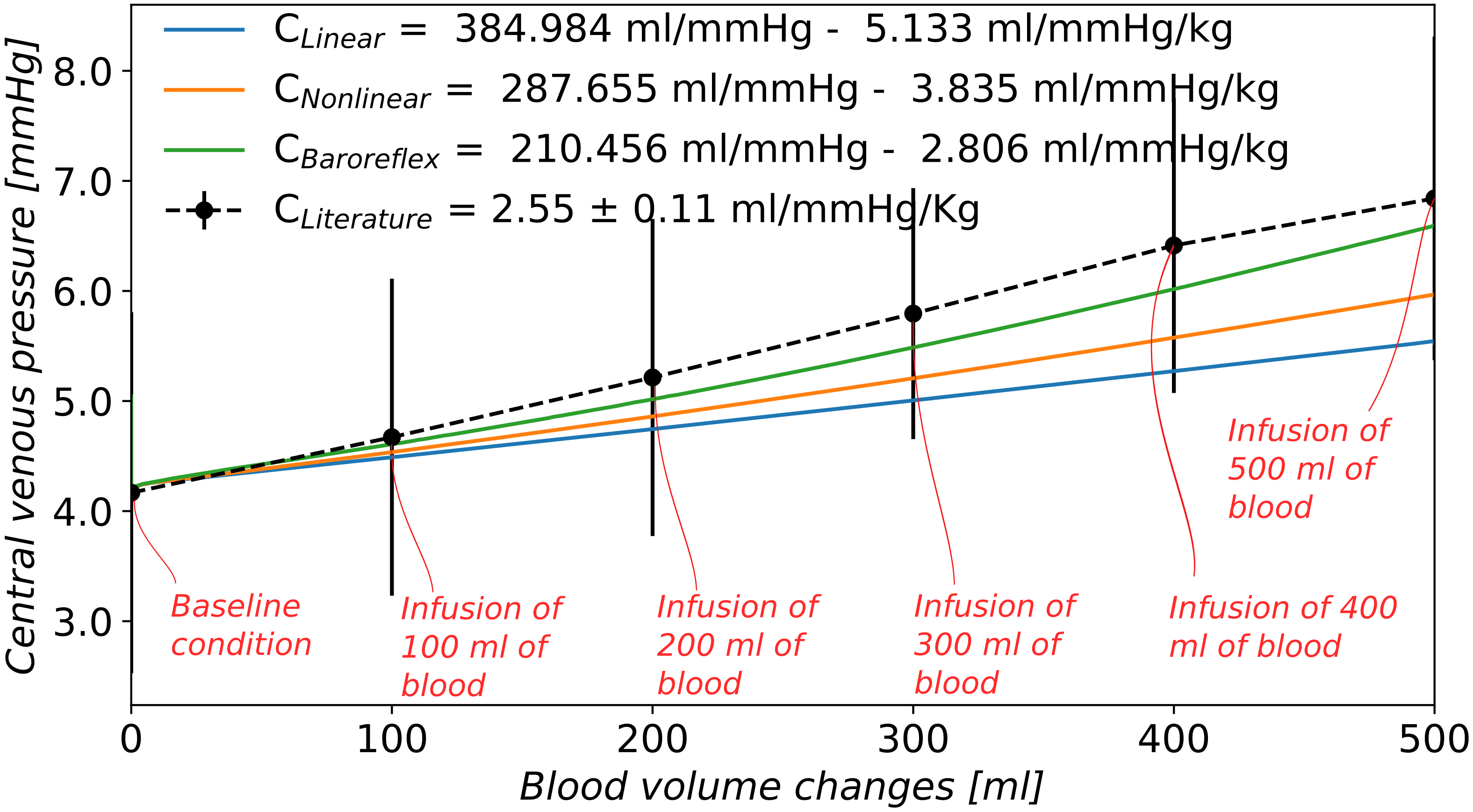
2.5. Determination of Total Effective Compliance
- (1)
- Linear case: linear resistances and compliances in 0D venous compartments were applied. The use of linear resistances implies that in Equation (7) during the entire simulation. With linear compliance, pressure in 0D venules compartments is evaluated with and in Equation (8); this is equivalent towhere is the unstressed volume of the linear pressure–volume relationship (see Figure 3) and is the basal value of compliance in Equation (8).
- (2)
- (3)
- Baroreflex case: in this case, the model presented in Section 2.4 was applied with parameters of Table 2 in conjunction with nonlinear resistances and compliances in venules/distal veins compartments.
3. Discussion
3.1. Control of Vascular Blood Volume
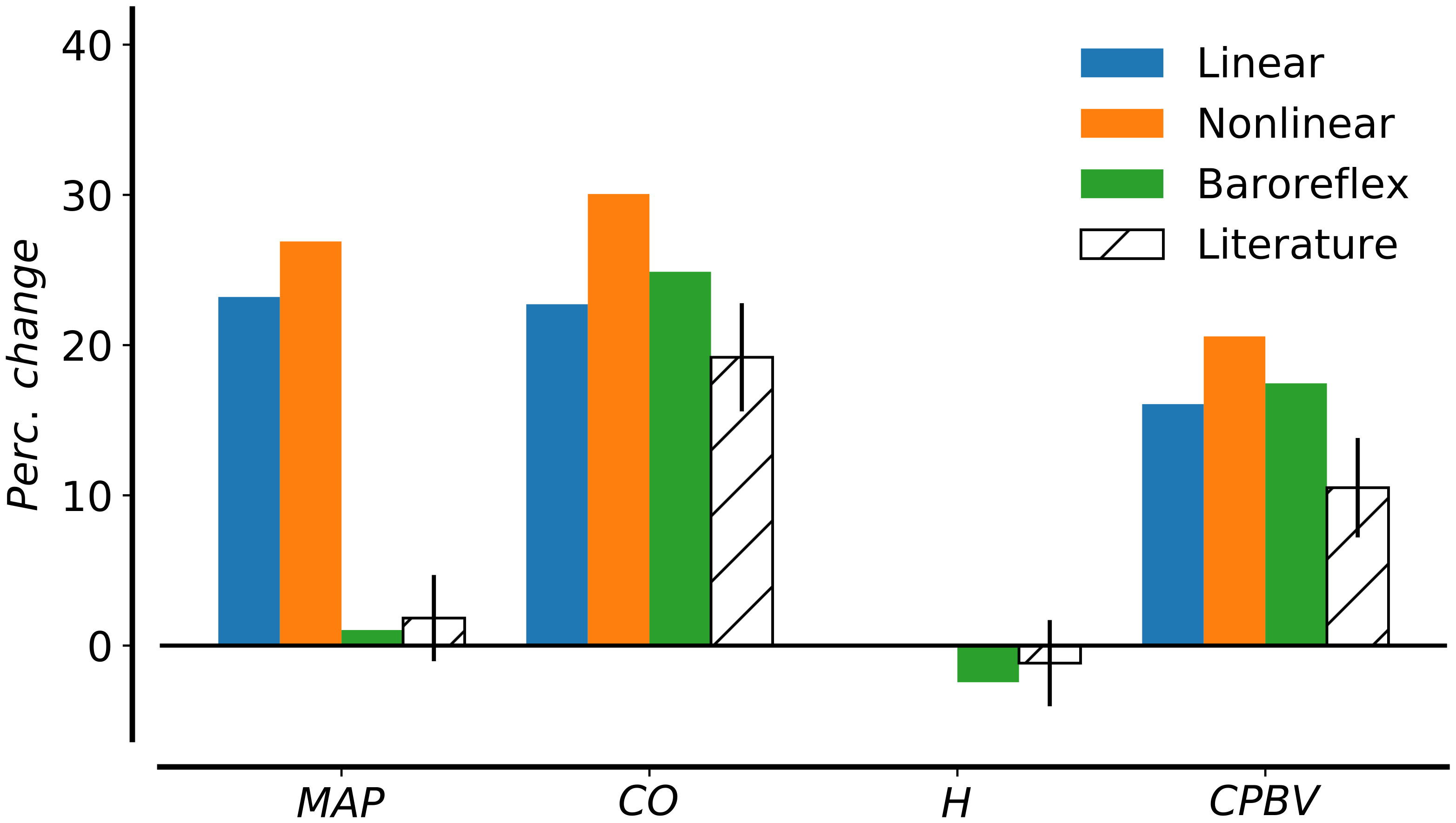
3.2. Nonlinearities in Venous Compartments
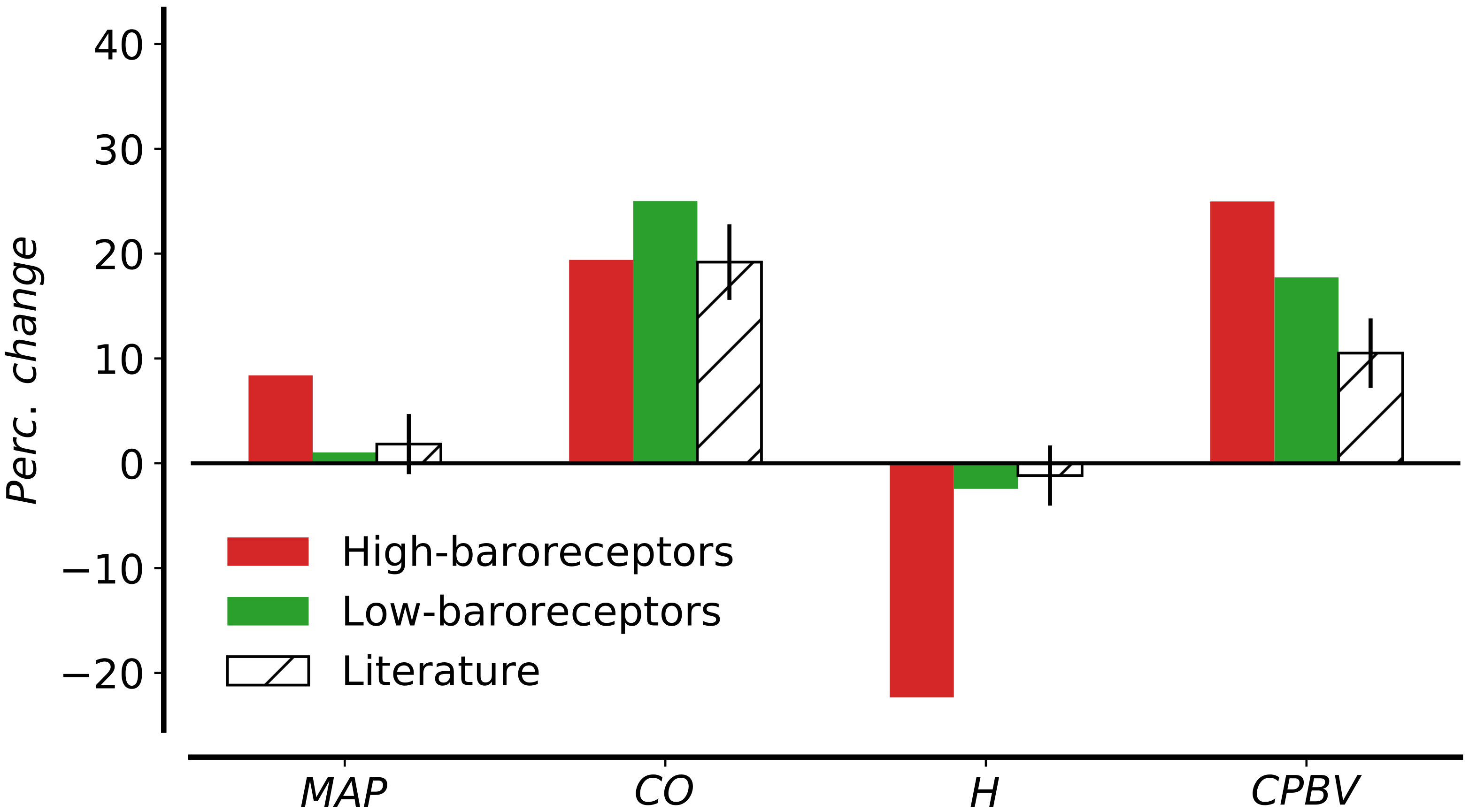
3.3. Baroreflex
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Safar, M.E.; London, G.M.; Simon, A.C.; Weiss, Y.A. (Eds.) Arterial and Venous Systems in Essential Hypertension. In Developments in Cardiovascular Medicine; Springer: Dordrecht, The Netherlands, 1987; Volume 63. [Google Scholar]
- Guyton, A.C.; Polizo, D.; Armstrong, G.G. Mean Circulatory Filling Pressure Measured Immediately after Cessation of Heart Pumping. Am. J. Physiol.-Leg. Content 1954, 179, 261–267. [Google Scholar] [CrossRef] [Green Version]
- Drees, J.A.; Rothe, C.F. Reflex Venoconstriction and Capacity Vessel Pressure-Volume Relationships in Dogs. Circ. Res. 1974, 34, 360–373. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Samar, R.E.; Coleman, T.G. Measurement of Mean Circulatory Filling Pressure and Vascular Capacitance in the Rat. Am. J. Physiol.-Heart Circ. Physiol. 1978, 234, H94–H100. [Google Scholar] [CrossRef] [PubMed]
- Shoukas, A.A.; Sagawa, K. Total Systemic Vascular Compliance Measured as Incremental Volume-Pressure Ratio. Circ. Res. 1971, 28, 277–289. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shoukas, A.A.; Sagawa, K. Control of Total Systemic Vascular Capacity by the Carotid Sinus Baroreceptor Reflex. Circ. Res. 1973, 33, 22–33. [Google Scholar] [CrossRef] [Green Version]
- Henry, J.P.; Gauer, O.H.; Sieker, H.O. The Effect of Moderate Changes in Blood Volume on Left and Right Atrial Pressures. Circ. Res. 1956, 4, 91–94. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Holtz, J.; Bassenge, E.; Kinadeter, H.; Kolin, A. Increased Effective Vascular Compliance and Venous Pooling of Intravascular Volume during Sustained Venodilation in Conscious Dogs. Basic Res. Cardiol. 1981, 76, 657–669. [Google Scholar] [CrossRef] [PubMed]
- Echt, M.; Duweling, J.; Gauer, O.H.; Lange, L. Effective Compliance of the Total Vascular Bed and the Intrathoracic Compartment Derived from Changes in Central Venous Pressure Induced by Volume Changes in Man. Circ. Res. 1974, 34, 61–68. [Google Scholar] [CrossRef] [Green Version]
- London, G.; Safar, M.; Weiss, Y.; Simon, C. Total Effective Compliance of the Vascular Bed in Essential Hypertension. Am. Heart J. 1978, 95, 325–330. [Google Scholar] [CrossRef]
- London, G.M.; Safar, M.E.; Simon, A.C.; Alexandre, J.M.; Levenson, J.A.; Weiss, Y.A. Total Effective Compliance, Cardiac Output and Fluid Volumes in Essential Hypertension. Circulation 1978, 57, 995–1000. [Google Scholar] [CrossRef] [Green Version]
- London, G.M.; Safar, M.E.; Payen, D.M.; Gitelman, R.C.; Guerin, A.M. Total, Peripheral and Intrathoracic Effective Compliances of the Vascular Bed in Normotensive and Hypertensive Patients. In Contributions to Nephrology; Bahlmann, J., Liebau, H., Eds.; S. Karger AG: Basel, Switzerland, 1982; Volume 30, pp. 144–153. [Google Scholar]
- Safar, M.E.; London, G.M.; Levenson, J.A.; Simon, A.C.; Chau, N.P. Rapid Dextran Infusion in Essential Hypertension. Hypertension 1979, 1, 615–623. [Google Scholar] [CrossRef] [Green Version]
- Safar, M.E.; London, G.M. Arterial and Venous Compliance in Sustained Essential Hypertension. Hypertension 1987, 10, 133–139. [Google Scholar] [CrossRef] [Green Version]
- Gauer, O.H.; Henry, J.P.; Sieker, H.O. Changes in Central Venous Pressure after Moderate Hemorrhage and Transfusion in Man. Circ. Res. 1956, 4, 79–84. [Google Scholar] [CrossRef] [Green Version]
- Warren, J.V.; Brannon, E.S.; Weens, H.S.; Stead, E. Effect of Increasing the Blood Volume and Right Atrial Pressure on the Circulation of Normal Subjects by Intravenous Infusions. Am. J. Med. 1948, 4, 193–200. [Google Scholar] [CrossRef]
- Koubenec, H.J.; Risch, W.D.; Gauer, O.H. Effective Compliance of the Circulation in the Upright Sitting Posture. Pflügers Arch. Eur. J. Physiol. 1978, 374, 121–124. [Google Scholar] [CrossRef] [PubMed]
- Witham, A.C.; Fleming, J.W.; Bloom, W.L. The effect of the intravenous administration of dextran on cardiac output and other circulatory dynamics. J. Clin. Investig. 1951, 30, 897–902. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pang, C.C.Y. Measurement of body venous tone. J. Pharmacol. Toxicol. Methods 2000, 44, 341–360. [Google Scholar] [CrossRef]
- Rothe, C.F. Reflex Control of Veins and Vascular Capacitance. Physiol. Rev. 1983, 63, 1281–1342. [Google Scholar] [CrossRef]
- Ludbrook, J.; Graham, W.F. The Role of Cardiac Receptor and Arterial Baroreceptor Reflexes in Control of the Circulation during Acute Change of Blood Volume in the Conscious Rabbit. Circ. Res. 1984, 54, 424–435. [Google Scholar] [CrossRef] [Green Version]
- Simon, A.C.; Safar, M.E.; Levenson, J.A.; London, G.M.; Levy, B.I.; Chau, N.P. An evaluation of large arteries compliance in man. Am. J. Physiol.-Heart Circ. Physiol. 1979, 237, H550–H554. [Google Scholar] [CrossRef]
- Sun, Y.; Beshara, M.; Lucariello, R.; Chiaramida, S. A Comprehensive Model for Right–Left Heart Interaction under the Influence of Pericardium and Baroreflex. Am. J. Physiol. 1997, 272, H1499–H1515. [Google Scholar] [CrossRef] [Green Version]
- Guyton, A.C.; Hall, J.E. Guyton and Hall TextBook of Medical Physiology, 12th ed.; Saunders/Elsevier: Amsterdam, The Netherlands, 2011. [Google Scholar]
- Ursino, M.; Antonucci, M.; Belardinelli, E. Role of Active Changes in Venous Capacity by the Carotid Baroreflex: Analysis with a Mathematical Model. Am. J. Physiol.-Heart Circ. Physiol. 1994, 267, H2531–H2546. [Google Scholar] [CrossRef] [PubMed]
- Safar, M.E.; London, G.M. Venous System in Essential Hypertension. Clin. Sci. 1985, 69, 497–504. [Google Scholar] [CrossRef] [PubMed]
- Mynard, J.; Smolich, J. One-Dimensional Haemodynamic Modeling and Wave Dynamics in the Entire Adult Circulation. Ann. Biomed. Eng. 2015, 43, 1443–1460. [Google Scholar] [CrossRef] [PubMed]
- Alfie, J.; Waisman, G.D.; Galarza, C.R.; Cámera, M.I. Contribution of Stroke Volume to the Change in Pulse Pressure Pattern with Age. Hypertension 1999, 34, 808–812. [Google Scholar] [CrossRef] [Green Version]
- Takatsu, H.; Gotoh, K.; Suzuki, T.; Ohsumi, Y.; Yagi, Y.; Tsukamoto, T.; Terashima, Y.; Nagashima, K.; Hirakama, S. Quantitative estimation of compliance of human systemic veins by occlusion plethysmography with radionucleotide: Methodology and the Effect of Nitroglycerin. Jpn. Circ. J. 1989, 53, 245–254. [Google Scholar] [CrossRef] [PubMed]
- Toro, E.F.; Celant, M.; Zhang, Q.; Contarino, C.; Agarwal, N.; Linninger, A.A.; Müller, L.O. Cerebrospinal fluid dynamics coupled to the global circulation in holistic setting: Mathematical models, numerical methods and applications. 2021; under review. [Google Scholar]
- Müller, L.O.; Toro, E.F. A Global Multiscale Mathematical Model for the Human Circulation with Emphasis on the Venous System. Int. J. Numer. Methods Biomed. Eng. 2014, 30, 681–725. [Google Scholar] [CrossRef]
- Müller, L.O.; Toro, E.F. Enhanced Global Mathematical Model for Studying Cerebral Venous Blood Flow. J. Biomech. 2014, 47, 3361–3372. [Google Scholar] [CrossRef]
- Barnard, A.L.; Hunt, W.; Timlake, W.; Varley, E. A Theory of Fluid Flow in Compliant Tubes. Biophys. J. 1966, 6, 717–724. [Google Scholar] [CrossRef] [Green Version]
- Hughes, T.J.; Lubliner, J. On the One-Dimensional Theory of Blood Flow in the Larger Vessels. Math. Biosci. 1973, 18, 161–170. [Google Scholar] [CrossRef]
- Toro, E.F.; Millington, R.C.; Nejad, L.A.M. Towards Very High–Order Godunov Schemes. In Godunov Methods: Theory and Applications; Toro, E.F., Ed.; Kluwer Academic/Plenum Publishers: New York, NY, USA, 2001; pp. 905–937. [Google Scholar]
- Formaggia, L.; Quarteroni, A. (Eds.) Cardiovascular Mathematics: Modeling and Simulation of the Circulatory System; Number 1 in MS & A: Modeling, Simulation & Applications; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Alastruey, J.; Khir, A.; Matthys, K.; Segers, P.; Sherwin, S.; Verdonck, P.; Parker, K.; Peiró, J. Pulse Wave Propagation in a Model Human Arterial Network: Assessment of 1-D Visco-Elastic Simulations against In Vitro Measurements. J. Biomech. 2011, 44, 2250–2258. [Google Scholar] [CrossRef] [PubMed]
- Toro, E.F.; Siviglia, A. Flow in Collapsible Tubes with Discontinuous Mechanical Properties: Mathematical Model and Exact Solutions. Commun. Comput. Phys. 2013, 13, 361–385. [Google Scholar] [CrossRef]
- Spilimbergo, A.; Toro, E.F.; Müller, L.O. One-Dimensional Blood Flow with Discontinuous Properties and Transport: Mathematical Analysis and Numerical Schemes. Commun. Comput. Phys. 2021, 29, 649–697. [Google Scholar] [CrossRef]
- Cattaneo, C. A Form of Heat-Conduction Equations which Eliminates the Paradox of Instantaneous Propagation. Comptes Rendus Mathématique l’Academie Sci. 1958, 247, 431–433. [Google Scholar]
- Montecinos, G.I.; Toro, E.F. Reformulations for General Advection-Diffusion-Reaction Equations and Locally Implicit ADER Schemes. J. Comput. Phys. 2014, 275, 415–442. [Google Scholar] [CrossRef]
- Montecinos, G.I.; Müller, L.O.; Toro, E.F. Hyperbolic Reformulation of a 1D Viscoelastic Blood Flow Model and ADER Finite Volume Schemes. J. Comput. Phys. 2014, 266, 101–123. [Google Scholar] [CrossRef]
- Müller, L.O.; Blanco, P.J.; Watanabe, S.M.; Feijóo, R.A. A High-Order Local Time Stepping Finite Volume Solver for One-Dimensional Blood Flow Simulations: Application to the ADAN Model. Int. J. Numer. Methods Biomed. Eng. 2015, 32, e02761. [Google Scholar] [CrossRef]
- Toro, E.F. The ADER Path to High-Order Godunov Methods. Continuum Mechanics, Applied Mathematics and Scientific Computing: Godunov’s Legacy—A Liber Amicorum to Professor Godunov; Springer: Berlin/Heidelberg, Germany, 2020; pp. 359–366. [Google Scholar]
- Müller, L.O.; Parés, C.; Toro, E.F. Well-Balanced High-Order Numerical Schemes for One-Dimensional Blood Flow in Vessels with Varying Mechanical Properties. J. Comput. Phys. 2013, 242, 53–85. [Google Scholar] [CrossRef]
- Müller, L.O.; Toro, E.F. Well-Balanced High-Order Solver for Blood Flow in Networks of Vessels with Variable Properties. Int. J. Numer. Methods Biomed. Eng. 2013, 29, 1388–1411. [Google Scholar] [CrossRef]
- Toro, E.; Montecinos, G. Advection-Diffusion-Reaction Equations: Hyperbolisation and High-Order ADER Discretizations. SIAM J. Sci. Comput. 2014, 36, A2423–A2457. [Google Scholar] [CrossRef]
- Dumbser, M.; Käser, M.; Toro, E.F. An Arbitrary High Order Discontinuous Galerkin Method for Elastic Waves on Unstructured Meshes v: Local Time Stepping and p–Adaptivity. Geophys. J. Int. 2007, 171, 695–717. [Google Scholar] [CrossRef] [Green Version]
- Liang, F.; Takagi, S.; Himeno, R.; Liu, H. Biomechanical Characterization of Ventricular-Arterial Coupling during Aging: A Multi-Scale Mode Study. J. Biomech. 2009, 42, 692–704. [Google Scholar] [CrossRef]
- Mynard, J.; Davidson, M.; Penny, D.; Smolich, J. A Simple, Versatile Valve Model for Use in Lumped Parameter and One-Dimensional Cardiovascular Models. Int. J. Numer. Methods Biomed. Eng. 2012, 28, 626–641. [Google Scholar] [CrossRef]
- Ursino, M.; Lodi, C. A Simple Mathematical Model of the Interaction between Intracranial Pressure and Cerebral Hemodynamics. J. Appl. Physiol. 1997, 82, 1256–1269. [Google Scholar] [CrossRef]
- Falz, R.; Fikenzer, S.; Hoppe, S.; Busse, M. Normal Values of Hemoglobin Mass and Blood Volume in Young, Active Women and Men. Int. J. Sport. Med. 2019, 40, 236–244. [Google Scholar] [CrossRef] [PubMed]
- Davy, K.P.; Seals, D.R. Total Blood Volume in Healthy Young and Older Men. J. Appl. Physiol. 1994, 76, 2059–2062. [Google Scholar] [CrossRef] [PubMed]
- Magder, S. Volume and Its Relationship to Cardiac output and Venous Return. Crit. Care 2016, 20, 271. [Google Scholar] [CrossRef] [Green Version]
- Greenway, C.V.; Lautt, W.W. Blood Volume, the Venous System, Preload, and Cardiac Output. Can. J. Physiol. Pharmacol. 1986, 64, 383–387. [Google Scholar] [CrossRef] [PubMed]
- Davis, T.L. Teaching Physiology through Interactive Simulation of Hemodynamics. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1991. [Google Scholar]
- Danielsen, M. Modeling of Feedback Mechanisms Which Control the Heart Function in a View to an Implementation in Cardiovascular Models. Ph.D. Thesis, Roskilde University Center, Roskilde, Denmark, 1998. [Google Scholar]
- Blanco, P.J.; Trenhago, P.R.; Fernandes, L.G.; Feijóo, R.A. On the Integration of the Baroreflex Control Mechanism in a Heterogeneous Model of the Cardiovascular System. Int. J. Numer. Methods Biomed. Eng. 2012, 28, 412–433. [Google Scholar] [CrossRef] [PubMed]
- Ursino, M.; Innocenti, M. Modeling Arterial Hypotension During Hemodialysis. Artif. Organs 2008, 21, 873–890. [Google Scholar] [CrossRef]
- Ursino, M.; Magosso, E. Acute Cardiovascular Response to Isocapnic Hypoxia. I. A Mathematical Model. Am. J. Physiol.-Heart Circ. Physiol. 2000, 279, H149–H165. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lim, E.; Chan, G.S.H.; Dokos, S.; Ng, S.C.; Latif, L.A.; Vandenberghe, S.; Karunanithi, M.; Lovell, N.H. A Cardiovascular Mathematical Model of Graded Head-Up Tilt. PLoS ONE 2013, 8, e77357. [Google Scholar] [CrossRef] [Green Version]
- Liang, F.; Liu, H. Simulation of Hemodynamic Responses to the Valsalva Maneuver: An Integrative Computational Model of the Cardiovascular System and the Autonomic Nervous System. J. Physiol. Sci. 2006, 56, 45–65. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Levick, J.R. An Introduction to Cardiovascular Physiology, 5th ed.; Hodder Arnold: London, UK, 2010. [Google Scholar]
- McEniery, C.; Ian, Y.; Ahmad Qasem, R.; Wilkinson, I.; Cockcroft, J. Normal Vascular Aging: Differential Effects on Wave Reflection and Aortic Pulse Wave Velocity: The Anglo-Cardiff Collaborative Trial (ACCT). J. Am. Coll. Cardiol. 2005, 46, 1753–1760. [Google Scholar] [CrossRef] [Green Version]
- Najjar, S.; Schulman, S.; Gerstenblith, G.; Fleg, J.; Kass, D.; O’Connor, F.; Becker, L.; Lakatta, E. Age and gender affect ventricular-vascular coupling during aerobic exercise. J. Am. Coll. Cardiol. 2004, 44, 611–617. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Guyton, A.C.; Lindsey, A.W.; Kaufmann, B.N.; Abernathy, J.B. Effect of Blood Transfusion and Hemorrhage on Cardiac Output and on the Venous Return Curve. Am. J. Physiol.-Leg. Content 1958, 194, 263–267. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Payne, S. A Model of the Interaction between Autoregulation and Neural Activation in the Brain. Math. Biosci. 2006, 204, 260–281. [Google Scholar] [CrossRef] [PubMed]
- Cirovic, S.; Walsh, C.; Fraser, W.D. Mathematical study of the role of non-linear venous compliance in the cranial volume-pressure test. Med. Biol. Eng. Comput. 2003, 41, 579–588. [Google Scholar] [CrossRef]
- Persson, P.B.; Kirchheim, H.R. (Eds.) Baroreceptor Reflexes; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar]
- Mark, A.L.; Mancia, G. Cardiopulmonary Baroreflexes in Humans. In Comprehensive Physiology; Terjung, R., Ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2011. [Google Scholar]
- Coleridge, H.M.; Coleridge, J.C.; Kaufman, M.P.; Dangel, A. Operational Sensitivity and Acute Resetting of Aortic Baroreceptors in Dogs. Circ. Res. 1981, 48, 676–684. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ottesen, J.; Olufsen, M. Functionality of the Baroreceptor Nerves in Heart Rate Regulation. Comput. Methods Programs Biomed. 2011, 101, 208–219. [Google Scholar] [CrossRef] [PubMed]
- Ricketts, J.H.; Head, G.A. A Five-Parameter Logistic Equation for Investigating Asymmetry of Curvature in Baroreflex Studies. Am. J. Physiol.-Regul. Integr. Comp. Physiol. 1999, 277, R441–R454. [Google Scholar] [CrossRef]
- Bainbridge, F.A. The Influence of Venous Filling upon the Rate of the Heart. J. Physiol. 1915, 50, 65–84. [Google Scholar] [CrossRef] [PubMed]
- Takeshita, A.; Mark, A.L.; Eckberg, D.L.; Abboud, F.M. Effect of Central Venous Pressure on Arterial Baroreflex Control of Heart Rate. Am. J. Physiol.-Heart Circ. Physiol. 1979, 236, H42–H47. [Google Scholar] [CrossRef] [PubMed]
- Ursino, M. Interaction between Carotid Baroregulation and the Pulsating Heart: A Mathematical Model. Am. J. Physiol.-Heart Circ. Physiol. 1998, 275, H1733–H1747. [Google Scholar] [CrossRef] [PubMed]
- Hosomi, H.; Sagawa, K. Sinovagal Interaction in Arterial Pressure Restoration after 10% Hemorrhage. Am. J. Physiol.-Regul. Integr. Comp. Physiol. 1979, 237, R203–R209. [Google Scholar] [CrossRef]
- Kumada, M.; Sagawa, K. Aortic Nerve Activity during Blood Volume Changes. Am. J. Physiol.-Leg. Content 1970, 218, 961–965. [Google Scholar] [CrossRef] [PubMed]
- Gupta, P.; Henry, J.; Sinclair, R.; Von Baumgarten, R. Responses of Atrial and Aortic Baroreceptors to Nonhypotensive Hemorrhage and to Tranfusion. Am. J. Physiol.-Leg. Content 1966, 211, 1429–1437. [Google Scholar] [CrossRef]
- Abboud, F.M.; Thames, M.D. Interaction of Cardiovascular Reflexes in Circulatory Control. In Comprehensive Physiology; American Cancer Society: Atlanta, GA, USA, 2011; Chapter 19; pp. 675–753. [Google Scholar]
- Trippodo, N.C. Total Circulatory Capacity in the Rat. Effects of Epinephrine and Vasopressin on Compliance and Unstressed Volume. Circ. Res. 1981, 49, 923–931. [Google Scholar] [CrossRef] [Green Version]
- Albanese, A.; Cheng, L.; Ursino, M.; Chbat, N.W. An integrated mathematical model of the human cardiopulmonary system: Model development. Am. J. Physiol.-Heart Circ. Physiol. 2016, 310, H899–H921. [Google Scholar] [CrossRef]


| Vascular Territory | Compliance [mL/mmHg] | Unstressed Volume [mL] |
|---|---|---|
| Systemic arterial circulation | 4 | 615 |
| Systemic venous circulation | 111 | 2500 |
| Pulmonary arterial circulation | 6.56 | 90 |
| Pulmonary venous circulation | 25.37 | 490 |
| Cardiac circulation | 50 |
| Actuator | [s] | [mmHg] | [mmHg] | |||
|---|---|---|---|---|---|---|
| H | 4 | 1.15 | 0.34 | 0.595 | 0.02 | 0 |
| E | 10 | 0.4 | 0 | 0.8 | 0.02 | 0 |
| R | 15 | 0.8 | 0 | 0.6 | 0.02 | 0.7 |
| C | 30 | −0.2 | 0 | 1.1 | 0.02 | 0 |
| V | 60 | −0.2 | 0 | 1.1 | 10.8 | 417 |
| Index | Current Value | Ref. Value | Ref. |
|---|---|---|---|
| SBP [mmHg] | 107.48 | 105 ± 8, 129 ± 3 | [10,64] |
| DBP [mmHg] | 76.18 | 71 ± 7, 76 ± 2 | [10,64] |
| MBP [mmHg] | 91.19 | 89 ± 8, 97 ± 2 | [10,64] |
| PP [mmHg] | 31.31 | 30 ± 6 | [64] |
| PP [mmHg] | 38.01 | 49 ± 9 | [64] |
| PP [mmHg] | 1.21 | 1.7 ± 0.14 | [64] |
| CO [mL/s] | 88.64 | ||
| C [mL/mmHg] | 1.91 | 1.7 | [28] |
| CVP [mmHg] | 4.21 | 4.2 ± 0.8 | [10] |
| H [beats/min] | 75 | 76 ± 4 | [10] |
| E [mmHg/mL] | 4.61 | 4.5 | [65] |
| E [mmHg/mL] | 2.80 | 2.3 | [65] |
| E/E | 0.60 | 0.58 | [65] |
| LV | 116.66 | 150 ± 67 | [27] |
| LV | 0.62 | 0.68 ± 0.12 | [27] |
| max. | 1511.27 | 1915 ± 410 | [27] |
| min. | −2632.04 | −2296 ± 530 | [27] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Celant, M.; Toro, E.F.; Müller, L.O. Total Effective Vascular Compliance of a Global Mathematical Model for the Cardiovascular System. Symmetry 2021, 13, 1858. https://doi.org/10.3390/sym13101858
Celant M, Toro EF, Müller LO. Total Effective Vascular Compliance of a Global Mathematical Model for the Cardiovascular System. Symmetry. 2021; 13(10):1858. https://doi.org/10.3390/sym13101858
Chicago/Turabian StyleCelant, Morena, Eleuterio F. Toro, and Lucas O. Müller. 2021. "Total Effective Vascular Compliance of a Global Mathematical Model for the Cardiovascular System" Symmetry 13, no. 10: 1858. https://doi.org/10.3390/sym13101858
APA StyleCelant, M., Toro, E. F., & Müller, L. O. (2021). Total Effective Vascular Compliance of a Global Mathematical Model for the Cardiovascular System. Symmetry, 13(10), 1858. https://doi.org/10.3390/sym13101858





