Small-Angle Scattering from Fractional Brownian Surfaces
Abstract
:1. Introduction
2. Theoretical Background
2.1. Small-Angle Scattering Technique
2.2. Small-Angle Scattering from Fractal Surfaces
2.3. Fractional Brownian Surfaces
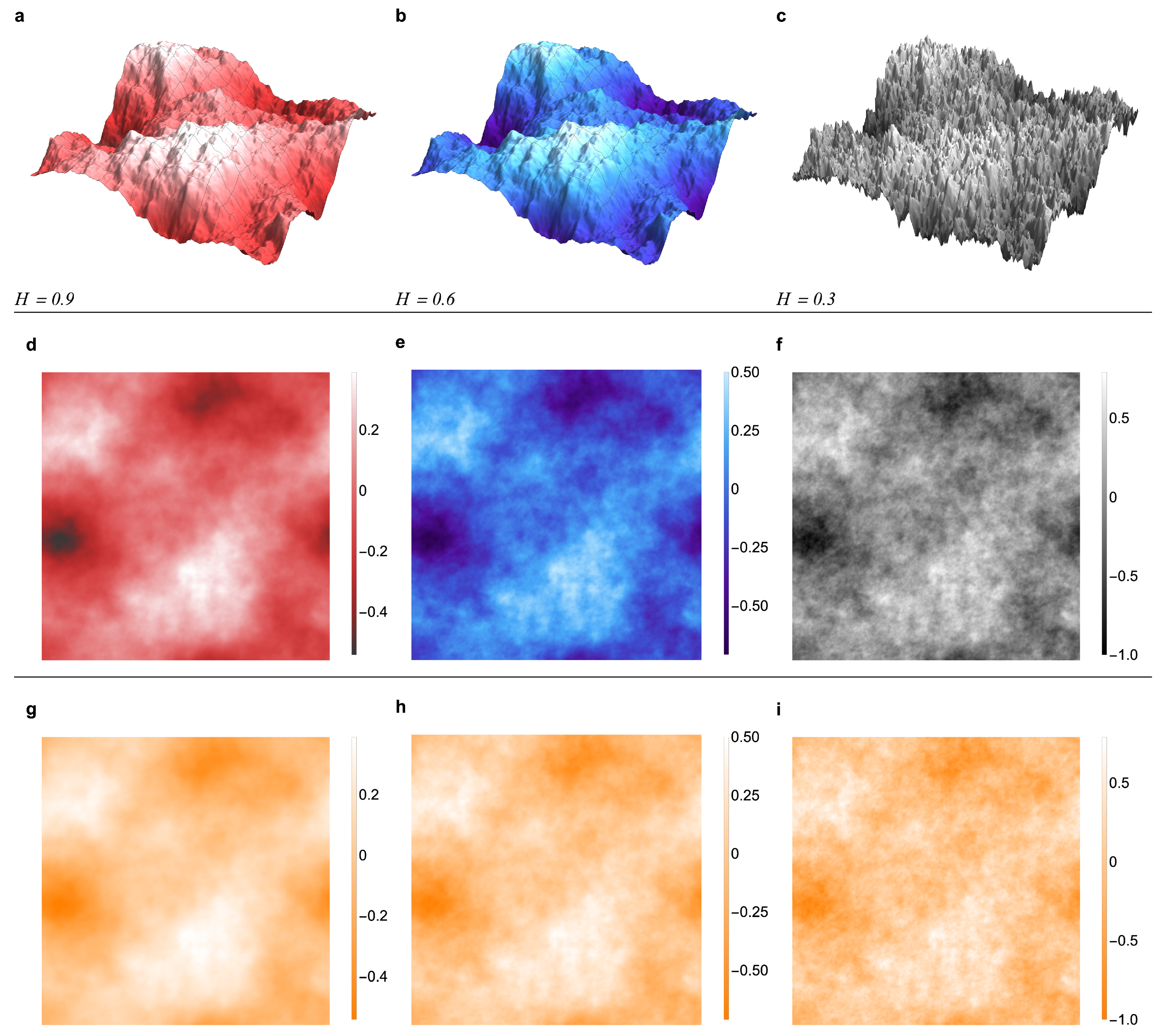
3. Methodology for Generating the Fractional Brownian Surfaces and for Calculating the Pair Distance Distribution Function
- Class I fBss (CI): distances between points are kept unchanged; thus, and z are of the same orders of magnitude. This corresponds to the classical structure of fBss, as shown in Figure 1, with a globular-like shape.
- Class II fBss (CII): distances between points are stretched by the same amount along x and y directions by a factor of b; thus, . This gives rise to fBss with rectangular, planar-like shapes.
- Class III fBss (CIII): distances between points are stretched along a single direction by a factor of b; thus, x or . This gives rise to fBss with rod-like shapes.
4. Results and Discussion
4.1. Pair-Distance Distribution Functions
4.2. Scattering Intensities
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zeng, P.; Tian, B.; Tian, Q.; Yao, W.; Li, M.; Wang, H.; Feng, Y.; Liu, L.; Wu, W. Screen-Printed, Low-Cost, and Patterned Flexible Heater Based on Ag Fractal Dendrites for Human Wearable Application. Adv. Mater. Technol. 2019, 4, 1800453. [Google Scholar] [CrossRef]
- Reinhardt, H.; Kroll, M.; Karstens, S.L.; Schlabach, S.; Hampp, N.A.; Tallarek, U. Nanoscaled Fractal Superstructures via Laser Patterning—A Versatile Route to Metallic Hierarchical Porous Materials. Adv. Mater. Interfaces 2021, 8, 2000253. [Google Scholar] [CrossRef] [Green Version]
- Kelesidis, G.A.; Pratsinis, S.E. A perspective on gas-phase synthesis of nanomaterials: Process design, impact and outlook. Chem. Eng. J. 2021, 421, 129884. [Google Scholar] [CrossRef]
- Liu, T.; Liu, P.; Guo, X.; Zhang, J.; Huang, Q.; Luo, Z.; Zhou, X.; Yang, Q.; Tang, Y.; Lu, A. Preparation, characterization and discussion of glass ceramic foam material: Analysis of glass phase, fractal dimension and self-foaming mechanism. Mater. Chem. Phys. 2020, 243, 122614. [Google Scholar] [CrossRef]
- Culcer, D.; Hu, X.; Das Sarma, S. Interface roughness, valley-orbit coupling, and valley manipulation in quantum dots. Phys. Rev. B 2010, 82, 205315. [Google Scholar] [CrossRef] [Green Version]
- Bonnín-Ripoll, F.; Martynov, Y.B.; Cardona, G.; Nazmitdinov, R.G.; Pujol-Nadal, R. Synergy of the ray tracing+carrier transport approach: On efficiency of perovskite solar cells with a back reflector. Sol. Energy Mater. Sol. Cells 2019, 200, 110050. [Google Scholar] [CrossRef]
- Jiang, G.; Hu, J.; Chen, L. Preparation of a Flexible Superhydrophobic Surface and Its Wetting Mechanism Based on Fractal Theory. Langmuir 2020, 36, 8435–8443. [Google Scholar] [CrossRef]
- Gonzalez-Torres, M.; Ramirez-Mata, A.; Melgarejo-Ramirez, Y.; Alvarez-Perez, M.A.; Jose Montesinos, J.; Leyva-Gomez, G.; Sanchez-Sanchez, R.; Eugenia-Baca, B.; Velasquillo, C. Assessment of biocompatibility and surface topography of poly(ester urethane)–silica nanocomposites reveals multifunctional properties. Mater. Lett. 2020, 276, 128269. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, J.; Li, K.; Hu, J. Surface characterization and biocompatibility of isotropic microstructure prepared by UV laser. J. Mater. Sci. Technol. 2021, 94, 136–146. [Google Scholar] [CrossRef]
- Vipul, S.; Anastasia, K.; Kyriacos, Y.; Kimmo, L.; Veikko, S. Flexible biodegradable transparent heaters based on fractal-like leaf skeletons. NPJ Flex. Electron. 2020, 4, 27. [Google Scholar] [CrossRef]
- Nazmitdinov, R.G.; Simonović, N.S.; Rost, J.M. Semiclassical analysis of a two-electron quantum dot in a magnetic field: Dimensional phenomena. Phys. Rev. B 2002, 65, 155307. [Google Scholar] [CrossRef] [Green Version]
- Nazmitdinov, R.G.; Simonović, N.S. Finite-thickness effects in ground-state transitions of two-electron quantum dots. Phys. Rev. B 2007, 76, 193306. [Google Scholar] [CrossRef] [Green Version]
- Mandelbrot, B.B.; Van Ness, J.W. Fractional Brownian Motions, Fractional Noises and Applications. SIAM Rev. 1968, 10, 422–437. [Google Scholar] [CrossRef]
- Calame, J.P.; Garven, M. Dielectric permittivity simulations of layered composites with rough interfacial surfaces. IEEE Trans. Dielectr. Electr. Insul. 2007, 14, 287–295. [Google Scholar] [CrossRef]
- Glyanko, M.S.; Volkov, A.V.; Fomchenkov, S.A. Assessment of surface roughness of substrates subjected to plasma-chemical etching. J. Phys. Conf. Ser. 2014, 541, 012100. [Google Scholar] [CrossRef] [Green Version]
- Zribi, M.; Ciarletti, V.; Taconet, O.; Paillé, J.; Boissard, P. Characterisation of the Soil Structure and Microwave Backscattering Based on Numerical Three-Dimensional Surface Representation: Analysis with a Fractional Brownian Model. Remote Sens. Environ. 2000, 72, 159–169. [Google Scholar] [CrossRef]
- Clivati-McIntyre, A.A.; McCoy, E.L. Fractional Brownian description of aggregate surfaces within undisturbed soil samples using penetration resistance measurements. Soil Tillage Res. 2006, 88, 144–152. [Google Scholar] [CrossRef]
- Mandelbrot, B.B. The Fractal Geometry of Nature; W.H. Freeman: San Francisco, CA, USA, 1982; p. 460. [Google Scholar]
- Arrault, J.; Arnéodo, A.; Davis, A.; Marshak, A. Wavelet Based Multifractal Analysis of Rough Surfaces: Application to Cloud Models and Satellite Data. Phys. Rev. Lett. 1997, 79, 75–78. [Google Scholar] [CrossRef]
- Majumdar, A.; Tien, C. Fractal characterization and simulation of rough surfaces. Wear 1990, 136, 313–327. [Google Scholar] [CrossRef]
- Liang, X.; Lin, B.; Han, X.; Chen, S. Fractal analysis of engineering ceramics ground surface. Appl. Surf. Sci. 2012, 258, 6406–6415. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, Y.; Chen, X.; Zhang, C.; Tan, Y. Two-stage method for fractal dimension calculation of the mechanical equipment rough surface profile based on fractal theory. Chaos Solitons Fractals 2017, 104, 495–502. [Google Scholar] [CrossRef]
- Dubuc, B.; Zucker, S.W.; Tricot, C.; Quiniou, J.F.; Wehbi, D.; Berry, M.V. Evaluating the fractal dimension of surfaces. Proc. R. Soc. Lond. A Math. Phys. Sci. 1989, 425, 113–127. [Google Scholar] [CrossRef]
- Schaefer, D.W.; Justice, R.S. How Nano Are Nanocomposites? Macromolecules 2007, 40, 8501–8517. [Google Scholar] [CrossRef]
- Anitas, E.M. Small-Angle Scattering from Fractals: Differentiating between Various Types of Structures. Symmetry 2020, 12, 65. [Google Scholar] [CrossRef] [Green Version]
- Cherny, A.Y.; Anitas, E.M.; Osipov, V.A.; Kuklin, A.I. Small-angle scattering from the Cantor surface fractal on the plane and the Koch snowflake. Phys. Chem. Chem. Phys. 2017, 19, 2261–2268. [Google Scholar] [CrossRef] [Green Version]
- Anitas, E.M.; Slyamov, A.; Szakacs, S. Microstructural characterization of surface fractals using small-angle scattering. Rom. J. Phys. 2018, 63, 104. [Google Scholar]
- Cherny, A.Y.; Anitas, E.M.; Osipov, V.A.; Kuklin, A.I. The structure of deterministic mass and surface fractals: Theory and methods of analyzing small-angle scattering data. Phys. Chem. Chem. Phys. 2019, 21, 12748–12762. [Google Scholar] [CrossRef] [PubMed]
- Feigin, L.A.; Svergun, D.I. Structure Analysis by Small-Angle X-ray and Neutron Scattering; Springer: Boston, MA, USA, 1987; p. 335. [Google Scholar] [CrossRef]
- Glatter, O.; May, R. Small-Angle Techniques. In International Tables for Crystallography Volume C: Mathematical, Physical and Chemical Tables; Prince, E., Ed.; Springer: Dordrecht, The Netherland, 2004; pp. 89–112. [Google Scholar]
- Bacon, G.E. Neutron Diffraction, 2nd ed.; Oxford University Press: London, UK, 1962; p. 438. [Google Scholar]
- Bracewell, R. The Fourier Transform and Its Applications, 3rd ed.; Mcgraw-Hill College: Singapore, 2000; p. 486. [Google Scholar]
- Pantos, E.; Bordas, J. Supercomputer simulation of small angle X-ray scattering, electron micrographs and X-ray diffraction patterns of macromolecular structures. Pure Appl. Chem. 1994, 66, 77–82. [Google Scholar] [CrossRef]
- Russ, J.C. Fractal Surfaces; Springer Science + Business Media: Raleigh, NC, USA, 1994; p. 309. [Google Scholar] [CrossRef]
- Martin, J.E.; Hurd, A.J. Scattering from fractals. J. Appl. Crystallogr. 1987, 20, 61–78. [Google Scholar] [CrossRef]
- Schmidt, P.W. Small-angle scattering studies of disordered, porous and fractal systems. J. Appl. Crystallogr. 1991, 24, 414–435. [Google Scholar] [CrossRef]
- Teixeira, J. Small-angle scattering by fractal systems. J. Appl. Crystallogr. 1988, 21, 781–785. [Google Scholar] [CrossRef] [Green Version]
- Bale, H.D.; Schmidt, P.W. Small-Angle X-Ray-Scattering Investigation of Submicroscopic Porosity with Fractal Properties. Phys. Rev. Lett. 1984, 53, 596–599. [Google Scholar] [CrossRef]
- Pfeifer, P.; Ehrburger-Dolle, F.; Rieker, T.P.; González, M.T.; Hoffman, W.P.; Molina-Sabio, M.; Rodríguez-Reinoso, F.; Schmidt, P.W.; Voss, D.J. Nearly Space-Filling Fractal Networks of Carbon Nanopores. Phys. Rev. Lett. 2002, 88, 115502. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Stein, M.L. Fast and Exact Simulation of Fractional Brownian Surfaces. J. Comput. Graph. Stat. 2002, 11, 587–599. [Google Scholar] [CrossRef]
- Saupe, D. Algorithms for random fractals. In The Science of Fractal Images; Peitgen, H.O., Saupe, D., Eds.; Springer: New York, NY, USA, 1988; pp. 71–113. [Google Scholar]
- Cherny, A.Y.; Anitas, E.M.; Osipov, V.A.; Kuklin, A.I. Scattering from surface fractals in terms of composing mass fractals. J. Appl. Crystallogr. 2017, 50, 919–931. [Google Scholar] [CrossRef] [Green Version]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anitas, E.M. Small-Angle Scattering from Fractional Brownian Surfaces. Symmetry 2021, 13, 2042. https://doi.org/10.3390/sym13112042
Anitas EM. Small-Angle Scattering from Fractional Brownian Surfaces. Symmetry. 2021; 13(11):2042. https://doi.org/10.3390/sym13112042
Chicago/Turabian StyleAnitas, Eugen Mircea. 2021. "Small-Angle Scattering from Fractional Brownian Surfaces" Symmetry 13, no. 11: 2042. https://doi.org/10.3390/sym13112042
APA StyleAnitas, E. M. (2021). Small-Angle Scattering from Fractional Brownian Surfaces. Symmetry, 13(11), 2042. https://doi.org/10.3390/sym13112042





