On Stability Switches and Bifurcation of the Modified Autonomous Van der Pol–Duffing Equations via Delayed State Feedback Control
Abstract
:1. Introduction
- Both the delay independent stable condition and the delay dependent stable interval of MAVPD in (2) are included in the framework of the decomposition method.
- Based on the adjoint operator theory, a lite bilinear form is introduced to reduce the amount of calculations on projection and adjoint eigenvectors.
- By solving the initial value problem of ordinary differential equation, a sophisticated procedure is addressed for the computation of Hopf bifurcation.
2. Problem Statement and Preliminaries
The Decomposition Method
3. Main Results
3.1. The Issue of Linear Analysis
3.2. The Issue of Periodical Solutions
| Algorithm 1 The calculations of the stability switches and Hopf bifurcation |
| 1: Construct the auxiliary polynomial of (8) by Lemma 1. |
| 2: Compute the PRRs of (10) by Lemma 2, and the critical delays in (19). |
| 3: Determine the delay stable intervals by Theorem 1 and Corollary 1. |
| 4: Compute the eigenvectors and in (28) and (29). |
| 5: Determine , substitute it into the Hassard formula in (34), and obtain . |
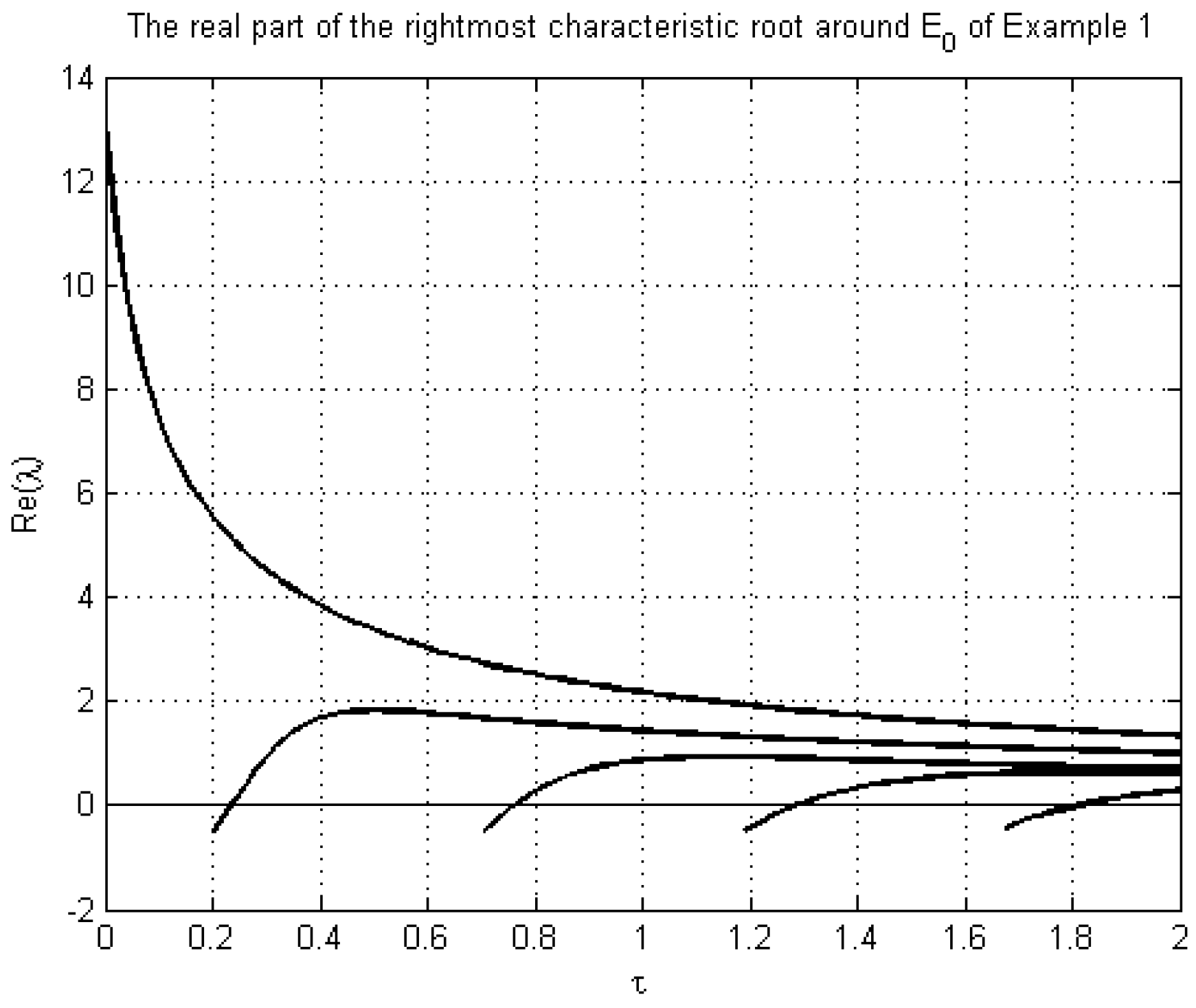
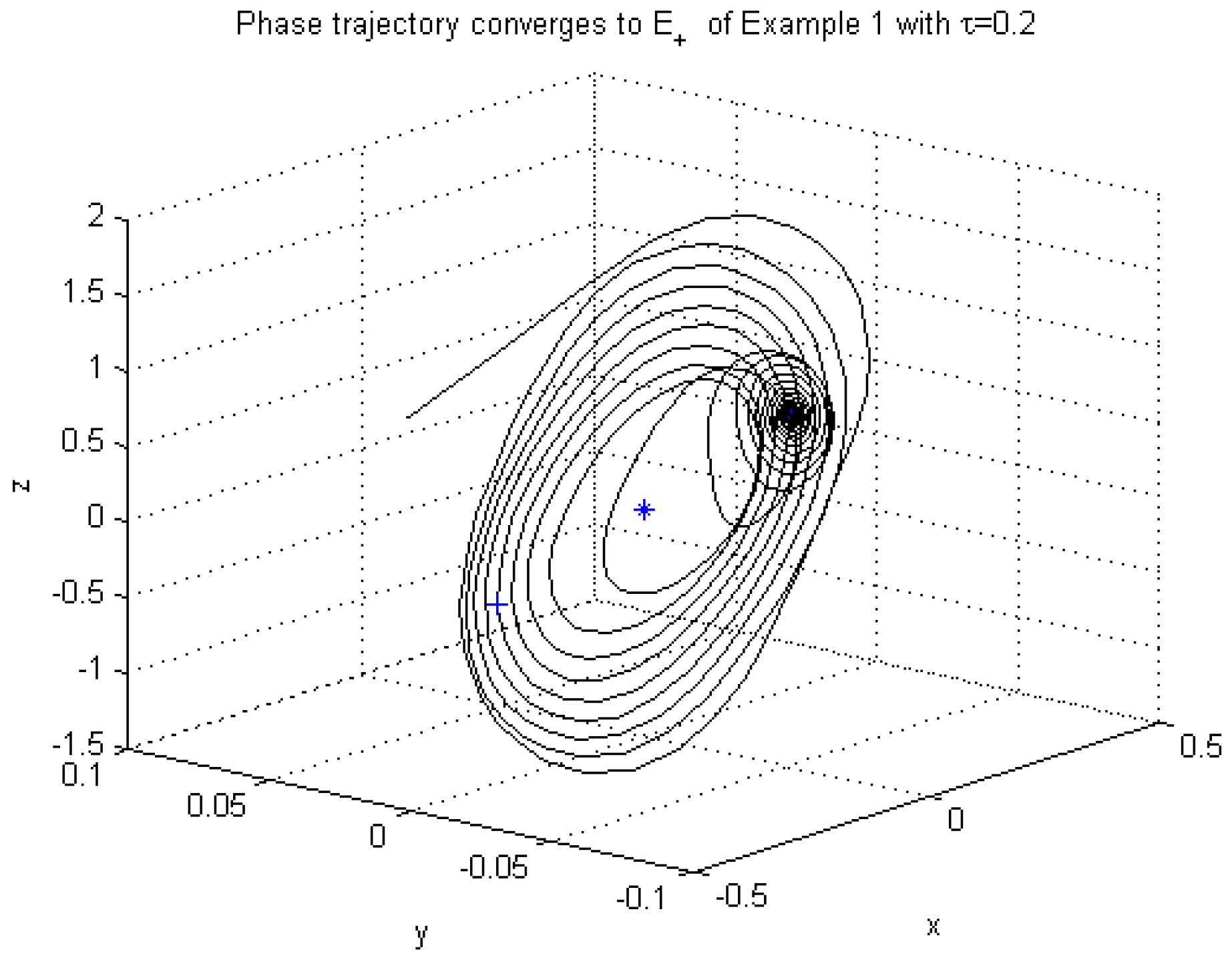
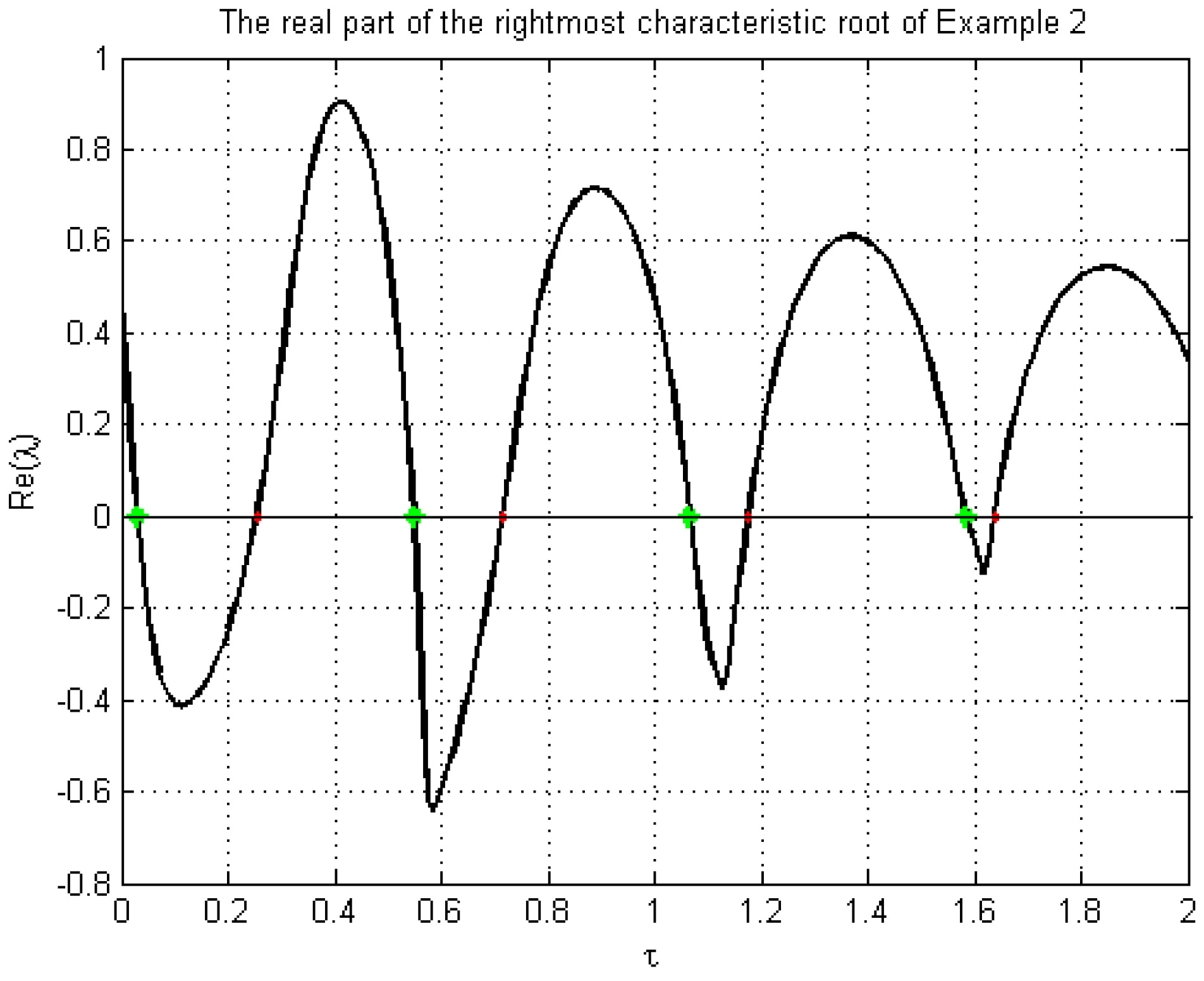
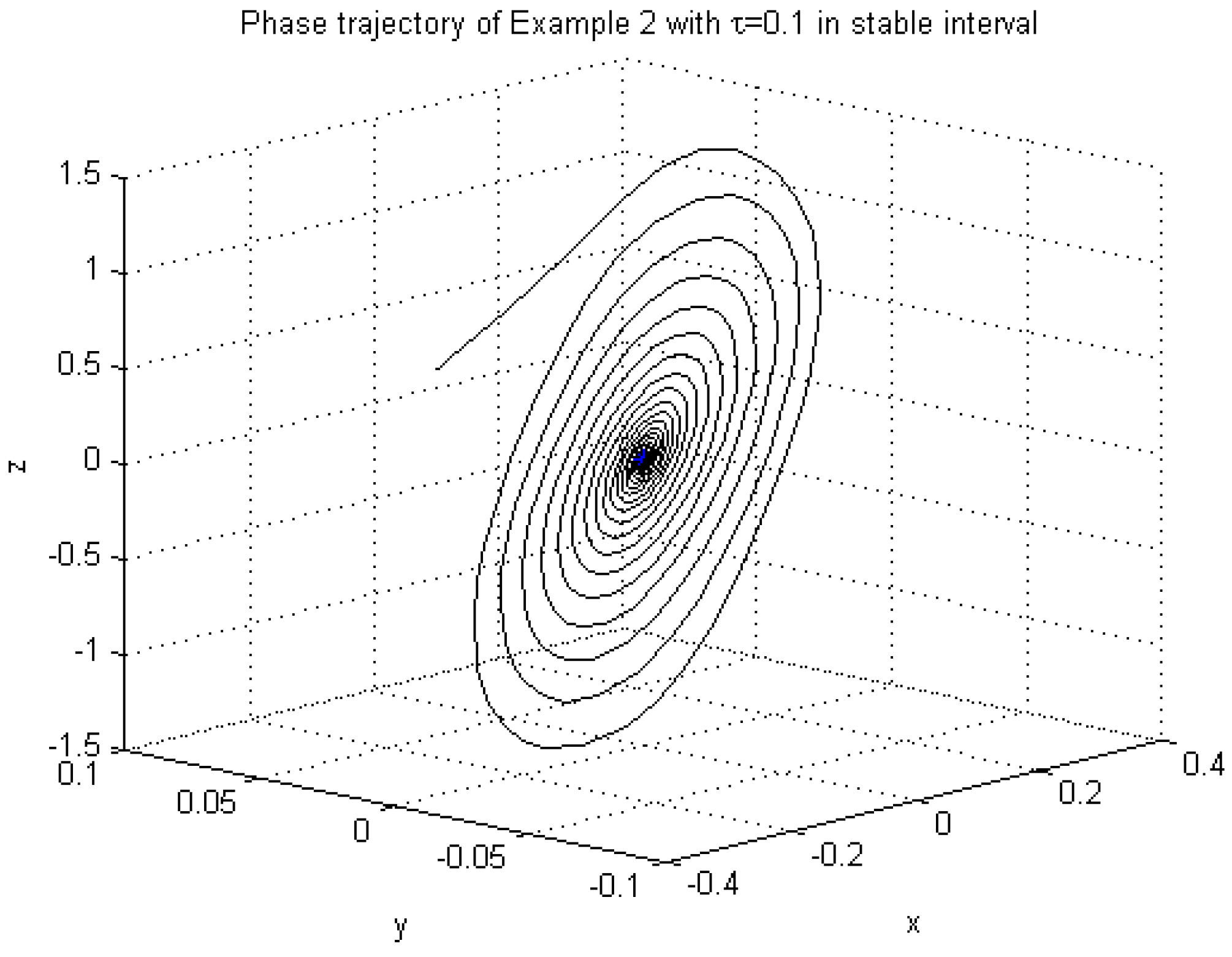
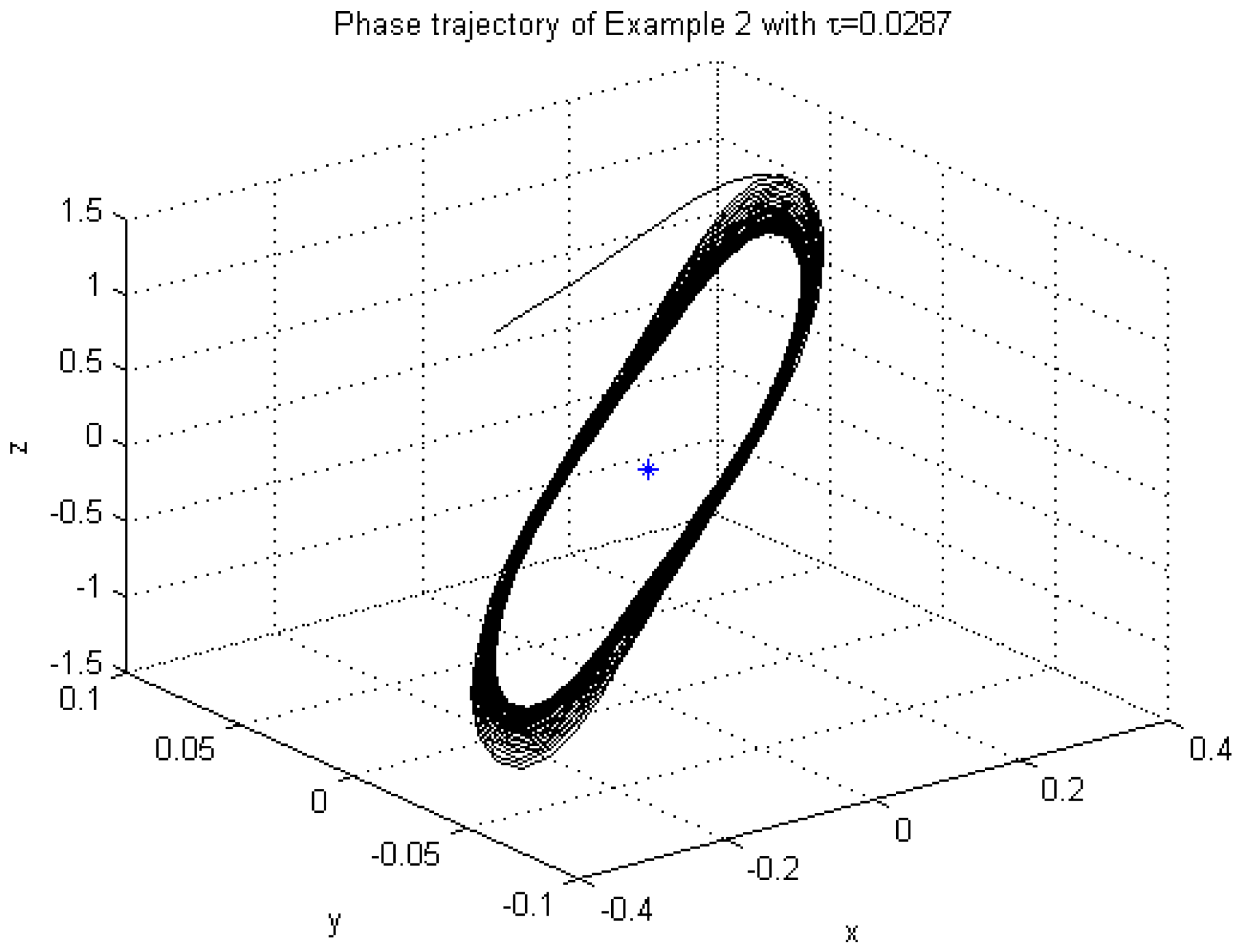
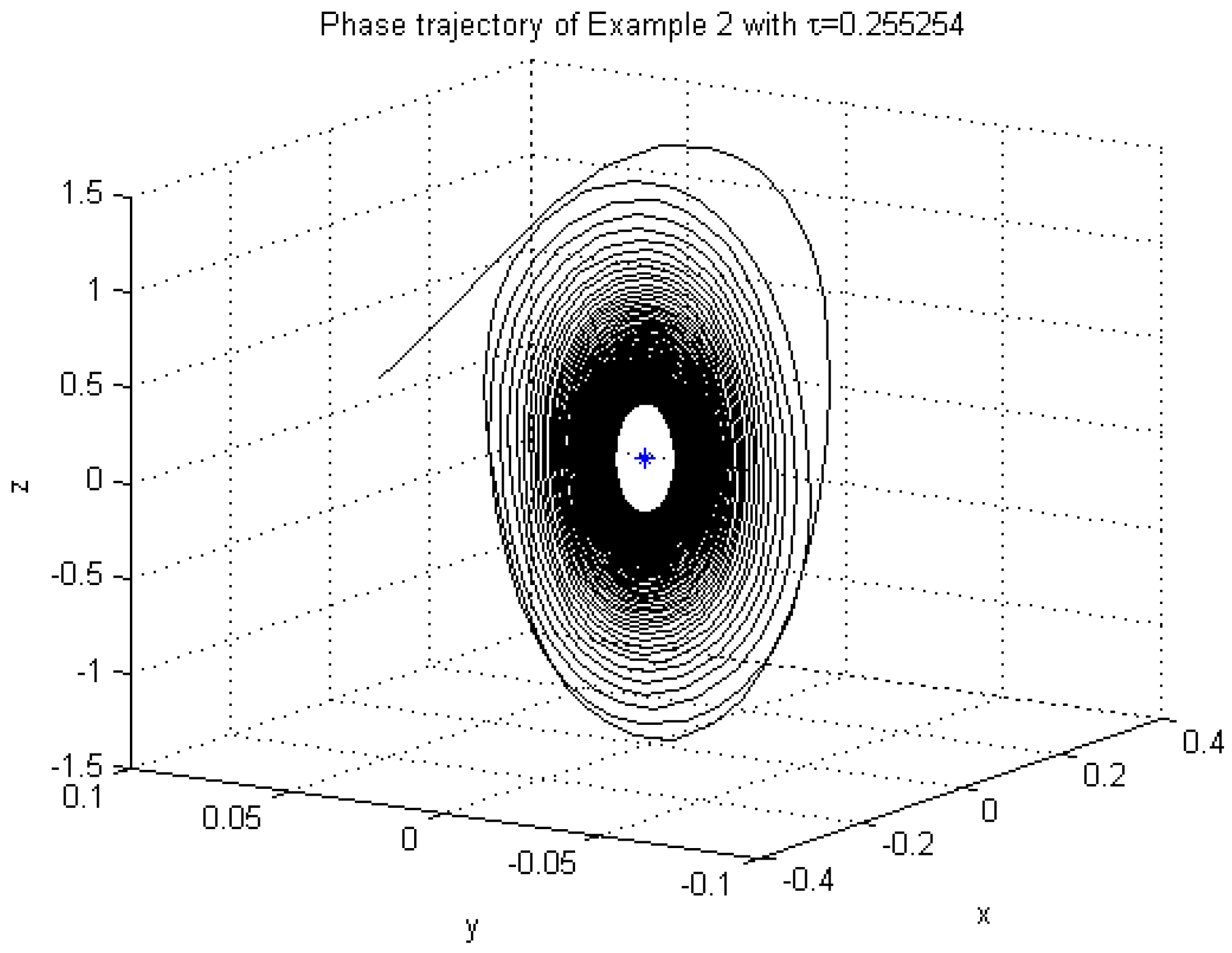
4. Numerical Examples
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| MAVPD | Modified autonomous Van der Pol–Duffing |
| PIR | Purely imaginary root |
| PRR | Positive real root |
| CD | Cross direction |
| CF | Cross frequency |
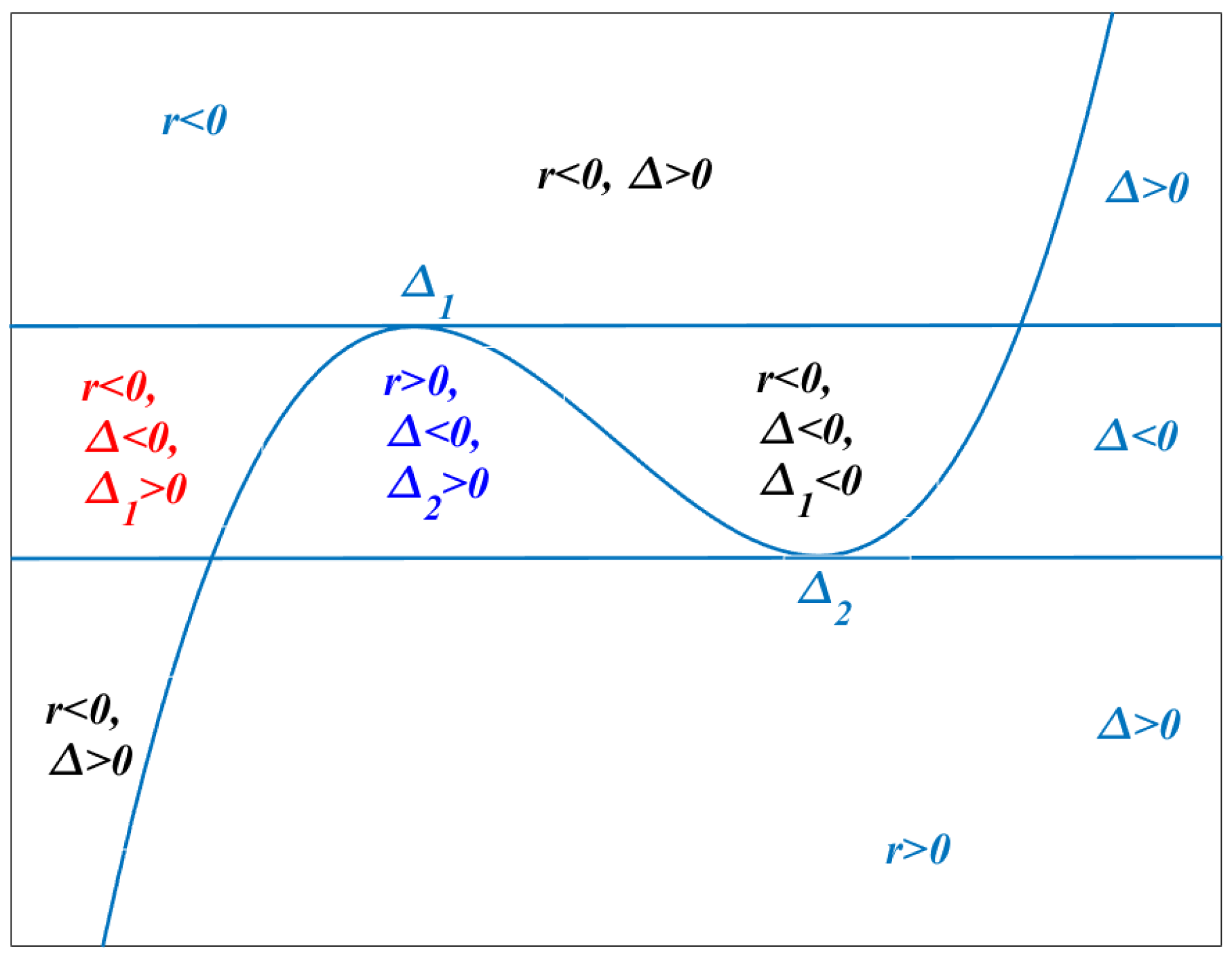
Appendix A. The Proof of Lemma 2
References
- Lebovitz, N.R. Bifurcation and stability problems in astrophysics. In Applications of Bifurcation Theory; Acadamic Press: New York, NY, USA, 1877; pp. 259–284. [Google Scholar]
- Shinriki, M.; Yamamoto, M.; Mori, S. Multimode oscillations in a modified van der Pol oscillator containing a positive nonlinear conductance. Proc. IEEE 1981, 69, 394–395. [Google Scholar] [CrossRef]
- King, G.P.; Gaito, S.T. Bistable chaos. I. Unfolding the cusp. Phys. Rev. A 1992, 46, 3092–3099. [Google Scholar] [CrossRef] [PubMed]
- Matouk, A.E.; Agiza, H.N. Bifurcations, chaos and synchronization in ADVP circuit with parallel resistor. J. Math. Anal. Appl. 2008, 341, 259–269. [Google Scholar] [CrossRef] [Green Version]
- Braga, D.C.; Mello, L.F.; Messias, M. Bifurcation analysis of a Van der Pol-Duffing circuit with parallel resistor. Math. Probl. Eng. 2009, 2009, 149563. [Google Scholar] [CrossRef] [Green Version]
- Fan, Q.J. Horseshoe in a modified Van der Pol-Duffing circuit. Appl. Math. Comput. 2009, 210, 436–440. [Google Scholar] [CrossRef]
- Vincent, U.E.; Nana Nbendjo, B.R.; Ajayi, A.A.; Njah, A.N.; McClintock, P.V.E. Hyperchaos and bifurcations in a driven Van der Pol-Duffing oscillator circuit. Int. J. Dyn. Control 2015, 3, 363–370. [Google Scholar] [CrossRef] [Green Version]
- Han, X.J.; Xia, F.B.; Ji, P.; Bi, Q.S.; Kurths, J. Hopf-bifurcation-delay-induced bursting patterns in a modified circuit system. Commun. Nonlinear Sci. Numer. Simul. 2016, 36, 517–527. [Google Scholar] [CrossRef]
- Miwadinou, C.H.; Hinvi, L.A.; Monwanou, A.V.; Chabi Orou, J. Nonlinear dynamics of a ϕ6 modified Duffing oscillator: Resonant oscillations and transition to chaos. Nonlinear Dyn. 2017, 88, 97–113. [Google Scholar] [CrossRef]
- Kumar, P.; Kumar, A.; Erlicher, S. A modified hybrid Van der Pol-Duffing-Rayleigh oscillator for modelling the lateral walking force on a rigid floor. Phys. D Nonlinear Phenom. 2017, 358, 1–14. [Google Scholar] [CrossRef]
- Rajagopal, K.; Pone, J.; Kingni, S.T.; Arun, S.; Karthikeyan, A. Analysis and electronic implementation of an absolute memristor autonomous Van der Pol-Duffing circuit. Eur. Phys. J. Spec. Top. 2019, 228, 2287–2299. [Google Scholar] [CrossRef]
- Rajagopal, K.; Khalaf, A.; Wei, Z.; Pham, V.T.; Alsaedi, A.; Hayat, T. Hyperchaos and coexisting attractors in a modified van der Pol-Duffing oscillator. Int. J. Bifurc. Chaos 2019, 29, 1950067. [Google Scholar] [CrossRef]
- Ma, X.D.; Yu, Y.; Wang, L.F. Complex mixed-mode vibration types triggered by the pitchfork bifurcation delay in a driven van der Pol-Duffing oscillator. Appl. Math. Comput. 2021, 411, 126522. [Google Scholar] [CrossRef]
- Panigoro, H.; Suryanto, A.; Kusumawinahyu, W.; Darti, I. Dynamics of an eco-epidemic predator-prey model involving fractional derivatives with power-law and mittag-leffler kernel. Symmetry 2021, 13, 785. [Google Scholar] [CrossRef]
- Isidori, A. Control of nonlinear systems via dynamic state feedback. In Algebraic and Geometric Methods in Nonlinear Control Theory; Riedel: Dordrecht, The Netherlands, 1986. [Google Scholar]
- Namajunas, A.; Pyragas, K.; Tamasevicius, A. An electronic analog of the Mackey-Glass system. Phys. Lett. A 1995, 201, 42–46. [Google Scholar] [CrossRef]
- Lee, M.S.; Hsu, C.S. On the τ decomposition method of stability analysis for retarded dynamical systems. SIAM J. Control 1969, 7, 249–259. [Google Scholar] [CrossRef]
- Cooke, K.; Driessche, P. On zeros of some transcendental equations. Funkcialaj Ekvacioj 1986, 29, 77–90. [Google Scholar]
- Hassard, B.D.; Kazarinoff, N.D.; Wan, Y.H. Theory and Applications of Hopf Bifurcation; Cambridge University Press: Cambridge, UK, 1981. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cai, T.-Y.; Jin, H.-L.; Yu, H.; Xie, X.-P. On Stability Switches and Bifurcation of the Modified Autonomous Van der Pol–Duffing Equations via Delayed State Feedback Control. Symmetry 2021, 13, 2336. https://doi.org/10.3390/sym13122336
Cai T-Y, Jin H-L, Yu H, Xie X-P. On Stability Switches and Bifurcation of the Modified Autonomous Van der Pol–Duffing Equations via Delayed State Feedback Control. Symmetry. 2021; 13(12):2336. https://doi.org/10.3390/sym13122336
Chicago/Turabian StyleCai, Tiao-Yang, Hui-Long Jin, Hong Yu, and Xiang-Peng Xie. 2021. "On Stability Switches and Bifurcation of the Modified Autonomous Van der Pol–Duffing Equations via Delayed State Feedback Control" Symmetry 13, no. 12: 2336. https://doi.org/10.3390/sym13122336




