Mixed Convection of Silica–Molybdenum Disulphide/Water Hybrid Nanoliquid over a Rough Sphere
Abstract
:1. Introduction
- –
- combined convective flow over a rough sphere;
- –
- impact of molybdenum-silica/water hybrid nanofluid on flow structures and heat patterns;
- –
- effect of surface roughness on hydro- and thermodynamics.
2. Mathematical Analysis
3. Method of Analysis
4. Results and Discussion
5. Conclusions
- –
- the hybrid nanofluid augments the temperature, as well as Nusselt number at the wall;
- –
- velocity distribution is reduced, while friction at the surface enhances in the case of hybrid nanofluid;
- –
- slip exists near the surface of the sphere owing to roughness, which yields steep jump in the liquid velocity close to the border;
- –
- for enhancing magnitudes of small parameter, the friction at the surface and the Nusselt number are enhanced;
- –
- skin-friction parameter and the Nusselt number are boosted for hybrid nanoliquid on comparison with mono nanofluid and the base fluid;
- –
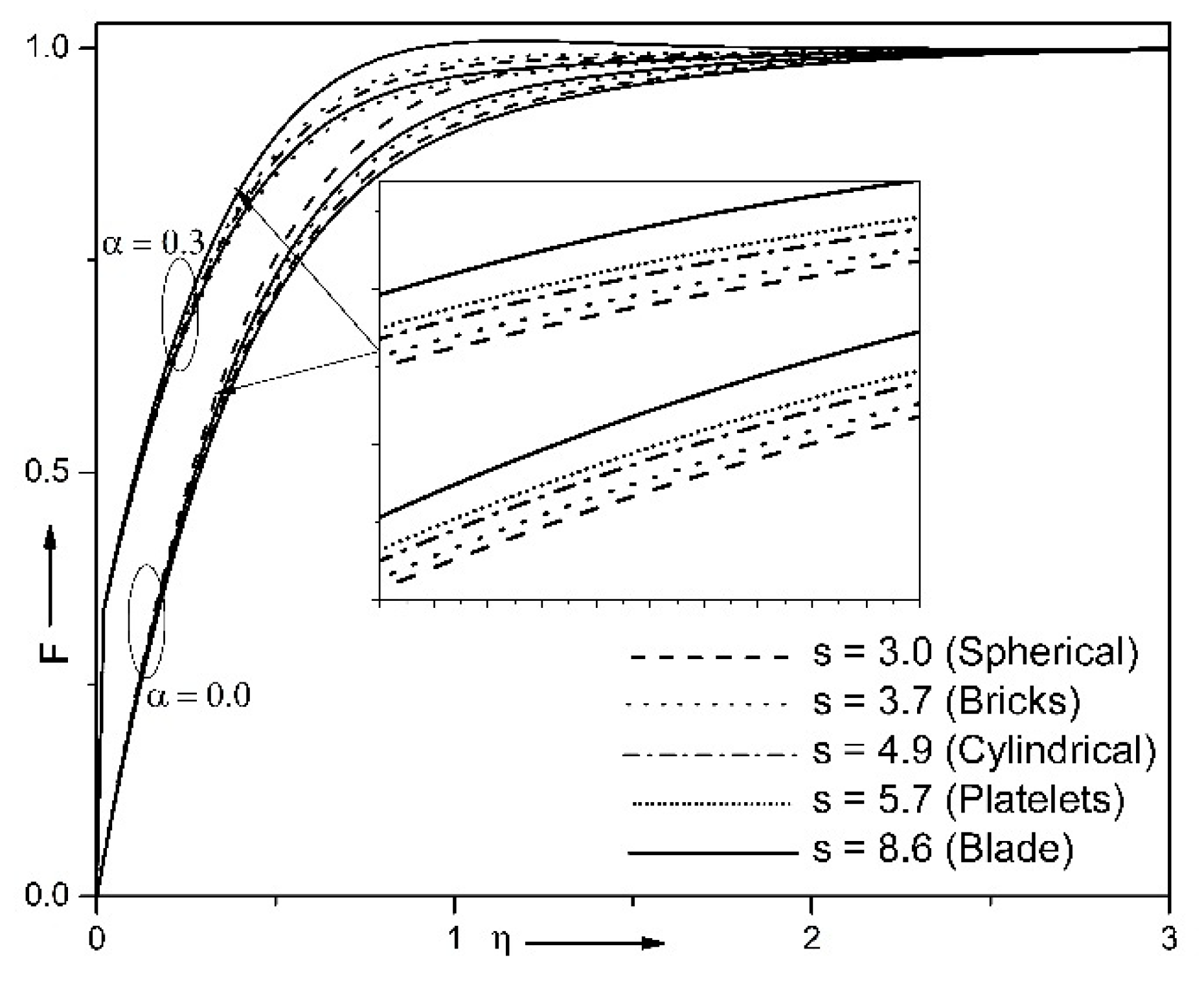
- skin-friction parameter and the Nusselt number have the highest values for blade-shaped nanoadditives and lowest value is found for spherical-formed nanoparticles.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| F | dimensionless velocity |
| Nu | Nusselt number |
| G | non-dimensional temperature |
| g | gravity acceleration |
| n | frequency parameter |
| v | y-velocity projection |
| r(x) | radius of the section normal to axis of the sphere |
| Pr | Prandtl number |
| U0 | reference velocity |
| f | dimensionless stream function |
| R | radius of the sphere |
| Tw | temperature at the wall |
| Re | Reynolds number |
| Gr | Grashof number |
| Ri | combined convection parameter |
| T | temperature |
| T∞ | ambient temperature |
| u | x-velocity projection |
| U∞ | free stream velocity constant |
| x, y | Cartesian coordinates |
| Ue | free stream velocity |
Greek symbols
| α | small parameter |
| step size in and η directions | |
| ε | velocity ratio parameter |
| , η | transformed variables |
| νf | kinematic viscosity |
| φ1 | volume fraction of silica nanoparticle |
| φ2 | volume fraction of molybdenum disulphide nanoparticle |
| ψ | dimensionless stream function |
Subscripts
| f | base fluid |
| hnf | hybrid nanofluid |
| nf | mono nanofluid |
| s1 | solid component for silica |
| s2 | solid component for molybdenum disulphide |
| , η | variables of the partial derivatives |
References
- Buongiorno, J. Convective transport in nanofluids. J. Heat Transf. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 250, 2002–2018. [Google Scholar] [CrossRef]
- Choi, S.U.S. Enhancing thermal conductivity of fluids with nanoparticles. In Proceedings of the 1995 ASME International Mechanical Engineering Congress and Exposition (FED 231/MD), San Francisco, CA, USA, 12–17 November 1995; Volume 6, pp. 99–105. [Google Scholar]
- Chu, Y.-M.; Nisar, K.S.; Khan, U.; Kasmaei, H.D.; Malaver, M.; Zaib, A.; Khan, I. Mixed convection in MHD water-based molybdenum disulfide-graphene oxide hybrid nanofluid through an upright cylinder with shape factor. Water 2020, 12, 1723. [Google Scholar] [CrossRef]
- Rostami, M.N.; Dinarvand, S.; Pop, I. Dual solutions for mixed convective stagnation-point flow of an aqueous silica-alumina hybrid nanofluid. Chin. J. Phys. 2018, 56, 2465–2478. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Miroshnichenko, I.V.; Sheremet, M.A. Numerical analysis of unsteady conjugate natural convection of hybrid water-based nanofluid in a semicircular cavity. J. Therm. Sci. Eng. Appl. 2017, 9, 041004. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Mixed convection flow over an exponentially stretching/shrinking vertical surface in a hybrid nanofluid. Alex. Eng. J. 2020, 59, 1881–1891. [Google Scholar] [CrossRef]
- Dinarvand, S.; Rostami, M.N.; Pop, I. A novel hybridity model for TiO2-CuO/water hybrid nanofluid flow over a static/moving wedge or corner. Sci. Rep. 2019, 9, 16290. [Google Scholar] [CrossRef]
- Acharya, N.; Das, K.; Kundu, P.K. Ramification of variable thickness on MHD TiO2 and Ag nanofluid flow over a slandering stretching sheet using NDM. Eur. Phys. J. Plus 2016, 131, 303. [Google Scholar] [CrossRef]
- Tlili, I.; Nabwey, H.A.; Samrat, S.P.; Sandeep, N. 3D MHD nonlinear radiative flow of CuO-MgO/methanol hybrid nanofluid beyond an irregular dimension surface with slip effect. Sci. Rep. 2020, 10, 9181. [Google Scholar] [CrossRef]
- Aladdin, N.A.L.; Bachok, N.; Pop, I. Cu-Al2O3/water hybrid nanofluid flow over a permeable moving surface in presence of hydromagnetic and suction effects. Alex. Eng. J. 2020, 59, 657–666. [Google Scholar] [CrossRef]
- Izadi, M.; Mohebbi, R.; Karimi, D.; Sheremet, M.A. Numerical simulation of natural convection heat transfer inside a ⊥ shaped cavity filled by a MWCNT-Fe3O4/water hybrid nanofluids using LBM. Chem. Eng. Process. Process Intensif. 2018, 125, 56–66. [Google Scholar] [CrossRef]
- Tassaddiq, A.; Khan, S.; Bilal, M.; Gul, T.; Mukhtar, S.; Shah, Z.; Bonyah, E. Heat and mass transfer together with hybrid nanofluid flow over a rotating disk. AIP Adv. 2020, 10, 055317. [Google Scholar] [CrossRef]
- Maraj, E.N.; Iqbal, Z.; Azhar, E.; Mehmood, Z. A comprehensive shape factor analysis using transportation of MoS2–SiO2/H2O inside an isothermal semi vertical inverted cone with porous boundary. Results Phys. 2018, 8, 633–641. [Google Scholar] [CrossRef]
- Ghadikolaei, S.S.; Gholinia, M.; Hoseini, M.E.; Ganji, D.D. Natural convection MHD flow due to MoS2-Ag nanoparticles suspended in C2H6O2-H2O hybrid base fluid with thermal radiation. J. Taiwan Inst. Chem. Eng. 2019, 97, 12–23. [Google Scholar] [CrossRef]
- Khan, U.; Shafiq, A.; Ziab, A.; Baleanu, D. Hybrid nanofluid on mixed convective radiative flow from an irregular variably thick moving surface with convex and concave effects. Case Stud. Therm. Eng. 2020, 21, 100660. [Google Scholar] [CrossRef]
- Saqid, M.; Ali, F.; Khan, I.; Ahmed Sheikh, N.; Bin Shafie, S. Convection in ethylene glycol-based molybdenum disulfide nanofluid. J. Therm. Anal. Calorim. 2019, 135, 523–532. [Google Scholar]
- Raza Shah, T.; Muhammad, A.; Mansoor Janjua, M. On aqua-based Silica (SiO2–Water) nanocoolant: Convective thermal potential and experimental precision evaluation in aluminum tube radiator. Nanomaterials 2020, 10, 1736. [Google Scholar] [CrossRef] [PubMed]
- Wang, H.; Yu, L.; Lee, Y.H.; Shi, Y.; Hsu, A.; Chin, M.L.; Li, L.J.; Dubey, M.; Kong, J.; Palacios, T. Integrated circuits based on bilayer MoS2 transistors. Nano Lett. 2012, 12, 4674–4680. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Das, S.; Chen, H.Y.; Penumatcha, A.V.; Appenzeller, J. High performance multilayer MoS2 transistors with scandium contacts. Nano Lett. 2012, 13, 100–105. [Google Scholar] [CrossRef]
- Radisavljevic, B.; Radenovic, A.; Brivio, J.; Giacometti, I.V.; Kis, A. Single-layer MoS2 transistors. Nat. Nanotechnol. 2011, 6, 147–150. [Google Scholar] [CrossRef]
- Castellanos-Gomez, A.; Poot, M.; Steele, G.A.; Van der Zant, H.S.; Agraıt, N.; Rubio-Bollinger, G. Mechanical properties of freely suspended semiconducting graphene-like layers based on MoS2. Nanoscale Res. Lett. 2012, 7, 233. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Winer, W.O. Molybdenum disulfide as a lubricant: A review of the fundamental knowledge. Wear 1967, 10, 422–452. [Google Scholar] [CrossRef] [Green Version]
- Kato, H.; Takama, M.; Iwai, Y.; Washida, K.; Sasaki, Y. Wear and mechanical properties of sintered copper–tin composites containing graphite or molybdenum disulfide. Wear 2003, 255, 573–578. [Google Scholar] [CrossRef]
- Carlisle, E.M.; Hawthorne, M.F.; Dunks, G.B. Silicon: An Essential Element for the Chick. Science 1972, 178, 619–621. [Google Scholar] [CrossRef]
- Shafie, S.; Gul, A.; Khan, I. Molybdenum disulfide nanoparticles suspended in water-based nanofluids with mixed convection and flow inside a channel filled with saturated porous medium. AIP Conf. Proc. 2016, 1775, 030042–030048. [Google Scholar]
- Bejan, A. Convection Heat Transfer; John Wiley and Sons: New York, NY, USA, 2013. [Google Scholar]
- Weigand, B.; Crawford, M.; Kays, W.M. Convective Heat and Mass Transfer, 4th ed.; McGraw Hill Education: New York, NY, USA, 2017. [Google Scholar]
- Shenoy, A.; Sheremet, M.; Pop, I. Convective Flow and Heat Transfer from Wavy Surfaces: Viscous Fluids, Porous Media and Nanofluids; CRC Press: New York, NY, USA, 2016. [Google Scholar]
- Salleh, M.Z.; Nazar, R.; Pop, I. Mixed convection boundary layer flow about a solid sphere with Newtonian heating. Arch. Mech. 2010, 62, 283–303. [Google Scholar]
- Rashad, A.M.; Chamkha, A.J.; El-Kabir, S.M.M. Effects of chemical reaction on heat and mass transfer by mixed convection flow about a sphere in a saturated porous media. Int. J. Numer. Methods Heat Fluid Flow 2011, 21, 418–433. [Google Scholar] [CrossRef] [Green Version]
- Rajakumar, J.; Saikrishnan, P.; Chamkha, A. Non-uniform mass transfer in MHD mixed convection flow of water over a sphere with variable viscosity and Prandtl number. Int. J. Numer. Methods Heat Fluid Flow 2016, 26, 2235–2251. [Google Scholar] [CrossRef]
- Roy, S.; Saikrishnan, P.; Pandey, B.D. Influence of double slot suction (injection) into water boundary layer flows over sphere. Int. Commun. Heat Mass Transf. 2009, 36, 646–650. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Grosan, T.; Pop, I. Mixed convection of a hybrid nanofluid flow along a vertical surface embedded in a porous medium. Int. Commun. Heat Mass Transf. 2020, 114, 104565. [Google Scholar] [CrossRef]
- Muhammad, K.; Hayat, T.; Alsaedi, A.; Dinarvand, B.; Momani, S. Mixed convective slip flow of hybrid nanofluid (MWCNTs + Cu + Water), nanofluid (MWCNTs + Water) and base fluid (Water): A comparative investigation. J. Therm. Anal. Calorim. 2020. [Google Scholar] [CrossRef]
- Bognar, G.; Klazly, M.; Hriczo, K. Nanofluid flow past a stretching plate. Processes 2020, 8, 827. [Google Scholar] [CrossRef]
- Bognar, G.; Hriczo, K. Similarity transformation approach for a heated ferrofluid flow in presence of magnetic field. Electron. J. Qual. Theory Differ. Equ. 2018, 42, 1–15. [Google Scholar] [CrossRef]
- Khan, U.; Zaib, A.; Khan, I.; Baleanu, D.; Nisar, K.S. Enhanced heat transfer in moderately ionized liquid due to hybrid MoS2/SiO2 nanofluids exposed by nonlinear radiation: Stability analysis. Crystals 2020, 10, 142. [Google Scholar] [CrossRef] [Green Version]
- Khan, U.; Zaib, A.; Mebarek-Oudina, F. Mixed convective magneto flow of SiO2–MoS2/C2H6O2 hybrid nanoliquids through a vertical stretching/shrinking wedge: Stability analysis. Arab. J. Sci. Eng. 2020, 45, 9061–9073. [Google Scholar] [CrossRef]
- Nikuradse, J. Stromungsgesetze in rauhen Rohren; VDI-Verlag: Berlin, Germany, 1933. [Google Scholar]
- Owen, P.R.; Thompson, W.R. Heat transfer across rough surfaces. J. Fluid Mech. 1963, 15, 321–324. [Google Scholar] [CrossRef]
- Dawson, D.A.; Trass, O. Mass transfer at rough surfaces. Int. J. Heat Mass Transf. 1972, 15, 1317–1336. [Google Scholar] [CrossRef]
- Sedahmed, G.H.; Shemilt, L.W. Forced convection mass transfer at rough surfaces annuli. Lett. Heat Mass Transf. 1972, 3, 499–512. [Google Scholar] [CrossRef]
- Patil, P.M.; Shashikant, A.; Hiremath, P.S.; Roy, S. Study of liquid oxygen and hydrogen diffusive flow past a sphere with rough surface. Int. J. Hydrogen Energy 2019, 44, 26624–26636. [Google Scholar] [CrossRef]
- Patil, P.M.; Kulkarni, M.; Hiremath, P.S. Nonlinear mixed convective nanofluid flow along moving vertical rough plate. Rev. Mex. Física 2020, 66, 153–161. [Google Scholar] [CrossRef]
- Devi, S.P.A.; Devi, S.S.U. Numerical investigation of hydromagnetic hybrid Cu-Al2O3/water nanofluid flow over a permeable stretching sheet with suction. Int. J. Nonlinear Sci. Numer. Simul. 2016, 17, 249–257. [Google Scholar] [CrossRef]
- Patil, P.M.; Shankar, H.F.; Hiremath, P.S.; Momoniat, E. Nonlinear mixed convective nanofluid flow about a rough sphere with the diffusion of liquid hydrogen. Alex. Eng. J. 2021, 60, 1043–1053. [Google Scholar] [CrossRef]
- Varga, R.S. Matrix Iterative Analysis; Prentice-Hall: Englewood Cliffs, NJ, USA, 2000. [Google Scholar]
- Hayat, T.; Nadeem, S.; Khan, A.U. Rotating flow of Ag-CuO/H2O hybrid nanofluid with radiation and partial slip boundary effects. Eur. Phys. J. E 2018, 41, 75. [Google Scholar] [CrossRef] [PubMed]
- Yousefi, M.; Dinarvand, S.; Yazdi, M.E.; Pop, I. Stagnation-point flow of an aqueous titania-copper hybrid nanofluid toward a wavy cylinder. Int. J. Numer. Methods Heat Fluid Flow 2018, 28, 1716–1735. [Google Scholar] [CrossRef]
- Patil, P.M.; Kulkarni, M.; Hiremath, P.S. Effects of surface roughness on mixed convective nanofluid flow past an exponentially stretching permeable surface. Chin. J. Phys. 2020, 64, 203–218. [Google Scholar] [CrossRef]
- Hamilton, R.; Crosser, O. Thermal conductivity of heterogeneous two-component systems. Ind. Eng. Chem. Fundam. 1962, 1, 187–191. [Google Scholar] [CrossRef]
- Patil, P.M.; Doddagoudar, S.H.; Hiremath, P.S. Impacts of surface roughness on mixed convection nanofluid flow with liquid hydrogen/nitrogen diffusion. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 2146–2174. [Google Scholar] [CrossRef]
- Nazar, R.; Amin, N.; Pop, I. Mixed convection boundary layer flow about an isothermal sphere in a micropolar fluid. Int. J. Therm. Sci. 2003, 42, 283–293. [Google Scholar] [CrossRef]
- Mohamed, M.K.A.; Sarif, N.M.; Noar, N.A.Z.M.; Salleh, M.Z.; Ishak, A. Viscous dissipation effect on the mixed convection boundary layer flow towards solid sphere. Trans. Sci. Technol. 2016, 3, 59–67. [Google Scholar]
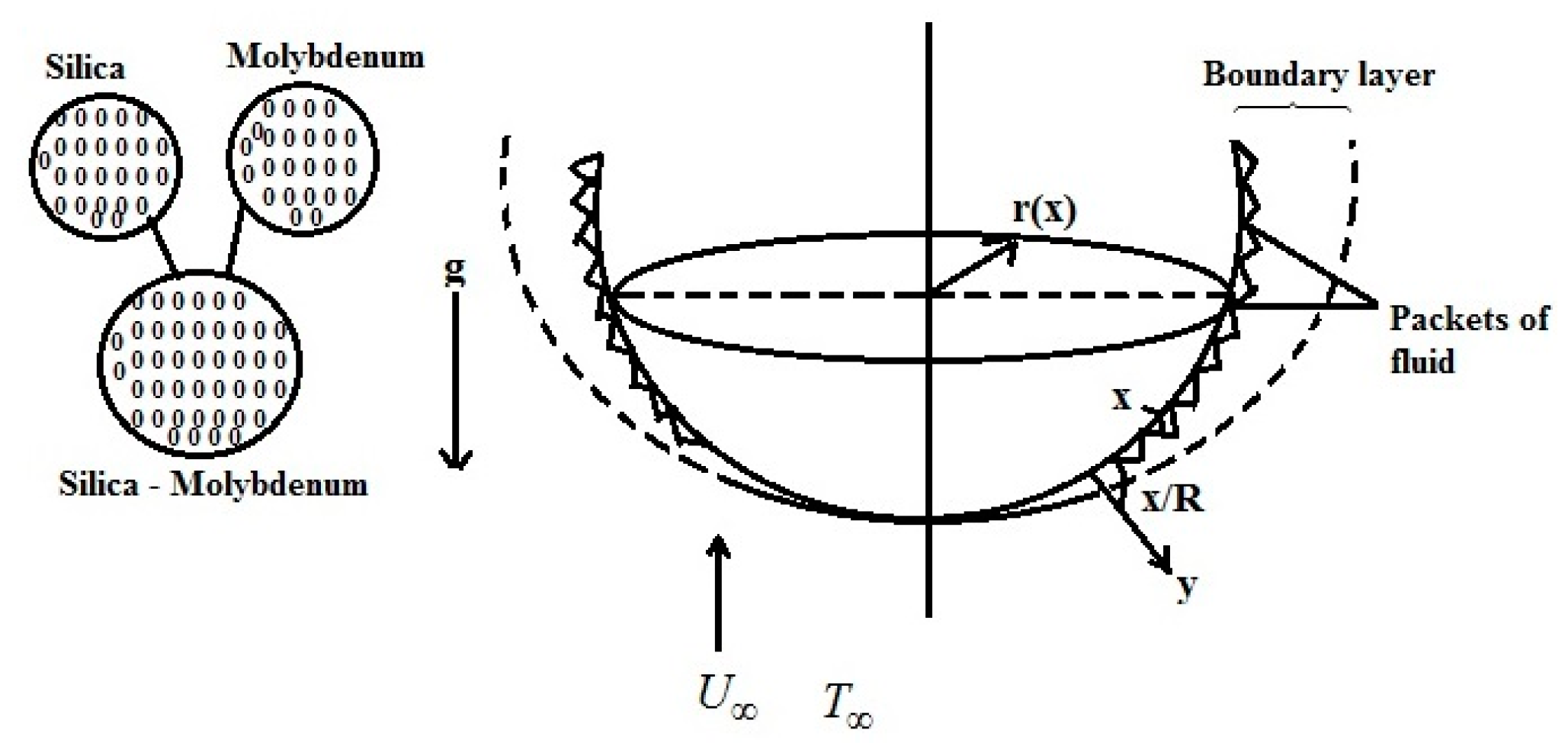
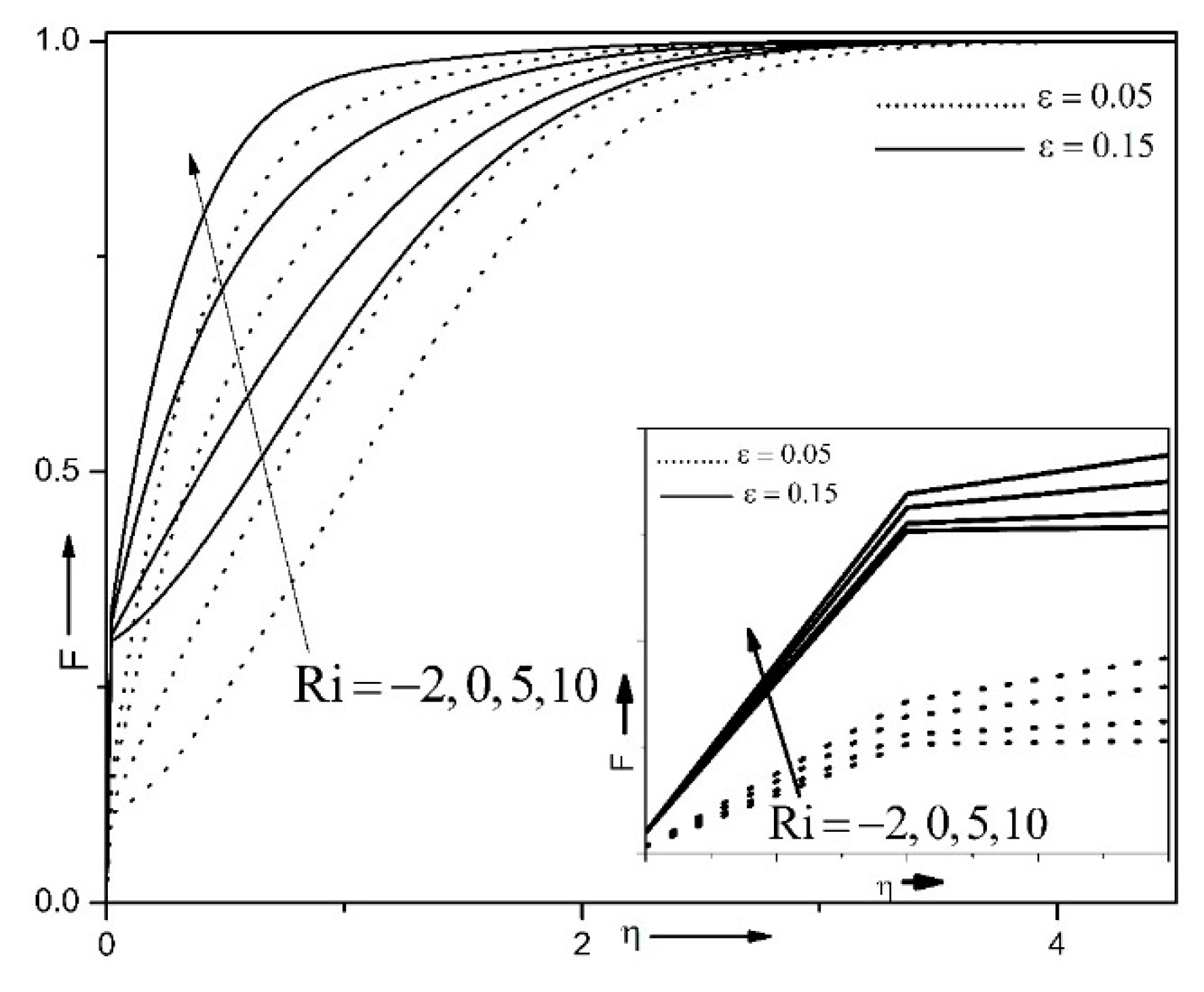
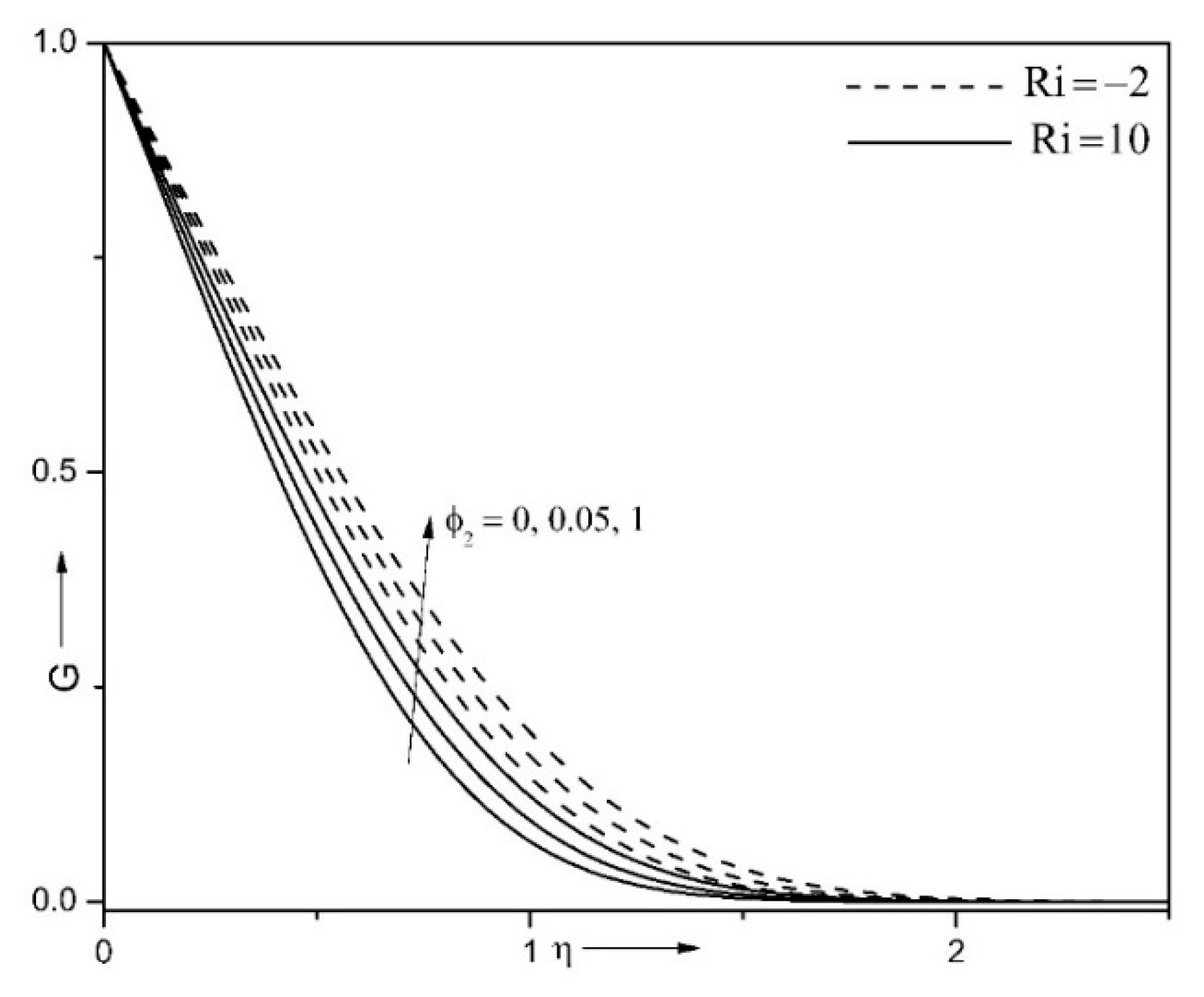
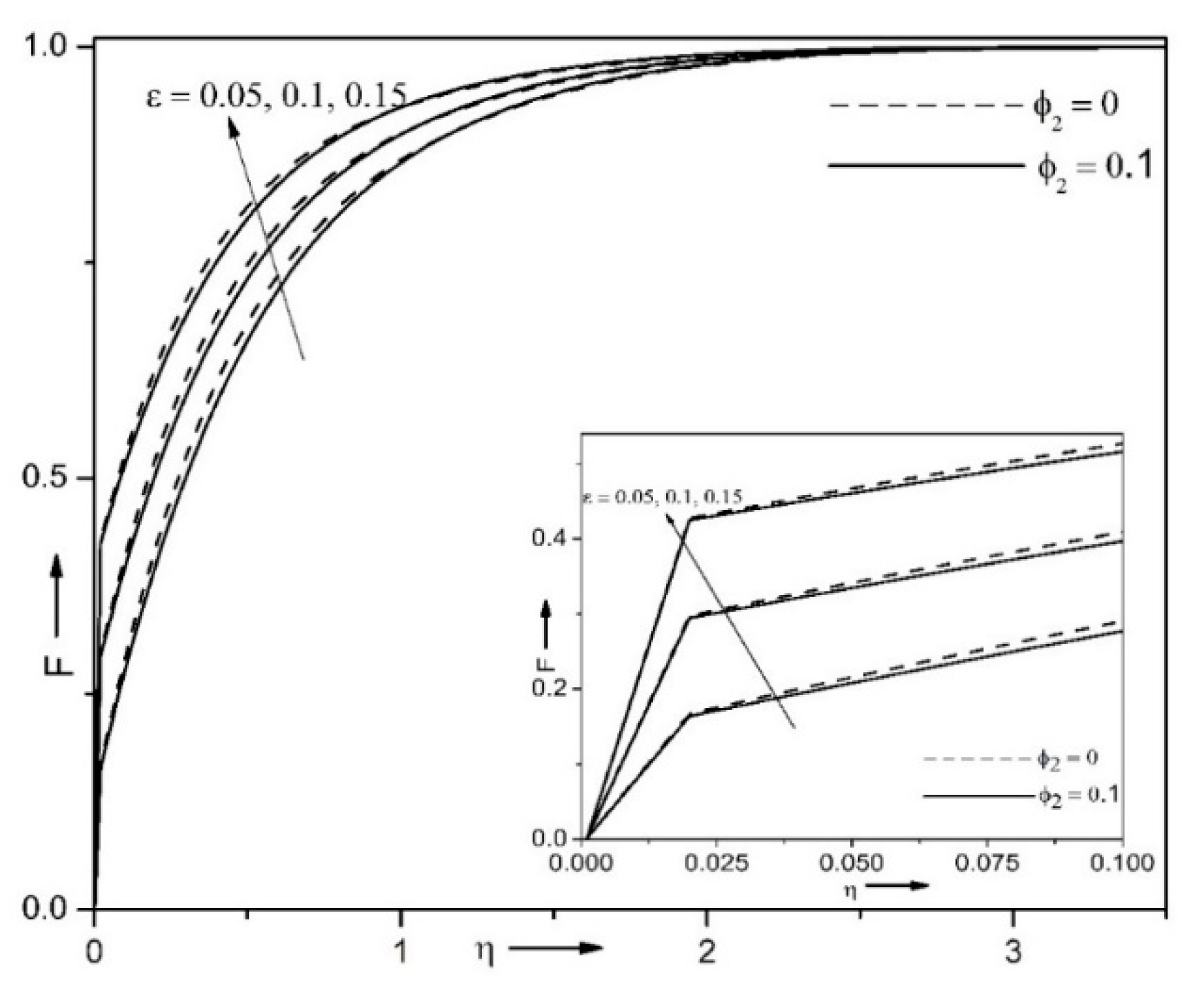

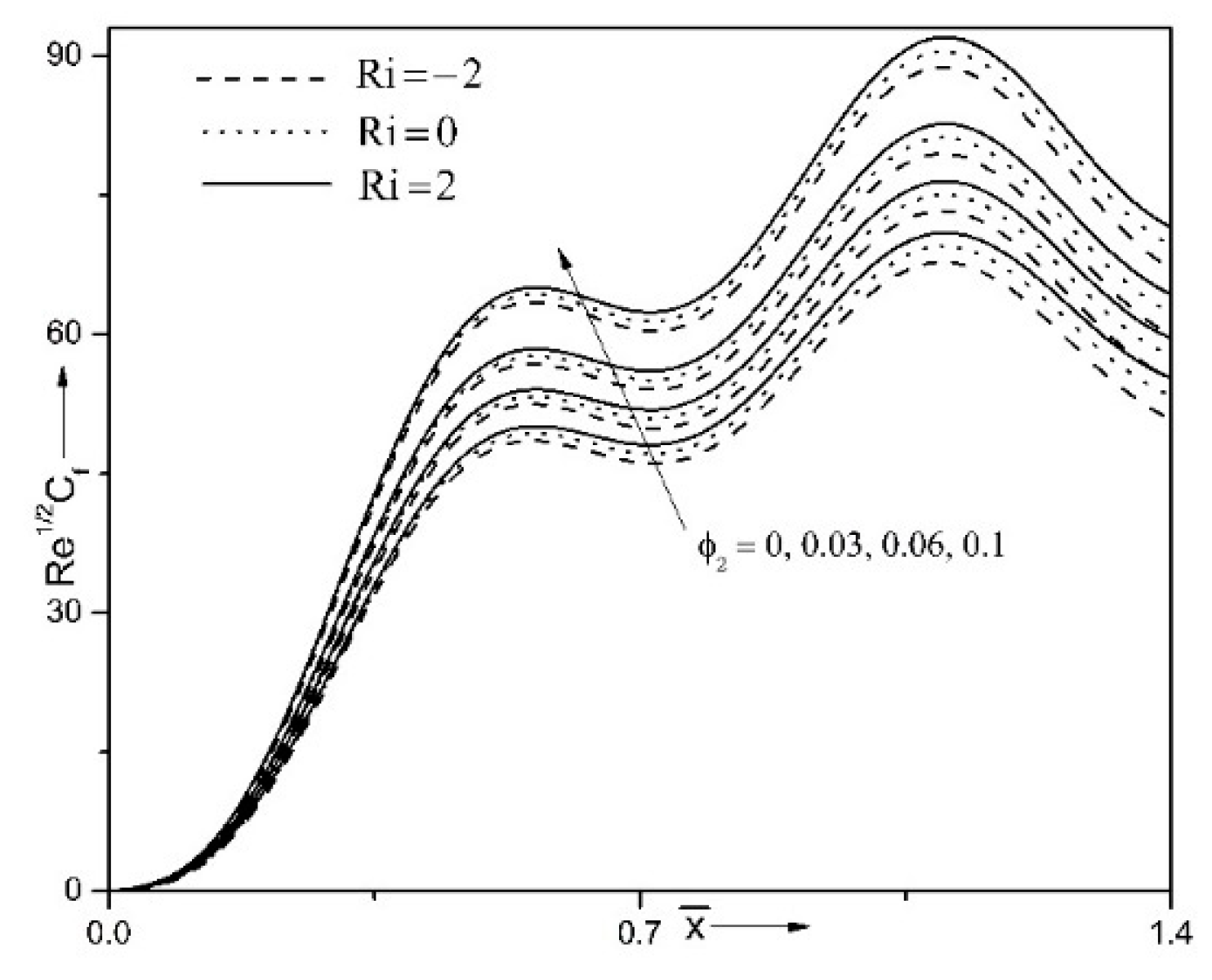
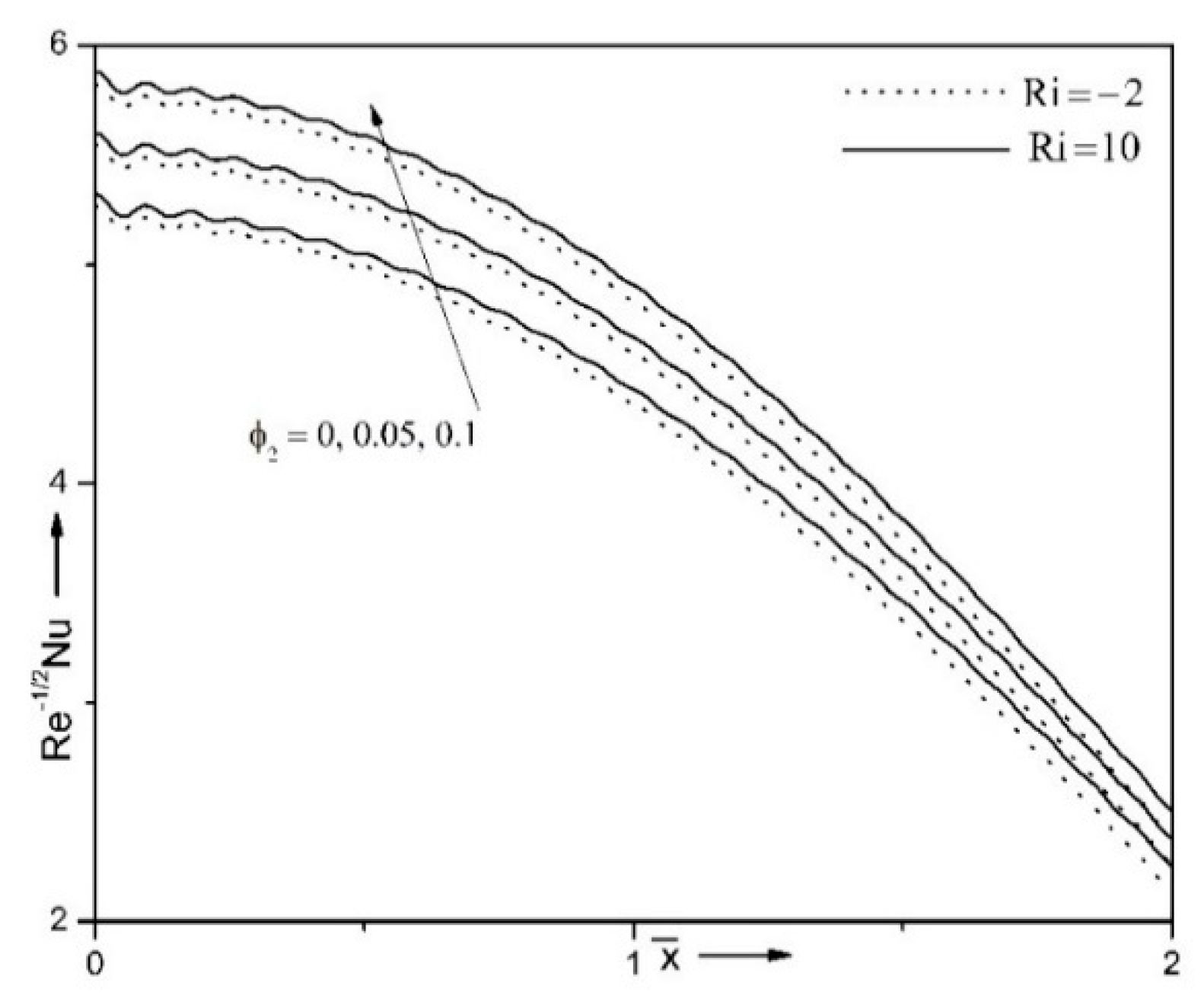
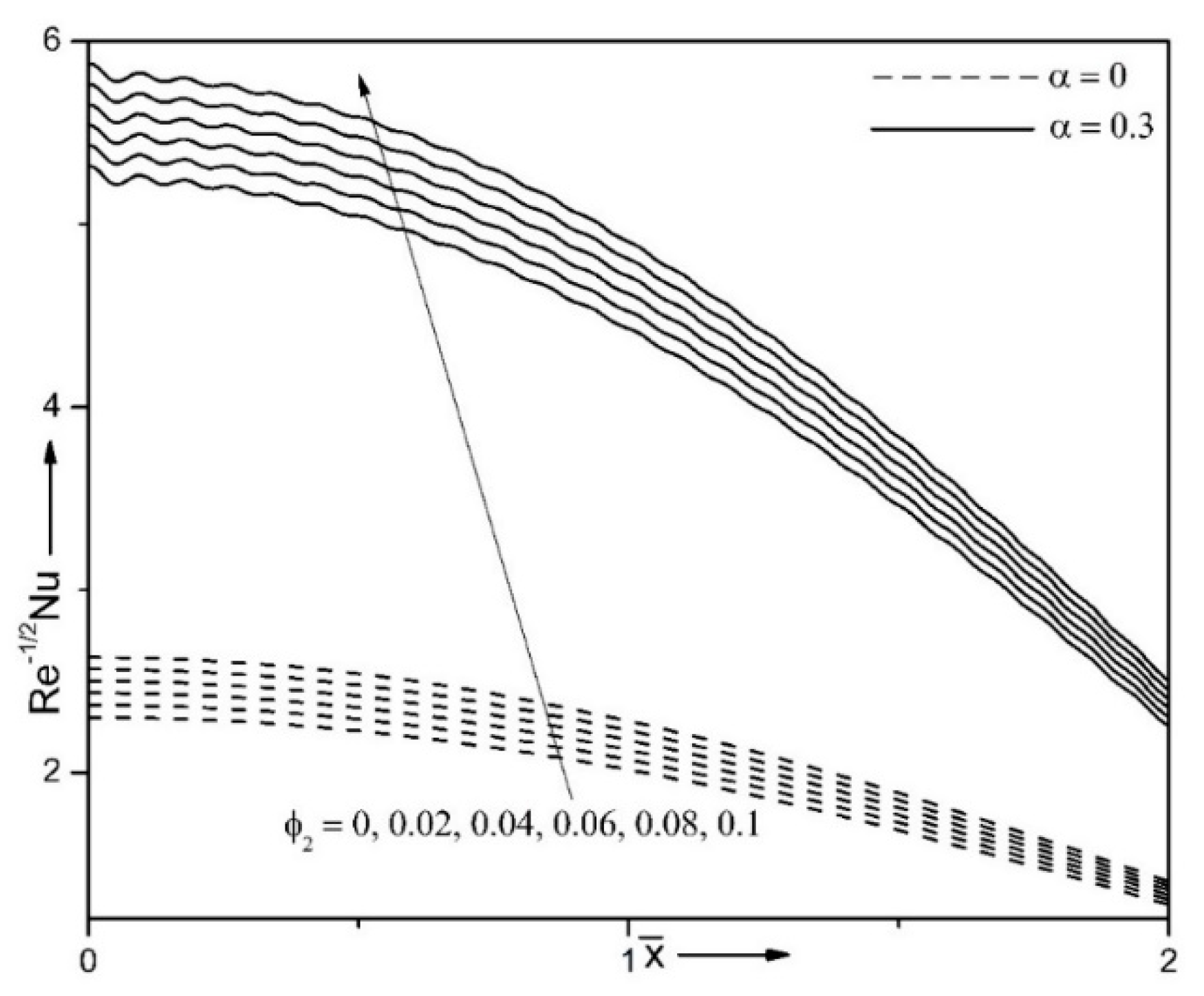
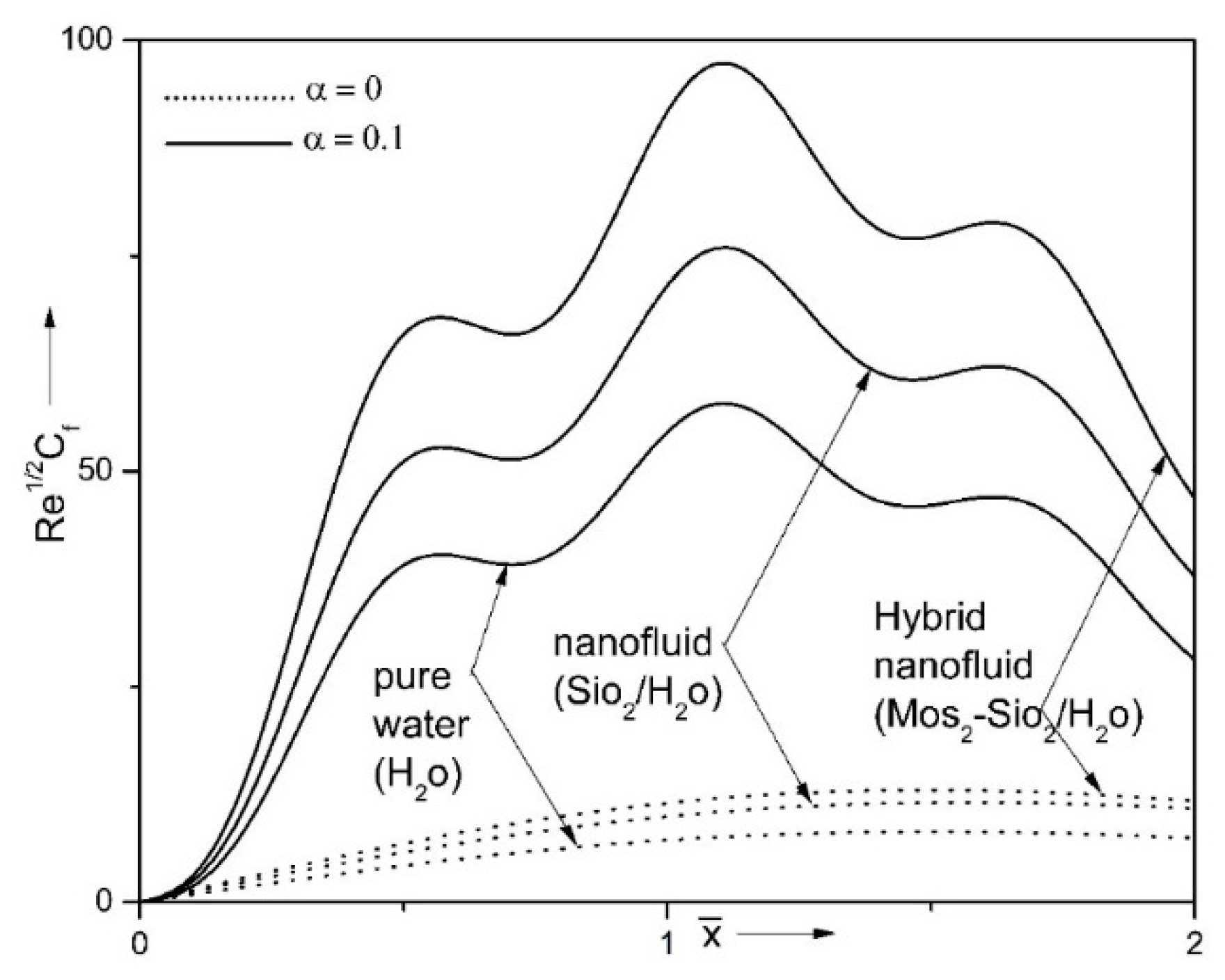

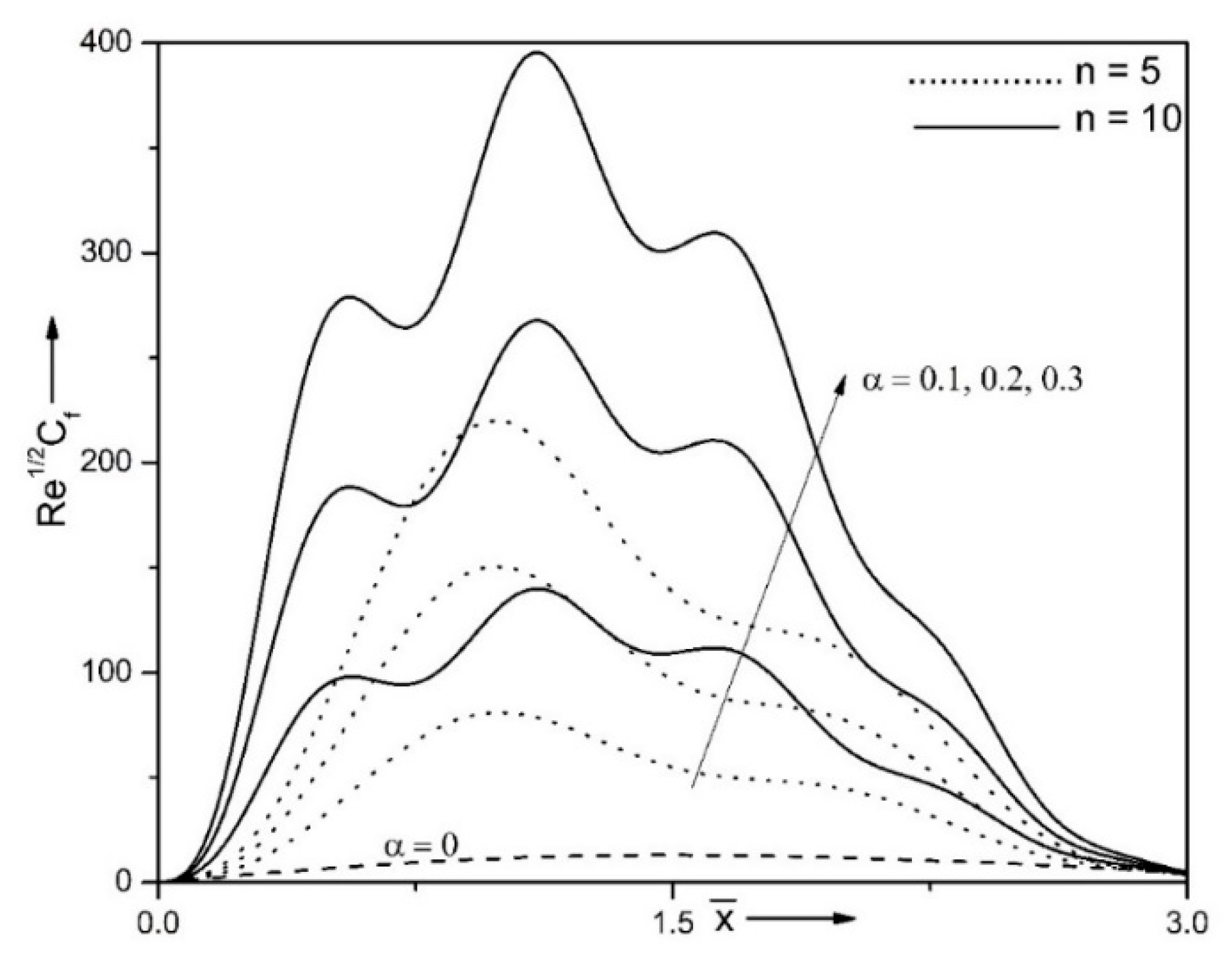
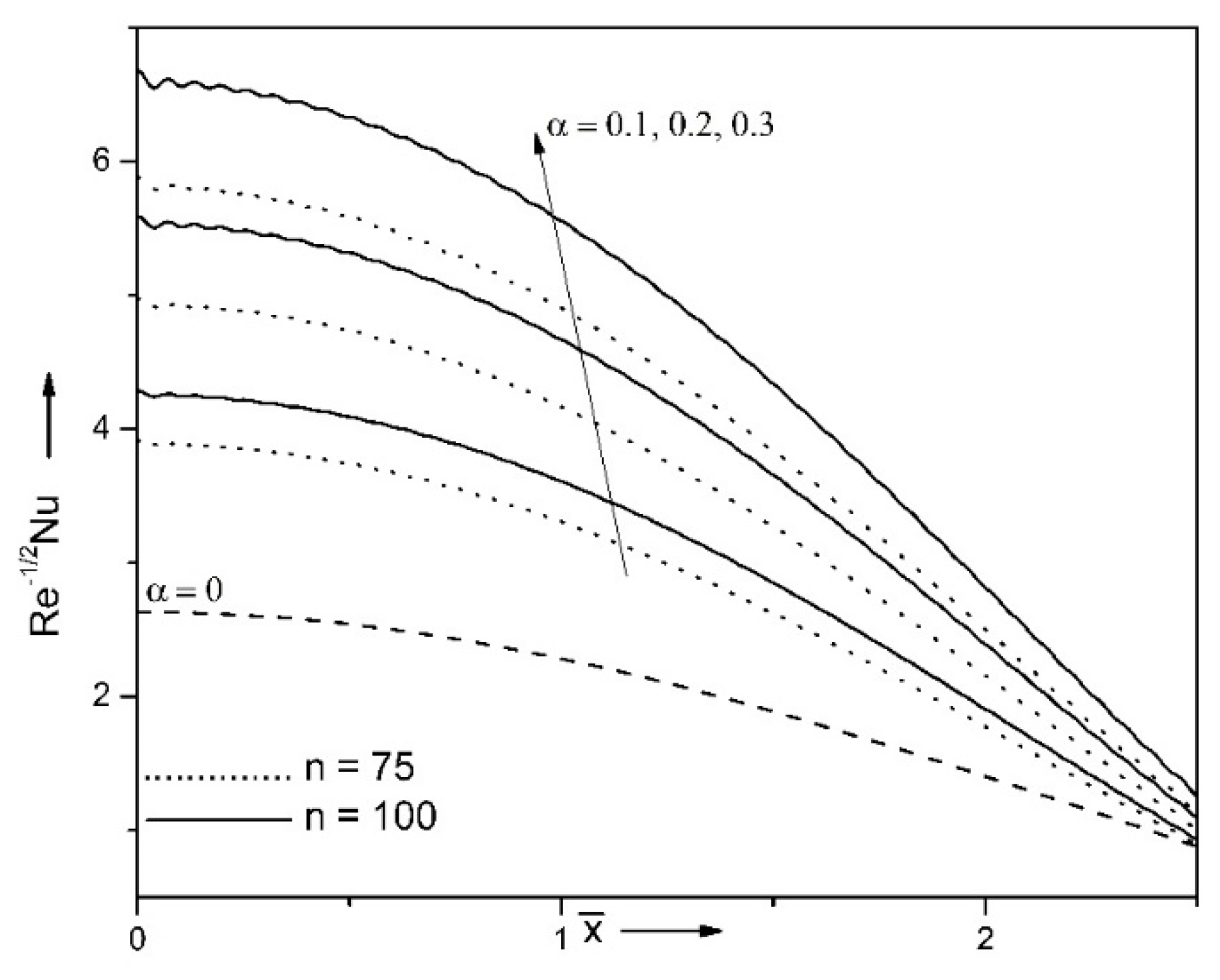
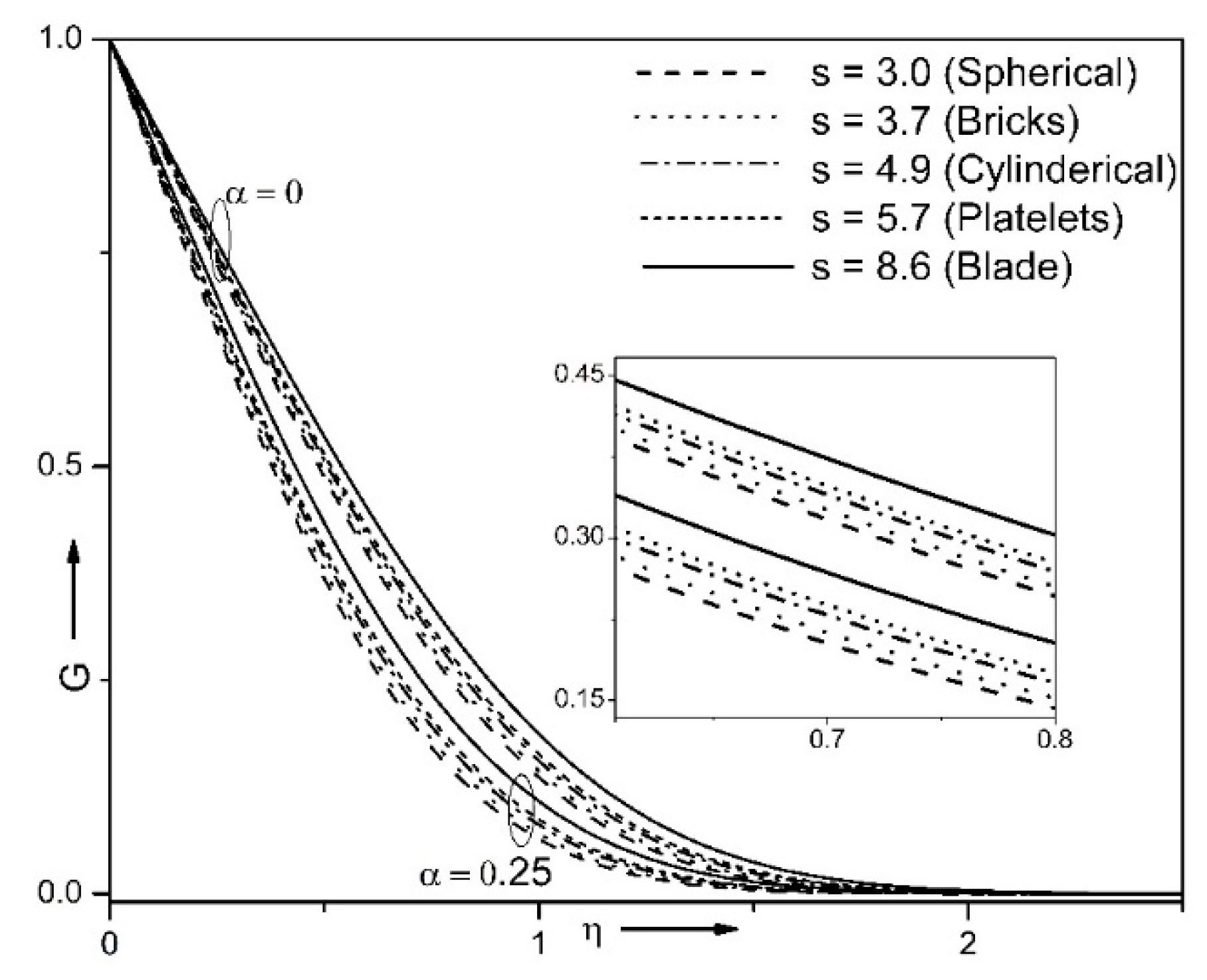
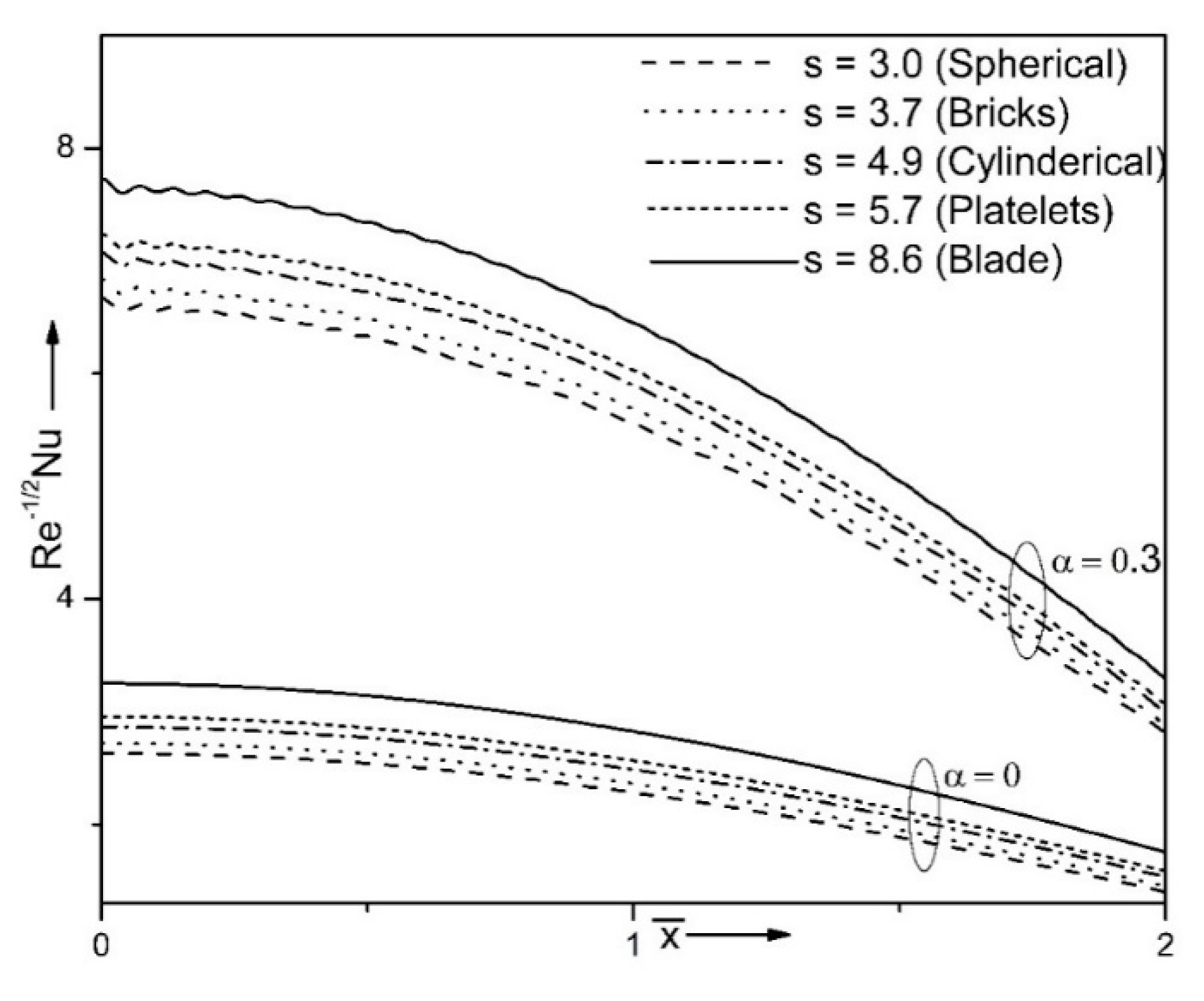

| Properties | Nanofluid | Hybrid Nanofluid |
|---|---|---|
| Dynamic viscosity | ||
| Density | ||
| Thermal conductivity | with | |
| Thermal expansion coefficient | ||
| Heat capacitance |
| Properties | Water | SiO2 | MoS2 |
|---|---|---|---|
| cp (J·kg−1·K−1) | 4179 | 730 | 397.746 |
| ρ (kg·m−3) | 997.1 | 2650 | 5060 |
| k (W·m−1·K−1) | 0.613 | 1.5 | 34.5 |
| Β × 10−5 (K−1) | 21.0 | 42.7 | 2.8242 |
| Nanoparticles Type | Geometry Parameter |
|---|---|
| Spherical | 3.0 |
| Bricks | 3.7 |
| Cylindrical | 4.9 |
| Platelets | 5.7 |
| Blade | 8.6 |
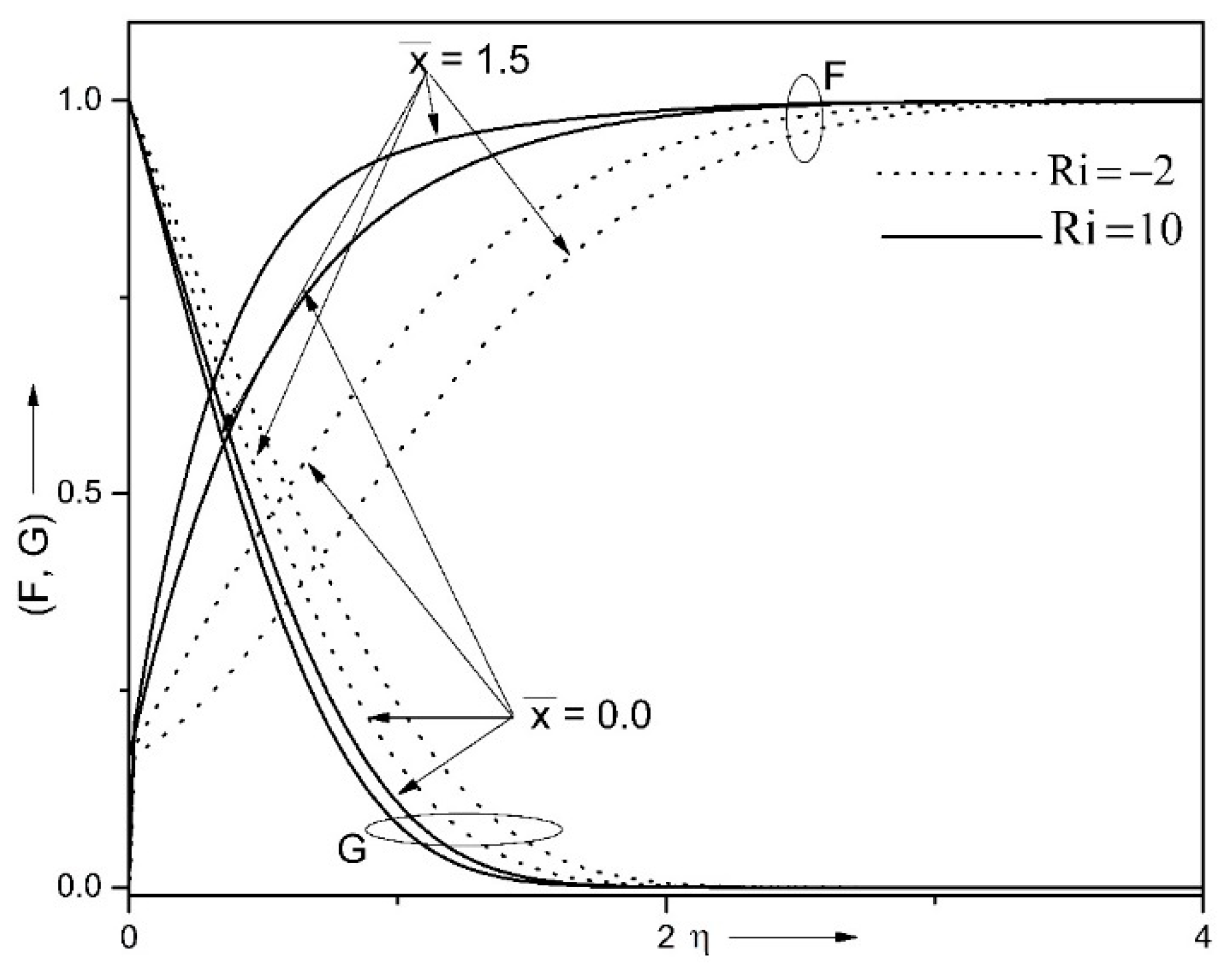
| Combined Convection Parameter (Ri) | Mono Nanofluid (φ1 = 0.1 and φ2 = 0.0) | Hybrid Nanofluid (φ1 = 0.1 and φ2 = 0.1) | |||
|---|---|---|---|---|---|
| Re1/2Cf | Re−1/2Nu | Re1/2Cf | Re−1/2Nu | ||
| Ri = −2 | smooth surface (α = 0) | 1.91924 | 1.73737 | 2.76707 | 2.07747 |
| Ri = 0 | 2.68659 | 1.85759 | 3.54184 | 2.18376 | |
| Ri = 3 | 3.71230 | 1.99284 | 4.60356 | 2.30996 | |
| Ri = 6 | 4.64655 | 2.10531 | 5.58725 | 2.41753 | |
| Ri = 10 | 5.79680 | 2.22851 | 6.81020 | 2.54115 | |
| Ri = −2 | rough surface (α = 0.25) | 115.67549 | 2.11400 | 150.75397 | 2.46100 |
| Ri = 0 | 116.33356 | 2.19692 | 151.43079 | 2.53750 | |
| Ri = 3 | 117.24895 | 2.30142 | 152.38640 | 2.63768 | |
| Ri = 6 | 118.10291 | 2.39003 | 153.28743 | 2.72483 | |
| Ri = 10 | 119.17278 | 2.49174 | 154.42511 | 2.82653 | |
| Nazar et al. [54] | Mohamed et al. [55] | Present Results | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Ri = −1 | Ri = 0 | Ri = 1 | Ri = −1 | Ri = 0 | Ri = 1 | Ri = −1 | Ri = 0 | Ri = 1 | |
| 00 | 0.7870 | 0.8162 | 0.8463 | 0.7858 | 0.8150 | 0.8406 | 0.7918 | 0.8180 | 0.8426 |
| 100 | 0.7818 | 0.8112 | 0.8371 | 0.7809 | 0.8103 | 0.8362 | 0.7778 | 0.8139 | 0.8385 |
| 200 | 0.7669 | 0.7974 | 0.8239 | 0.7615 | 0.7967 | 0.8232 | 0.7729 | 0.8008 | 0.8254 |
| 300 | 0.7422 | 0.7746 | 0.8024 | 0.7719 | 0.7741 | 0.8018 | 0.7497 | 0.7792 | 0.8054 |
| 400 | 0.7076 | 0.7429 | 0.7725 | 0.7074 | 0.7425 | 0.7721 | 0.7134 | 0.7475 | 0.7737 |
| 500 | 0.6624 | 0.7022 | 0.7345 | 0.6624 | 0.7032 | 0.7354 | 0.6754 | 0.7081 | 0.7377 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Patil, P.M.; Shankar, H.F.; Sheremet, M.A. Mixed Convection of Silica–Molybdenum Disulphide/Water Hybrid Nanoliquid over a Rough Sphere. Symmetry 2021, 13, 236. https://doi.org/10.3390/sym13020236
Patil PM, Shankar HF, Sheremet MA. Mixed Convection of Silica–Molybdenum Disulphide/Water Hybrid Nanoliquid over a Rough Sphere. Symmetry. 2021; 13(2):236. https://doi.org/10.3390/sym13020236
Chicago/Turabian StylePatil, Prabhugouda M., Hadapad F. Shankar, and Mikhail A. Sheremet. 2021. "Mixed Convection of Silica–Molybdenum Disulphide/Water Hybrid Nanoliquid over a Rough Sphere" Symmetry 13, no. 2: 236. https://doi.org/10.3390/sym13020236
APA StylePatil, P. M., Shankar, H. F., & Sheremet, M. A. (2021). Mixed Convection of Silica–Molybdenum Disulphide/Water Hybrid Nanoliquid over a Rough Sphere. Symmetry, 13(2), 236. https://doi.org/10.3390/sym13020236








