Self-Assembly of Hydrogen-Bonded Cage Tetramers of Phosphonic Acid
Abstract
:1. Introduction
2. Experimental
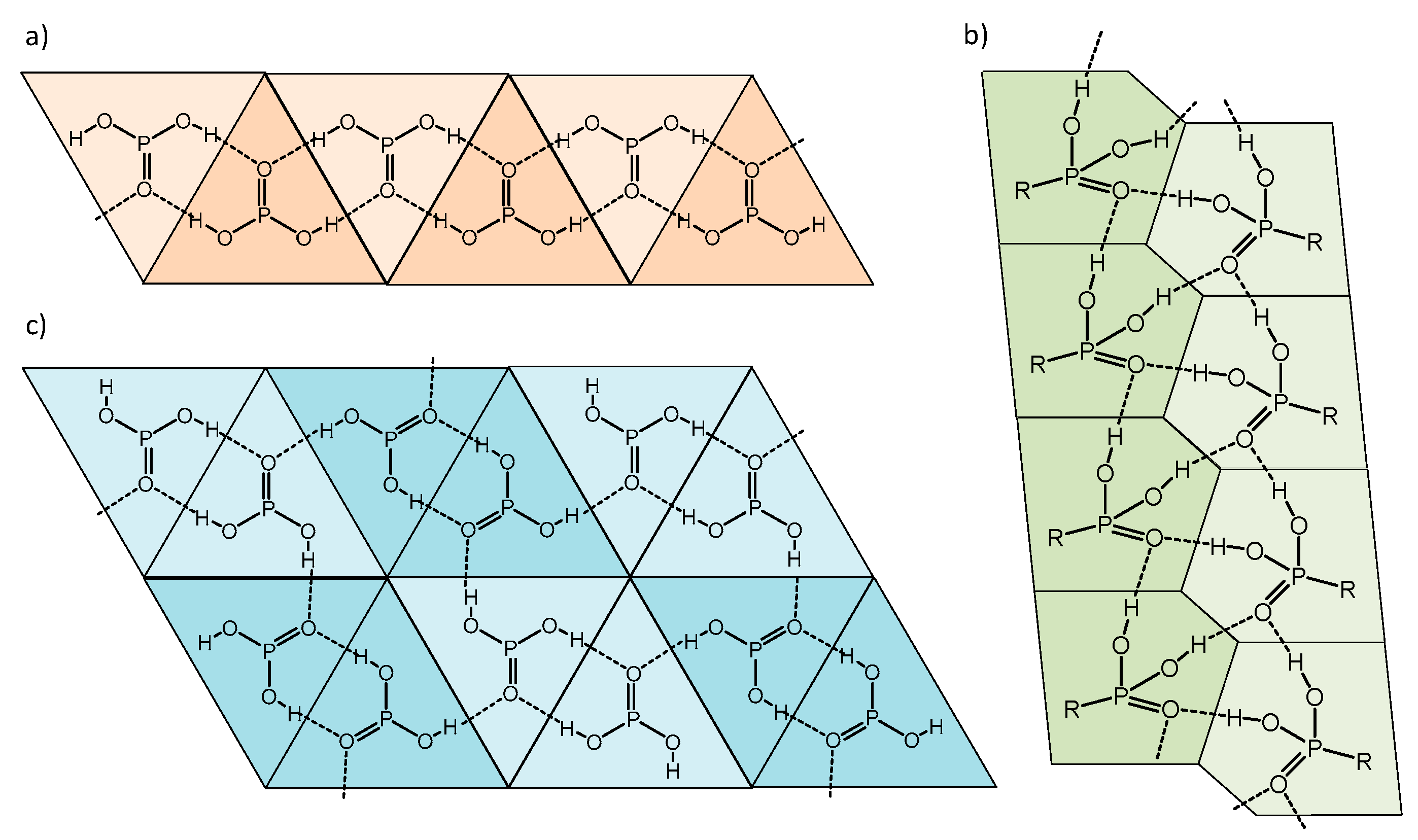
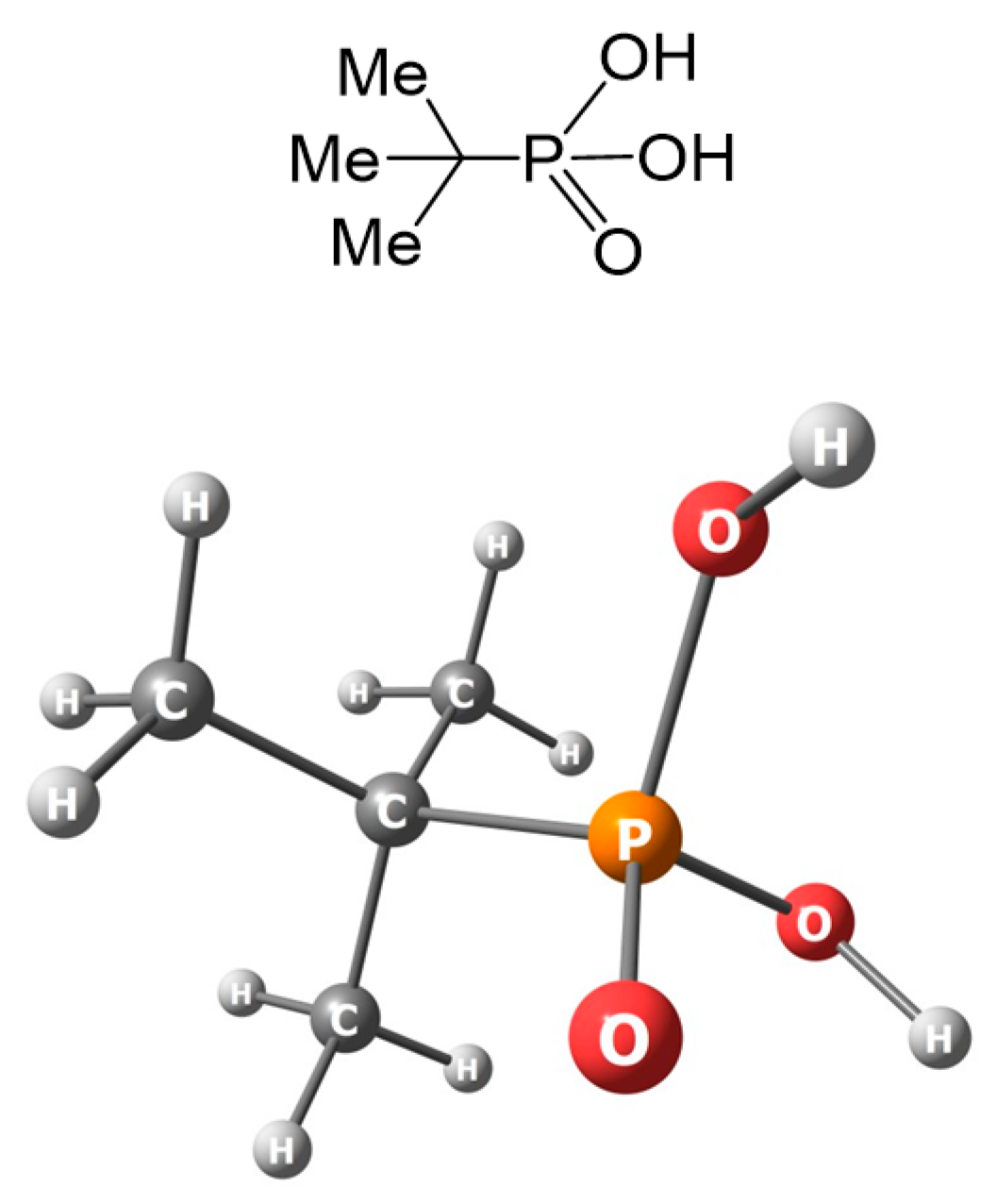
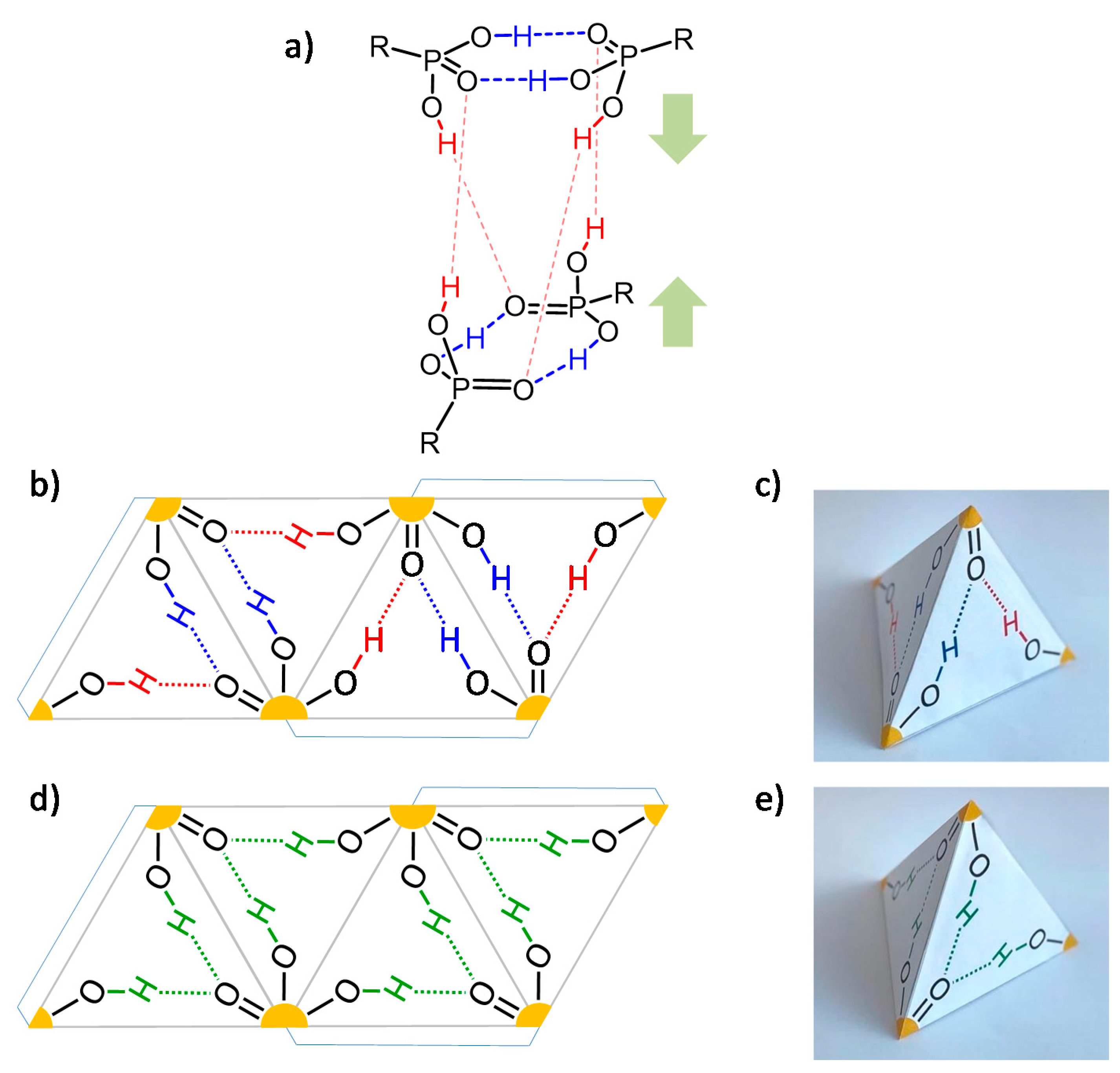
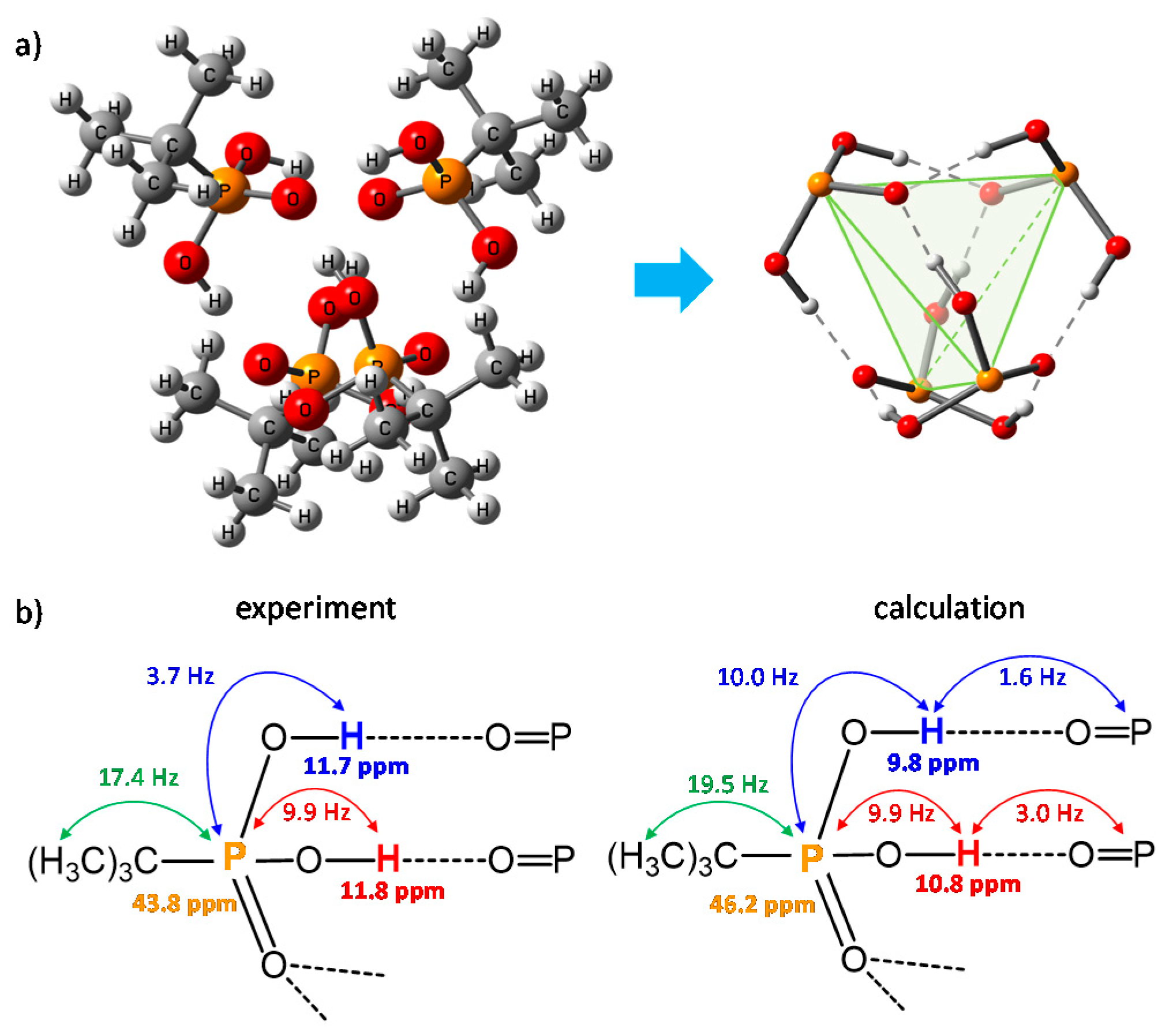
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Klein, O.; Aguilar–Parrilla, F.; Lopez, J.; Jagerovic, N.; Elguero, J.; Limbach, H.-H. Dynamic NMR Study of the Mechanisms of Double, Triple, and Quadruple Proton and Deuteron Transfer in Cyclic Hydrogen Bonded Solids of Pyrazole Derivatives. J. Am. Chem. Soc. 2004, 126, 11718–11732. [Google Scholar] [CrossRef] [Green Version]
- Castaneda, J.P.; Denisov, G.S.; Kucherov, S.Y.; Schreiber, V.M.; Shurukhina, A.V. Infrared and Ab Initio Studies of Hydrogen Bonding and Proton Transfer in the Complexes Formed by Pyrazoles. J. Mol. Struct. 2003, 660, 25–40. [Google Scholar] [CrossRef]
- Limbach, H.-H.; Seiffert, W. Dynamic Processes in Systems with Hydrogen Bonds. I. 1H–NMR Spectroscopic Study of the Cis-Trans Equilibrium and the Hydrogen Bond Association of N,Nˊ–bis(pentadeuterophenyl)–1–amino–3–iminopropene in Carbon Disulfide. Ber. Bunsen Ges. Phys. Chem. 1974, 78, 532–537. [Google Scholar]
- Limbach, H.-H.; Seiffert, W. Dynamic Processes in Systems with Hydrogen Bonds. II. 1H–NMR Spectroscopic Study of the Direct and Indirect Intermolecular Proton Exchange of N,Nˊ–bis(pentadeuterophenyl)–1–amino–3–iminopropene in Carbon Disulfide. Ber. Bunsen Ges. Phys. Chem. 1974, 78, 641–647. [Google Scholar]
- Lopez, J.; Maennle, F.; Wawer, I.; Buntkowsky, G.; Limbach, H.-H. NMR Studies of Double Proton Transfer in Hydrogen Bonded Cyclic N,Nˊ-Bis-arylformamidine Dimers: Conformational Control, Kinetic HH/HD/DD Isotope Effects and Tunneling. Phys. Chem. Chem. Phys. 2007, 9, 4498–4513. [Google Scholar] [CrossRef]
- Torres, V.; Lopez, J.-M.; Langer, U.; Buntkowsky, G.; Vieth, H.-M.; Elguero, J.; Limbach, H.-H. Kinetics of Coupled Double Proton and Deuteron Transfer in Hydrogen-Bonded Ribbons of Crystalline Pyrazole-4-carboxylic Acid. Z. Phys. Chem. 2012, 226, 1125–1147. [Google Scholar] [CrossRef] [Green Version]
- Xue, Q.; Horsewill, A.; Johnson, M.; Trommsdorff, H. Tunneling Dynamics of Double Proton Transfer in Formic Acid and Benzoic Acid Dimers. J. Chem. Phys. 2004, 120, 11107–11119. [Google Scholar] [CrossRef]
- Tolstoy, P.M.; Schah-Mohammedi, P.; Smirnov, S.N.; Golubev, N.S.; Denisov, G.S.; Limbach, H.-H. Characterization of Fluxional Hydrogen-Bonded Complexes of Acetic Acid and Acetate by NMR: Geometries and Isotope and Solvent Effects. J. Am. Chem. Soc. 2004, 126, 5621–5634. [Google Scholar] [CrossRef]
- Tolstoy, P.M.; Smirnov, S.N.; Shenderovich, I.G.; Golubev, N.S.; Denisov, G.S.; Limbach, H.-H. NMR Studies of Solid State—Solvent and H/D Isotope Effects on Hydrogen Bond Geometries of 1:1 Complexes of Collidine with Carboxylic Acids. J. Mol. Struct. 2004, 700, 19–27. [Google Scholar] [CrossRef]
- Gilli, G.; Gilli, P. The Nature of the Hydrogen Bond. Outline of a Comprehensive Hydrogen Bond Theory; Oxford University Press: Oxford, UK, 2009; p. 251. [Google Scholar]
- Asfin, R.E.; Denisov, G.S.; Mielke, Z.; Tokhadze, K.G. Particular Features of the ν(OH) Absorption Band of Strongly Hydrogen-Bonded Complexes in the Gas Phase, Low-Temperature Matrices, and Crystalline Films at 12–600 K. Opt. Spectrosc. 2005, 99, 56–67. [Google Scholar] [CrossRef]
- Detering, C.; Tolstoy, P.M.; Golubev, N.S.; Denisov, G.S.; Limbach, H.-H. Vicinal H/D Isotope Effects in NMR Spectra of Complexes with Coupled Hydrogen Bonds. Phosphoric Acids. Doklady Phys. Chem. 2001, 379, 1–4. [Google Scholar] [CrossRef]
- Giba, I.S.; Mulloyarova, V.V.; Denisov, G.S.; Tolstoy, P.M. Influence of Hydrogen Bonds in 1:1 Complexes of Phosphinic Acids with Substituted Pyridines on 1H and 31P NMR Chemical Shifts. J. Phys. Chem. A 2019, 123, 2252–2260. [Google Scholar] [CrossRef] [PubMed]
- Mulloyarova, V.V.; Giba, I.S.; Kostin, M.A.; Denisov, G.S.; Shenderovich, I.G.; Tolstoy, P.M. Cyclic Trimers of Phosphinic Acids in Polar Aprotic Solvent: Symmetry, Chirality and H/D Isotope Effects on NMR Chemical Shifts. Phys. Chem. Chem. Phys. 2018, 20, 4901–4910. [Google Scholar] [CrossRef] [PubMed]
- Giba, I.S.; Mulloyarova, V.V.; Denisov, G.S.; Tolstoy, P.M. Sensitivity of 31P NMR Chemical Shifts to Hydrogen Bond Geometry and Molecular Conformation for Complexes of Phosphinic Acids with Pyridines. Magn. Reson. Chem. 2021. [Google Scholar] [CrossRef] [PubMed]
- Mulloyarova, V.V.; Giba, I.S.; Denisov, G.S.; Tolstoy, P.M. Conformational Mobility and Proton Transfer in Hydrogen-bonded Dimers and Trimers of Phosphinic and Phosphoric acids. J. Phys. Chem A 2019, 123, 6761–6771. [Google Scholar] [CrossRef] [PubMed]
- Asfin, R.E.; Denisov, G.S.; Tokhadze, K.G. The Infrared Spectra and Enthalpies of Strongly Bound Dimers of Phosphinic Acids in the Gas Phase. (CH2Cl)2POOH and (C6H5)2POOH. J. Mol. Struct. 2002, 608, 161–168. [Google Scholar] [CrossRef]
- Asfin, R.E.; Denisov, G.S.; Poplevchenkov, D.N.; Tokhadze, K.G.; Velikanova, T.V. IR ν(OH) Band and Dimerization of Phosphorous Acids in the Gas Phase and Solid State. Pol. J. Chem. 2002, 76, 1223–1231. [Google Scholar]
- Mó, O.; Yáñez, M.; Gonzalez, L.; Elguero, J. Spontaneous Self-ionization in the Gas Phase: A Theoretical Prediction. Chem. Phys. Chem. 2001, 2, 465–467. [Google Scholar] [CrossRef]
- Ahmadi, I.; Rahemi, H.; Tayyari, S.F. Structural, Potential Surface and Vibrational Spectroscopy Studies of Hypophosphorous Acid in the Gas Phase and Chain Conformation. A Theoretical Study. J. Korean Chem. Soc. 2005, 49, 129–137. [Google Scholar] [CrossRef] [Green Version]
- Gonzalez, L.; Mó, O.; Yáñez, M.; Elguero, J. Very Strong Hydrogen Bonds in Neutral Molecules: The phosphinic acid dimers. J. Chem. Phys. 1998, 109, 2685–2693. [Google Scholar] [CrossRef]
- Picazo, O.; Alkorta, I.; Elguero, J.; Mó, O.; Yáñez, M. Chiral Recognition in Phosphinic Acid Dimers. J. Phys. Org. Chem. 2005, 18, 491–497. [Google Scholar] [CrossRef] [Green Version]
- Rekik, N.; Ghalla, H.; Hanna, G. Explaining the Structure of the OH Stretching Band in the IR Spectra of Strongly Hydrogen-bonded Dimers of Phosphinic Acid and Their Deuterated Analogs in the Gas Phase: A Computational Study. J. Phys. Chem. A 2012, 116, 4495–4509. [Google Scholar] [CrossRef]
- Yue, B.; Yan, L.; Han, S.; Xie, L. Proton Transport Pathways in an Acid–Base Complex Consisting of a Phosphonic Acid Group and a 1,2,3-Triazolyl Group. J. Phys. Chem. B 2013, 117, 7941–7949. [Google Scholar] [CrossRef] [PubMed]
- Fedorova, I.V.; Krishtal, S.P.; Kiselev, M.G.; Safonova, L.P. Structure of Orthophosphoric Acid-N,N-dimethylformamide Complexes. Russian J. Phys. Chem. 2006, 80, 7–13. [Google Scholar] [CrossRef]
- Yoo, T.; Nguyen, H.D.; Nilsson, M. Molecular Dynamics Investigations of Di-Butyl-Phosphoric Acid—Parameterization and Dimerization. J. Phys. Chem. B 2018, 122, 12040–12048. [Google Scholar] [CrossRef] [PubMed]
- Solka, J.L.; Reis, A.H., Jr.; Mason, G.W.; Lewey, S.M.; Peppard, D.F. Sterically Hindered Solvent Extractants-III: The Molecular and Crystal Structure and Heavy-Element Extraction Properties of the Di-t-pentylphosphinic Acid Dimer. J. Inorg. Nucl. Chem. 1978, 40, 663–668. [Google Scholar] [CrossRef]
- Fenske, D.; Mattes, R.; Lons, J.; Tebbe, K.F. Die Kristallstruktur von Diphenylphosphinsaure. Chem. Ber. 1973, 106, 1139–1144. (In German) [Google Scholar] [CrossRef]
- Reis, A.H., Jr.; Peterson, S.W.; Druyan, M.E.; Gebert, E.; Mason, G.W.; Peppard, D.F. Sterically Hindered Solvent Extractants. 2. A Neutron-Diffraction Study of the Di-tertbutylphosphinic Acid Dimer Showing Strong Asymmetric Hydrogen Bonding. Inorg. Chem. 1976, 15, 2748–2752. [Google Scholar] [CrossRef]
- Druyan, M.E.; Reis, A.H., Jr.; Gebert, E.; Peterson, S.W.; Mason, G.W.; Peppard, D.F. Dimeric Structure of di-tert-butylphosphinic Acid. J. Amer. Chem. Soc. 1976, 98, 4801–4805. [Google Scholar] [CrossRef]
- Bruckmann, J.; Kruger, C.; Lehmann, C.W.; Leitner, W.; Rust, J.; Six, C. Ethylenebis(phosphonic acid). Acta Cryst. C 1999, 55, 695–696. [Google Scholar] [CrossRef] [Green Version]
- Fatila, E.M.; Pink, M.; Twum, E.B.; Karty, J.A.; Flood, A.H. Phosphate–Phosphate Oligomerization Drives Higher Order Co-assemblies with Stacks of Cyanostar Macrocycles. Chem Sci. 2018, 9, 2863–2872. [Google Scholar] [CrossRef] [Green Version]
- Mulloyarova, V.V.; Ustimchuk, D.O.; Filarowski, A.; Tolstoy, P.M. H/D Isotope Effects on 1H NMR Chemical Shifts in Cyclic Heterodimers and Heterotrimers of Phosphinic and Phosphoric Acids. Molecules 2020, 25, 1907. [Google Scholar] [CrossRef] [Green Version]
- Merz, K.; Knüfer, A. Cyclohexylphosphonic Acid. Acta Cryst. 2002, C58, o187–o188. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Belabassi, Y.; Gushwa, A.F.; Richards, A.F.; Montchamp, J.-L. Structural Analogues of Bioactive Phosphonic Acids: First Crystal Structure Characterization of Phosphonothioic and Boranophosphonic Acids. Elem. Phosphorus Sulfur Silicon Relat. Elem. 2008, 183, 2214–2228. [Google Scholar] [CrossRef]
- Aragoni, M.C.; Arca, M.; Blake, A.J.; Lippolis, V.; Schroder, M.; Wilson, C. 4-Methoxyphenylphosphonic Acid: Reactivity of Lawesson’s Reagent. Acta Cryst. 2002, C58, o260–o262. [Google Scholar] [CrossRef] [PubMed]
- Mahmoudkhania, A.H.; Langer, V. Phenylphosphonic Acid as a Building Block for Two-Dimensional Hydrogen-Bonded Supramolecular Arrays. J. Mol. Struct. 2002, 609, 97–108. [Google Scholar] [CrossRef]
- Desiraju, G.R. Supramolecular Synthons in Crystal Engineering—A New Organic Synthesis. Angew. Chem Int. Ed. Engl. 1995, 34, 2311–2327. [Google Scholar] [CrossRef]
- Etter, M. Encoding and Decoding Hydrogen-Bond Patterns of Organic Compounds. Acc. Chem. Res. 1990, 23, 120–126. [Google Scholar] [CrossRef]
- Aiello, D.; Folliet, N.; Laurent, G.; Testa, F.; Gervais, C.; Babonneau, F.; Azaïs, T. Solid state NMR Characterization of Phenylphosphonic Acid Encapsulated in SBA-15 and Aminopropyl-modified SBA-15. Micropor. Mesopor. Mat. 2013, 166, 109–116. [Google Scholar] [CrossRef]
- Kreuer, K.; Paddison, S.; Spohr, E.; Schuster, M. Transport in Proton Conductors for Fuel-Cell Applications: Simulations, Elementary Reactions, and Phenomenology. Chem. Rev. 2004, 104, 4637–4678. [Google Scholar] [CrossRef] [Green Version]
- Ye, G.; Janzen, N.; Goward, G. Solid-State NMR Study of Two Classic Proton Conducting Polymers: Nafion and Sulfonated Poly(ether ether ketone)s. Macromolecules 2006, 39, 3283–3290. [Google Scholar] [CrossRef]
- Thompson, E.; Capehart, T.; Fuller, T.; Jorne, J. Investigation of Low-Temperature Proton Transport in Nafion Using Direct Current Conductivity and Differential Scanning Calorimetry. Electrochem. Soc. 2006, 153, A2351–A2362. [Google Scholar] [CrossRef]
- Zhao, R.; Rupper, P.; Gaan, S. Recent Development in Phosphonic Acid-Based Organic Coatings on Aluminum. Coatings 2017, 7, 133. [Google Scholar] [CrossRef] [Green Version]
- Gawalt, E.S.; Avaltroni, M.J.; Koch, N.; Schwart, J. Self-Assembly and Bonding of Alkanephosphonic Acids on the Native Oxide Surface of Titanium. Langmuir 2001, 17, 5736–5738. [Google Scholar] [CrossRef]
- Adden, N.; Gamble, L.J.; Castner, D.G.; Hoffmann, A.; Gross, G.; Menzel, H. Phosphonic Acid Monolayers for Binding of Bioactive Molecules to Titanium Surfaces. Langmuir 2006, 22, 8197–8204. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wan, X.; Lieberman, I.; Asyuda, A.; Resch, S.; Seim, H.; Kirsch, P.; Zharnikov, M. Thermal Stability of Phosphonic Acid Self-Assembled Monolayers on Alumina Substrates. J. Phys. Chem. C 2020, 124, 2531–2542. [Google Scholar] [CrossRef]
- Cao, L.; Peng, Y.; Li, Z. Phosphonic Acid Self-assembled Monolayer Improved Properties of n-type Organic Field-effect Transistors in Air Ambient. RSC Adv. 2016, 6, 89794–89798. [Google Scholar] [CrossRef]
- Bamba, T.; Ohtake, T.; Ohata, Y.; Nie, H.-Y.; Ban, T.; Yamamoto, S.-I. Octadecylphosphonic Acid Self-assembled Monolayers Obtained Using Rapid Dipping Treatments. Trans. Mat. Res. Soc. Jpn. 2018, 43, 305–309. [Google Scholar] [CrossRef] [Green Version]
- Siegel, J.S.; Anet, F.A.I. Dichlorofluoromethane-d: A Versatile Solvent for VT-NMR Experiments. J. Org. Chem. 1988, 53, 2629–2630. [Google Scholar] [CrossRef]
- Harris, R.K.; Becker, E.D.; Cabral de Menezes, S.M.; Goodfellow, R.; Granger, P. NMR Nomenclature. Nuclear Spin Properties and Conventions for Chemical Shifts (IUPAC Recommendations 2001). Pure Appl. Chem. 2001, 73, 1795–1818. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian, Inc.: Wallingford, CT, USA. 2016. Available online: http://gaussian.com/citation/ (accessed on 20 January 2021).
- Arey, J.S.; Aeberhard, P.C.; Lin, I.-C.; Rothlisberger, U. Hydrogen Bonding Described Using Dispersion-Corrected Density Functional Theory. J. Phys. Chem. B 2009, 113, 4726–4732. [Google Scholar] [CrossRef] [PubMed]
- Latypov, S.; Polyancev, F.; Yakhvarov, D.; Sinyashina, O. Quantum Chemical Calculations of 31P NMR Chemical Shifts: Scopes and Limitations. Phys. Chem. Chem. Phys. 2015, 17, 6976–6987. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dennington, R.; Keith, T.A.; Millam, J.M. GaussView, Version 5; Semichem Inc.: Shawnee Mission, KS, USA, 2009. [Google Scholar]
- Chemcraft, Version 1.8. Available online: www.chemcraftprog.com (accessed on 20 January 2021).
- Budzelaar, P.H.M. gNMR; Adept Scientific: Letchworth, UK, 2002. [Google Scholar]
- Biegler-König, F.; Schönbohm, J.; Bayles, D. AIM2000—A Program to Analyze and Visualize Atoms in Molecules. J. Comp. Chem. 2001, 22, 545–559. [Google Scholar]
- Biegler-König, F.; Schönbohm, J. An Update to the AIM2000—Program for Atoms in Molecules. J. Comp. Chem. 2002, 23, 1489–1494. [Google Scholar] [CrossRef]
- Shenderovich, I.G.; Burtsev, A.P.; Denisov, G.S.; Golubev, N.S.; Limbach, H.-H. Influence of the Temperature-dependent Dielectric Constant on the H/D Isotope Effects on the NMR Chemical Shifts and the Hydrogen Bond Geometry of the Collidine-HF Complex in CDF3/CDClF2 Solution. Magn. Reson. Chem. 2001, 39, 91–99. [Google Scholar] [CrossRef]
- Dohnal, V.; Tkadlecova, M. A Simple Relation between 1H NMR Data and Mixing Enthalpy for Systems with Complex Formation by Hydrogen Bonding. J. Phys. Chem. B 2002, 106, 12307–12310. [Google Scholar] [CrossRef]
- Ishikawa, R.; Kojima, C.; Ono, A.; Kainosho, M. Developing Model Systems for the NMR Study of Substituent Effects on the N—H···N Hydrogen Bond in Duplex DNA. Magn. Reson. Chem. 2001, 39, 159–165. [Google Scholar] [CrossRef]
- Limbach, H.-H.; Tolstoy, P.M.; Perez-Hernandez, N.; Guo, J.; Shenderovich, I.G.; Denisov, G.S. OHO Hydrogen Bond Geometries and NMR Chemical Shifts: From Equilibrium Structures to Geometric H/D Isotope Effects with Applications for Water, Protonated Water and Compressed Ice. Israel J. Chem. 2009, 49, 199–216. [Google Scholar] [CrossRef]
- Sorgenfrei, N.; Hioe, J.; Greindl, J.; Rothermel, K.; Morana, F.; Lokesh, N.; Gschwind, R.M. NMR Spectroscopic Characterization of cCharge Assisted Strong Hydrogen Bonds in Brønsted Acid Catalysis. J. Am. Chem. Soc. 2016, 138, 16345–16354. [Google Scholar] [CrossRef] [Green Version]
- Silski-Devlin, A.M.; Petersen, J.P.; Liu, J.; Turner, G.A.; Poutsma, J.C.; Kandel, S.A. Hydrogen-bonded Tetramers of Carbamazepine. J. Phys. Chem. C 2020, 124, 5213–5219. [Google Scholar] [CrossRef]
- Dilovic, I.; Matkovic-Calogovic, D.; Kos, M.I. N-Benzyloxy-1H-benzotriazole-1-Carboxamide: A Hydrogen-bonded Tetramer Based upon a Rare R(4)4(20) Structural motif. Acta Cryst. C 2008, 64, 434–436. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Xue, M.; Marshall, L.J.; de Mendoza, J. Hydrogen-bonded Cyclic Tetramers Based on Ureidopyrimidinones Attached to a 3,6-carbazolyl Spacer. Org. Lett. 2011, 13, 3186–3189. [Google Scholar] [CrossRef] [PubMed]
- Sagan, B.L.; Walmsley, J.A. Evidence for the Existence of Hydrogen-bonded Cyclic Tetramers in Aqueous Solutions of Tetramethylammonium Guanosine 5′-monophosphate. Biochem. Biophys. Res. Comm. 1985, 128, 980–986. [Google Scholar] [CrossRef]
- Larsen, R.W.; Suhm, M.A. The Benefits of Alternation and Alkylation: Large Amplitude Hydrogen Bond Librational Modes of Alcohol Trimers and Tetramers. Phys. Chem. Chem. Phys. 2010, 12, 8152–8157. [Google Scholar] [CrossRef] [PubMed]
- Mella, M.; Harris, K.D.M. Pathways for Hydrogen Bond Switching in a Tetrameric Methanol Cluster. Phys. Chem. Chem. Phys. 2009, 11, 11340–11346. [Google Scholar] [CrossRef]
- Denisov, G.S.; Tokhadze, K.G. Ultrastrong Hydrogen Bond in Gas Phase. Dimer of Dimethylphosphinic Acid. Dokl. Phys. Chem. 1994, 337, 117–119. [Google Scholar]
- Espinosa, E.; Molins, E.; Lecomte, C. Hydrogen Bond Strengths Revealed by Topological Analyses of Experimentally Observed Electron Densities. Chem. Phys. Lett. 1998, 285, 170–173. [Google Scholar] [CrossRef]
- Mata, I.; Alkorta, I.; Espinosa, E.; Molins, E. Relationships between Interaction Energy, Intermolecular Distance and Electron Density Properties in Hydrogen Bonded Complexes under External Electric Fields. Chem. Phys. Lett. 2011, 507, 185–189. [Google Scholar] [CrossRef]
- Kumar, G.A.; McAllister, M.A. Theoretical Investigation of the Relationship between Proton NMR Chemical Shift and Hydrogen Bond Strength. J. Org. Chem. 1998, 63, 6968–6972. [Google Scholar] [CrossRef]
- Tupikina, E.Y.; Sigalov, M.; Shenderovich, I.G.; Mulloyarova, V.V.; Denisov, G.S.; Tolstoy, P.M. Correlations of NHN hydrogen bond energy with geometry and 1H NMR chemical shift difference of NH protons for aniline complexes. J. Chem. Phys. 2019, 150, 114305. [Google Scholar] [CrossRef] [PubMed]
- Denisov, G.S.; Bureiko, S.F.; Kucherov, S.Y.; Tolstoy, P.M. Correlation Relationships between the Energy and Spectroscopic Parameters of Complexes with F···HF Hydrogen Bond. Doklady Phys. Chem. 2017, 475, 115–118. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Giba, I.S.; Tolstoy, P.M. Self-Assembly of Hydrogen-Bonded Cage Tetramers of Phosphonic Acid. Symmetry 2021, 13, 258. https://doi.org/10.3390/sym13020258
Giba IS, Tolstoy PM. Self-Assembly of Hydrogen-Bonded Cage Tetramers of Phosphonic Acid. Symmetry. 2021; 13(2):258. https://doi.org/10.3390/sym13020258
Chicago/Turabian StyleGiba, Ivan S., and Peter M. Tolstoy. 2021. "Self-Assembly of Hydrogen-Bonded Cage Tetramers of Phosphonic Acid" Symmetry 13, no. 2: 258. https://doi.org/10.3390/sym13020258
APA StyleGiba, I. S., & Tolstoy, P. M. (2021). Self-Assembly of Hydrogen-Bonded Cage Tetramers of Phosphonic Acid. Symmetry, 13(2), 258. https://doi.org/10.3390/sym13020258






