Effect of Natural Wind on the Transiting Test for Measuring the Aerodynamic Coefficients of Structures
Abstract
:1. Introduction
2. Experimental Methodology
2.1. Introduction of the Test and Model
2.2. Equipment and Data Acquisition System
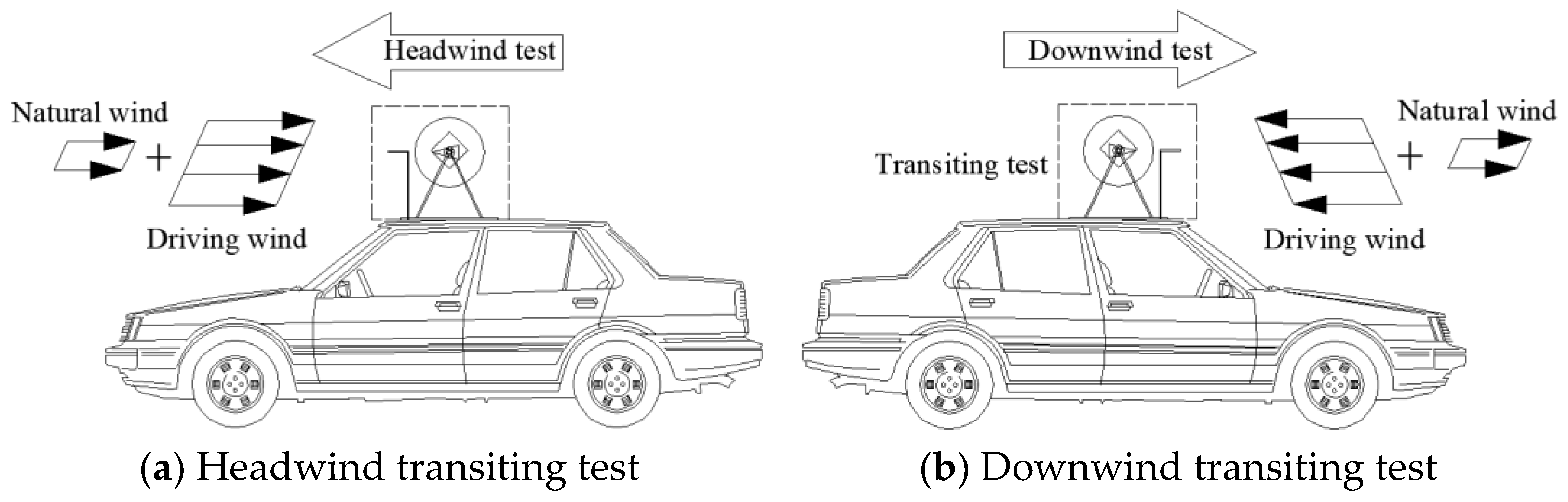
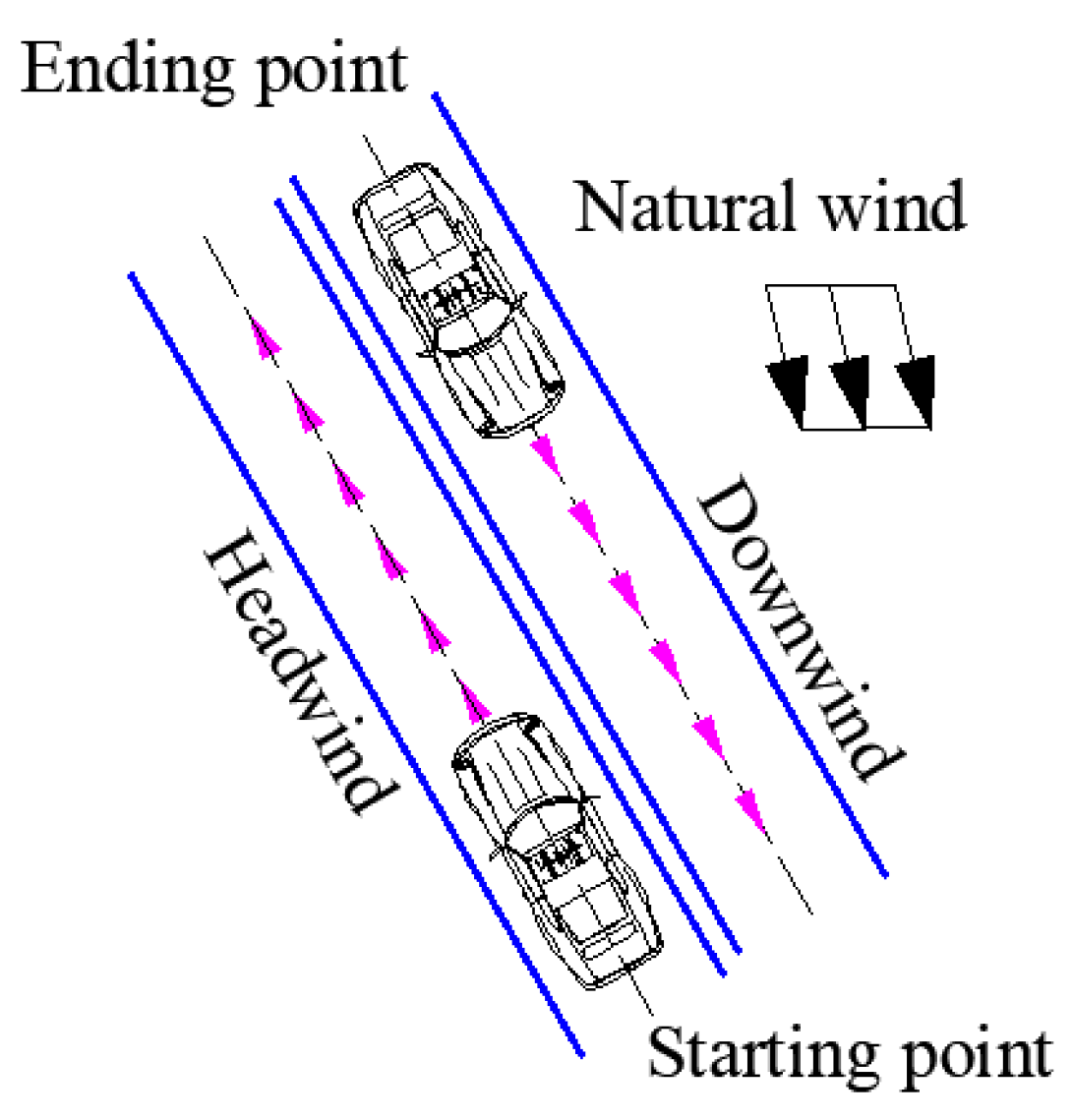
2.3. Test Methods and Strategies
2.4. Measurement of Natural Wind
3. Analysis of Data
3.1. Data of Force
3.2. Data of Pressure
3.3. Data of Natural Wind
4. Results
4.1. Effect of Natural Wind on the Driving Wind of a Moving Vehicle
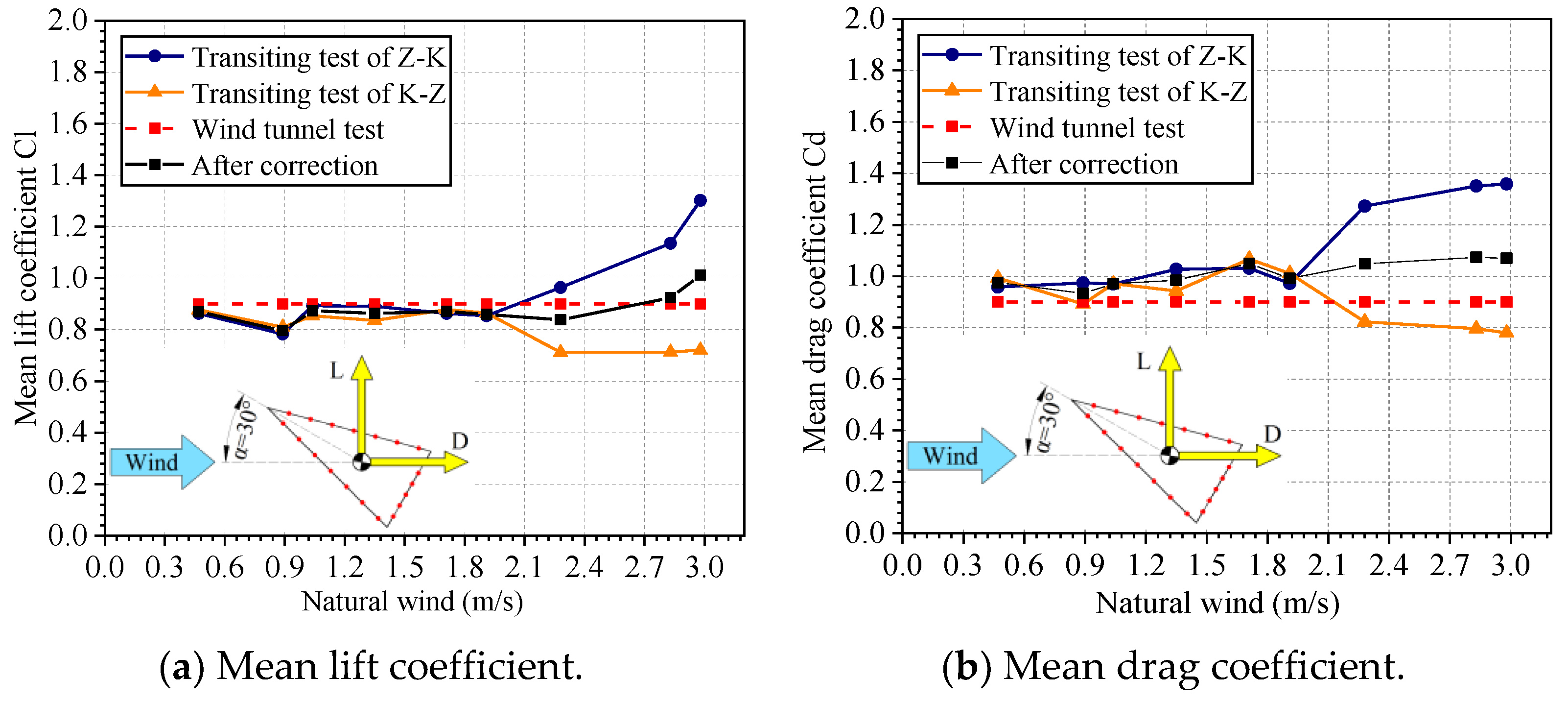
4.2. Effect of Natural Wind on the Mean Aerodynamic Force Coefficients
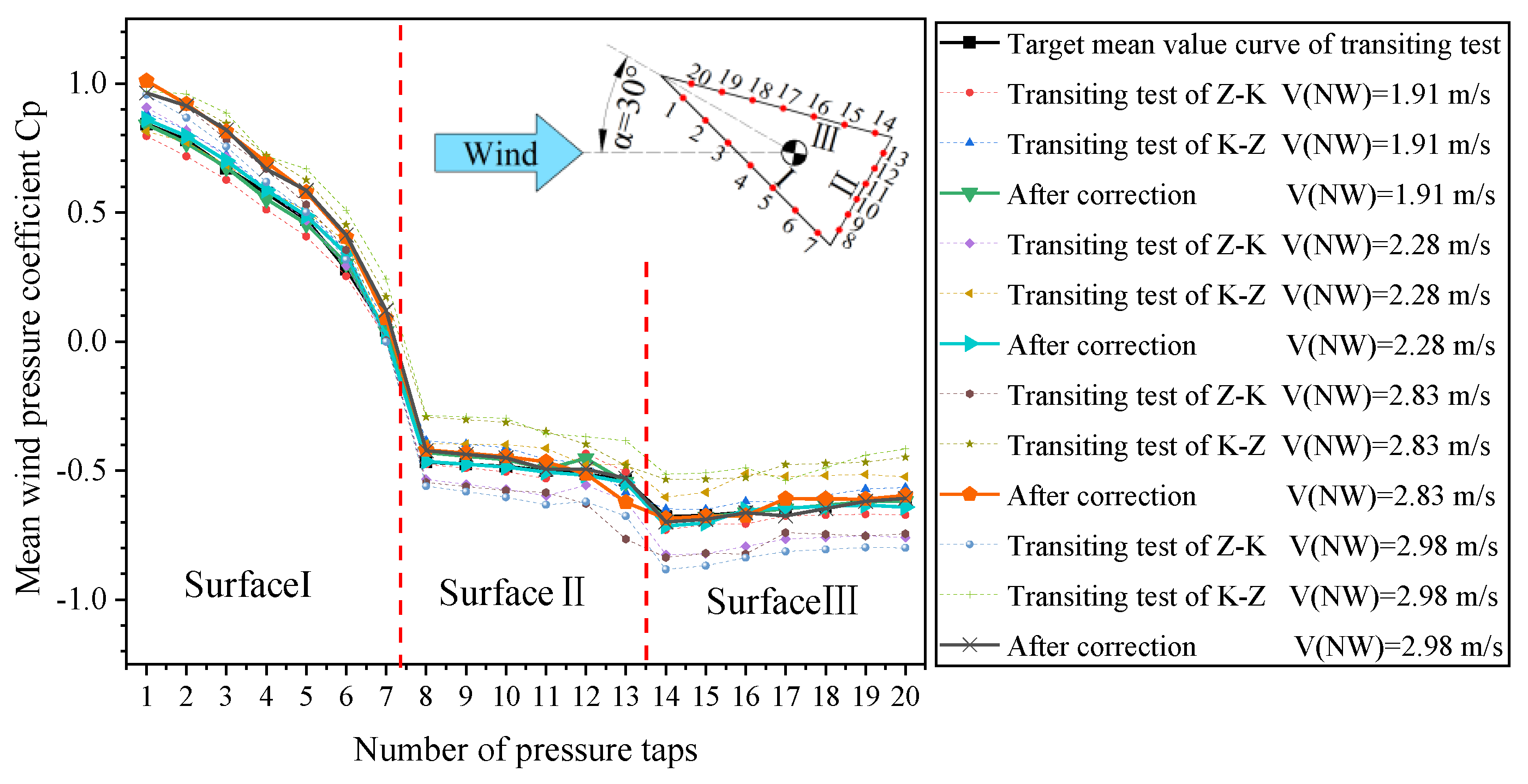
4.3. Effect of Natural Wind on the Mean Aerodynamic Pressure Coefficient
5. Discussion
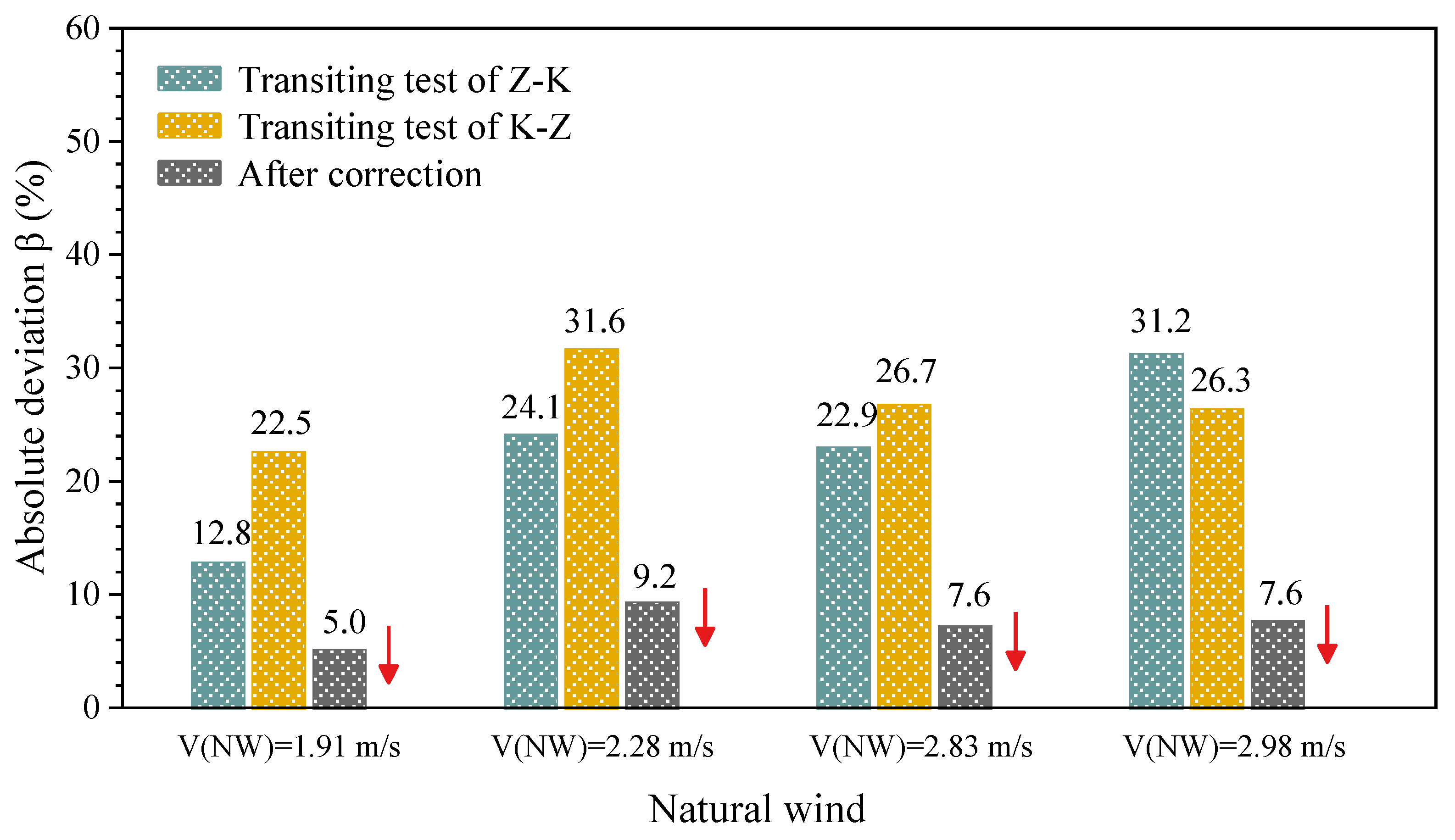
5.1. Correction of Natural Wind on the Mean Aerodynamic Force Coefficients
5.2. Correction of Natural Wind on the Mean Aerodynamic Pressure Coefficient
6. Conclusions
- (1)
- The wind field of the driving vehicle will be significantly affected only when the mean natural wind speed is greater than 1.71 m/s. The influence of natural wind in the range of 0–3.0 m/s on the mean velocity error of the driving wind is less than 10%.
- (2)
- The effect of natural wind on the aerodynamic force coefficients is limited and negligible when the mean natural wind speed is smaller than 1.91 m/s.
- (3)
- When mean natural wind speed is smaller than 1.71 m/s, the weak natural wind has a limited impact on the mean aerodynamic pressure coefficients and the impact can be ignored. Moreover, natural wind has no effect on the curve trend of the aerodynamic pressure coefficients. Natural wind has less influence on the positive wind pressure on the windward side that is lower than the negative pressure on the leeward side.
- (4)
- In summary, to improve the accuracy of the test results, it is recommended that the transiting test of aerodynamic coefficient is carried out under natural wind conditions of less than 1.71 m/s. If the transiting test is carried out under the condition that the average natural wind speed is greater than 1.71 m/s, it is recommended to use the correction method of taking the average value from the two-direction round-trip measurement.
- (5)
- The influence and correction of this research that are based on the segment model, and the natural wind influence analysis and correction that are based on the aeroelastic model transiting test, need further research.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Aliabadi, S.K.; Rasekh, S. Effect of sudden change in free stream velocity on the wind turbine airfoil performance based on boundary element method. Eng. Anal. Bound. Elem. 2019, 101, 360–370. [Google Scholar] [CrossRef]
- Wan, J.W.; Wang, Q.; Liao, H.L.; Li, M.S. Study on aerodynamic coefficients and responses of the integrated catwalk of Halogaland Bridge. Wind Struct. 2017, 25, 215–232. [Google Scholar] [CrossRef]
- Cai, M.; Zhou, L.; Lei, H.; Huang, H. Wind tunnel test investigation on unsteady aerodynamic coefficients of iced 4-bundle conductors. Adv. Civ. Eng. 2019, 1, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Sheng, R.; Perret, L.; Calmet, I.; Demouge, F.; Guilhot, J. Wind tunnel study of wind effects on a high-rise building at a scale of 1:300. J. Wind Eng. Ind. Aerodyn. 2018, 174, 391–403. [Google Scholar] [CrossRef]
- Park, B.H.; Han, Y.O. Steady aerodynamic characteristics of two-dimensional naca0012 airfoil for one revolution angle of attack. Int. J. Aeronaut. Space Sci. 2018, 19, 291–304. [Google Scholar] [CrossRef]
- Wang, Y.; Shen, S.; Li, G.; Huang, D.; Zheng, Z. Investigation on aerodynamic performance of vertical axis wind turbine with different series airfoil shapes. Renew. Energy 2018, 126, 801–818. [Google Scholar] [CrossRef]
- Alonso, G.; Meseguer, J. A parametric study of the galloping stability of two dimensional triangular cross-section bodies. J. Wind Eng. Ind. Aerodyn. 2006, 94, 241–253. [Google Scholar] [CrossRef]
- Alonso, G.; Meseguer, J.; Grande, I.P. Galloping stability of triangular cross sectional bodies: A systematic approach. J. Wind Eng. Ind. Aerodyn. 2007, 95, 928–940. [Google Scholar] [CrossRef]
- Giappino, S.; Melzi, S.; Tomasini, G. High-speed freight trains for intermodal transportation: Wind tunnel study on the aerodynamic coefficients of container wagons. J. Wind Eng. Ind. Aerodyn. 2018, 175, 111–119. [Google Scholar] [CrossRef]
- He, X.; Zou, Y.; Wang, H.; Han, Y.; Shi, K. Aerodynamic characteristics of a trailing rail vehicles on viaduct based on still wind tunnel experiments. J. Wind Eng. Ind. Aerod. 2014, 135, 22–33. [Google Scholar] [CrossRef]
- Noguchi, Y.; Suzuki, M.; Baker, C.; Nakade, K. Numerical and experimental study on the aerodynamic force coefficients of railway vehicles on an embankment in crosswind. J. Wind Eng. Ind. Aerodyn. 2019, 184, 90–105. [Google Scholar] [CrossRef]
- Li, J.; Li, Q.; Hu, S. Monitoring of wind pressures on gable roof of a low-rise building during tropical cyclones and comparisons with wind tunnel test results. Struct. Control. Health Monit. 2019, 38, e2380. [Google Scholar] [CrossRef]
- Ma, C.; Liu, Y.; Yeung, N.; Li, Q. Experimental Study of Across-Wind Aerodynamic Behavior of a Bridge Tower. J. Bridge. Eng. 2019, 24, 04018116. [Google Scholar] [CrossRef]
- Álvarez, A.; Nieto, F.; Nguyen, D.; Owen, J.; Hernandez, S. 3D LES simulations of a static and vertically free-to-oscillate 4:1 rectangular cylinder: Effects of the grid resolution. J. Wind Eng. Ind. Aerodyn. 2019, 192, 31–44. [Google Scholar] [CrossRef]
- Guo, P.; Li, S.; Wang, D. Effects of aerodynamic interference on the iced straddling hangers of suspension bridges by wind tunnel tests. J. Wind Eng. Ind. Aerodyn. 2019, 184, 162–173. [Google Scholar] [CrossRef]
- Guo, P.; Li, S.L.; Wang, C.Q.; Wang, D.W. Influence of catwalk design parameters on the galloping of constructing main cables in long-span suspension bridges. J. Vibroeng. 2017, 19, 4671–4684. [Google Scholar] [CrossRef]
- Meng, F.-Q.; He, B.-J.; Zhu, J.; Zhao, D.-X.; Darko, A.; Zhao, Z.-Q. Sensitivity analysis of wind pressure coefficients on CAARC standard tall buildings in CFD simulations. J. Build. Eng. 2018, 16, 146–158. [Google Scholar] [CrossRef]
- Rašuo, B. On boundary layer control in two-dimensional transonic wind tunnel testing. In Proceedings of the IUTAM Symposium on One Hundred Years of Boundary Layer Research, 2006; Volume 129, pp. 473–482. Available online: https://num.math.uni-goettingen.de/bail/documents/proceedings/rasuo.pdf (accessed on 1 June 2021).
- Argentini, T.; Diana, G.; Rocchi, D.; Somaschini, C. A case-study of double multi-modal bridge flutter: Experimental result and numerical analysis. J. Wind Eng. Ind. Aerod. 2016, 151, 25–36. [Google Scholar] [CrossRef]
- Wang, H.; Mao, J.-X.; Spencer, B.F. A monitoring-based approach for evaluating dynamic responses of riding vehicle on long-span bridge under strong winds. Eng. Struct. 2019, 189, 35–47. [Google Scholar] [CrossRef]
- Zhang, J.; Li, Q. Field measurements of wind pressures on a 600 m high skyscraper during a landfall typhoon and comparison with wind tunnel test. J. Wind Eng. Ind. Aerodyn. 2018, 175, 391–407. [Google Scholar] [CrossRef]
- Rašuo, B. 2012 Scaling between Wind Tunnels–Results Accuracy in Two-Dimensional Testing. Trans. Jpn. Soc. Aeronaut. Space Sci. 2012, 55, 109–115. [Google Scholar] [CrossRef] [Green Version]
- Ocokoljić, G.; Damljanović, D.; Vuković, Đ.; Rašuo, B. Contemporary frame of measurement and assessment of wind-tunnel flow quality in a low-speed facility. FME Trans. 2018, 46, 429–442. [Google Scholar] [CrossRef]
- Zocca, M.; Gori, G.; Guardone, A. Blockage and Three-Dimensional Effects in Wind-Tunnel Testing of Ice Accretion over Wings. J. Aircr. 2017, 54, 759–767. [Google Scholar] [CrossRef]
- Rašuo, B. The influence of Reynolds and Mach numbers on two-dimensional wind-tunnel testing: An experience. Aeronaut. J. 2011, 115, 249–254. [Google Scholar] [CrossRef]
- Rašuo, B. On Status of Wind Tunnel Wall Correction. In Proceedings of the 25th ICAS Congress, Hamburg, Germany, September 2006; Available online: https://www.icas.org/ICAS_ARCHIVE/ICAS2006/PAPERS/416.PDF (accessed on 1 June 2021).
- Ocokoljić, G.; Rašuo, B.; Kozić, M. Supporting system interference on aerodynamic characteristics of an aircraft model in a low-speed wind tunnel. Aerosp. Sci. Technol. 2017, 64, 133–146. [Google Scholar] [CrossRef]
- Linić, S.L.; Ocokoljić, G.J.; Ristić, S.S.; Lučanin, V.J.; Kozić, M.S.; Rašuo, B.P.; Jegdić, B.V. Boundary-layer transition detection by thermography and numerical method around bionic train model in wind tunnel test. Therm. Sci. 2018, 22, 1137–1148. [Google Scholar] [CrossRef] [Green Version]
- Meier, G.E.A.; Sreenivasan, K.R.; Heinemann, H.-J. (Eds.) IUTAM Symposium on One Hundred Years of Boundary Layer Research Book Series: Solid Mechanics and Its Applications 129; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar] [CrossRef]
- Li, S.; Liu, L.; Wu, H.; Jiang, N.; Zheng, S.; Guo, P. New Test Method of Wind Pressure Coefficient Based on CAARC Standard Model Determined Using Vehicle Driving Wind. Exp. Tech. 2019, 43, 707–717. [Google Scholar] [CrossRef]
- Li, S.; Liang, J.; Zheng, S.; Jiang, N.; Liu, L.; Guo, P. A Novel Test Method for Aerodynamic Coefficient Measurements of Structures Using Wind Generated by a Moving Vehicle. Exp. Tech. 2019, 43, 677–693. [Google Scholar] [CrossRef]
- Li, S.; Wan, R.; Wang, D.; Guo, P. Effect of end plates on transiting test for measuring the aerodynamic coefficient of structures using wind generated by a moving vehicle. J. Wind Eng. Ind. Aerodyn. 2019, 190, 273–286. [Google Scholar] [CrossRef]
- Guo, P.; Wang, D.W.; Li, S.L.; Liu, L.L.; Wang, X.D. Transiting test method for galloping of iced conductor using wind generated by a moving vehicle. Wind Struct. 2019, 28, 155–170. [Google Scholar] [CrossRef]
- Li, S.; Chu, Y.; Liu, X.; Liu, L.; Guo, P.; Wang, X.; Li, P. Investigation on passive simulation method and factors influencing the type-C-terrain wind profile of a structural wind-resistant moving-vehicle tester. J. Build. Eng. 2020, 32, 101482. [Google Scholar] [CrossRef]
- Li, B.; Yang, Q.; Yang, J. Wind characteristics near ground in south-eastern coast area of China based on field measurement. Geomat. Nat. Haz. Risk 2016, 7, 57–69. [Google Scholar] [CrossRef] [Green Version]
- Nappo, C.J.; Hiscox, A.L.; Miller, D.R. A Note on Turbulence Stationarity and Wind Persistence Within the Stable Planetary Boundary Layer. Bound. Lay. Meteorol. 2010, 136, 165–174. [Google Scholar] [CrossRef]
- Yu, M.; Liu, J.; Liu, D.; Chen, H.; Zhang, J. Investigation of aerodynamic effects on the high-speed train exposed to longitudinal and lateral wind velocities. J. Fluid Struct. 2016, 61, 347–361. [Google Scholar] [CrossRef]
- Altinisik, A. Aerodynamic coast-down analysis of a passenger car for various configurations. Int. J. Automot. Technol. 2017, 18, 245–254. [Google Scholar] [CrossRef]
- Páscoa, J.C.; Brójo, F.P.; Santos, F.C.; Fael, P.O. An innovative experimental on-road testing method and its demonstration on a prototype vehicle. J. Mech. Sci. Technol. 2012, 26, 1663–1670. [Google Scholar] [CrossRef]
- McAuliffe, B.R.; Chuang, D. Track-Based Aerodynamic Testing of a Heavy-Duty Vehicle: Coast-Down Measurements SAE. Int. J. Commer. Veh. 2016, 9, 381–396. [Google Scholar] [CrossRef]
- Ji, L.; Tan, H.; Kato, S.; Bu, Z.; Takahashi, T. Wind tunnel investigation on influence of fluctuating wind direction on cross natural ventilation. Build. Environ. 2011, 46, 2490–2499. [Google Scholar] [CrossRef]
- Kozmar, H.; Butler, K.; Kareem, A. Downslope Gusty Wind Loading of Vehicles on Bridges. J. Bridge. Eng. 2015, 20, 04015008. [Google Scholar] [CrossRef]
- Baker, C.J. The simulation of unsteady aerodynamic cross wind forces on trains. J. Wind Eng. Ind. Aerodyn. 2010, 98, 88–99. [Google Scholar] [CrossRef]
- Veldhuizen, R.; Van Raemdonck, G.; Van Der Krieke, J. Fuel economy improvement by means of two European tractor semi-trailer combinations in a platooning formation. J. Wind Eng. Ind. Aerodyn. 2019, 188, 217–234. [Google Scholar] [CrossRef]
- McAuliffe, B.R.; Belluz, L.; Belzile, M. Measurement of the On-Road Turbulence Environment Experienced by Heavy Duty Vehicles SAE. Int. J. Commer. Veh. 2014, 7, 685–702. [Google Scholar] [CrossRef]
- Zhang, X.L.; Liu, P.F. Virtual Test System for Coast-down Resistance of Motor Vehicle with Compensation of Wind Speed and Direction. Trans. Chin. Soc. Agric. Mach. 2017, 48, 390–397. [Google Scholar]
- Yan, L.; Zhu, L.D.; Flay, R. Comparison of force-balance and pressure measurements on deck strips on a stationary bridge model. J. Wind Eng. Ind. Aerod. 2017, 164, 96–107. [Google Scholar] [CrossRef]
- Ganapathi, C.S.; Harikrishna, P.; Rajan, S.S. Effects of upstream terrain characteristics on aerodynamic coefficients of structures. Arch. Civ. Mech. Eng. 2017, 17, 776–785. [Google Scholar] [CrossRef]
- Fiedler, M.; Berg, W.; Ammon, C.; Loebsin, C.; Sanftleben, P.; Samer, M.; Von Bobrutzki, K.; Kiwan, A.; Saha, C.K. Air velocity measurements using ultrasonic anemometers in the animal zone of a naturally ventilated dairy barn. Biosyst. Eng. 2013, 116, 276–285. [Google Scholar] [CrossRef]
- Wang, H.; Wu, T.; Tao, T.; Li, A.; Kareem, A. Measurements and analysis of non-stationary wind characteristics at Sutong Bridge in Typhoon Damrey. J. Wind Eng. Ind. Aerod. 2016, 151, 100–106. [Google Scholar] [CrossRef] [Green Version]
- GB50009-2012 Load Code for the Design of Building Structures Beijing; China Architecture and Building Press. Available online: https://www.chinesestandard.net/PDF.aspx/GB50009-2012 (accessed on 1 June 2021).
- Fu, J.; Zheng, Q.; Huang, Y.; Wu, J.; Pi, Y.; Liu, Q. Design optimization on high-rise buildings considering occupant comfort reliability and joint distribution of wind speed and direction. Eng. Struct. 2018, 156, 460–471. [Google Scholar] [CrossRef]
- Li, Q.; Zhi, L.; Hu, F. Boundary layer wind structure from observations on a 325m tower. J. Wind Eng. Ind. Aerod. 2010, 98, 818–832. [Google Scholar] [CrossRef]
- Liu, Z.; Zheng, C.; Wu, Y.; Song, Y. Investigation on characteristics of thousand-meter height wind profiles at non-tropical cyclone prone areas based on field measurement. Build. Environ. 2018, 130, 62–73. [Google Scholar] [CrossRef]
- Duncan, B.; D’Alessio, L.; Gargoloff, J.; Alajbegovic, A. Vehicle aerodynamics impact of on-road turbulence. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2017, 231, 1148–1159. [Google Scholar] [CrossRef] [Green Version]
- Chevula, S.; Sanz-Andres, A.; Franchini, S. Franchini, Estimation of the correction term of pitot tube measurements in unsteady (gusty) flows. Flow. Meas. Instrum. 2015, 46, 179–188. [Google Scholar] [CrossRef]
- Wordley, S.; Saunders, J.W. On-road Turbulence. SAE Int. J. Passeng. Cars Mech. Syst. 2008, 1, 341–360. [Google Scholar] [CrossRef]
- Ricciardelli, F.; De Grenet, E.T.; Hangan, H. Pressure distribution, aerodynamic forces and dynamic response of box bridge sections. J. Wind Eng. Ind. Aerod. 2002, 90, 1135–1150. [Google Scholar] [CrossRef]

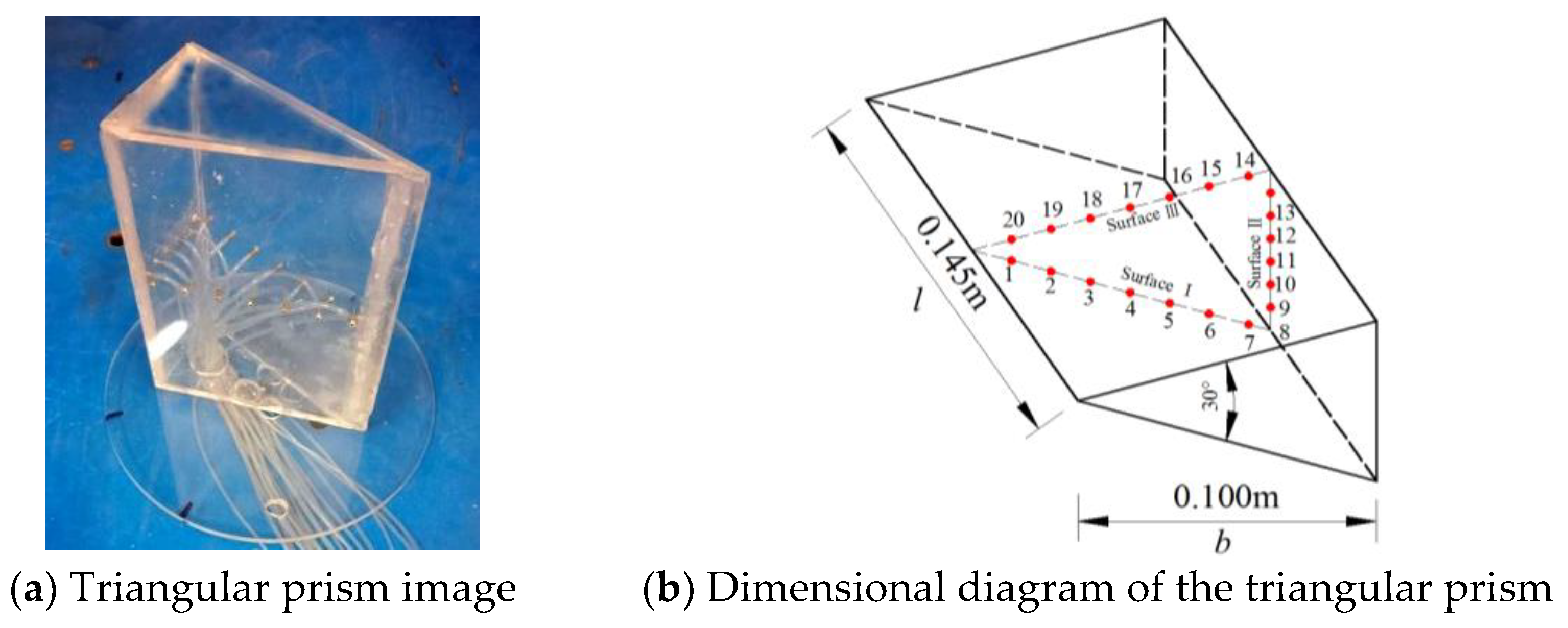
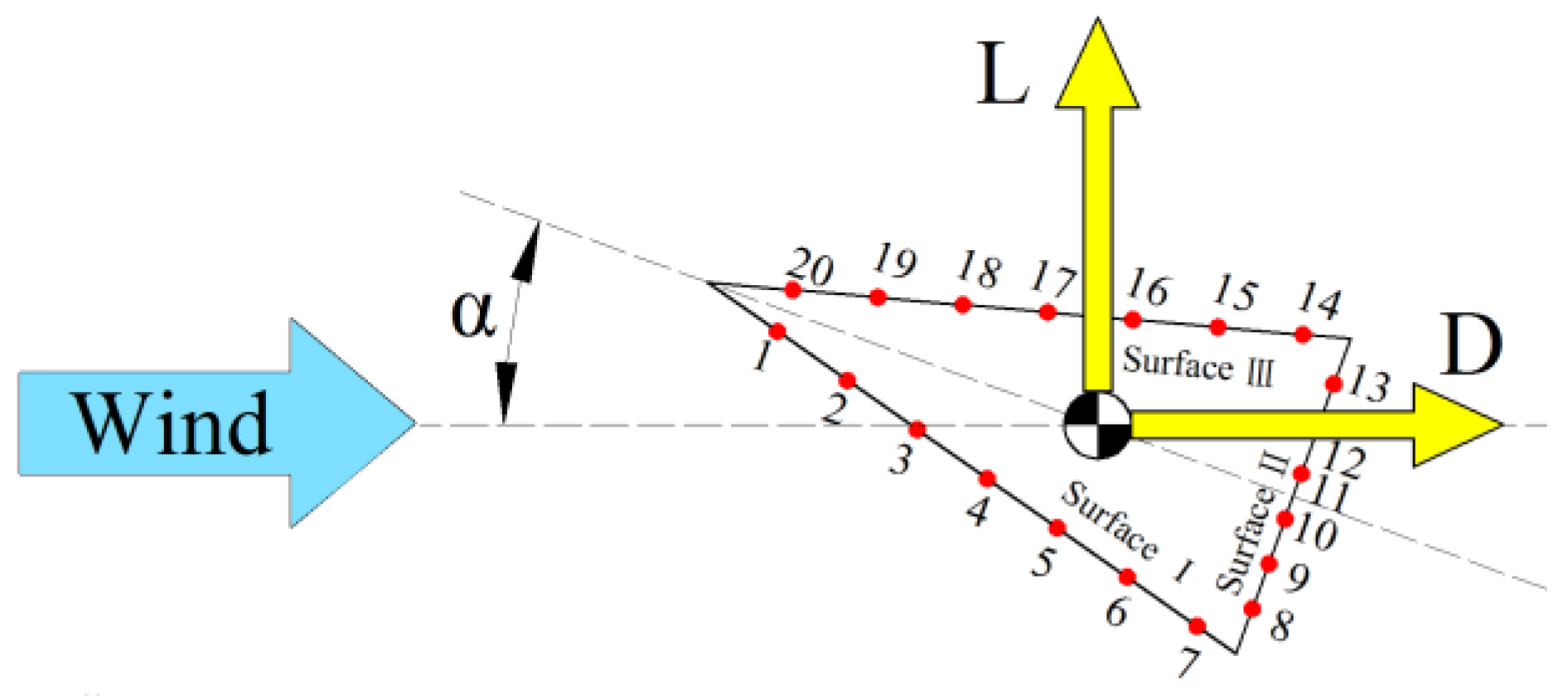


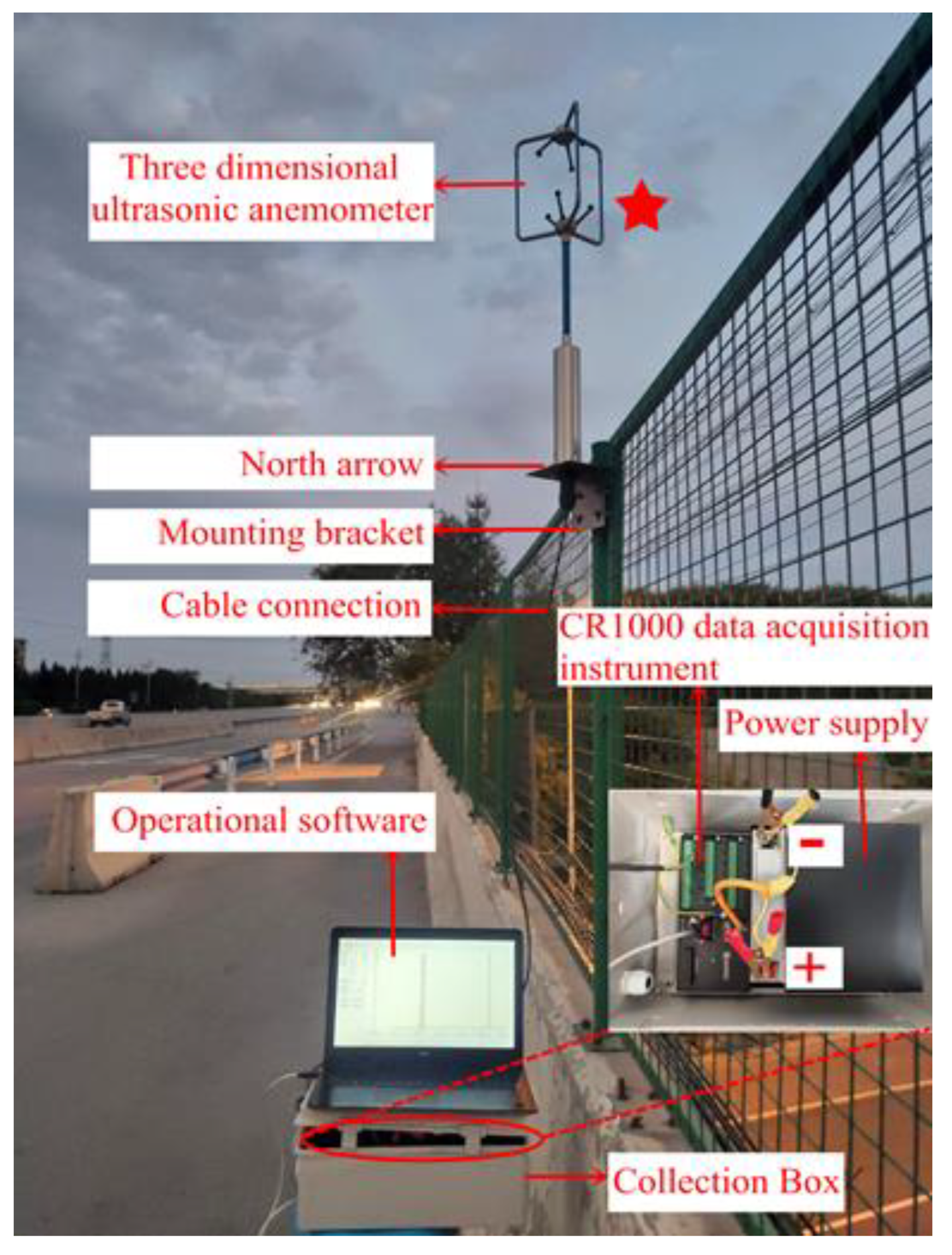
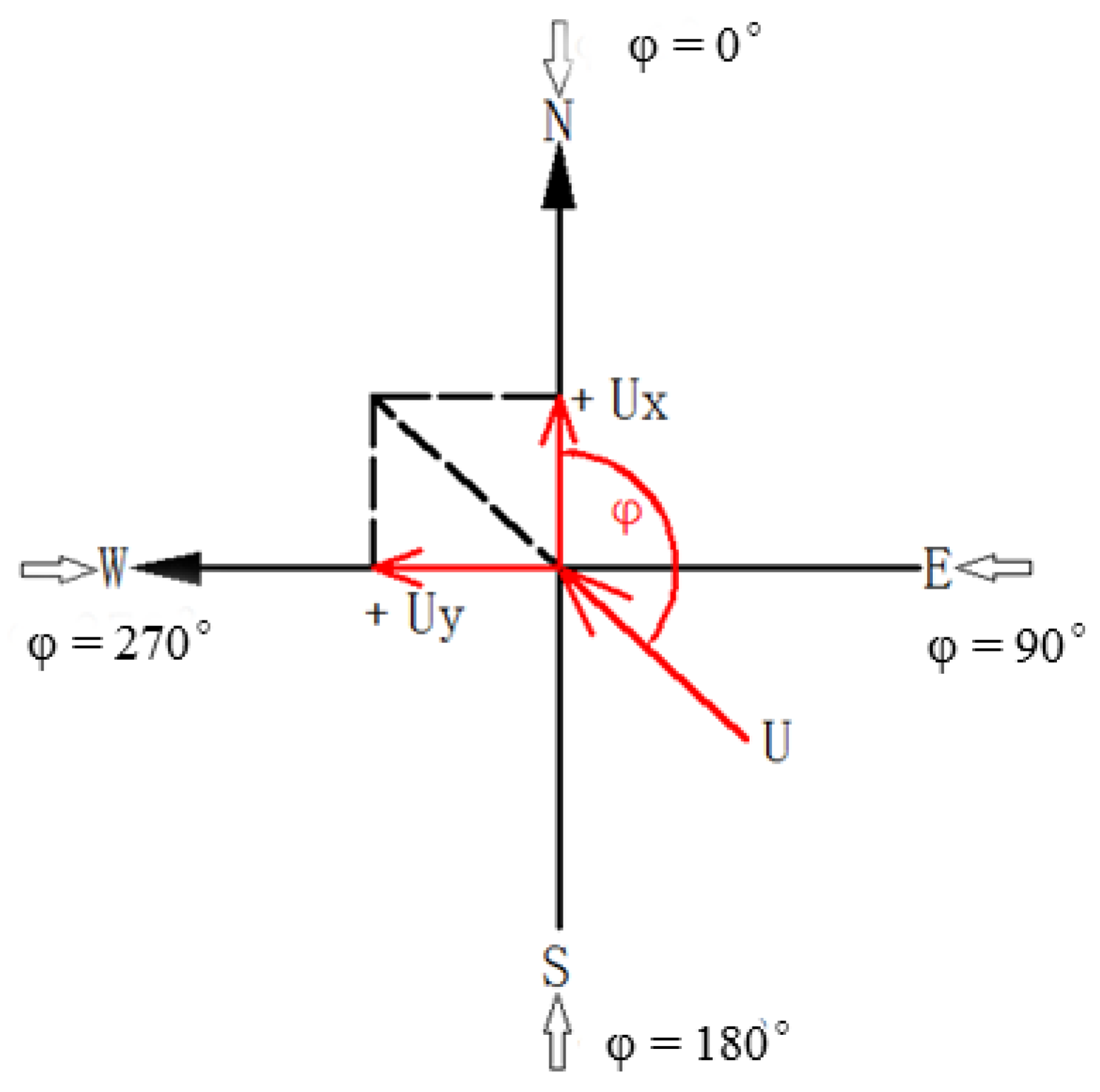

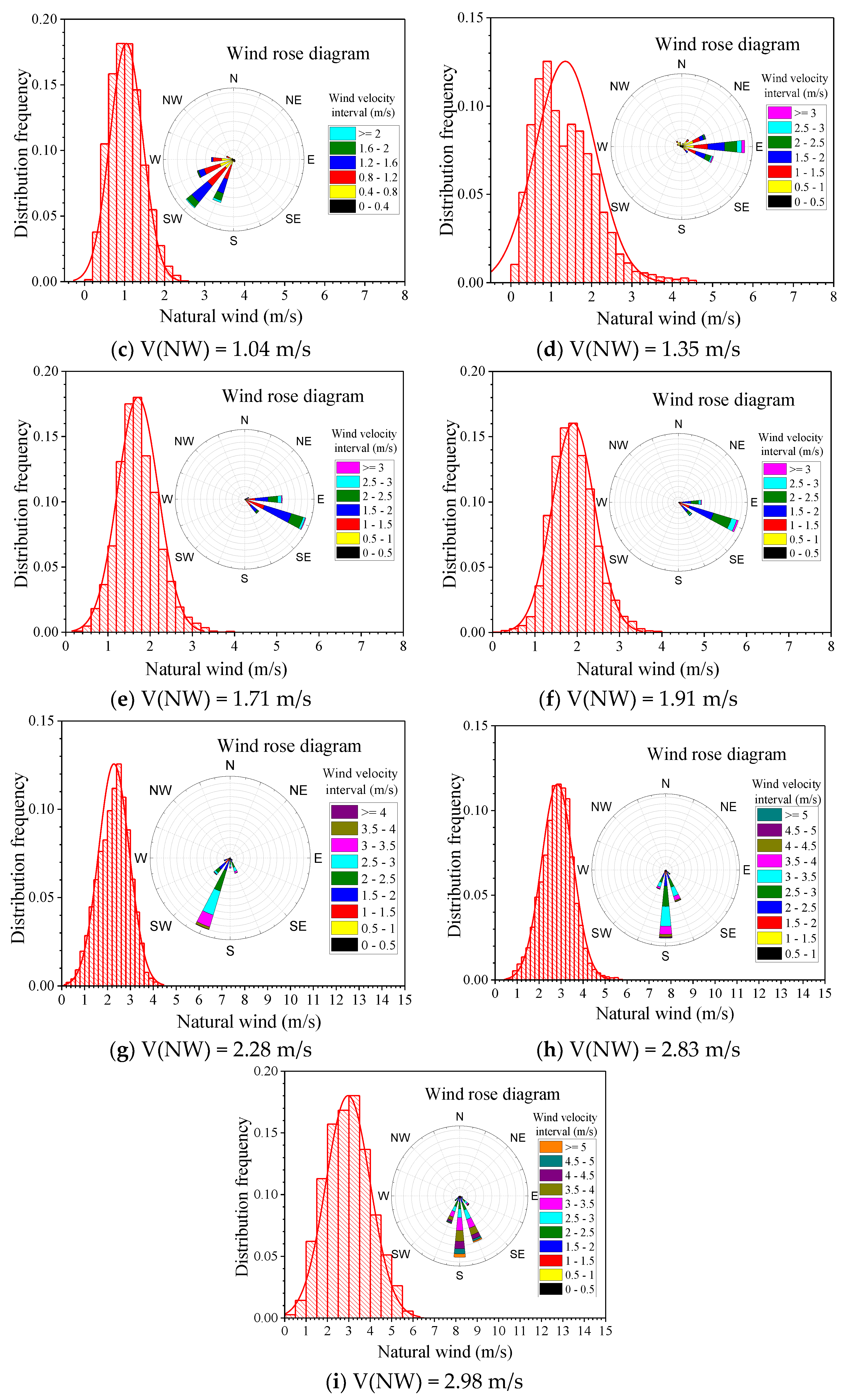


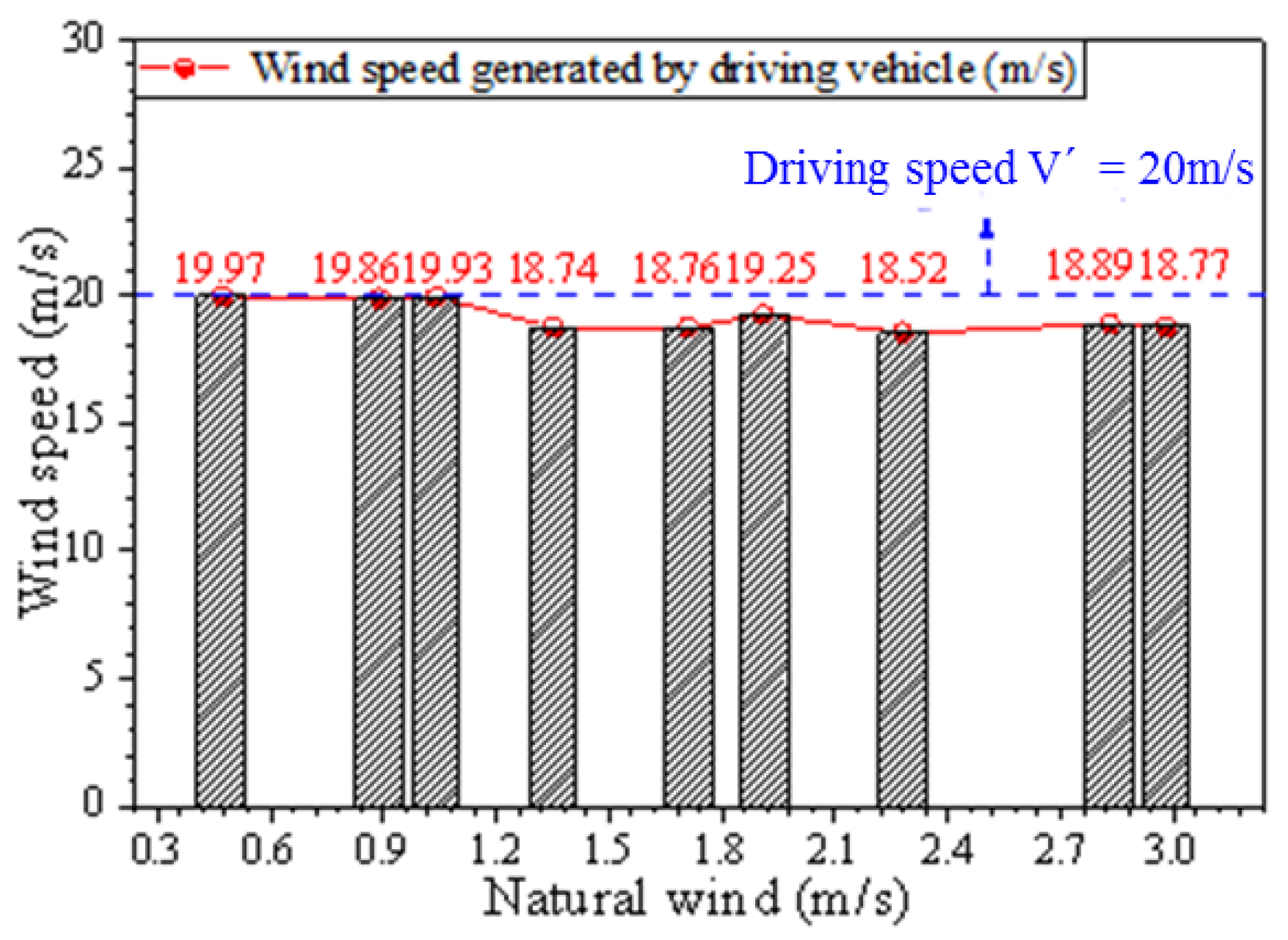


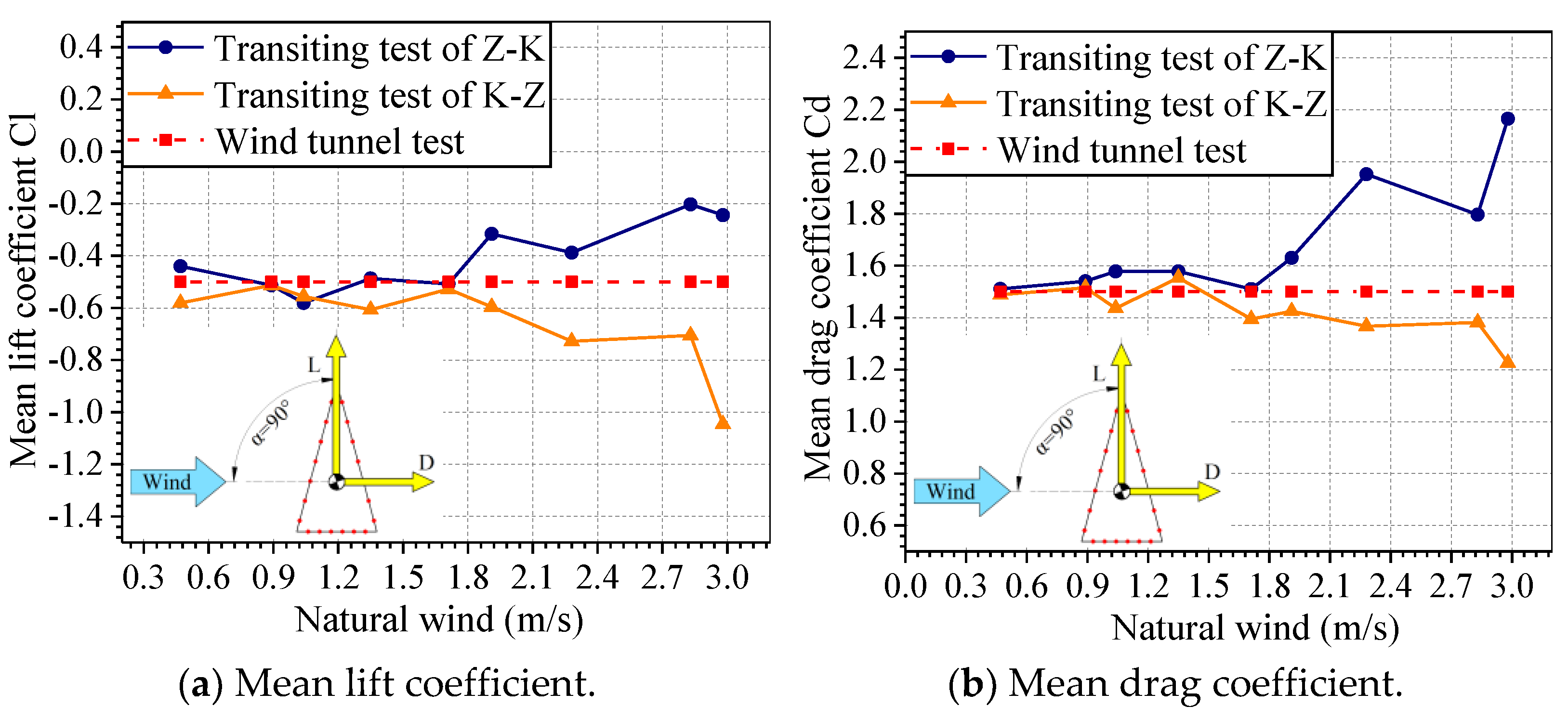
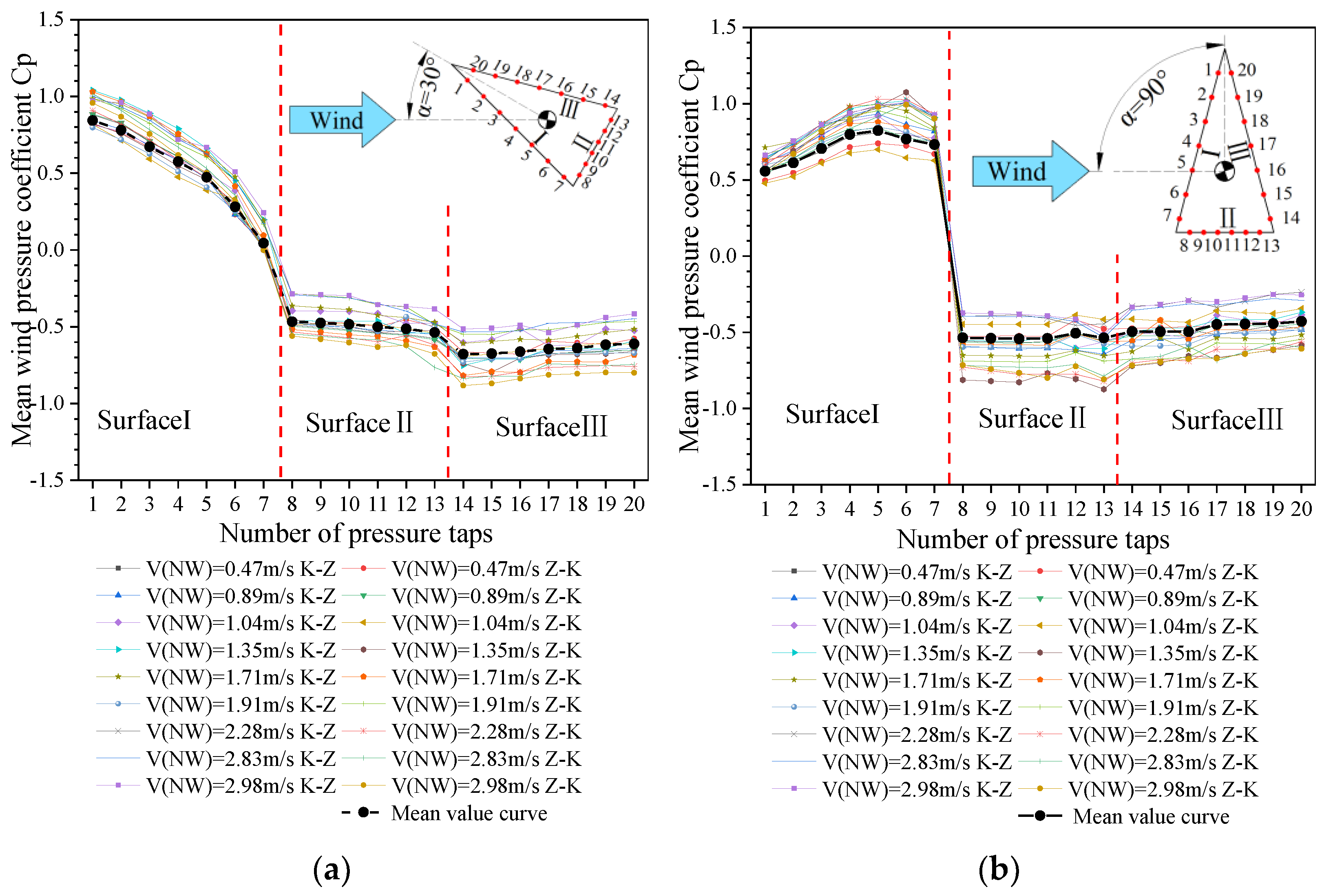




| Driving Speed | Model Placement | Wind Attack Angle α | Test Route | Range of Natural Wind Speed |
|---|---|---|---|---|
| 72 km/h (20 m/s) | Horizontal | 30° | K–Z | 0–3.0 m/s |
| Z–K | ||||
| 90° | K–Z | |||
| Z–K |
| Case | Test Date | ||
|---|---|---|---|
| 1 | 20 May 2019 | 0.47 m/s | 0.33 m/s |
| 2 | 26 Sep. 2019 | 0.89 m/s | 0.62 m/s |
| 3 | 21 May 2019 | 1.04 m/s | 0.73 m/s |
| 4 | 28 Aug. 2019 | 1.35 m/s | 0.95 m/s |
| 5 | 29 Aug. 2019 | 1.71 m/s | 1.20 m/s |
| 6 | 29 Aug. 2019 | 1.91 m/s | 1.34 m/s |
| 7 | 16 Apr. 2019 | 2.28 m/s | 1.60 m/s |
| 8 | 14 May 2019 | 2.83 m/s | 1.98 m/s |
| 9 | 14 May 2019 | 2.98 m/s | 2.08 m/s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, G.; Liu, X.; Liu, L.; Li, S. Effect of Natural Wind on the Transiting Test for Measuring the Aerodynamic Coefficients of Structures. Symmetry 2021, 13, 1493. https://doi.org/10.3390/sym13081493
Zhu G, Liu X, Liu L, Li S. Effect of Natural Wind on the Transiting Test for Measuring the Aerodynamic Coefficients of Structures. Symmetry. 2021; 13(8):1493. https://doi.org/10.3390/sym13081493
Chicago/Turabian StyleZhu, Guangxia, Xin Liu, Lulu Liu, and Shengli Li. 2021. "Effect of Natural Wind on the Transiting Test for Measuring the Aerodynamic Coefficients of Structures" Symmetry 13, no. 8: 1493. https://doi.org/10.3390/sym13081493
APA StyleZhu, G., Liu, X., Liu, L., & Li, S. (2021). Effect of Natural Wind on the Transiting Test for Measuring the Aerodynamic Coefficients of Structures. Symmetry, 13(8), 1493. https://doi.org/10.3390/sym13081493






