The Energy of the Ground State of the Two-Dimensional Hamiltonian of a Parabolic Quantum Well in the Presence of an Attractive Gaussian Impurity
Abstract
:1. Introduction
2. Preliminaries
- (i)
- (ii)
3. Calculation of the Ground State Energy
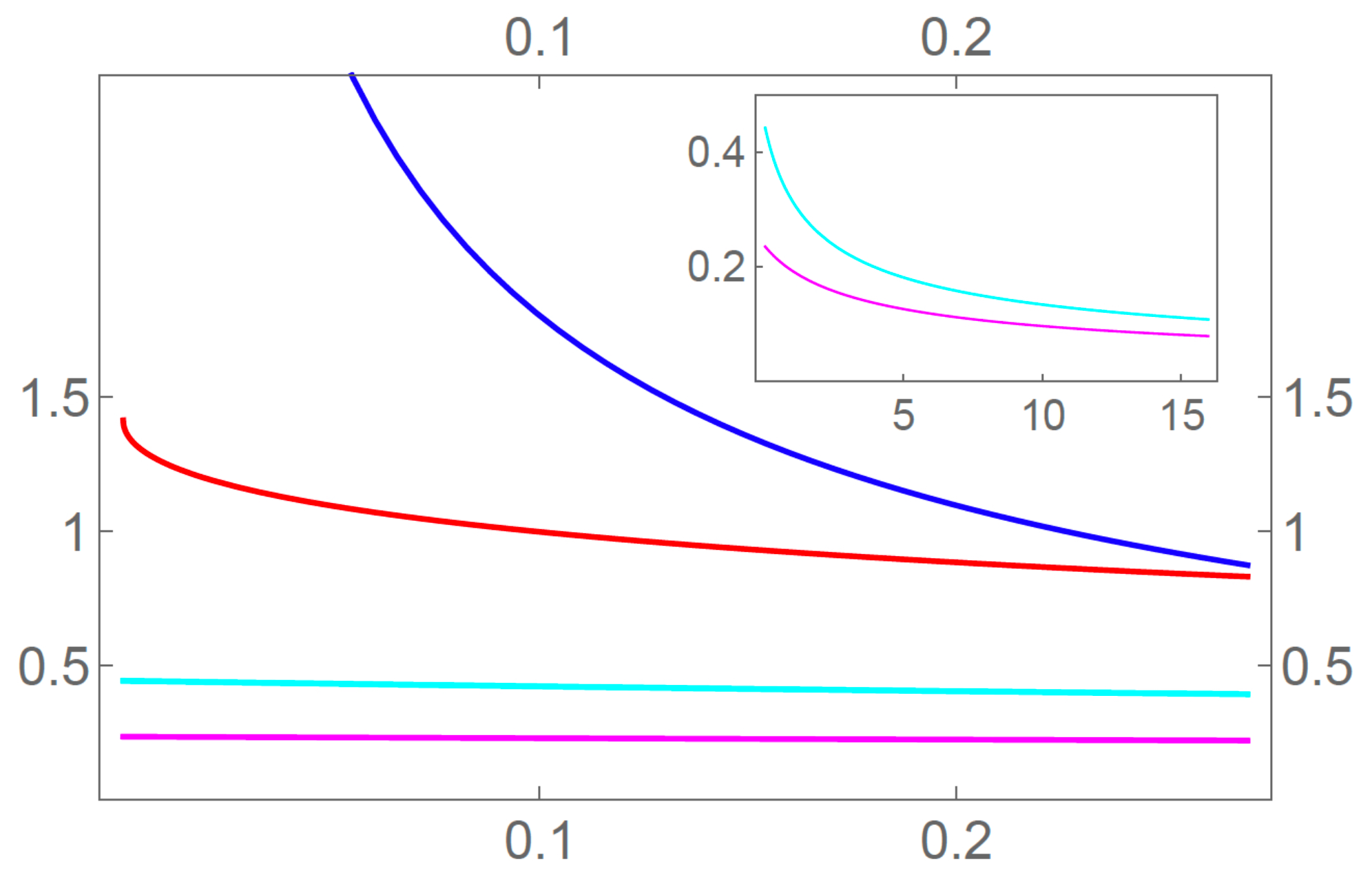
4. Final Remarks
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Some Mathematical Results
Appendix A.1. First Theorem
Appendix A.2. Second Theorem
Appendix A.3. Third Theorem
References
- Albeverio, S.; Gesztesy, F.; Høegh-Krohn, R.; Holden, H. Solvable Models in Quantum Mechanics, 2nd ed.; AMS Chelsea Series; AMS Chelsea: Providence, RI, USA, 2004. [Google Scholar]
- Albeverio, S.; Kurasov, P. Singular Perturbations of Differential Operators; Cambridge UP: Cambridge, UK, 1999. [Google Scholar]
- Seba, P. Some remarks on the δ-interaction in one dimension. Czechoslov. J. Phys. 1986, 36, 667–673. [Google Scholar] [CrossRef]
- Bräunlich, G.; Hainzl, C.; Seiringer, R. On contact interactions as limits of short-range potentials. Met. Funct. Anal. Top. 2013, 19, 364–375. [Google Scholar]
- Bohm, A. Quantum Mechanics. Foundations and Applications; Springer: New York, NY, USA, 1994. [Google Scholar]
- Kurasov, P. Distribution theory for discontinuous test functions and differential operators with generalized coefficients. J. Math. Anal. Appl. 1996, 201, 297–323. [Google Scholar] [CrossRef] [Green Version]
- Albeverio, S.; Kurasov, P. Pseudo-differential operators with point interactions. Lett. Math. Phys. 1997, 41, 79–92. [Google Scholar] [CrossRef]
- Albeverio, S.; Fassari, S.; Rinaldi, F. The discrete spectrum of the spinless onedimensional Salpeter Hamiltonian perturbed by δ-interactions. J. Phys. A Math. Theor. 2015, 48, 185301. [Google Scholar] [CrossRef]
- Erman, F.; Gadella, M.; Uncu, H. One-dimensional semirelativistic Hamiltonian with multiple Dirac delta potentials. Phys. Rev. D 2017, 95, 045004. [Google Scholar] [CrossRef] [Green Version]
- Muñoz-Castañeda, J.M.; Nieto, L.M.; Romaniega, C. Hyperspherical δ-δ′ potentials. Ann. Phys. 2019, 400, 246–261. [Google Scholar] [CrossRef] [Green Version]
- Antonie, J.P.; Gesztesy, F.; Shabani, J. Exactly solvable models of sphere interaction in quantum mechanics. J. Phys. A Math. Gen. 1987, 20, 3687–3712. [Google Scholar] [CrossRef]
- Nyeo, S.-L. Regularization methods for delta-function potential in two-dimensional quantum mechanics. Am. J. Phys. 2000, 68, 571–575. [Google Scholar] [CrossRef] [Green Version]
- Jackiw, R. Diverse Topics in Mathematical Physics; Word Scientific: Singapore, 1995; pp. 41–48. [Google Scholar]
- Goszdinsky, P.; Tarrach, R. Learning Quantum Field Theory from elementary quantum mechanics. Am. J. Phys. 1991, 59, 70–74. [Google Scholar] [CrossRef]
- Erman, F.; Uncu, H. Green’s function formulation of multiple nonlinear Dirac delta-function potential in one dimension. Phys. Lett. A 2020, 384, 126227. [Google Scholar] [CrossRef] [Green Version]
- Fassari, S.; Popov, I.; Rinaldi, F. On the behaviour of the two-dimensional Hamiltonian −Δ + λ[δ( + ) + δ( − )] as the distance between the two centers vanishes. Phys. Scr. 2020, 95, 075209. [Google Scholar] [CrossRef]
- Klaus, M. A remark about weakly coupled one-dimensional Schrödinger operators. Helv. Phys. Acta 1979, 52, 223–229. [Google Scholar]
- Klaus, M. Some applications of the Birman-Schwinger principle. Helv. Phys. Acta 1982, 55, 49–68. [Google Scholar]
- Fassari, S. An estimate regarding one-dimensional point interactions. Helv. Phys. Acta 1995, 68, 121–125. [Google Scholar]
- Holden, H. On coupling constant thresholds in two dimensions. J. Oper. Theory 1985, 14, 263–276. [Google Scholar]
- Fassari, S.; Klaus, M. Coupling constant thresholds of perturbed periodic Hamiltonians. J. Math. Phys. 1998, 39, 4369–4416. [Google Scholar] [CrossRef] [Green Version]
- Fassari, S.; Gadella, M.; Glasser, M.L.; Nieto, L.M. Rinaldi, F. Level crossings of eigenvalues of the Schrödinger Hamiltonian of the isotropic harmonic oscillator perturbed by a central point interaction in different dimensions. Nanosyst. Phys. Chem. Math. 2018, 9, 179–186. [Google Scholar] [CrossRef]
- Fassari, S.; Inglese, G. Spectroscopy of a three-dimensional isotropic harmonic oscillator with a δ-type perturbation. Helv. Phys. Acta 1996, 69, 130–140. [Google Scholar]
- Albeverio, S.; Fassari, S.; Rinaldi, F. Spectral properties of a symmetric three-dimensional quantum dot with a pair of identical attractive δ-impurities symmetrically situated around the origin. Nanosyst. Phys. Chem. Math. 2016, 7, 268–289. [Google Scholar] [CrossRef] [Green Version]
- Albeverio, S.; Fassari, S.; Rinaldi, F. Spectral properties of a symmetric three-dimensional quantum dot with a pair of identical attractive δ-impurities symmetrically situated around the origin II. Nanosyst. Phys. Chem. Math. 2016, 7, 803–815. [Google Scholar] [CrossRef] [Green Version]
- Albeverio, S.; Fassari, S.; Rinaldi, F. A remarkable spectral feature of the Schrödinger Hamiltonian of the harmonic oscillator perturbed by an attractive δ-interaction centred at the origin: Double degeneracy and level crossings. J. Phys. A Math. Theor. 2013, 46, 385305. [Google Scholar] [CrossRef]
- Fassari, S.; Gadella, M.; Glasser, M.L.; Nieto, L.M. Spectroscopy of a one-dimensional V-shaped quantum well with a point impurity. Ann. Phys. 2018, 389, 48–62. [Google Scholar] [CrossRef] [Green Version]
- Fassari, S.; Gadella, M.; Nieto, L.M.; Rinaldi, F. Spectral properties of the 2D Schrödinger Hamiltonian with various solvable confinements in the presence of a central point perturbation. Phys. Scr. 2019, 94, 055202. [Google Scholar] [CrossRef] [Green Version]
- Sasaki, R.; Znojil, M. One-dimensional Schrödinger equation with non-analytic potential V(x) = −g2exp(−|x|) and its exact Bessel-function solvability. J. Phys. A Math. Theor. 2016, 49, 445303. [Google Scholar] [CrossRef] [Green Version]
- Fassari, S.; Gadella, M.; Nieto, L.M.; Rinaldi, F. On the spectrum of the 1D Schrödinger Hamiltonian perturbed by an attractive Gaussian potential. Acta Polytech. 2017, 57, 385–390. [Google Scholar] [CrossRef] [Green Version]
- Muchatibaya, G.; Fassari, S.; Rinaldi, F.; Mushanyu, J. A note on the discrete spectrum of Gaussian wells (I): The ground state energy in one dimension. Adv. Math. Phys. 2016, 2016, 2125769. [Google Scholar] [CrossRef] [Green Version]
- Fernández, F.M. Quantum Gaussian wells and barriers. Am. J. Phys. 2011, 79, 752–754. [Google Scholar] [CrossRef]
- Nandi, S. The quantum Gaussian well. Am. J. Phys. 2010, 78, 1341–1345. [Google Scholar] [CrossRef] [Green Version]
- Fassari, S.; Nieto, L.M.; Rinaldi, F. The two lowest eigenvalues of the harmonic oscillator in the presence of a Gaussian perturbation. Eur. Phys. J. Plus 2020, 135, 728. [Google Scholar] [CrossRef]
- Fassari, S.; Rinaldi, F. Exact calculation of the trace of the Birman-Schwinger operator of the one-dimensional harmonic oscillator perturbed by an attractive Gaussian potential. Nanosyst. Phys. Chem. Math. 2019, 10, 608–615. [Google Scholar] [CrossRef] [Green Version]
- Harrison, P. Quantum Wells, Wires, Dots; Wiley: Chichester, UK, 2009. [Google Scholar]
- Albeverio, S.; Fassari, S.; Gadella, M.; Nieto, L.M.; Rinaldi, F. The Birman-Schwinger operator for a parabolic quantum well in a zero-thickness layer in the presence of a two-dimensional attractive Gaussian impurity. Front. Phys. 2019, 7, 102. [Google Scholar] [CrossRef] [Green Version]
- Reed, M.; Simon, B. Fourier Analysis, Self-Adjointness, Methods in Modern Mathematical Physics; Academic Press: New York, NY, USA, 1975. [Google Scholar]
- Duclos, P.; Štovicek, P.; Tušek, M. On the two-dimensional Coulomb-like potential with a central point interaction. J. Phys. A Math. Theor. 2010, 43, 474020. [Google Scholar] [CrossRef] [Green Version]
- Reed, M.; Simon, B. Functional Analysis, Methods in Modern Mathematical Physics; Academic Press: New York, NY, USA, 1972. [Google Scholar]
- Simon, B. Trace Ideals and Their Applications; Cambridge University Press: Cambridge, UK, 1979. [Google Scholar]
- Arfken, G.B. Mathematical Methods for Physicists, 3rd ed.; Academic Press: New York, NY, USA, 1985. [Google Scholar]
- Correggi, M.; Dell’Antonio, G.; Finco, D. Spectral analysis of a two-body problem with zero-range perturbation. J. Funct. Anal. 2008, 255, 502–531. [Google Scholar] [CrossRef] [Green Version]
- Wang, W.-M. Pure Point Spectrum of the Floquet Hamiltonian for the Quantum Harmonic Oscillator Under Time Quasi-Periodic Perturbations. Commun. Math. Phys. 2008, 277, 459–496. [Google Scholar] [CrossRef]
- Fassari, S.; Inglese, G. On the spectrum of the harmonic oscillator with a δ-type perturbation. Helv. Phys. Acta 1994, 67, 650–659. [Google Scholar]
- Mityagin, B.S.; Siegl, P. Root system of singular perturbations of the harmonic oscillator type operators. Lett. Math. Phys. 2016, 106, 147–167. [Google Scholar] [CrossRef] [Green Version]
- Simon, B. The bound state of weakly coupled Schrödinger operators in one and two dimensions. Ann. Phys. 1976, 97, 279–288. [Google Scholar] [CrossRef]
- Gesztesy, F.; Kirsten, K. On traces and modified Fredholm determinants for half-line Schrödinger operators with purely discrete spectra. Q. Appl. Math. 2019, 77, 615–630. [Google Scholar] [CrossRef] [Green Version]
- Reed, M.; Simon, B. Analysis of Operators, Methods in Modern Mathematical Physics; Academic Press: New York, NY, USA, 1978. [Google Scholar]
- Klaus, M.; Simon, B. Coupling constant thresholds in nonrelativistic quantum mechanics. I. Short-range two-body case. Ann. Phys. 1980, 130, 251–281. [Google Scholar] [CrossRef]
- Reed, M.; Simon, B. Scattering Theory, Methods in Modern Mathematical Physics; Academic Press: New York, NY, USA, 1979. [Google Scholar]
- Fassari, S. On the bound states of non-relativistic Kronig-Penney Hamiltonians with short range impurities. Helv. Phys. Acta 1990, 63, 849–883. [Google Scholar]
- Fassari, S. On the bound states of relativistic Kronig-Penney Hamiltonians with short range impurities. Helv. Phys. Acta 1990, 63, 884–907. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fassari, S.; Gadella, M.; Nieto, L.M.; Rinaldi, F. The Energy of the Ground State of the Two-Dimensional Hamiltonian of a Parabolic Quantum Well in the Presence of an Attractive Gaussian Impurity. Symmetry 2021, 13, 1561. https://doi.org/10.3390/sym13091561
Fassari S, Gadella M, Nieto LM, Rinaldi F. The Energy of the Ground State of the Two-Dimensional Hamiltonian of a Parabolic Quantum Well in the Presence of an Attractive Gaussian Impurity. Symmetry. 2021; 13(9):1561. https://doi.org/10.3390/sym13091561
Chicago/Turabian StyleFassari, Silvestro, Manuel Gadella, Luis Miguel Nieto, and Fabio Rinaldi. 2021. "The Energy of the Ground State of the Two-Dimensional Hamiltonian of a Parabolic Quantum Well in the Presence of an Attractive Gaussian Impurity" Symmetry 13, no. 9: 1561. https://doi.org/10.3390/sym13091561
APA StyleFassari, S., Gadella, M., Nieto, L. M., & Rinaldi, F. (2021). The Energy of the Ground State of the Two-Dimensional Hamiltonian of a Parabolic Quantum Well in the Presence of an Attractive Gaussian Impurity. Symmetry, 13(9), 1561. https://doi.org/10.3390/sym13091561