Symmetry and Combinatorial Concepts for Cyclopolyarenes, Nanotubes and 2D-Sheets: Enumerations, Isomers, Structures Spectra & Properties
Abstract
:1. Introduction
2. Combinatorial Methods: Generalized Character Cycle Indices, Sheehan’s Method, Euler’s Totient Function and Character Based Enumerations
3. Applications to Polyarenes Enumeration & Spectroscopy
3.1. Enumeration of Hetero-Substituted Polyarenes and Polysubstituted Polyarenes
- Case (1): m even, n odd, D: protons:
- Case (2): m even, n even, D: protons:
- Case (3): m odd, n odd, D: protons:
- Case (4): m odd, n even, D: protons:
- Case (1): m even
- Case (2): m odd
- Case (1): m even, n odd, protons:
- Case (2): m even, n even, protons:
- Case (3): m odd, n odd, protons:
- Case (4): m odd, n even, protons:
3.2. Applications to 13C, Proton NMR and Multiple Quantum NMR of Polyarenes
(n + 4)/2 if n is even.
α48 + 5α47β + 109α46β2 + 1467α45β3 + 16398α44β4 +142945α43β5 +1024059α42β6 +6137527α41β7 + 31453488α40β8 + 139767835α39β9 + 545091767α38β10 + 1882967013α37β11 + 5805816362α36β12 +16077455055α35β13 + 40193661777α34β14 + 91105252497α33β15 + 187904675706α32β16 + 353702280690α31β17 + 609154167250α30β18 + 961821475230α29β19 + 1394641595644α28β20 + 1859521084850α27β21 + 2282140209534α26β22 + 2579809646726α25β23 + 2687302591938α24β24 +…
α24 + 3α23β + 31α22β2 + 181α21β3 + 934α20β4 + 3597α19β5 + 11395α18β6 + 29007α17β7+ 61698α16β8 + 109298α15β9 + 164110α14β10 + 208474α13β11 + 226150α12β12 +…
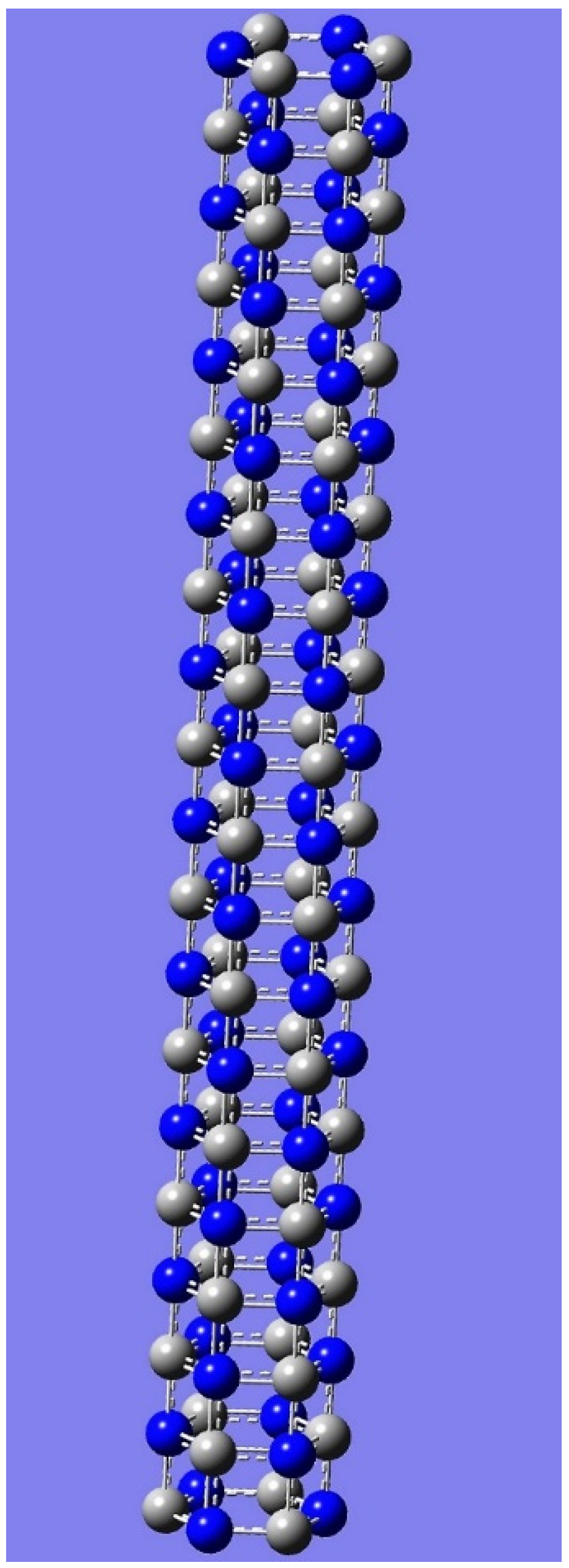
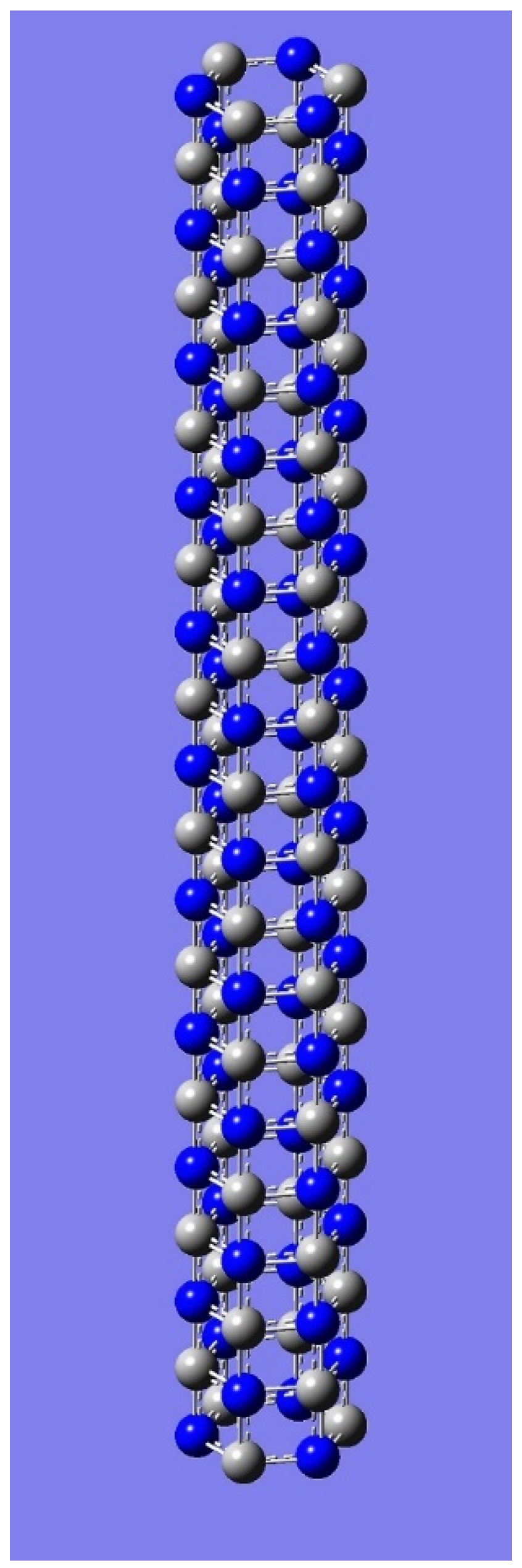
4. Applications to Nanotubes: Enumerations & Chirality
- (a)
- all vertices of the top/bottom layers are colored with a single color (white) while the vertices of the central layers are colored with different colors;
- (b)
- all vertices of the central layers are colored with a single color (white) while the vertices of the top/bottom layers are colored with different colors;
- (c)
- all vertices of the central layers and vertices of the top/bottom layers are colored with different colors chosen from a single set of colors without making any distinction between vertices of the central layers and vertices of the top/bottom layers.
- (d)
- all vertices of the central layers are colored with colors chosen from one set while all vertices of the top/bottom layers are colored with colors chosen from a different set of colors thus making a distinction between vertices of the central layers and vertices of the top/bottom layers.
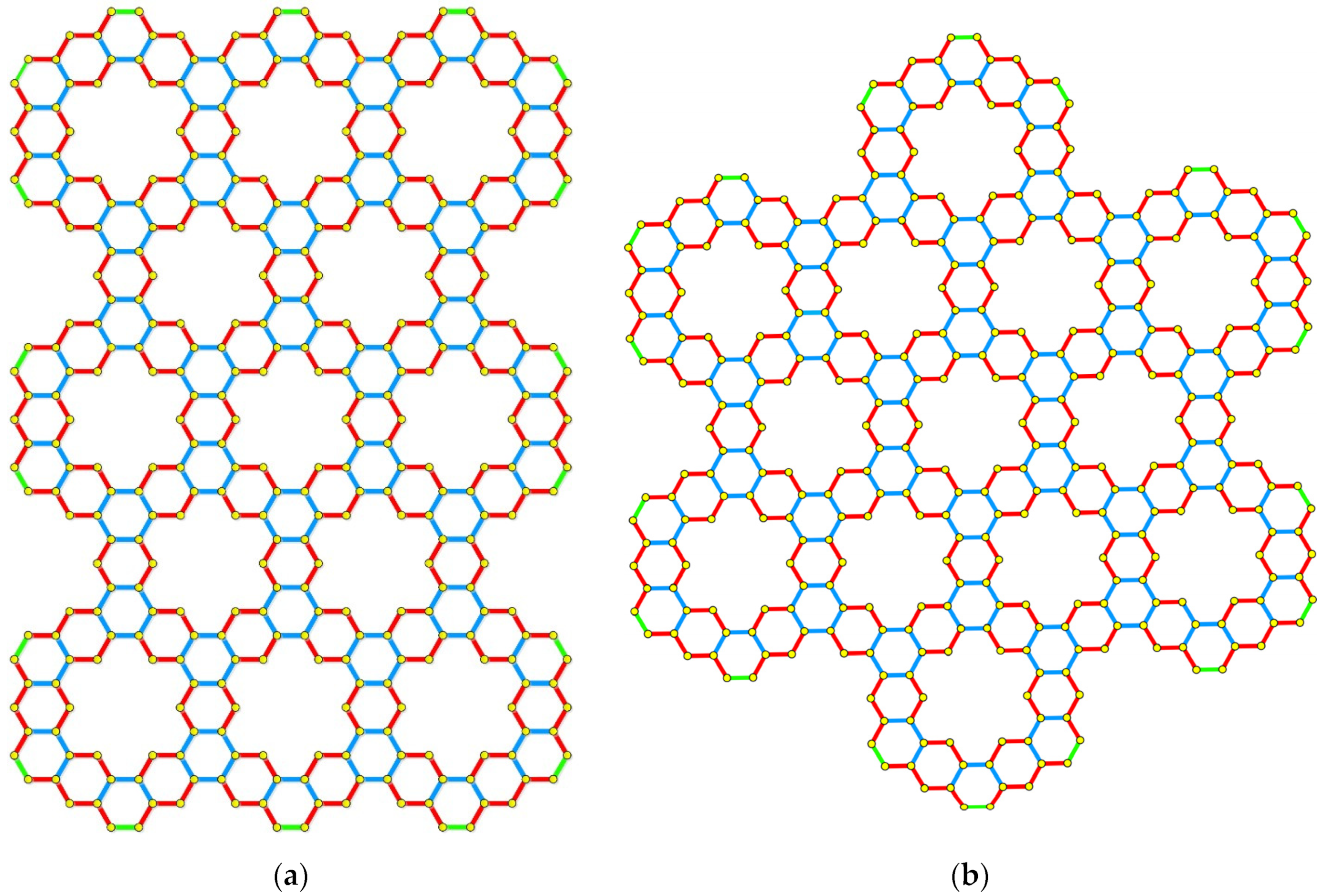
5. Applications to Tessellations of Kekulenes, Nanobands, C60 Polymers, Spectroscopy & Topology
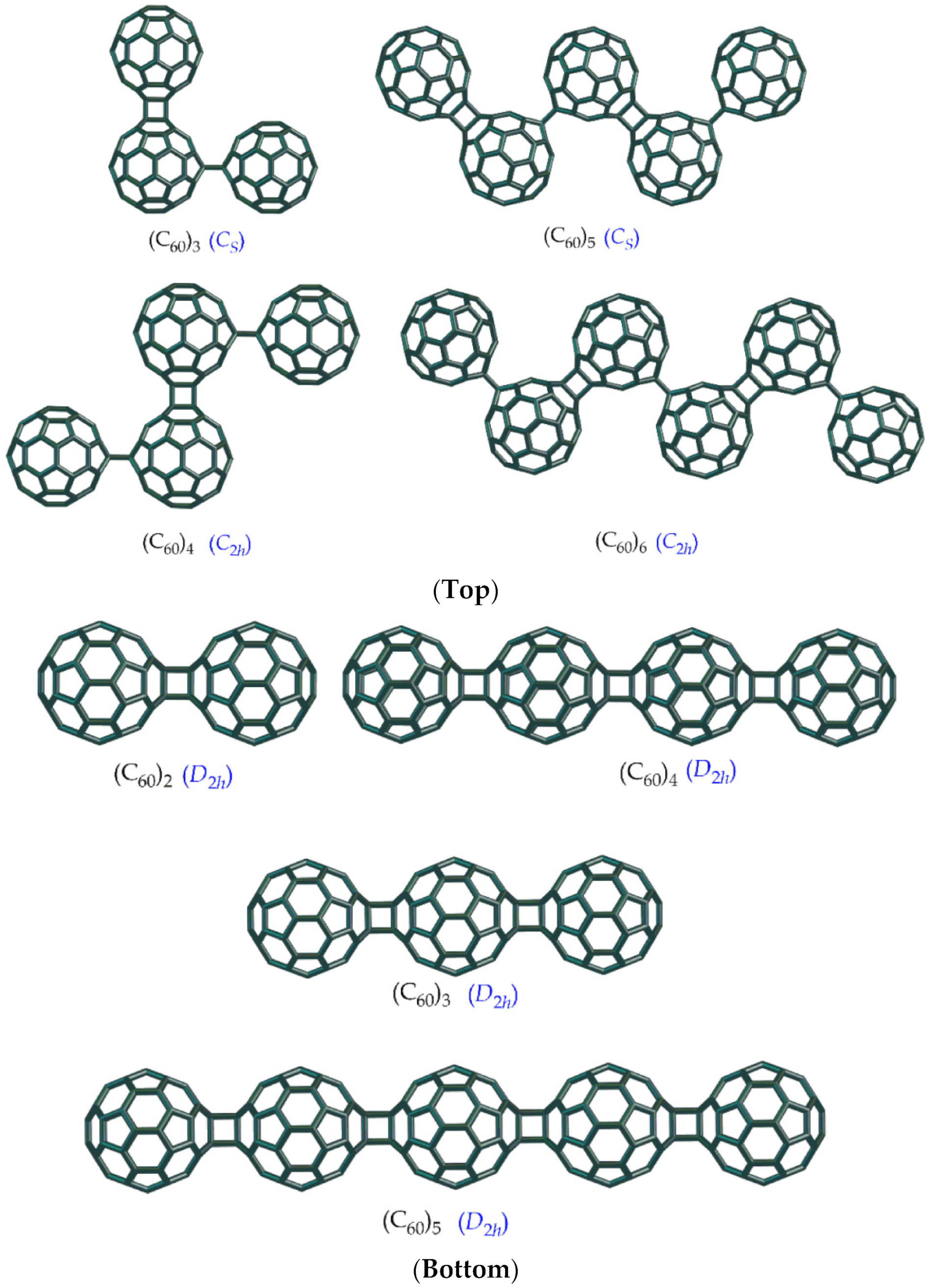
6. Conclusions, Helical Structures, Fullerene Polymers and Other Structural Derivatives & Future Perspectives
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Diederich, F.; Staab, H.A. Benzenoid versus Annulenoid Aromaticity: Synthesis and Properties of Kekulene. Angew. Chem. Int. Ed. Engl. 1978, 17, 372–374. [Google Scholar] [CrossRef]
- Schweitzer, D.; Hausser, K.H.; Vogler, H.; Diederich, F.; Staab, H.A. Electronic Properties of Kekulene. Mol. Phys. 1982, 46, 1141–1153. [Google Scholar] [CrossRef] [Green Version]
- Staab, H.A.; Diederich, F. Cycloarenes a New Class of Aromatic Compounds, I. Synthesis of Kekulene. Chem. Ber. 1983, 116, 3487–3503. [Google Scholar] [CrossRef]
- Miyoshi, H.; Nobusue, S.; Shimizu, A.; Tobe, Y. Non-alternant non-benzenoid kekulenes: The birth of a new kekulene family. Chem. Soc. Rev. 2015, 44, 6560–6577. [Google Scholar] [CrossRef]
- Funhoff, D.J.; Staab, H.A. Cyclodecakisbenzene, a new cycloarene. Angew. Chem. Int. Ed. Engl. 1986, 25, 742–744. [Google Scholar] [CrossRef]
- Kumar, B.; Viboh, R.L.; Bonifacio, M.C.; Thompson, W.B.; Buttrick, J.C.; Westlake, B.C.; Kim, M.-S.; Zoellner, R.W.; Varganov, S.A.; Mörschel, P.; et al. Septulene: The heptagonal homologue of kekulenes. Angew. Chem. Int. Ed. 2012, 51, 12795–12800. [Google Scholar] [CrossRef]
- Buttrick, J.C.; King, B.T. Kekulenes, cycloarenes, and heterocycloarenes: Addressing electronic structure and aromaticity through experiments and calculations. Chem. Soc. Rev. 2017, 46, 7–20. [Google Scholar] [CrossRef]
- Liu, C.; Sandoval-Salinas, M.E.; Hong, Y.; Gopalakrishna, T.Y.; Phan, H.; Aratani, N.; Herng, T.S.; Ding, J.; Yamada, H.; Kim, D.; et al. Macrocyclic polyradicaloids with unusual super-ring structure and global aromaticity. Chem 2018, 4, 1586–1595. [Google Scholar] [CrossRef] [Green Version]
- Nakakuki, Y.; Hirose, T.; Matsuda, K. Synthesis of a helical analogue of kekulene: A flexible π-expanded helicene with large helical diameter acting as a soft molecular spring. J. Am. Chem. Soc. 2018, 140, 15461–15469. [Google Scholar] [CrossRef]
- Pozo, I.; Majzik, Z.; Pavlicek, N.; Melle-Franco, M.; Guitián, E.; Peña, D.; Gross, L.; Pérez, D. Revisiting kekulene: Synthesis and single-molecule imaging. J. Am. Chem. Soc. 2019, 141, 15488–15493. [Google Scholar] [CrossRef] [Green Version]
- Arejdal, M. Prediction of the magnetocaloric behaviors of the kekulene structure for the magnetic refrigeration. Results Phys. 2020, 18, 103342. [Google Scholar] [CrossRef]
- Ji, L.; Shu, Y.; Wenxiang, W.; Lingxu, L.; Hongda, L.; Soleymani, H. Potential application of kekulene nanoring in the Li-ion batteries: DFT studies. Comput. Theor. Chem. 2020, 1181, 112796. [Google Scholar] [CrossRef]
- Hou, H.; Zhao, X.J.; Tang, C.; Ju, Y.Y.; Deng, Z.Y.; Wang, X.R.; Feng, L.B.; Lin, D.H.; Hou, X.; Narita, A.; et al. Synthesis and assembly of extended quintulene. Nat. Commun. 2020, 11, 3976. [Google Scholar] [CrossRef]
- Yang, L.; Zhang, N.; Han, Y.; Zou, Y.; Qiao, Y.; Chang, D.; Zhao, Y.; Lu, X.; Wu, J.; Liu, Y. A sulfur-containing hetero-octulene: Synthesis, host–guest properties, and transistor applications. Chem. Commun. 2020, 56, 9990–9993. [Google Scholar] [CrossRef]
- Swager, T.M.; Lu, R. A Missing Member of the Cycloarene Family: Quintulene. Synfacts 2020, 16, 1289. [Google Scholar]
- Di Giovannantonio, M.; Yao, X.; Eimre, K.; Urgel, J.I.; Ruffieux, P.; Pignedoli, C.A.; Müllen, K.; Fasel, R.; Narita, A. Large-cavity coronoids with different inner and outer edge structures. J. Am. Chem. Soc. 2020, 142, 12046–12050. [Google Scholar] [CrossRef]
- Fan, W.; Han, Y.; Wang, X.; Hou, X.; Wu, J. Expanded Kekulenes. J. Am. Chem. Soc. 2021, 143, 13908–13916. [Google Scholar] [CrossRef]
- Lu, X.; An, D.; Han, Y.; Zou, Y.; Qiao, Y.; Zhang, N.; Chang, D.; Wu, J.; Liu, Y. A cyclopenta-fused dibenzo [b,d] thiophene-co-phenanthrene macrocyclic tetraradicaloid. Chem. Sci. 2021, 12, 3952–3957. [Google Scholar] [CrossRef]
- Fan, W.; Han, Y.; Dong, S.; Li, G.; Lu, X.; Wu, J. Facile Synthesis of Aryl-Substituted Cycloarenes via Bismuth (III) Triflate-Catalyzed Cyclization of Vinyl Ethers. CCS Chem. 2021, 3, 1445–1452. [Google Scholar] [CrossRef]
- Martinez-Castro, J.; Bolat, R.; Fan, Q.; Werner, S.; Arefi, H.H.; Esat, T.; Sundermeyer, J.; Wagner, C.; Gottfried, J.M.; Temirov, R.; et al. Disentangling the Complex Electronic Structure of an Adsorbed Nanographene: Cycloarene C108. arXiv arXiv:2110.11449.
- Liu, H.; Zhuang, G.; Wang, S.; Huang, P.; Chen, M.; Yang, S.; Du, P. Synthesis and Photophysical Properties of [3]Cyclo-1,8-pyrenes via [4+2] Cycloaddition Reaction. J. Org. Chem. 2021, 86, 7038–7045. [Google Scholar] [CrossRef]
- Su, J.; Fan, W.; Mutombo, P.; Peng, X.; Song, S.; Ondráček, M.; Golub, P.; Brabec, J.; Veis, L.; Telychko, M.; et al. On-Surface synthesis and characterization of [7]triangulene quantum ring. Nano Lett. 2020, 21, 861–867. [Google Scholar] [CrossRef]
- Itami, K.; Krzeszewski, M.; Ito, H. Infinitene: A Helically Twisted Figure-Eight [12]Circulene Topoisomer. 2021. Available online: https://chemrxiv.org/engage/api-gateway/chemrxiv/assets/orp/resource/item/6165026b35b4062ec310d759/original/infinitene-a-helically-twisted-figure-eight-12-circulene-topoisomer.pdf (accessed on 20 November 2021).
- Tang, M.C.; Wei, Y.C.; Chu, Y.C.; Jiang, C.X.; Huang, Z.X.; Wu, C.C.; Chao, T.H.; Hong, P.H.; Cheng, M.J.; Chou, P.T.; et al. [2, 2](5, 8) Picenophanedienes: Syntheses, Structural Analyses, Molecular Dynamics, and Reversible Intramolecular Structure Conversion. J. Am. Chem. Soc. 2020, 142, 20351–20358. [Google Scholar] [CrossRef]
- Chen, H.; Miao, Q. Recent advances and attempts in synthesis of conjugated nanobelts. J. Phys. Org. Chem. 2020, 33, e4145. [Google Scholar] [CrossRef]
- Maghsoumi, A.; Beser, U.; Feng, X.; Narita, A.; Müllen, K.; Castiglioni, C.; Tommasini, M. Raman spectroscopy of holey nanographene C216. J. Raman Spectrosc. 2021, 52, 2301–2316. [Google Scholar] [CrossRef]
- Kiel, G.R.; Bergman, H.M.; Tilley, T.D. Site-selective [2+2+n] cycloadditions for rapid, scalable access to alkynylated polycyclic aromatic hydrocarbons. Chem. Sci. 2020, 11, 3028–3035. [Google Scholar] [CrossRef] [Green Version]
- Beser, U.; Kastler, M.; Maghsoumi, A.; Wagner, M.; Castiglioni, C.; Tommasini, M.; Narita, A.; Feng, X.; Klaus Müllen, M. Hexakis(4-iodophenyl)-peri-hexabenzocoronene—A Versatile Building Block for Highly Ordered Discotic Liquid Crystalline Materials. J. Am. Chem. Soc. 2004, 126, 177–186. [Google Scholar] [CrossRef]
- Saito, S.; Osuka, A. Expanded Porphyrins: Intriguing Structures, Electronic Properties, and Reactivities. Angew. Chem. Int. Ed. 2011, 50, 4342–4373. [Google Scholar] [CrossRef]
- Haags, A.; Reichmann, A.; Fan, Q.; Egger, L.; Kirschner, H.; Naumann, T.; Werner, S.; Vollgraff, T.; Sundermeyer, J.; Eschmann, L.; et al. Kekulene: On-Surface Synthesis, Orbital Structure, and Aromatic Stabilization. ACS Nano 2020, 14, 15766–15775. [Google Scholar] [CrossRef]
- Xu, M.; Liang, T.; Shi, M.; Chen, H. Graphene-like two-dimensional materials. Chem. Rev. 2013, 113, 3766–3798. [Google Scholar] [CrossRef]
- Majewski, M.A.; Hong, Y.; Lis, T.; Gregoliński, J.; Chmielewski, P.J.; Cybińska, J.; Kim, D.; Stępień, M. Octulene: A Hyperbolic Molecular Belt that Binds Chloride Anions. Angew. Chem. Int. Ed. 2016, 55, 14072–14076. [Google Scholar] [CrossRef] [PubMed]
- Beser, U.; Kastler, M.; Maghsoumi, A.; Wagner, M.; Castiglioni, C.; Tommasini, M.; Narita, A.; Feng, X.; Klaus Müllen, M. A C216-Nanographene Molecule with Defined Cavity as Extended Coronoid. J. Am. Chem. Soc. 2016, 138, 4322–4325. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, J.; Zhuang, G.; Chen, M.; Lu, D.; Li, Z.; Huang, Q.; Jia, H.; Cui, S.; Shao, X.; Yang, S.; et al. Selective Synthesis of Conjugated Chiral Macrocycles: Sidewall Segments of (−)/(+)-(12,4) Carbon Nanotubes with Strong Circularly Polarized Luminescence. Angew. Chem. 2020, 132, 1636–1643. [Google Scholar] [CrossRef]
- Fan, W.; Matsuno, T.; Han, Y.; Wang, X.; Zhou, Q.; Isobe, H.; Wu, J. Synthesis and Chiral Resolution of Twisted Carbon Nanobelts. J. Am. Chem. Soc. 2021, 143, 15924–15929. [Google Scholar] [CrossRef] [PubMed]
- Zhong, Q.; Ihle, A.; Ahles, S.; Wegner, H.A.; Schirmeisen, A.; Ebeling, D. Constructing covalent organic nanoarchitectures molecule by molecule via scanning probe manipulation. Nat. Chem. 2021, 13, 1133–1139. [Google Scholar] [CrossRef] [PubMed]
- Thess, A.; Lee, R.; Nikolaev, P.; Dai, H.; Petit, P.; Robert, J.; Xu, C.; Lee, Y.H.; Kim, S.G.; Rinzler, A.G.; et al. Crystalline ropes of metallic carbon nanotubes. Science 1996, 273, 483–487. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bandow, S.; Rao, A.M.; Williams, K.A.; Thess, A.; Smalley, R.E.; Eklund, P.C. Purification of single-wall carbon nanotubes by microfiltration. J. Phys. Chem. B 1997, 101, 8839–8842. [Google Scholar] [CrossRef]
- Liu, Y.; Dobrinsky, A.; Yakobson, B.I. Graphene edge from armchair to zigzag: The origins of nanotube chirality? Phys. Rev. Lett. 2010, 105, 235502. [Google Scholar] [CrossRef] [Green Version]
- Rodriguez, K.R.; Malone, M.A.; Nanney, W.A.; Maddux, C.J.; Coe, J.V.; Martínez, H.L. Generalizing thermodynamic properties of bulk single-walled carbon nanotubes. AIP Adv. 2014, 4, 127149. [Google Scholar] [CrossRef] [Green Version]
- Okada, S. Energetics of nanoscale graphene ribbons: Edge geometries and electronic structures. Phys. Rev. B 2008, 77, 041408. [Google Scholar] [CrossRef]
- Wang, Y.; Yan, L.; Dastafkan, K.; Zhao, C.; Zhao, X.; Xue, Y.; Huo, J.; Li, S.; Zhai, Q. Lattice Matching Growth of Conductive Hierarchical Porous MOF/LDH Heteronanotube Arrays for Highly Efficient Water Oxidation. Adv. Mater. 2021, 33, 2006351. [Google Scholar] [CrossRef]
- Burdanova, M.G.; Tsapenko, A.P.; Kharlamova, M.V.; Kauppinen, E.I.; Gorshunov, B.P.; Kono, J.; Lloyd-Hughes, J. A Review of the Terahertz Conductivity and Photoconductivity of Carbon Nanotubes and Heteronanotubes. Adv. Opt. Mater. 2021, 2101042. [Google Scholar] [CrossRef]
- Xiang, R.; Maruyama, S. Heteronanotubes: Challenges and Opportunities. Small Sci. 2021, 1, 2000039. [Google Scholar] [CrossRef]
- Xiang, R.; Inoue, T.; Zheng, Y.; Kumamoto, A.; Qian, Y.; Sato, Y.; Liu, M.; Tang, D.; Gokhale, D.; Guo, J.; et al. One-dimensional van der Waals heterostructures. Science 2020, 367, 537–542. [Google Scholar] [CrossRef] [Green Version]
- Cambré, S.; Liu, M.; Levshov, D.; Otsuka, K.; Maruyama, S.; Xiang, R. Nanotube-Based 1D Heterostructures Coupled by van der Waals Forces. Small 2021, 17, 2102585. [Google Scholar] [CrossRef]
- Sundararajan, J.P.; Bakharev, P.; Niraula, I.; Fouetio Kengne, B.A.; MacPherson, Q.; Sargent, M.; Hare, B.; McIlroy, D.N. Observation of Surface Plasmon Polariton Pumping of Optical Eigenmodes of Gold-Decorated Gallium Nitride Nanowires. Nano Lett. 2012, 12, 5181–5185. [Google Scholar] [CrossRef]
- Duan, X.; Wang, J.; Lieber, C.M. Synthesis and optical properties of gallium arsenide nanowires. Appl. Phys. Lett. 2000, 76, 1116–1118. [Google Scholar] [CrossRef] [Green Version]
- Hu, Y.; Kuemmeth, F.; Lieber, C.M.; Marcus, C.M. Hole spin relaxation in Ge–Si core–shell nanowire qubits. Nat. Nano Technol. 2012, 7, 47–50. [Google Scholar] [CrossRef] [Green Version]
- Zhang, H.J.; Wang, K.X.; Wu, X.Y.; Jiang, Y.M.; Zhai, Y.B.; Wang, C.; Wei, X.; Chen, J.S. MoO2/Mo2C heteronanotubes function as high-performance Li-ion battery electrode. Adv. Funct. Mater. 2014, 4, 3399–3404. [Google Scholar] [CrossRef]
- Ayala, P.; Arenal, R.; Loiseau, A.; Rubio, A.; Pichler, T. The physical and chemical properties of heteronanotubes. Rev. Mod. Phys. 2010, 82, 1843. [Google Scholar] [CrossRef] [Green Version]
- Chen, X.K.; Xie, Z.X.; Zhang, Y.; Deng, Y.X.; Zou, T.H.; Liu, J.; Chen, K.Q. Highly efficient thermal rectification in carbon/boron nitride heteronanotubes. Carbon 2019, 148, 532–539. [Google Scholar] [CrossRef]
- Hu, C.; Michaud-Rioux, V.; Yao, W.; Guo, H. Theoretical design of topological heteronanotubes. Nano Lett. 2019, 19, 4146–4150. [Google Scholar] [CrossRef]
- Zhong, R.L.; Sun, S.L.; Xu, H.L.; Qiu, Y.Q.; Su, Z.M. Multilithiation effect on the first hyperpolarizability of carbon–boron–nitride heteronanotubes: Activating segment versus connecting pattern. J. Phys. Chem. C 2014, 118, 14185–14191. [Google Scholar] [CrossRef]
- Zhong, R.L.; Sun, S.L.; Xu, H.L.; Qiu, Y.Q.; Su, Z.M. BN segment doped effect on the first hyperpolarizibility of heteronanotubes: Focused on an effective connecting pattern. J. Phys. Chem. C 2013, 117, 10039–10044. [Google Scholar] [CrossRef]
- Zhang, Z.Y.; Miao, C.; Guo, W. Nano-solenoid: Helicoid carbon–boron nitride hetero-nanotube. Nanoscale 2013, 5, 11902–11909. [Google Scholar] [CrossRef] [Green Version]
- Xu, X.; Wei, Y.; Liu, B.; Li, W.; Zhang, G.; Jiang, Y.; Tian, W.Q.; Liu, L. Chiral heteronanotubes: Arrangement-dominated chiral interface states and conductivities. Nanoscale 2019, 1, 8699–8705. [Google Scholar] [CrossRef]
- Zhong, R.L.; Sun, S.L.; Xu, H.L.; Qiu, Y.Q.; Su, Z.M. Helical carbon segment in carbon-boron-nitride heteronanotubes: Structure and nonlinear optical properties. ChemPlusChem 2014, 79, 732–736. [Google Scholar] [CrossRef]
- Meng, G.; Han, F.; Zhao, X.; Chen, B.; Yang, D.; Liu, J.; Xu, Q.; Kong, M.; Zhu, X.; Jung, Y.J.; et al. A general synthetic approach to interconnected nanowire/nanotube and nanotube/nanowire/nanotube heterojunctions with branched topology. Angew. Chem. 2009, 121, 7302–7306. [Google Scholar] [CrossRef]
- Silva, R.J.; Nitsche, H. Environmental actinide science. MRS Bull. 2001, 26, 707–713. [Google Scholar] [CrossRef]
- Schwaiger, L.K.; Parsons-Moss, T.; Hubaud, A.; Tueysuez, H.; Balasubramanian, K.; Yang, P.; Nitsche, H. Actinide and lanthanide complexation by organically modified mesoporous silica. Abstr. Pap. Am. Chem. Soc. 2010, 239, 1155. [Google Scholar]
- Cao, Z.; Balasubramanian, K. Theoretical studies of UO2(H2O)n2+, NpO2(H2O)n+, and PuO2 (H2O)n2+ complexes (n= 4–6) in aqueous solution and gas phase. J. Chem. Phys. 2005, 123, 114309. [Google Scholar] [CrossRef] [PubMed]
- Balasubramanian, K.; Chaudhuri, D. Computational modeling of environmental plutonyl mono-, di-and tricarbonate complexes with Ca counterions: Structures and spectra: PuO2(CO3)22−, PuO2(CO3)2Ca, and PuO2(CO3)3Ca3. Chem. Phys. Lett. 2008, 450, 196–202. [Google Scholar] [CrossRef]
- Knopp, R.; Panak, P.J.; Wray, L.A.; Renninger, N.S.; Keasling, J.D.; Nitsche, H. Laser spectroscopic studies of interactions of U(VI) with bacterial phosphate species. Chem.—Eur. J. 2003, 9, 2812–2818. [Google Scholar] [CrossRef] [PubMed]
- Cao, Z.; Balasubramanian, K.; Calvert, M.G.; Nitsche, H. Solvation effects on isomeric preferences of curium (III) complexes with multidentate phosphonopropionic acid ligands: CmH2PPA2+ and CmHPPA+ complexes. Inorg. Chem. 2009, 48, 9700–9714. [Google Scholar] [CrossRef]
- Aihara, J. Graph Theory of Ring-Current Diamagnetism. Bull. Chem. Soc. Jpn. 2018, 91, 274–303. [Google Scholar] [CrossRef]
- Aihara, J. Graph Theory of Aromatic Stabilization. Bull. Chem. Soc. Jpn. 2016, 89, 1425–1454. [Google Scholar] [CrossRef] [Green Version]
- Aihara, J. Graph-Theoretical Formulation of London Diamagnetism. J. Am. Chem. Soc. 1979, 101, 5913–5917. [Google Scholar] [CrossRef]
- Aihara, J.; Horikawa, T. Graph–Theoretical Formula for Ring Currents Induced in a Polycyclic Conjugated System. Bull. Chem. Soc. Jpn. 1983, 56, 1853–1854. [Google Scholar] [CrossRef] [Green Version]
- Aihara, J.J. Magnetotropism of biphenylene and related hydrocarbons. A circuit current analysis. J. Am. Chem. Soc. 1985, 107, 298–302. [Google Scholar] [CrossRef]
- Aihara, J. Circuit Resonance Energy: A Key Quantity That Links Energetic and Magnetic Criteria of Aromaticity. J. Am. Chem. Soc. 2006, 128, 2873–2879. [Google Scholar] [CrossRef]
- Aihara, J.; Kanno, H.; Ishida, T. Magnetic resonance energies of heterocyclic conjugated molecules. J. Phys. Chem. A 2007, 111, 8873–8876. [Google Scholar] [CrossRef]
- Aihara, J. Topological resonance energy, bond resonance energy, and circuit resonance energy. J. Phys. Org. Chem. 2008, 21, 79–85. [Google Scholar] [CrossRef]
- Dias, J.R. Valence-Bond Determination of Diradical Character of Polycyclic Aromatic Hydrocarbons: From Acenes to Rectangular Benzenoids. J. Phys. Chem. A 2013, 117, 4716–4725. [Google Scholar] [CrossRef]
- Aihara, J.; Makino, M.; Ishida, T.; Dias, J.R. Analytical study of superaromaticity in cycloarenes and related coronoid hydrocarbons. J. Phys. Chem. A 2013, 117, 4688–4697. [Google Scholar] [CrossRef]
- Dias, J.R. Search for singlet-triplet bistability or biradicaloid properties in polycyclic conjugated hydrocarbons: A valence-bond analysis. Mol. Phys. 2013, 6, 735–751. [Google Scholar] [CrossRef]
- Dias, J.R. What Do We Know about C24H14 Benzenoid, Fluoranthenoid, and Indacenoid Compounds? Polycycl. Aromat. Comp. 2014, 34, 177–190. [Google Scholar] [CrossRef]
- Dias, J.R. Nonplanarity Index for Fused Benzenoid Hydrocarbons. Polycycl. Aromat. Comp. 2014, 34, 161–176. [Google Scholar] [CrossRef]
- Dias, J.R. Perimeter topology of benzenoid polycyclic hydrocarbons. J. Chem. Inf. Model. 2005, 45, 562–571. [Google Scholar] [CrossRef]
- Dias, J.R. Isomers and chemical graphs of substituted cycloalkanes. Match 1982, 13, 315–324. [Google Scholar]
- Aihara, J. On the Number of Aromatic Sextets in a Benzenoid Hydrocarbon. Bull. Chem. Soc. Jpn. 1976, 49, 1429–1430. [Google Scholar] [CrossRef] [Green Version]
- Balaban, A.T. To be or not to be Aromatic. Rev. Roum. De Chim. 2015, 60, 121–140. [Google Scholar]
- Randić, M. On the role of kekule valence structures. Pure Appl. Chem. 1983, 55, 347–354. [Google Scholar] [CrossRef]
- Randić, M. Aromaticity and Conjugation. J. Am. Chem. Soc. 1977, 99, 444–450. [Google Scholar] [CrossRef]
- Randić, M. Aromaticity of Polycyclic Conjugated Hydrocarbons. Chem. Rev. 2003, 103, 3449–3606. [Google Scholar] [CrossRef]
- Vogler, H. Structures and 1H-chemical shifts of conjugation deficient hydrocarbons. Int. J. Quantum Chem. 1986, 30, 97–107. [Google Scholar] [CrossRef]
- Aihara, J. Is superaromaticity a fact or an artifact? The kekulene Problem. J. Am. Chem. Soc. 1992, 114, 865–868. [Google Scholar] [CrossRef]
- Clar, E. The Aromatic Sextet; Wiley: London, UK, 1972. [Google Scholar]
- Aihara, J. Aromaticity and superaromaticity in cyclopolyacenes. J. Chem. Soc. Perkin Trans. 1994, 2, 971–974. [Google Scholar] [CrossRef]
- Aihara, J. Lack of Superaromaticity in Carbon Nanotubes. J. Phys. Chem. 1994, 98, 9773–9776. [Google Scholar] [CrossRef]
- Aihara, J. Hückel-like rule of superaromaticity for charged paracyclophanes. Chem. Phys. Lett. 2003, 381, 147–153. [Google Scholar] [CrossRef]
- Aihara, J. A Simple Method for Estimating the Superaromatic Stabilization Energy of a Super-Ring Molecule. J. Phys. Chem. A 2008, 112, 4382–4385. [Google Scholar] [CrossRef]
- Aihara, J. Macrocyclic Conjugation Pathways in Porphyrins. J. Phys. Chem. A 2008, 112, 5305–5311. [Google Scholar] [CrossRef] [PubMed]
- Dias, J.R.; Aihara, J. Antiaromatic holes in graphene and related graphite defects. Mol. Phys. 2009, 107, 71–80. [Google Scholar] [CrossRef]
- Makino, M.; Aihara, J. Macrocyclic aromaticity of porphyrin units in fully conjugated oligoporphyrins. J. Phys. Chem. A 2012, 116, 8074–8084. [Google Scholar] [CrossRef] [PubMed]
- Aihara, J.; Nakagami, Y.; Sekine, R.; Makino, M. Validity and Limitations of the Bridged Annulene Model for Porphyrins. J. Phys. Chem. A 2012, 116, 11718–11730. [Google Scholar] [CrossRef] [PubMed]
- Dias, J.R. Structure and Electronic Characteristics of Coronoid Polycyclic Aromatic Hydrocarbons as Potential Models of Graphite Layers with Hole Defects. J. Phys. Chem. A 2008, 112, 12281–12292. [Google Scholar] [CrossRef]
- Cyvin, S.J.; Brunvoll, J.; Cyvin, B.N. Theory of Coronoid Hydrocarbons; Lecture Notes in Chemistry; Springer: Berlin, Germany, 1991. [Google Scholar]
- Cyvin, S.J.; Brunvoll, J.; Chen, R.S.; Cyvin, B.N.; Zhang, F.J. Theory of Coronoid Hydrocarbons II; Lecture Notes in Chemistry; Springer: Berlin, Germany, 1994; Volume 62. [Google Scholar]
- Gutman, I.; Milun, M.; Trinajstić, N. Graph theory and molecular orbitals. 19. Nonparametric resonance energies of arbitrary conjugated systems. J. Am. Chem. Soc. 1977, 99, 1692–1704. [Google Scholar] [CrossRef]
- Aihara, J.; Kanno, H. Local Aromaticities in Large Polyacene Molecules. J. Phys. Chem. A 2005, 109, 3717–3721. [Google Scholar] [CrossRef]
- Sekine, R.; Nakagami, Y.; Aihara, J. Aromatic Character of Polycyclic π Systems Formed by Fusion of Two or More Rings of the Same Size. J. Phys. Chem. A 2011, 115, 6724–6731. [Google Scholar] [CrossRef]
- Randić, M. Graph theoretical approach to π-electron currents in polycyclic conjugated hydrocarbons. Chem. Phys. Lett. 2010, 500, 123–127. [Google Scholar] [CrossRef]
- Randić, M.; Balaban, A.T.; Plavšić, D. Applying the conjugated circuits method to Clar structures of [n]phenylenes for determining resonance energies. Phys. Chem. Chem. Phys. 2011, 13, 20644–20648. [Google Scholar] [CrossRef]
- Balasubramanian, K.; Kaufman, J.J.; Koski, W.S.; Balaban, A.T. Graph theoretical characterization and computer generation of certain carcinogenic benzenoid hydrocarbons and identification of bay region. J. Comput. Chem. 1980, 1, 149–157. [Google Scholar] [CrossRef]
- Balasubramanian, K. Graph-Theoretical Characterization of Fullerene Cages. Polycycl. Aromat. Compd. 2006, 3, 247–259. [Google Scholar] [CrossRef]
- Basak, S.C.; Mills, D.; Mumtaz, M.M.; Balasubramanian, K. Use of topological indices in predicting aryl hydrocarbon receptor binding potency of dibenzofurans: A hierarchical QSAR Approach. Indian J. Chem. A 2003, 42, 1385–1391. [Google Scholar]
- Arockiaraj, M.; Clement, J.; Balasubramanian, K. Analytical expressions for topological properties of polycyclic benzenoid networks. J. Chemom. 2016, 30, 682–697. [Google Scholar] [CrossRef]
- Arockiaraj, M.; Clement, J.; Balasubramanian, K. Topological Indices and Their Applications to Circumcised Donut Benzenoid Systems, Kekulenes and Drugs. Polycycl. Aromat. Compd. 2018, 40, 280–303. [Google Scholar] [CrossRef]
- Hosoya, H.; Hosoi, K.; Gutman, I. A topological index for the total π–electron energy. Theor. Chim. Acta 1975, 38, 37–47. [Google Scholar] [CrossRef]
- Balasubramanian, K. Combinatorial Enumeration of Chemical Isomers. Indian J. Chem. Sect. B—Org. Chem. Incl. Med. Chem. 1978, 16, 1094–1096. [Google Scholar]
- Arockiaraj, M.; Klavzar, S.; Mushtaq, S.; Balasubramanian, K. Topological Characterization of the Full k-Subdivision of a Family of Partial Cubes and Their Applications to α-Types of Novel Graphyne and Graphdiyne Materials. Polycycl. Aromat. Compd. 2021, 41, 1902–1924. [Google Scholar] [CrossRef]
- Dias, J.R. Concealed Coronoid Hydrocarbons with Enhanced Antiaromatic Circuit Contributions as Models for Schottky Defects in Graphenes. Open Org. Chem. J. 2011, 5, 112–116. [Google Scholar] [CrossRef] [Green Version]
- Balasubramanian, K. Applications of Combinatorics and Graph Theory to Quantum Chemistry & Spectroscopy. Chem. Rev. 1985, 85, 599–618. [Google Scholar]
- Ramaraj, R.; Balasubramanian, K. Computer Generation of Matching Polynomials of Graphs and Lattices. J. Comput. Chem. 1985, 6, 122–141. [Google Scholar] [CrossRef]
- Balasubramanian, K. Spectra of chemical trees. Int. J. Quantum Chem. 1982, 21, 581–590. [Google Scholar] [CrossRef]
- Balasubramanian, K.; Randić, M. Characteristic Polynomials of Structures with Pending Bonds. Theor. Chim. Acta 1982, 61, 307–323. [Google Scholar] [CrossRef]
- Balasubramanian, K. Characteristic polynomials of organic polymers and periodic structures. J. Comput. Chem. 1985, 6, 656–661. [Google Scholar] [CrossRef]
- Balasubramanian, K. Computer Generation of Distance Polynomials of Graphs. J. Comput. Chem. 1990, 11, 829–836. [Google Scholar] [CrossRef]
- Manoharan, M.; Balakrishnarajan, M.M.; Venuvanalingam, P.; Balasubramanian, K. Topological resonance energy predictions of the stability of fullerene Clusters. Chem. Phys. Lett. 1994, 222, 95–100. [Google Scholar] [CrossRef]
- Balasubramanian, K. Matching Polynomials of Fullerene Clusters. Chem. Phys. Lett. 1993, 201, 306–314. [Google Scholar] [CrossRef]
- Balasubramanian, K. Nuclear-spin statistics of C60, C60H60 and C60D60. Chem. Phys. Lett. 1991, 183, 292–296. [Google Scholar] [CrossRef]
- Balasubramanian, K. Topological Peripheral Shapes and Distance-Based Characterization of Fullerenes C20-C720: Existence of Isoperipheral Fullerenes. Polycycl. Aromat. Compd. 2020, 1–19. [Google Scholar] [CrossRef]
- Balasubramanian, K. Combinatorial enumeration of isomers of superaromatic polysubstituted cycloarenes and coronoid hydrocarbons with applications to NMR. J. Phys. Chem. A 2018, 122, 8243–8257. [Google Scholar] [CrossRef] [PubMed]
- Balasubramanian, K. Combinatorics of NMR and ESR spectral simulations. J. Chem. Inf. Comput. Sci. 1992, 32, 296–298. [Google Scholar] [CrossRef]
- Hu, C.; Guo, H. 2N-rule: Searching topological phases and robust edge modes in carbon nanotubes. Appl. Phys. Lett. 2020, 117, 083101. [Google Scholar] [CrossRef]
- Huilgol, M.I.; Sriram, V.; Balasubramanian, K. Tensor and Cartesian products for nanotori, nanotubes and zig–zag polyhex nanotubes and their applications to 13C NMR spectroscopy. Mol. Phys. 2021, 119, e1817594. [Google Scholar] [CrossRef]
- Balasubramanian, K. Group theoretical analysis of vibrational modes and rovibronic levels of extended aromatic C48N12 azafullerene. Chem. Phys. Lett. 2004, 391, 64–68. [Google Scholar] [CrossRef]
- Balasubramanian, K.; Ottorino, O.; Cataldo, F.; Putz, M.V. Combinatorics of chiral and stereo isomers of substituted nanotubes: Applications of Eulerian character indices and comparison with bondonic formalism. Fuller. Nanotub. Carbon Nanostruct. 2021, 1–19. [Google Scholar] [CrossRef]
- Balasubramanian, K.; Ori, O.; Cataldo, F.; Ashrafi, A.R.; Putz, M.V. Face colorings and chiral face colorings of icosahedral giant fullerenes: C80 to C240. Fuller. Nanotub. Carbon Nanostruct. 2021, 29, 1–12. [Google Scholar] [CrossRef]
- Balasubramanian, K. Combinatorics of Edge Symmetry: Chiral and Achiral Edge Colorings of Icosahedral Giant Fullerenes: C80, C180, and C240. Symmetry 2020, 12, 8130. [Google Scholar] [CrossRef]
- Balasubramanian, K. Symmetry, Combinatorics, Artificial Intelligence, Music and Spectroscopy. Symmetry 2021, 13, 1850. [Google Scholar] [CrossRef]
- Huilgol, M.I.; Divya, B.; Balasubramanian, K. Distance degree vector and scalar sequences of corona and lexicographic products of graphs with applications to dynamic NMR and dynamics of nonrigid molecules and proteins. Theor. Chem. Acc. 2021, 140, 25. [Google Scholar] [CrossRef]
- Balasubramanian, K. Combinatorics of Supergiant Fullerenes: Enumeration of Polysubstituted Isomers, Chirality, Nuclear Magnetic Resonance, Electron Spin Resonance Patterns, and Vibrational Modes from C70 to C150000. J. Phys. Chem. A 2020, 124, 10359–10383. [Google Scholar] [CrossRef]
- Besalú, E.; Gironés, X.; Amat, L.; Carbó-Dorca, R. Molecular quantum similarity and the fundamentals of QSAR. Acc. Chem. Res. 2002, 35, 289–295. [Google Scholar] [CrossRef]
- Carbó-Dorca, R.; Mezey, P.G. Advances in Molecular Similarity; Elsevier: Amsterdam, The Netherlands, 1999; p. 296. [Google Scholar]
- Carbó-Dorca, R.; Chakraborty, T. Divagations about the periodic table: Boolean hypercube and quantum similarity connections. J. Comput. Chem. 2019, 40, 2653–2663. [Google Scholar] [CrossRef]
- Basak, S.C.; Gute, B.D.; Mills, D.; Hawkins, D.M. Quantitative molecular similarity methods in the property/toxicity estimation of chemicals: A comparison of arbitrary versus tailored similarity spaces. J. Mol. Struct. THEOCHEM 2003, 622, 127–145. [Google Scholar] [CrossRef]
- Mezey, P.G. Natural Molecular Fragments, Functional Groups, and Holographic Constraints on Electron Densities. Phys. Chem. Chem. Phys. 2012, 14, 8516–8522. [Google Scholar] [CrossRef]
- Mezey, P.G. Fuzzy Electron Density Fragments in Macromolecular Quantum Chemistry, Combinatorial Quantum Chemistry, Functional Group Analysis, and Shape-Activity Relations. Acc. Chem. Res. 2014, 47, 2821–2827. [Google Scholar] [CrossRef]
- Balasubramanian, K. Mathematical and computational techniques for drug discovery: Promises and developments. Curr. Top. Med. Chem. 2018, 18, 2774–2799. [Google Scholar] [CrossRef]
- Balasubramanian, K.; Gupta, S.P. Quantum molecular dynamics, topological, group theoretical and graph theoretical studies of protein-protein interactions. Curr. Top. Med. Chem. 2019, 19, 426–443. [Google Scholar] [CrossRef]
- Basak, S.C.; Grunwald, G.D.; Gute, B.D.; Balasubramanian, K.; Opitz, D. Use of statistical and neural net approaches in predicting toxicity of chemicals. J. Chem. Inf. Comput. Sci. 2000, 40, 885–890. [Google Scholar] [CrossRef]
- Kavitha, S.R.J.; Abraham, J.; Arockiaraj, M.; Jency, J.; Balasubramanian, K. Topological Characterization and Graph Entropies of Tessellations of Kekulene Structures: Existence of Isentropic Structures and Applications to Thermochemistry, Nuclear Magnetic Resonance, and Electron Spin Resonance. J. Phys. Chem. A 2021, 125, 8140–8158. [Google Scholar] [CrossRef]
- Arockiaraj, M.; Clement, J.; Paul, D.; Balasubramanian, K. Relativistic distance-based topological descriptors of Linde type A zeolites and their doped structures with very heavy elements. Mol. Phys. 2021, 119, e1798529. [Google Scholar] [CrossRef]
- Arockiaraj, M.; Paul, D.; Klavžar, S.; Clement, J.; Tigga, S.; Balasubramanian, K. Relativistic Distance Based and Bond Additive Topological Descriptors of Zeolite RHO Materials. J. Mol. Struct. 2022, 1250, e131798. [Google Scholar] [CrossRef]
- Arockiaraj, M.; Clement, J.; Paul, D.; Balasubramanian, K. Quantitative structural descriptors of sodalite materials. J. Mol. Struct. 2021, 1223, e128766. [Google Scholar] [CrossRef]
- Xu, W.; Pei, X.; Diercks, C.S.; Lyu, H.; Ji, Z.; Yaghi, O.M. A metal–organic framework of organic vertices and polyoxometalate linkers as a solid-state electrolyte. J. Am. Chem. Soc. 2019, 141, 17522–17526. [Google Scholar] [CrossRef] [PubMed]
- Liang, W.; Wied, P.; Carraro, F.; Sumby, C.J.; Nidetzky, B.; Tsung, C.; Falcaro, P.; Doonan, C.J. Metal–organic framework-based enzyme biocomposites. Chem. Rev. 2021, 121, 1077–1129. [Google Scholar] [CrossRef]
- Balasubramanian, K. Enumeration of the isomers of the gallium arsenide clusters (GamAsn). Chem. Phys. Lett. 1988, 150, 71–77. [Google Scholar] [CrossRef]
- Balasubramanian, K. Electronic structure of (GaAs)2. Chem. Phys. Lett. 1990, 171, 58–62. [Google Scholar] [CrossRef]
- Pitzer, K.S. (Ed.) Molecular Structure and Statistical Thermodynamics: Selected Papers of Kenneth S. Pitzer; World Scientific: Singapore, 1993; Volume 1. [Google Scholar]
- Bishea, G.A.; Morse, M.D. Resonant two-photon ionization spectroscopy of jet-cooled Au3. J. Chem. Phys. 1991, 95, 8779–8792. [Google Scholar] [CrossRef]
- Abraham, J.; Arockiaraj, M.; Jency, J.; Kavitha, S.R.J.; Balasubramanian, K. Graph Entropies, Enumeration of Circuits, Walks and Topological Properties of Three Classes of Isoreticular Metal Organic Frameworks. J. Math. Chem. 2021, in press. [Google Scholar]
- Balasubramanian, K. Generalization of the Harary–Palmer power group theorem to all irreducible representations of object and color groups-color combinatorial group theory. J. Math. Chem. 2014, 52, 703–728. [Google Scholar] [CrossRef]
- Pόlya, G.; Read, R.C. Combinatorial Enumeration of Groups, Graphs, and Chemical Compounds; Springer: New York, NY, USA, 2012; p. 148. [Google Scholar] [CrossRef]
- Sheehan, J. On Pόlya’s Theorem. Can. J. Math. 1967, 19, 792–799. [Google Scholar] [CrossRef]
- Bloom, G.S.; Kennedy, J.W.; Quintas, L.V. Some Problems Concerning Distance and Path Degree Sequences. In Graph Theory; Springer: Berlin/Heidelberg, Germany, 1983; pp. 179–190. [Google Scholar]
- Balasubramanian, K. Topochemie-2020-A Computational Package for Computing Topological Indices, Spectral Polynomials, Walks and Distance Degree Sequences and Combinatorial Generators. J. Phys. Chem. A 2021, 125, 8140–8158. [Google Scholar]
- Arockiaraj, M.; Prabhu, S.; Arulperumjothi, M.; Kavitha, S.R.J.; Balasubramanian, K. Topological characterization of hexagonal and rectangular tessellations of kekulenes as traps for toxic heavy metal ions. Theor. Chem. Acc. 2021, 140, 43. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Ori, O.; Tukhbatullina, A.A.; Shepelevich, I.S. Covalently Bonded Fullerene Nano-Aggregates (C60)n: Digitalizing Their Energy–Topology–Symmetry. Symmetry 2021, 13, 1899. [Google Scholar] [CrossRef]
- Matamala, A.R. Pólya’s Combinatorial Method and the isomer enumeration problem. Bol. Soc. Chil. Quím. 2002, 47, 105–112. [Google Scholar] [CrossRef]
- White, D.E. Counting patterns with a given automorphism group. Proc. Am. Math. Soc. 1975, 47, 41–44, Erratum in Proc. Am. Math. Soc. 1975, 50, 504. [Google Scholar] [CrossRef]
- Harary, F.; Palmer, E.M. Graphical Enumeration; Elsevier: Amsterdam, The Netherlands, 2014; p. 271. ISBN 978-0-12-324245-7. [Google Scholar] [CrossRef]
- Robinson, R.W.; Harary, F.; Balaban, A.T. The numbers of chiral and achiral alkanes and monosubstituted alkanes. Tetrahedron 1976, 32, 355–361. [Google Scholar] [CrossRef] [Green Version]
- Harary, F.; Mezey, P.G. Chiral and achiral square-cell configurations; the degree of chirality. In New Developments in Molecular Chirality; Springer: Dordrecht, Germany, 1991; pp. 241–256. [Google Scholar]
- Harary, F.; Palmer, E. The power group enumeration theorem. J. Comb. Theory 1966, 1, 157–173. [Google Scholar] [CrossRef] [Green Version]
- Gessel, I.M. Symmetric functions and P-recursiveness. J. Comb. Theory Ser. A 1990, 53, 257–285. [Google Scholar] [CrossRef] [Green Version]
- Read, R.C. The use of S-functions in combinatorial analysis. Can. J. Math. 1968, 20, 808–841. [Google Scholar] [CrossRef]
- Stanley, R.P. Graph colorings and related symmetric functions: Ideas and applications A description of results, interesting applications, & notable open problems. Discret. Math. 1998, 193, 267–286. [Google Scholar]
- Klein, D.J.; Babiċ, D.; Trinajstiċ, N. Enumeration in chemistry. In Chemical Modelling: Applications & Theory; Hinchliffe, A., Ed.; Royal Society of Chemistry: Cambridge, UK, 2002; Volume 2, pp. 56–95. [Google Scholar]



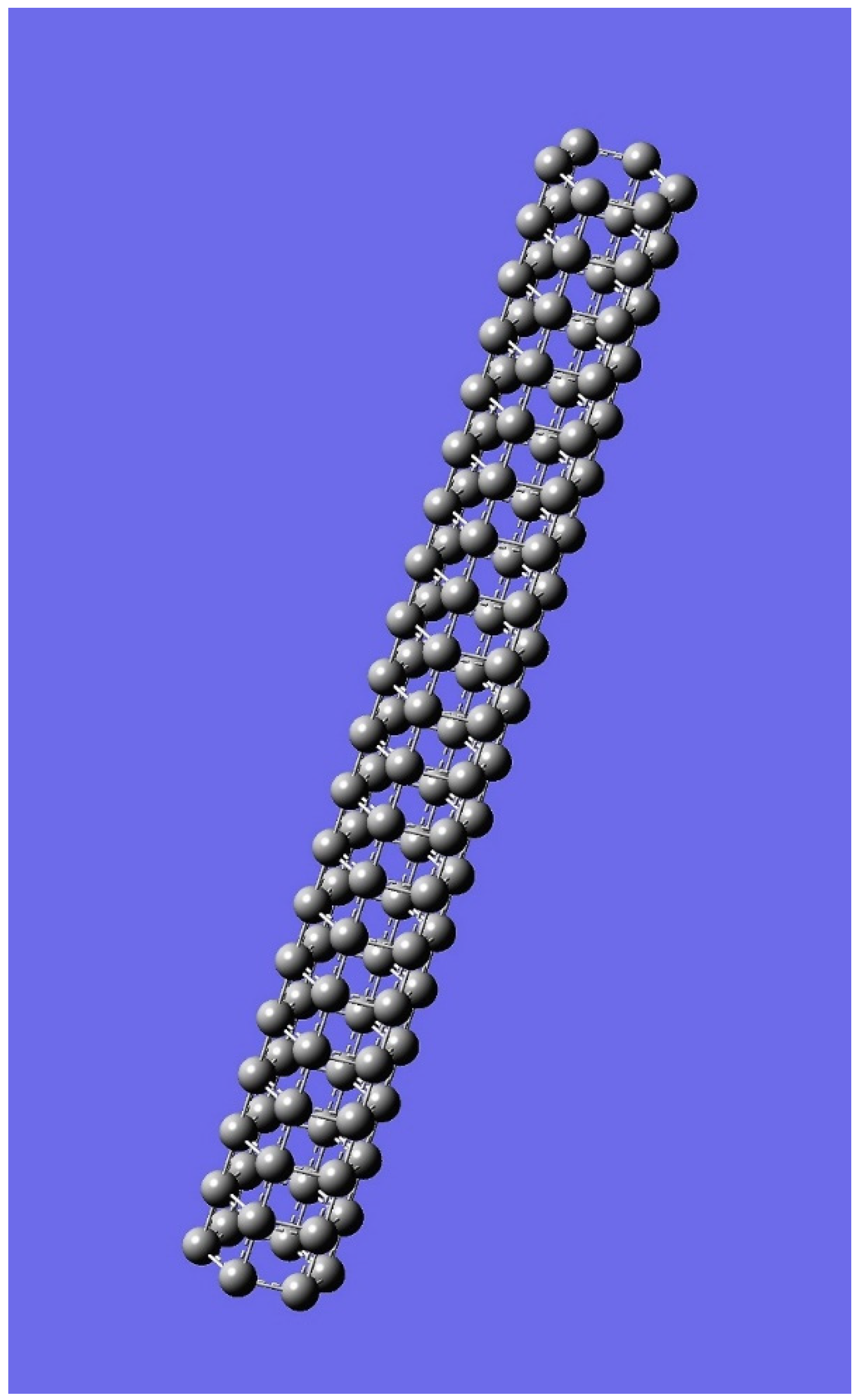

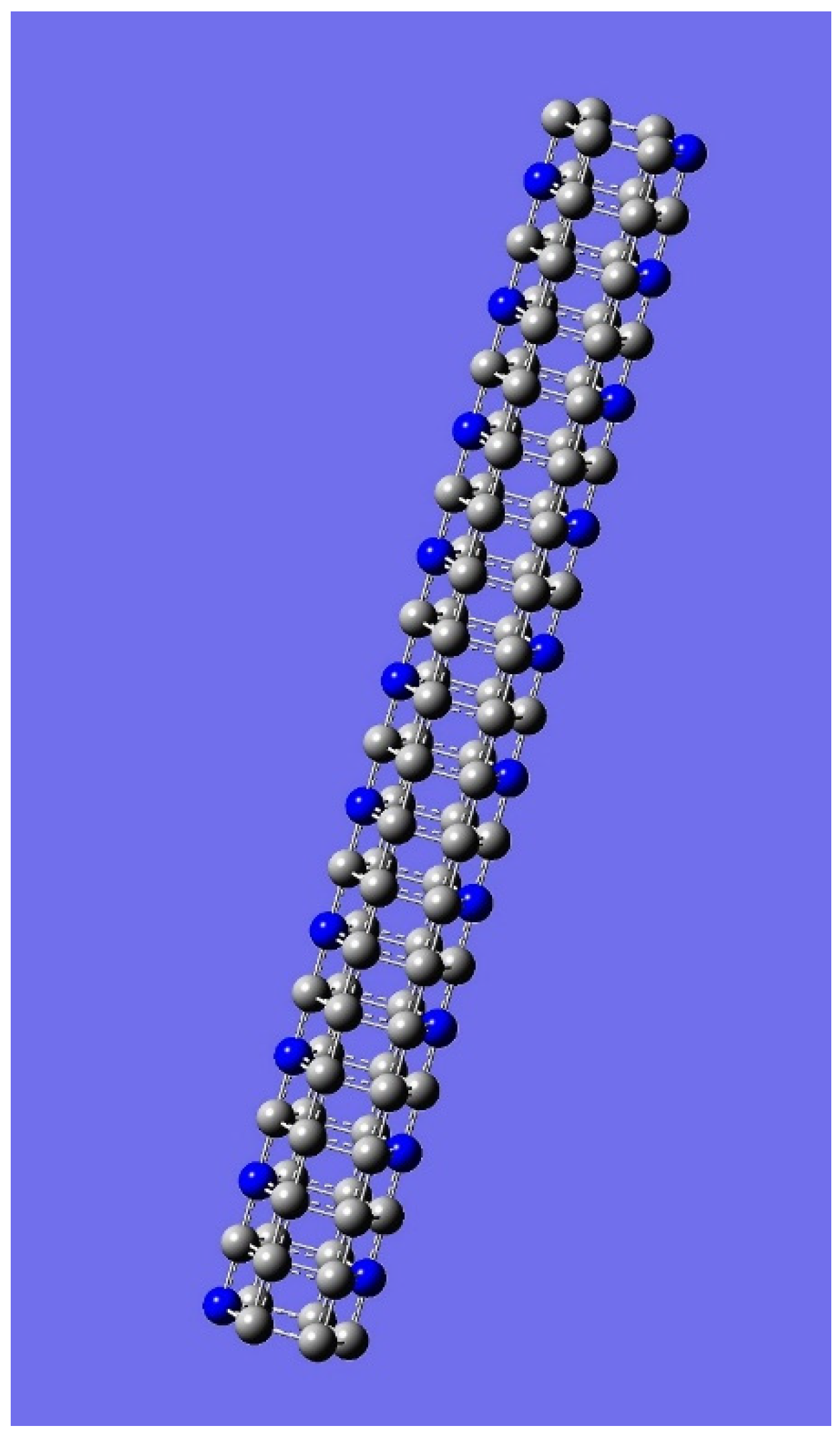
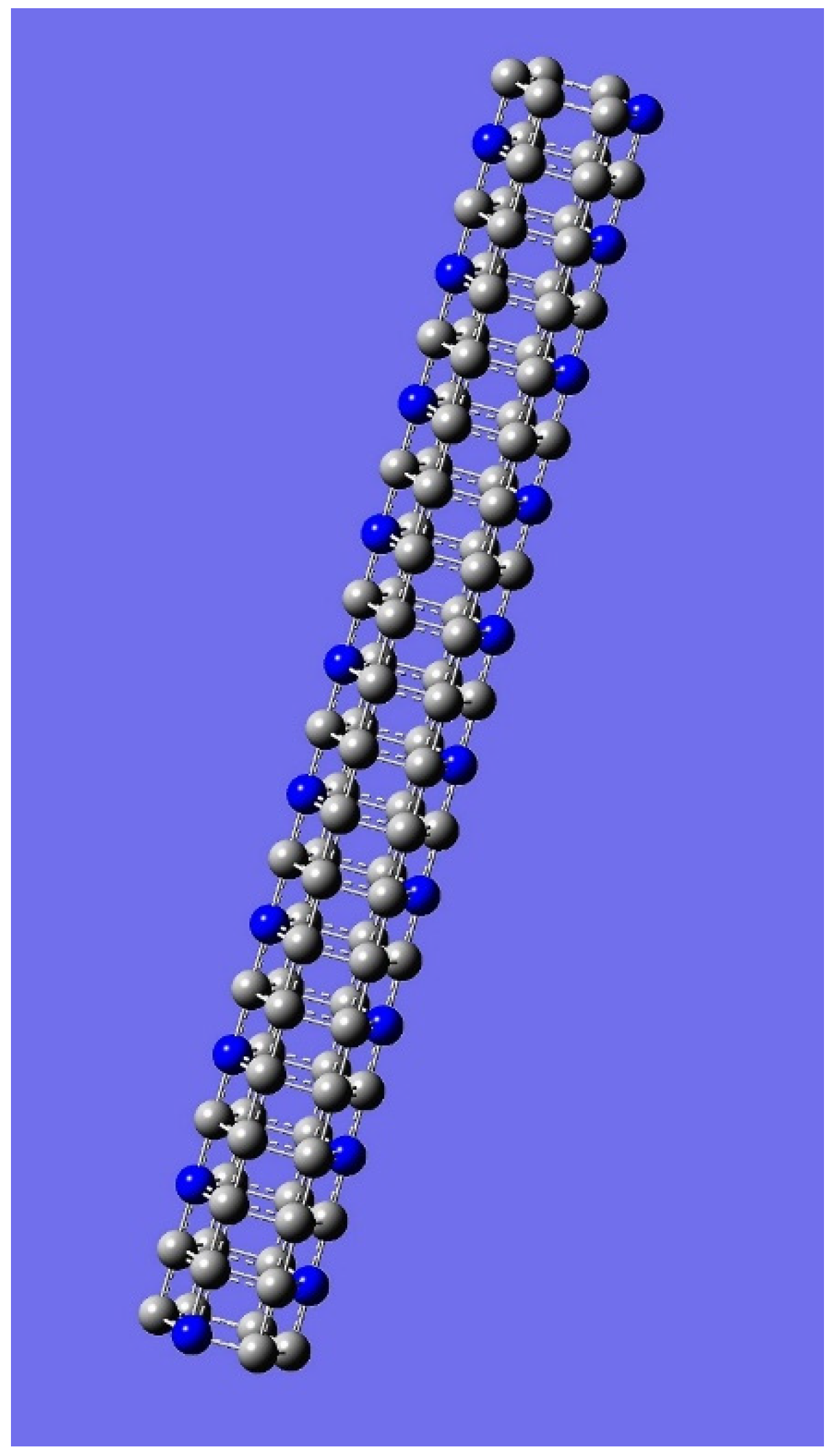

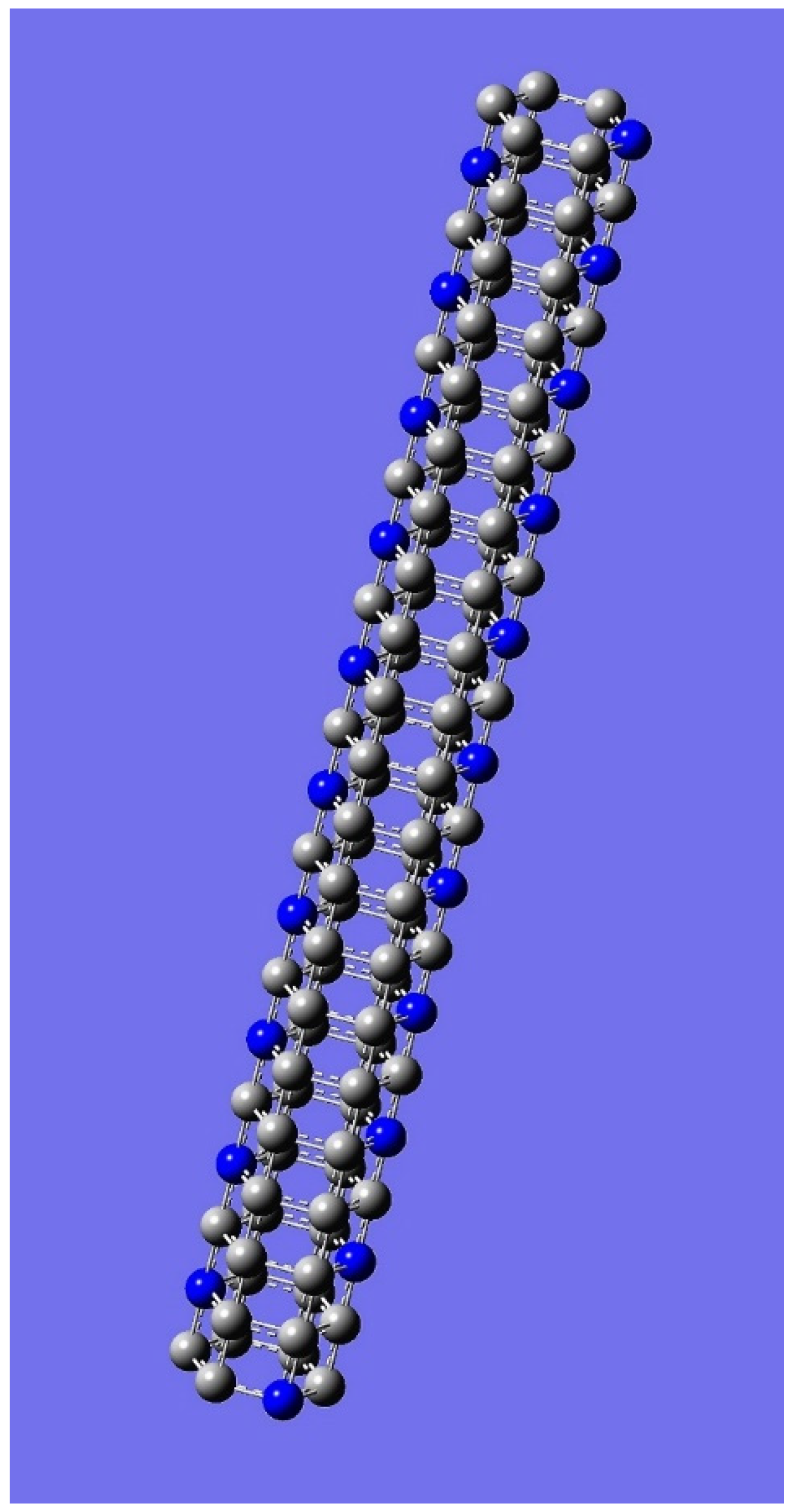
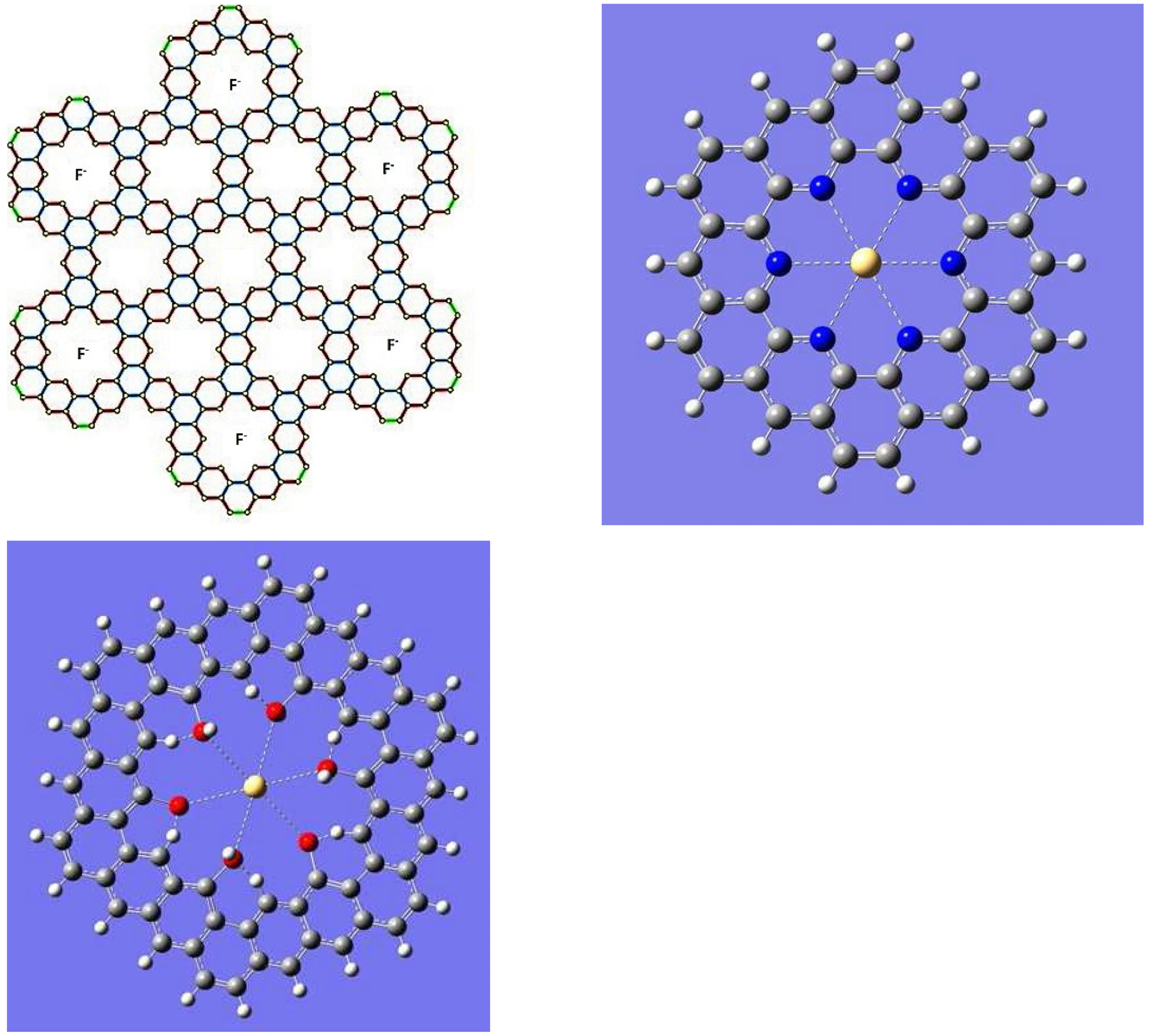


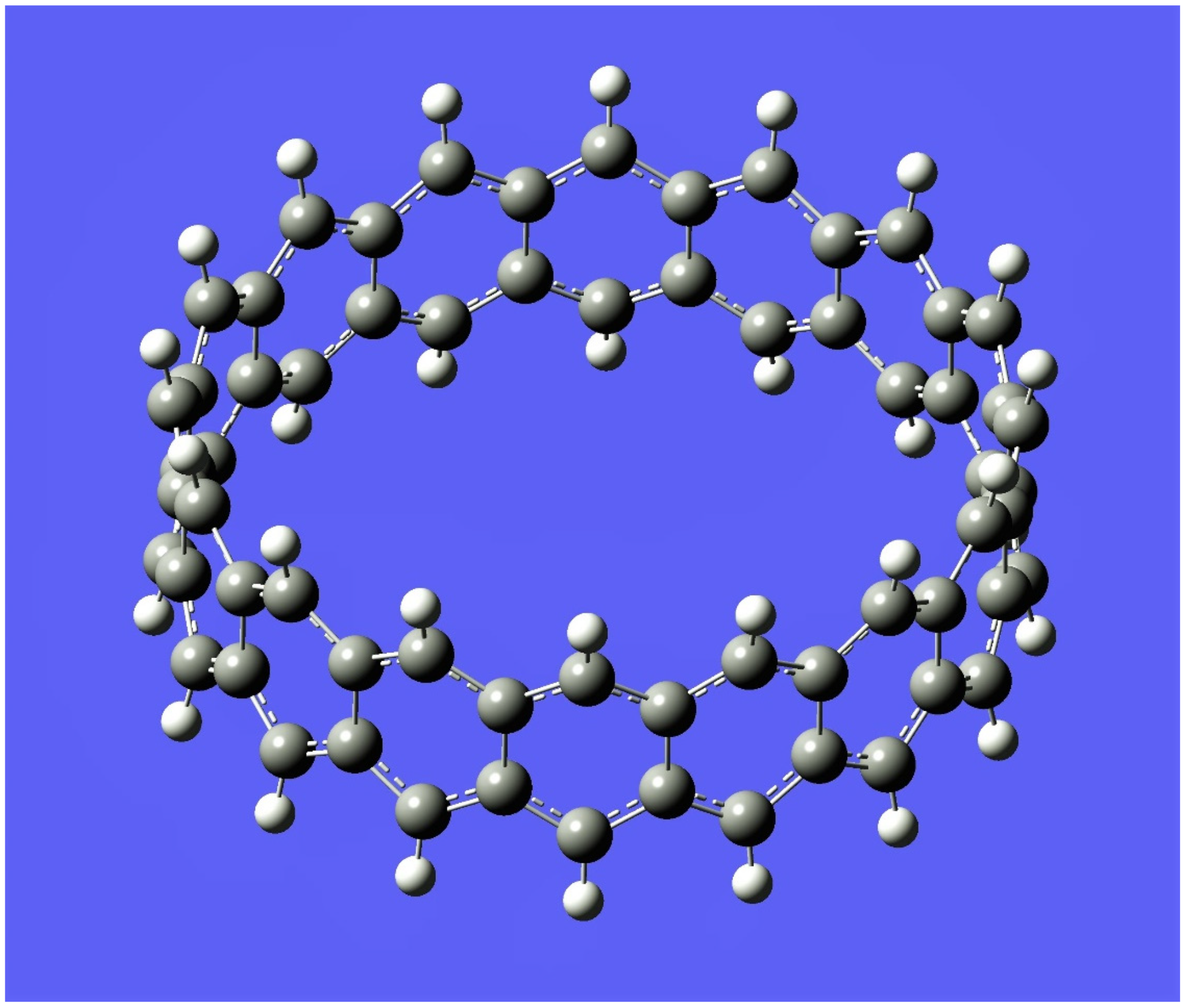
| n | M | Name | Formulae |
|---|---|---|---|
| 1 | 6 | Kekulene | C48H24 |
| 1 | 7 | Septulene | C56H28 |
| 1 | 8 | Octulene | C64H32 |
| 1 | 9 | Nonulene | C72H36 |
| 2 | 6 | 2-Kekulene | C90H30 |
| 2 | 7 | 2-Septulene | C105H35 |
| 2 | 8 | 2-Octulene | C120H40 |
| 2 | 9 | 2-Nonulene | C135H45 |
| … | … | … | … |
| n | N | ncir-m-cycloarene | Cm(n2+4n+3)Hm(n+3) |
| Coefficient | Vector | Coefficient | Vector | Coefficient | |
|---|---|---|---|---|---|
| 1 | 48 0 0 | 103561517895 | 37 9 2 | 88851068896152860 | 29 10 9 |
| 5 | 47 1 0 | 383177575979 | 36 10 2 | 234243726928693460 | 28 11 9 |
| 109 | 46 2 0 | 1254034278774 | 35 11 2 | 546568695999885020 | 27 12 9 |
| 1467 | 45 3 0 | 11184050659015080 | 29 14 5 | 1135181137598290860 | 26 13 9 |
| 16398 | 44 4 0 | 21622497914498712 | 28 15 5 | 2108193541021176180 | 25 14 9 |
| 142945 | 43 5 0 | 37839371323512066 | 27 16 5 | 3513655901417556180 | 24 15 9 |
| 1024059 | 42 6 0 | 60097825014257646 | 26 17 5 | 5270483851893361530 | 23 16 9 |
| 6137527 | 41 7 0 | 86807969439172982 | 25 18 5 | 7130654622923896350 | 22 17 9 |
| 31453488 | 40 8 0 | 114221012397502010 | 24 19 5 | 8715244538987104690 | 21 18 9 |
| 139767835 | 39 9 0 | 137065214861482908 | 23 20 5 | 9632638700903207990 | 20 19 9 |
| 545091767 | 38 10 0 | 150119044840161540 | 22 21 5 | 257668099806453820 | 28 10 10 |
| 1882967013 | 37 11 0 | 5364479296722 | 36 6 6 | 655882435174711976 | 27 11 10 |
| 5805816362 | 36 12 0 | 27588742194948 | 35 7 6 | 1475735479246515564 | 26 12 10 |
| 16077455055 | 35 13 0 | 120700745906895 | 34 8 6 | 2951470957321010124 | 25 13 10 |
| 40193661777 | 34 14 0 | 455980568919225 | 33 9 6 | 5270483852702315676 | 24 14 10 |
| 91105252497 | 33 15 0 | 1504735876402143 | 32 10 6 | 8432774162750027376 | 23 15 10 |
| 187904675706 | 32 16 0 | 4377413392313808 | 31 11 6 | 12122112859683551010 | 22 16 10 |
| 353702280690 | 31 17 0 | 11308317935237736 | 30 12 6 | 15687440169664356522 | 21 17 10 |
| 609154167250 | 30 18 0 | 26096118180492360 | 29 13 6 | 18302013532416736122 | 20 18 10 |
| 961821475230 | 29 19 0 | 54056244829095960 | 28 14 6 | 19265277401095105380 | 19 19 10 |
| 1394641595644 | 28 20 0 | 100904990136381816 | 27 15 6 | 1609893249573173544 | 26 11 11 |
| 1859521084850 | 27 21 0 | 170277170927824422 | 26 16 6 | 3488102040361648164 | 25 12 11 |
| 2282140209534 | 26 22 0 | 260423908201122666 | 25 17 6 | 6707888538605476404 | 24 13 11 |
| 2579809646726 | 25 23 0 | 361699872642264870 | 24 18 6 | 11499237494286660144 | 23 14 11 |
| 2687302591938 | 24 24 0 | 456884049357215480 | 23 19 6 | 17632164157359925680 | 22 15 11 |
| 191 | 46 1 1 | 525416656969664460 | 22 20 6 | 24244225715985790086 | 21 16 11 |
| 4349 | 45 2 1 | 550436497519708228 | 21 21 6 | 29948749413533412462 | 20 17 11 |
| 64927 | 44 3 1 | 137943703250100 | 34 7 7 | 33276388237117085060 | 19 18 11 |
| 713757 | 43 4 1 | 586260729113235 | 33 8 7 | 7266879251045544564 | 24 12 12 |
| 6136471 | 42 5 1 | 2149622651528715 | 32 9 7 | 13415777076559133640 | 23 13 12 |
| 42952525 | 41 6 1 | 6878792458628112 | 31 10 7 | 22040205198015185064 | 22 14 12 |
| 251570847 | 40 7 1 | 19385687790199632 | 30 11 7 | 32325634287639864600 | 21 15 12 |
| 1257841915 | 39 8 1 | 48464219421777720 | 29 12 7 | 42427395004047433380 | 20 16 12 |
| 5450620065 | 38 9 1 | 108112489398538680 | 28 13 7 | 49914582355035448050 | 19 17 12 |
| 20712315283 | 37 10 1 | 216224978713510800 | 27 14 7 | 52687614710209402350 | 18 18 12 |
| 69668620785 | 36 11 1 | 389204961579264336 | 26 15 7 | 23735605596070004280 | 22 13 13 |
| 209005757019 | 35 12 1 | 632458062469307646 | 25 16 7 | 37298808793141434264 | 21 14 13 |
| 562707643953 | 34 13 1 | 930085385883855630 | 24 17 7 | 52218332309646863976 | 20 15 13 |
| 1366575493563 | 33 14 1 | 1240113847767543320 | 23 18 7 | 65272915386631793610 | 19 16 13 |
| 3006465804441 | 32 15 1 | 1501190447237652600 | 22 19 7 | 72952081902429966390 | 18 17 13 |
| 6012931267794 | 31 16 1 | 1651309491932965436 | 21 20 7 | 55948213191205903656 | 20 14 14 |
| 10964756631510 | 30 17 1 | 2418325503348480 | 32 8 8 | 74597617584478171920 | 19 15 14 |
| 18274593959490 | 29 18 1 | 8598490570300620 | 31 9 8 | 88584670884029471910 | 18 16 14 |
| 27892800895190 | 28 19 1 | 26655320766887340 | 30 10 8 | 93795533874004088610 | 17 17 14 |
| 39049920855330 | 27 20 1 | 72696329114759460 | 29 11 8 | 94490315606290645536 | 18 15 15 |
| 50207040787046 | 26 21 1 | 175682795387902440 | 28 12 8 | 106301605056802235190 | 17 16 15 |
| 59335593422274 | 25 22 1 | 378393712685968980 | 27 13 8 | 112945455375981823980 | 16 16 16 |
| 64495210129142 | 24 23 1 | 729759303148399260 | 26 14 8 | ||
| 97657 | 44 2 2 | 1264916124783420252 | 25 15 8 | ||
| 1427470 | 43 3 2 | 1976431445242048080 | 24 16 8 | ||
| 15343647 | 42 4 2 | 2790256157185981770 | 23 17 8 | ||
| 128856651 | 41 5 2 | 3565327312427067330 | 22 18 8 | ||
| 880512171 | 40 6 2 | 4128273729405627230 | 21 19 8 | ||
| 5031358420 | 39 7 2 | 4334687416503640196 | 20 20 8 | ||
| 24527846695 | 38 8 2 | 29617022995256180 | 30 9 9 |
| Coefficient | Vector (x, y, z) | Coefficient | Vector (x, y, z) |
|---|---|---|---|
| 1 | 24 0 0 | 4292790 | 16 4 4 |
| 3 | 23 1 0 | 13731492 | 15 5 4 |
| 31 | 22 2 0 | 34329060 | 14 6 4 |
| 181 | 21 3 0 | 68650260 | 13 7 4 |
| 934 | 20 4 0 | 111559140 | 12 8 4 |
| 3597 | 19 5 0 | 148738030 | 11 9 4 |
| 11395 | 18 6 0 | 163616810 | 10 10 4 |
| 29007 | 17 7 0 | 41190876 | 14 5 5 |
| 61698 | 16 8 0 | 96108684 | 13 6 5 |
| 109298 | 15 9 0 | 178483956 | 12 7 5 |
| 164110 | 14 10 0 | 267723414 | 11 8 5 |
| 208474 | 13 11 0 | 327216106 | 10 9 5 |
| 226150 | 12 12 0 | 208237164 | 12 6 6 |
| 49 | 22 1 1 | 356962872 | 11 7 6 |
| 519 | 21 2 1 | 490830354 | 10 8 6 |
| 3573 | 20 3 1 | 545356070 | 9 9 6 |
| 17785 | 19 4 1 | 560936856 | 10 7 7 |
| 67443 | 18 5 1 | 701168970 | 9 8 7 |
| 202149 | 17 6 1 | 788825460 | 8 8 8 |
| [λ] | A1g | A1u |
|---|---|---|
| 120 0 | 1 | 0 |
| 119 1 | 10 | 0 |
| 118 2 | 430 | 200 |
| 117 3 | 13140 | 10270 |
| 116 4 | 358580 | 327000 |
| 115 5 | 8076302 | 7805200 |
| 114 6 | 153228940 | 151186610 |
| 113 7 | 2485281890 | 2472015520 |
| 112 8 | 35049847010 | 34972263360 |
| 111 9 | 435895574270 | 435487149890 |
| 110 10 | 4837163423172 | 4835187982608 |
| 109 11 | 48366143812740 | 48357338386240 |
| 108 12 | 439304123944130 | 439267535249120 |
| 107 13 | 3649522483044260 | 3649380289048000 |
| 106 14 | 27892495987962020 | 27891975424030480 |
| 105 15 | 197106031753154350 | 197104231299671570 |
| 104 16 | 1293505382317706270 | 1293499470458272960 |
| 103 17 | 7913200546609255430 | 7913182073007147040 |
| 102 18 | 45281066731050988200 | 45281011600933676850 |
| 101 19 | 243087762638665331530 | 243087605202533277680 |
| 100 20 | 1227593019502352645116 | 1227592588139680180808 |
| 99 21 | 5845680586016149192080 | 5845679450165058565230 |
| 98 22 | 26305561521626686809770 | 26305558641388642521880 |
| 97 23 | 112084563868390564619870 | 112084556825344707148480 |
| 96 24 | 453008439719843093324185 | 453008423084819935550720 |
| 95 25 | 1739552395580465510782712 | 1739552357586305907062080 |
| 94 26 | 6356056802607579048149000 | 6356056718581715136103840 |
| 93 27 | 22128493997304209628417280 | 22128493817196173268087480 |
| 92 28 | 73498212093520559324283920 | 73498211718937259300046560 |
| 91 29 | 233166741598000966442884040 | 233166740841488289311513600 |
| 90 30 | 707272449109079345066275200 | 707272447624069094082039400 |
| 89 31 | 2053371625707638595138215800 | 2053371622872379689408576320 |
| 88 32 | 5710939832691184420347395710 | 5710939827422078705100951680 |
| 87 33 | 15229172884919521763241151730 | 15229172875382280514199523400 |
| 86 34 | 38968765907621805546447563950 | 38968765890797737924153754120 |
| 85 35 | 95751824795384727236237065030 | 95751824766445595988072443312 |
| 84 36 | 226080697423665918179837712780 | 226080697375100662367959790680 |
| 83 37 | 513264286027563834692713658510 | 513264285948013123131287760080 |
| 82 38 | 1121077256300109181440804823910 | 1121077256172862735028690940680 |
| 81 39 | 2357136795263310515884594697290 | 2357136795064474082599295897160 |
| 80 40 | 4773202010358707257612086114170 | 4773202010055058999556359513408 |
| 79 41 | 9313564898191311432444888679940 | 9313564897737983810337193741600 |
| 78 42 | 17518372070312058850710272631220 | 17518372069650191620702994780120 |
| 77 43 | 31777512127415150509871599803740 | 31777512126469853524471252843840 |
| 76 44 | 55610646222809929010711390106200 | 55610646221488836306052022553040 |
| 75 45 | 93920202509422472913833055244500 | 93920202507615427898786969026968 |
| 74 46 | 153130764960751689179624148240700 | 153130764958331858395428437922800 |
| 73 47 | 241099502278311827209649491672420 | 241099502275138843239010973758880 |
| 72 48 | 366672159714557209135122371215500 | 366672159710482373202785259933120 |
| 71 49 | 538783581620959439539367888026550 | 538783581615833385238761111253600 |
| 70 50 | 765072685901281807653064962794306 | 765072685894964033815883701208344 |
| 69 51 | 1050099764962022784801615163283710 | 1050099764954393034471851047572500 |
| 68 52 | 1393401611199060099344586013233020 | 1393401611190030163150578065198920 |
| 67 53 | 1787760557764276241571586760097890 | 1787760557753801778758977126918480 |
| 66 54 | 2218147358706984761747143844517710 | 2218147358695075055554709343916900 |
| 65 55 | 2661776830447873212340258083935022 | 2661776830434598506818581481178240 |
| 64 56 | 3089562392483687387382849613409250 | 3089562392469181632858390724145920 |
| 63 57 | 3468982335419907262008024739287820 | 3468982335404366657697752965321340 |
| 62 58 | 3768032536748931605487595238564080 | 3768032536732607374058506770897600 |
| 61 59 | 3959627411498706582271887678528880 | 3959627411481893631161976216665600 |
| 60 60 | 4025621201690294701317661934726204 | 4025621201673315584661128579342944 |
| [λ] | A1g | A1u |
|---|---|---|
| 126 0 | 1 | 0 |
| 125 1 | 11 | 0 |
| 124 2 | 473 | 220 |
| 123 3 | 15253 | 11910 |
| 122 4 | 436673 | 398560 |
| 121 5 | 10348033 | 10004800 |
| 120 6 | 206573169 | 203879874 |
| 119 7 | 3527122728 | 3508834432 |
| 118 8 | 52385696704 | 52274232236 |
| 117 9 | 686407979303 | 685795703012 |
| 116 10 | 8028934612915 | 8025849284552 |
| 115 11 | 84659644031796 | 84645309793480 |
| 114 12 | 811283921490655 | 811221894648266 |
| 113 13 | 7114189337715709 | 7113938271431488 |
| 112 14 | 57421136296522190 | 57420179480527816 |
| 111 15 | 428742633734011054 | 428739188570867700 |
| 110 16 | 2974395957907605578 | 2974384184884777864 |
| 109 17 | 19246072541004160475 | 19246034252371767360 |
| 108 18 | 116545605020781110873 | 116545486120454041778 |
| 107 19 | 662469593565669880140 | 662469240221433288120 |
| 106 20 | 3544211884087688220237 | 3544210876693600321224 |
| 105 21 | 17889830252498885495425 | 17889827492074590075716 |
| 104 22 | 85383277805294190143784 | 85383270521181826240644 |
| 103 23 | 386080901136593900270904 | 386080882599144920683392 |
| 102 24 | 1656930517051376784683269 | 1656930471481634923631114 |
| 101 25 | 6760276470775467874719927 | 6760276362433949041317696 |
| 100 26 | 26261073896914647611843439 | 26261073647477690543603952 |
| 99 27 | 97263236471662895274416233 | 97263235914956931417312702 |
| 98 28 | 343895014286357966923112919 | 343895013080626398572379156 |
| 97 29 | 1162127978543417947038420093 | 1162127976007021782564392832 |
| 96 30 | 3757547129116693561369533113 | 3757547123929752463745097544 |
| 95 31 | 11636274977618676741474605088 | 11636274967299175559427516672 |
| 94 32 | 34545191334481858494269819958 | 34545191314493091129392663952 |
| 93 33 | 98401454094672584680155613591 | 98401454056952557263117170000 |
| 92 34 | 269156918536182307562667604533 | 269156918466792562463927930652 |
| 91 35 | 707498185837591596996314883276 | 707498185713084020804419188984 |
| 90 36 | 1788398191930007116460908717629 | 1788398191711979048251416225608 |
| 89 37 | 4350157764075290579543044835397 | 4350157763702510856253493800128 |
| 88 38 | 10188527394682682072242746308316 | 10188527394060064806948032860284 |
| 87 39 | 22989497710884551633808458024272 | 22989497709868307190203644545008 |
| 86 40 | 50002157520879567765636859278504 | 50002157519257903388142469358316 |
| 85 41 | 104882574311653493200042912232931 | 104882574309122653904387904539904 |
| 84 42 | 212262352772955598204815146300663 | 212262352769091372968466328442032 |
| 83 43 | 414652037974188760093034977579044 | 414652037968414603187921841451176 |
| 82 44 | 782184526177360507348845920120703 | 782184526168914032231473122983532 |
| 81 45 | 1425314025477099466617348186776881 | 1425314025465000766616408261522640 |
| 80 46 | 2509792088337944983451863878699918 | 2509792088320970669250957680677753 |
| 79 47 | 4271986533338402240952986091515568 | 4271986533315071146968456514703232 |
| 78 48 | 7030977836115966289850178437041004 | 7030977836084542036709394169335632 |
| 77 49 | 11192168800343594818507662954115382 | 11192168800302112216589382096345792 |
| 76 50 | 17235939952524034981288300451784128 | 17235939952470353920645281657293988 |
| 75 51 | 25684930125323990978539590227007264 | 25684930125255882540509198550606740 |
| 74 52 | 37045572296133623677675596842206368 | 37045572296048886307617780234533304 |
| 73 53 | 51724006602141374421656431169602456 | 51724006602037978956693506504856184 |
| 72 54 | 69923194110294214696088434473985680 | 69923194110170466866523001472260968 |
| 71 55 | 91535817744376799116378300971826720 | 91535817744231511058933713108728176 |
| 70 56 | 116054340354469299983499331537508704 | 116054340354301950607134075303190832 |
| 69 57 | 142522874119515507211110040589336016 | 142522874119326379203129178324865584 |
| 68 58 | 169553074383553918092169927083932224 | 169553074383344188394338933483678944 |
| 67 59 | 195417102679343529229105477101092448 | 195417102679115305059831028491385312 |
| 66 60 | 218215764658594707645979394768009504 | 218215764658350990916595981027726176 |
| 65 61 | 236102302745360626194077729265514496 | 236102302745105211277102095497067808 |
| 64 62 | 247526607716907796799690373591361056 | 247526607716645097621485771818723552 |
| 63 63 | 251455601490191198999846211143625568 | 251455601489926026768523441574344800 |
| TI | AHK(2) | RK(3,3) |
|---|---|---|
| Zagreb-1 | 2520 | 2520 |
| Zagreb-2 | 3366 | 3366 |
| Randić | 177.8786 | 177.8786 |
| Atom-bond connectivity | 322.9188 | 322.9188 |
| Padmakar Ivan | 168,480 | 168,480 |
| Wiener | 1,140,348 | 1,185,720 |
| Hyper-Wiener | 12,876,702 | 14,183,508 |
| Schultz | 589,684 | 6,134,528 |
| Gutman | 7,622,832 | 7,934,112 |
| Harary | 5635.002 | 5565.1216 |
| Balaban | 0.3237 | 0.3120 |
| Mostar | 80,256 | 79,920 |
| Szeged | 10,338,816 | 10,325,272 |
| Harmonic Szeged | 66,834.8034 | 67,043.7870 |
| Edge Partitions | (22)18(23)252(33)198 | (22)18(23)252(33)198 |
| Vertex Partitions | 641228 | 24488 |
| Spectral Pattern | 1 2 2 1 1 1 2 2 2 1 2 1 2 1 2 1 2 1 1 2 | 112421154.154211124 |
| 2 2 1 1 1 2 2 1 1 1 2 2 1 2 1 2 1 2 2 1 | ||
| 1 2 2 1 1 2 2 1 1 2 1 2 2 1 2 1 2 2 1 1 | ||
| 2 2 1 1 2 2 1 1 2 2 1 2 1 2 1 1 1 1 2 2 | ||
| 2 2 2 1 1 2 2 1 1 2 2 1 2 1 1 2 1 2 2 1 | ||
| 2 2 1 2 1 1 1 2 2 1 2 2 1 2 1 1 2 1 2 | ||
| 2 1 2 1 1 2 1 2 2 1 2 2 1 1 1 2 1 2 2 1 | ||
| 2 2 1 2 1 1 2 1 2 2 1 1 2 2 1 1 2 2 2 2 | ||
| 2 1 1 1 1 2 1 2 1 2 2 1 1 2 2 1 1 2 2 1 | ||
| 1 2 2 1 2 1 2 2 1 2 1 1 2 2 1 1 2 2 1 1 | ||
| 2 2 1 2 1 2 1 2 2 1 1 1 2 2 1 1 1 2 2 2 | ||
| 1 1 2 1 2 1 2 1 2 1 2 2 2 1 1 1 2 2 1 | ||
| Spectral Difference | 0.009612 | |
| RMS Spectral Difference | 0.013128 | |
| HOMO-LUMO | 0.755β a | 0.744β |
| Eπ | 524.4053β | 524.4139β |
| 13C-NMR | 32 signals | 92 signals |
| Proton NMR | 14 signals 1:1:1:1:2:2:2:2:2:2:2:2:2:2 | 38 signals 1:1:1:1:2:2:2:2:2:2:2:2:2:2: 2:2:2:2:2:2:2:2:2:2: 2:2:2:2:2:2:2:2:2:2: |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Balasubramanian, K. Symmetry and Combinatorial Concepts for Cyclopolyarenes, Nanotubes and 2D-Sheets: Enumerations, Isomers, Structures Spectra & Properties. Symmetry 2022, 14, 34. https://doi.org/10.3390/sym14010034
Balasubramanian K. Symmetry and Combinatorial Concepts for Cyclopolyarenes, Nanotubes and 2D-Sheets: Enumerations, Isomers, Structures Spectra & Properties. Symmetry. 2022; 14(1):34. https://doi.org/10.3390/sym14010034
Chicago/Turabian StyleBalasubramanian, Krishnan. 2022. "Symmetry and Combinatorial Concepts for Cyclopolyarenes, Nanotubes and 2D-Sheets: Enumerations, Isomers, Structures Spectra & Properties" Symmetry 14, no. 1: 34. https://doi.org/10.3390/sym14010034
APA StyleBalasubramanian, K. (2022). Symmetry and Combinatorial Concepts for Cyclopolyarenes, Nanotubes and 2D-Sheets: Enumerations, Isomers, Structures Spectra & Properties. Symmetry, 14(1), 34. https://doi.org/10.3390/sym14010034





