Performance Analysis of Continuous Variable Quantum Teleportation with Noiseless Linear Amplifier in Seawater Channel
Abstract
:1. Introduction
2. The NLA-Based CVQT under Seawater
2.1. The NLA-Based CVQT Protocol
2.2. Optical Propagation Characteristics of Seawater Channel
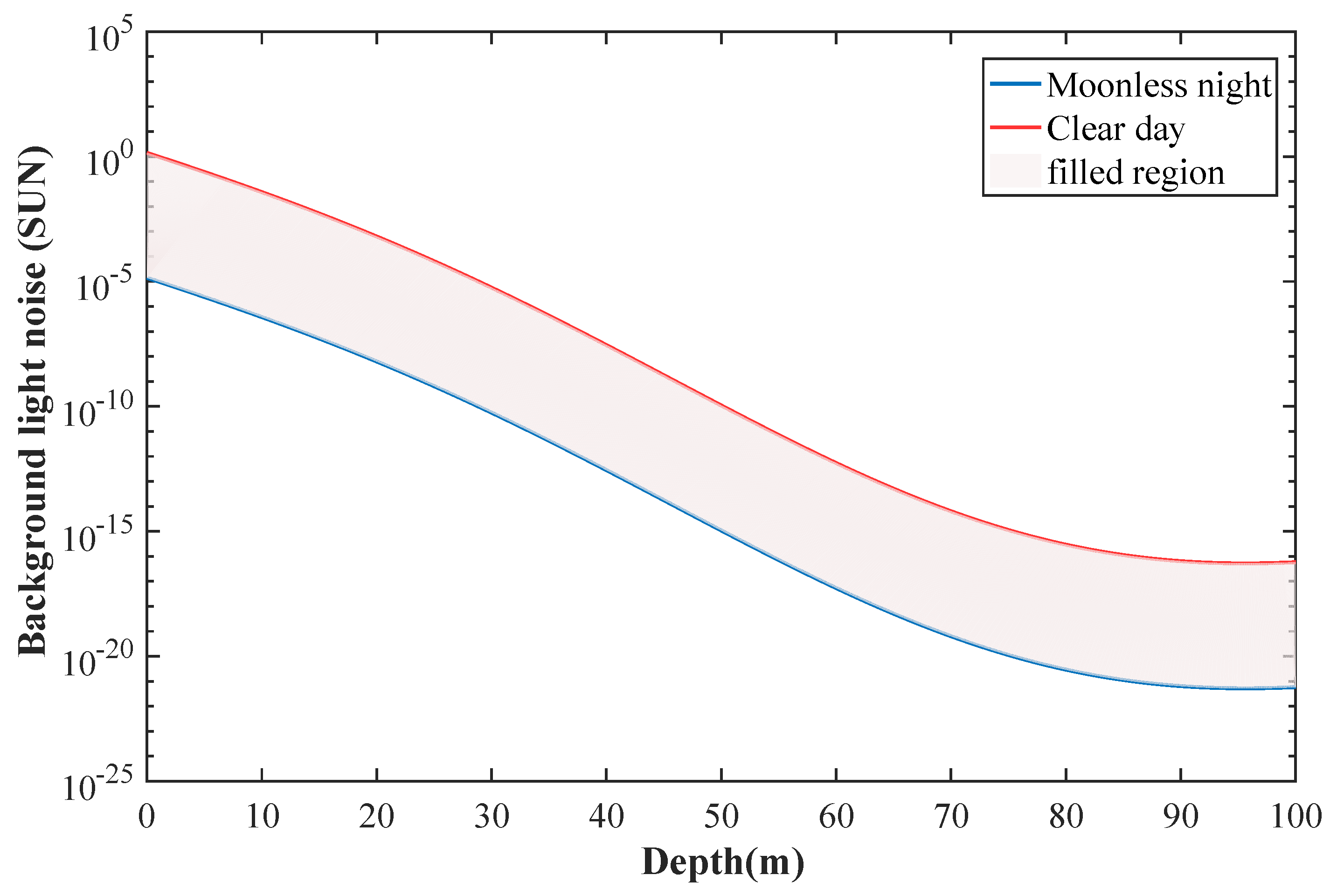
3. Effect of Excess Noise
4. Performance Analysis
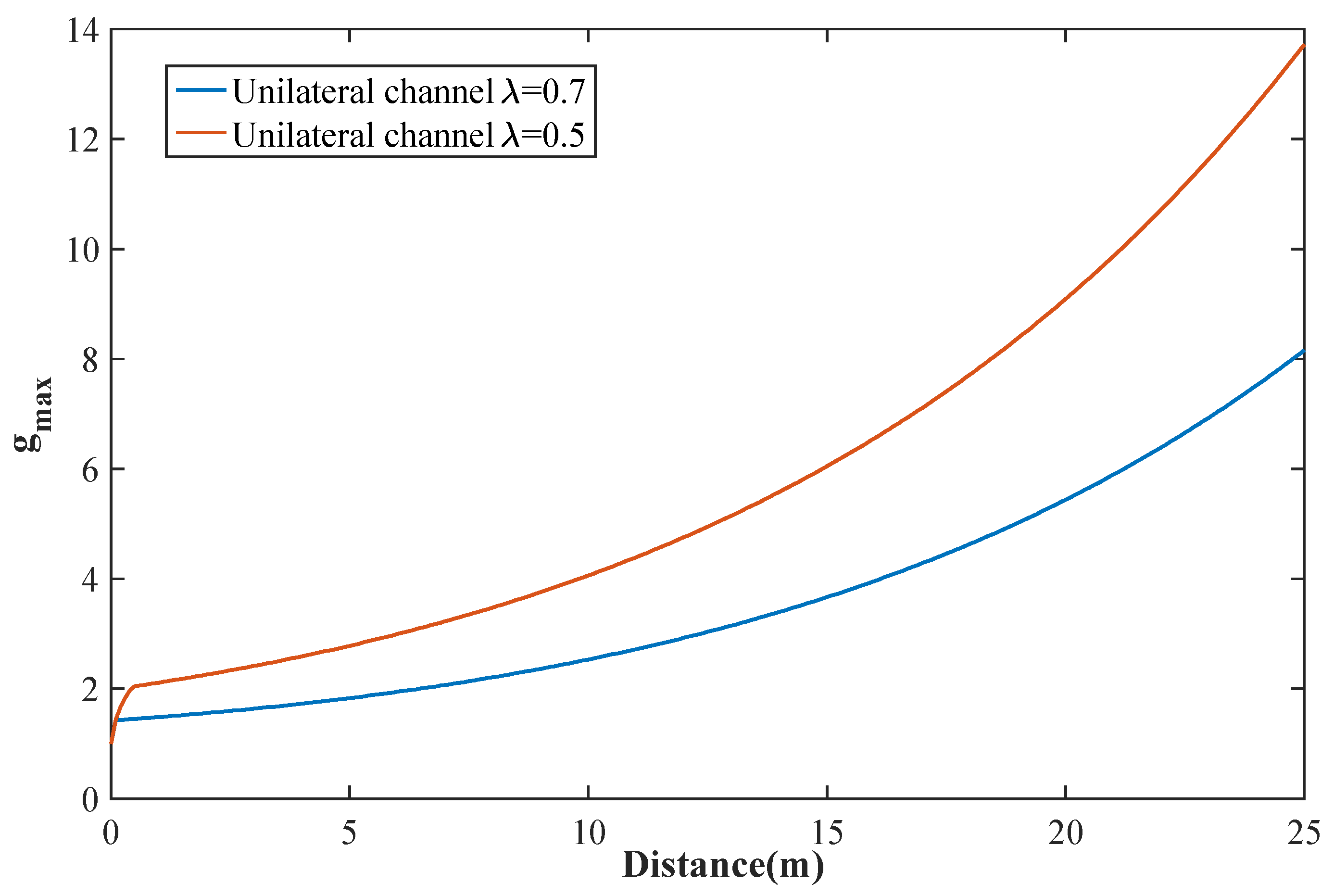
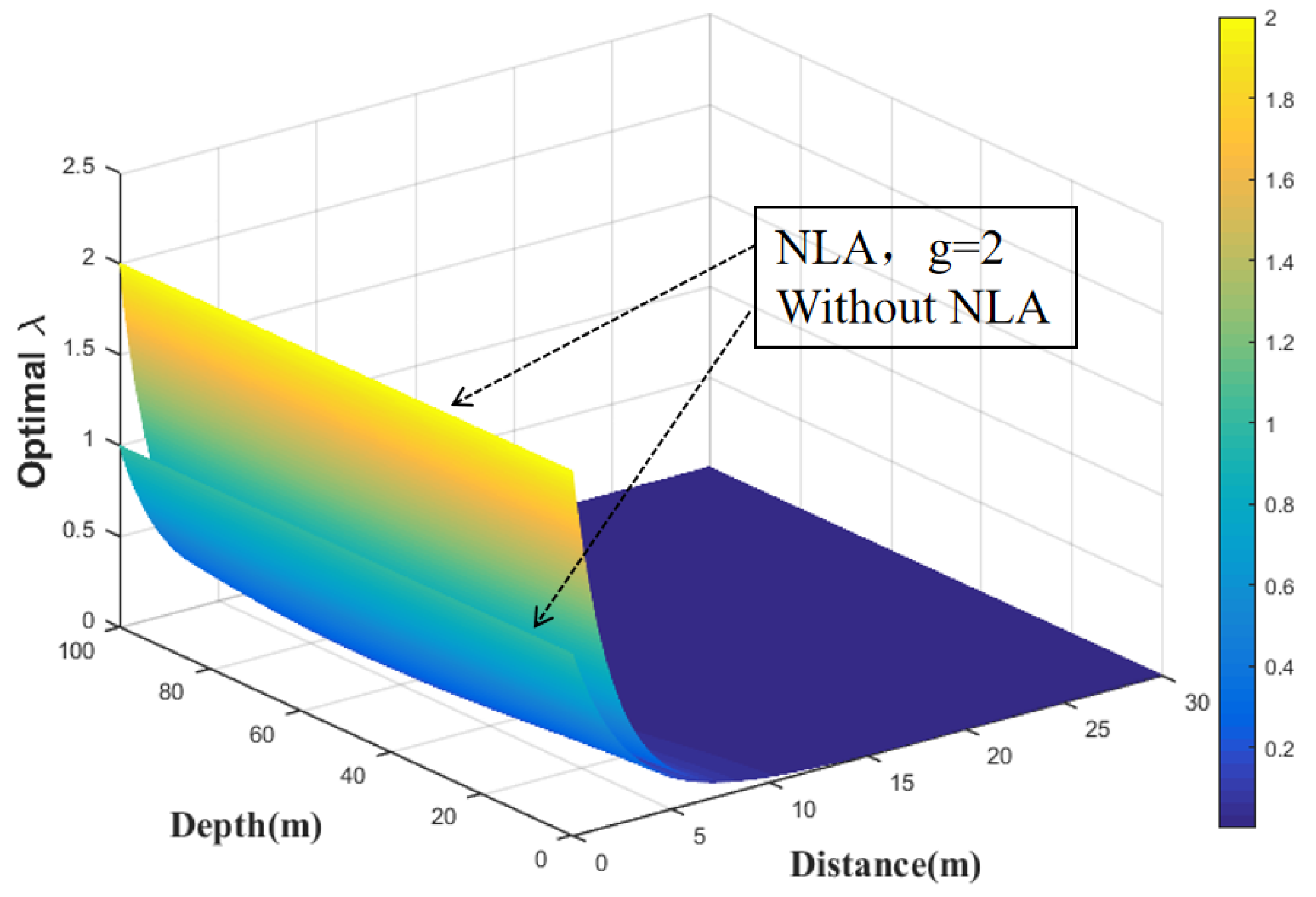
4.1. The Gain of NLA under Seawater Channel
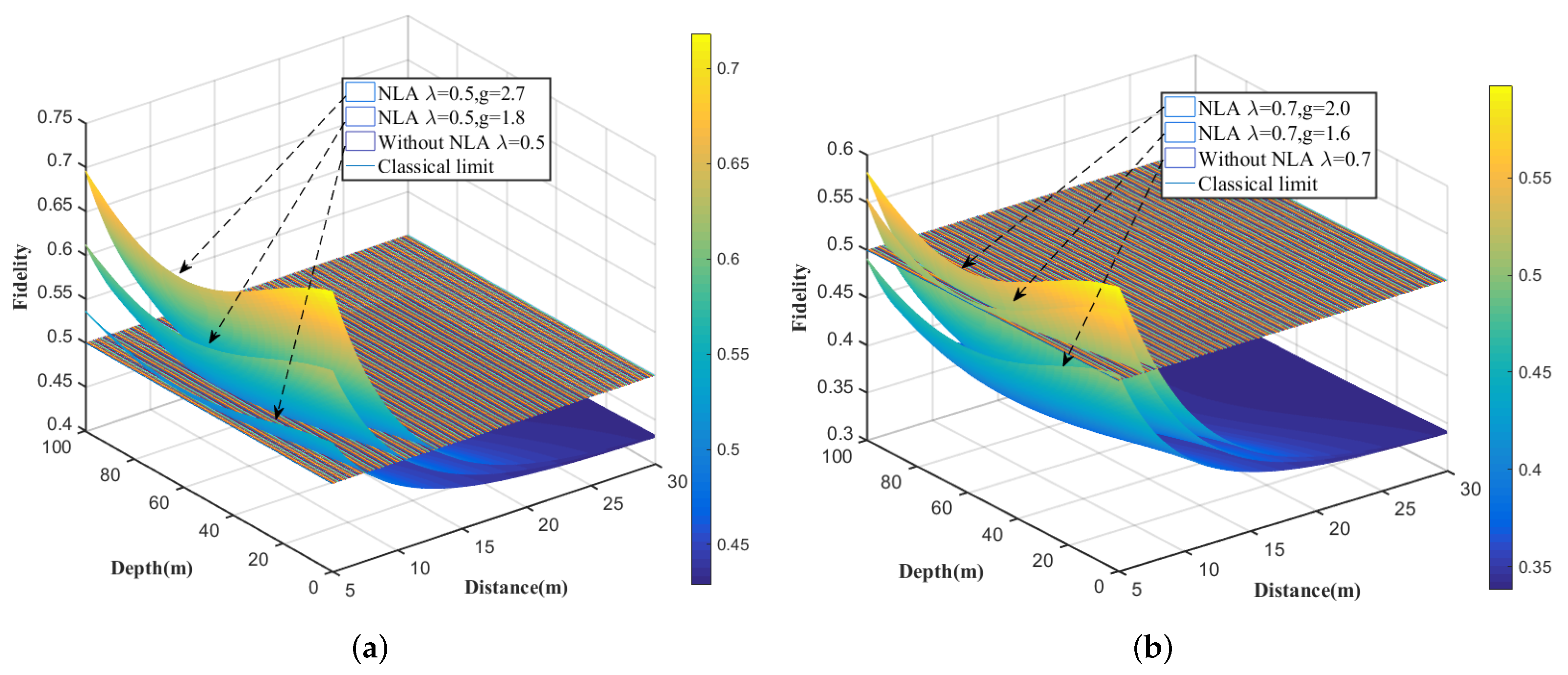
4.2. Performance Improvement under Seawater Lossy Channel
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. The Absorption Coefficient of the Seawater Channel
| Parameters | Value | Description |
|---|---|---|
| Fulvic acid spectral absorption coefficient | ||
| Fulvic acid absorption slope coefficient | ||
| Humic acid spectral absorption coefficient | ||
| Humic acid absorption slope coefficient | ||
| Mineral and detritus spectral absorption coefficient | ||
| Mineral and detritus absorption slope coefficient |
Appendix B. The Scattering Coefficient of the Seawater Channel
| Function | Formula |
|---|---|
References
- Bennett, C.H.; Brassard, G.; Crépeau, C.; Jozsa, R.; Peres, A.; Wootters, W.K. Teleporting an unknown quantum state via dual classical and Einstein-Podolsky-Rosen channels. Phys. Rev. Lett. 1993, 70, 1895–1899. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bouwmeester, D.; Pan, J.W.; Mattle, K.; Eibl, M.; Weinfurter, H.; Zeilinger, A. Experimental quantum teleportation. Nature 1997, 390, 575–579. [Google Scholar] [CrossRef] [Green Version]
- Hu, X.M.; Zhang, C.; Liu, B.H.; Cai, Y.; Ye, X.J.; Guo, Y.; Xing, W.B.; Huang, C.X.; Huang, Y.F.; Li, C.F.; et al. Experimental high-dimensional quantum teleportation. Phys. Rev. Lett. 2020, 125, 230501. [Google Scholar] [CrossRef] [PubMed]
- Marcikic, I.; De Riedmatten, H.; Tittel, W.; Zbinden, H.; Gisin, N. Long-distance teleportation of qubits at telecommunication wavelengths. Nature 2003, 421, 509–513. [Google Scholar] [CrossRef] [PubMed]
- Yin, J.; Ren, J.G.; Lu, H.; Cao, Y.; Yong, H.L.; Wu, Y.P.; Liu, C.; Liao, S.K.; Zhou, F.; Jiang, Y.; et al. Quantum teleportation and entanglement distribution over 100-kilometre free-space channels. Nature 2012, 488, 185–188. [Google Scholar] [CrossRef] [PubMed]
- Ren, J.G.; Xu, P.; Yong, H.L.; Zhang, L.; Liao, S.K.; Yin, J.; Liu, W.Y.; Cai, W.Q.; Yang, M.; Pan, J.W.; et al. Ground-to-satellite quantum teleportation. Nature 2017, 549, 70–73. [Google Scholar] [CrossRef] [Green Version]
- Braunstein, S.L.; Kimble, H.J. Teleportation of continuous quantum variables. Phys. Rev. Lett. 1998, 80, 869. [Google Scholar] [CrossRef] [Green Version]
- Lee, N.; Benichi, H.; Takeno, Y.; Takeda, S.; Webb, J.; Huntington, E.; Furusawa, A. Teleportation of nonclassical wave packets of light. Science 2011, 332, 330–333. [Google Scholar] [CrossRef] [Green Version]
- Huo, M.R.; Qin, J.L.; Cheng, J.L.; Yan, Z.H.; Qin, Z.Z.; Su, X.L.; Jia, X.J.; Xie, C.D.; Peng, K.C. Deterministic quantum teleportation through fiber channels. Sci. Adv. 2018, 4, eaas9401. [Google Scholar] [CrossRef] [Green Version]
- Rudolph, T.; Sanders, B.C. Requirement of optical coherence for continuous-variable quantum teleportation. Phys. Rev. Lett. 2001, 87, 077903. [Google Scholar] [CrossRef] [Green Version]
- Zhao, H.; Feng, J.X.; Sun, J.K.; Li, Y.J.; Zhang, K.S. Real time deterministic quantum teleportation over 10 km of single optical fiber channel. Opt. Express 2022, 30, 3770–3782. [Google Scholar] [CrossRef] [PubMed]
- Jin, X.M.; Ren, J.G.; Yang, B.; Yi, Z.H.; Zhou, F.; Xu, X.F.; Wang, S.K.; Yang, D.; Hu, Y.F.; Jiang, S.; et al. Experimental free-space quantum teleportation. Nat. Photon. 2010, 4, 376–381. [Google Scholar] [CrossRef]
- Zuo, Z.Y.; Wang, Y.J.; Liao, Q.; Guo, Y. Overcoming the uplink limit of satellite-based quantum communication with deterministic quantum teleportation. Phys. Rev. A 2021, 104, 022615. [Google Scholar] [CrossRef]
- Ma, X.S.; Kropatschek, S.; Naylor, W.; Scheidl, T.; Kofler, J.; Herbst, T.; Zeilinger, A.; Ursin, R. Experimental quantum teleportation over a high-loss free-space channel. Opt. Express 2012, 20, 23126–23137. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Q.; Goebel, A.; Wagenknecht, C.; Chen, Y.A.; Zhao, B.; Yang, T.; Mair, A.; Schmiedmayer, J.; Pan, J.W. Experimental quantum teleportation of a two-qubit composite system. Nat. Phys. 2006, 2, 678–682. [Google Scholar] [CrossRef] [Green Version]
- Barasinski, A.; Cernoch, A.; Lemr, K. Demonstration of controlled quantum teleportation for discrete variables on linear optical devices. Pys. Rev. Lett. 2019, 122, 170501. [Google Scholar] [CrossRef]
- Rajiuddin, S.; Baishya, A.; Behera, B.K.; Panigrahi, P.K. Experimental realization of quantum teleportation of an arbitrary two-qubit state using a four-qubit cluster state. Quantum Inf. Process. 2020, 19, 87. [Google Scholar] [CrossRef]
- Chatterjee, Y.; Devrari, V.; Behera, B.K.; Panigrahi, P.K. Experimental realization of quantum teleportation using coined quantum walks. Quantum Inf. Process. 2020, 19, 31. [Google Scholar] [CrossRef] [Green Version]
- Simon, C. Towards a global quantum network. Nat. Photon. 2017, 11, 678–680. [Google Scholar] [CrossRef] [Green Version]
- Liao, Q.; Haijie, L.; Lingjin, Z.; Guo, Y. Quantum secret sharing using discretely modulated coherent states. Phys. Rev. A 2021, 103, 032410. [Google Scholar] [CrossRef]
- Ji, L.; Gao, J.; Yang, A.L.; Feng, Z.; Lin, X.F.; Li, Z.G.; Jin, X.M. Towards quantum communications in free-space seawater. Opt. Express 2017, 25, 19795–19806. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hu, C.Q.; Yan, Z.Q.; Gao, J.; Jiao, Z.Q.; Li, Z.M.; Shen, W.G.; Chen, Y.; Ren, R.J.; Qiao, L.F.; Yang, A.L.; et al. Transmission of photonic polarization states through 55 m water: Towards air-to-sea quantum communication. Photon. Res. 2019, 7, A40–A44. [Google Scholar] [CrossRef]
- Haltrin, V.I. Chlorophyll-based model of seawater optical properties. Appl. Opt. 1999, 38, 6826–6832. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gilerson, A.; Zhou, J.; Hlaing, S.; Ioannou, I.; Schalles, J.; Gross, B.; Moshary, F.; Ahmed, S. Fluorescence component in the reflectance spectra from coastal waters. Dependence on water composition. Opt. Express 2007, 15, 15702. [Google Scholar] [CrossRef]
- Gariano, J.; Djordjevic, I.B. Theoretical study of a submarine to submarine quantum key distribution systems. Opt. Express 2019, 27, 3055. [Google Scholar] [CrossRef]
- Guo, Y.; Xie, C.L.; Huang, P.; Li, J.W.; Zhang, L.; Huang, D.; Zeng, G.H. Channel-parameter estimation for satellite-to-submarine continuous-variable quantum key distribution. Phys. Rev. A 2018, 97, 052326. [Google Scholar] [CrossRef]
- Liao, Q.; Xiao, G.; Xu, C.; Xu, Y.; Guo, Y. Discretely modulated continuous-variable quantum key distribution with untrusted entanglement source. Phys. Rev. A 2020, 102, 032604. [Google Scholar] [CrossRef]
- Braunstein, S.L.; Fuchs, C.A.; Kimble, H.J. Criteria for continuous-variable quantum teleportation. J. Mod. Opt. 2000, 47, 267–278. [Google Scholar] [CrossRef]
- Weedbrook, C.; Pirandola, S.; Garcia-Patron, R.; Cerf, N.J.; Ralph, T.C.; Shapiro, J.H.; Lloyd, S. Gaussian quantum information. Rev. Mod. Phys. 2012, 84, 621–669. [Google Scholar] [CrossRef]
- Chizhov, A.V.; Knoll, L.; Welsch, D.G. Continuous-variable quantum teleportation through lossy channels. Rev. Mod. Phys. 2002, 65, 022310. [Google Scholar] [CrossRef] [Green Version]
- Feng, Z.; Li, S.B.; Xu, Z.Y. Experimental underwater quantum key distribution. Opt. Express 2021, 29, 8725–8736. [Google Scholar] [CrossRef] [PubMed]
- Prieur, L.; Sathyendranath, S. An optical classification of coastal and oceanic waters based on the specific spectral absorption curves of phytoplankton pigments, dissolved organic matter, and other particulate materials. J. Geophys. Res. 1981, 26, 671. [Google Scholar] [CrossRef]
- Uitz, J.; Claustre, H.; Morel, A.; Hooker, S.B. Vertical distribution of phytoplankton communities in open ocean: An assessment based on surface chlorophyll. J. Geophys. Res.-Oceans 2006, 111, C08005. [Google Scholar] [CrossRef]
- Ruan, X.C.; Zhang, H.; Zhao, W.; Wang, X.X.; Li, X.; Guo, Y. Security analysis of discrete modulated continuous-variable quantum key distribution over seawater channel. Appl. Sci. 2019, 9, 4956. [Google Scholar] [CrossRef] [Green Version]
- Huang, D.; Huang, P.; Lin, D.K.; Zeng, G.H. Long-distance continuous-variable quantum key distribution by controlling excess noise. Sci. Rep. 2016, 6, 19201. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jaruwatanadilok, S. Underwater wireless optical communication channel modeling and performance evaluation using vector radiative transfer theory. IEEE J. Sel. Areas Commun. 2008, 26, 1620–1627. [Google Scholar] [CrossRef]
- Miao, E.L.; Han, Z.F.; Gong, S.S.; Zhang, T.; Diao, D.S.; Guo, G.C. Background noise of satellite-to-ground quantum key distribution. New J. Phys. 2005, 7, 215. [Google Scholar]
- Blandino, R.; Leverrier, A.; Barbieri, M.; Etesse, J.; Grangier, P.; Tualle-Brouri, R. Improving the maximum transmission distance of continuous-variable quantum key distribution using a noiseless amplifier. Phys. Rev. A 2012, 86, 012327. [Google Scholar] [CrossRef] [Green Version]
- Xu, B.J.; Tang, C.M.; Chen, H.; Zhang, W.Z.; Zhu, F.C. Improving the maximum transmission distance of four-state continuous-variable quantum key distribution by using a noiseless linear amplifier. Phys. Rev. A 2013, 87, 062311. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.C.; Li, Z.Y.; Weedbrook, C.; Marshall, K.; Pirandola, S.; Yu, S.; Guo, H. Noiseless linear amplifiers in entanglement-based continuous-variable quantum key distribution. Entropy 2015, 17, 4547–4562. [Google Scholar] [CrossRef] [Green Version]
- Hofmann, K.; Semenov, A.A.; Vogel, W.; Bohmann, M. Quantum teleportation through atmospheric channels. Phys. Scr. 2019, 94, 125104. [Google Scholar] [CrossRef] [Green Version]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, H.; Liu, X.; Zhang, H.; Ruan, X.; Guo, Y. Performance Analysis of Continuous Variable Quantum Teleportation with Noiseless Linear Amplifier in Seawater Channel. Symmetry 2022, 14, 997. https://doi.org/10.3390/sym14050997
Wu H, Liu X, Zhang H, Ruan X, Guo Y. Performance Analysis of Continuous Variable Quantum Teleportation with Noiseless Linear Amplifier in Seawater Channel. Symmetry. 2022; 14(5):997. https://doi.org/10.3390/sym14050997
Chicago/Turabian StyleWu, Hao, Xu Liu, Hang Zhang, Xinchao Ruan, and Ying Guo. 2022. "Performance Analysis of Continuous Variable Quantum Teleportation with Noiseless Linear Amplifier in Seawater Channel" Symmetry 14, no. 5: 997. https://doi.org/10.3390/sym14050997
APA StyleWu, H., Liu, X., Zhang, H., Ruan, X., & Guo, Y. (2022). Performance Analysis of Continuous Variable Quantum Teleportation with Noiseless Linear Amplifier in Seawater Channel. Symmetry, 14(5), 997. https://doi.org/10.3390/sym14050997






