Symmetric Difference Operator in Quantum Calculus
Abstract
:1. Introduction
2. Preliminaries of the q-Difference Operator
3. Preliminaries of the h-Difference Operator
4. Fundamental Theorems in Quantum Calculus
4.1. Fundamental Theorems Related to the q-Difference Symmetric Operator
4.2. Fundamental Theorems Related to -Difference Symmetric Operators
5. Mixed Symmetric Operators in Quantum Calculus
5.1. Symmetric Difference Operators of q and h
5.2. Symmetric Difference Operators of and h
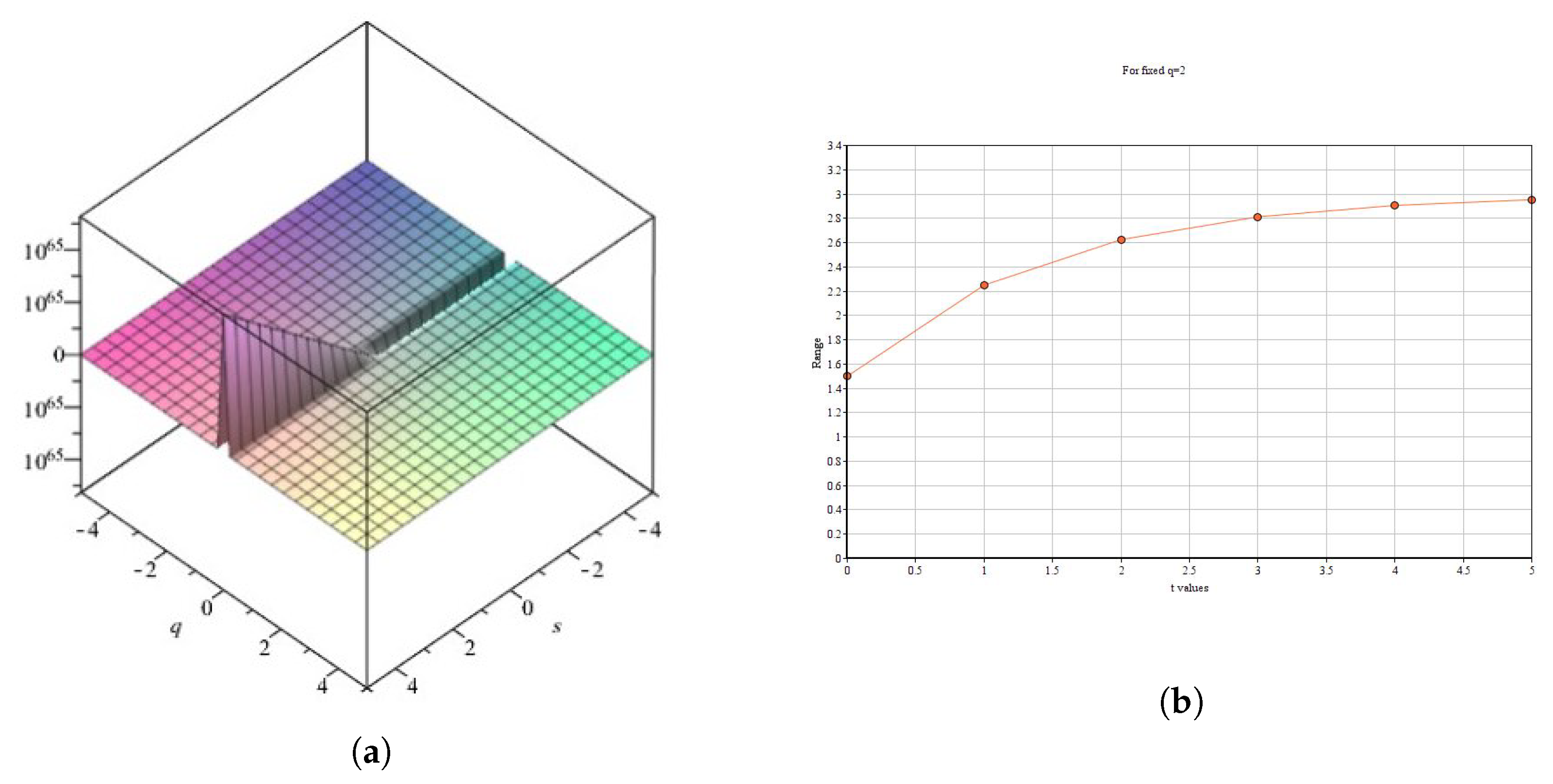
5.3. Value Stability Analysis
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Akca, H.; Benbourenane, J.; Eleuch, H. The q-derivative and differential equation. J. Phys. Conf. Ser. 2019, 1411, 012002. [Google Scholar] [CrossRef] [Green Version]
- Adams, C.R. On the Linear Ordinary q-Difference Equation. Ann. Math. 1928, 30. [Google Scholar] [CrossRef]
- Carmichael, R.D. The General Theory of Linear q-Difference Equations. Am. J. Math. 1912, 34, 147. [Google Scholar] [CrossRef]
- Jackson, F.H. q-Difference equations. Am. J. Math. 1910, 32, 305–314. [Google Scholar] [CrossRef]
- Mason, T.E. On Properties of the Solutions of Linear q-Difference Equations with Entire Function Coefficients. Am. J. Math. 1915, 37, 439. [Google Scholar] [CrossRef]
- Trjitzinsky, W.J. Analytic Theory of Linear q-Difference Equations. Acta Math. 1933, 61, 1–38. [Google Scholar] [CrossRef]
- Hahn, W. Geometric difference equations. Germanic, 1980; not published. [Google Scholar]
- Artur, M.C.; da Cruz, B. Symmetric Quantum Calculus; Department of Mathematics at the University of Aveiro (Departamento de Matemática da Universidade de Aveiro): Aveiro, Portugal, 2012. [Google Scholar]
- Liouville, J. Mémoire sur quelques questions de géométrie et de mécanique, et sur un nouveau genre de calcul pour résoudre ces questions. J. l’École Polytech. 1832, 13, 1–69. [Google Scholar]
- Michele, C. Linear Models of Dissipation whose Q is almost Frequency Independent—II. Geophys. J. Int. 1967, 13, 529–539. [Google Scholar] [CrossRef]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; John Wiley and Sons, Inc.: New York, NY, USA, 1993. [Google Scholar]
- Atanackovic, T.M.; Dolicanin, C.; Kacapor, E. Internal Variable Theory in Viscoelasticity: Fractional Generalizations and Thermodynamical Restrictions. Mathematics 2022, 10, 1708. [Google Scholar] [CrossRef]
- Luchko, Y. Fractional Derivatives and the Fundamental Theorem of Fractional Calculus. Fract. Calc. Appl. Anal. 2020, 23, 939–966. [Google Scholar] [CrossRef]
- Ortigueira, M.D. A New Look at the Initial Condition Problem. Mathematics 2022, 10, 1771. [Google Scholar] [CrossRef]
- Tarasov, V.E. Fractional Dynamics with Depreciation and Obsolescence: Equations with Prabhakar Fractional Derivatives. Mathematics 2022, 10, 1540. [Google Scholar] [CrossRef]
- Luchko, Y. General Fractional Integrals and Derivatives with the Sonine Kernels. Mathematics 2021, 9, 594. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Hilfer, R.; Luchko, Y. Desiderata for Fractional Derivatives and Integrals. Mathematics 2019, 7, 149. [Google Scholar] [CrossRef] [Green Version]
- Yamamoto, M. Fractional Calculus and Time-Fractional Differential Equations: Revisit and Construction of a Theory. Mathematics 2022, 10, 698. [Google Scholar] [CrossRef]
- Miller, K.S.; Ross, B. Fractional Difference Calculus, in Univalent Functions, Fractional Calculus, and the Applications (Koriyama, 1988); Horwood: Chichester, UK, 1989; pp. 139–152. [Google Scholar]
- Agarwal, R.P. Certain Fractional q-Integrals and q-Derivatives. Math. Proc. Camb. Philos. Soc. 1969, 66, 365–370. [Google Scholar] [CrossRef]
- Al-Salam, W.A. Some Fractional q-Integrals and q-Derivatives. Proc. Edinb. Math. Soc. 1966, 15, 135–140. [Google Scholar] [CrossRef] [Green Version]
- Al-Salam, W.A.; Verma, A. A fractional Leibniz q-formula. Pac. J. Math. 1975, 60, 1–9. [Google Scholar]
- Abdi, W.H. On certain q-difference equations and q-Laplace transform. Proc. Nat. Inst. Sci. India Part A 1962, 28, 1–15. [Google Scholar]
- Abdi, W.H. On q-Laplace transform. Proc. Nat. Acad. Sci. India 1961, 29, 389–408. [Google Scholar]
- Hahn, W. Beitrage Zur Theorie Der Heineschen Reihen. Die 24 Integrale Der Hypergeometri schen q-Differenzengleichung. Das q-Analogon Der Laplace-Transformation. Math. Nachrichten 1949, 2, 340–379. [Google Scholar] [CrossRef]
- Jackson, F.H. A generalization of the functions Γ(n) and xn. Proc. Roy. Soc. Lond. 1904, 74, 64–72. [Google Scholar] [CrossRef] [Green Version]
- Jackson, F.H. A q-form of Taylors theorem. Mess. Math 1909, 38, 62–64. [Google Scholar]
- Jackson, F.H. On q-definite integrals. Quart. J. Pure Appl. Math. 1910, 41, 193–203. [Google Scholar]
- Jackson, F.H. Transformations of q-series. Mess. Math. 1910, 39, 193–203. [Google Scholar]
- De Sole, A.; Kac, V. On integral representations of q-gamma and q-beta functions. Rend. Mat. Acc. Lincei 2005, 16, 11–29. [Google Scholar]
- McAnally, D.S. q-Exponential and q-Gamma Functions. II. q-Gamma Functionsa. J. Math. Phys. 1995, 36, 574–595. [Google Scholar] [CrossRef] [Green Version]
- McAnally, D.S. q-Exponential and q-Gamma Functions. I. q-Exponential Functions. J. Math. Phys. 1995, 36, 546–573. [Google Scholar] [CrossRef]
- Ernst, T. The History of q-Calculus and a New Method. Licentiate Thesis, Uppsala University, Uppsala, Sweden, 2000. [Google Scholar]
- Koornwinder, T.H. Compact quantum groups and q-special functions. In Representation of Lie Groups and Quantum Groups; Baldona, V., Picardello, M.A., Eds.; PitmanResearch Notes in Mathematics Series 311; Longman Scientific and Technical: London, UK, 1994; pp. 46–128. [Google Scholar]
- Koornwinder, T.H. q-special functions, a tutorial. arXiv 2013, arXiv:math/9403216. [Google Scholar]
- Andrews, G.E.; Askey, R. Special Functions; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Carlson, B.C. Special Functions of Applied Mathematics; Academic Press: New York, NY, USA, 1977. [Google Scholar]
- Agarwal, R.P. Difference Equations and Inequalities; Marcel Dekker: New York, NY, USA, 2000. [Google Scholar]
- Jackson, F.H. XI.—On q-Functions and a Certain Difference Operator. Trans. R. Soc. Edinb. 1909, 46, 253–281. [Google Scholar] [CrossRef]
- Popenda, J.; Szmanda, B. On the Oscillation of Solutions of certain Difference Equation. Demonstr. Math. 1984, 17, 153–164. [Google Scholar] [CrossRef]
- Maria Susai Manuel, M.; Chandrasekar, V. Solutions and Applications of Certain Class of α-Difference Equations. Int. J. Appl. Math. 2011, 24, 943–954. [Google Scholar]
- Rexma Sherine, V.; Gerly, T.G.; Britto Antony Xavier, G. Infinite Series of Fractional order of Fibonacci Delta Operator and its Sum. Adv. Math. Sci. J. 2020, 9, 5891–5900. [Google Scholar] [CrossRef]
- Britto Antony Xavier, G.; Gerly, T.G.; Begum, N.H. Finite series of polynomials and polynomial factorials arising from generalized q-difference operator. Far East J. Math. Sci. (FJMS) 2014, 94, 47–63. [Google Scholar]
- Soundarya; Gerly, T.G.; Rexma Sherine, V. Oscillation Theory of q-Difference Equation. J. Comput. Math. 2021, 5, 83–91. [Google Scholar] [CrossRef]
- Britto Antony Xavier, G.; Gerly, T.G.; Vasanth Kumar, S.U. Multi-series solution of generalized q-alpha difference equation. Int. J. Appl. Eng. Res. 2015, 10, 97–101. [Google Scholar]
- Artur, M.C.; da Cruz, B.; Martins, N. The q-Symmetric Variational Calculus. Comput. Math. Appl. 2012, 64, 2241–2250. [Google Scholar] [CrossRef] [Green Version]
- Goodrich, C.; Peterson, A.C. Discrete Fractional Calculus; Springer International Publishing: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Kac, V.; Cheung, P. Quantum Calculus; Springer: New York, NY, USA, 2002. [Google Scholar]
- Maria Susai Manuel, M.; Britto Antony Xavier, G.; Thandapani, E. Theory of Generalized Difference Operator and Its Applications. Far East J. Math. Sci. 2006, 20, 163–171. [Google Scholar]


| ∃ | there exists |
| ∋ | such that |
| q-difference operator | |
| q-alpha difference operator | |
| Inverse q-difference operator | |
| Inverse of q-alpha difference operator | |
| h-difference operator | |
| Inverse of h-difference operator | |
| Inverse of h-difference operator with respect to the variable t |
| Values | Values | ||
|---|---|---|---|
| 2.81 (by Example 7) | 12.19 | ||
| 2.44 | 18.19 | ||
| 1.97 | 19.78 (by Example 8) | ||
| 1.5 | 18.19 | ||
| 0.93 | 15.04 | ||
| 0.76 | 11.54 | ||
| 0.52 | 8.39 | ||
| 0.34 | 5.85 | ||
| 0.22 | 3.94 | ||
| 0.14 | 2.58 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, W.; Rexma Sherine, V.; Gerly, T.G.; Britto Antony Xavier, G.; Julietraja, K.; Chellamani, P. Symmetric Difference Operator in Quantum Calculus. Symmetry 2022, 14, 1317. https://doi.org/10.3390/sym14071317
Zhao W, Rexma Sherine V, Gerly TG, Britto Antony Xavier G, Julietraja K, Chellamani P. Symmetric Difference Operator in Quantum Calculus. Symmetry. 2022; 14(7):1317. https://doi.org/10.3390/sym14071317
Chicago/Turabian StyleZhao, Weidong, V. Rexma Sherine, T. G. Gerly, G. Britto Antony Xavier, K. Julietraja, and P. Chellamani. 2022. "Symmetric Difference Operator in Quantum Calculus" Symmetry 14, no. 7: 1317. https://doi.org/10.3390/sym14071317
APA StyleZhao, W., Rexma Sherine, V., Gerly, T. G., Britto Antony Xavier, G., Julietraja, K., & Chellamani, P. (2022). Symmetric Difference Operator in Quantum Calculus. Symmetry, 14(7), 1317. https://doi.org/10.3390/sym14071317






