Laplace Residual Power Series Method for Solving Three-Dimensional Fractional Helmholtz Equations

Abstract

In the present study, the exact solutions of the fractional three-dimensional (3D) Helmholtz equation (FHE) are obtained using the Laplace residual power series method (LRPSM). The fractional derivative is calculated using the Caputo operator. First, we introduce a novel method that combines the Laplace transform tool and the residual power series approach. We specifically give the specifics of how to apply the suggested approach to solve time-fractional nonlinear equations. Second, we use the FHE to evaluate the method’s efficacy and validity. Using 2D and 3D plots of the solutions, the derived and precise solutions are compared, confirming the suggested method’s improved accuracy. The results for nonfractional approximate and accurate solutions, as well as fractional approximation solutions for various fractional orders, are indicated in the tables. The relationship between the derived solutions and the actual solutions to each problem is examined, showing that the solution converges to the actual solution as the number of terms in the series solution of the problems increases. Two examples are shown to demonstrate the effectiveness of the suggested approach in solving various categories of fractional partial differential equations. It is evident from the estimated values that the procedure is precise and simple and that it can therefore be further extended to linear and nonlinear issues.

1. Introduction

A number of phenomena can be described using fractional derivatives (FD), which generalize integer derivatives and change the order of derivatives from integer to real or even complex. Fractional calculus (FC) has produced a unique mathematical approach to solutions for countless applications in a variety of scientific fields [1,2,3]. Numerous applications, including ecology, signal and image processing, economics, and mechanics, accurately depend on FC. Continuous-time random walk, anomalous diffusion, control, and vibration are the main topics of FC [4]. Recently, many books on FC have been published, and in each of them, the subject’s history is discussed in some form [5,6,7,8]. It comes naturally to use fractional derivatives when mathematically modeling viscoelastic materials, according to Podliubny [7], who surveyed numerous applications that have resulted from FC. Numerous fractional derivative forms were taken into consideration: Caputo, Riemann–Liouville, ErdélyiKober, Hadamard, Marchaud, Riesz, and Grünwald–Letnikov are just some examples. The most common definitions used for the differ-integral of fractional order are the Riemann–Liouville, Grünwald–Letnikov, and the Caputo definition. To explain fractional differential equations, a variety of strategies were proposed, for example, collocation method [9], monotone iterative method [10], Yang transform decomposition method [11,12], trapezoidal method [13] homotopy analysis method [14], Elzaki transform decomposition method [15,16], homotopy perturbation transform method [17,18], auxiliary equation method [19], fractional variational iteration method [20,21], and many more [22,23,24,25,26].

The Helmholtz equation is a potential second-order elliptic partial differential equation theory that follows naturally from the wave equation [27]. In most cases, we present it as

$$\nabla u+{\lambda }^{2}u=0,$$

(1)

where the wave number is $\lambda$, and the Laplace operator is ${\nabla }^2$. When $\lambda =0$, the Helmholtz equation is the same as Laplace’s equation. The Helmholtz equation (HE) is a generalization of Laplace’s equation.

The wave solution is $u(\xi ,\kappa )$ for a harmonic source $f(\xi ,\kappa )$ vibrating at a specific fixed frequency $\varpi >0$ using an appropriate scalar HE over an assumed region W in a 2D nonhomogeneous isotropic medium with speed c.

$$\frac{{\partial }^2}{\partial {\xi }^2}u(\xi ,\kappa )+\frac{{\partial }^2}{\partial {\kappa }^2}u(\xi ,\kappa )+\lambda u(\xi ,\kappa )=-f(\xi ,\kappa ),$$

(2)

where $u(\xi ,\kappa )$ is differentiable function over the boundary of W, $f(\xi ,\kappa )$ is a known function, $\lambda >0$ is a constant, $\sqrt{\lambda }=\frac{\varpi }{c}$ is the wave number and the wavelength is $\frac{2\pi }{\sqrt{\lambda }}$ [28].

The HE must have a singular solution if it models a physical reality. In real life, there are numerous applications for the HE. Some of them include the following: when the temperature is changed while the pressure remains constant, it is used in the field of optics to calculate changes in enthalpy; CHELS, or the combined Helmholtz equation-least squares method, is utilized in seismology, elastic waves, electromagnetism, the scientific study of earthquakes, medical imaging, volcanic eruptions, and tsunamis. Using FRDTM, Abuasad et al. [29] achieved an accurate solution for two-dimensional FHE. In order to find the solution of HE, the higher-order compact difference (HOC) method with consistent mesh sizes was applied by Ghaffar et al. [30]. Gupta et al. [31] found the approximative solution of a multidimensional partial differential Helmholtz problem with fractional space derivatives [32,33,34].

Numerous physical issues, such as fluid confined by thermally conducting walls or flows with shear viscosity, have a wide range of applications for conservation equations, which are frequently converted into the Helmholtz equation. In the former case, Nguyen and Delcarte [35] studied the Helmholtz problem with mixed derivatives using a spectral collocation method, including local fractional integral transforms [36], double-layer potentials for a generalized biaxially symmetric HE [37], the variational iteration method (VIM) [38], the cylindrical coordinates of the Cantorian and Cantor-type, and the diffusion and Helmholtz equations connected to local fractional derivative operators [39].

Because the power series approach is increasingly widespread, it was applied more frequently to address fractional issues when the standard derivative was updated to a fractional derivative. The fractional RPS and fractional DTM techniques are used to develop and address numerous key problems in various science and engineering disciplines. The method was first introduced by Omar Abu Arqub, who used it to solve first- and second-order fuzzy differential equations [40]. The RPSM offers a quick and efficient approach to creating the solution as a series for both linear and nonlinear equations. The power series expansion without perturbation, discretization, or linearization is the foundation of the innovative analytical method known as RPSM. In this study, we aimed to enhance the efficiency of the RPSM approach by adding the Laplace transform. The Laplace residual power series method (LRPSM) is the name given to this RPSM advancement. Solving three-dimensional Helmholtz equations describe the construction of this novel approach.

The purpose of this study was to employ LRPSM to the fractional 3D Helmholtz equation with $\xi$ space of the type:

$$D_{\xi }^{\varrho }u+\frac{{\partial }^2}{\partial {\kappa }^2}u(\xi ,\kappa ,\varphi )+\frac{{\partial }^2}{\partial {\varphi }^2}u(\xi ,\kappa ,\varphi )+\lambda u(\xi ,\kappa ,\varphi )=0$$

(3)

with the initial condition

$$u(0,\kappa ,\varphi )=\psi \left(\kappa \varphi \right),$$

(4)

where $\psi \left(\kappa \varphi \right)$ is a given function and $1<\varrho \le 2.$

The importance of this study is finding an accurate solution to the 3D FHE using a comparably new method and comparing the accurate solution of non-FHE to tenth-order approximations for a range of fractional derivative values. Researchers can use this study as a fundamental reference to examine this strategy and employ it in many applications to get accurate and approximative results in a few easy steps. The unique aspect of this study is the implementation of LRPSM for three-dimensional FHE with modest and easy steps. In Section 2, we provide straightforward definitions and properties of fractional calculus. Section 3 contains the proposed approach, whereas Section 4 provides accurate solutions to two cases of 3D FHE.

2. Preliminaries

In this section, we explain the basic concept associated with fractional calculus in addition to Laplace transform theorems.

Definition 1 

([41]). In the Caputo sense, the fractional derivative is

$${}^C{D}_{\varsigma }^{\varrho }u(\xi ,\varsigma )=J_{\varsigma }^{k-\varrho }{u}^k(\xi ,\varsigma ),k-1<\varrho \le k,\varsigma >0$$

(5)

with $k\in \mathbb{N}$ and $J_{\varsigma }^{\varrho }$ is the Riemann–Liouville (RL) integral operator as

$$J_{\varsigma }^{\varrho }u(\xi ,\varsigma )=\frac{1}{\Gamma \left(\varrho \right)}{\int }_0^{\varsigma }{(\varsigma -t)}^{\varrho -1}u(\xi ,t)dt.$$

(6)

Definition 2 

([41]). The function $u(\xi ,\varsigma )$ Laplace transform (LT) is

$$u(\xi ,\upsilon )={\mathit{L}}_{\varsigma }\left\{u(\xi ,\varsigma )\right\}={\int }_0^{\infty }{e}^{-\upsilon \varsigma }u(\xi ,\varsigma )d\varsigma ,\upsilon >\varrho$$

(7)

employing inverse LT as

$$u(\xi ,\varsigma )={\mathit{L}}_{\varsigma }^{-1}\left\{u(\xi ,\upsilon )\right\}={\int }_{j-i\infty }^{j+i\infty }{e}^{\upsilon \varsigma }u(\xi ,\upsilon )d\upsilon ,j=Re\left(\upsilon \right)>j_0.$$

(8)

Lemma 1. 

Consider that $u(\xi ,\varsigma )$ is a piecewise continuous function having $U(\xi ,\upsilon )={\mathit{L}}_{\varsigma }\left\{u(\xi ,\varsigma )\right\}$, then the following properties hold:

(i) 

${\displaystyle {\mathit{L}}_{\varsigma }\left\{J_{\varsigma }^{\varrho }u(\xi ,\varsigma )\right\}=\frac{U(\xi ,\upsilon )}{{\upsilon }^{\varrho }},\varrho >0;}$

(ii) 

${\displaystyle {\mathit{L}}_{\varsigma }\left\{D_{\varsigma }^{\varrho }u(\xi ,\varsigma )\right\}={\upsilon }^{\varrho }U(\xi ,\upsilon )-\sum _{k=0}^{m-1}{\upsilon }^{\varrho -k-1}{u}^k(\xi ,0),m-1<\varrho \le m;}$

(iii) 

${\displaystyle {\mathit{L}}_{\varsigma }\left\{D_{\varsigma }^{n\varrho }u(\xi ,\varsigma )\right\}={\upsilon }^{n\varrho }U(\xi ,\upsilon )-\sum _{k=0}^{n-1}{\upsilon }^{(n-k)\varrho -1}{D}_{\varsigma }^{k\varrho }u(\xi ,0),0<\varrho \le 1.}$

The proof of this Lemma is given in [21].

Theorem 1. 

Consider $u(\xi ,\varsigma )$ is a piecewise continuous on $I\times [0,\infty )$ having exponential order ϑ. Let us assume that the function $U(\xi ,\upsilon )={\mathit{L}}_{\varsigma }\left\{u(\xi ,\varsigma )\right\}$ has the fractional expansion as:

$$U(\xi ,\upsilon )=\sum _{n=0}^{\infty }\frac{f_n\left(\xi \right)}{{\upsilon }^{1+n\varrho }},0<\varrho \le 1,\xi \in I,\upsilon >\vartheta .$$

(9)

Thus, $f_n\left(\xi \right)=D_{\varsigma }^{n\varrho }u(\xi ,0)$.

The proof of this Theorem can be seen in [41].

Remark 1. 

By employing inverse LT to (9) given as [41]:

$$u(\xi ,\varsigma )=\sum _{i=0}^{\infty }\frac{D_{\varsigma }^{\varrho }u(\xi ,0)}{\Gamma (1+i\varrho )}{\varsigma }^{i\left(\vartheta \right)},0<\vartheta \le 1,\varsigma \ge 0,$$

(10)

which is similar to the fractional Taylor’s formula stated in [42].

The subsequent Theorem describes and establishes the convergence of the FPS in Theorem 1.

3. LRPSM Idea

Consider the following general fractional differential equation

$$D_{\varsigma }^{\varrho }u(\xi ,\varsigma )=c{D}_{\xi }^{2}u(\xi ,\varsigma )+au(\xi ,\varsigma )-b{u}^4(\xi ,\varsigma )$$

(11)

subjected to the initial condition

$$u(\xi ,\varsigma )=f_0\left(\xi \right).$$

(12)

First, employ the LT to (11), we get

$$\mathbf{L}\left\{D_{\varsigma }^{\varrho }u(\xi ,\varsigma )\right\}=c\mathbf{L}\left\{D_{\xi }^{2}u(\xi ,\varsigma )\right\}+a\mathbf{L}\left\{u(\xi ,\varsigma )\right\}-b\mathbf{L}\left\{u^4(\xi ,\varsigma )\right\}.$$

(13)

From the statement that $\mathbf{L}\left\{D_1^{a}u(\xi ,\varsigma )\right\}={\upsilon }^a\mathbf{L}\left\{u(\xi ,\varsigma )\right\}-{\upsilon }^{a-1}u(\xi ,0)$ and by using (12), we have

$$U(\xi ,\upsilon )=\frac{f_0\left(\xi \right)}{\upsilon }+\frac{c}{{\upsilon }^a}{D}_{\upsilon }^{2}U(\xi ,\upsilon )+\frac{a}{{\upsilon }^a}U(\xi ,\upsilon )-\frac{b}{{\upsilon }^a}\mathbf{L}\left\{{\left({\mathbf{L}}^{-1}\left\{U(\xi ,\upsilon )\right\}\right)}^a\right\}$$

(14)

with $U(\xi ,\upsilon )=\mathbf{L}\left\{u(\xi ,\varsigma )\right\}$.

Second, we describe the altered function $U(\xi ,\upsilon )$ as

$$U(\xi ,\upsilon )=\sum _{n=0}^{\infty }\frac{f_{\upsilon }\left(\xi \right)}{{\upsilon }^{n\varrho +1}}.$$

(15)

The kth-truncated series of (15) is stated as

$$U_k(\xi ,\upsilon )=\sum _{n=0}^k\frac{f_{\upsilon }\left(\xi \right)}{{\upsilon }^{n\varrho +1}}=\frac{f_0\left(\xi \right)}{\upsilon }+\sum _{n=1}^k\frac{f_k\left(\xi \right)}{{\upsilon }^{n\varrho +1}}.$$

(16)

As stated in [43], from the Laplace residual function definition

$$\begin{array}{cc}\hfill {\mathbf{L}Res}_k(\xi ,\upsilon )& =U_k(\xi ,\upsilon )-\frac{f_0\left(\xi \right)}{\upsilon }-\frac{c}{{\upsilon }^{\varrho }}{D}_{\upsilon }^2{U}_k(\xi ,\upsilon )-\frac{a}{{\upsilon }^{\varrho }}{U}_k(\xi ,\upsilon )\hfill \\ \hfill & +\frac{b}{{\upsilon }^{\varrho }}\mathbf{L}\left\{{\left({\mathbf{L}}^{-1}\left\{U_k(\xi ,\upsilon )\right\}\right)}^q\right\}.\hfill \end{array}$$

(17)

Third, we provide a few characteristics of the typical residual power series approach [43]:

(i)

$\mathbf{L}\Re (\xi ,\upsilon )=0$ and ${lim}_{k\to \infty }\mathbf{L}\Re {\upsilon }_k(\xi ,\upsilon )=\mathbf{L}\Re (\xi ,\upsilon )$ for each $\upsilon >0$;

(ii)

If ${lim}_{\upsilon \to \infty }\upsilon \mathbf{L}\Re (\xi ,\upsilon )=0$, then ${lim}_{\upsilon \to \infty }\upsilon \mathbf{L}\Re (\xi ,\upsilon )=0;$

(iii)

${lim}_{\upsilon \to \infty }{\upsilon }^{k\varrho +1}\mathbf{L}\Re (\xi ,\upsilon )={lim}_{\upsilon \to \infty }{\upsilon }^{k\varrho +1}\mathbf{L}{\Re }_k(\xi ,\upsilon )=0$ for $0<\varrho \le 1$ and $k\in \mathbb{N}.$

We now perform a further iterative solution of the system to obtain the coefficient values $f_n\left(\xi \right)$

$$\underset{\upsilon \to \infty }{lim}\left({\upsilon }^{ka+1}{\mathbf{L}Res}_k(\xi ,\upsilon )\right)=0$$

for $0<\varrho \le 1$ and $k\in \mathbb{N}.$

Finally, we employ inverse LT to $U_k(\xi ,\upsilon )$ for obtaining the kth approximations $u_k(\xi ,\varsigma )$.

4. Numerical Problems

In this section, We examine the LRPSM significance for extracting the 3D FHE’s closed form solution.

Example 1. 

Let us consider 3D FHE of the form

$$D_{\xi }^{\varrho }u+u_{\kappa \kappa }+u_{\varphi \varphi }-u=0$$

(18)

with the initial condition

$$u(0,\kappa ,\varphi )=\kappa +\varphi .$$

(19)

Taking the LT to (18) and by utilizing (19), we have

$$U(\upsilon ,\kappa ,\varphi )-\frac{\kappa +\varphi }{\upsilon }+\frac{1}{{\upsilon }^{\varrho }}{\mathbf{L}}_{\xi }\left\{{\mathbf{L}}_{\xi }^{-1}\left\{U_{\kappa \kappa }\right\}+{\mathbf{L}}_{\xi }^{-1}\left\{U_{\varphi \varphi }\right\}-{\mathbf{L}}_{\xi }^{-1}\left\{U\right\}\right\}=0.$$

(20)

The kth-truncated series is stated as

$$U(\upsilon ,\kappa ,\varphi )=\frac{\kappa +\varphi }{\upsilon }+\sum _{n=1}^k\frac{f_n(\upsilon ,\kappa ,\varphi )}{{\upsilon }^{n\varrho +1}},k=1,2,3,\dots ,$$

(21)

thus, the kth LRFs are:

$$\begin{array}{cc}\hfill {\mathbf{L}}_{t}Re{s}_{u,k}(\upsilon ,\kappa ,\varphi )& =U_k(\upsilon ,\kappa ,\varphi )-\frac{\kappa +\varphi }{\upsilon }\hfill \\ \hfill & +\frac{1}{{\upsilon }^{\varrho }}{\mathbf{L}}_{\xi }\left\{{\mathbf{L}}_{\xi }^{-1}\left\{U_{\kappa \kappa ,k}\right\}+{\mathbf{L}}_{\xi }^{-1}\left\{U_{\varphi \varphi ,k}\right\}-{\mathbf{L}}_{\xi }^{-1}\left\{U_k\right\}\right\}.\hfill \end{array}$$

(22)

To obtain $f_k(\upsilon ,\kappa ,\varphi )$, the kth-truncated series (21) is now inserted into the kth Laplace residual function (22). The derived equation is then multiplied by ${\upsilon }^{k\varrho +1}$ and now we solve the relation

$$\underset{\upsilon \to \infty }{lim}\left({\upsilon }^{k\varrho +1}{\mathbf{L}}_{t}Re{s}_{u,k}(\upsilon ,\kappa ,\varphi )\right)=0,k=1,2,3,\dots .$$

Some values are as:

$$\begin{array}{cc}\hfill f_1(\upsilon ,\kappa ,\varphi )& =\kappa +\varphi ,\hfill \\ \hfill f_2(\upsilon ,\kappa ,\varphi )& =\kappa +\varphi ,\hfill \\ \hfill f_3(\upsilon ,\kappa ,\varphi )& =\kappa +\varphi ,\hfill \\ \hfill f_4(\upsilon ,\kappa ,\varphi )& =\kappa +\varphi ,\hfill \\ \hfill f_5(\upsilon ,\kappa ,\varphi )& =\kappa +\varphi ,\hfill \\ \hfill f_6(\upsilon ,\kappa ,\varphi )& =\kappa +\varphi ,\hfill \\ \hfill f_7(\upsilon ,\kappa ,\varphi )& =\kappa +\varphi ,\hfill \\ \hfill f_8(\upsilon ,\kappa ,\varphi )& =\kappa +\varphi \hfill \end{array}$$

and so on.

By inserting the values for $f_k(\upsilon ,\kappa )$ with $k=1,2,3,\dots$ in (21), we can now obtain

$$\begin{array}{cc}\hfill U(\upsilon ,\kappa ,\varphi )& =\frac{\kappa +\varphi }{\upsilon }+\frac{\kappa +\varphi }{{\upsilon }^{\varrho +1}}+\frac{\kappa +\varphi }{{\upsilon }^{2\varrho +1}}+\frac{\kappa +\varphi }{{\upsilon }^{3\varrho +1}}+\frac{\kappa +\varphi }{{\upsilon }^{4\varrho +1}}\hfill \\ \hfill & +\frac{\kappa +\varphi }{{\upsilon }^{5\varrho +1}}+\frac{\kappa +\varphi }{{\upsilon }^{6\varrho +1}}+\frac{\kappa +\varphi }{{\upsilon }^{7\varrho +1}}+\frac{\kappa +\varphi }{{\upsilon }^{8\varrho +1}}+\dots .\hfill \end{array}$$

(23)

When we take the inverse of LT, we have

$$\begin{array}{cc}\hfill u(\xi ,\kappa ,\varphi )& =(\kappa +\varphi )+(\kappa +\varphi )\frac{{\varsigma }^{\varrho }}{\Gamma (\varrho +1)}+(\kappa +\varphi )\frac{{\varsigma }^{2\varrho }}{\Gamma (2\varrho +1)}\hfill \\ \hfill & +(\kappa +\varphi )\frac{{\varsigma }^{3\varrho }}{\Gamma (3\varrho +1)}+(\kappa +\varphi )\frac{{\varsigma }^{4\varrho }}{\Gamma (4\varrho +1)}+(\kappa +\varphi )\frac{{\varsigma }^{5\varrho }}{\Gamma (5\varrho +1)}\hfill \\ \hfill & +(\kappa +\varphi )\frac{{\varsigma }^{6\varrho }}{\Gamma (6\varrho +1)}+(\kappa +\varphi )\frac{{\varsigma }^{7\varrho }}{\Gamma (7\varrho +1)}+(\kappa +\varphi )\frac{{\varsigma }^{8\varrho }}{\Gamma (8\varrho +1)}+\dots .\hfill \end{array}$$

Taking $\varrho =2$, we have

$$u(\xi ,\kappa ,\varphi )=(\kappa +\varphi )cosh\left(\xi \right).$$

(24)

In Figure 1, exact and proposed approach tenth-order approximate solution at $\varrho =2$ and $\kappa =0.01$ for Example 1. Figure 2, suggested approach to solution at $\varrho =1.8,1.6$ and $\kappa =0.01$ for Example 1. Figure 3, the suggested approach tenth-order analytical solution at numerous values of ϱ and $\kappa =0.01$ for Example 1. In

Table 1. The exact solution and proposed method tenth-order approximate solution of Example 1 at different fractional-orders of $\varrho$ and $\kappa =0.01$.

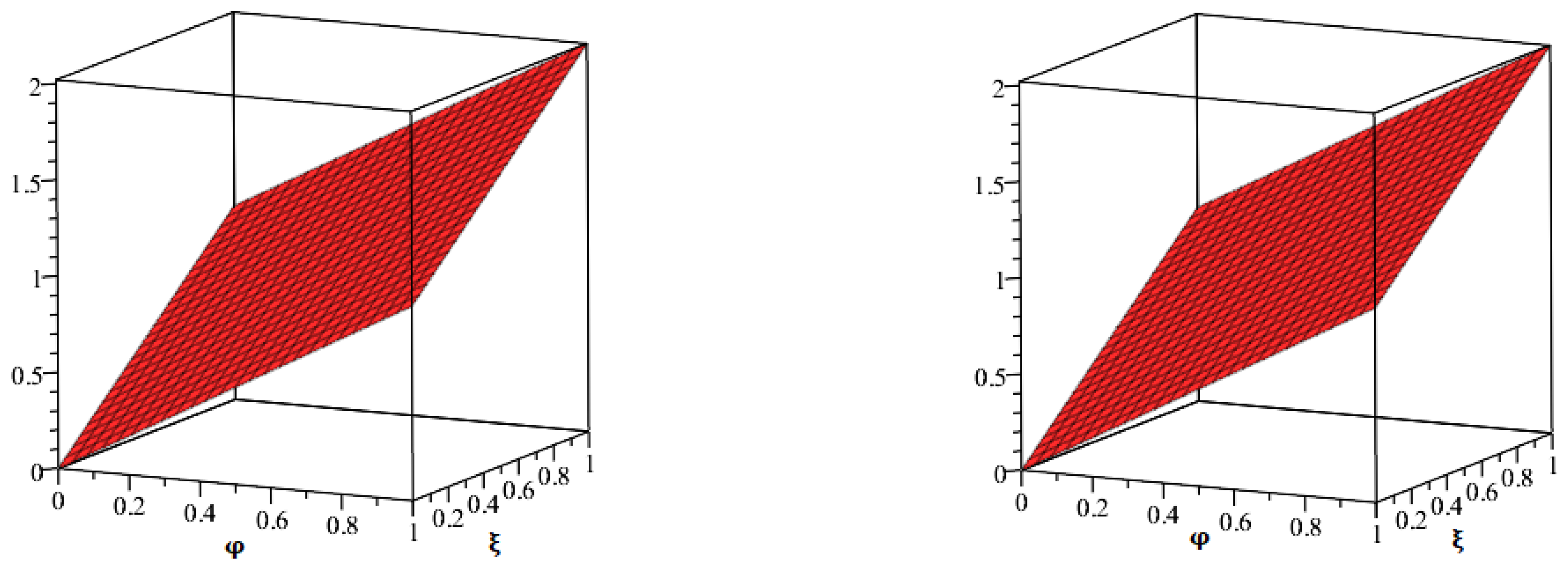
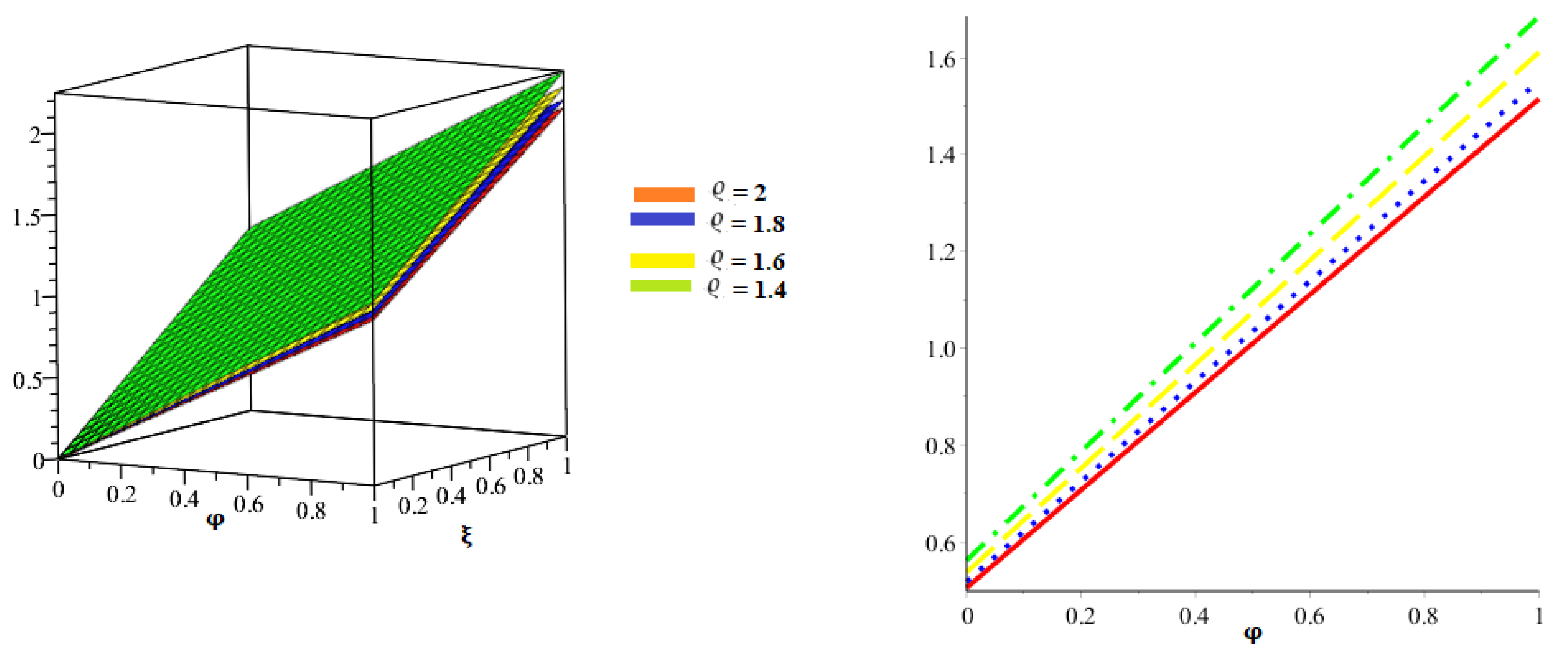
$(\mathit{\varphi },\mathit{\xi })$	$\mathit{u}(\mathit{\xi },\mathit{\kappa },\mathit{\varphi })$ at $\mathit{\varrho }=1.5$	$\mathit{u}(\mathit{\xi },\mathit{\kappa },\mathit{\varphi })$ at $\mathit{\varrho }=1.75$	$\mathit{LRPSM}$ at $\mathit{\varrho }=2$	Exact Solution
(0.2, 0.01)	0.2102371	0.2100072	0.2100000	0.2100000
(0.4, 0.01)	0.4104630	0.4100141	0.4100000	0.4100000
(0.6, 0.01)	0.6106889	0.6100209	0.6100000	0.6100000
(0.2, 0.02)	0.2103355	0.2100121	0.2100000	0.2100000
(0.4, 0.02)	0.4106550	0.4100237	0.4100000	0.4100000
(0.6, 0.02)	0.6109746	0.6100353	0.6100000	0.6100000
(0.2, 0.03)	0.2104110	0.2100164	0.2100000	0.2100000
(0.4, 0.03)	0.4108025	0.4100321	0.4100000	0.4100000
(0.6, 0.03)	0.6111940	0.6100478	0.6100000	0.6100000
(0.2, 0.04)	0.2104747	0.2100204	0.2100000	0.2100000
(0.4, 0.04)	0.4109269	0.4100399	0.4100000	0.4100000
(0.6, 0.04)	0.6113790	0.6100593	0.6100000	0.6100000
(0.2, 0.05)	0.2105309	0.2100241	0.2100000	0.2100000
(0.4, 0.05)	0.4110365	0.4100471	0.4100000	0.4100000
(0.6, 0.05)	0.6115421	0.6100701	0.6100000	0.6100000

, the exact solution and proposed method tenth-order approximate solution of Example 1 at different fractional-orders of ϱ and $\kappa =0.01$.

Example 2. 

Let us consider 3D FHE with ξ space fractional derivative of the form

$$D_{\xi }^{\varrho }u+u_{\kappa \kappa }+u_{\varphi \varphi }+5u=0$$

(25)

with the initial condition

$$u(0,\kappa ,\varphi )=\kappa +\varphi .$$

(26)

Taking the LT to (25) and by utilizing (26), we obtain

$$U(\upsilon ,\kappa ,\varphi )-\frac{\kappa +\varphi }{\upsilon }+\frac{1}{{\upsilon }^{\varrho }}{\mathbf{L}}_{\xi }\left\{{\mathbf{L}}_{\xi }^{-1}\left\{U_{\kappa \kappa }\right\}+{\mathbf{L}}_{\xi }^{-1}\left\{U_{\varphi \varphi }\right\}+5{\mathbf{L}}_{\xi }^{-1}\left\{U\right\}\right\}=0.$$

(27)

The kth-truncated series is stated as

$$U(\upsilon ,\kappa ,\varphi )=\frac{\kappa +\varphi }{\upsilon }+\sum _{n=1}^k\frac{f_n(\upsilon ,\kappa ,\varphi )}{{\upsilon }^{n\varrho +1}},k=1,2,3,\dots .$$

(28)

Thus, the kth LRFs are

$$\begin{array}{cc}\hfill {\mathbf{L}}_{t}Re{s}_{u,k}(\upsilon ,\kappa ,\varphi )& =U_k(\upsilon ,\kappa ,\varphi )-\frac{\kappa +\varphi }{\upsilon }\hfill \\ \hfill & +\frac{1}{{\upsilon }^{\varrho }}{\mathbf{L}}_{\xi }\left\{{\mathbf{L}}_{\xi }^{-1}\left\{U_{\kappa \kappa ,k}\right\}+{\mathbf{L}}_{\xi }^{-1}\left\{U_{\varphi \varphi ,k}\right\}+5{\mathbf{L}}_{\xi }^{-1}\left\{U_k\right\}\right\}.\hfill \end{array}$$

(29)

To obtain $f_k(\upsilon ,\kappa ,\varphi )$, the kth-truncated series (28) is now inserted into the kth Laplace residual function (29). The derived equation is then multiplied by ${\upsilon }^{k\varrho +1}$ and now we solve the relation

$$\underset{\upsilon \to \infty }{lim}\left({\upsilon }^{k\varrho +1}{\mathbf{L}}_{t}Re{s}_{u,k}(\upsilon ,\kappa ,\varphi )\right)=0,k=1,2,3,\dots .$$

Some values are as:

$$\begin{array}{cc}\hfill f_1(\upsilon ,\kappa ,\varphi )& =-5\kappa +\varphi ,\hfill \\ \hfill f_2(\upsilon ,\kappa ,\varphi )& =25\kappa +\varphi ,\hfill \\ \hfill f_3(\upsilon ,\kappa ,\varphi )& =-125\kappa +\varphi ,\hfill \\ \hfill f_4(\upsilon ,\kappa ,\varphi )& =625\kappa +\varphi ,\hfill \\ \hfill f_5(\upsilon ,\kappa ,\varphi )& =-3125\kappa +\varphi ,\hfill \\ \hfill f_6(\upsilon ,\kappa ,\varphi )& =\mathrm{15,625}\kappa +\varphi ,\hfill \\ \hfill f_7(\upsilon ,\kappa ,\varphi )& =-\mathrm{78,125}\kappa +\varphi ,\hfill \\ \hfill f_8(\upsilon ,\kappa ,\varphi )& =\mathrm{390,625}\kappa +\varphi \hfill \end{array}$$

and so on.

By inserting the values for $f_k(\upsilon ,\kappa )$ with $k=1,2,3,\dots$ in (28), we now acquire

$$\begin{array}{cc}\hfill U(\upsilon ,\kappa ,\varphi )& =\frac{\kappa +\varphi }{\upsilon }-\frac{5\kappa +\varphi }{{\upsilon }^{\varrho +1}}+\frac{25}{{\upsilon }^{2\varrho +1}}-\frac{125}{{\upsilon }^{3\varrho +1}}+\frac{625}{{\upsilon }^{4\varrho +1}}\hfill \\ \hfill & -\frac{3125}{{\upsilon }^{5\varrho +1}}+\frac{\mathrm{15,625}}{{\upsilon }^{6\varrho +1}}-\frac{\mathrm{78,125}}{{\upsilon }^{7\varrho +1}}+\frac{\mathrm{390,625}}{{\upsilon }^{8\varrho +1}}-\cdots .\hfill \end{array}$$

(30)

When we take the inverse of LT, we have

$$\begin{array}{cc}\hfill u(\xi ,\kappa ,\varphi )& =(\kappa +\varphi )-5(\kappa +\varphi )\frac{{\varsigma }^{\varrho }}{\Gamma (\varrho +1)}+25(\kappa +\varphi )\frac{{\varsigma }^{2\varrho }}{\Gamma (2\varrho +1)}-125(\kappa +\varphi )\frac{{\varsigma }^{3\varrho }}{\Gamma (3\varrho +1)}\hfill \\ \hfill & +625(\kappa +\varphi )\frac{{\varsigma }^{4\varrho }}{\Gamma (4\varrho +1)}-3125\frac{{\varsigma }^{5\varrho }}{\Gamma (5\varrho +1)}+\mathrm{15,625}\frac{{\varsigma }^{6\varrho }}{\Gamma (6\varrho +1)}\hfill \\ \hfill & -\mathrm{78,125}\frac{{\varsigma }^{7\varrho }}{\Gamma (7\varrho +1)}+\mathrm{390,625}\frac{{\varsigma }^{8\varrho }}{\Gamma (8\varrho +1)}-\cdots .\hfill \end{array}$$

Taking $\varrho =2$, we have

$$u(\xi ,\kappa ,\varphi )=(\kappa +\varphi )cos\left(\sqrt{5}\xi \right).$$

(31)

In Figure 4, exact and proposed approach tenth-order approximate solution at $\varrho =2$ and $\kappa =0.01$ for Example 2. Figure 5, suggested approach to solution at $\varrho =1.8,1.6$ and $\kappa =0.01$ for Example 2. Figure 6, the suggested approach tenth-order analytical solution at numerous values of ϱ and $\kappa =0.01$ for Example 2. In

Table 2. Exact and proposed method tenth-order approximate solution at numerous orders of $\varrho$ and $\kappa =0.01$ of Example 2.

$(\mathit{\varphi },\mathit{\xi })$	$\mathit{u}(\mathit{\xi },\mathit{\kappa },\mathit{\varphi })$ at $\mathit{\varrho }=1.5$	$\mathit{u}(\mathit{\xi },\mathit{\kappa },\mathit{\varphi })$ at $\mathit{\varrho }=1.75$	$\mathit{LRPSM}$ at $\mathit{\varrho }=2$	Exact Solution
(0.2, 0.01)	0.2088204	0.2099810	0.2099994	0.2099994
(0.4, 0.01)	0.4076970	0.4099466	0.4099989	0.4099989
(0.6, 0.01)	0.6065736	0.6099392	0.6099984	0.6099984
(0.2, 0.02)	0.2083348	0.2099813	0.2099989	0.2099989
(0.4, 0.02)	0.4067490	0.4099235	0.4099979	0.4099979
(0.6, 0.02)	0.6051632	0.6099176	0.6099969	0.6099969
(0.2, 0.03)	0.2079635	0.2099392	0.2099984	0.2099984
(0.4, 0.03)	0.4060240	0.4099608	0.4099969	0.4099969
(0.6, 0.03)	0.6040845	0.6099978	0.6099954	0.6099954
(0.2, 0.04)	0.2076512	0.2098978	0.2099979	0.2099979
(0.4, 0.04)	0.4054143	0.4099005	0.4099959	0.4099959
(0.6, 0.04)	0.6031774	0.6099032	0.6099939	0.6099939
(0.2, 0.05)	0.2073767	0.2099792	0.2099973	0.2099973
(0.4, 0.05)	0.4048784	0.4099642	0.4099948	0.4099948
(0.6, 0.05)	0.6023800	0.6099492	0.6099923	0.6099923

, the exact solution and proposed method tenth-order approximate solution of Example 1 at different fractional-orders of ϱ and $\kappa =0.01$.

5. Conclusions

In order to solve several significant nonlinear temporal-fractional models, a novel method combining the Laplace transform operator and residual power series was described in this paper. The advantage of the new technique is that it requires less computation to determine the result in series form, whose coefficients are established in a series of algebraic steps. Two separate physical models were solved using the suggested method, and graphs and tables showed that it was accurate. Finally, we demonstrated that the Laplace residual power series approach could handle fractional nonlinear equations with excellent accuracy and simple computation operations. Graphs and tables were used to display the results that were obtained. We determined from the graphs and tables that the exact and analytical solutions are closely related to one another. Using the existing method, smaller calculations have greater accuracy and can be used to expand the Laplace transform residual power series schemes to higher dimensional physical applications in a future study. Additionally, the suggested approach can be applied to analyze many fractional problems related to the propagation of nonlinear phenomena in plasma physics, for instance, studying the impact of the temporal fractional on the solitary waves, conoidal waves, and rogue waves [44,45,46,47] in different plasma models in addition to other oscillations in fluid mechanics many fields of science [48,49,50,51,52].