Symmetries and Dynamics of Generalized Biquaternionic Julia Sets Defined by Various Polynomials
Abstract
:1. Introduction
2. Preliminaries
2.1. Biquaternions and Their Properties
2.2. Julia Sets within Biquaternions
2.2.1. Power Polynomials
2.2.2. Monic Higher-Degree Polynomials
3. Symmetry of Biquaternionic Julia Sets
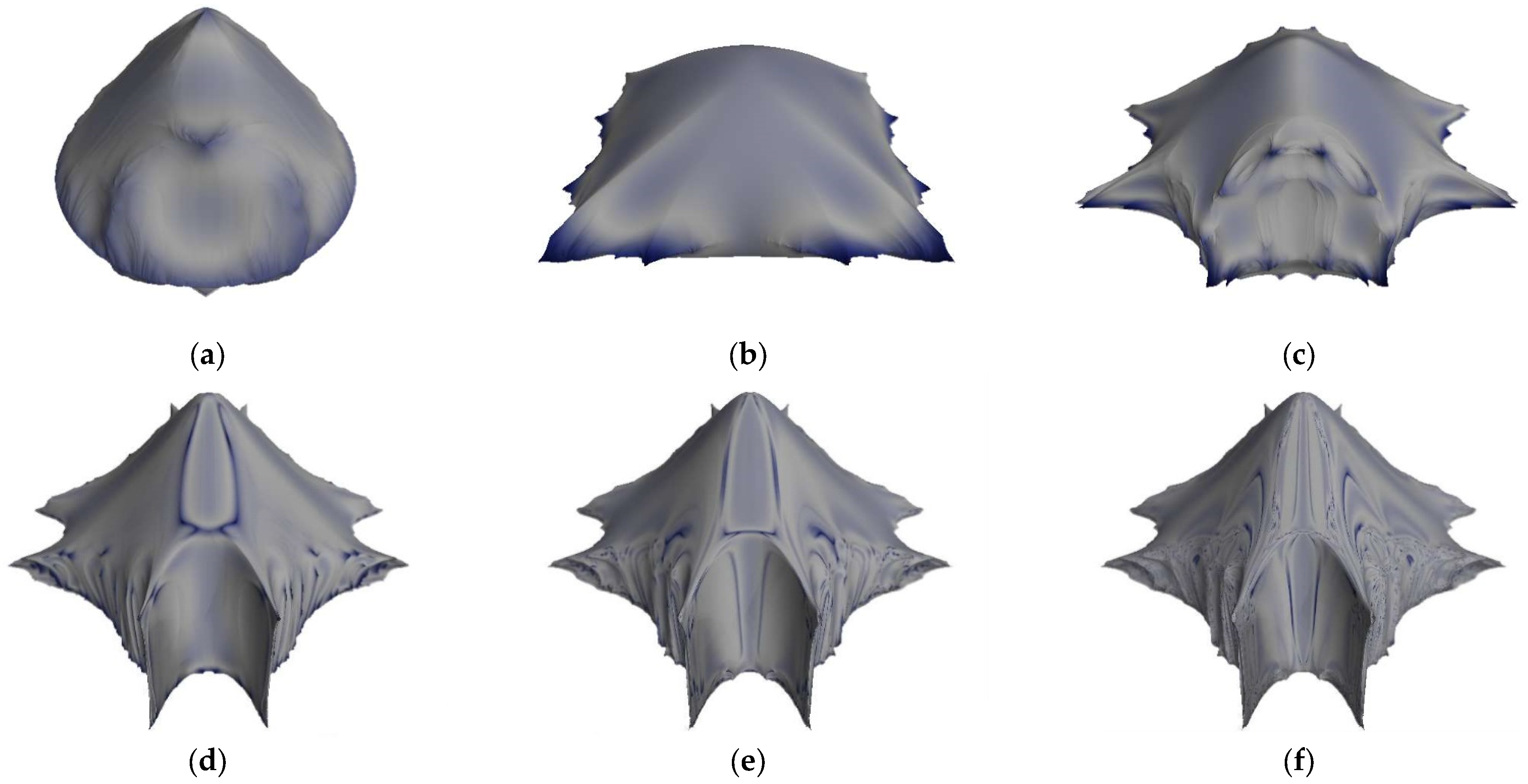
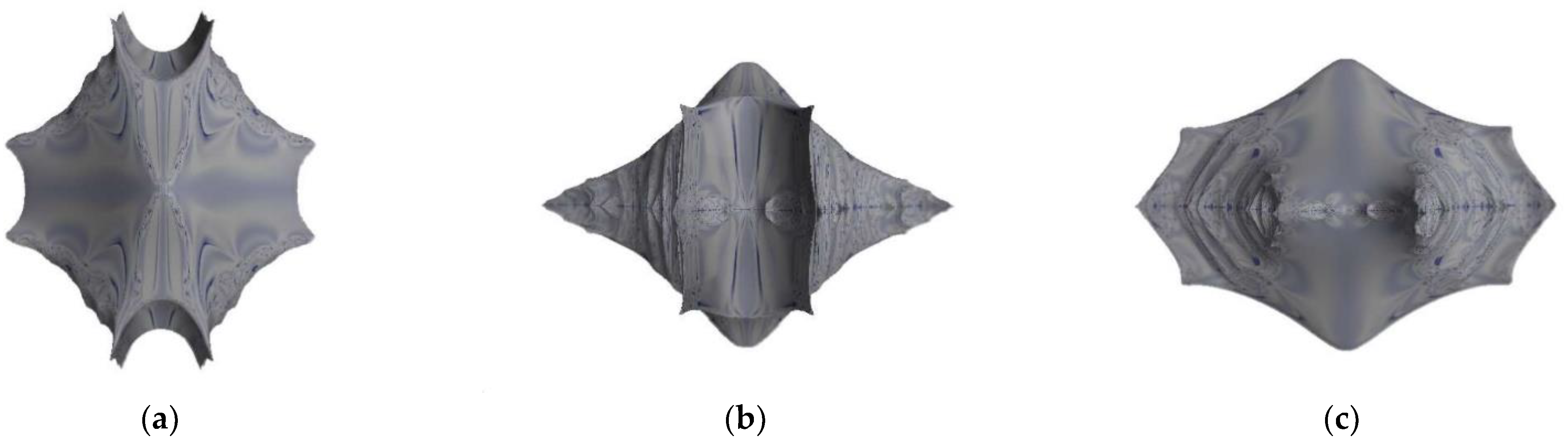
3.1. Symmetry of Biquaternionic Julia Sets Defined by Power Polynomials
3.2. Symmetry of Biquaternionic Julia Sets Defined by Monic Higher-Degree Polynomials
4. Stability of Biquaternionic Julia Sets
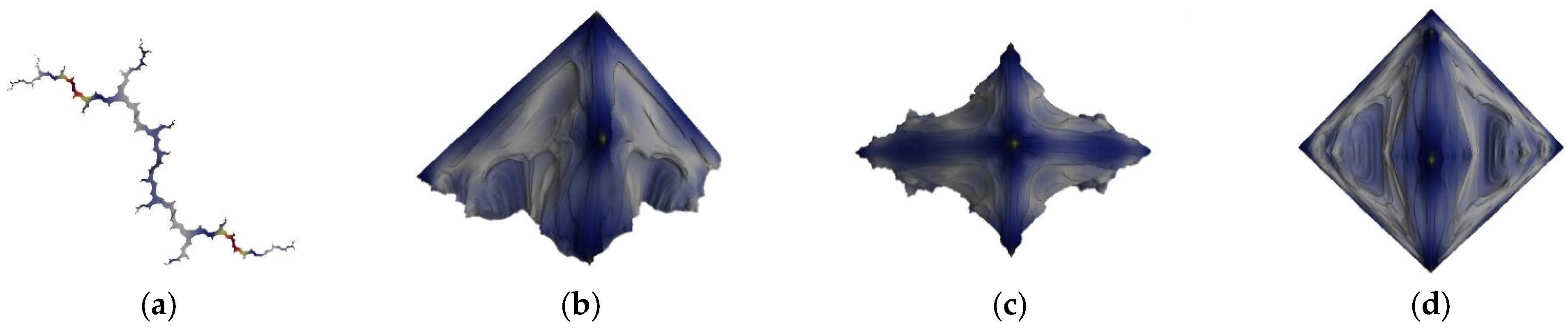
4.1. Stability of Biquaternionic Julia Sets Defined by Power Polynomials
4.1.1. 1-Cycle Stability
4.1.2. 2-Cycle Stability
4.1.3. 3-Cycle Stability
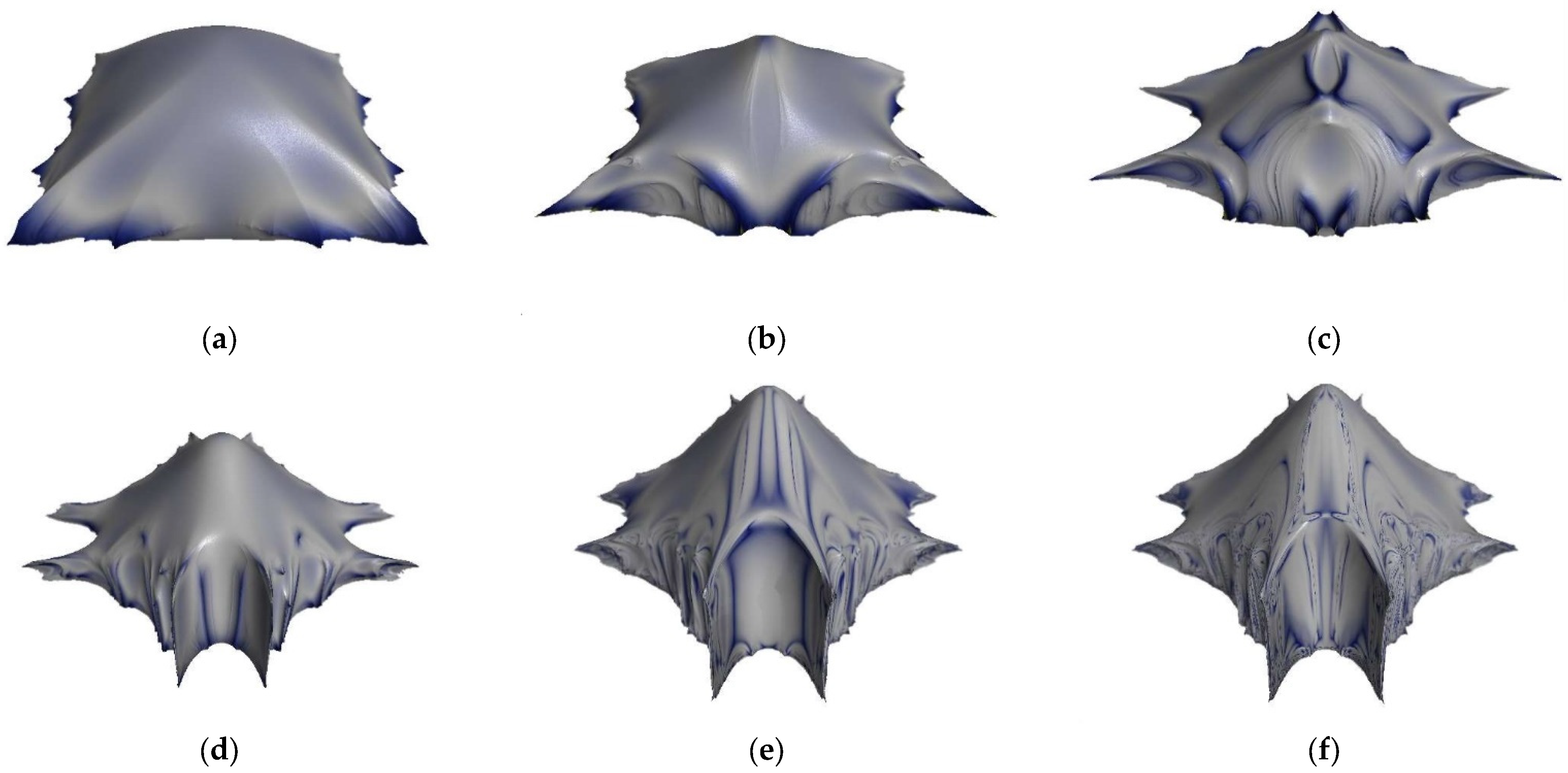
4.2. Stability of Biquaternionic Julia Sets Defined by Monic Higher-Degree Polynomials
4.2.1. 1-Cycle Stability
4.2.2. 2-Cycle Stability
4.2.3. 3-Cycle Stability
5. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
List of Symbols
| complex elements of a biquaternion | |
| complex elements of a biquaternion | |
| constant value of the Julia/Mandelbrot set | |
| complex number space | |
| bicomplex number space | |
| multicomplex number space | |
| bicomplex number space | |
| biquaternion number space | |
| complex elements of the constant value of the biquaternionic Julia set | |
| vector part of constant value of the biquaternionic Julia set | |
| real elements of the extended representation of a biquaternion | |
| real elements of the extended representation of a biquaternion | |
| quaternion number space | |
| imaginary units | |
| invariant unit matrix | |
| imaginary unit | |
| generalized biquaternionic Julia set | |
| natural number space | |
| Landau symbol | |
| octonion number space | |
| power of the iterated variable of the Julia/Mandelbrot set | |
| biquaternion | |
| real number space | |
| scalar parts of the iterated variable of the biquaternionic Julia set | |
| sedenion number space | |
| complex elements of vector parts of biquaternions | |
| vector parts of the iterated variable of the biquaternionic Julia set | |
| complex elements of vector parts of the iterated variable of the biquaternionic Julia set | |
| complex elements of scalar parts of the iterated variable of the biquaternionic Julia set | |
| complex elements of vector parts of the iterated variable of the biquaternionic Julia set | |
| iterated variable of the Julia/Mandelbrot set | |
| small perturbation parameter | |
| eigenvalues | |
| biquaternionic root of −1 | |
| Pauli matrices | |
| arbitrary complex numbers | |
| symmetry plane along the axes of imaginary values and | |
| symmetry plane along the axes of reals and imaginary values | |
| symmetry plane along the axes of reals and imaginary values |
Appendix A
References
- Mandelbrot, B.B. The Fractal Geometry of Nature; Freeman and Company: New York, NY, USA, 1983. [Google Scholar]
- Falconer, K. Fractal Geometry: Mathematical Foundations and Applications, 3rd ed.; John Wiley and Sons: Chichester, UK, 2014. [Google Scholar]
- Norton, A.V. Generation and display of geometric fractals in 3-D. Comput. Graph. 1982, 16, 61–67. [Google Scholar] [CrossRef]
- Norton, A.V. Julia sets in the quaternions. Comput. Graph. 1989, 13, 267–278. [Google Scholar] [CrossRef]
- Holbrook, J.A.R. Quaternionic Fatou-Julia sets. Ann. Sci. Math. Que. 1987, 11, 79–94. [Google Scholar]
- Griffin, C.J.; Joshi, G.C. Octonionic Julia sets. Chaos Solitons Fract. 1992, 2, 11–24. [Google Scholar] [CrossRef]
- Griffin, C.J.; Joshi, G.C. Transition points in octonionic Julia sets. Chaos Solitons Fract. 1993, 3, 67–88. [Google Scholar] [CrossRef]
- Griffin, C.J.; Joshi, G.C. Associators in generalized octonionic maps. Chaos Solitons Fract. 1993, 3, 307–319. [Google Scholar] [CrossRef]
- Katunin, A. A Concise Introduction to Hypercomplex Fractals; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Dixon, S.L.; Steele, K.L.; Burton, R.P. Generation and graphical analysis of Mandelbrot and Julia sets in more than four dimensions. Comput. Graph. 1996, 20, 451–456. [Google Scholar] [CrossRef]
- Wang, X.Y.; Sun, Y.Y. The general quaternionic M-J sets on the mapping z ← zα + c (α ∈ N). Comput. Math. Appl. 2007, 53, 1718–1732. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.Y.; Jin, T. Hyperdimensional generalized M–J sets in hypercomplex number space. Nonlinear Dyn. 2013, 73, 843–852. [Google Scholar] [CrossRef]
- Rochon, D. A generalized Mandelbrot set for bicomplex numbers. Fractals 2000, 8, 355–368. [Google Scholar] [CrossRef]
- Wang, X.Y.; Song, W.J. The generalized M–J sets for bicomplex numbers. Nonlinear Dyn. 2013, 72, 17–26. [Google Scholar] [CrossRef]
- Garant-Pelletier, V.; Rochon, D. On a generalized Fatou-Julia theorem in multicomplex spaces. Fractals 2009, 17, 241–255. [Google Scholar] [CrossRef]
- Parisé, P.O.; Rochon, D. A study of dynamics of the tricomplex polynomial ηp + c. Nonlinear Dyn. 2015, 82, 157–171. [Google Scholar] [CrossRef]
- Brouillette, G.; Rochon, D. Characterization of the principal 3D slices related to the multicomplex Mandelbrot set. Adv. Appl. Clifford Algebr. 2019, 29, 39. [Google Scholar] [CrossRef] [Green Version]
- Gintz, T.W. Artist’s statement CQUATS—A non-distributive quad algebra for 3D renderings of Mandelbrot and Julia sets. Comput. Graph. 2002, 26, 367–370. [Google Scholar] [CrossRef]
- Bogush, A.A.; Gazizov, A.Z.; Kurochkin, Y.A.; Stosui, V.T. Symmetry properties of quaternionic and biquaternionic analogs of Julia sets. Ukr. J. Phys. 2003, 48, 295–299. [Google Scholar]
- Katunin, A. Analysis of 4D hypercomplex generalizations of Julia sets. In Proceedings of the Computer Vision and Graphics, Warsaw, Poland, 19–21 September 2016; Chmielewski, L.J., Datta, A., Kozera, R., Wojciechowski, K., Eds.; Lecture Notes in Computer Science. Springer: Cham, Switzerland, 2016; Volume 9972, pp. 627–635. [Google Scholar]
- Katunin, A. The generalized biquaternionic M-J sets. J. Geom. Graph. 2018, 22, 49–58. [Google Scholar]
- Rosenfeld, B. Geometry of Lie Groups; Springer: Dordrecht, The Netherlands, 1997. [Google Scholar]
- Francis, M.R.; Kosowsky, A. The construction of spinors in geometric algebra. Ann. Phys. 2005, 317, 383–409. [Google Scholar] [CrossRef] [Green Version]
- Sangwine, S.J.; Alfsmann, D. Determination of the biquaternion divisors of zero, including the idempotents and nilpotents. Adv. Appl. Clifford Algebr. 2010, 20, 401–410. [Google Scholar] [CrossRef] [Green Version]
- Sangwine, S.J.; Ell, T.A.; Le Bihan, N. Fundamental representations and algebraic properties of biquaternions or complexified quaternions. Adv. Appl. Clifford Algebras 2011, 21, 607–636. [Google Scholar] [CrossRef] [Green Version]
- Branner, B.; Fagella, N. Homeomorphisms between limbs of the Mandelbrot set. J. Geom. Anal. 1999, 9, 327–390. [Google Scholar] [CrossRef] [Green Version]
- Zireh, A. A generalized Mandelbrot set of polynomials of type Ed for bicomplex numbers. Georgian Math. J. 2008, 15, 189–194. [Google Scholar] [CrossRef]
- Katunin, A.; Fedio, K. On a visualization of the convergence of the boundary of generalized Mandelbrot set to (n − 1)-sphere. J. Appl. Math. Comput. Mech. 2015, 14, 63–69. [Google Scholar] [CrossRef]
- Katunin, A. On the convergence of multicomplex M-J sets to the Steinmetz hypersolids. J. Appl. Math. Comput. Mech. 2016, 15, 67–74. [Google Scholar] [CrossRef] [Green Version]
- Gomatam, J.; Doyle, J.; Steves, B.; McFarlane, I. Generalization of the Mandelbrot set: Quaternionic quadratic maps. Chaos Solitons Fract. 1995, 5, 971–986. [Google Scholar] [CrossRef]
- Berezin, A.V.; Kurochkin, U.A.; Tolkachov, E.A. Quaternions in Relativistic Physics, 2nd ed.; Editorial URSS: Moscow, Russia, 2003. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Katunin, A. Symmetries and Dynamics of Generalized Biquaternionic Julia Sets Defined by Various Polynomials. Symmetry 2023, 15, 43. https://doi.org/10.3390/sym15010043
Katunin A. Symmetries and Dynamics of Generalized Biquaternionic Julia Sets Defined by Various Polynomials. Symmetry. 2023; 15(1):43. https://doi.org/10.3390/sym15010043
Chicago/Turabian StyleKatunin, Andrzej. 2023. "Symmetries and Dynamics of Generalized Biquaternionic Julia Sets Defined by Various Polynomials" Symmetry 15, no. 1: 43. https://doi.org/10.3390/sym15010043






