Applications of First-Order Differential Subordination for Subfamilies of Analytic Functions Related to Symmetric Image Domains
Abstract
:1. Introduction
- 1.
- is convex, or is starlike.
- 2.
- .
2. Subordination Results for the Class
- 1.
- for
- 2.
- for
- 3.
- for
- 4.
- for
- 5.
- for
- 6.
- for
- 1.
- for
- 2.
- for
- 3.
- for
- 4.
- for
- 5.
- for
- 6.
- for
- 1.
- for
- 2.
- for
- 3.
- for
- 4.
- for
- 5.
- for
- 6.
- for
- 1.
- for
- 2.
- for
- 3.
- for
- 4.
- for
- 5.
- for
- 6.
- for
- 1.
- for
- 2.
- for
- 3.
- for
- 4.
- for
- 5.
- for
- 6.
- for
- 1.
- for
- 2.
- for
- 3.
- for
- 4.
- for
- 5.
- for
- 6.
- for
3. Subordination Results for Class
- 1.
- for
- 2.
- for
- 3.
- for
- 4.
- for
- 5.
- for
- 6.
- for
- for
- for
- for
- for
- for
- for
- 1.
- for
- 2.
- for
- 3.
- for
- 4.
- for
- 5.
- for
- 6.
- for
- for
- for
- for
- for
- for
- for
- for
- for
- for
- for
- for
- for
- 1.
- for
- 2.
- for
- 3.
- for
- 4.
- for
- 5.
- for
- 6.
- for
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Khan, M.F.; Al-shbeil, I.; Khan, S.; Khan, N.; Haq, W.U.; Gong, J. Applications of a q-Differential Operator to a Class of Harmonic Mappings Defined by q-Mittag–Leffler Functions. Symmetry 2022, 14, 1905. [Google Scholar] [CrossRef]
- Ma, W.; Minda, D. A Unified Treatment of Some Special Classes of Univalent Functions. In Proceeding of Conference on Complex Analytic; Li, Z., Ren, F., Yang, L., Zhang, S., Eds.; International Press: New York, NY, USA, 1994; pp. 157–169. [Google Scholar]
- Janowski, W. Extremal problems for a family of functions with a positive real part and for some related families. Ann. Polonici Math. 1971, 23, 159–177. [Google Scholar] [CrossRef]
- Ur Rehman, M.S.; Ahmad, Q.Z.; Al-Shbeil, I.; Ahmad, S.; Khan, A.; Khan, B.; Gong, J. Coefficient Inequalities for Multivalent Janowski Type q-Starlike Functions Involving Certain Conic Domains. Axioms 2022, 11, 494. [Google Scholar] [CrossRef]
- Sokól, J.; Kanas, S. Radius of convexity of some subclasses of strongly starlike functions. Zesz. Nauk. Politech. Rzeszowskiej Mat. 1996, 19, 101–105. [Google Scholar]
- Cho, N.E.; Kumar, V.; Kumar, S.S.; Ravichandran, V. Radius problems for starlike functions associated with the sine function. Bull. Iran. Math. Soc. 2019, 45, 213–232. [Google Scholar] [CrossRef]
- Wani, L.A.; Swaminathan, A. Starlike and convex functions associated with a Nephroid domain. Bull. Malays. Math. Sci. Soc. 2021, 44, 79–104. [Google Scholar] [CrossRef]
- Mendiratta, R.; Nagpal, S.; Ravichandran, V. On a subclass of strongly starlike functions associated with an exponential function. Bull. Malays. Math. Sci. Soc. 2015, 38, 365–386. [Google Scholar] [CrossRef]
- Raina, R.K.; Sokól, J. On Coefficient estimates for a certain class of starlike functions, Hacettepe. J. Math. Statist. 2015, 44, 1427–1433. [Google Scholar]
- Kumar, S.S.; Arora, K. Starlike functions associated with a petal shaped domain. arXiv 2020, arXiv:2010.10072. [Google Scholar]
- Ali, R.M.; Cho, N.E.; Ravichandran, V.; Kumar, S.S. Differential subordination for functions associated with the lemniscate of Bernoulli. Taiwan. J. Math. 2012, 16, 1017–1026. [Google Scholar] [CrossRef]
- Kumar, S.S.; Kumar, V.; Ravichandran, V.; Cho, N.E. Sufficient conditions for starlike functions associated with the lemniscate of Bernoulli. J. Inequal. Appl. 2013, 176. [Google Scholar] [CrossRef]
- Kumar, S.; Ravichandran, V. Subordinations for functions with positive real part. Complex Anal. Oper. Theory 2018, 12, 1179–1191. [Google Scholar] [CrossRef]
- Paprocki, E.; Sokól, J. The extremal problems in some subclass of strongly starlike functions. Zeszyty Nauk. Politech. Rzeszowskiej Mat 1996, 20, 89–94. [Google Scholar]
- Raza, M.; Sokól, J.; Mushtaq, S. Differential subordinations for analytic functions. Iran. J. Sci. Technol. Trans. A Sci. 2019, 43, 883–890. [Google Scholar] [CrossRef]
- Shi, L.; Srivastava, H.M.; Khan, M.G.; Khan, N.; Ahmad, B.; Khan, B.; Mashwani, W.K. Certain subclasses of analytic multivalent functions associated with petal-shape domain. Axioms 2021, 10, 291. [Google Scholar] [CrossRef]
- Gupta, P.; Nagpal, S.; Ravichandran, V. Inclusion relations and radius problems for a subclass of starlike functions. arXiv 2020, arXiv:2012.1351v1. [Google Scholar]
- Gandhi, S. Radius estimates for three leaf function and convex combination of starlike functions. In Mathematical. Analysis 1: Approximation Theory. ICRAPAM; Deo, N., Gupta, V., Acu, A., Agrawal, P., Eds.; Springer: Singapore, 2018; Volume 306. [Google Scholar]
- Miller, S.S.; Mocanu, P.T. Differential Subordinations Theory and Its Applications; Series of Monographs and Textbooks in Pure and Applied Mathematics; Marcel Dekker Inc.: New York, NY, USA, 2000; Volume 225. [Google Scholar]
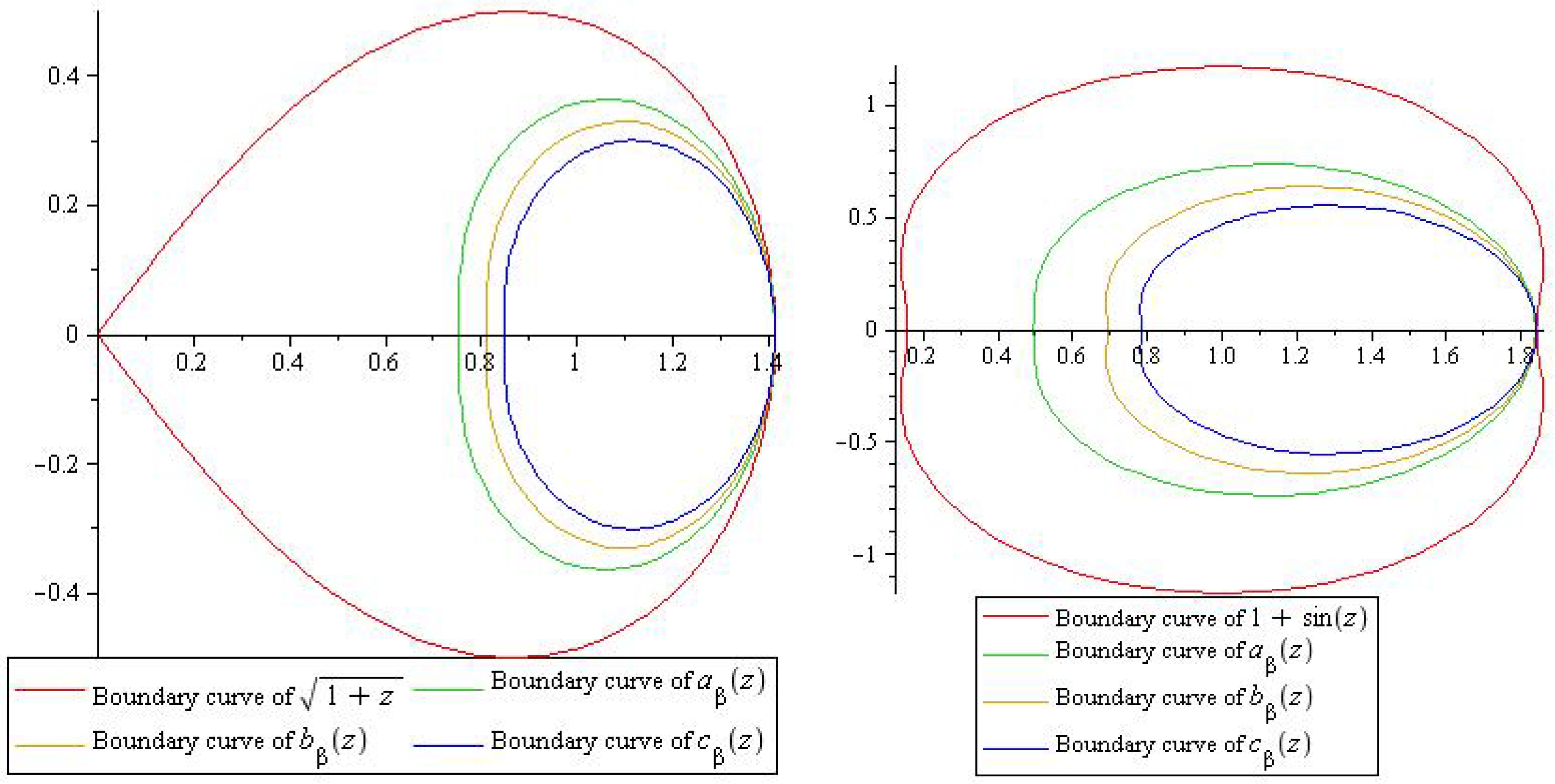
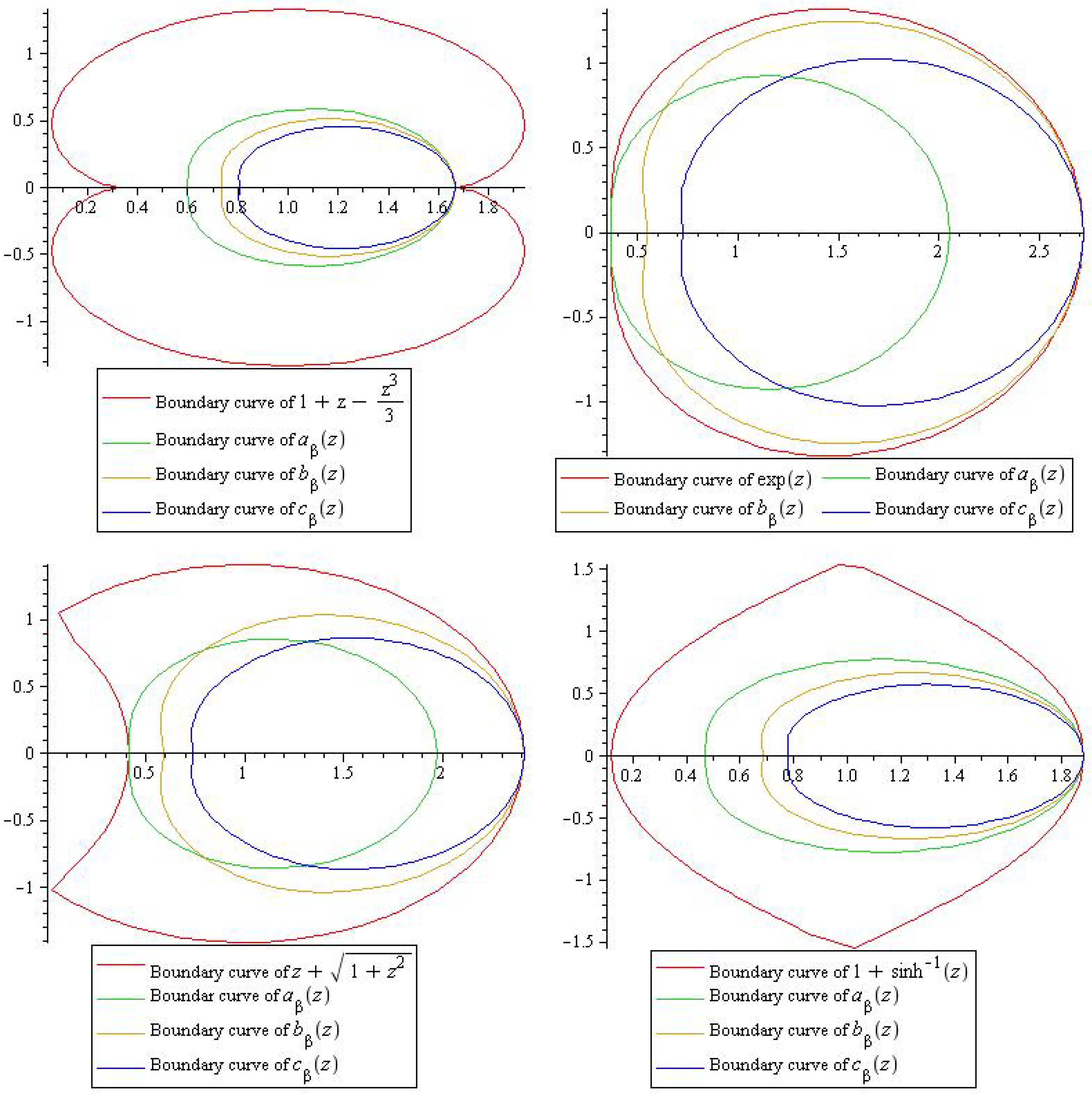
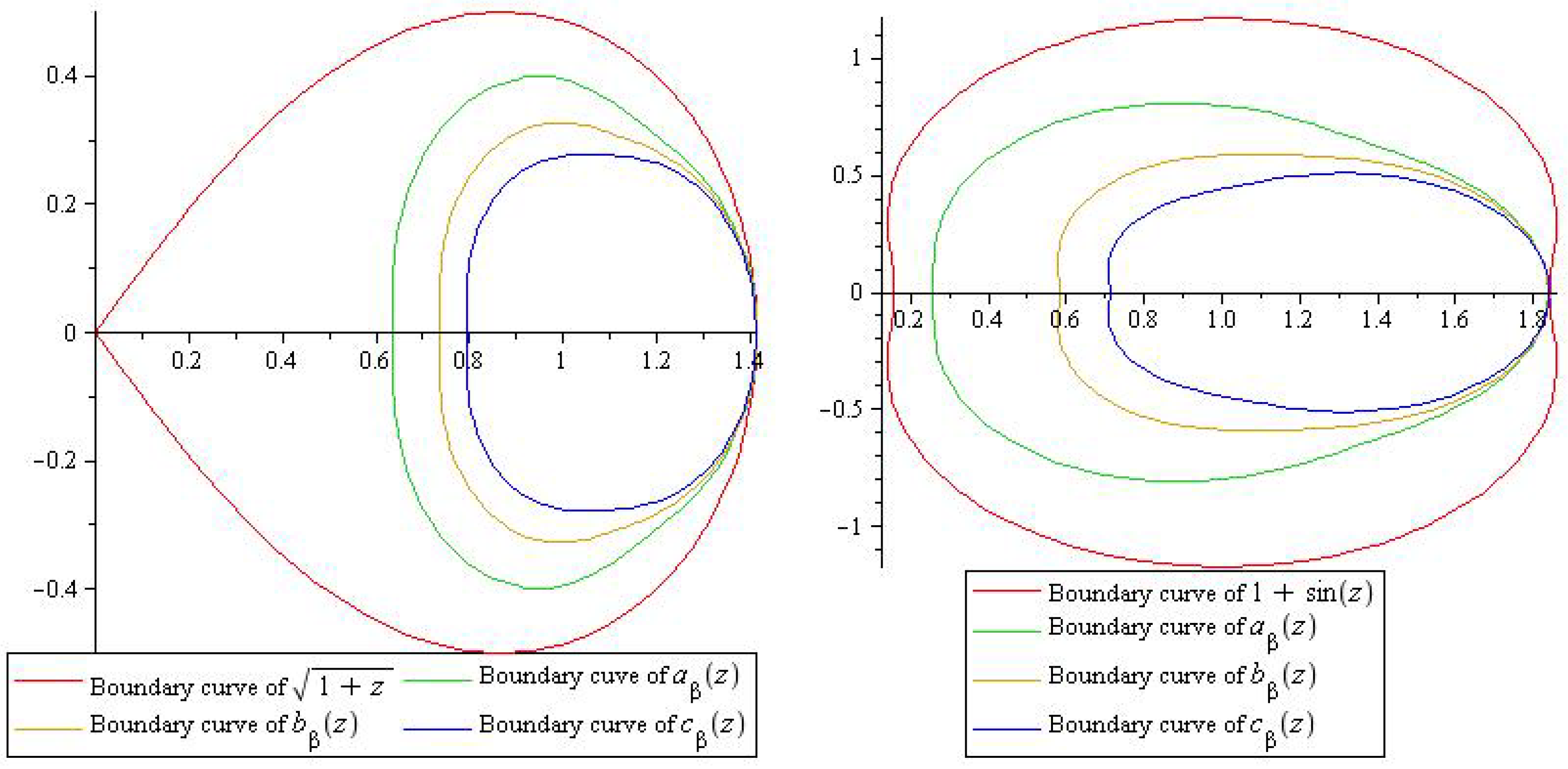

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, M.G.; Khan, B.; Gong, J.; Tchier, F.; Tawfiq, F.M.O. Applications of First-Order Differential Subordination for Subfamilies of Analytic Functions Related to Symmetric Image Domains. Symmetry 2023, 15, 2004. https://doi.org/10.3390/sym15112004
Khan MG, Khan B, Gong J, Tchier F, Tawfiq FMO. Applications of First-Order Differential Subordination for Subfamilies of Analytic Functions Related to Symmetric Image Domains. Symmetry. 2023; 15(11):2004. https://doi.org/10.3390/sym15112004
Chicago/Turabian StyleKhan, Muhammad Ghaffar, Bilal Khan, Jianhua Gong, Fairouz Tchier, and Ferdous M. O. Tawfiq. 2023. "Applications of First-Order Differential Subordination for Subfamilies of Analytic Functions Related to Symmetric Image Domains" Symmetry 15, no. 11: 2004. https://doi.org/10.3390/sym15112004





