Halo Orbits under Some Perturbations in cr3bp

Abstract

The general idea of this paper is to study the effect of mass variation of a test particle on periodic orbits in the restricted three-body model. In the circular restricted three-body problem (cr3bp), two bigger bodies (known as primary and secondary or sometime only primaries) are placed at either side of the origin on abscissa while moving in circular orbits around their common center of mass (here origin), while the third body (known as smallest body or infinitesimal body or test particle) is moving in space and varies its mass according to Jeans law. Using the Lindstedt–Poincaré method, we determine equations of motion and their solutions under various perturbations. The time-series and halo orbits around one of the collinear critical points of this model are drawn under the effects of the solar radiation pressure of the primary and the oblateness of the secondary. In general, these two dynamical properties are symmetrical.

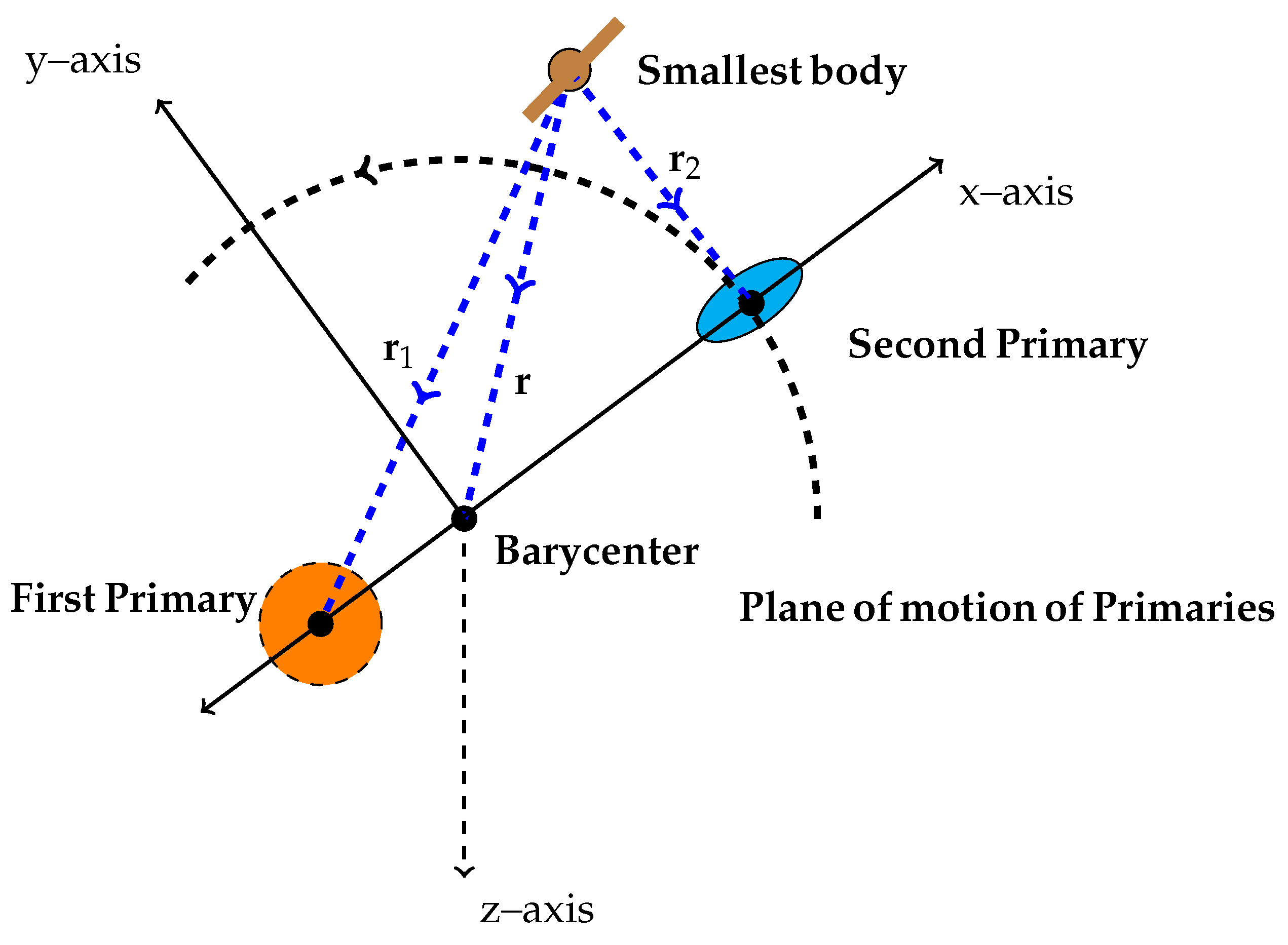
1. Introduction

The study of periodic orbits, which are symmetrical in general, of a test particle in the restricted problem frame is one of the most interesting research topics. Periodic orbits are investigated in the circular restricted three-body problem (cr3bp) and four or more body problems. The generalization of periodic orbits is known as “halo orbits”, which is actually performed in a three-dimensional around one of the collinear equilibrium points obtained in the restricted problem. Many scientists have investigated this problem as follows.

Farquhar et al. [1] conducted an investigation into the development of the halo orbits around the collinear points for the Sun–Earth system. Breakwell and Brown [2] performed the halo orbits in the restricted three-body problem and observed that as the orbits moved near to the Moon they become shorter, and in both cases a narrow band of stable orbits appeared roughly half-way to the Moon. Richardson [3] developed the halo orbits formula for the ISEE-3 mission. Howell [4] numerically investigated the halo orbits in the restricted three-body problem and found that the orbits increase in size as increases the mass ratio. Cielaszyk and Wie [5] developed a simple iterative numerical method to determine the halo orbits in a circular restricted three-body frame. Further starting with a first-order reference trajectory, they generated large, complex, and quasi-periodic Lissajous trajectories. Gomez et al. [6] studied the transfer between halo orbits of the same family by two methods: the first was the geometry of the phase space around these solutions, and the second was the Floquet theory for periodic orbits around the libration point ${\displaystyle L_1}$ of the Sun–Earth system.

Serban et al. [7] performed the trajectory correction maneuvers for a halo orbit mission to balance for the launch velocity errors produced by the launcher’s inaccuracies. Rahmani et al. [8] used the numerical method to control spacecrafts on periodic halo orbits around collinear equilibrium points in the restricted three-body problem. The control approach was based on the optimal control theory. Farres and Jorba [9] described the behavior of the trajectory of a solar sail when no control on the sail orientation was applied. Tiwary and Kushvah [10] extended the approach to find the Lissajous and the halo orbits around the collinear Lagrangian points in the restricted three-body problem where one of the primaries was taken as the Sun as a radiation source and other one was the Earth as an oblate body. Due to these effects, they observed that the time period of the orbit increases around ${\displaystyle L_1}$ and decreases around ${\displaystyle L_2}$. They also computed the halo orbits using the Lindstedt–Poincaré method.

Pal and Kushvah [11] studied the effect of solar wind drag, Poynting–Robertson drag, and radiation pressure in the restricted three-body problem frame. They used the perturbation technique to find the Lagrangian points and showed that the collinear Lagrangian points deviate from the line joining the primaries, while the triangular Lagrangian points remain unchanged. They observed that due to drag forces, the triangular Lagrangian points are unstable because in the classical case these triangular points are always stable for an interval of mass ratio. They also computed the halo orbits with the use of the Linstedt-Poincaré method. Bucciarelli et al. [12] investigated the influence of the various parameters used on the existence of bifurcations to halo orbits, both numerically and analytically, using qualitative techniques. Chidambararaj and Sharma [13] studied, numerically as well as analytically, the family of halo orbits in the three-dimensional photo-gravitational restricted three-body problem. They observed that due to radiation pressure and oblateness, the size and the orbital period of the halo orbit around ${\displaystyle L_1}$ increases.

Srivastava et al. [14] computed halo orbits around ${\displaystyle L_1}$ and ${\displaystyle L_2}$ by the analytic approximation of the solution. They used the Lindstedt–Poincaré method in the photo-gravitational circular restricted three-body problem frame. They noticed that the time period of the halo orbits increases around ${\displaystyle L_1}$ and ${\displaystyle L_2}$, accounting for oblateness and solar radiation pressure. They also observed that the stability of halo orbits is a weak function in view of the amplitude and the mass reduction factor. For any mission from the Earth, Nath and Ramanan [15] performed a design towards the halo orbits into two steps: firstly, one can chose a halo orbit for a specified size and then an optimal transfer trajectory design from the Earth parking orbit to the chosen halo orbit. Yingjing et al. [16] used an improved numerical method to perform the halo orbits and Lissajous orbits in the Earth–Moon system. Boudad et al. [17] illustrated the dynamics of resonance near the halo orbits in the bi-circular four-body model. Numerically, Sharma and SubbaRao [18] revealed the location of equilibrium points in the restricted three-body problem frame, when the bigger primary is taken as oblate.

Abdulraheem and Singh [19] investigated the existence of equilibrium points and their stability in the restricted three-body problem under the effect of many perturbations as oblateness of the primaries, solar radiation pressure, Coriolis, and centrifugal forces. They observed that there five equilibrium points exist, out of which three collinear equilibrium points are always unstable and two triangular equilibrium points are stable for some values of the mass ratio. Ceccaroni et al. [20] and Farres and Jorba [21] illustrated the halo orbits in the restricted problem. The periodic solutions using the Lindstedt–Poincaré method in the restricted problem have been studied by [22,23]. Ansari et al. [24] studied the hill problem under the quantized corrections.

Unlike the previous study, we have considered the variable mass of the smallest body. Since the mass of a celestial body varies (gain or loss) with time, this study may be useful to our research community. Some researchers have studied the restricted problem with variable mass. These studies are detailed below.

Refs. [25,26,27,28,29,30,31,32,33] studied the effect of variation of mass in the restricted problem. They found that due to mass-variation effects, all of the equilibrium points become unstable. They also made a numerical investigation for these models for the location of the equilibrium points, the regions of prohibited and allowed motion, the Poincaré surfaces of the section, and the basins of attraction.

Gravitationally bound three-body systems have been studied for hundreds of years and are common in our Galaxy. They show complex orbital interactions, which can constrain the compositions, masses, and interior structures of the bodies and test theories of gravity. Pulsars are famous as precise clocks in the areas of fundamental physics under extreme conditions ([34,35,36,37]), which are attributed to the exceptionally regular rotation and stable integrated pulse profiles. A triple system containing a millisecond pulsar PSR B 1620-26, a white dwarf, and a planetary-mass object in an orbit of several decades shows only weak interactions. Additionally, PSR J 0337+1715 was found to be a millisecond pulsar in a hierarchical triple system with two other stars. Highly accurate binary-pulsar timing plays an important role in the test of General Relativity.

Recently, Gao et al. [38] investigated the equilibrium equations of test particles in a Boson–Fermi system using the Newtonian approximation method. Fu et al. [39] performed a study of the scattering of test particles (Dirac spinors) in rotating spheroids. However, the above test particles are different from the test particles mentioned in this work theoretically (or conceptually). The mass of the former usually keeps constant (ignoring general relativistic effects), while the mass of the latter can vary, but both can be used to test gravitational properties.

The arrangement of the paper is as follows. The introduction of the paper is presented in Section 1. The equations of motion and its solutions are performed in Section 2, while Section 3 presents the numerical studies for time-series and halo orbits. Section 4 contains the conclusion.

2. Equations of Motion

As is commonly known, the restricted three-body problem consists of two massive bodies (the primaries) and a smaller body (infinitesimal body). We assume that the first primary is radiating with radiation parameter ${\displaystyle q}$ and the second primary is oblate in shape with oblateness factor ${\displaystyle A_2}$. We suppose that the equatorial plane of the oblate body coincides with the plane of motion (see Figure 1). Following the procedure adopted by [14] and shifting the origin to one of the critical points ${\displaystyle L_1}$ or ${\displaystyle L_2}$ (i.e., ${\displaystyle x={\displaystyle \frac{X+\mu \mp \gamma -1}{\gamma }}}$, ${\displaystyle y={\displaystyle \frac{Y}{\gamma }}}$ and ${\displaystyle z={\displaystyle \frac{Z}{\gamma }}}$, where ${\displaystyle \gamma }$ is the distance between the critical point and the second body ${\displaystyle m_2}$), we can write the equations of motion of the smallest body with constant mass as:

$$\begin{array}{ccc}\ddot{x}-2n\dot{y}\hfill & =& (n^2+2c_2)x+\frac{3}{2}{c}_3(2x^2-y^2-z^2)+2c_{4}x(2x^2-3y^2-3z^2),\hfill \\ \\ \ddot{y}+2n\dot{x}\hfill & =& (n^2-c_2)y-3c_{3}xy-\frac{3}{2}{c}_{4}y(4x^2-y^2-z^2),\hfill \\ \\ \ddot{z}\hfill & =& -c_{2}z-3c_{3}xz-\frac{3}{2}{c}_{4}z(4x^2-y^2-z^2),\hfill \end{array}$$

(1)

where

$$\begin{array}{ccc}{n}^2\hfill & =& 1+\frac{3}{2}{A}_2,m_1=1-\mu ,m_2=\mu \left(\mathrm{say}\right)\hfill \\ \\ c_m\hfill & =& \frac{1}{{\gamma }^3}\left\{{(\pm 1)}^m\left(\mu +\frac{3\mu A_2}{2{\gamma }^2}\right)+{(-1)}^m\frac{q(1-\mu ){\gamma }^{m+1}}{{(1\mp \gamma )}^{m+1}}\right\},m=0,1,2,3,4,\dots \hfill \end{array}$$

(2)

As we are interested in studying the effect of mass variation, we will suppose that the mass of the smallest body varies according to Jean’s law. Hence, we will use the method given by [40]. Then, we can write the equations of motion by the assumption that the variation of mass originates from one point and has negligible momenta as:

$$\begin{array}{ccccc}\ddot{x}-2n\dot{y}\hfill & +& {\displaystyle \frac{\dot{m}}{m}}(\dot{x}-ny)\hfill & =& (n^2+2c_2)x+\frac{3}{2}{c}_3(2x^2-y^2-z^2)\hfill \\ \\ \hfill & & & & +2c_{4}x(2x^2-3y^2-3z^2),\hfill \\ \\ \ddot{y}+2n\dot{x}\hfill & +& {\displaystyle \frac{\dot{m}}{m}}(\dot{y}+nx)\hfill & =& (n^2-c_2)y-3c_{3}xy-\frac{3}{2}{c}_{4}y(4x^2-y^2-z^2),\hfill \\ \\ \ddot{z}\hfill & +& {\displaystyle \frac{\dot{m}}{m}}\dot{z}\hfill & =& -c_{2}z-3c_{3}xz-\frac{3}{2}{c}_{4}z(4x^2-y^2-z^2).\hfill \end{array}$$

(3)

Since we assume that we are in variable mass case, we will use Jean’s law [41] and Meshcherskii space-time transformations [42] given by:

$$\begin{array}{ccc}m\hfill & =& m_0{e}^{-{\alpha }_{1}t},\hfill \\ \\ (x,y,z)\hfill & =& {\alpha }_2^{-1/2}(\xi ,\eta ,\zeta ).\hfill \end{array}$$

(4)

The velocity and the acceleration components can be written as:

$$\begin{array}{ccc}(\dot{x},\dot{y},\dot{z})\hfill & =& {\alpha }_2^{-1/2}\left[\left(\dot{\xi }+{\displaystyle \frac{{\alpha }_1}{2}}\xi \right),\left(\dot{\eta }+{\displaystyle \frac{{\alpha }_1}{2}}\eta \right),\left(\dot{\zeta }+{\displaystyle \frac{{\alpha }_1}{2}}\zeta \right)\right],\hfill \\ (\ddot{x},\ddot{y},\ddot{z})\hfill & =& {\alpha }_2^{-1/2}\left[\left(\ddot{\xi }+{\alpha }_1\dot{\xi }+{\displaystyle \frac{{\alpha }_1^2}{4}}\xi \right),\left(\ddot{\eta }+{\alpha }_1\dot{\eta }+{\displaystyle \frac{{\alpha }_1^2}{4}}\eta \right),\left(\ddot{\zeta }+{\alpha }_1\dot{\zeta }+{\displaystyle \frac{{\alpha }_1^2}{4}}\zeta \right)\right],\hfill \end{array}$$

(5)

where ${\displaystyle {\alpha }_1}$ is variation constant and ${\displaystyle {\alpha }_2={\displaystyle \frac{m}{m_0}}}$.

Utilizing Equations (3)–(5), we obtain

$$\begin{array}{ccc}\ddot{\xi }-2n\dot{\eta }\hfill & =& V_1,\hfill \\ \\ \ddot{\eta }+2n\dot{\xi }\hfill & =& V_2,\hfill \\ \\ \ddot{\zeta }\hfill & =& V_3.\hfill \end{array}$$

(6)

To solve these equations, we will use the successive approximation method where some secular terms arise. To avoid these secular terms, we will introduce a frequency connection ${\displaystyle \omega }$ and a new time-independent variable ${\displaystyle \tau }$ by the relation ${\displaystyle \tau =\omega t}$. The equations of motion become:

$$\begin{array}{ccc}{\omega }^2{\xi }^{″}-2n\omega {\eta }^{\prime }\hfill & =& V_1,\hfill \\ \\ {\omega }^2{\eta }^{″}+2n\omega {\xi }^{\prime }\hfill & =& V_2,\hfill \\ \\ {\omega }^2{\zeta }^{″}\hfill & =& V_3.\hfill \end{array}$$

(7)

where

$$\begin{array}{ccc}{V}_1\hfill & =& \frac{1}{4}{\alpha }_1^2\xi +(n^2+2c_2)\xi +\frac{3}{2}{c}_3{\alpha }_2^{-1/2}(2{\xi }^2-{\eta }^2-{\zeta }^2)+2c_4{\alpha }_2^{-1}\xi (2{\xi }^2-3{\eta }^2-3{\zeta }^2),\hfill \\ \\ V_2\hfill & =& \frac{1}{4}{\alpha }_1^2\eta +(n^2-c_2)\eta -3c_3{\alpha }_2^{-1/2}\xi \eta -\frac{3}{2}{c}_4{\alpha }_2^{-1}\eta (4{\xi }^2-{\eta }^2-{\zeta }^2),\hfill \\ \\ V_3\hfill & =& \frac{1}{4}{\alpha }_1^2\zeta -c_2\zeta -3c_3{\alpha }_2^{-1/2}\xi \zeta -\frac{3}{2}{c}_4{\alpha }_2^{-1}\zeta (4{\xi }^2-{\eta }^2-{\zeta }^2).\hfill \end{array}$$

(8)

To find the better approximate solutions for the nonlinear system in the neighborhood of equilibrium points, we will use the perturbation techniques of Lindstedt–Poincaré by assuming that the solutions are of the form: ${\displaystyle \xi \left(\tau \right)=\sum _{s=1}^{\infty }{ϵ}^s{\xi }_s\left(\tau \right),}$${\displaystyle \eta \left(\tau \right)=\sum _{s=1}^{\infty }{ϵ}^s{\eta }_s\left(\tau \right),}$${\displaystyle \zeta \left(\tau \right)=\sum _{s=1}^{\infty }{ϵ}^s{\zeta }_s\left(\tau \right)}$ and also ${\displaystyle \omega =1+\sum _{s=1}^{\infty }{ϵ}^s{\omega }_s}$.

By putting these values in Equation (7) and equating the coefficients of ascending powers of ${\displaystyle ϵ}$ to zero, the coefficients of ${\displaystyle ϵ}$ will be

$$\begin{array}{ccc}{\xi }_1^{″}-2n{\eta }_1^{\prime }-B_1{\xi }_1\hfill & =& 0,\hfill \\ \\ {\eta }_1^{″}+2n{\xi }_1^{\prime }-B_2{\eta }_1\hfill & =& 0,\hfill \\ \\ {\zeta }_1^{″}+B_3{\zeta }_1\hfill & =& 0.\hfill \end{array}$$

(9)

Following the procedure given in [43], the approximate solution of Equation (9) will be

$$\begin{array}{ccc}{\xi }_1\hfill & =& -B_{\xi }\cos {\tau }_1,\hfill \\ \\ {\eta }_1\hfill & =& k{B}_{\xi }\sin {\tau }_1,\hfill \\ \\ {\zeta }_1\hfill & =& B_{\zeta }\sin {\tau }_2.\hfill \end{array}$$

(10)

where ${\displaystyle B_{\xi }}$ and ${\displaystyle B_{\zeta }}$ are arbitrary constants.

The coefficients of ${\displaystyle {ϵ}^2}$ after setting ${\displaystyle {\omega }_1=0}$ will be

$$\begin{array}{ccc}{\xi }_2^{″}-2n{\eta }_2^{\prime }-B_1{\xi }_2\hfill & =& B_4+B_5\cos 2{\tau }_1+B_6\cos 2{\tau }_2,\hfill \\ \\ {\eta }_2^{″}+2n{\xi }_2^{\prime }-B_2{\eta }_2\hfill & =& D_1\sin 2{\tau }_1,\hfill \\ \\ {\zeta }_2^{″}+B_3{\zeta }_2\hfill & =& E_1\sin ({\tau }_1+{\tau }_2)+E_1\sin ({\tau }_2-{\tau }_1).\hfill \end{array}$$

(11)

The approximate solution of Equation (11) will be

$$\begin{array}{ccc}{\xi }_2\hfill & =& B_{20}+B_{21}\cos 2{\tau }_1+B_{22}\cos 2{\tau }_2,\hfill \\ \\ {\eta }_2\hfill & =& D_{21}\sin 2{\tau }_1+D_{22}\sin 2{\tau }_2,\hfill \\ \\ {\zeta }_2\hfill & =& E_{21}\sin ({\tau }_2+{\tau }_1)+E_{22}\sin ({\tau }_2-{\tau }_1).\hfill \end{array}$$

(12)

The coefficients of ${\displaystyle {ϵ}^3}$ (by assuming ${\displaystyle {\tau }_1={\tau }_2}$) will be

$$\begin{array}{ccc}{\xi }_3^{″}-2n{\eta }_3^{\prime }-B_1{\xi }_3\hfill & =& {\delta }_1\cos 3{\tau }_1+{\delta }_2\cos {\tau }_1,\hfill \\ \\ {\eta }_3^{″}+2n{\xi }_3^{\prime }-B_2{\eta }_3\hfill & =& {\delta }_3\sin 3{\tau }_1+{\delta }_4\sin {\tau }_1,\hfill \\ \\ {\zeta }_3^{″}+B_3{\zeta }_3\hfill & =& \left\{\begin{array}{cc}{(-1)}^{i/2}{\delta }_5\sin 3{\tau }_1,\hfill & i=0,2;\hfill \\ {(-1)}^{(i-1)/2}{\delta }_5\cos 3{\tau }_1,\hfill & i=1,3.\hfill \end{array}\right.\hfill \end{array}$$

(13)

The approximate solution of Equation (13) will be

$$\begin{array}{ccc}{\xi }_3\hfill & =& B_{31}\cos 3{\tau }_1+B_{32}\cos {\tau }_1,\hfill \\ \\ {\eta }_3\hfill & =& D_{31}\sin 3{\tau }_1+D_{32}\sin {\tau }_1,\hfill \\ \\ {\zeta }_3\hfill & =& \left\{\begin{array}{cc}{(-1)}^{i/2}{E}_{31}\sin 3{\tau }_1,\hfill & i=0,2;\hfill \\ {(-1)}^{(i-1)/2}{E}_{32}\cos 3{\tau }_1,\hfill & i=1,3.\hfill \end{array}\right.\hfill \end{array}$$

(14)

Finally, the approximate solution of Equation (7) (by assuming ${\displaystyle {\tau }_1=\tau }$) will be

$$\begin{array}{ccc}\xi \left(\tau \right)\hfill & =& B_{20}+(B_{32}-B_{\xi })\cos \tau +(B_{21}+B_{22})\cos 2\tau +B_{31}\cos 3\tau ,\hfill \\ \\ \eta \left(\tau \right)\hfill & =& (k{B}_{\xi }+D_{32})\sin \tau +(D_{21}+D_{22})\sin 2\tau +D_{31}\sin 3\tau ,\hfill \\ \\ \zeta \left(\tau \right)\hfill & =& \left\{\begin{array}{cc}{(-1)}^{i/2}\{B_{\zeta }\sin \tau +E_{21}\sin 2\tau +E_{31}\sin 3\tau \},\hfill & i=0,2;\hfill \\ \\ {(-1)}^{(i-1)/2}\{B_{\zeta }\cos \tau +E_{21}\cos 2\tau +E_{22}+E_{32}\cos 3\tau \},\hfill & i=1,3.\hfill \end{array}\right.\hfill \end{array}$$

(15)

All constants and coefficients are given in Appendix A.

3. Numerical Studies

In this section, we numerically performed the time series and halo orbits for various parameters by using Equation (15). Here, we took the following numerical values of the constants as ${\displaystyle \mu =0.004}$, ${\displaystyle A_2=0.002}$, ${\displaystyle q=0.99}$, ${\displaystyle \gamma =0.107715}$, ${\displaystyle {\alpha }_1=0.2}$, ${\displaystyle {\alpha }_2=0.4}$, ${\displaystyle B_{\xi }}$ = 206,000,000 and ${\displaystyle B_{\zeta }}$ = 110,000,000 (see [14]). The dynamical behavior explains the dynamical motion of the third body given in Figure 2, Figure 3 and Figure 4. In all these figures, the sub-figures represented by (a) are for different values of the mass parameter ${\displaystyle {\alpha }_2}$ (=0.4 (blue), 0.8 (red), and 1 (black)), the sub-figures represented by (b) are for different values of the oblateness parameter ${\displaystyle A_2}$ (=0 (blue), 0.002 (red), and 0.005 (black)), the sub-figures represented by (c) are for the different values of ${\displaystyle \gamma }$ (=0.107715 (blue), 0.157715 (red), and 0.207715 (black)) and the sub-figures represented by (d) are for different values of the solar radiation factor ${\displaystyle q}$ (=0.75 (blue), 0.85 (red), and 0.95 (black)).

3.1. Time Series

In this subsection, we will show the variation of the amplitude and phase angle towards both the axes ${\displaystyle \xi }$ and ${\displaystyle \eta }$ with time that we presented in Figure 2 and Figure 3, respectively. Figure 2a,c show that as we increase the values of ${\displaystyle {\alpha }_2}$ and ${\displaystyle \gamma }$, the amplitude decreases, while Figure 2b shows that as we increase the value of ${\displaystyle A_2}$, the amplitude increases. Additionally, by increasing the value of ${\displaystyle q}$, there is negligible change in the amplitude, as shown in the Figure 2d.

While Figure 3 shows 90 degrees phase angle from Figure 2, and Figure 3a–c show that as we increase the values of ${\displaystyle {\alpha }_2}$, ${\displaystyle A_2}$, and ${\displaystyle \gamma }$, the amplitude changes randomly and the value of ${\displaystyle q}$ increases in Figure 3d, there is negligible change in the amplitude. Over all, these parameters affected the amplitude.

3.2. Halo Orbits

These orbits explain some facts of the dynamical motion of the third body. Figure 4 represents the halo orbits around one of the collinear critical points, i.e., either ${\displaystyle L_1}$ or ${\displaystyle L_2}$ for various values of the parameters used and given in the Figure 4a–d. We observed significant changes in Figure 4a–c, while there is no significant change in Figure 4d (i.e., due to solar radiation pressure).

4. Conclusions

The mass-variation effects of the smallest body in the restricted problem are investigated by shifting the origin to one of the collinear critical points under the effects of radiation of the primary and the oblateness of the secondary. We used Jean’s law and Meshcherskii space time transformation to evaluate the equations of motion, which clearly depend on the mass variation parameters and the other perturbations parameters used. We then evaluated the solutions of these equations by using the Lindstedt–Poincaré method. Finally, we illustrated the time-series and halo orbits for the various values of the parameters introduced. For the time-series, we observed that the amplitude varies with the variation of these parameters. Further, we drew the halo orbits and found that they are periodic but change their shapes and sizes with the variation of the parameters. In all of the cases, solar radiation factor ${\displaystyle q}$ has no significant effect.

Finally, we think that using halo orbits, scientists can regularly and simultaneously see the Earth and the dark side of the Moon in the Earth–Moon system. They can design interplanetary trajectories for any interplanetary mission. We also think that space agencies can launch various satellite around collinear Lagrangian points in the Sun–Earth system to investigate the solar corona.