Multiple-Attribute Decision Making Based on Intuitionistic Hesitant Fuzzy Connection Set Environment

Abstract

The intuitionistic hesitant fuzzy set (IHFS) is an enriched version of hesitant fuzzy sets (HFSs) that can cover both fuzzy sets (FSs) and intuitionistic fuzzy sets (IFSs). By assigning membership and non-membership grades as subsets of [0, 1], the IHFS can model and handle situations more proficiently. Another related theory is the theory of set pair analysis (SPA), which considers both certainties and uncertainties as a cohesive system and represents them from three aspects: identity, discrepancy, and contrary. In this article, we explore the suitability of combining the IHFS and SPA theories in multi-attribute decision making (MADM) and present the hybrid model named intuitionistic hesitant fuzzy connection number set (IHCS). To facilitate the design of a novel MADM algorithm, we first develop several averaging and geometric aggregation operators on IHCS. Finally, we highlight the benefits of our proposed work, including a comparative examination of the recommended models with a few current models to demonstrate the practicality of an ideal decision in practice. Additionally, we provide a graphical interpretation of the devised attempt to exhibit the consistency and efficiency of our approach.

1. Introduction

The technique for picking the most acceptable alternative based on the provided criteria is known as decision making (DM). Various DM procedures are originated via one feature only, but most often, these are dependent upon multiple attributes. The area of DM is then called multiple-attribute DM (MADM). MADM is a highly crucial testing domain, which helps choose the correct option associated with several leading features [1,2,3]. Mainly, the DM uses crisp figures to describe the preferences concerning the choices in usual MADM problems. Nevertheless, due to the inadequacy of statistics, shortage of time, and lack of data and quality values, fuzzy values may be used to specify the preferences exclusively for the attribute values.

The idea of fuzzy sets (FSs) was introduced by Zadeh [4] in 1965 and, after they have come into existence, several researchers have applied them to solve various problems and developed symmetrical decision models [5,6,7]. Every fuzzy set has a pair of each component with a function of membership, where this function provides a membership grade in $[0,1]$. Later, several extensions of this fuzzy set have been proposed by the researchers. Atanassov [8,9] proposed an intuitionistic fuzzy set (IFS) in 1986. The IFS expresses ambiguous and complex information with the aid of the membership as well as non-membership grades, where the sum of both grades cannot exceed 1. The IFS has been getting a lot of attention since its initiation. For instance, Garg and Arora [10] stated a decision-making algorithm for the DMPs (“decision-making problems”) with “interval-valued intuitionistic fuzzy soft set” features. Zeng et al. [11] defined the generalized probabilistic ordered operators for DMPs. Eom and Lee [12] elaborated the intuitionistic fuzzy theta-compact space. In Refs. [13,14], the authors have defined an application of the intuitionistic fuzzy pairs to solve the different DMPs. Bustince and Burillo [15] and Park et al. [16] defined the correlation coefficients for an interval-valued IFS. Atanassov [17] stated some novel topological operators over the IFS. In terms of ranking the different pairs of IFSs, several researchers have defined various measures such as the accuracy function [18], similarity measures [19], and grey relational analysis [20]-based algorithms for DMPs. Jiang et al. [21] defined the distance measures based on the transformation techniques of IFSs and their application to the “pattern recognition” problems. In [22,23], the authors have defined some generalized aggregation operators to aggregate the different pairs of IFSs and their applications to the DMPs.

In the above-mentioned literature, all the studies considered the input as a real number. However, to address the uncertainties in a more generalized manner, Torra [24,25] presented the concept of the hesitant fuzzy set (HFS). An HFS agrees to the membership grade holding a set of possible values of the interval from 0 to 1. An HFS is also an expanded form of the FS. The idea of the HFS is broadly applied in several complications. Most scholars performed a critical investigation on HF data accumulation procedures and their effects in DMPs. For instance, Mahmood et al. [26] defined the aggregation operators of the pairs of the bipolar-valued hesitant fuzzy set to solve the DMPs. Mockor et al. [27] explored the relationship of HFSs with some generalized form of fuzzy sets. Alcantud [28] ranked the different pairs of HFSs for multi-agent decisions. Zhang et al. [29] presented an extended LINMAP method with HFS information for green-supplier selection problems. Xia et al. [30] stated some operators for HFSs. Shaheen et al. [31] defined the concept of hesitant fuzzy rough sets. Sun and Ouyang [32] stated a TOPSIS-based decision-making algorithm for the DMPs. Ni et al. [33] stated a projection method for DMPs using the dual HFS. Liu and Sun [34] proposed generalized power operators for DMPs using the pairs of HFSs. Tahir et al. [35] described the concept of an IHFS (“intuitionistic hesitant fuzzy set”) which is a fusion of the IFS and the HFS. In the IHFS, the grades reflect in the form of a set of possible values from $[0,1]$. Admittedly, the IHFS has developed as a potent tool for explaining the fuzziness of the DM complexities.

Aside from the aforementioned ideas, Zhao [36] suggested another approach, recognized as set pair analysis (SPA), to cope with uncertainty. This concept harmonizes the system of assurance and apprehension in a specific evaluation. The most important part of this concept is the CN (“connection number”); it is split into three kinds of senses: identity, inconsistency, and opposition. The fundamental properties of the CNs are defined by Jiang et al. [37]. Later, several researchers showed interest in explaining DM difficulties using set pair analysis (SPA) [38,39,40]. However, Kumar and Garg [41,42] outlined the different formulae of connection numbers to resolve the DM problems applying the TOPSIS method. Zhang [43] developed the relationship between fuzzy sets and the theory of SPA. After considering the relationship between both theories, Hu et al. [44] defined a dynamic stochastic multiple-attribute DM course. Jia et al. [45] determined the distance measures for MADM based on CNs.

The two foremost steps involved in MADM are, firstly, the aggregation phase to determine the total value of the selected option by combining the given performance values of all the attributes through the suitable integration process; and, secondly, the manipulation phase for choosing the best option. In this paper [46], the authors employ many approaches to support the MD process, such as a combination of the DEA window analysis with the Malmquist index approach to assess the efficiency of the cybersecurity industry, and a framework based on multicriteria decision making (MCDM) is provided that integrates the spherical fuzzy analytical hierarchical process (SF-AHP) and the grey complex proportional assessment (G-COPRAS), in which spherical fuzzy sets and grey numbers are used to express the ambiguous linguistic evaluation statements of experts [47]. For the aggregation phase, during the last twenty years, experts have worked hard to develop aggregation operators, used to tackle DM difficulties. For example, Xu and Yager [48] established a few fundamental geometric aggregation (GA) operators named the IF-WGA operator and IF-OWG operator, which are applied for DM based upon the intuitionistic fuzzy set. Zhang [49] established some aggregation operators and aggregated the hesitant fuzzy environment. Yager [50] demonstrated a power aggregation operator and confirmed its role in MADM. Ahmmad et al. [51] used the Aczel–Alsina Average aggregation operator for medical diagnosis. Mehmood et al. [52] produced the Hamacher Choquet-integral aggregation operators for ranking. Moreover, Tahir et al. [35] established some AOs for IHFSs and PHFSs for decision making.

The above-described aggregation operators are extensively applied to aggregate the preference values for getting the best results. However, during the research, these operators exceeded the association between the data provided. Through research, we have explored the idea that the CN of the theory of SPA can be applied extensively for MADM. Considering this aspect, we define the intuitionistic hesitant fuzzy connection number set (IHCS) by merging the prominent features of the IHFS and the SPA, and the applications of fuzzy logic in different fields should also be mentioned in the literature review. The related works were suggested as follows [53,54,55,56]. In addition, this article establishes a sequence of aggregation operators. The inspiration and attainments of this paper are presented below:

• The origination of some PA operators, such as the IHCPA, IHCPWA, IHCPOWA, IHCPHA, IHCPG, IHCPWG, IHCPOWG, and IHCPHG operators, and verifying their properties;
• Proposing a novel MADM technique that involves the developed operations;
• Furnishing specific mathematical examples to validate the consistency and supremacy of the presented approach.

The rest of the article is organized as follows: In Section 2, we briefly overview the concept of the HFS and SPA theory. In Section 3, we defined the concept of IHFCNS (“intuitionistic hesitant fuzzy connection number set”) and stated its properties. In Section 4, we proposed an MADM algorithm with the stated operators to address the DMPs. To validate the performance of the proposed algorithm, a numerical example is given in Section 5. Finally, a conclusion is drawn in Section 6. The detailed flow chart of the proposed study is shown in Figure 1.

2. Preliminaries

This section includes a few fundamental concepts related to the present studies on the intuitionistic hesitant fuzzy set and the theory of SPA.

Definition 1

([35]). The supporting mappings for an IHFS are $\varphi$, $\psi$: $\mathcal{F}\to \left[0,1\right].$With the aid of these mappings, IHFS ${\mathcal{Z}}_i$ over $\mathcal{F}$ is described as below:

$${\mathcal{Z}}_i=\left\{\left({\varsigma }_i,{\varphi }_{\mathcal{Z}}\left({\varsigma }_i\right),{\psi }_{\mathcal{Z}}\left({\varsigma }_i\right)\right)|{\varsigma }_i\in \mathcal{F}\right\}$$

(1)

where $0\le \mathit{max}\left({\varphi }_{\mathcal{Z}}\left({\varsigma }_i\right)\right)+\mathit{max}\left({\psi }_{\mathcal{Z}}\left({\varsigma }_i\right)\right)\le 1$. ${\varphi }_{\mathcal{Z}}\left({\varsigma }_i\right)$ and ${\psi }_{\mathcal{Z}}\left({\varsigma }_i\right)$ are collections of certain amounts in $\left[0, 1\right]$, representing the membership grades and non-membership grades of the component ${\varsigma }_i\in \mathcal{F}$.

Remark 1.

• For each ${\varsigma }_i\in \mathcal{F}$, ${\mathcal{Z}}_i=({\varphi }_{\mathcal{Z}}\left({\varsigma }_i\right),{\psi }_{\mathcal{Z}}\left({\varsigma }_i\right))$ is the IHFE.
• It is assumed that the largest element will be repeated to make the lengths of the two IHFEs the same in the IHFSs.
• The elements in the IHFSs will be arranged in ascending order for comparison purposes.

Definition 2

([35]). For IHFS  $\mathcal{Z}=\left({\varphi }_{\mathcal{Z}},{\psi }_{\mathcal{Z}}\right)$, the score function ($\mathcal{S}c\left(\mathcal{Z}\right)$ ) as well as accuracy function ($\mathcal{T}\left(\mathcal{Z}\right)$) are expressed as:

$$\mathcal{S}c\left(\mathcal{Z}\right)=\frac{\mathcal{S}c\left({\varphi }_{\mathcal{Z}}\right)-\mathcal{S}c\left({\psi }_{\mathcal{Z}}\right)}{2},\mathcal{S}c\left(\mathcal{Z}\right)\in \left[-1, 1\right]$$

$$\mathcal{T}\left(\mathcal{Z}\right)=\frac{\mathcal{S}c\left({\varphi }_{\mathcal{Z}}\right)+\mathcal{S}c\left({\psi }_{\mathcal{Z}}\right)}{2},\mathcal{T}\left(\mathcal{Z}\right)\in \left[0, 1\right]$$

where $\mathcal{S}c\left({\varphi }_{\mathcal{Z}}\right)=\frac{sum of all elements in \left({\varphi }_{\mathcal{Z}}\right)}{order of \left({\varphi }_{\mathcal{Z}}\right)}$ and $\mathcal{S}c\left({\psi }_{\mathcal{Z}}\right)=\frac{sum of all elements in \left({\psi }_{\mathcal{Z}}\right)}{order of \left({\psi }_{\mathcal{Z}}\right)}$.

Definition 3

([35]). An IHFS ${\mathcal{Z}}_1$ will be preferred over ${\mathcal{Z}}_2$, denoted by ${\mathcal{Z}}_1>{\mathcal{Z}}_2$, based upon the following criteria:

(i)

$\mathcal{S}c\left({\mathcal{Z}}_1\right)>\mathcal{S}c\left({\mathcal{Z}}_2\right)$;

(ii)

$\mathcal{S}c\left({\mathcal{Z}}_1\right)=\mathcal{S}c\left({\mathcal{Z}}_2\right)$ and $\mathcal{T}\left({\mathcal{Z}}_1\right)>\mathcal{T}\left({\mathcal{Z}}_2\right)$

Definition 4

([35]). The intuitionistic hesitant fuzzy power average and geometric operators are denoted and defined, respectively, as follows:

$$IHFPA({\mathcal{Z}}_1{\mathcal{Z}}_2\dots .,{\mathcal{Z}}_n)$$

$$=\bigcup _{\begin{array}{c}{\mathrm{h}}_{\mathrm{i}}\mathrm{\epsilon }{\mathsf{\varphi }}_{\mathrm{i}}\\ {\mathrm{f}}_{\mathrm{i}}\mathrm{\epsilon }{\mathsf{\psi }}_{\mathrm{i}}\end{array}}\left(1-\prod _{\mathrm{i}=1}^{\mathrm{n}}(1-{\left({\mathrm{h}}_{\mathrm{i}}\right)}^{\frac{\left(1+\mathrm{T}\left({\mathcal{Z}}_{\mathrm{i}}\right)\right)}{{\sum }_{\mathrm{i}=1}^{\mathrm{n}}\left(1+\mathrm{T}\left({\mathcal{Z}}_{\mathrm{i}}\right)\right)}}),\prod _{\mathrm{i}=1}^{\mathrm{n}}{\left({\mathrm{f}}_{\mathrm{i}}\right)}^{\frac{\left(1+\mathrm{T}\left({\mathcal{Z}}_{\mathrm{i}}\right)\right)}{{\sum }_{\mathrm{i}=1}^{\mathrm{n}}\left(1+\mathrm{T}\left({\mathcal{Z}}_{\mathrm{i}}\right)\right)}}\right)$$

and

$$IHFPG ({\mathcal{Z}}_1{\mathcal{Z}}_2\dots .,{\mathcal{Z}}_n)$$

$$=\bigcup _{\begin{array}{c}{\mathrm{h}}_{\mathrm{i}}\mathrm{\epsilon }{\mathsf{\varphi }}_{\mathrm{i}}\\ {\mathrm{f}}_{\mathrm{i}}\mathrm{\epsilon }{\mathsf{\psi }}_{\mathrm{i}}\end{array}}\left(\prod _{\mathrm{i}=1}^{\mathrm{n}}{\left({\mathrm{h}}_{\mathrm{i}}\right)}^{\frac{\left(1+\mathrm{T}\left({\mathcal{Z}}_{\mathrm{i}}\right)\right)}{\sum _{\mathrm{i}=1}^{\mathrm{n}}\left(1+\mathrm{T}\left({\mathcal{Z}}_{\mathrm{i}}\right)\right)}},1-\prod _{\mathrm{i}=1}^{\mathrm{n}}(1-{\left({\mathrm{f}}_{\mathrm{i}}\right)}^{\frac{\left(1+\mathrm{T}\left({\mathcal{Z}}_{\mathrm{i}}\right)\right)}{\sum _{\mathrm{i}=1}^{\mathrm{n}}\left(1+\mathrm{T}\left({\mathcal{Z}}_{\mathrm{i}}\right)\right)}})\right)$$

where

$$T\left({\mathcal{Z}}_i\right)=\bigcup _{\begin{array}{c}{\varphi }_i\epsilon {\mathcal{Z}}_i\\ {\psi }_i\epsilon {\mathcal{Z}}_i\end{array}}\left(\sum _{\begin{array}{c}i=1\\ i\ne j\end{array}}^{n}Sup\left({\mathcal{Z}}_i,{\mathcal{Z}}_j\right)\right)$$

The theory of SPA is based on a pair of sets that are interrelated and unified under a certain construction. The examples may include the system and surroundings, the system and engineering, military problems, state security, etc. SPA analyzes the common attributes and establishes a connection degree ($\mathcal{J}=\alpha +\beta i+\gamma j$) of two such sets according to the given settings. The theory is further extended for more than two sets.

Definition 5

([36]). The connection degree is defined as

$$\mathcal{J}=\frac{\mathit{S}}{\mathit{N}}+\frac{\mathit{F}}{\mathit{N}}\mathit{i}+\frac{\mathit{P}}{\mathit{N}}\mathit{j}$$

$$\mathcal{J}=\alpha +\beta i+\gamma$$

(2)

where N represents “all attributes”, S represents “identity attributes”, P represents “contrary attributes”, and $\mathit{F}=\mathit{N}-\mathit{S}-\mathit{P}$ represents the amount of “mutual attributes” of the two sets that are neither identity nor contrary. $\alpha =\frac{\mathit{S}}{\mathit{N}},\beta =\frac{\mathit{F}}{\mathit{N}},\gamma =\frac{\mathit{P}}{\mathit{N}}$ are, respectively, called the identity degree, discrepancy degree, and contrary degree of the two sets. According to the given circumstances, $i$ (contrary degree) and $j$ (discrepancy degree) can be specified from $[-1, 1]$. Clearly, $\alpha +\beta +\gamma =1$.

3. Proposed IHCS and Aggregation Operators

This portion includes the establishment of a new concept by combining the IHFS and CN of the theory of SPA, called the intuitionistic hesitant fuzzy connection number set (IHCS). Based on this definition, a sequence of new aggregation operators is developed. These proposed operators and their properties have been analyzed and their suitability is portrayed in some newly developed algorithm.

Definition 6.

An intuitionistic hesitant fuzzy CN set (IHCS) corresponding to IHFS  $\mathcal{Z}=\left\{\left({\varsigma }_i,\varphi \left({\varsigma }_i\right),\psi \left({\varsigma }_i\right)\right)|{\varsigma }_i\in \mathcal{F}\right\}$ is denoted and defined as

$${\mathcal{J}}_{\mathcal{Z}}=\left\{\right({\varsigma }_i,{\alpha }_{\mathcal{Z}}\left({\varsigma }_i\right)+{\beta }_{\mathcal{Z}}\left({\varsigma }_i\right)i+{\gamma }_{\mathcal{Z}}\left({\varsigma }_i\right)j|{\varsigma }_i\in \mathcal{F}\}$$

(3)

where ${\alpha }_{\mathcal{Z}}\left({\varsigma }_i\right)=\frac{1}{m}{\sum }_{l=1}^m\left(\left\{h_l\left(1-f_l\right)\right\}\right)$, ${\beta }_{\mathcal{Z}}\left({\varsigma }_i\right)=\frac{1}{m}{\sum }_{l=1}^m\left(\left\{1-h_l\left(1-f_l\right)-f_l\left(1-h_l\right)\right\}\right)$, and ${\gamma }_{\mathcal{Z}}\left({\varsigma }_i\right)=\frac{1}{m}{\sum }_{l=1}^m\left(\left\{f_l\left(1-h_l\right)\right\}\right)$, and $h_l\in {\varphi }_{\mathcal{Z}}\left({\varsigma }_i\right)$ and $f_l\in {\psi }_{\mathcal{Z}}\left({\varsigma }_i\right)$ are arbitrary elements of the membership and non-membership sets. Here, ${\alpha }_{\mathcal{Z}}\left({\varsigma }_i\right)$, ${\beta }_{\mathcal{Z}}\left({\varsigma }_i\right)$, and ${\gamma }_{\mathcal{Z}}\left({\varsigma }_i\right)$ signify the “identity,” “discrepancy”, and “contrary” degrees.

For a particular $k,{\alpha }_{\mathcal{Z}}\left({\varsigma }_k\right)+{\beta }_{\mathcal{Z}}\left({\varsigma }_k\right)i+{\gamma }_{\mathcal{Z}}\left({\varsigma }_k\right)j$, this represents the intuitionistic hesitant fuzzy connection number IHCN. Without loss of generality, it will be written as ${\alpha }_k+{\beta }_{k}i+{\gamma }_{k}j$.

Remark 2.

• For each ${\varsigma }_i\in \mathcal{F},{\mathcal{J}}_{\mathcal{Z}}={\alpha }_{\mathcal{Z}}\left({\varsigma }_i\right)+{\beta }_{\mathcal{Z}}\left({\varsigma }_i\right)i+{\gamma }_{\mathcal{Z}}\left({\varsigma }_i\right)j$ will be considered as an intuitionistic hesitant fuzzy connection number element.
• Throughout the article, the lengths of the IHFEs will be kept similar by repeating the maximum value in the smaller one.
• $h_l$, $f_l$ $(l=\mathrm{1,2},\dots ,m)$ represents the IHFEs in ascending order.
• The components of the IHCS are considered as IHCEs.

Definition 7.

For two IHCEs ${\mathcal{J}}_1={\alpha }_1+{\beta }_{1}i+{\gamma }_{1}j$ and  ${\mathcal{J}}_2={\alpha }_2+{\beta }_{2}i+{\gamma }_2\left({\varsigma }_i\right)j$, we have

i.

${\mathcal{J}}_1\left({\varsigma }_i\right)={\mathcal{J}}_2(.{\varsigma }_i)\iff {\alpha }_1\left({\varsigma }_i\right)={\alpha }_2\left({\varsigma }_i\right),{\beta }_1\left({\varsigma }_i\right)={\beta }_1\left({\varsigma }_i\right),{\gamma }_1\left({\varsigma }_i\right)={\gamma }_2\left({\varsigma }_i\right)$;

ii.

${\mathcal{J}}_1\left({\varsigma }_i\right)\le {\mathcal{J}}_2\left({\varsigma }_i\right) if {\alpha }_1\left({\varsigma }_i\right)\le {\alpha }_2\left({\varsigma }_i\right),{\gamma }_1\left({\varsigma }_i\right)\ge {\gamma }_2\left({\varsigma }_i\right)$;

iii.

${{\mathcal{J}}_1\left({\varsigma }_i\right)}^c={\gamma }_1\left({\varsigma }_i\right)+{\beta }_1\left({\varsigma }_i\right)i+{\alpha }_1\left({\varsigma }_i\right)j$ represents the complement of the IHCE ${\mathcal{J}}_1\left({\varsigma }_i\right).$

Definition 8.

Let ${\mathcal{J}}_1={\alpha }_1+{\beta }_{1}i+{\gamma }_{1}j$ and ${\mathcal{J}}_2={\alpha }_2+{\beta }_{2}i+{\gamma }_{2}j$ be two IHCEs; then,

i.

${\mathcal{J}}_1⨁{\mathcal{J}}_2=(1-(1-{\alpha }_1\left)\right(1-{\alpha }_2\left)\right)+(\left({\beta }_1+{\gamma }_1)\left({\beta }_2+{\gamma }_2\right)-{\beta }_1{\beta }_2\right)i+\left({\beta }_1{\beta }_2\right)j$;

ii.

${\mathcal{J}}_1\otimes {\mathcal{J}}_2={\alpha }_1{\alpha }_2+\left(1-\left(1-{\beta }_1\right)\left(1-{\beta }_2\right)\right)i+\left(\left({\alpha }_1+{\gamma }_1\right)\left({\alpha }_2+{\gamma }_2\right)-{\alpha }_1{\alpha }_2\right)j$;

iii.

${{\mathcal{J}}_1}^{\lambda }={a_1}^{\lambda }+\left(1-{\left(1-b_1\right)}^{\lambda }\right)i+\left({\left(a_1+c_1\right)}^{\lambda }-a_1^{\lambda }\right)j,\lambda >0.$

It is straightforward to prove that these operators generate IHCEs.

Definition 9.

For a group of IHCEs  ${\mathcal{J}}_i(i=\mathrm{1,2},\dots ,n)$, the power averaging operator IHCPA:  ${\mathcal{J}}^n\to \mathcal{J}$ is defined as

$$IHCPA\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots {\mathcal{J}}_n\right)=\frac{\begin{array}{c}n\\ ⨁\\ i=1\end{array}(1+T({\mathcal{J}}_i)){\mathcal{J}}_i}{\sum _{i=1}^n(1+T({\mathcal{J}}_i\left)\right)}$$

(4)

$$\begin{gathered} \begin{array}{l}=(1-{\displaystyle \prod _{i=1}^n}\left({1-{(\alpha }_i)}^{\frac{(1+T({\mathcal{J}}_i\left)\right))}{{\sum }_{i=1}^n(1+T({\mathcal{J}}_i\left)\right)}}\right))\\ +({\displaystyle \prod _{i=1}^n}{\left({\beta }_i+{\gamma }_i\right)}^{\frac{\left(1+T\left({\mathcal{J}}_i\right)\right)}{{\sum }_{i=1}^n(1+T({\mathcal{J}}_i\left)\right)}}-{\displaystyle \prod _{i=1}^n}{\left({\beta }_i\right)}^{\frac{\left(1+T\left({\mathcal{J}}_i\right)\right)}{ \\ {\sum }_{i=1}^n(1+T({\mathcal{J}}_i\left)\right)}})i\\ +\left(\prod _{i=1}^n{\left({\beta }_i\right)}^{\frac{\left(1+T\left({\mathcal{J}}_i\right)\right)}{{\sum }_{i=1}^n(1+T({\mathcal{J}}_i\left)\right)}}\right)\end{array} \end{gathered}$$

(5)

wherever $T\left({\mathcal{J}}_i\right)$ is the $Sup$ of the $ith$ biggest IHCE (${\mathcal{J}}_i$) by all the other IHCEs, that is,

$$T\left({\mathcal{J}}_i\right)={\sum }_{\begin{array}{c}j=1\\ j\ne i\end{array}}^{n}Sup({\mathcal{J}}_i,{\mathcal{J}}_j)$$

Here, $Sup({\mathcal{J}}_i,{\mathcal{J}}_j)$ is the $Sup$ for ${\mathcal{J}}_i$ from ${\mathcal{J}}_j$, and it is calculated by:

$$Sup\left({\mathcal{J}}_i,{\mathcal{J}}_j\right)=1-d\left({\mathcal{J}}_i,{\mathcal{J}}_j\right)$$

$Sup$ satisfies the given properties:

i.

$Sup ({\mathcal{J}}_i,{\mathcal{J}}_j)\in [0,1]$;

ii.

$Sup ({\mathcal{J}}_i,{\mathcal{J}}_j)=Sup ({\mathcal{J}}_j,{\mathcal{J}}_i)$;

iii.

$Sup ({\mathcal{J}}_i,{\mathcal{J}}_j)\ge Sup ({\mathcal{J}}_s,{\mathcal{J}}_t),if d({\mathcal{J}}_i-{\mathcal{J}}_j)<d({\mathcal{J}}_s-{\mathcal{J}}_t)$ where $d$ is distance.

The support ($Sup$) amount is a similarity indicator.

Definition 10.

Let  ${\mathcal{J}}_i$  be a group of IHCEs and  $\omega ={({\omega }_1,{\omega }_2,\dots ,{\omega }_n)}^T$  by the weight vector of  ${\mathcal{J}}_i$,  ${\omega }_i>0$, and  $\sum _{i=1}^n{\omega }_i=1.$  The power weight averaging operator IHCPWA:  ${\mathcal{J}}^n\to \mathcal{J}$  is defined as

$$IHCPWA\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=\frac{\begin{array}{c}n\\ ⨁\\ i=1\end{array}({\omega }_i(1+T({\mathcal{J}}_i){\mathcal{J}}_i)}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_i))}$$

$$\begin{array}{l}=1-{\displaystyle \prod _{i=1}^n}\left({1-{(\alpha }_i)}^{\frac{{\omega }_i(1+T({\mathcal{J}}_i\left)\right)}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_i\left)\right)}}\right)\\ +\left({\displaystyle \prod _{i=1}^n}{\left({\beta }_i+{\gamma }_i\right)}^{\frac{{\omega }_i\left(1+T\left({\mathcal{J}}_i\right)\right)}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_i\left)\right)}}-{\displaystyle \prod _{i=1}^n}{\left({\beta }_i\right)}^{\frac{{\omega }_i\left(1+T\left({\mathcal{J}}_i\right)\right)}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_i\left)\right)}}\right)i\\ +\left({\displaystyle \prod _{i=1}^n}{\left({\beta }_i\right)}^{\frac{{\omega }_i\left(1+T\left({\mathcal{J}}_i\right)\right)}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_i\left)\right)}}\right)j\end{array}$$

(6)

The following properties can be easily justified for the IHCPWA operators.

Property 1: (Idempotency)

Suppose  ${\mathcal{J}}_i$ $\left(i=1,2,3\cdots ,n\right)$  are  ${\mathcal{J}}_i=\mathcal{J}$  for each  $i$  ; then,

$$IHCPWA\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=\mathcal{J}$$

Property 2: (Boundedness)

Suppose  ${\mathcal{J}}_i$ is a collection of IHCEs, and permits 

$${\mathcal{J}}^{-}=\underset{i}{\mathit{min}}{\mathcal{J}}_i,{\mathcal{J}}^{+}=\underset{i}{\mathit{max}}{\mathcal{J}}_i(i=1,2,\dots ,n)$$

then

$${\mathcal{J}}^{-}\le IHCPWA\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)\le {\mathcal{J}}^{+}$$

Property 3: (Monotonicity)

Suppose  ${\mathcal{J}}_i and {{\mathcal{J}}^{\prime }}_i$ are collections of IHCEs; if  ${\mathcal{J}}_i\le {{\mathcal{J}}^{\prime }}_i$  for all  $i$  , then

$$IHCPWA\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)\le IH\mathrm{C}PWA\left({{\mathcal{J}}^{\prime }}_1,{{\mathcal{J}}^{\prime }}_2,\dots ,{{\mathcal{J}}^{\prime }}_n\right)$$

Definition 11.

Let  ${\mathcal{J}}_i$ be a group of IHCEs; the power order weight averaging operator of dimension  $“n”$, related with the weight vector  $\omega ={({\omega }_1,{\omega }_2,\dots ,{\omega }_i)}^T$  such that  ${\omega }_i>0$  and  $\sum _{i=1}^n{\omega }_i=1$  , IHCPOWA:  ${\mathcal{J}}^n\to \mathcal{J}$, is defined as

$$IHCPOWA\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=\frac{\begin{array}{c}n\\ ⨁\\ i=1\end{array}({\omega }_i(1+T({\mathcal{J}}_{\sigma (i)}){\mathcal{J}}_{\sigma (i)}))}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_{\sigma (i)}))}$$

$$\begin{array}{l}=1-{\displaystyle \prod _{i=1}^n}\left({1-\left({\alpha }_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i(1+T({\mathcal{J}}_{\sigma \left(i\right)}\left)\right)}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_{\sigma \left(i\right)}\left)\right)}}\right)\\ +\left({\displaystyle \prod _{i=1}^n}{\left({\beta }_{\sigma \left(i\right)}+{\gamma }_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i\left(1+T\left({\mathcal{J}}_{\sigma \left(i\right)}\right)\right)}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_{\sigma \left(i\right)}\left)\right)}}-{\displaystyle \prod _{i=1}^n}{\left({\beta }_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i\left(1+T\left({\mathcal{J}}_{\sigma \left(i\right)}\right)\right)}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_{\sigma \left(i\right)}\left)\right)}}\right)i\\ +\left({\displaystyle \prod _{i=1}^n}{\left({\beta }_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i\left(1+T\left({\mathcal{J}}_{\sigma \left(i\right)}\right)\right)}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_{\sigma \left(i\right)}\left)\right)}}\right)j\end{array}$$

(7)

where $\sigma \left(1\right),\sigma \left(2\right)$,…,$\sigma \left(n\right)$ indicates a permutation of $(\mathrm{1,2},\dots ,n)$, in which ${\mathcal{J}}_{\sigma (i-1)}\ge {\mathcal{J}}_{\sigma \left(i\right)},$ ${\omega }_i\left(i=\mathrm{1,2},\dots ,n\right)$ is a group of weights so that

$${\omega }_i=g\left(\frac{R_i}{TV}\right)-g\left(\frac{R_{i-1}}{TV}\right),R_i={\sum }_{i=1}^n{V}_{\sigma \left(i\right)},TV={\sum }_{i=1}^n{V}_{\sigma \left(i\right)},V_{\sigma \left(i.\right)}=1.+T\left({\mathcal{J}}_{\sigma \left(i\right)}\right)$$

and $T\left({\mathcal{J}}_{\sigma \left(j\right)}\right)$ implies the $Sup$ of the $j$th largest IHCE $T\left({\mathcal{J}}_{\sigma \left(j\right)}\right)$ by all the other (IHCEs), that is,

$$T\left({\mathcal{J}}_{\sigma \left(j\right)}\right)=\sum _{\begin{array}{c}i=1\\ i\ne j\end{array}}^{n}Sup({\mathcal{J}}_{\sigma \left(j\right)},{\mathcal{J}}_{\sigma \left(i\right)})$$

where $\sum _{\begin{array}{c}i=1\\ i\ne j\end{array}}^{n}Sup({\mathcal{J}}_{\sigma \left(j\right)},{\mathcal{J}}_{\sigma \left(i\right)})$ shows the $Sup$ of the $j$th is the biggest IHCE ${\mathcal{J}}_{\sigma \left(j\right)}$, for the $i$th largest IHCE ${\mathcal{J}}_{\sigma \left(i\right)}$.

Some properties of the IHCPOWA operator are as follows:

Property 4: (Idempotency)

Suppose  ${\mathcal{J}}_i\left(i=1,2,3\cdots ,n\right)$ are  ${\mathcal{J}}_i=\mathcal{J}$  for every  $i$; then,

$$\mathrm{I}\mathrm{H}CPOWA\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=\mathcal{J}$$

Property 5: (Boundedness)

Let  ${\mathcal{J}}_i$ be a group of IHCEs and allow

$${\mathcal{J}}^{-}=\underset{i}{\mathit{min}}{\mathcal{J}}_i,{\mathcal{J}}^{+}=\underset{i}{\mathit{max}}{\mathcal{J}}_i (i=1,2,\dots ,n)$$

then

$${\mathcal{J}}^{-}\le IHCPOWA(\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)\le {\mathcal{J}}^{+}$$

Property 6: (Monotonicity)

Let  ${\mathcal{J}}_i and {{\mathcal{J}}^{\prime }}_i$ be two set of IHCEs; if  ${\mathcal{J}}_i\le {{\mathcal{J}}^{\prime }}_i$  for all  $i$  , then

$$IHCPOWA\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)\le IH\mathrm{C}POWA\left({{\mathcal{J}}^{\prime }}_1,{{\mathcal{J}}^{\prime }}_2,\dots ,{{\mathcal{J}}^{\prime }}_n\right)$$

Property 7: (Commutativity)

Let  ${\mathcal{J}}_i and {{\mathcal{J}}^{\prime }}_i$ be two set of IHCEs; if  ${\mathcal{J}}_i\le {{\mathcal{J}}^{\prime }}_i$  for all  $i$, then 

$$IHCPOWA\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=IH\mathrm{C}POWA\left({{\mathcal{J}}^{\prime }}_1,{{\mathcal{J}}^{\prime }}_2,\dots ,{{\mathcal{J}}^{\prime }}_n\right)$$

where ${{\mathcal{J}}^{\prime }}_j$ is a permutation of ${\mathcal{J}}_j$.

Definition 12.

Suppose  ${\mathcal{J}}_i$ is group of IHCEs; the power hybrid averaging operator IHCPHA:  ${\mathcal{J}}^n\to \mathcal{J}$  is defined as

$$IHCNPHA\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=\frac{\begin{array}{c}n\\ ⨁\\ i=1\end{array}({\omega }_i(1+T({\dot{\mathcal{J}}}_{\sigma \left(i\right)}){\dot{\mathcal{J}}}_{\sigma \left(i\right)}))}{\sum _{i=1}^n{\omega }_i(1+T({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\left)\right)}$$

$$\begin{array}{l}=1-{\displaystyle \prod _{i=1}^n}\left({1-\left({\dot{\alpha }}_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i(1+T({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\left)\right)}{\sum _{i=1}^n{\omega }_i(1+T({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\left)\right)}}\right)\\ +({\displaystyle \prod _{i=1}^n}{\left({\dot{\beta }}_{\sigma \left(i\right)}+{\dot{\gamma }}_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i\left(1+T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right)\right)}{\sum _{i=1}^n{\omega }_i(1+T({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\left)\right)}}\\ -{\displaystyle \prod _{i=1}^n}{\left({\dot{\beta }}_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i\left(1+T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right)\right)}{\sum _{i=1}^n{\omega }_i(1+T({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\left)\right)}})i\\ +\left({\displaystyle \prod _{i=1}^n}{\left({\dot{\beta }}_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i\left(1+T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right)\right)}{\sum _{i=1}^n{\omega }_i(1+T({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\left)\right)}}\right)j\end{array}$$

(8)

where $\omega ={({\omega }_1,{\omega }_2,\dots ,{\omega }_i)}^T$ is a represented weight vector, where ${\omega }_i\in [0, 1]$ and $\sum _{i=1}^n{\omega }_i=1$ and ${\mathcal{J}}_{\sigma \left(i\right)}$ is the $i$th largest objects in the IHCE arguments ${\dot{\mathcal{J}}}_i$ ($\dot{\mathcal{J}}$ = ($n{\omega }_i$) ${\mathcal{J}}_i$, $i=1,2,\dots ,n)$, $\omega =({\omega }_1,{\omega }_2,$ … , ${\omega }_n$) is the weighting vector of the IHCE arguments ${\mathcal{J}}_i(i=1,2,\dots ,n)$, ${\omega }_i\in [0, 1]$, and $\sum _{i=1}^n{\omega }_i=1$ with ${\omega }_i$ being a group such that

$${\omega }_i=g\left(\frac{R_i}{TV}\right)-g\left(\frac{R_{i-1}}{TV}\right),R_i={\sum }_{i=1}^j{V}_{\sigma \left(i\right)},TV={\sum }_{i=1}^n{V}_{\sigma \left(i\right)},V_{\sigma \left(i\right)}=1+T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right)$$

and $T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right)$ is the $Sup$ of the $j$th biggest IHCEs ${\dot{\mathcal{J}}}_{\sigma \left(i\right)}$ by all the other (IHCEs), that is,

$$T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right)=\sum _{\begin{array}{c}i=1\\ i\ne j\end{array}}^{n}Sup({\dot{\mathcal{J}}}_{\sigma \left(j\right)},{\mathcal{J}}_{\sigma \left(i\right)})$$

where $\sum _{\begin{array}{c}i=1\\ i\ne j\end{array}}^{n}Sup({\dot{\mathcal{J}}}_{\sigma \left(j\right)},{\mathcal{J}}_{\sigma \left(i\right)})$ shows the $Sup$ of the $j$th biggest IHCE ${\dot{\mathcal{J}}}_{\sigma \left(j\right)}$, for the $i$th biggest IHCE ${\dot{\mathcal{J}}}_{\sigma \left(i\right)}$. Specifically, the IHCPHA is reduced to the IHCPWA operator if $\omega ={(\frac{1}{n},\frac{1}{n},\dots ,\frac{1}{n})}^T$ and the IHCPHA is reduced to the IHCPOWA operator if $\omega =(\frac{1}{n},\frac{1}{n},\dots ,\frac{1}{n})$.

Definition 13.

Suppose  ${\mathcal{J}}_i$  is family of IHCEs; the power geometric operator IHCPG:  ${\mathcal{J}}^n\to \mathcal{J}$  is defined as

$$IHCPG\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=\begin{array}{c}n\\ \otimes \\ i=1\end{array}{\left(\mathcal{J}\right)}^{\frac{1+T\left({\mathcal{J}}_i\right)}{\sum _{i=1}^n(1+T({\mathcal{J}}_i\left)\right)}}$$

(9)

$$=\prod _{i=1}^n{\left({\alpha }_i\right)}^{\frac{\left(1+T\left({\mathcal{J}}_i\right)\right)}{\sum _{i=1}^n(1+T({\mathcal{J}}_i\left)\right)}}+(1-\prod _{i=1}^n{(1-{\beta }_i)}^{\frac{(1+T({\mathcal{J}}_i\left)\right)}{\sum _{i=1}^n(1+T({\mathcal{J}}_i\left)\right)}})i+\left(\prod _{i=1}^n{\left({\alpha }_i+{\gamma }_i\right)}^{\frac{\left(1+T\left({\mathcal{J}}_i\right)\right)}{\sum _{i=1}^n(1+T({\mathcal{J}}_i\left)\right)}}-\prod _{i=1}^n{\left({\alpha }_i\right)}^{\frac{\left(1+T\left({\mathcal{J}}_i\right)\right)}{\sum _{i=1}^n(1+T({\mathcal{J}}_i\left)\right)}}\right)j$$

(10)

where

$$T\left({\mathcal{J}}_i\right)={\sum }_{\begin{array}{c}j=1\\ i\ne j\end{array}}^{n}Sup ({\mathcal{J}}_i,{\mathcal{J}}_j)$$

Definition 14.

Let  ${\mathcal{J}}_i$  be a group of IHCEs and  $\omega ={({\omega }_1,{\omega }_2,\dots ,{\omega }_n)}^T$  be the weight vector of  ${\mathcal{J}}_i$,  ${\omega }_i>0$  and  $\sum _{i=1}^n{\omega }_i=1.$  The power weight geometric operator IHCPWG:  ${\mathcal{J}}^n\to \mathcal{J}$  is defined as 

$$IHCPWG\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=\begin{array}{c}n\\ \otimes \\ i=1\end{array}{\left(\mathcal{J}\right)}^{\frac{{\omega }_i(1+T\left({\mathcal{J}}_i\right))}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_i\left)\right)}}$$

$$=\prod _{i=1}^n{\left({\alpha }_i\right)}^{\frac{{\omega }_i(1+T\left({\mathcal{J}}_i\right))}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_i\left)\right)}}+(1-\prod _{i=1}^n{(1-{\beta }_i)}^{\frac{{\omega }_i(1+T\left({\mathcal{J}}_i\right))}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_i\left)\right)}})i+\left(\prod _{i=1}^n{\left({\alpha }_i+{\gamma }_i\right)}^{\frac{{\omega }_i(1+T\left({\mathcal{J}}_i\right))}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_i\left)\right)}}-\prod _{i=1}^n{\left({\alpha }_i\right)}^{\frac{{\omega }_i(1+T\left({\mathcal{J}}_i\right))}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_i\left)\right)}}\right)j$$

(11)

The following characteristics can be easily proven for the IHCPWG operator.

Property 8: (Idempotency)

When ${\mathcal{J}}_i\left(i=1,2,3\cdots ,n\right)$ are ${\mathcal{J}}_i=\mathcal{J}$ for each $i$, then 

$$IHCPWG\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=\mathcal{J}$$

Property 9: (Boundedness)

Suppose ${\mathcal{J}}_i$ is a group of IHCEs, and permits 

$${\mathcal{J}}^{-}=\underset{i}{\mathit{min}}{\mathcal{J}}_i,{\mathcal{J}}^{+}=\underset{i}{\mathit{max}}{\mathcal{J}}_i (i=1,2,\dots ,n)$$

then

$${\mathcal{J}}^{-}\le IHCPWG\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)\le {\mathcal{J}}^{+}$$

Property 10: (Monotonicity)

Let ${\mathcal{J}}_i and {{\mathcal{J}}^{\prime }}_i$ be two sets of IHCEs; if ${\mathcal{J}}_i\le {{\mathcal{J}}^{\prime }}_i$ for all $i$, then 

$$IHCPWG\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)\le IH\mathrm{C}PWG\left({{\mathcal{J}}^{\prime }}_1,{{\mathcal{J}}^{\prime }}_2,\dots ,{{\mathcal{J}}^{\prime }}_n\right)$$

Definition 15.

Let  ${\mathcal{J}}_i$  be group of IHCEs; the power order weight geometric operator of dimension  $‘n’$,  related with the weight vector  $\omega ={({\omega }_1,{\omega }_2,\dots ,{\omega }_i)}^T$  such that  ${\omega }_i>0$  and  $\sum _{i=1}^n{\omega }_i=1$,  IHCPOWG:  ${\mathcal{J}}^n\to \mathcal{J}$,  is defined as 

$$IHCPOWG\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=\begin{array}{c}n\\ \otimes \\ i=1\end{array}{\left({\mathcal{J}}_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i(1+T\left({\mathcal{J}}_{\sigma \left(i\right)}\right))}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_{\sigma \left(i\right)}\left)\right)}}$$

$$\begin{array}{c}={\displaystyle \prod _{i=1}^n}\left({\left({\alpha }_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i(1+T\left({\mathcal{J}}_{\sigma \left(i\right)}\right))}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_{\sigma \left(i\right)}\left)\right)}}\right)\\ +(1-{\displaystyle \prod _{i=1}^n}{(1-{\beta }_{\sigma \left(i\right)})}^{\frac{{\omega }_i(1+T\left({\mathcal{J}}_{\sigma \left(i\right)}\right))}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_{\sigma \left(i\right)}\left)\right)}})i\\ +\left({\displaystyle \prod _{i=1}^n}{\left({\alpha }_{\sigma \left(i\right)}+{\gamma }_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i(1+T\left({\mathcal{J}}_{\sigma \left(i\right)}\right))}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_{\sigma \left(i\right)}\left)\right)}}-{\displaystyle \prod _{i=1}^n}{\left({\alpha }_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i(1+T\left({\mathcal{J}}_{\sigma \left(i\right)}\right))}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_{\sigma \left(i\right)}\left)\right)}}\right)j\end{array}$$

(12)

where $\sigma \left(1\right),\sigma \left(2\right)$,…,$\sigma \left(n\right)$ indicates a permutation of (1, 2, …, $n$), where ${\mathcal{J}}_{\sigma (i-1)}\ge {\mathcal{J}}_{\sigma \left(i\right)},$ and ${\omega }_i\left(i=\mathrm{1,2},\dots ,n\right)$ is group of weights so that

$${\omega }_i=g\left(\frac{R_i}{TV}\right)-g\left(\frac{R_{i-1}}{TV}\right),R_j=\sum _{i=1}^j{V}_{\sigma \left(i\right)},TV=\sum _{i=1}^n{V}_{\sigma \left(i\right)},V_{\sigma \left(i.\right)}=1.+T\left({\mathcal{J}}_{\sigma \left(i\right)}\right)$$

and $T\left({\mathcal{J}}_{\sigma \left(i\right)}\right)$ implies the $Sup$ of the $i\mathrm{th}$ biggest IHCE ${\mathcal{J}}_{\sigma \left(i\right)}$ by all the other (IHCEs), that is,

$$T\left({\mathcal{J}}_{\sigma \left(i\right)}\right)=\sum _{\begin{array}{c}j=1\\ i\ne j\end{array}}^{n}Sup({\mathcal{J}}_{\sigma \left(i\right)},{\mathcal{J}}_{\sigma \left(j\right)})$$

where $\sum _{\begin{array}{c}j=1\\ i\ne j\end{array}}^{n}Sup({\mathcal{J}}_{\sigma \left(i\right)},{\mathcal{J}}_{\sigma \left(j\right)})$ shows the $Sup$ of the $i$th is the biggest IHCE ${\mathcal{J}}_{\sigma \left(i\right)}$, for the $j$th largest IHCE ${\mathcal{J}}_{\sigma \left(j\right)}$.

Some properties of the IHCPOWG operator are as follows:

Property 11: (Idempotency)

Let ${\mathcal{J}}_i$ $\left(i=1,2,3\cdots ,n\right)$ be ${\mathcal{J}}_i=\mathcal{J}$ for every $i$; then, 

$$IHCPOWG\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=\mathcal{J}$$

Property 12: (Boundedness)

Suppose ${\mathcal{J}}_i$ is a group of IHCEs, and permits

$${\mathcal{J}}^{-}=\underset{i}{\mathit{min}}{\mathcal{J}}_i,{\mathcal{J}}^{+}=\underset{i}{\mathit{max}}{\mathcal{J}}_i(i=1,2,\dots ,n)$$

then

$${\mathcal{J}}^{-}\le IHCPOWG\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)\le {\mathcal{J}}^{+}$$

Property 13: (Monotonicity)

Let ${\mathcal{J}}_i and {{\mathcal{J}}^{\prime }}_i$ be two sets of IHCEs; if ${\mathcal{J}}_i\le {{\mathcal{J}}^{\prime }}_i$ for all $i$, then

$$IHCPOWG\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)\le IH\mathrm{C}POWG\left({{\mathcal{J}}^{\prime }}_1,{{\mathcal{J}}^{\prime }}_2,\dots ,{{\mathcal{J}}^{\prime }}_n\right)$$

Property 14: (Commutativity)

Let ${\mathcal{J}}_i and {{\mathcal{J}}^{\prime }}_i$ be two sets of IHCEs; if ${\mathcal{J}}_i\le {{\mathcal{J}}^{\prime }}_i$ for all $i$, then

$$IHCPOWG\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=IH\mathrm{C}POWG\left({{\mathcal{J}}^{\prime }}_1,{{\mathcal{J}}^{\prime }}_2,\dots ,{{\mathcal{J}}^{\prime }}_n\right)$$

where ${{\mathcal{J}}^{\prime }}_j$ is a permutation of ${\mathcal{J}}_j$.

Definition 16.

Suppose${\mathcal{J}}_i$  is group of IHCEs; the power hybrid geometric operator of objects  $‘n’$   IHCPHG:  ${\mathcal{J}}^n\to \mathcal{J}$  is defined as 

$$IHCPHG\left({\mathcal{J}}_1,{\mathcal{J}}_2,\dots ,{\mathcal{J}}_n\right)=\begin{array}{c}n\\ \otimes \\ i=1\end{array}{\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i(1+T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right))}{\sum _{i=1}^n{\omega }_i(1+T({\dot{\dot{\mathcal{J}}}}_{\sigma \left(i\right)}\left)\right)}}$$

$$\begin{array}{c}={\displaystyle \prod _{i=1}^n}{\left({\dot{\alpha }}_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i(1+T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right))}{\sum _{i=1}^n{\omega }_i(1+T({\dot{\dot{\mathcal{J}}}}_{\sigma \left(i\right)}\left)\right)}}+(1-{\displaystyle \prod _{i=1}^n}{(1-{\dot{\beta }}_{\sigma \left(i\right)})}^{\frac{{\omega }_i(1+T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right))}{\sum _{i=1}^n{\omega }_i(1+T({\dot{\dot{\mathcal{J}}}}_{\sigma \left(i\right)}\left)\right)}})i\\ +\left({\displaystyle \prod _{i=1}^n}{\left({\dot{\alpha }}_{\sigma \left(i\right)}+{\dot{\gamma }}_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i(1+T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right))}{\sum _{i=1}^n{\omega }_i(1+T({\dot{\dot{\mathcal{J}}}}_{\sigma \left(i\right)}\left)\right)}}-{\displaystyle \prod _{i=1}^n}{\left({\dot{\alpha }}_{\sigma \left(i\right)}\right)}^{\frac{{\omega }_i(1+T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right))}{\sum _{i=1}^n{\omega }_i(1+T({\dot{\dot{\mathcal{J}}}}_{\sigma \left(i\right)}\left)\right)}}\right)j\end{array}$$

(13)

where $\omega ={({\omega }_1,{\omega }_2,\dots ,{\omega }_i)}^T$ is a represented weight vector, where ${\omega }_i\in [0,1]$ and $\sum _{i=1}^n{\omega }_i=1$, and ${\mathcal{J}}_{\sigma \left(i\right)}$ is the $i$th largest object in the IHCEs arguments ${\dot{\mathcal{J}}}_i$ ($\dot{\mathcal{J}}$ = ($n{\omega }_i$)${\mathcal{J}}_i$, $i=1,2,\dots ,n)$, $\omega =({\omega }_1,{\omega }_2,$ … , ${\omega }_n$) is the weighting vector of the IHCE influences ${\mathcal{J}}_i(i=1,2,\dots ,n)$, ${\omega }_i\in [0,1]$. $\sum _{i=1}^n{\omega }_i=1$, as well as ${\omega }_i$, is a group where

$${\omega }_i=g\left(\frac{R_i}{TV}\right)-g\left(\frac{R_{i-1}}{TV}\right),R_i={\sum }_{i=1}^j{V}_{\sigma \left(i\right)},TV={\sum }_{i=1}^n{V}_{\sigma \left(i\right)},V_{\sigma \left(i\right)}=1+T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right)$$

and $T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right)$ is the $Sup$ of the $j$th biggest IHCEs ${\dot{\mathcal{J}}}_{\sigma \left(i\right)}$ by all the other IHCEs, that is,

$$T\left({\dot{\mathcal{J}}}_{\sigma \left(i\right)}\right)=\sum _{\begin{array}{c}j=1\\ i\ne j\end{array}}^{n}Sup({\dot{\mathcal{J}}}_{\sigma \left(i\right)},{\mathcal{J}}_{\sigma \left(j\right)})$$

where ${\sum }_{\begin{array}{c}j=1\\ i\ne j\end{array}}^{n}Sup({\dot{\mathcal{J}}}_{\sigma \left(i\right)},{\mathcal{J}}_{\sigma \left(j\right)})$ shows the $Sup$ of the $j$th largest IHCE ${\dot{\mathcal{J}}}_{\sigma \left(j\right)}$, for the $i$th biggest IHCE ${\dot{\mathcal{J}}}_{\sigma \left(i\right)}$. Specifically, the IHCPHG is reduced to the IHCPWG operator if $\omega ={(\frac{1}{n},\frac{1}{n},\dots ,\frac{1}{n})}^T$ and the IHCPHG is reduced to the IHCNPOWG operator if $\omega =(\frac{1}{n},\frac{1}{n},\dots ,\frac{1}{n})$.

Theorem 1.

The IHCPA operator aggregates the “$n$” IHCEs and again generates IHCE.

Proof 1.

The proof is straightforward. □

Remark 3.

Similarly, the IHCPWA, IHCPOWA, IHCPHA, IHCPG IHCPWG, IHCPOWG, and IHCPHG also generate the IHCE.

4. Proposed MADM Algorithm Based on IHCS

This section includes the execution of the proposed operators to DMPs through IHF data. The proposed concepts are applied to denote the MADM challenges for the promising computation of developing technology companies with IHF data. Suppose $\mathfrak{H}=\{{\mathfrak{H}}_1,{\mathfrak{H}}_2,\dots ,{\mathfrak{H}}_m\}$ is the distinctive collection of alternatives, and $\mathfrak{G}$ $=\{{\mathfrak{G}}_1,{\mathfrak{G}}_2,\dots ,{\mathfrak{G}}_n\}$ is the collection of attributes. Suppose $\omega$ $=\left({\omega }_t\right)(t=\mathrm{1,2},\dots n)$ is the weight vector of the attributes, such that ${\omega }_t\ge 0$, $\sum _{t=1}^n{\omega }_t=1$. Therefore, both the IHCPWA and IHCWG operators are applied to the MADM problems for the possible calculation of emerging technology commercialization by the IHF environment.

Step 1. Arrange all data about the alternative in matrix $\mathcal{M}={\left({\check{\mathsf{{\rm Y}}}}_{it}\right)}_{m\times n}$ in such a way:

$$\mathcal{M}=\begin{array}{c}\\ {\mathfrak{H}}_1\\ {\mathfrak{H}}_2\\ \begin{array}{c}⋮\\ {\mathfrak{H}}_m\end{array}\end{array}\begin{array}{c}\begin{array}{ccc}{\mathfrak{G}}_1& {\mathfrak{G}}_2& \begin{array}{cc}\cdots & {\mathfrak{G}}_n\end{array}\end{array}\\ \left(\begin{array}{ccc}\begin{array}{cc}\begin{array}{c}{\check{\mathsf{{\rm Y}}}}_{11}\\ {\check{\mathsf{{\rm Y}}}}_{21}\end{array}& \begin{array}{c}{\check{\mathsf{{\rm Y}}}}_{12}\\ {\check{\mathsf{{\rm Y}}}}_{22}\end{array}\end{array}& \cdots & \begin{array}{c}{\check{\mathsf{{\rm Y}}}}_{1n}\\ {\check{\mathsf{{\rm Y}}}}_{2n}\end{array}\\ \begin{array}{cc}⋮& \ddots \end{array}& \ddots & ⋮\\ \begin{array}{cc}{\check{\mathsf{{\rm Y}}}}_{m1}& {\check{\mathsf{{\rm Y}}}}_{m2}\end{array}& \cdots & {\check{\mathsf{{\rm Y}}}}_{mn}\end{array}\right)\end{array}$$

Step 2. Formulate the IHCEs ${\mathcal{J}}_{it}={\alpha }_{it}+{\beta }_{it}i+{\gamma }_{it}j$ from the IHFEs given in ${\check{\mathsf{{\rm Y}}}}_{it}$, by using Definition 6.

Step 3. Compute the supports:

$$Sup\left({\mathcal{J}}_{it},{\mathcal{J}}_{ik}\right)=1-d\left({\mathcal{J}}_{it},{\mathcal{J}}_{ik}\right),\left(t,k=1,2,\dots ,n\right)$$

(14)

where

$$d\left({\mathcal{J}}_{it},{\mathcal{J}}_{ik}\right)=\frac{1}{3}\left(\left|{\alpha }_{it}-{\alpha }_{ik}\right|+\left|{\beta }_{it}-{\beta }_{ik}\right|+\left|{\gamma }_{it}-{\gamma }_{ik}\right|\right)$$

(15)

Step 4. Utilize the weights ${\omega }_j$ of attribute ${\mathfrak{G}}_j$ to calculate the weighted $Sup$ T (${\mathcal{J}}_{it}$) of the IHCEs ${\mathcal{J}}_{it}$ by other IHCEs ${\mathcal{J}}_{ik}$ $(t,k=1,2,\dots ,n,t\ne k)$

$$T\left({\mathcal{J}}_{it}\right)={\sum }_{\begin{array}{c}k=1\\ k\ne j\end{array}}^n{\omega }_{j}Sup({\mathcal{J}}_{it},{\mathcal{J}}_{ik})$$

(16)

and calculate the weight ${\xi }_{it}$ connected with the IHCEs ${\mathcal{J}}_{it},(i=1,2,\dots ,n,t=1,2,\dots ,m)$

$${\xi }_{it}=\frac{{\omega }_t\left(\right(1+T\left({\mathcal{J}}_{it}\right))}{\sum _{i=1}^n{\omega }_t(1+T({\mathcal{J}}_{it}\left)\right)}$$

(17)

where ${\xi }_{it}\ge 0$ and $\sum _{i=1}^n{\xi }_{it}=1.$

Step 5. Use the decision data, the IHCPWA operator, and IHCPWG operators

$${\mathcal{J}}_t=IHCPWA\left({\mathcal{J}}_{i1},{\mathcal{J}}_{i2},\dots ,{\mathcal{J}}_{in}\right)=\frac{\begin{array}{c}n\\ ⨁\\ i=1\end{array}({\omega }_i(1+T({\mathcal{J}}_i){\mathcal{J}}_i)}{\sum _{i=1}^n{\omega }_i(1+T({\mathcal{J}}_i))}$$

$$=1-\prod _{i=1}^n\left({1-{(\alpha }_{it})}^{{\xi }_{it}}\right)+\left(\prod _{i=1}^n{\left({\beta }_{it}+{\gamma }_{it}\right)}^{{\xi }_{it}}-\prod _{i=1}^n{\left({\beta }_{it}\right)}^{{\xi }_{it}}\right)i+\left(\prod _{i=1}^n{\left({\beta }_{it}\right)}^{{\xi }_{it}}\right)j$$

(18)

and

$$IHCPW{G}_{\omega }\left({\mathcal{J}}_{t1},{\mathcal{J}}_{t2},\dots ,{\mathcal{J}}_{tn}\right)=\begin{array}{c}n\\ \otimes \\ i=1\end{array}{\left(\mathcal{J}\right)}^{{\xi }_{it}}$$

$$=\prod _{i=1}^n{\left({\alpha }_{it}\right)}^{{\xi }_{it}}+(1-\prod _{i=1}^n{(1-{\beta }_{it})}^{{\xi }_{it}})i+\left(\prod _{i=1}^n{\left({\alpha }_{it}+{\gamma }_{it}\right)}^{{\xi }_{it}}-\prod _{i=1}^n{\left({\alpha }_{it}\right)}^{{\xi }_{it}}\right)j$$

(19)

to obtain the complete preference elements ${\mathcal{J}}_i$of ${\mathfrak{H}}_t\left(t=1,2,\dots ,m\right).$

Step 6. Calculate the scores $Sc\left({\mathcal{J}}_i\right)$ for the total IHCEs ${\mathcal{J}}_i$ to rank the ${\mathfrak{H}}_i$ each, then choose the best one(s).

$$Sc\left({\mathcal{J}}_i\right)=\frac{1+({\alpha }_i-{\gamma }_i)(1+{\beta }_i)}{2}$$

(20)

Step 7. Rank the alternative ${\mathfrak{H}}_i$ values and select the biggest, considering $\left({\mathcal{J}}_i\right)$ $(i=1,2,\dots ,n)$.

5. Illustrative Example

In this section, we illustrate a mathematical problem to express the potential evaluation of companies of emerging technology by IHF information, demonstrating the method suggested in this paper. In a state of Pakistan, the government wants to contest an election and to overcome fraud and make it fair. The government decided to use technology for this election, so the government decided to develop an electronic voting machine (EVM). To complete this task, the government invites some electronics companies and configures five attributes for selecting the best company for the effort—“project cost” $\left({\mathfrak{G}}_1\right)$,“completion time” $\left({\mathfrak{G}}_2\right)$, “technical capability” $\left({\mathfrak{G}}_3\right)$, “business status” $\left({\mathfrak{G}}_4\right)$, and “company background” $\left({\mathfrak{G}}_5\right)$—and allocate the weights of the relative importance of every attribute. The weight vector relating to it has been given as $\omega ={\left(\mathrm{0.25,0.3,0.1,0.2,0.15}\right)}^T$ based on the decision maker’s preferences. The four electronics companies have taken in this structure of the alternatives, namely, “Noor Manufacturers Ltd.” (${\mathfrak{H}}_1$), “F&I Electronics Ltd.” (${\mathfrak{H}}_2$), “Pakistan Software Company” (${\mathfrak{H}}_3$), and “Sial Group of Technological Companies” (${\mathfrak{H}}_4$), who are interested in these tasks. The major purpose of the task is to select the best company for the project. The steps for the suggested technique are executed as follows:

• Step 1: The specialist has calculated all alternatives for the distinct attributes based on the IHFSs as given in Table 1.

  Table 1. IHF information corresponding to attributes.

  ${\mathfrak{G}}_{\mathbf{1}}$	${\mathfrak{G}}_{\mathbf{2}}$	${\mathfrak{G}}_{\mathbf{3}}$	${\mathfrak{G}}_{\mathbf{4}}$	${\mathfrak{G}}_{\mathbf{5}}$
  $\left(\right\{\mathrm{0.2,0.1}\},\{\mathrm{0.1,0.4}\left\}\right)$	$\left(\right\{\mathrm{0.1,0.2}\},\{\mathrm{0.6,0.3}\left\}\right)$	($\left\{\mathrm{0.1,0.3}\right\},\left\{\mathrm{0.5,0.1}\right\}$)	($\left\{\mathrm{0.2,0.4}\right\},\left\{\mathrm{0.1,0.2}\right\}$)	$\left(\left\{\mathrm{0.2,0.1}\right\},\left\{\mathrm{0.5,0.1}\right\}\right)$
  $\left(\left\{\mathrm{0.1,0.0}\right\},\left\{\mathrm{0.2,0.2}\right\}\right)$	$\left(\left\{\mathrm{0.0,0.1}\right\},\left\{\mathrm{0.1,0.2}\right\}\right)$	$\left(\right\{\mathrm{0.1,0.1}\},\{\mathrm{0.1,0.3}\left\}\right)$	$\left(\right\{\mathrm{0.1,0.2}\},\{\mathrm{0.1,0.3}\left\}\right)$	$\left(\left\{\mathrm{0.1,0.4}\right\},\left\{\mathrm{0.1,0.2}\right\}\right)$
  $\left(\left\{\mathrm{0.3,0.2}\right\},\left\{\mathrm{0.2,0.1}\right\}\right)$	($\left\{\mathrm{0.1,0.2}\right\},\left\{\mathrm{0.0,0.1}\right\}$)	$\left(\left\{\mathrm{0.2,0.5}\right\},\left\{\mathrm{0.2,0.1}\right\}\right)$	$\left(\right\{\mathrm{0.0,0.6}\},\{\mathrm{0.2,0.1}\left\}\right)$	$\left(\left\{\mathrm{0.2,0.2}\right\},\left\{\mathrm{0.5,0.2}\right\}\right)$
  $\left(\left\{\mathrm{0.3,0.1}\right\},\left\{\mathrm{0.2,0.5}\right\}\right)$	($\left\{\mathrm{0.3,0.5}\right\},\left\{\mathrm{0.1,0.1}\right\}$)	$\left(\left\{\mathrm{0.1,0.0}\right\},\left\{\mathrm{0.1,0.2}\right\}\right)$	$\left(\right\{\mathrm{0.3,0.2}\},\{\mathrm{0.2,0.2}\left\}\right)$	$\left(\left\{\mathrm{0.3,0.5}\right\},\left\{\mathrm{0.2,0.2}\right\}\right)$

  Table 1. IHF information corresponding to attributes.

  ${\mathfrak{G}}_{\mathbf{1}}$	${\mathfrak{G}}_{\mathbf{2}}$	${\mathfrak{G}}_{\mathbf{3}}$	${\mathfrak{G}}_{\mathbf{4}}$	${\mathfrak{G}}_{\mathbf{5}}$
  $\left(\right\{\mathrm{0.2,0.1}\},\{\mathrm{0.1,0.4}\left\}\right)$	$\left(\right\{\mathrm{0.1,0.2}\},\{\mathrm{0.6,0.3}\left\}\right)$	($\left\{\mathrm{0.1,0.3}\right\},\left\{\mathrm{0.5,0.1}\right\}$)	($\left\{\mathrm{0.2,0.4}\right\},\left\{\mathrm{0.1,0.2}\right\}$)	$\left(\left\{\mathrm{0.2,0.1}\right\},\left\{\mathrm{0.5,0.1}\right\}\right)$
  $\left(\left\{\mathrm{0.1,0.0}\right\},\left\{\mathrm{0.2,0.2}\right\}\right)$	$\left(\left\{\mathrm{0.0,0.1}\right\},\left\{\mathrm{0.1,0.2}\right\}\right)$	$\left(\right\{\mathrm{0.1,0.1}\},\{\mathrm{0.1,0.3}\left\}\right)$	$\left(\right\{\mathrm{0.1,0.2}\},\{\mathrm{0.1,0.3}\left\}\right)$	$\left(\left\{\mathrm{0.1,0.4}\right\},\left\{\mathrm{0.1,0.2}\right\}\right)$
  $\left(\left\{\mathrm{0.3,0.2}\right\},\left\{\mathrm{0.2,0.1}\right\}\right)$	($\left\{\mathrm{0.1,0.2}\right\},\left\{\mathrm{0.0,0.1}\right\}$)	$\left(\left\{\mathrm{0.2,0.5}\right\},\left\{\mathrm{0.2,0.1}\right\}\right)$	$\left(\right\{\mathrm{0.0,0.6}\},\{\mathrm{0.2,0.1}\left\}\right)$	$\left(\left\{\mathrm{0.2,0.2}\right\},\left\{\mathrm{0.5,0.2}\right\}\right)$
  $\left(\left\{\mathrm{0.3,0.1}\right\},\left\{\mathrm{0.2,0.5}\right\}\right)$	($\left\{\mathrm{0.3,0.5}\right\},\left\{\mathrm{0.1,0.1}\right\}$)	$\left(\left\{\mathrm{0.1,0.0}\right\},\left\{\mathrm{0.1,0.2}\right\}\right)$	$\left(\right\{\mathrm{0.3,0.2}\},\{\mathrm{0.2,0.2}\left\}\right)$	$\left(\left\{\mathrm{0.3,0.5}\right\},\left\{\mathrm{0.2,0.2}\right\}\right)$

• Step 2: The IHCE for every IHFS is calculated by using Definition 6, and thus, the calculated results are summarized in Table 2.

  Table 2. Developed IHCEs.

  ${\mathfrak{G}}_{\mathbf{1}}$	${\mathfrak{G}}_{\mathbf{2}}$	${\mathfrak{G}}_{\mathbf{3}}$	${\mathfrak{G}}_{\mathbf{4}}$	${\mathfrak{G}}_{\mathbf{5}}$
  $0.12+0.66\mathit{i}+0.22\mathit{j}$	$0.09+0.52i+0.39j$	$0.16+0.58i+0.26j$	$0.25+0.65i+0.1j$	$0.095+0.66\mathit{i}+0.245\mathit{j}$
  $0.04+0.77\mathit{i}+0.19\mathit{j}$	$0.04+0.82i+0.14j$	$0.08+0.74i+0.18j$	$0.115+0.72i+0.165j$	$0.205+0.69i+0.105j$
  $0.21+0.68\mathit{i}+0.11\mathit{j}$	$0.14+0.82i+0.04j$	$0.305+0.59i+0.105j$	$0.27+0.61i+0.12j$	$0.13+0.59i+0.28j$
  $0.145+0.56\mathit{i}+0.295\mathit{j}$	$0.36+0.58i+0.06j$	$0.045+0.81i+0.145j$	$0.2+0.65i+0.15j$	$0.32+0.56i+0.12j$

  Table 2. Developed IHCEs.

  ${\mathfrak{G}}_{\mathbf{1}}$	${\mathfrak{G}}_{\mathbf{2}}$	${\mathfrak{G}}_{\mathbf{3}}$	${\mathfrak{G}}_{\mathbf{4}}$	${\mathfrak{G}}_{\mathbf{5}}$
  $0.12+0.66\mathit{i}+0.22\mathit{j}$	$0.09+0.52i+0.39j$	$0.16+0.58i+0.26j$	$0.25+0.65i+0.1j$	$0.095+0.66\mathit{i}+0.245\mathit{j}$
  $0.04+0.77\mathit{i}+0.19\mathit{j}$	$0.04+0.82i+0.14j$	$0.08+0.74i+0.18j$	$0.115+0.72i+0.165j$	$0.205+0.69i+0.105j$
  $0.21+0.68\mathit{i}+0.11\mathit{j}$	$0.14+0.82i+0.04j$	$0.305+0.59i+0.105j$	$0.27+0.61i+0.12j$	$0.13+0.59i+0.28j$
  $0.145+0.56\mathit{i}+0.295\mathit{j}$	$0.36+0.58i+0.06j$	$0.045+0.81i+0.145j$	$0.2+0.65i+0.15j$	$0.32+0.56i+0.12j$

  Now, we apply the proposed operators to show the possible assessment of the emerging technology companies of the participants developing technology enterprises.
• Step 3: Using Equation (14), we calculate the supports $Sup ({\mathcal{J}}_{kt},{\mathcal{J}}_{kl})$ among the IHCEs. $(k=1,2,3,4;t,l=1,2,3,4,5)$
• Step 4: Evaluate the weights ${\xi }_{it}(i=1,2,3,4,t=1,2,3,4,5)$ by applying Equation (17) that are associated with the IHCEs, that include $R={\gamma }_{4\times 5}$

  $$\xi =\left[\begin{array}{c}0.266868\\ 0.263069\\ 0.266225\\ 0.260804\end{array}\begin{array}{c}0.34021\\ 0.345547\\ 0.338625\\ 0.343185\end{array}\begin{array}{c}0.075676\\ 0.074877\\ 0.07573\\ 0.074549\end{array}\begin{array}{c}0.18803\\ 0.191087\\ 0.19356\\ 0.192559\end{array}\begin{array}{c}0.129215\\ 0.125421\\ 0.12686\\ 0.128904\end{array}\right]$$
• Step 5: Utilize the IHCPWA operators and IHCPWG operators given in Equations (18) and (19), respectively, to obtain all IHCEs ${\mathcal{J}}_{it}(i=1,2,3,4,t=1,2,3,4,5)$ of the emerging enterprises ${\mathfrak{H}}_i$. The aggregating values are reflected in Table 3.

  Table 3. Aggregate the finding of the developing enterprises by IHCPWA and IHCPWG operators.

  	IHFCNPWA	IHFCNPWG
  ${\mathfrak{H}}_{\mathbf{1}}$	$0.136176+0.262893i+0.600931j$	$0.123867+0.609392i+0.266741$
  ${\mathfrak{H}}_{\mathbf{2}}$	$0.079838+0.156161i+0.76399j$	$0.063278+0.770114i+0.166608j$
  ${\mathfrak{H}}_{\mathbf{3}}$	$0.1972+0.113411i+0.68939j$	$0.185977+0.712337i+0.101686j$
  ${\mathfrak{H}}_{\mathbf{4}}$	$0.251833+0.148609i+0.599558j$	$0.213909+0.610781i+0.17531j$

  Table 3. Aggregate the finding of the developing enterprises by IHCPWA and IHCPWG operators.

  	IHFCNPWA	IHFCNPWG
  ${\mathfrak{H}}_{\mathbf{1}}$	$0.136176+0.262893i+0.600931j$	$0.123867+0.609392i+0.266741$
  ${\mathfrak{H}}_{\mathbf{2}}$	$0.079838+0.156161i+0.76399j$	$0.063278+0.770114i+0.166608j$
  ${\mathfrak{H}}_{\mathbf{3}}$	$0.1972+0.113411i+0.68939j$	$0.185977+0.712337i+0.101686j$
  ${\mathfrak{H}}_{\mathbf{4}}$	$0.251833+0.148609i+0.599558j$	$0.213909+0.610781i+0.17531j$

• Step 6: By Equation (20), the values obtained by applying the score function are produced in Table 4.

  Table 4. Results after applying score function.

  	IHCPWA	IHCPWG
  ${\mathfrak{H}}_{\mathbf{1}}$	$0.206531$	$0.38503$
  ${\mathfrak{H}}_{\mathbf{2}}$	$0.1045$	$0.408546$
  ${\mathfrak{H}}_{\mathbf{3}}$	$0.225995$	$0.572167$
  ${\mathfrak{H}}_{\mathbf{4}}$	$0.3003$	$0.531087$

  Table 4. Results after applying score function.

  	IHCPWA	IHCPWG
  ${\mathfrak{H}}_{\mathbf{1}}$	$0.206531$	$0.38503$
  ${\mathfrak{H}}_{\mathbf{2}}$	$0.1045$	$0.408546$
  ${\mathfrak{H}}_{\mathbf{3}}$	$0.225995$	$0.572167$
  ${\mathfrak{H}}_{\mathbf{4}}$	$0.3003$	$0.531087$

• Step 7: Agreeing to the score values given in Table 4 and evaluating the formula of score functions, the rank of the emerging technology companies is stated in Table 5. Recall that this idea $“>“$ indicates “preference.”

  Table 5. Ranking of the best attributes.

  	Ranking
  IHCPWA	${\mathfrak{H}}_4>{\mathfrak{H}}_3>{\mathfrak{H}}_1>{\mathfrak{H}}_2$
  IHCPWG	${\mathfrak{H}}_3>{\mathfrak{H}}_4>{\mathfrak{H}}_2>{\mathfrak{H}}_1$

  Table 5. Ranking of the best attributes.

  	Ranking
  IHCPWA	${\mathfrak{H}}_4>{\mathfrak{H}}_3>{\mathfrak{H}}_1>{\mathfrak{H}}_2$
  IHCPWG	${\mathfrak{H}}_3>{\mathfrak{H}}_4>{\mathfrak{H}}_2>{\mathfrak{H}}_1$

Comparative Study

Now, we will evaluate the execution of the suggested method with the current operators, power averaging aggregation, and power geometric aggregation based on intuitionistic hesitant fuzzy data as proposed by Tahir et al. [35]. We will consider the data of Example 1 for this comparative study, and the outcomes are presented in

Table 6. Comparative study with some existing approach discussed.

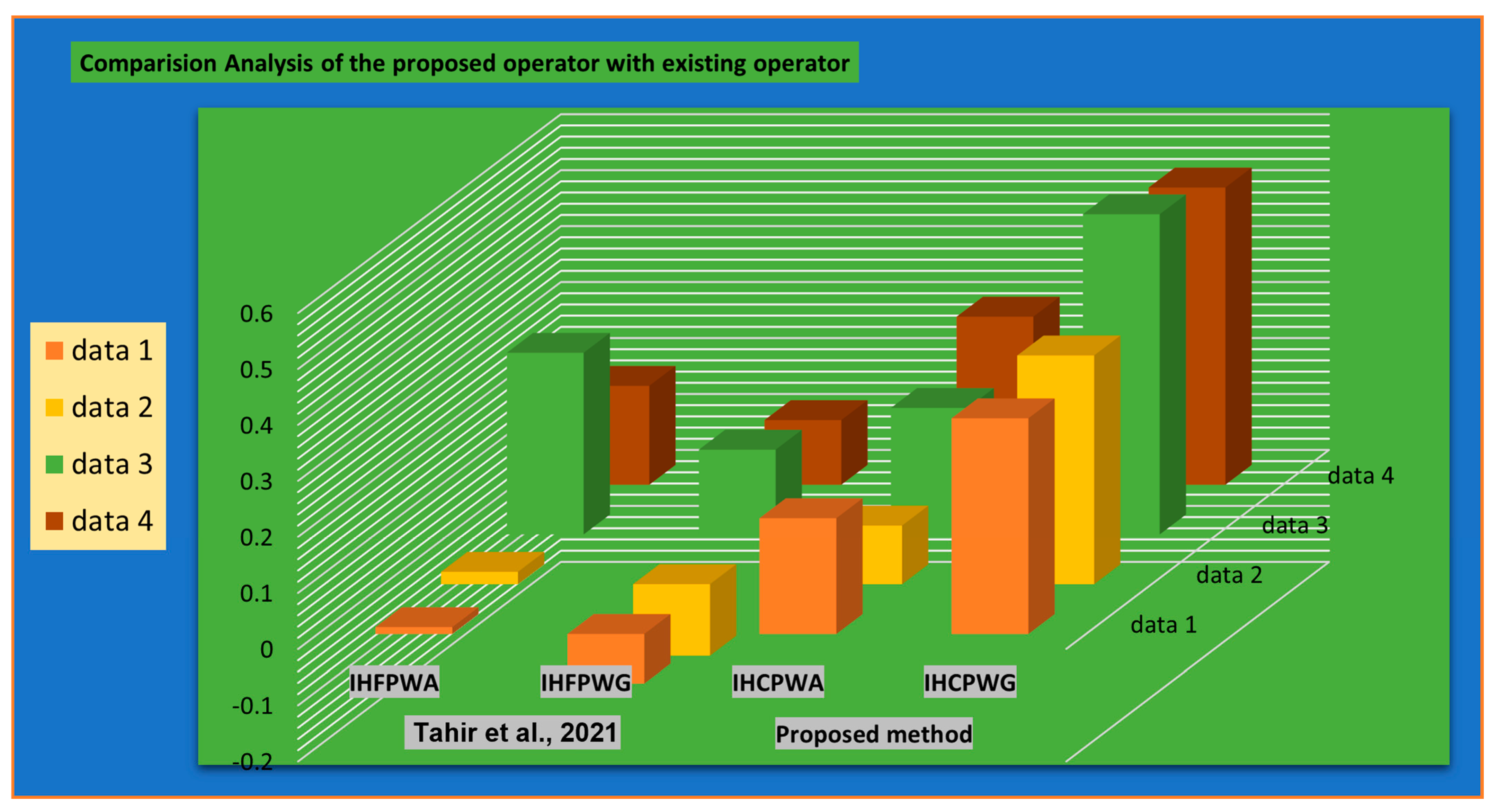
Methods		Ranking
Tahir et al. [35]	IHFPWA	${\mathfrak{H}}_3>{\mathfrak{H}}_4>{\mathfrak{H}}_2>{\mathfrak{H}}_1$
	IHFPWG	${\mathfrak{H}}_3>{\mathfrak{H}}_4>{\mathfrak{H}}_1>{\mathfrak{H}}_2$
Proposed method	IHCPWA	${\mathfrak{H}}_4>{\mathfrak{H}}_3>{\mathfrak{H}}_1>{\mathfrak{H}}_2$
	IHCPWG	${\mathfrak{H}}_3>{\mathfrak{H}}_4>{\mathfrak{H}}_2>{\mathfrak{H}}_1$

.

By comparison, we obtain the existing approach and the proposed method and their ranking results in Table 6 and Figure 2, which show the geometrical representation that will be helpful for understanding this comparative analysis.

The advantages and benefits of the proposed notion defined in this paper is a generalized form of the CN defined for the IFS. If we have a singleton set, then the IHFS reduces to the IFS and Definition 6 for the IHFCS reduces to the IFCNS. Therefore, the IHCS is capable of coping with the ambiguity and vagueness of the environment.

6. Conclusions

We have proposed an extension of the intuitionistic hesitant fuzzy set IHFS by integrating it with the CN of SPA, resulting in the intuitionistic hesitant fuzzy connection number set (IHCS). This development has filled certain existing gaps in MADM by facilitating the design of a novel MADM algorithm. The primary contribution of this manuscript is the presentation of novel aggregation operators and basic operational laws of the IHCNs. We explored the concept of power aggregation operators and developed a series of novel operators, including the IHCPA, IHCPG, IHCPWA, IHCPWG, IHCPOWA, IHCPOWG, IHCPHA, and IHCPHG, and verified their novelty with the deserved properties. We validated the proposed methodology by applying it to a real-life problem and demonstrating its feasibility and competency. Additionally, we conducted a comparison analysis of our approach with some existing AO models to establish its validity, authenticity, and effectiveness, and presented a graphical interpretation of the proposed approach. These operators and techniques have numerous applications, including networking analysis, risk assessment, cognitive science, and other areas in uncertain situations. We plan to construct more broadly applicable information metrics to comprehend the information that affects our daily life and investigate our novel techniques in the context of multi-criteria development in the fuzzy environment. We also intend to examine the idea behind our suggested methods within the perspective of square root fuzzy information [57] and ongoing research using a Pythagorean fuzzy system [58].