A Soliton Solution for the Kadomtsev–Petviashvili Model Using Two Novel Schemes
Abstract
1. Introduction
2. General Description and Application of the Proposed Methods
2.1. The Modified Sardar Sub-Equation Technique
- Case 1:
- If , and , then
- If , and , then
- Case 2:
- For constants and , let , and ; then,
- Case 3:
- For constants and , let and ; then,
- For constants and , let , and ; then,
- For constants and , let , and ; then,
- For constants and , let , and ; then,
- For constants and , let , and ; then,
- Case 4:
- Let , and ; then,
- Let , and ; then,
- Case 5:
- Let , , and ; then,
- Let , , and ; then,
- Let , , and ; then,
- Let , , and ; then,
- Let , , and ; then,
- Case 6:
- Let and then,
- Let and then,
- Case 7:
- Let , and ; then,
- Let , and ; then,
2.2. Application of the Modified Sardar Sub-Equation Technique
- Case 1:
- If , and , then
- If , and , then
- Case 2:
- For constants and , let , and ; then,
- Case 3:
- For constants and , let and ; then,
- For constants and , let , and ; then,
- For constants and , let and ; then,
- For constants and , let and ; then,
- For constants and , let and ; then,
- Case 4:
- Let , and ; then,
- Let , and ; then,
- Case 5:
- Let , , and ; then,
- Let , and ; then,
- Let , and ; then,
- Let and ; then,
- Let , and ; then,
- Case 6:
- Let and then,
- Let and then,
- Case 7:
- Let and ; then,
- Let and ; then,
3. Darboux Transformation
3.1. The N-Fold
3.2. Asymptotic State Analysis and Solutions to Bright–Dark Multi-Soliton Systems
3.2.1. Dynamic Analysis and One-Soliton Solutions
3.2.2. Analysis of Two-Soliton Solutions Asymptotically
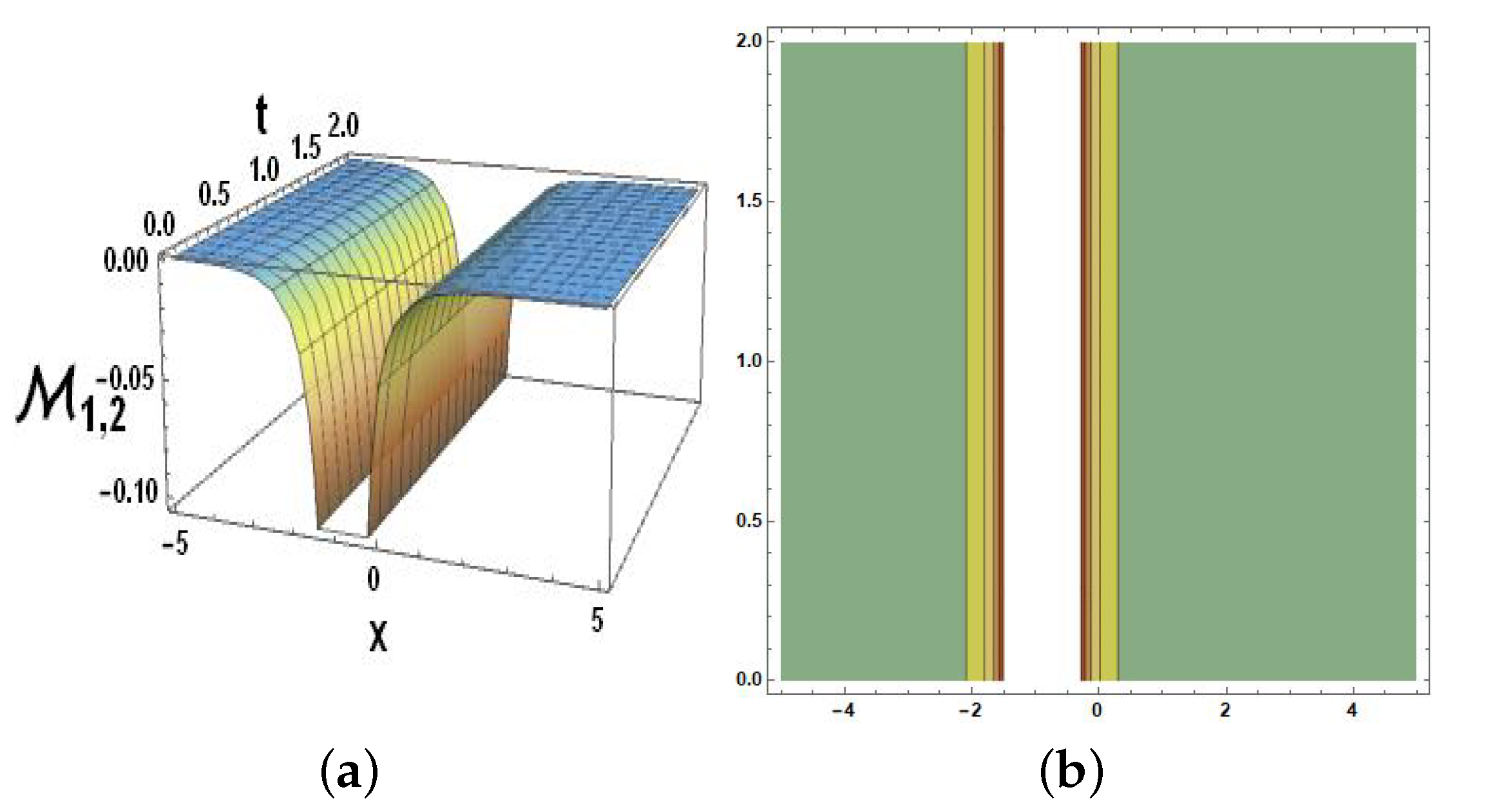
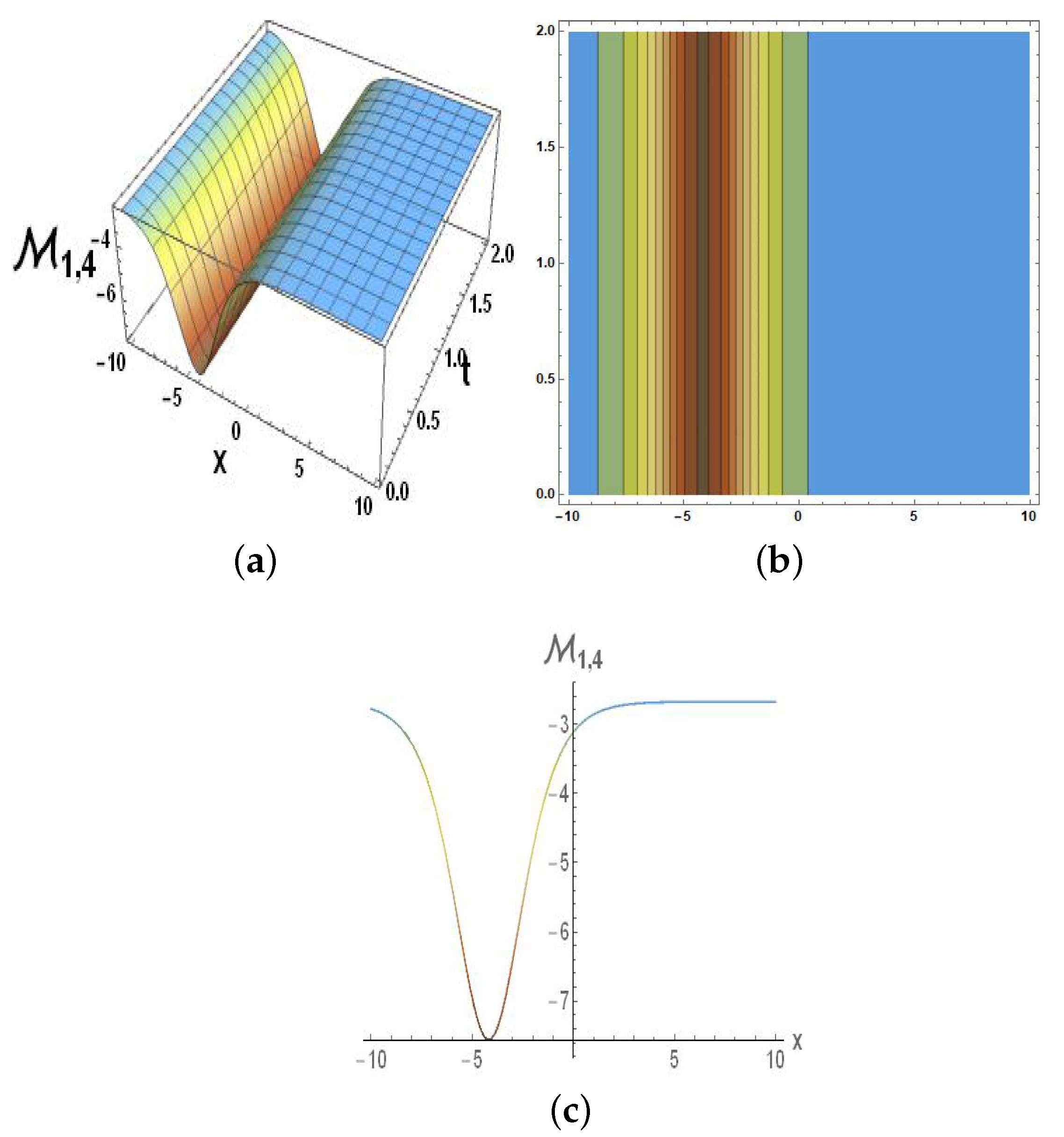
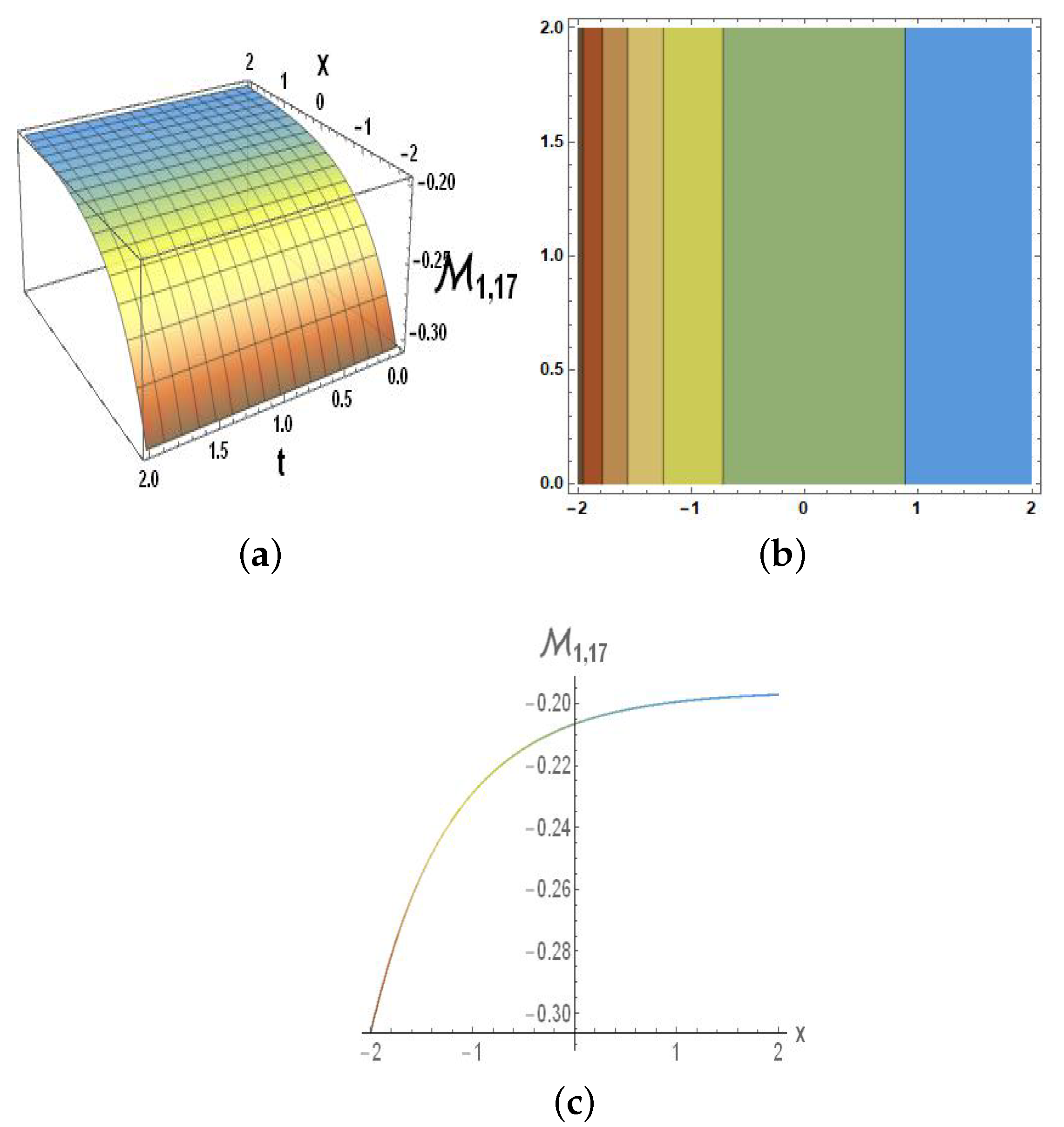
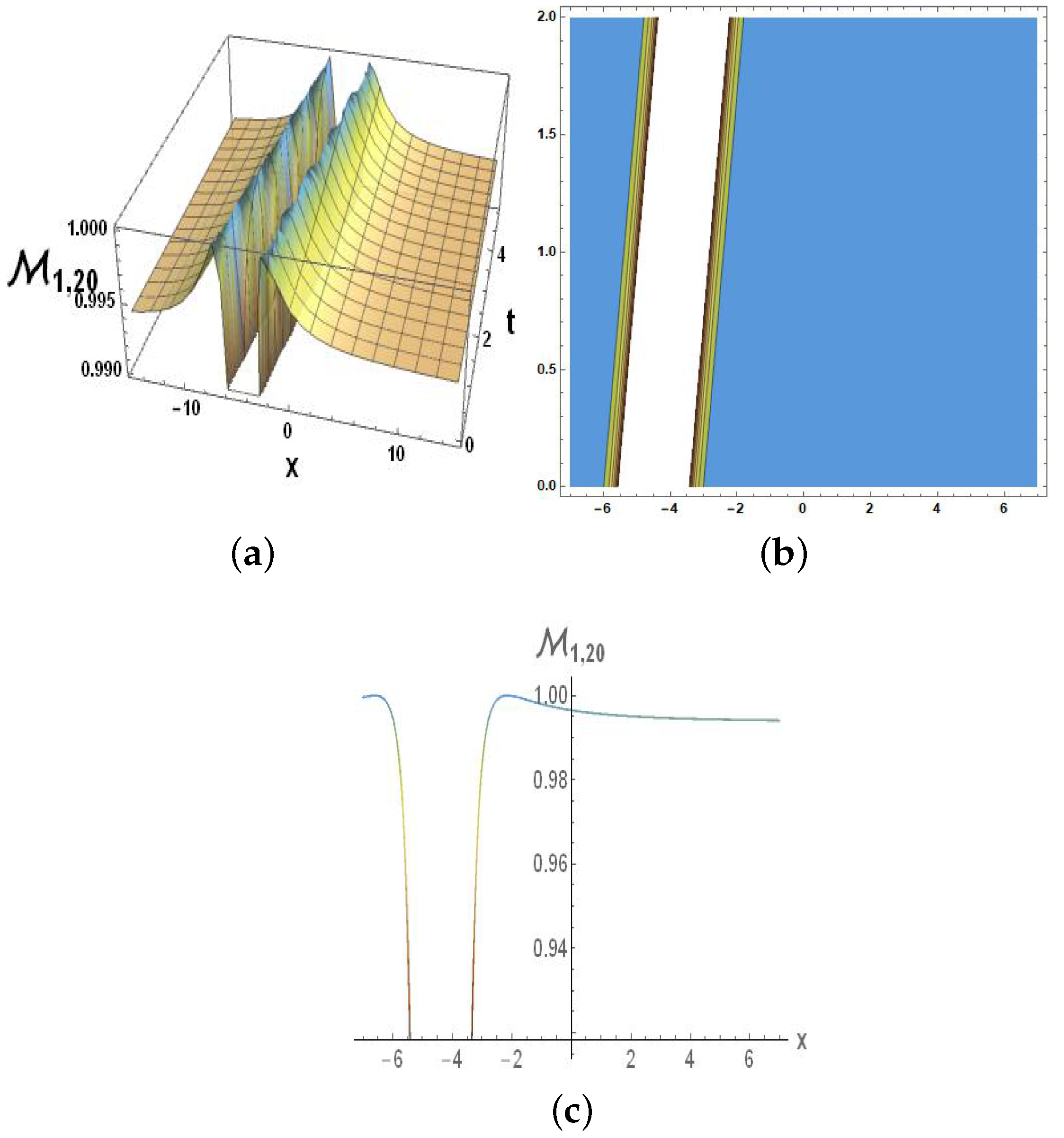
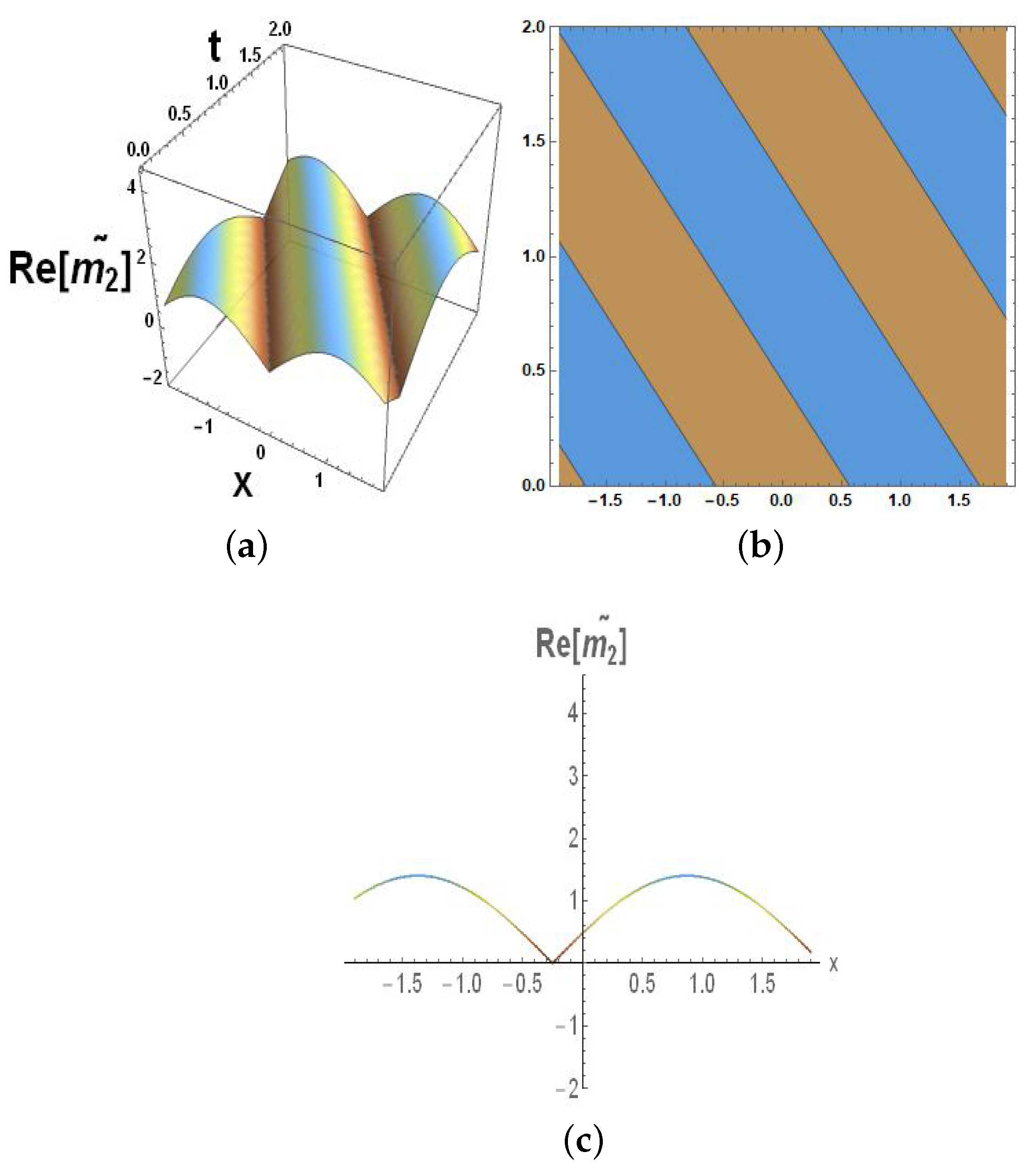
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jin, H.Y.; Wang, Z.A. Global stabilization of the full attraction-repulsion Keller-Segel system. Discret. Contin. Dyn. Syst. Ser. A 2020, 40, 3509–3527. [Google Scholar] [CrossRef]
- Triki, H.; Wazwaz, A.M. On soliton solutions for the Fitzhugh–Nagumo equation with time-dependent coefficients. Appl. Math. Model. 2013, 37, 3821–3828. [Google Scholar] [CrossRef]
- Guo, C.; Hu, J. Fixed-Time Stabilization of High-Order Uncertain Nonlinear Systems: Output Feedback Control Design and Settling Time Analysis. J. Syst. Sci. Complex. 2023. [CrossRef]
- Xie, X.; Huang, L.; Marson, S.M.; Wei, G. Emergency response process for sudden rainstorm and flooding: Scenario deduction and Bayesian network analysis using evidence theory and knowledge meta-theory. Nat. Hazards 2023, 117, 3307–3329. [Google Scholar] [CrossRef]
- Rafiq, M.H.; Raza, N.; Jhangeer, A. Dynamic study of bifurcation, chaotic behavior and multi-soliton profiles for the system of shallow water wave equations with their stability. Chaos Solitons Fractals 2023, 171, 113436. [Google Scholar] [CrossRef]
- Zhong, Q.; Han, S.; Shi, K.; Zhong, S.; Kwon, O. Co-Design of Adaptive Memory Event-Triggered Mechanism and Aperiodic Intermittent Controller for Nonlinear Networked Control Systems. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 4979–4983. [Google Scholar] [CrossRef]
- Guo, C.; Hu, J. Time base generator based practical predefined-time stabilization of high-order systems with unknown disturbance. IEEE Trans. Circuits Syst. II Express Briefs 2023. [Google Scholar] [CrossRef]
- Alotaibi, M.F.; Raza, N.; Rafiq, M.H.; Soltani, A. New solitary waves, bifurcation and chaotic patterns of Fokas system arising in monomode fiber communication system. Alex. Eng. J. 2023, 67, 583–595. [Google Scholar] [CrossRef]
- Malik, S.; Hashemi, M.S.; Kumar, S.; Rezazadeh, H.; Mahmoud, W.; Osman, M.S. Application of new Kudryashov method to various nonlinear partial differential equations. Opt. Quantum Electron. 2023, 55, 8. [Google Scholar] [CrossRef]
- Wang, L.; She, A.; Xie, Y. The dynamics analysis of Gompertz virus disease model under impulsive control. Sci. Rep. 2023, 13, 10180. [Google Scholar] [CrossRef]
- Raza, N.; Arshed, S.; Wazwaz, A.M. Structures of interaction between lump, breather, rogue and periodic wave solutions for new (3+1)-dimensional negative order KdV-CBS model. Phys. Lett. A 2023, 458, 128589. [Google Scholar] [CrossRef]
- Shakeel, M.; Shah, N.A.; Chung, J.D. Application of modified exp-function method for strain wave equation for finding analytical solutions. Ain Shams Eng. J. 2023, 14, 101883. [Google Scholar] [CrossRef]
- Ali, A.; Ahmad, J.; Javed, S. Solitary wave solutions for the originating waves that propagate of the fractional Wazwaz-Benjamin-Bona-Mahony system. Alex. Eng. J. 2023, 69, 121–133. [Google Scholar] [CrossRef]
- Rafiq, M.H.; Jhangeer, A.; Raza, N. The analysis of solitonic, supernonlinear, periodic, quasiperiodic, bifurcation and chaotic patterns of perturbed Gerdjikov–Ivanov model with full nonlinearity. Commun. Nonlinear Sci. Numer. Simul. 2023, 116, 106818. [Google Scholar] [CrossRef]
- Liu, Q.; Peng, H.; Wang, Z. Convergence to nonlinear diffusion waves for a hyperbolic-parabolic chemotaxis system modelling vasculogenesis. J. Differ. Equations 2022, 314, 251–286. [Google Scholar] [CrossRef]
- Malomed, B.A.; Mihalache, D. Nonlinear waves in optical and matter-wave media: A topical survey of recent theoretical and experimental results. Rom. J. Phys. 2019, 64, 106. [Google Scholar]
- Cárdenas-Castillo, L.F.; Camacho-Guardian, A. Strongly Interacting Bose Polarons in Two-Dimensional Atomic Gases and Quantum Fluids of Polaritons. Atoms 2023, 11, 3. [Google Scholar] [CrossRef]
- Chen, J.; Pelinovsky, D.E. Periodic waves in the discrete mKdV equation: Modulational instability and rogue waves. Phys. Nonlinear Phenom. 2023, 9, 133652. [Google Scholar] [CrossRef]
- Gu, Y.; Zia, S.M.; Isam, M.; Manafian, J.; Hajar, A.; Abotaleb, M. Bilinear method and semi-inverse variational principle approach to the generalized (2+ 1)-dimensional shallow water wave equation. Results Phys. 2023, 9, 106213. [Google Scholar] [CrossRef]
- Butt, A.R.; Raza, N.; Inc, M.; Alqahtani, R.T. Complexitons, Bilinear forms and Bilinear Bäcklund transformation of a (2+1)- dimensional Boiti–Leon–Manna–Pempinelli model describing incompressible fluid. Chaos Solitons Fractals 2023, 168, 113201. [Google Scholar] [CrossRef]
- Wazwaz, A.M.; Alyousef, H.A.; El-Tantawy, S. An extended Painlevé integrable Kadomtsev–Petviashvili equation with lumps and multiple soliton solutions. Int. J. Numer. Methods Heat Fluid Flow 2023, 33, 2533–2543. [Google Scholar] [CrossRef]
- Yang, S.X.; Wang, Y.F.; Zhang, X. Conservation laws, Darboux transformation and localized waves for the N-coupled nonautonomous Gross–Pitaevskii equations in the Bose–Einstein condensates. Chaos Solitons Fractals 2023, 169, 113272. [Google Scholar] [CrossRef]
- Shen, Y.; Tian, B.; Zhou, T.Y.; Gao, X.T. N-fold Darboux transformation and solitonic interactions for the Kraenkel–Manna–Merle system in a saturated ferromagnetic material. Nonlinear Dyn. 2023, 111, 2641–2649. [Google Scholar] [CrossRef]
- Yang, Y.; Xia, T.; Liu, T. Darboux transformation and exact solution to the nonlocal Kundu–Eckhaus equation. Appl. Math. Lett. 2023, 141, 108602. [Google Scholar] [CrossRef]
- Alquran, M. Optical bidirectional wave-solutions to new two-mode extension of the coupled KdV–Schrodinger equations. Opt. Quantum Electron. 2021, 53, 588. [Google Scholar] [CrossRef]
- Ablowitz, M.J. Nonlinear Waves and the Inverse Scattering Transform. Optik 2023, 278, 170710. [Google Scholar] [CrossRef]
- Alquran, M. Physical properties for bidirectional wave solutions to a generalized fifth-order equation with third-order time-dispersion term. Results Phys. 2021, 28, 104577. [Google Scholar] [CrossRef]
- Ran, X.; Wang, X.H.; Wei, T.F.; Qu, S.W.; Wang, B.Z. Inverse-Designed Superstrate for Arbitrary Shaped-Beam Radiation Pattern Based on Inverse Scattering Method. IEEE Trans. Antennas Propag. 2023, 71, 3828–3835. [Google Scholar]
- Alquran, M. New interesting optical solutions to the quadratic–cubic Schrodinger equation by using the Kudryashov-expansion method and the updated rational sine–cosine functions. Opt. Quantum Electron. 2022, 54, 666. [Google Scholar] [CrossRef]
- Ali, M.R.; Khattab, M.A.; Mabrouk, S.M. Optical soliton solutions for the integrable Lakshmanan-Porsezian-Daniel equation via the inverse scattering transformation method with applications. Optik 2023, 272, 170256. [Google Scholar] [CrossRef]
- Wazwaz, A.M.; El-Tantawy, S.A. A new (3+1)-dimensional generalized Kadomtsev–Petviashvili equation. Nonlinear Dyn. 2016, 84, 1107–1112. [Google Scholar] [CrossRef]












| Solitons | Intensity | Dimensions | Speed | Wave Numbers | Main Phases | Energies |
|---|---|---|---|---|---|---|
| 0 | ||||||
| 0 |
| Soliton | Amplitude | Width | Velocity | Wave Numbers | Primary Phase | Energy |
|---|---|---|---|---|---|---|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, A.; Javed, S.; Nadeem, M.; Iambor, L.F.; Mureşan, S. A Soliton Solution for the Kadomtsev–Petviashvili Model Using Two Novel Schemes. Symmetry 2023, 15, 1364. https://doi.org/10.3390/sym15071364
Ali A, Javed S, Nadeem M, Iambor LF, Mureşan S. A Soliton Solution for the Kadomtsev–Petviashvili Model Using Two Novel Schemes. Symmetry. 2023; 15(7):1364. https://doi.org/10.3390/sym15071364
Chicago/Turabian StyleAli, Asghar, Sara Javed, Muhammad Nadeem, Loredana Florentina Iambor, and Sorin Mureşan. 2023. "A Soliton Solution for the Kadomtsev–Petviashvili Model Using Two Novel Schemes" Symmetry 15, no. 7: 1364. https://doi.org/10.3390/sym15071364
APA StyleAli, A., Javed, S., Nadeem, M., Iambor, L. F., & Mureşan, S. (2023). A Soliton Solution for the Kadomtsev–Petviashvili Model Using Two Novel Schemes. Symmetry, 15(7), 1364. https://doi.org/10.3390/sym15071364








