Longitudinal–Transverse Vibration of a Functionally Graded Nanobeam Subjected to Mechanical Impact and Electromagnetic Actuation
Abstract
1. Introduction
2. Derivation of the Governing Equations
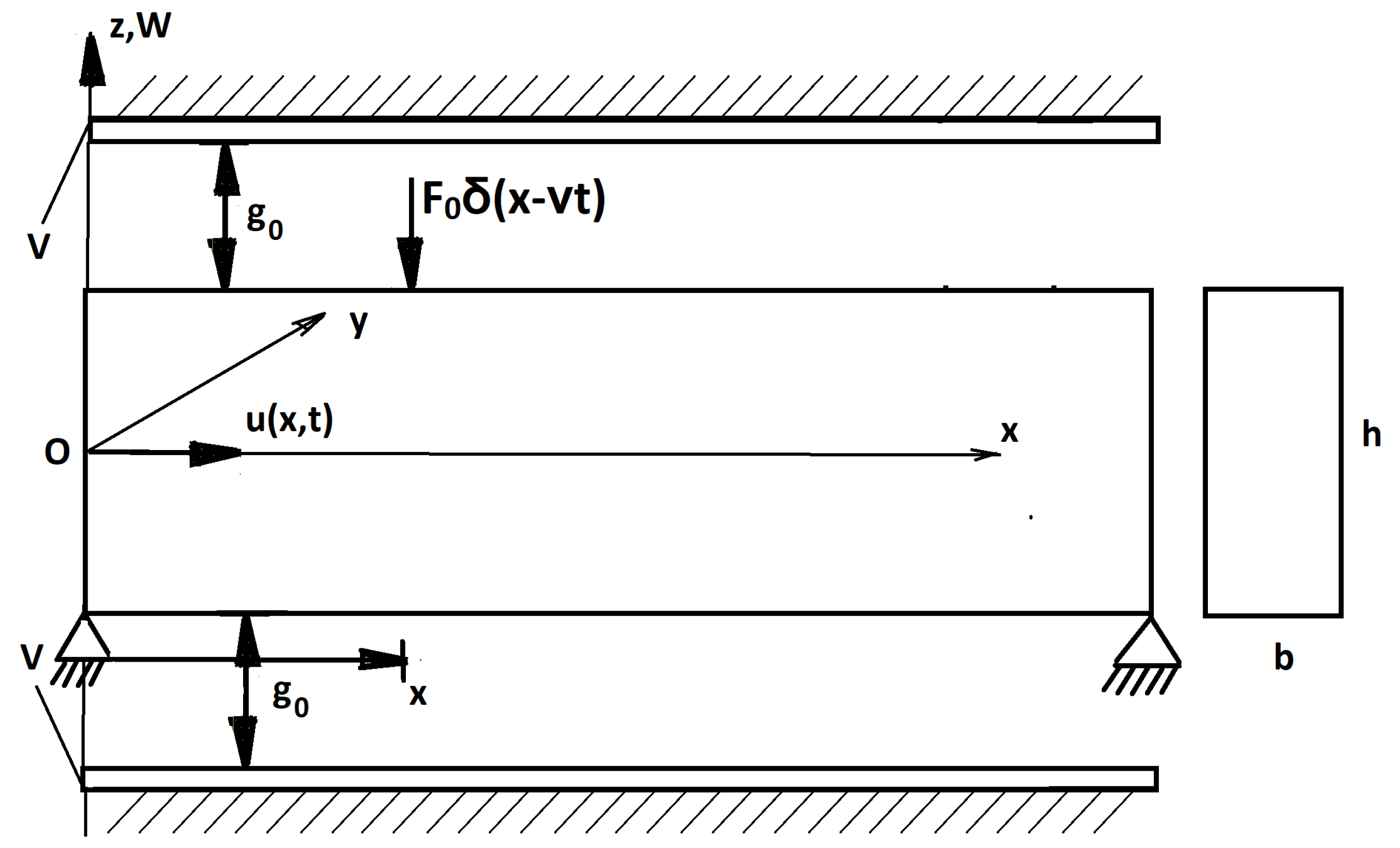
2.1. Modeling of the Functionally Nanobeam
2.2. Kinematic Relations
2.3. The Governing Equation for FG Nanobeam
3. Solution Procedure
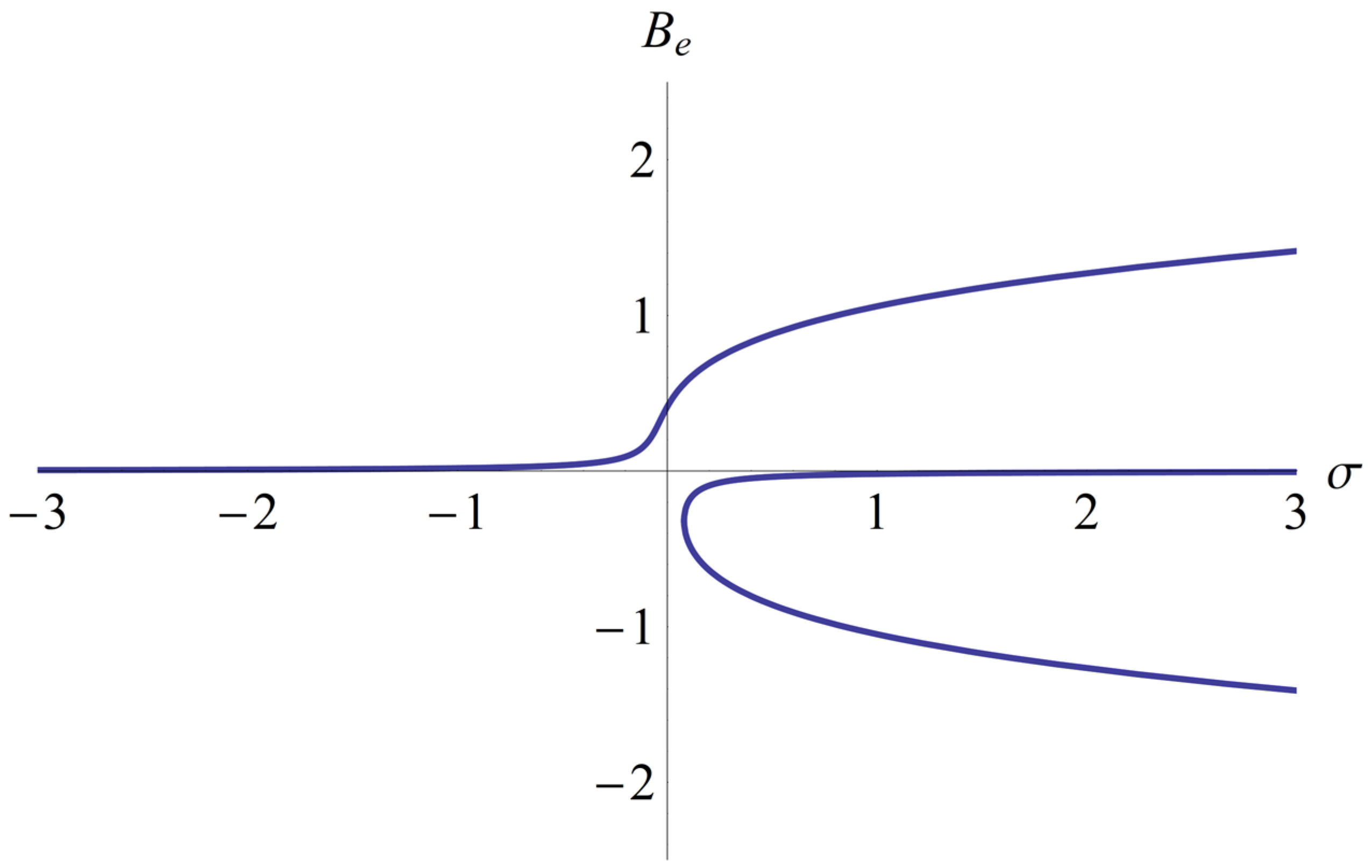
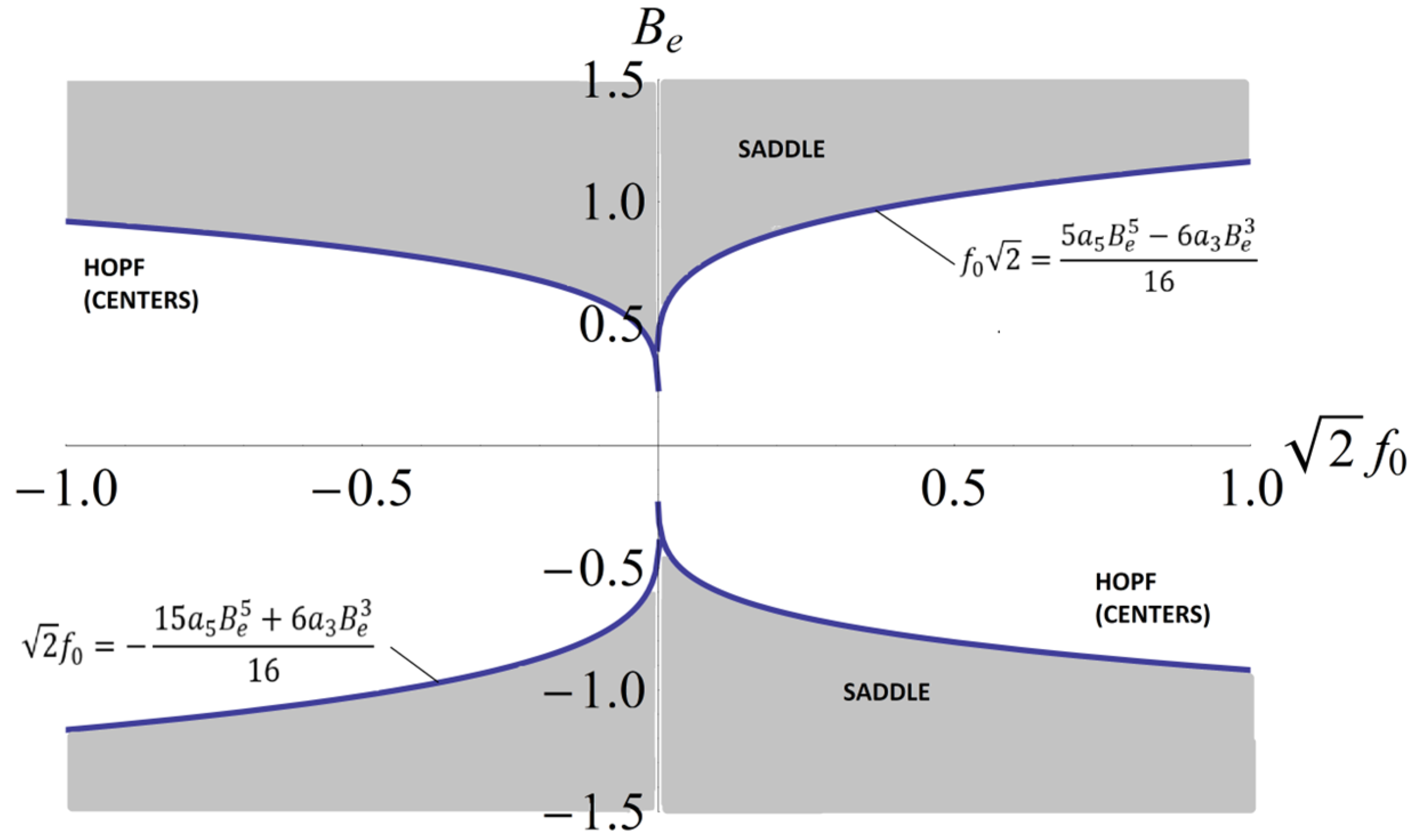
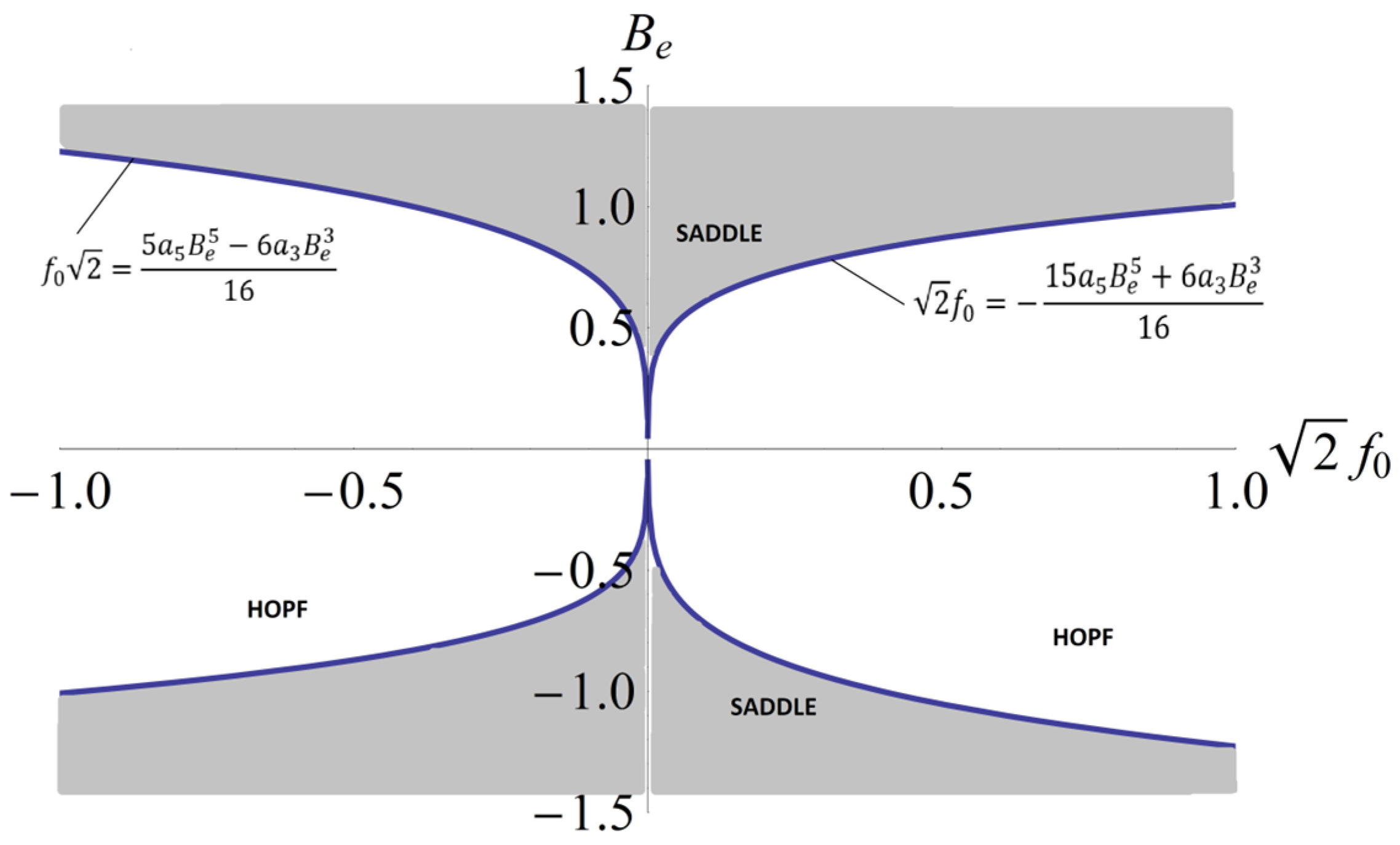
4. Stability of the Steady–State Motion for the FG Nanobeam
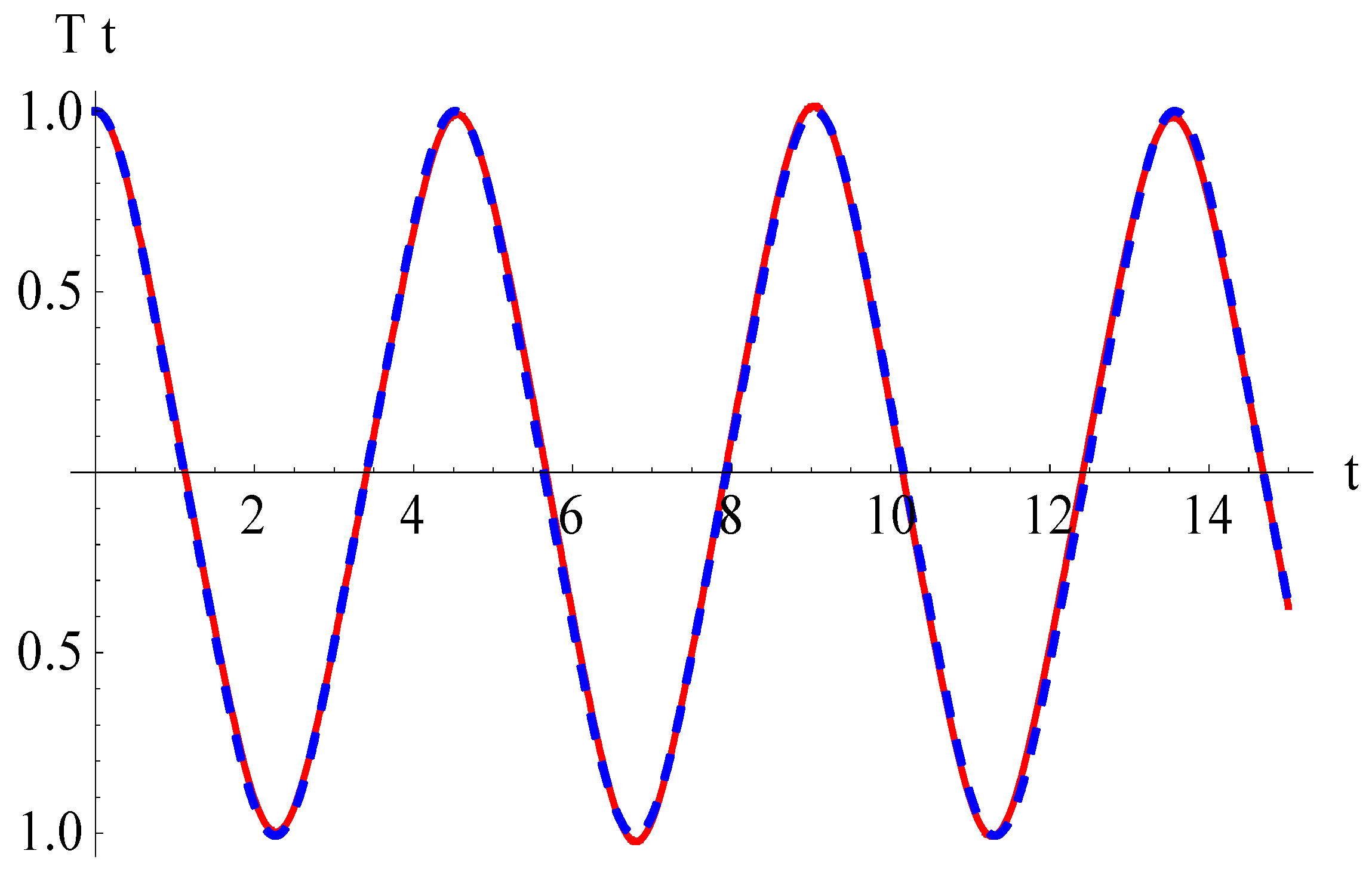
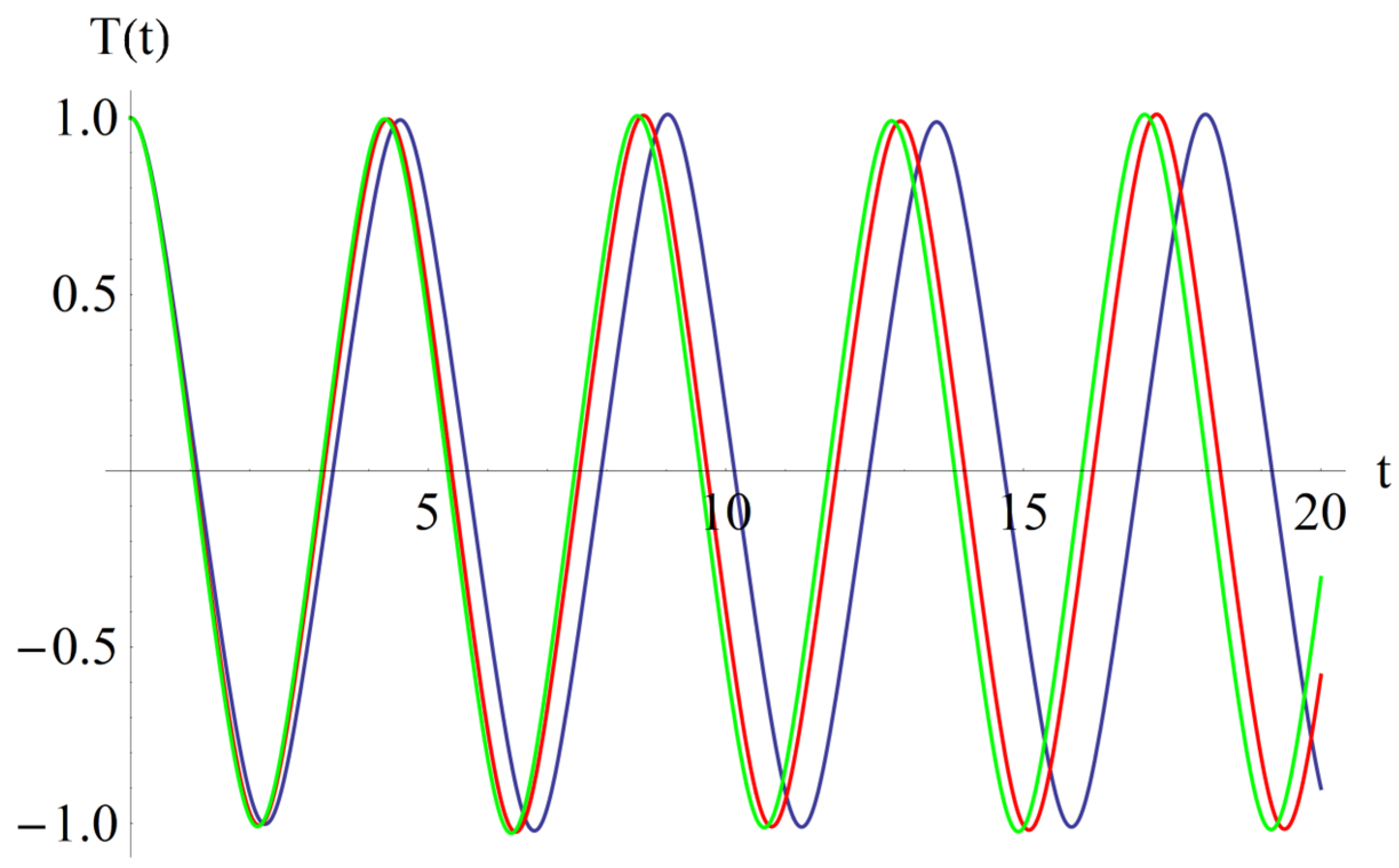
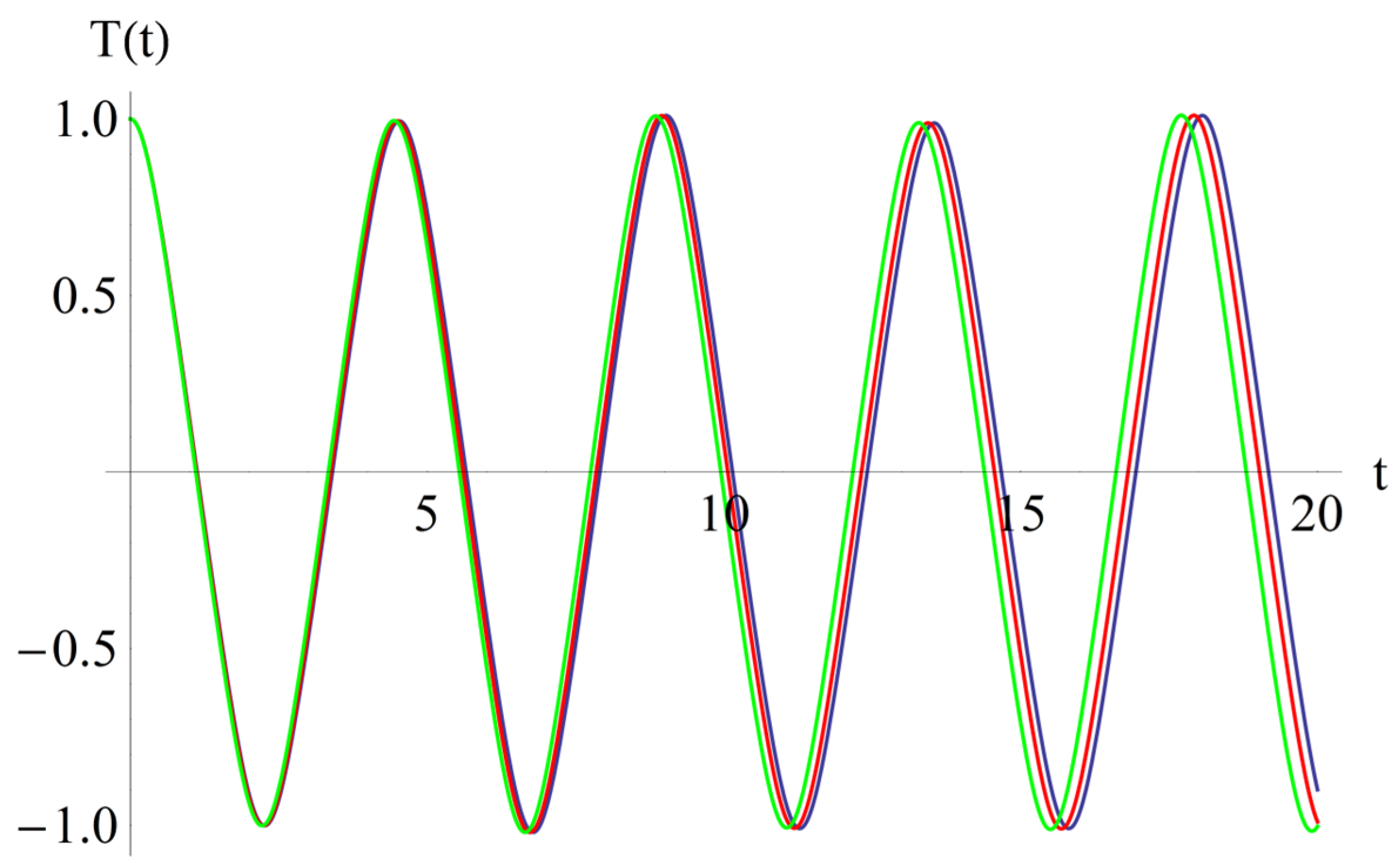
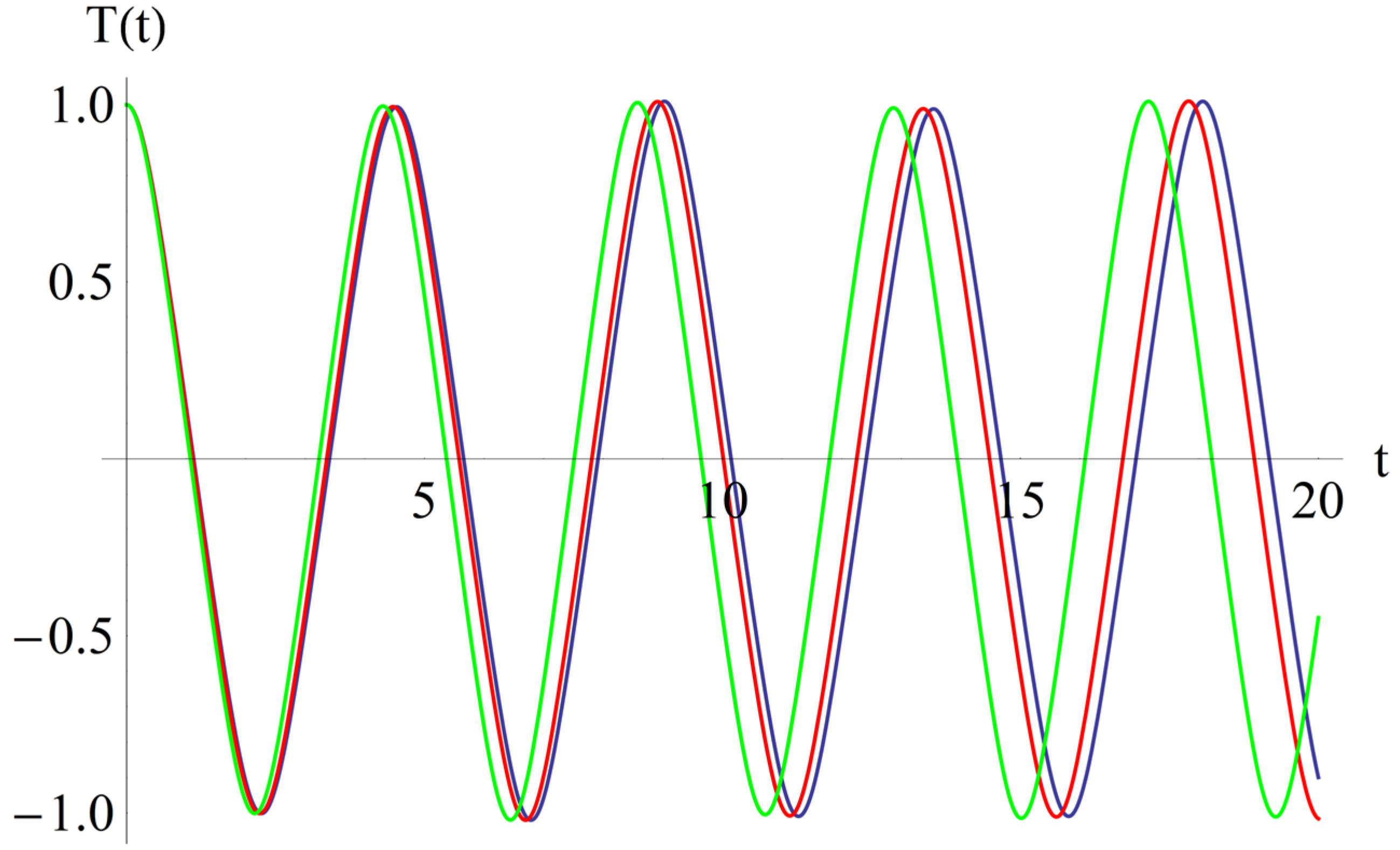
5. Numerical Examples
6. Global Stability by the Lyapunov Function
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Miyamoto, Y.; Kaysser, W.A.; Rabin, B.H.; Kawasaki, A.; Ford, R.G. Functionally Graded Materials; Springer: New York, NY, USA, 1999. [Google Scholar]
- Alimoradzadeh, M.; Salemi, M.; Esfarjani, S.M. Nonlinear dynamic response of an axially functionally graded (AFG) beam resting on nonlinear elastic foundation subjected to moving load. Nonlinear Eng. 2019, 8, 250–260. [Google Scholar] [CrossRef]
- Shafiei, H.; Setoodei, A.R. An analytical study on the nonlinear forced vibration of functionally graded carbon nanotube-reinforced composite beams on nonlinear viscoelastic foundation. Arch. Mech. 2020, 72, 81–107. [Google Scholar]
- Ansari, R.; Gholami, R.; Shojaei, M.F.; Mohammadi, V.; Darabi, M.A. Coupled longitudinal-transverse-rotational free vibration of post-buckled functionally graded first-order shear deformable micro- and nano-beams on the Mindlin’s strain gradient theory. Appl. Math. Model. 2016, 40, 9872–9891. [Google Scholar] [CrossRef]
- Mu, L.; Zhao, G. Fundamental frequency analysis of sandwich beams functionally graded face and metallic foam core. Shock Vibr. 2016, 2016, 3287645. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Barati, M.R. A nonlocal higher-order shear deformation beam theory for vibration analysis of size-dependent functionally graded nanobeams. Arab. J. Sci. Eng. 2016, 41, 1679–1690. [Google Scholar] [CrossRef]
- Gangnian, X.; Lian, M.; Youzni, W.; Quan, Y.; Weijie, Y. Differential quadrature method of nonlinear bending of functionally graded beam. Mater. Sci. Eng. 2008, 307, 012058. [Google Scholar] [CrossRef]
- Reddy, J.N.; Ruoco, E.; Loya, J.A.; Neves, A.M.A. Theories and analysis of functionally graded beams. Appl. Sci. 2021, 11, 7159. [Google Scholar] [CrossRef]
- Fattani, A.M.; Sahmani, S.; Ahmed, N.A. Nonlocal strain gradient beam model for nonlinear secondary resonance analysis of functionally graded porous micro/nano-beams under periodic hard excitations. Mech. Based Des. Struct. Mach. 2019, 48, 1–30. [Google Scholar]
- Long, N.V.; Nguyen, V.L.; Tran, M.T.; Thai, D.K. Exact solution for nonlinear static behaviors of functionally graded beams with porosities resting on elastic foundation using neutral surface concept. J Mech. Eng. Sci. 2022, 236, 481–495. [Google Scholar] [CrossRef]
- Wu, J.; Chen, L.; We, R.; Chen, X. Nonlinear forced vibration of bidirectional functionally graded porous material beam. Shock Vibr. 2021, 2021, 6675125. [Google Scholar] [CrossRef]
- Alhaifi, K.; Arshid, E.; Khorshidvand, A.R. Large deflection analysis of functionally graded saturated porous rectangular plates on nonlinear elastic foundation via GDQM. Steel Comp. Struct. 2021, 39, 795–809. [Google Scholar]
- Dang, V.H.; Nguyen, T.H. Buckling and nonlinear vibration of functionally graded porous microbeam resting on elastic foundation. Mech. Adv. Compos. Struct. 2022, 9, 75–88. [Google Scholar]
- Yas, M.H.; Rahimi, S. Thermal vibration of functionally graded porous nanocomposite beams reinforced with graphene platelets. Appl. Math. Mech. 2020, 41, 1209–1226. [Google Scholar] [CrossRef]
- Yang, W.D.; Yang, F.P.; Wang, X. Coupling influences of nonlocal stress and strain gradient on dynamic pull-in of functionally graded nanotubes reinforced nano-actuator with damping effects. Sens. Actuators A Phys. 2016, 248, 101021. [Google Scholar] [CrossRef]
- Kashyzadeh, K.R.; Asfarjani, A.A. Finite element study in the vibration of functionally graded beam with different temperature conditions. Adv. Mater. 2016, 5, 57–65. [Google Scholar] [CrossRef]
- Nguyen, D.K.; Bui, V.T. Dynamic analysis of functionally graded Timoshenko beams in thermal environment using a higher-order hierarchical beam element. Math. Probl. Eng. 2017, 2017, 7025750. [Google Scholar] [CrossRef]
- Fan, J.; Huang, J. Haar wavelet method for nonlinear vibration of functionally graded CHT-reinforced composite beams resting on nonlinear elastic foundations in thermal environment. Shock Vibr. 2018, 2018, 9597541. [Google Scholar]
- Shafiei, N.; Hamisi, M.; Ghadiri, M. Vibration analysis of rotary tapered axially functionally graded Timoshenko nanobeams in thermal environment. J. Solid Mech. 2020, 12, 16–32. [Google Scholar]
- Zhou, Z.; Chen, M.; Jia, W. Free vibration analysis of axially functionally graded double-tapered Timoshenko beam by NURBS approach. In Proceedings of the 30th International Ocean and Polar Engineering Conference, Shanghai, China, 11–16 October 2020. [Google Scholar]
- Sari, M.S.; Al-Kouz, W.G.; Atieh, A.M. Transverse vibration of functionally graded tapered double nanobeams resting on elastic foundation. Appl. Sci. 2020, 10, 493. [Google Scholar] [CrossRef]
- Su, Z.; Jin, G.; Ye, T. Vibration analysis and transient response of a functionally graded piezoelectric curved beam with boundary conditions. Smart Mater. Struct. 2016, 25, 065003. [Google Scholar] [CrossRef]
- Nasirzadeh, R.; Benjat, B.; Kharazi, M. Finite element study on thermal buckling of functionally graded piezoelectric beams considering inverse effects. J. Theor. Appl. Mech. 2018, 56, 1097–1108. [Google Scholar] [CrossRef]
- Ma, X.; Wang, S.; Zhou, B.; Xue, S. Study of the electromechanical behavior of functionally graded piezoelectric composite beams. J. Mech. 2020, 36, 841–848. [Google Scholar] [CrossRef]
- Singh, A.; Kumari, P. Two-dimensional free vibration analysis of axially functionally graded beams integrated with piezoelectric layers: A piezoelectric approach. Int. J. Appl. Mech. 2020, 12, 2050037. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, M.; Su, Y.; Zhou, Z. Coupling analysis of flexoelectric effect of functionally graded piezoelectric cantilever nanobeams. Micromachines 2021, 12, 595. [Google Scholar] [CrossRef]
- El Knoudoar, Y.; Adri, A.; Oufassafte, O.; Rifai, S.; Benamar, R. Nonlinear forced vibration analysis of piezoelectric functionally graded beams in thermal environment. Int. J. Eng. 2021, 34, 2587–2597. [Google Scholar]
- Nazmul, I.M.; Nahed, S.; Indromil, D. Analytical solutions for vibration of bi-directional functionally graded nonlocal nanobeams. Results Eng. 2023, 2023, 101046. [Google Scholar]
- Fang, L.; Yin, B.; Zhang, X.; Yang, B. Size-dependent vibration of functionally graded rotating nanobeams with different boundary conditions based on nonlocal elasticity theory. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2021, 236, 2756–2774. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Marin, M.; Askar, S.S. Analysis of the magneto-thermoelastic vibrations of rotating Euler–Bernoulli nanobeams using the nonlocal elasticity model. Bound. Value Probl. 2023, 2023, 21. [Google Scholar] [CrossRef]
- Lal, R.; Dangi, C. Thermomechanical vibration of bi-directional functionally graded non-uniform Timoshenko nanobeam using nonlocal elasticity theory. Compos. Part B Eng. 2019, 172, 724–742. [Google Scholar] [CrossRef]
- Sun, L.; Zhang, C.; Yu, Y. A boundary knot method for 3D time harmonic elastic wave problems. Appl. Math. Lett. 2020, 104, 106210. [Google Scholar] [CrossRef]
- Yu, Y.; Zhang, C.; Chen, Z.; Lim, C.W. Relaxation and mixed mode oscillations in a shape memory alloy oscillator driven by parametric and external excitations. Chaos Solitons Fractals 2020, 140, 110145. [Google Scholar] [CrossRef]
- Chen, D.; Wang, B.; Chen, Z.; Yu, Y. Parametrically excited vibrations in a nonlinear damped triple-well oscillator with resonant frequency. J. Vibr. Eng. Techn. 2022, 10, 781–788. [Google Scholar] [CrossRef]
- Malikan, M.; Wiczenbach, T.; Eremeyev, V.A. Thermal buckling of functionally graded piezomagnetic micro- and nanobeams presenting the flexomagnetic effect. Contin. Mech. Thermodyn. 2022, 34, 1051–1066. [Google Scholar] [CrossRef]
- Jalaei, M.H.; Thai, H.T.; Civalek, O. On viscoelastic transient response of magnetically imperfect functionally graded nanobeams. Int. J. Eng. Sci. 2022, 172, 103629. [Google Scholar] [CrossRef]
- Al-Zahrani, M.A.; Asiri, S.A.; Ahmed, K.I.; Eltaher, M.A. Free vibration analysis of 2D functionally graded strip beam using finite element method. J. Appl. Comput. Mech. 2022, 8, 1422–1430. [Google Scholar]
- Malikan, M.; Eremeyev, V.A. On time-dependent nonlinear dynamic response of micro-elastic solids. Int. J. Eng. Sci. 2023, 182, 103793. [Google Scholar] [CrossRef]
- Civalek, O.; Uzun, B.; Yaylı, M.O. On nonlinear stability analysis of saturated embedded porous nanobeams. Int. J. Eng. Sci. 2023, 190, 103898. [Google Scholar] [CrossRef]
- Lim, C.W.; Zhang, G.; Reddy, J.N. A higher-order nonlocal elasticity and strain gradient theory and its applications in wave propagation. J. Mech. Phys. Solids 2015, 78, 298–313. [Google Scholar] [CrossRef]
- Herisanu, N.; Marinca, V. An efficient analytical approach to investigate the dynamics of a misaligned multirotor system. Mathematics 2020, 8, 1083. [Google Scholar] [CrossRef]
- Marinca, V.; Herisanu, N.; Marinca, B. Optimal Auxiliary Functions Method for Nonlinear Dynamical Systems; Springer: Cham, Switzerland, 2021. [Google Scholar]
- Herisanu, N.; Marinca, V. An effective analytical approach to nonlinear free vibration of elastically actuated microtubes. Meccanica 2021, 56, 812–823. [Google Scholar] [CrossRef]
- Marinca, B.; Marinca, V.; Bogdan, C. Dynamical SEIR epidemic model by Optimal Auxiliary Functions Method. Chaos Solitons Fractals 2021, 147, 110949. [Google Scholar] [CrossRef] [PubMed]
- Herisanu, N.; Marinca, B.; Marinca, V. Nonlinear vibration of double-walled carbon nanotubes subjected to mechanical impact and embedded on Winkler-Pasternak foundation. Materials 2022, 15, 8599. [Google Scholar] [CrossRef] [PubMed]
- Rand, R. Lectures Notes on Nonlinear Vibrations; Version 45; Cornell University: Ithaca, NY, USA, 2003. [Google Scholar]
- Luo, S.; Ma, H.; Li, F.; Ouakad, N.M. Dynamical analysis and chaos control of MEMS resonator by using the analog circuit. Nonl. Dyn. 2022, 108, 1–16. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Herisanu, N.; Marinca, B.; Marinca, V. Longitudinal–Transverse Vibration of a Functionally Graded Nanobeam Subjected to Mechanical Impact and Electromagnetic Actuation. Symmetry 2023, 15, 1376. https://doi.org/10.3390/sym15071376
Herisanu N, Marinca B, Marinca V. Longitudinal–Transverse Vibration of a Functionally Graded Nanobeam Subjected to Mechanical Impact and Electromagnetic Actuation. Symmetry. 2023; 15(7):1376. https://doi.org/10.3390/sym15071376
Chicago/Turabian StyleHerisanu, Nicolae, Bogdan Marinca, and Vasile Marinca. 2023. "Longitudinal–Transverse Vibration of a Functionally Graded Nanobeam Subjected to Mechanical Impact and Electromagnetic Actuation" Symmetry 15, no. 7: 1376. https://doi.org/10.3390/sym15071376
APA StyleHerisanu, N., Marinca, B., & Marinca, V. (2023). Longitudinal–Transverse Vibration of a Functionally Graded Nanobeam Subjected to Mechanical Impact and Electromagnetic Actuation. Symmetry, 15(7), 1376. https://doi.org/10.3390/sym15071376







