Strong Necessary Conditions and the Cauchy Problem
Abstract
:1. Introduction
2. A Brief Introduction to the Bogomolny Equations
3. A Presentation of the Strong Necessary Conditions Method
- making a certain part of the dual equations linearly dependent—the remaining equations are just the Bogomolny equations;
- obtaining a condition for the potential of the given field-theoretical model. The Bogomolny decomposition (the Bogomolny equations) exists only for this model, which potentially satisfies such a condition.
4. The Case of Ordinary Differential Equations
5. The Case of Partial Differential Equations
5.1. Field Equations and the Cauchy Problem Associated with Homotopy Group
5.2. Field Equations and the Cauchy Problem for the Restricted Baby Skyrme Model
6. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Adomian, G. Solving Frontiers Problems of Physics: The Decomposition Method; Springer Science+Business Media: Dordrecht, The Netherlands, 1994. [Google Scholar]
- Atmaja, A.N.; Ramadhan, H.S. Bogomol’nyi equations of classical solutions. Phys. Rev. 2014, D90, 105009. [Google Scholar] [CrossRef]
- Atmaja, A.N. A method for BPS equations of vortices. Phys. Lett. 2017, B768, 351. [Google Scholar] [CrossRef]
- Benci, V.; Fortunato, D. Variational Methods in Nonlinear Field Equations. Solitary Waves, Hylomorphic Solutions and Vortices; Springer International Publishing: Cham, Switzerland, 2014. [Google Scholar]
- Bluman, G.W.; Cheviakov, A.F.; Anco, S.C. Applications of Symmetry Methods to Partial Differential Equations; Springer Science+Business Media: Dordrecht, The Netherlands, 2010. [Google Scholar]
- Cieśliński, J. The Darboux-Bianchi-Bäcklund transformation and soliton surfaces. In Proceedings of the Nonlinearity & Geometry, Proceedings of First Non-Orthodox School; Cieśliński, J., Wójcik, D., Eds.; PWN: Warszawa, Poland, 1998; p. 81. [Google Scholar]
- Conte, R. Exact solutions of nonlinear partial differential equations by singularity analysis. In Lecture Notes in Physics, Proceedings of the Direct and Inverse Methods in Nonlinear Evolution Equations; Greco, A.M., Ed.; Springer: Berlin/Heidelberg, Germany, 2003; Volume 632, p. 1. [Google Scholar]
- Debnath, L. Nonlinear Partial Differential Equations for Scientists and Engineers; Springer Science+Business Media, Birkhäuser: Basel, Switzerland, 2012. [Google Scholar]
- Doliwa, A. Minimal surfaces, holomorphic curves and Toda systems. In Proceedings of the Nonlinearity & Geometry, Proceedings of First Non-Orthodox School; Cieśliński, J., Wójcik, D., Eds.; PWN: Warszawa, Poland, 1998; p. 227. [Google Scholar]
- Elzaki, T.M.; Biazar, J. Homotopy Perturbation Method and Elzaki Transform for Solving System of Nonlinear Partial Differential Equations. World Appl. Sci. J. 2013, 24, 944. [Google Scholar]
- Feng, Z.S. The first-integral method to study the Burgers–Korteweg–de Vries equation. J. Phys. A Math. Gen. 2002, 35, 343. [Google Scholar] [CrossRef]
- Fushchich, W.I.; Shtelen, W.M.; Serov, N. Symmetry Analysis and Exact Solutions of Equations of Nonlinear Mathematical Physics; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1993. [Google Scholar]
- Gaeta, G. Nonlinear Symmetries and Nonlinear Equations; Springer Science+Business Media: Dordrecht, The Netherlands, 1994. [Google Scholar]
- Goldstein, P. Painleve test: Standard gun of integrability hunter. In Proceedings of the Nonlinearity & Geometry, Proceedings of First Non-Orthodox School; Cieśliński, J., Wójcik, D., Eds.; PWN: Warszawa, Poland, 1998; p. 207. [Google Scholar]
- Gu, C.; Hu, H.; Zhou, Z. Darboux Transformations in Integrable Systems. Theory and Their Applications to Geometry; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Kudryashov, N.A. Analytic Theory of Nonlinear Differential Equations; Moscow-Izhevsk, Institute of Computer Studies: Izhevsk, Russia, 2004. (In Russian) [Google Scholar]
- Magri, F.; Falqui, G.; Pedroni, M. The method of Poisson pairs in the theory of nonlinear PDEs. In Lecture Notes in Physics, Proceedings of the Direct and Inverse Methods in Nonlinear Evolution Equations; Greco, A.M., Ed.; Springer: Berlin/Heidelberg, Germany, 2003; Volume 632, p. 85. [Google Scholar]
- Meleshko, S.V. Methods for Constructing Exact Solutions of Partial Differential Equations; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Musette, M. Nonlinear superposition formulae of integrable partial differential equations by the singular manifold method. In Lecture Notes in Physics, Proceedings of the Direct and Inverse Methods in Nonlinear Evolution Equations; Greco, A.M., Ed.; Springer: Berlin/Heidelberg, Germany, 2003; Volume 632, p. 137. [Google Scholar]
- Krasil’shchik, I. Symmetries and recursion operators for soliton equations. In Proceedings of the Nonlinearity & Geometry, Proceedings of First Non-Orthodox School; Cieśliński, J., Wójcik, D., Eds.; PWN: Warszawa, Poland, 1998; p. 141. [Google Scholar]
- Nieszporski, M.; Sym, A. Weingarten congruences and non-auto-Backlund transformations for hyperbolic surfaces. In Proceedings of the Nonlinearity & Geometry, Proceedings of First Non-Orthodox School; Cieśliński, J., Wójcik, D., Eds.; PWN: Warszawa, Poland, 1998; p. 37. [Google Scholar]
- Olver, P.J. Applications of Lie Groups to Differential Equations; Springer: Berlin/Heidelberg, Germany, 1986. [Google Scholar]
- Polyanin, A.D.; Zaitsev, V.F. Handbook of Nonlinear Partial Differential Equations; CRC Press Taylor & Francis Group: Boca Raton, FL, USA, 2012. [Google Scholar]
- Rajchel, K.; Szczȩsny, J. New method to solve certain differential equations. Ann. Univ. Paedagog. Crac. Stud. Math. 2016, XV, 107. [Google Scholar] [CrossRef]
- Rubina, L.I.; Ul’yanov, O.N. On one approach to solving nonhomogeneous partial differential equations. Vestn. Udmurtsk. Univ. Mat. Mekh. Komp. Nauki 2017, 27, 355. [Google Scholar] [CrossRef]
- Satsuma, J. Hirota bilinear method for nonlinear partial differential equations. In Lecture Notes in Physics, Proceedings of the Direct and Inverse Methods in Nonlinear Evolution Equations; Greco, A.M., Ed.; Springer: Berlin/Heidelberg, Germany, 2003; Volume 632, p. 171. [Google Scholar]
- Stȩpień, T. Some decomposition method for analytic solving of certain nonlinear partial differential equations in physics with applications. J. Comp. Appl. Math. 2010, 233, 1607. [Google Scholar] [CrossRef]
- Stȩpień, T. Some Exact Soluions for ABC equations and Martínez Alonso Shabat Equations. J. Geom. Symm. Phys. 2023, 66, 47. [Google Scholar] [CrossRef]
- Vitanov, N.K.; Dimitrova, Z.I.; Vitanov, K.N. Modified method of simplest equation for obtaining exact analytical solutions of nonlinear partial differential equations: Further development of the methodology with applications. Appl. Math. Comp. 2015, 269, 363–378. [Google Scholar] [CrossRef]
- Wietecha, T.; Sokalski, K. Plus-minus algorithm—A method for derivation of the Bäcklund transformations. J. Symb. Comp. 2009, 44, 1511. [Google Scholar] [CrossRef]
- Winternitz, P. Lie groups, singularities and solutions of nonlinear partial differential equations. In Lecture Notes in Physics, Proceedings of the Direct and Inverse Methods in Nonlinear Evolution Equations; Greco, A.M., Ed.; Springer: Berlin/Heidelberg, Germany, 2003; Volume 632, p. 223. [Google Scholar]
- Zakharov, V.E.; Shabat, A.B. A scheme for integrating the nonlinear equations of mathematical physics by the method of the inverse scattering problem I. Funct. Anal. Appl. 1977, 11, 226. [Google Scholar]
- Zhang, Z.Y.; Zhong, J.; Dou, S.S.; Liu, J.; Peng, D.; Gao, T. First integral method and exact solutions to nonlinear partial differential equations arising in mathematical physics. Rom. Rep. Phys. 2013, 65, 1155. [Google Scholar]
- Fedorchuk, V.M.; Fedorchuk, V.I. Reduction of the (1+ 3)-Dimensional Inhomogeneous Monge–Ampère Equation to First-Order Partial Differential Equations. Ukr. Math. J. 2022, 74, 472. [Google Scholar] [CrossRef]
- Adam, C.; Santamaria, F. The first-order Euler-Lagrange equations and some of their uses. J. High Energ. Phys. 2016, 2016, 047. [Google Scholar] [CrossRef]
- Sokalski, K. Instantons in Anisotropic Ferromagnets. Acta Phys. Pol. 1979, A56, 571. [Google Scholar]
- Sokalski, K. Dynamical stability of instantons. Phys. Lett. 1981, A81, 102. [Google Scholar] [CrossRef]
- Sokalski, K. Instantons in Three-Dimensional Heisenberg Ferromagnets. Acta Phys. Pol. 1984, A65, 457. [Google Scholar]
- Jochym, P.T.; Sokalski, K. Variational approach to the Bogomolny separation. J. Phys. A Math. Gen. 1993, 26, 3837. [Google Scholar] [CrossRef]
- Sokalski, K.; Stȩpień, T.; Sokalska, D. The existence of Bogomolny decomposition by means of strong necessary conditions. J. Phys. 2002, A35, 6157. [Google Scholar] [CrossRef]
- Sokalski, K.; Wietecha, T.; Lisowski, Z. Variational Approach to the Bäcklund Transformations. Acta Phys. Pol. 2001, B32, 17. [Google Scholar]
- Sokalski, K.; Wietecha, T.; Lisowski, Z. A Concept of Strong Necsseary Conditions in Nonlinear Field Theory. Acta Phys. Pol. 2001, B32, 2771. [Google Scholar]
- Sokalski, K.; Wietecha, T.; Lisowski, Z. Unified Variational Approach to the Bäcklund Transformations and the Bogomolny Decomposition. Int. J. Theor. Phys. Group Theory Nonlinear Opt. NOVA 2002, 9, 331. [Google Scholar]
- Sokalski, K.; Wietecha, T.; Sokalska, D. Existence of dual equations by means of strong necessary conditions-Analysis of integrability of partial differential nonlinear equations. J. Nonlinear Math. Phys. 2005, 12, 31. [Google Scholar] [CrossRef]
- Stȩpień, T. Bogomolny Decomposition in the Context of the Concept of Strong Necessary Conditions. Ph.D. Dissertation, Jagiellonian University, Kraków, Poland, Marian Smoluchowski Institute of Physics, Department of Mathematics, Physics and Astronomy, Cracow, Poland, 2003. [Google Scholar]
- Stȩpień, T.; Sokalska, D.; Sokalski, K. The Bogomolny decomposition for systems of two generalized nonlinear partial differential equations of the second order. J. Nonlinear Math. Phys. 2009, 16, 25. [Google Scholar] [CrossRef]
- Stȩpień, T. On Bogomolny Decompositions for the Baby Skyrme Models. In Proceedings of the Geometric Methods in Physics, XXXI Workshop; Białowieża, Poland, 24–30 June 2012, Kielanowski, P., Ali, S.T., Odesskii, A., Odzijewicz, A., Schlichenmaier, M., Voronov, T., Eds.; Trends in Mathematics; Birkhäuser, Springer: Basel, Switzerland, 2013; pp. 229–237. [Google Scholar]
- Stȩpień, T. The Existence of Bogomolny Decompositions for Gauged O(3) Nonlinear “Sigma” Model and for Gauged Baby Skyrme Models. Acta Phys. Pol. B 2015, 46, 999. [Google Scholar] [CrossRef]
- Stȩpień, T. Bogomolny equation for the BPS Skyrme model from strong necessary conditions. J. Phys. A 2016, 49, 175202. [Google Scholar] [CrossRef]
- Stȩpień, T. Bogomolny equations in certain generalized baby BPS Skyrme models. J. Phys. A 2018, 51, 015208. [Google Scholar] [CrossRef]
- Stȩpień, T. Bogomolny equations for the BPS Skyrme models with impurity. J. High Energ. Phys. 2020, 2020, 140. [Google Scholar] [CrossRef]
- Stȩpień, T.; Pomorski, K. Bogomolny approach in description of superconducting structures. arXiv 2023, arXiv:2308.09172. [Google Scholar]
- Szafirski, B.; Institute of Mathematics, Jagiellonian University, Kraków, Poland. Personal Communication, 2001.
- Stȩpień, T. Strong necessary conditions and Cauchy problem. arXiv 2019, arXiv:1912.02609v2. [Google Scholar]
- Bogomolny, E.B. The stability of classical solutions. Sov. J. Nucl. Phys. 1976, 24, 449. [Google Scholar]
- Belavin, A.A.; Polyakov, A.M.; Schwartz, A.S.; Tyupkin, Y.S. Pseudoparticle solutions of the Yang-Mills equations. Phys. Lett. 1975, B59, 85. [Google Scholar] [CrossRef]
- Hosoya, A. On Vanishing of Energy-Momentum Tensor for a Class of Instanton-Like Solutions. Prog. Theor. Phys. 1978, 59, 1781. [Google Scholar] [CrossRef]
- Białynicki-Birula, I. On the stability of solitons. In Lecture Notes in Physics, Proceedings of the Nonlinear Problems in Theoretical Physcis, Jaca, Spain, June 1978; Rañada, A.F., Ed.; Birkhäuser, Springer: Basel, Switzerland, 1979; Volume 98, p. 15. [Google Scholar]
- Meissner, K.A. Classical Field Theory; PWN: Warszawa, Poland, 2013. (In Polish) [Google Scholar]
- Belavin, A.A.; Polyakov, A.M. Metastable states of two dimensional isotropic ferromagnets. JETP Lett. 1975, 22, 245. [Google Scholar]
- Balakrishnan, R.; Bishop, A.R.; Dandoloff, R. Geometric phase in the classical continuous antiferromagnetic Heisenberg spin chain. Phys. Rev. Lett. 1990, 64, 2107. [Google Scholar] [CrossRef]
- Balakrishnan, R.; Dandoloff, R.; Saxena, A. Exact hopfion vortices in a 3D Heisenberg ferromagnet. Phys. Lett. A 2023, 480, 128975. [Google Scholar] [CrossRef]
- Felsager, B. Geometry, Particles and Fields; Springer Science+Business Media: New York, NY, USA, 1998. [Google Scholar]
- Morandi, G. The Role of Topology in Classical and Quantum Physics; Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 1992. [Google Scholar]
- Arodź, H.; Hadasz, L. Lectures on Classical and Quantum Theory of Fields; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Bogoliubov, N.N.; Shirkov, D.V. Introduction to the Theory of Quantized Fields. In Nikolai Nikolaevich Bogoliubov. Collection of Scientific Works; Sukhanov, A.D., Ed.; Nauka: Moscow, Russia, 2008; Volume 10. (In Russian) [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Mechanics; PWN: Warszawa, Poland, 2012. (In Polish) [Google Scholar]
- Gignoux, C.; Silvestre-Brac, B. Solved Problems in Lagrangian and Hamiltonian Mechanics; Springer Science+Business Media B.V.: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Knudsen, J.M.; Hjorth, P.G. Elements of Newtonian Mechanics. Including Nonlinear Dynamics; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Cieśliński, J.L. On the exact discretization of the classical harmonic oscillator equation. J. Diff. Equ. Appl. 2013, 17, 1673. [Google Scholar] [CrossRef]
- Saxena, A.; Kevrekidis, P.; Cuevas-Maraver, J. Nonlinearity and Topology. In Emerging Frontiers in Nonlinear Science; Kevrekidis, P., Cuevas-Maraver, J., Saxena, A., Luo, A.C.J., Eds.; Nonlinear Systems and Complexity Series; Springer Nature Switzerland AG: Cham, Switzerland, 2020; Chapter 2, Volume 32, pp. 25–53. [Google Scholar]
- Skyrme, T.H.R. A non-linear field theory. Proc. R. Soc. Lond. 1961, A260, 127–138. [Google Scholar]
- Skyrme, T.H.R. A unified field theory of mesons and baryons. Nucl. Phys. 1962, 31, 556. [Google Scholar] [CrossRef]
- Makhankov, V.G.; Rybakov, Y.P.; Sanyuk, V.I. The Skyrme Model: Fundamentals, Methods, Applications; Springer: Berlin/Heidelberg, Germany, 1993. [Google Scholar]
- Sondhi, S.L.; Karlhede, A.; Kivelson, S.A.; Rezayi, E.H. Skyrmions and the crossover from the integer to fractional quantum Hall effect at small Zeeman energies. Phys. Rev. B 1993, 47, 16419. [Google Scholar] [CrossRef]
- Schwindt, O.; Weidig, T. Towards a phase diagram of the 2D Skyrme model. Europhys. Lett. 2001, 55, 633. [Google Scholar] [CrossRef]
- Weidig, T. The baby Skyrme models and their multi-skyrmions. Nonlinearity 1999, 12, 1489. [Google Scholar] [CrossRef]
- Hu, H.; Hu, K. Existence of Solutions for a Baby-Skyrme Model. J. Appl. Math. 2014, 2014, 1. [Google Scholar] [CrossRef]
- Adam, C.; Romańczukiewicz, T.; Sanchez-Guillen, J.; Wereszczyński, A. Investigation of restricted baby Skyrme models. Phys. Rev. 2010, D81, 085007. [Google Scholar] [CrossRef]
- Speight, J.M. Compactons and semi-compactons in the extreme baby Skyrme model. J. Phys. A 2010, 43, 405201. [Google Scholar] [CrossRef]
- Sanyal, A.K. Degenerate Hamiltonian operator in higher-order canonical gravity—The problem and a remedy. Ann. Phys. 2019, 411, 167971. [Google Scholar] [CrossRef]
- Del Castillo, G.F.T.; Mirón, C.A.; Rojas, R.I.B. Variational symmetries of Lagrangians. Riv. Mexic. Fis. E 2013, 59, 140. [Google Scholar]
- Arodź, H. Lectures on Field Theory. Delivered at Institute of Physics, Jagiellonian University 1997/1998. Unpublished.
- Kiselev, V.V.; Batalov, S.V. Nonlinear interference of solitons and waves in the magnetic domain structure. Theor. Math. Phys. 2023, 214, 369. [Google Scholar] [CrossRef]
- Dimopoulos, S.; Eguchi, T. Virial theorems on instantons. Phys. Lett. 1977, 66B, 480. [Google Scholar] [CrossRef]
- De Vega, H.J.; Schaposnik, F.A. Classical vortex solution of the Abelian Higgs model. Phys. Rev. D 1976, 14, 1100. [Google Scholar] [CrossRef]
- Belavin, A.A.; Burlankov, D.E. The renormalisable theory of gravitation and the einstein equations. Phys. Lett. 1976, 58A, 7. [Google Scholar] [CrossRef]
- Faddeev, L.D.; Jackiw, R. Hamiltonian reduction of unconstrained and constrained systems. Phys. Rev. Lett. 1988, 60, 1692. [Google Scholar] [CrossRef]
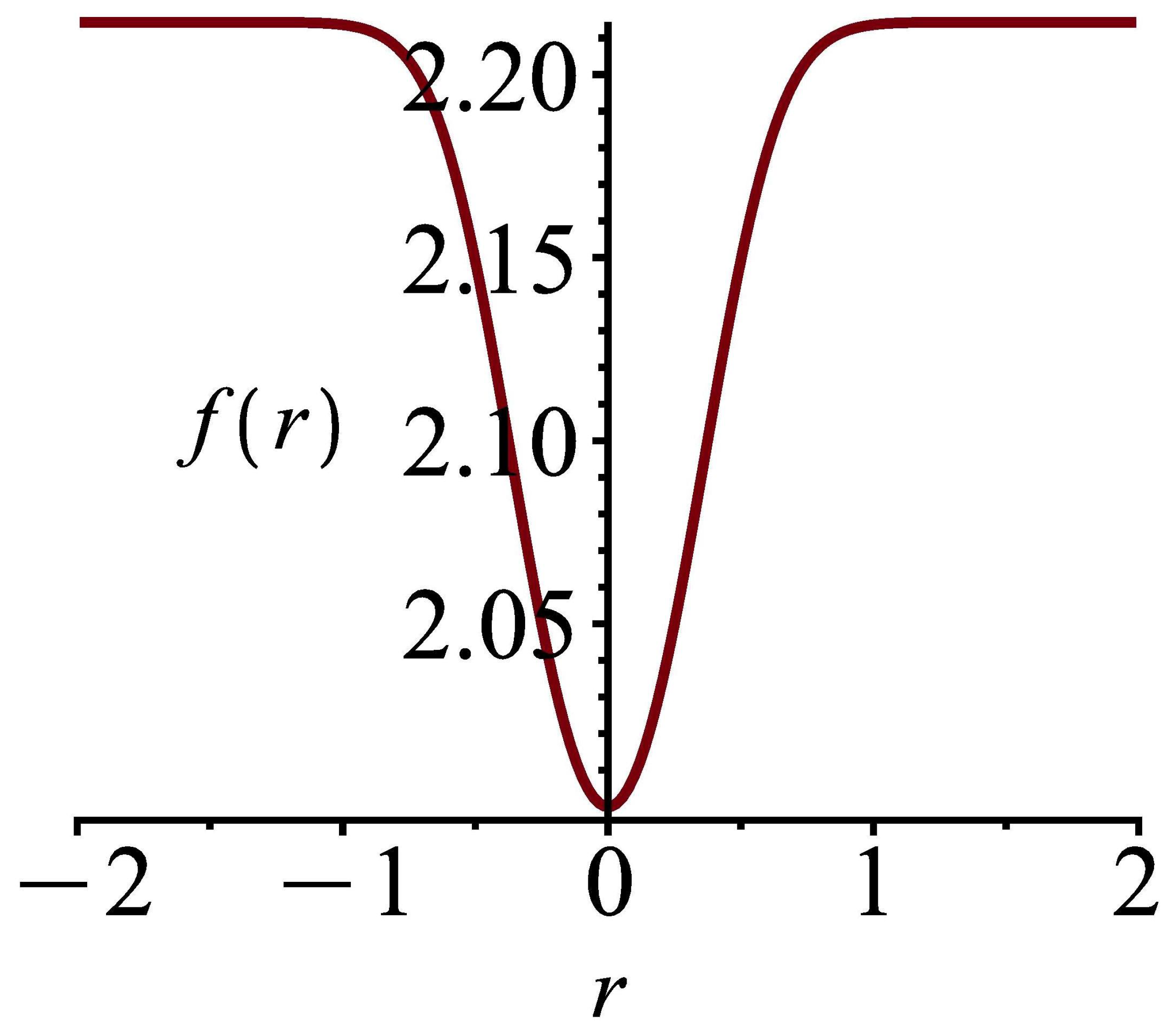
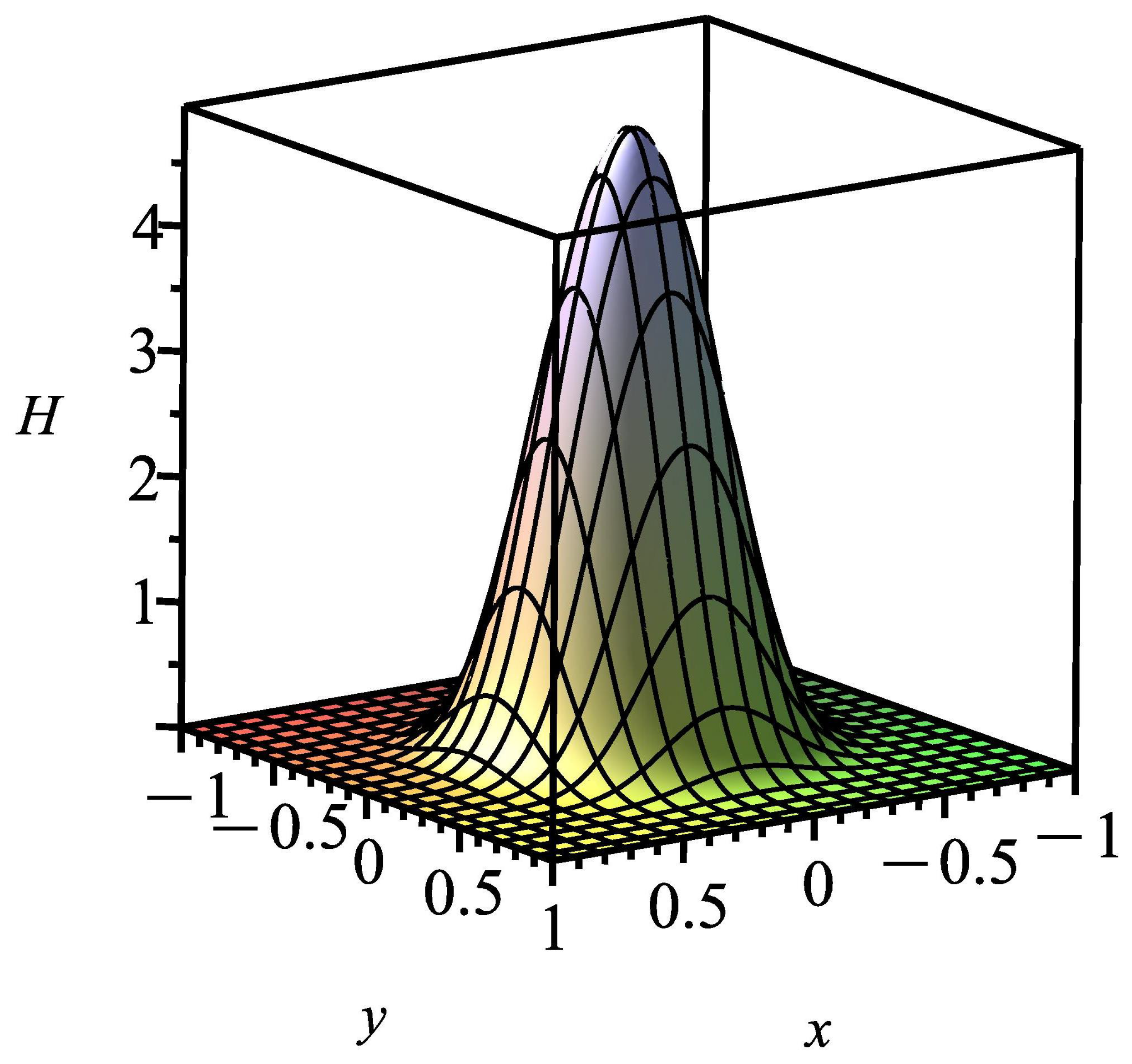
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stȩpień, Ł.T. Strong Necessary Conditions and the Cauchy Problem. Symmetry 2023, 15, 1622. https://doi.org/10.3390/sym15091622
Stȩpień ŁT. Strong Necessary Conditions and the Cauchy Problem. Symmetry. 2023; 15(9):1622. https://doi.org/10.3390/sym15091622
Chicago/Turabian StyleStȩpień, Łukasz T. 2023. "Strong Necessary Conditions and the Cauchy Problem" Symmetry 15, no. 9: 1622. https://doi.org/10.3390/sym15091622
APA StyleStȩpień, Ł. T. (2023). Strong Necessary Conditions and the Cauchy Problem. Symmetry, 15(9), 1622. https://doi.org/10.3390/sym15091622






