Constraints on Phase Transitions in Neutron Star Matter
Abstract
:1. Introduction
2. Equation of State of Neutron Star Matter
2.1. Observational Constraints
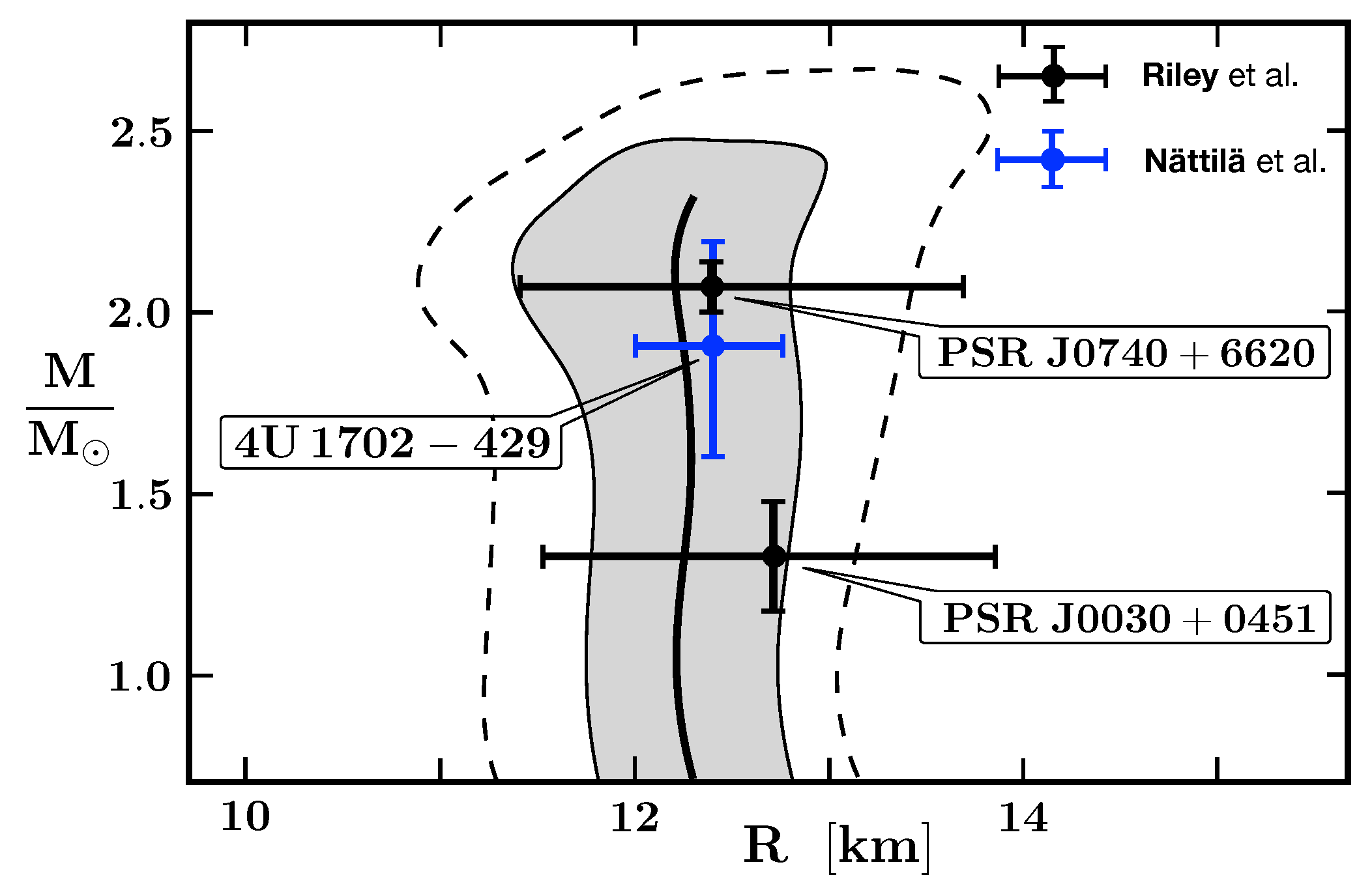
2.1.1. Neutron Star Masses and Radii
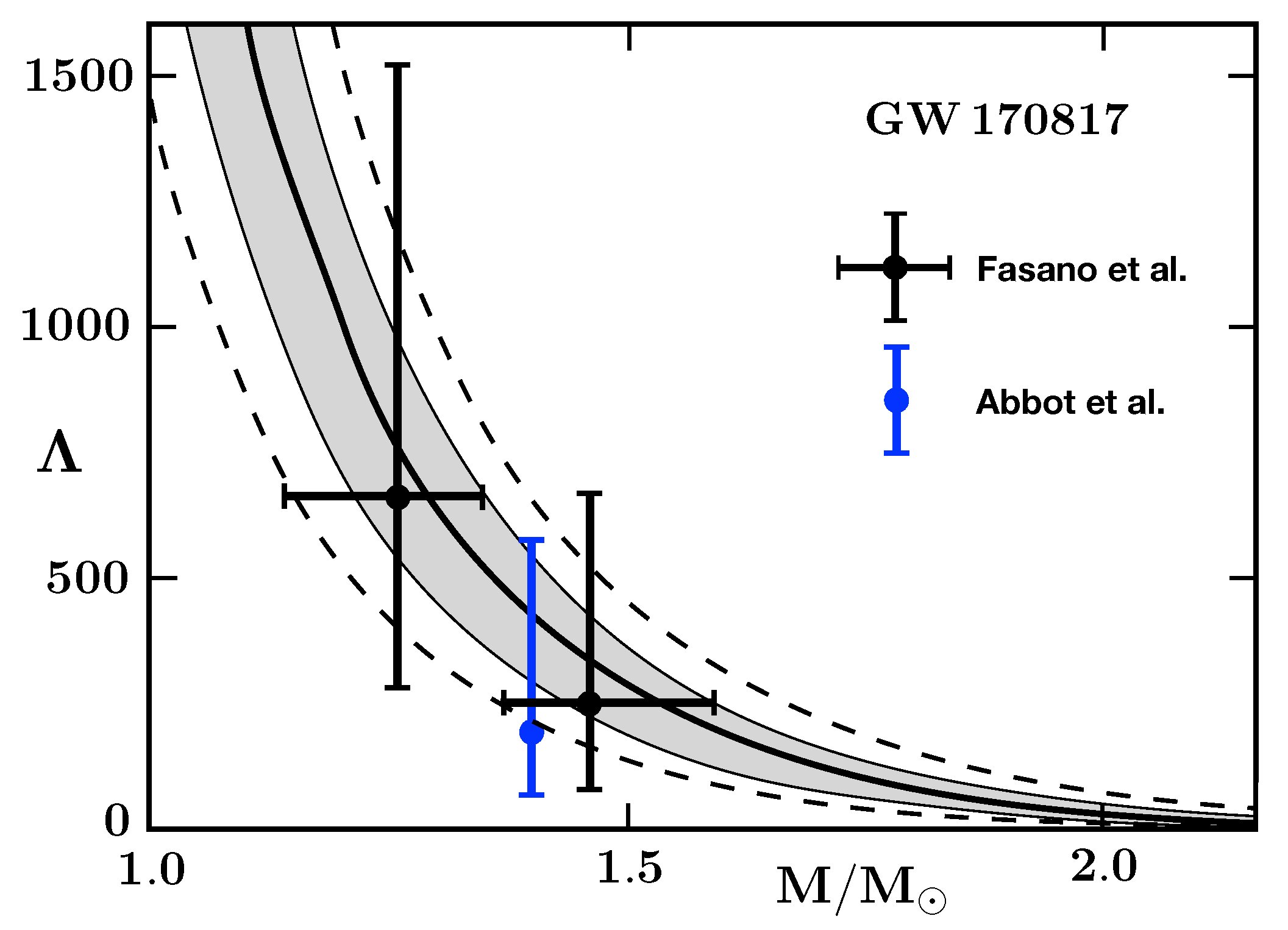
2.1.2. Binary Neutron Star Mergers and Tidal Deformabilities
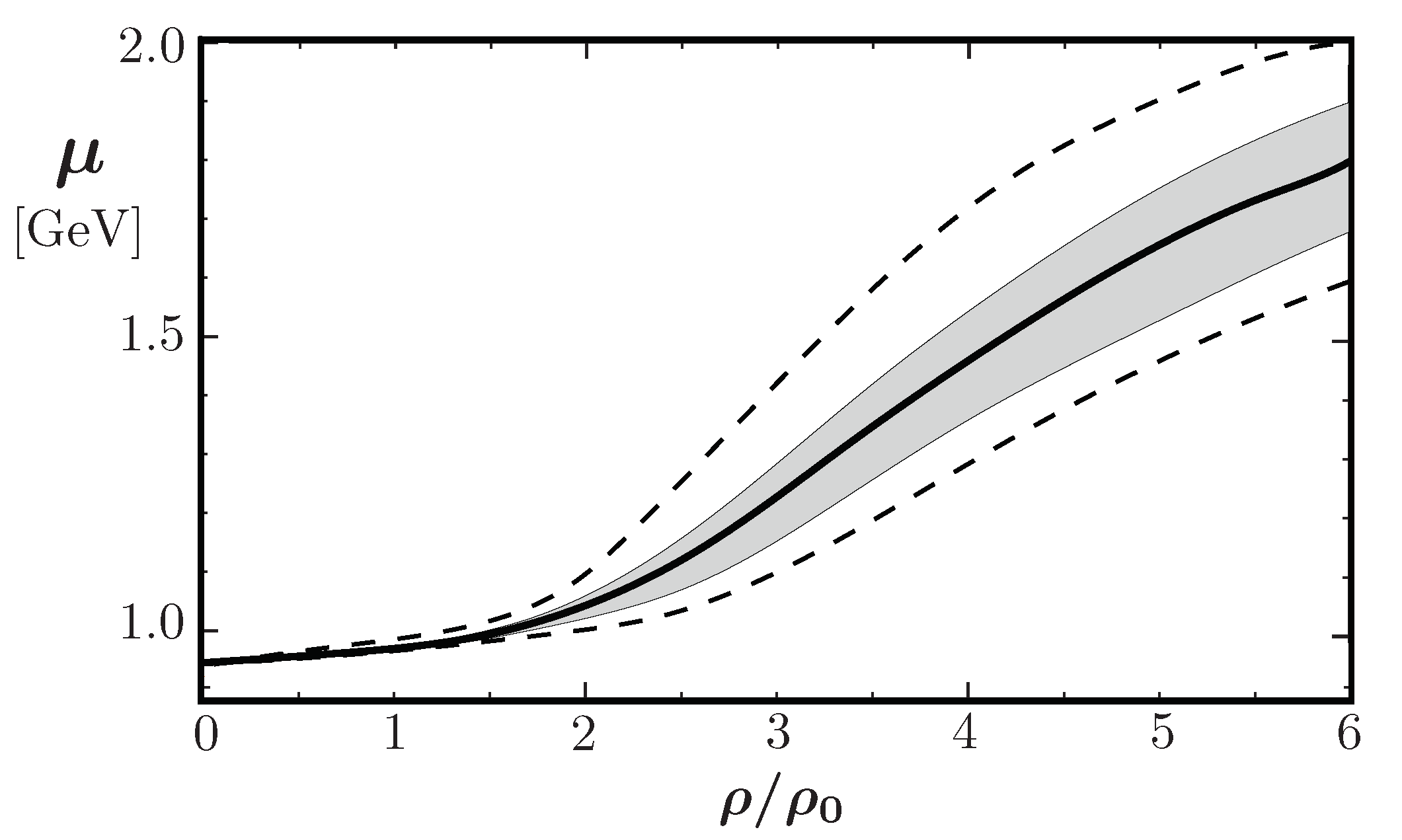
2.2. Inference of Sound Velocity and the EoS in Neutron Stars
2.3. Selected Neutron Star Properties
3. Constraints on Phase Transitions in Neutron Stars
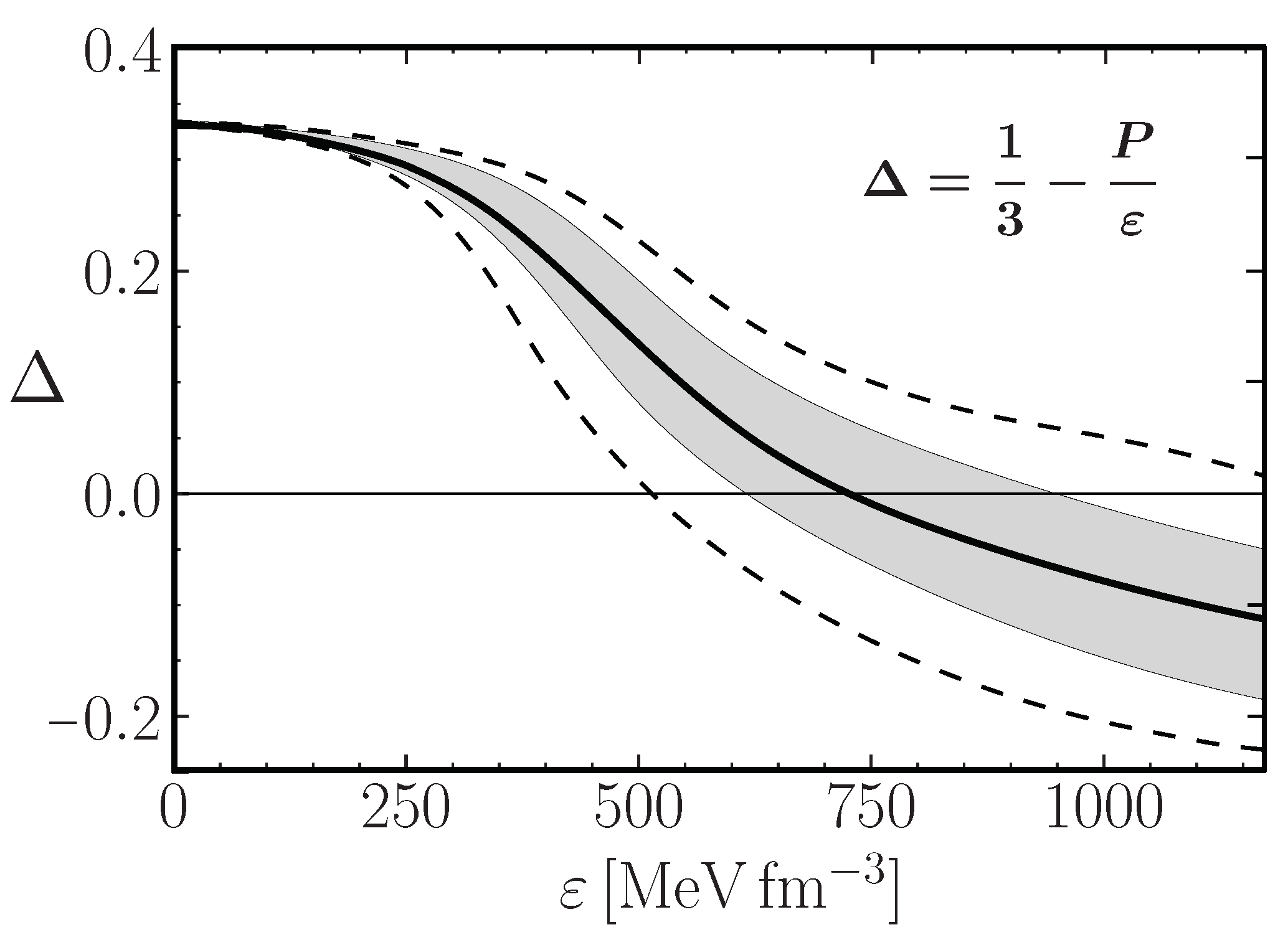
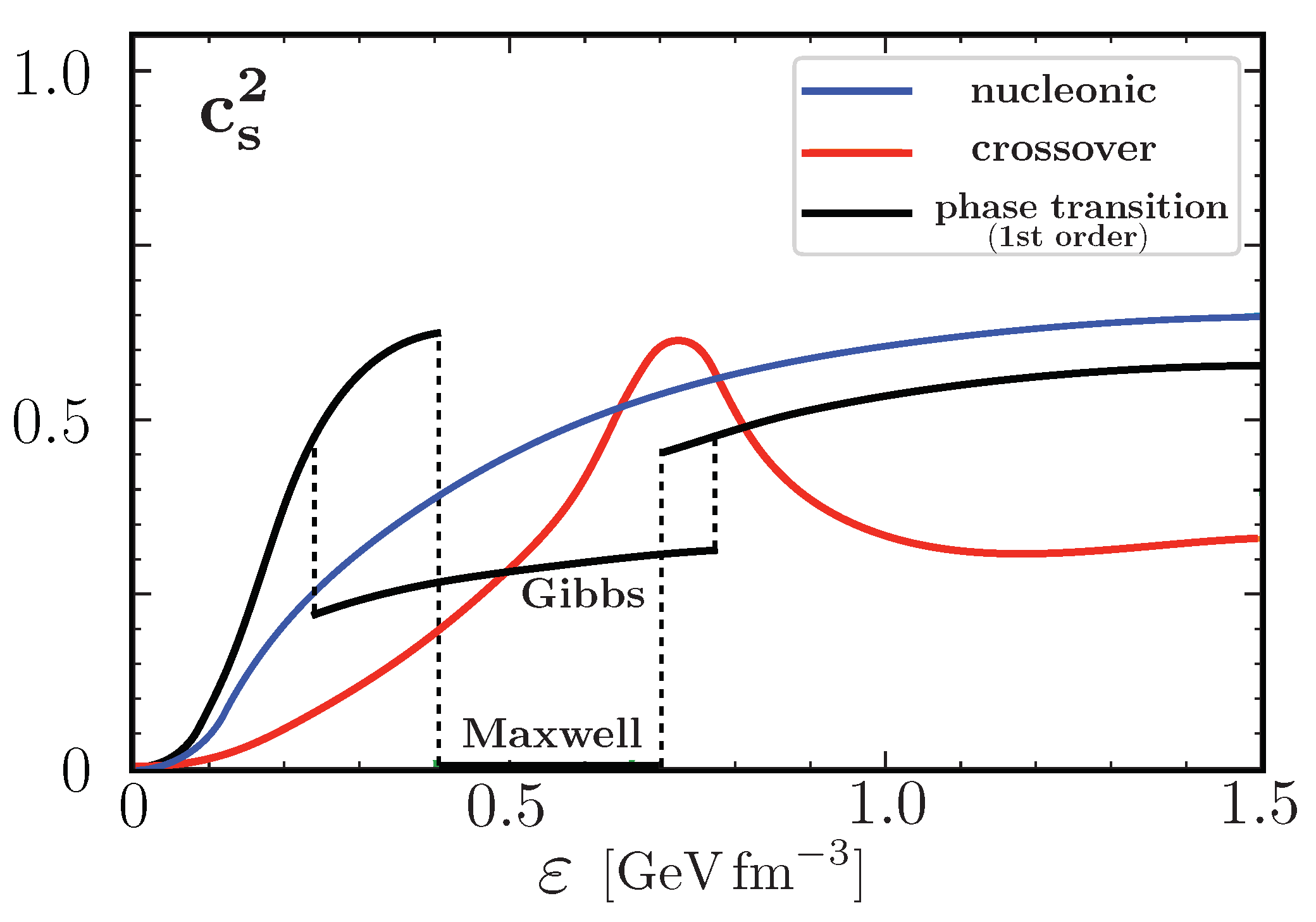
3.1. Evidence against a Very Low Squared Sound Speed in Neutron Stars
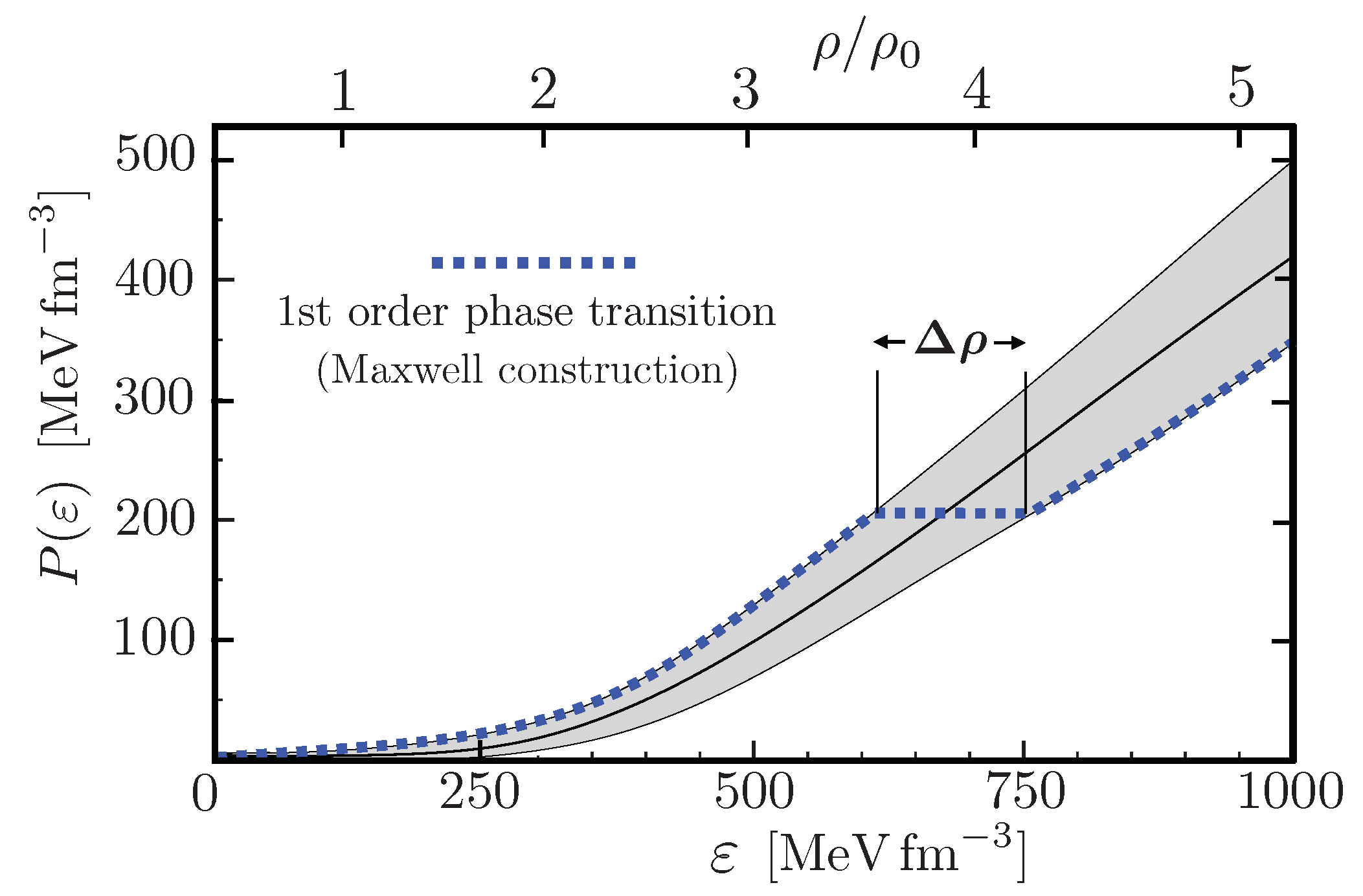
3.2. Evidence against a Strong First-Order Phase Transition in the Cores of Neutron Stars
3.3. Intermediate Summary
4. Phenomenology and Models
4.1. Reminder of Low-Energy Nucleon Structure and a Two-Scale Scenario
4.2. Quark–Hadron Continuity and Crossover
4.3. Restoration of Chiral Symmetry in Dense Matter: From First-Order Phase Transition to Crossover
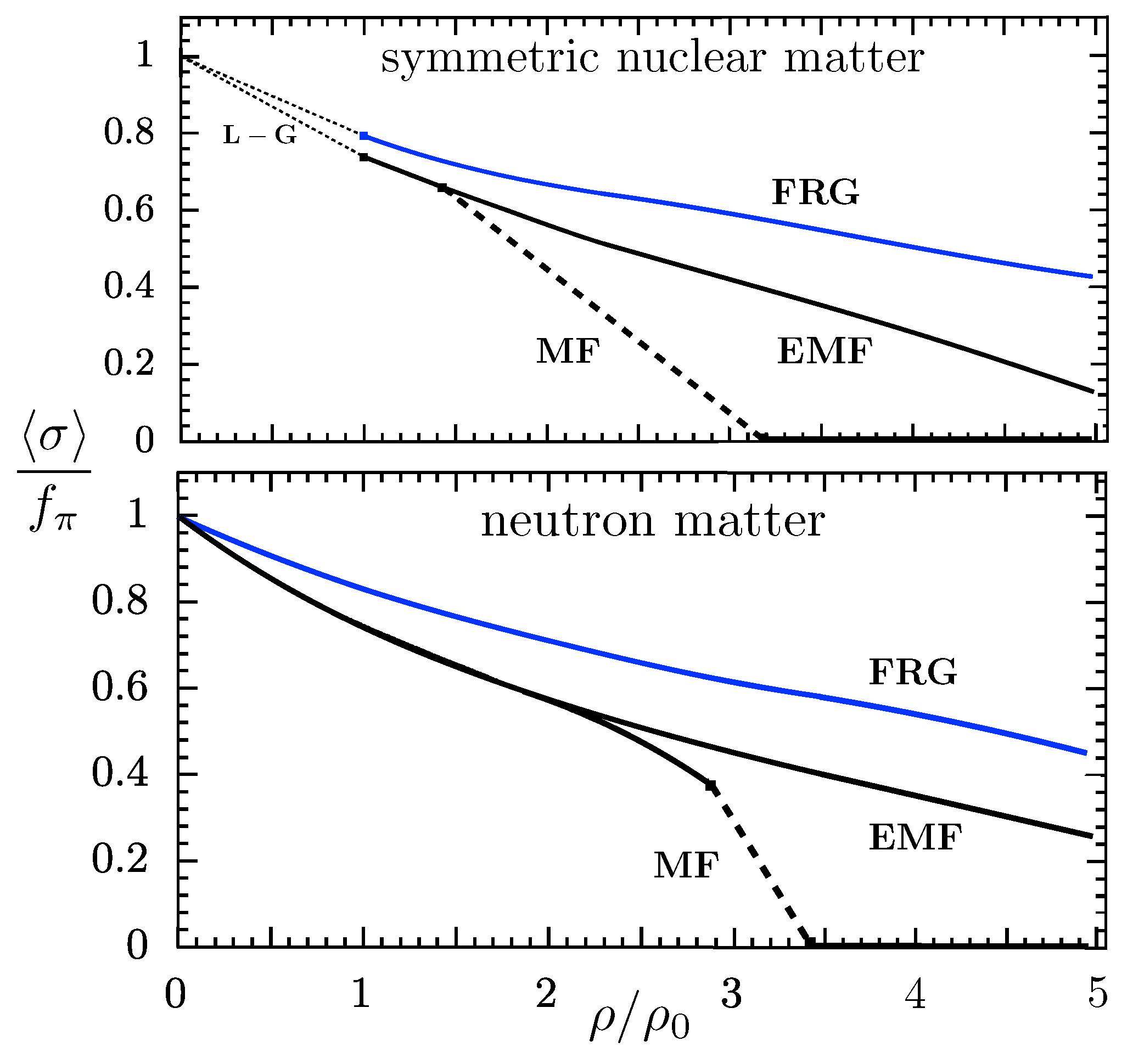
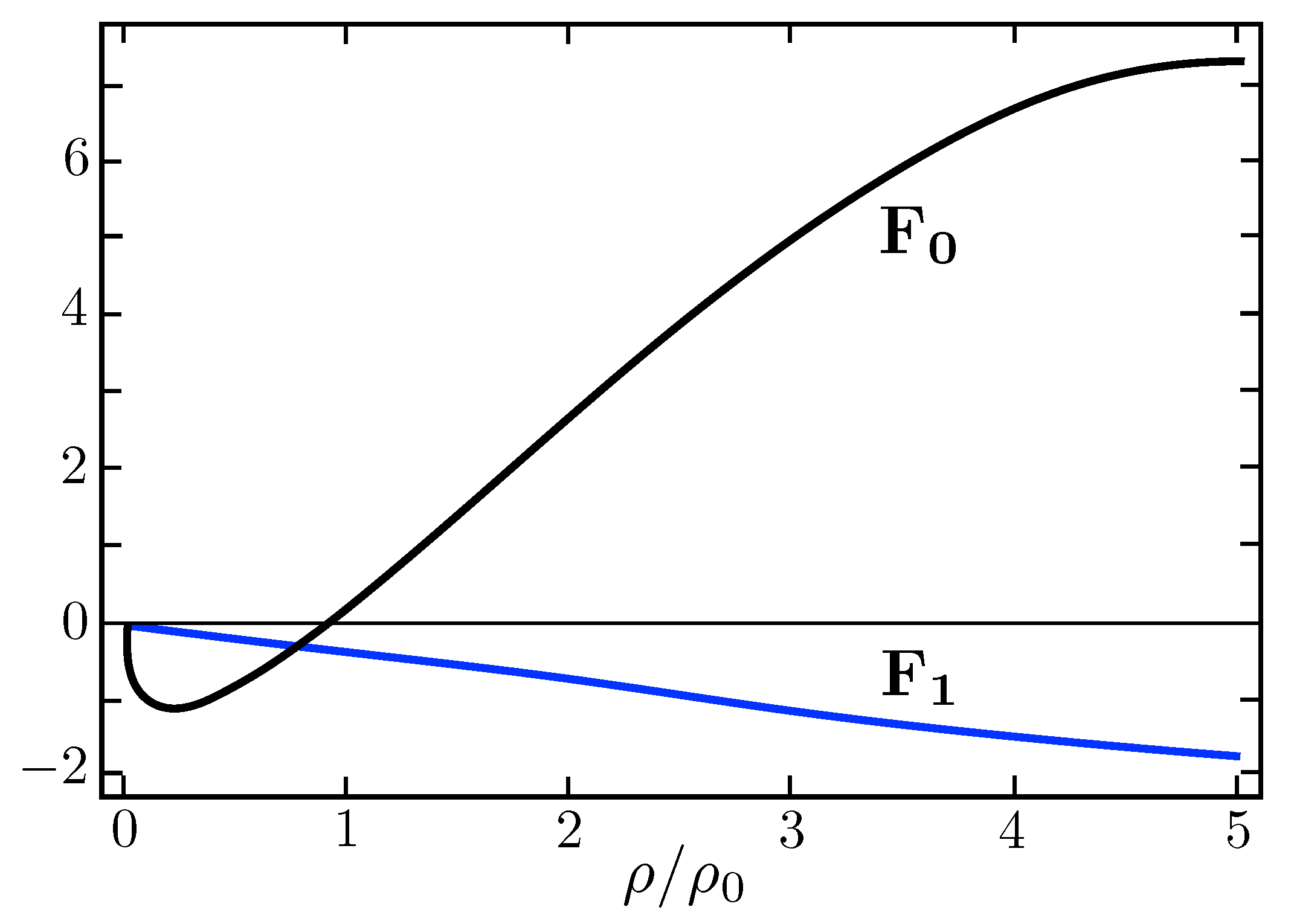
4.4. Dense Baryonic Matter: A Fermi Liquid Picture
5. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lovato, A.; Dore, T.; Pisarski, R.D.; Schenke, B.; Chatziioannou, K.; Read, J.S.; Landry, P.; Danielewicz, P.; Lee, D.; Pratt, S.; et al. Long range plan: Dense matter theory for heavy-ion collisions and neutron stars. arXiv 2022, arXiv:2211.02224. [Google Scholar]
- Fukushima, K.; Hatsuda, T. The phase diagram of dense QCD. Rep. Prog. Phys. 2011, 74, 014001. [Google Scholar] [CrossRef]
- Aarts, G.; Aichelin, J.; Allton, C.; Athenodorou, A.; Bachtis, D.; Bonanno, C.; Brambilla, N.; Bratkovskaya, E.; Bruno, M.; Caselle, M.; et al. Phase transitions in particle physics—Results and perspectives from lattice quantum chromo-dynamics. Prog. Part. Nucl. Phys. 2023, 133, 104070. [Google Scholar]
- Demorest, P.B.; Pennucci, T.; Ransome, S.M.; Roberts, M.S.E. A two-solar mass neutron star measured using Shapiro delay. Nature 2010, 467, 1081–1083. [Google Scholar] [CrossRef]
- Antoniadis, J.; Freire, P.C.C.; Wex, N.; Tauris, T.M.; Lynch, R.S.; van Kerkwijk, M.H.; Kramer, M.; Bassa, C.; Dhillon, V.S.; Driebe, T.; et al. A massive pulsar in a compact relativistic binary. Science 2013, 340, 448–502. [Google Scholar] [CrossRef]
- Fonseca, E.; Pennucci, T.; Ellis, J.A.; Stairs, I.H.; Nice, D.J.; Ransom, S.M.; Demorest, P.B.; Arzoumanian, Z.; Crowther, K.; Dolch, T.; et al. The NANOGrav nine-year data set: Mass and geometric measurements of binary millisecond pulsars. Astrophys. J. 2016, 832, 167–189. [Google Scholar]
- Arzoumanian, Z.; Brazier, A.; Burke-Spolaor, S.; Chamberlin, S.; Chatterjee, S.; Christy, B.; Cordes, J.M.; Cornish, N.J.; Crawford, F.; Cromartie, H.T.; et al. The NANOGrav 11-year data set: High-precision timing of 45 millisecond pulsars. Astrophys. J. Suppl. 2018, 235, 37–78. [Google Scholar] [CrossRef]
- Cromartie, H.T.; Fonseca, E.; Ransom, S.M.; Demorest, P.B.; Arzoumanian, Z.; Blumer, P.R.; Brook, P.R.; DeCesar, M.E.; Dolch, T.; Ellis, J.A.; et al. Relativsitic Shapiro delay measurements of an extremely massive millisecond pulsar. Nat. Astron. 2020, 4, 72–76. [Google Scholar] [CrossRef]
- Fonseca, E.; Cromartie, H.T.; Pennucci, T.T.; Ray, P.S.; Kirichenko, A.Y.; Ransom, S.M.; Demorest, P.B.; Stairs, I.H.; Arzoumanian, Z.; Guillemot, L.; et al. Refined mass and geometric measurements of the high-mass PSR J0740+6620. Astrophys. J. Lett. 2021, 915, L12. [Google Scholar] [CrossRef]
- Riley, T.E.; Watts, A.L.; Bogdanov, S.; Ray, P.S.; Ludlam, R.M.; Guillot, S.; Arzoumanian, Z.; Baker, C.L.; Bilous, A.V.; Chakrabarty, D.; et al. A NICER view of PSR J0030+0451: Millisecond pulsar parameter estimation. Astrophys. J. Lett. 2019, 887, L21. [Google Scholar]
- Miller, M.C.; Lamb, F.K.; Dittmann, A.J.; Bogdanov, S.; Arzumanian, Z.; Gendreau, K.C.; Guillot, S.; Harding, A.K.; Ho, W.C.G.; Lattimer, J.M.; et al. PSR J0030+0451 mass and radius from NICER data and implications for the properties of neutron stars. Astrophys. J. Lett. 2019, 887, L24. [Google Scholar] [CrossRef]
- Riley, T.E.; Watts, A.L.; Ray, P.S.; Bogdanov, S.; Guillot, S.; Morsink, S.M.; Bilous, A.V.; Arzoumanian, Z.; Choudury, D.; Daneva, J.S.; et al. A NICER view of the massive pulsar PSR J0740+6620 informed by radio timing and XMM-Newton spectroscopy. Astrophys. J. Lett. 2021, 918, L27. [Google Scholar]
- Miller, M.C.; Lamb, F.K.; Dittmann, A.J.; Bogdanov, S.; Arzumanian, Z.; Gendreau, K.C.; Guillot, S.; Ho, W.C.G.; Lattimer, J.M.; Loewenstein, M.; et al. The radius of PSR J0740+6620 from NICER and XMM-Newton data. Astrophys. J. Lett. 2021, 918, L28. [Google Scholar] [CrossRef]
- Salmi, T.; Vinciguerra, S.; Choudury, D.; Riley, T.E.; Watts, A.L.; Remillard, R.A.; Ray, P.S.; Bogdanov, S.; Guillot, S.; Arzumanian, Z.; et al. The radius of PSR J0740+6620 from NICER with NICER background estimates. Astrophys. J. 2022, 941, 150–173. [Google Scholar]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. (LIGO Scientific Collaboration and Virgo Collaboration). Properties of the binary neutron star merger GW170817. Phys. Rev. 2019, X9, 011001. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. (LIGO Scientific Collaboration and Virgo Collaboration). GW170817: Measurement of neutron star radii and equation of state. Phys. Rev. Lett. 2018, 121, 161101. [Google Scholar] [CrossRef] [PubMed]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; et al. (LIGO Scientific Collaboration and Virgo Collaboration). GW190425: Observation of a compact binary coalescence with total mass ∼ 3.4 M⊙. Astrophys. J. Lett. 2020, 892, L3. [Google Scholar] [CrossRef]
- Annala, E.; Gorda, T.; Kurkela, A.; Nättilä, J.; Vuorinen, A. Evidence for quark-matter cores in massive neutron stars. Nature Phys. 2020, 16, 907. [Google Scholar] [CrossRef]
- Raaijmakers, G.; Greif, S.K.; Hebeler, K.; Hinderer, T.; Nissanke, S.; Schwenk, A.; Riley, T.E.; Watts, A.; Lattimer, J.M.; Ho, W.C.G. Constraints on the dense matter equation of state and neutron star properties from NICER’s mass-radius estimate of PSR J0740+6620 and multimessenger observations. Astrophys. J. Lett. 2021, 918, L29. [Google Scholar]
- Pang, P.T.H.; Tews, I.; Coughlin, M.W.; Bulla, M.; Van Den Broeck, C.; Dietrich, T. Nuclear physics multimessenger constraints on the neutron star equation of state: Adding NICER’s PSR J0740+6620 measurement. Astrophys. J. 2021, 922, 14. [Google Scholar] [CrossRef]
- Legred, I.; Chatziiannou, K.; Essick, R.; Han, S.; Landry, P. Impact of the PSR J0740+6620 radius constraint on the properties of high-density matter. Phys. Rev. 2021, D104, 063003. [Google Scholar] [CrossRef]
- Biswas, B.; Datta, S. Constraining neutron star properties with a new equation of state insensitive approach. Phys. Rev. 2022, D106, 043012. [Google Scholar] [CrossRef]
- Ecker, C.; Rezzolla, L. A general, scale-independent description of the sound speed in neutron stars. Astrophys. J. Lett. 2022, 939, L35. [Google Scholar] [CrossRef]
- Altiparmak, S.; Ecker, C.; Rezzolla, L. On the sound speed in neutron stars. Astrophys. J. Lett. 2022, 939, L34. [Google Scholar] [CrossRef]
- Huth, S.; Pang, P.T.H.; Tews, I.; Dietrich, T.; Le Fevre, A.; Schwenk, A.; Trautmann, W.; Agarwal, K.; Bulla, M.; Coughlin, M.W.; et al. Constraining neutron star matter with microscopic and macroscopic collisions. Nature 2022, 606, 276–280. [Google Scholar] [CrossRef] [PubMed]
- Annala, E.; Gorda, T.; Hirvonen, J.; Komoltsev, O.; Kurkela, A.; Nättilä, J.; Vuorinen, A. Strongly interacting matter exhibits deconfined behavior in massive neutron stars. arXiv 2023, arXiv:2303.11356. [Google Scholar] [CrossRef] [PubMed]
- Somasundran, R.; Tews, I.; Margueron, J. Investigating signatures of phase transitions in neutron-star cores. Phys. Rev. 2023, C107, 025801. [Google Scholar]
- Essick, R.; Legred, I.; Chatziioannou, K.; Han, S.; Landry, P. Phase transition phenomenology with nonparametric representations of the neutron star equation of state. Phys. Rev. 2023, D108, 043013. [Google Scholar] [CrossRef]
- Brandes, L.; Weise, W.; Kaiser, N. Inference of the sound speed and related properties of neutron stars. Phys. Rev. 2023, D107, 014011. [Google Scholar] [CrossRef]
- Lim, Y.; Holt, J.W. Neutron star radii, deformabilities, and moments of inertia from experimental and ab-initio theory constraints of the 208Pb neutron skin thickness. Galaxies 2022, 10, 99. [Google Scholar] [CrossRef]
- Brandes, L.; Weise, W.; Kaiser, N. Evidence against a strong first-order phase transition in neutron star cores: Impact of new data. Phys. Rev. 2023, D108, 094014. [Google Scholar]
- Mroczek, D.; Miller, M.C.; Noronha-Hostler, J.; Yunes, N. Nontrivial features in the speed of sound inside neutron stars. arXiv 2023, arXiv:2309.02345. [Google Scholar]
- Han, M.-Z.; Huang, Y.-J.; Tang, S.-P.; Fan, Y.-Z. Plausible presence of new state in neutron stars with masses above 0.98 MTOV. Sci. Bull. 2023, 68, 913–919. [Google Scholar] [CrossRef] [PubMed]
- Drischler, C.; Han, S.; Reddy, S. Large and massive neutronn stars: Implications for the sound speed within QCD of dense matter. Phys. Rev. 2022, C105, 035808. [Google Scholar]
- Gorda, T.; Kurkela, A.; Paatelainen, R.; Säppi, S.; Vuorinen, A. Soft interactions in cold quark matter. Phys. Rev. Lett. 2021, 127, 162003. [Google Scholar] [PubMed]
- Komoltsev, O.; Kurkela, A. How perturbative QCD constrains the equation of state at neutron-star densities. Phys. Rev. Lett. 2022, 128, 202701. [Google Scholar]
- Romani, R.W.; Kandel, D.; Filippenko, A.W.; Brink, T.G.; Zheng, W.K. PSR J0952–0607: The fastest and heaviest known galactic neutron star. Astrophys. J. Lett. 2022, 934, L17. [Google Scholar]
- Fasano, M.; Abdelsalhin, T.; Maselli, A.; Ferrari, V. Constraining the neutron star equation of state using multiband independent measurements of radii and tidal deformabilities. Phys. Rev. Lett. 2019, 123, 141101. [Google Scholar] [CrossRef]
- Essick, R.; Tews, I.; Landry, P.; Reddy, S.; Holz, D.E. Direct astrophysical tests of chiral effective field theory at supranuclear densities. Phys. Rev. 2020, C102, 055803. [Google Scholar] [CrossRef]
- Komoltsev, O.; Somasundaram, R.; Gorda, T.; Kurkela, A.; Margueron, J.; Tews, I. Equation of state at neutron-star densities and beyond from perturbative QCD. arXiv 2023, arXiv:2312.14127. [Google Scholar]
- Akmal, A.; Pandharipande, V.R.; Ravenhall, D.G. The equation of state of nucleon matter and neutron star structure. Phys. Rev. 1998, C58, 1804–1828. [Google Scholar]
- Fujimoto, Y.; Fukushima, K.; McLerran, L.D.; Praszalowicz, M. Trace anomaly as signature of conformality in neutron stars. Phys. Rev. Lett. 2022, 129, 252702. [Google Scholar] [CrossRef]
- Marczenko, M.; McLerran, L.D.; Redlich, K.; Sasaki, C. Reaching percolation and conformal limits in neutron stars. Phys. Rev. 2023, C107, 025802. [Google Scholar] [CrossRef]
- Rho, M. Dense baryonic matter predicted in “pseudo-conformal model”. Symmetry 2023, 15, 1271. [Google Scholar] [CrossRef]
- Ma, Y.-L.; Yang, W.-C. Topology and emergent symmetries in dense compact star matter. Symmetry 2023, 15, 776. [Google Scholar] [CrossRef]
- Nättilä, J.; Miller, M.C.; Steiner, A.W.; Kajava, J.J.E.; Suleimanov, V.F.; Poutanen, J. Neutron star mass and radius measurements from atmospheric model fits to x-ray burst cooling tail spectra. Astron. Astrophys. 2017, 608, A31. [Google Scholar]
- Sumiyoshi, K.; Kojo, T.; Furusawa, S. Equation of state in neutron stars and supernovae. In Handbook of Nuclear Physics; Tanihata, I., Toki, H., Kajino, T., Eds.; Springer: Singapore, 2023; Volume 5, pp. 3127–3177. [Google Scholar]
- Holt, J.W.; Rho, M.; Weise, W. Chiral symmetry and effective field theories for hadronic, nuclear and stellar matter. Phys. Reports 2016, 621, 2–75. [Google Scholar]
- McLerran, L.; Reddy, S. Quarkyonic matter and neutron stars. Phys. Rev. Lett. 2019, 122, 122701. [Google Scholar] [CrossRef]
- Baym, G.; Furusawa, S.; Hatsuda, T.; Kojo, T.; Togashi, H. New neutron star equation of state with quark-hadron crossover. Astrophys. J. 2019, 885, 42–49. [Google Scholar]
- Fukushima, K.; Kojo, T.; Weise, W. Hard-core deconfinement and soft-surface delocalization from nuclear to quark matter. Phys. Rev. 2020, D102, 096017. [Google Scholar] [CrossRef]
- Kojo, T.; Baym, G.; Hatsuda, T. Implications of NICER for neutron star matter: The QHC21 Equation of State. Astrophys. J. 2022, 934, 46–58. [Google Scholar] [CrossRef]
- Han, S.; Mamun, M.A.A.; Lalit, S.; Constantinou, C.; Prakash, M. Treating quarks within neutron stars. Phys. Rev. 2019, D100, 103022. [Google Scholar] [CrossRef]
- Wellenhofer, C.; Holt, J.W.; Kaiser, N.; Weise, W. Nuclear thermodynamics from chiral low-momentum interactions. Phys. Rev. 2014, C89, 064009. [Google Scholar]
- Brandes, L.; Kaiser, N.; Weise, W. Fluctuations and phases in baryonic matter. Eur. Phys. J. 2021, A57, 243. [Google Scholar] [CrossRef]
- Gorda, T.; Hebeler, K.; Kurkela, A.; Schwenk, A.; Vuorinen, A. Constraints on strong phase transitions in neutron stars. Astrophys. J. 2023, 955, 100. [Google Scholar] [CrossRef]
- Thomas, A.W.; Weise, W. The Structure of the Nucleon; Wiley-VCH: Berlin, Germany, 2001; pp. 232–246. [Google Scholar]
- Lin, Y.H.; Hammer, H.W.; Meißner, U.-G. New insights into the nucleon’s electromagnetic structure. Phys. Rev. Lett. 2022, 128, 052002. [Google Scholar]
- Kaiser, N.; Passemar, E. Spectral functions of nucleon form factors: Three-pion continua at low energies. Eur. Phys. J. 2019, A55, 16. [Google Scholar] [CrossRef]
- Brown, G.E.; Rho, M.; Weise, W. Phenomenological delineation of the quark-gluon structure from nucleon electromagnetic form factors. Nucl. Phys. 1986, A454, 669–690. [Google Scholar] [CrossRef]
- Meissner, U.-G.; Kaiser, N.; Weise, W. Nucleons as Skyrme solitons with vector mesons. Nucl. Phys. 1987, A466, 685–723. [Google Scholar] [CrossRef]
- Hill, R.J.; Kammel, P.; Marciano, W.J.; Sirlin, A. Nucleon axial radius and muonic hydrogen—A new analysis and review. Rep. Prog. Phys. 2018, 81, 096301. [Google Scholar] [CrossRef]
- Kharzeev, D.E. Mass radius of the proton. Phys. Rev. 2021, D104, 054015. [Google Scholar]
- Benhar, O. Testing the paradigm of nuclear many-body theory. Particles 2023, 6, 611–621. [Google Scholar] [CrossRef]
- Fujimoto, Y.; Kojo, T.; McLerran, L.D. Momentum shell in quarkyonic matter from explicit duality: A solvable model analysis. arXiv 2023, arXiv:2306.04304. [Google Scholar]
- Stephanov, M.; Rajagopal, K.; Shuryak, E. Signatures of the tricritical point in QCD. Phys. Rev. Lett. 1998, 81, 4816–4819. [Google Scholar]
- Fukushima, K. Spectral functions in the σ channel near the critical end point. Phys. Rev. 2003, C67, 025003. [Google Scholar]
- Fischer, C.S.; Luecker, J.; Welzbacher, C.A. Phase structure of three and four flavor QCD. Phys. Rev. 2014, D90, 034022. [Google Scholar]
- Asakawa, M.; Yazaki, K. Chiral restoration at finite density and temperature. Nucl. Phys. 1989, A504, 668–684. [Google Scholar]
- Klimt, S.; Lutz, M.F.M.; Weise, W. Chiral phase transition in the SU(3) Nambu and Jona-Lasinio model. Phys. Lett. 1990, B249, 386–390. [Google Scholar]
- Scavenius, O.; Mocsy, A.; Mishustin, I.N.; Rischke, D.H. Chiral phase transition within effective models with constituent quarks. Phys. Rev. 2001, C64, 045202. [Google Scholar] [CrossRef]
- Rößner, S.; Ratti, C.; Weise, W. Polyakov loop, diquarks and the two-flavour phase diagram. Phys. Rev. 2007, D75, 034007. [Google Scholar]
- Rößner, S.; Hell, T.; Ratti, C.; Weise, W. The chiral and deconfinement crossover transitions: PNJL model beyond mean field. Nucl. Phys. 2008, A814, 118–143. [Google Scholar]
- Hell, T.; Rößner, S.; Christoforetti, M.; Weise, W. Thermodynamics of the three-flavor nonlocal Polyakov–Nambu–Jona-Lasinio model. Phys. Rev. 2010, D81, 074023. [Google Scholar]
- Drews, M.; Weise, W. Functional renormalization group studies of nuclear and neutron matter. Prog. Part. Nucl. Phys. 2017, 93, 69–107. [Google Scholar]
- Skokov, V.; Friman, B.; Nakano, E.; Redlich, K.; Schaefer, B.-J. Vacuum fluctuations and thermodynamics od chiral models. Phys. Rev. 2010, D82, 034029. [Google Scholar]
- Zacci, A.; Schaffner-Bielich, J. Effects of renormalizing the chiral SU(2) quark-meson model. Phys. Rev. 2018, D97, 074011. [Google Scholar] [CrossRef]
- Gupta, U.S.; Tiwari, V.K. Revisiting the phase structure of the Polyakov-quark-meson model in the presence of vacuum fermion fluctuation. Phys. Rev. 2012, D85, 014010. [Google Scholar]
- Eser, J.; Blaizot, J.-P. Thermodynamics of the parity-doublet model: Symmetric nuclear matter and the chiral transition. arXiv 2023, arXiv:2309.06566. [Google Scholar]
- Lonardoni, D.; Lovato, A.; Gandolfi, S.; Pederiva, F. Hyperon puzzle: Hints from Quantum Monte Carlo calculations. Phys. Rev. Lett. 2015, 114, 092301. [Google Scholar] [CrossRef]
- Gerstung, D.; Kaiser, N.; Weise, W. Hyperon-nucleon three-body forces and strangeness in neutron stars. Eur. Phys. J. 2020, A56, 175. [Google Scholar]
- Leong, J.; Motta, T.F.; Thomas, A.W.; Guichon, P.A.M. Dense nuclear matter with phenomenological short distance repulsion. Phys. Rev. 2023, C108, 015804. [Google Scholar]
- Baym, G.; Chin, S.A. Landau theory of relativistic Fermi liquids. Nucl. Phys. 1976, A262, 527–538. [Google Scholar]
- Friman, B.; Weise, W. Neutron star matter as a relativistic Fermi liquid. Phys. Rev. 2023, C100, 065807. [Google Scholar]
- Baym, G.; Pethick, C. Landau Fermi-Liquid Theory: Concepts and Applications; Wiley: New York, NY, USA, 1991; p. 117. [Google Scholar]




| 1.9 | 2.0 | 2.1 | 2.2 | 2.3 | |
|---|---|---|---|---|---|
| 500.9 | 229.8 | 15.0 | 3.6 | 2.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brandes, L.; Weise, W. Constraints on Phase Transitions in Neutron Star Matter. Symmetry 2024, 16, 111. https://doi.org/10.3390/sym16010111
Brandes L, Weise W. Constraints on Phase Transitions in Neutron Star Matter. Symmetry. 2024; 16(1):111. https://doi.org/10.3390/sym16010111
Chicago/Turabian StyleBrandes, Len, and Wolfram Weise. 2024. "Constraints on Phase Transitions in Neutron Star Matter" Symmetry 16, no. 1: 111. https://doi.org/10.3390/sym16010111
APA StyleBrandes, L., & Weise, W. (2024). Constraints on Phase Transitions in Neutron Star Matter. Symmetry, 16(1), 111. https://doi.org/10.3390/sym16010111





