Effect of Calcium and Fullerene Symmetry Spatial Minimization on Angiogenesis
Abstract
:1. Introduction
2. Experimental Section
2.1. Synthesis of Nanoparticles (ACP-NP)
2.2. Nanoparticle Characterization
2.3. Stimulation of BJ Cells with Nanoparticles (ACP-NPs)
2.4. Stimulation of HUVEC Cells with Nanoparticles (ACP-NPs)
2.5. HUVEC Cells Tube-like Formation Assay
2.6. Optical Microscopic Images
2.7. Digital Image Processing and Three-Dimensional Reconstruction of Angiogenic Networks
2.8. Statistical Data Analysis
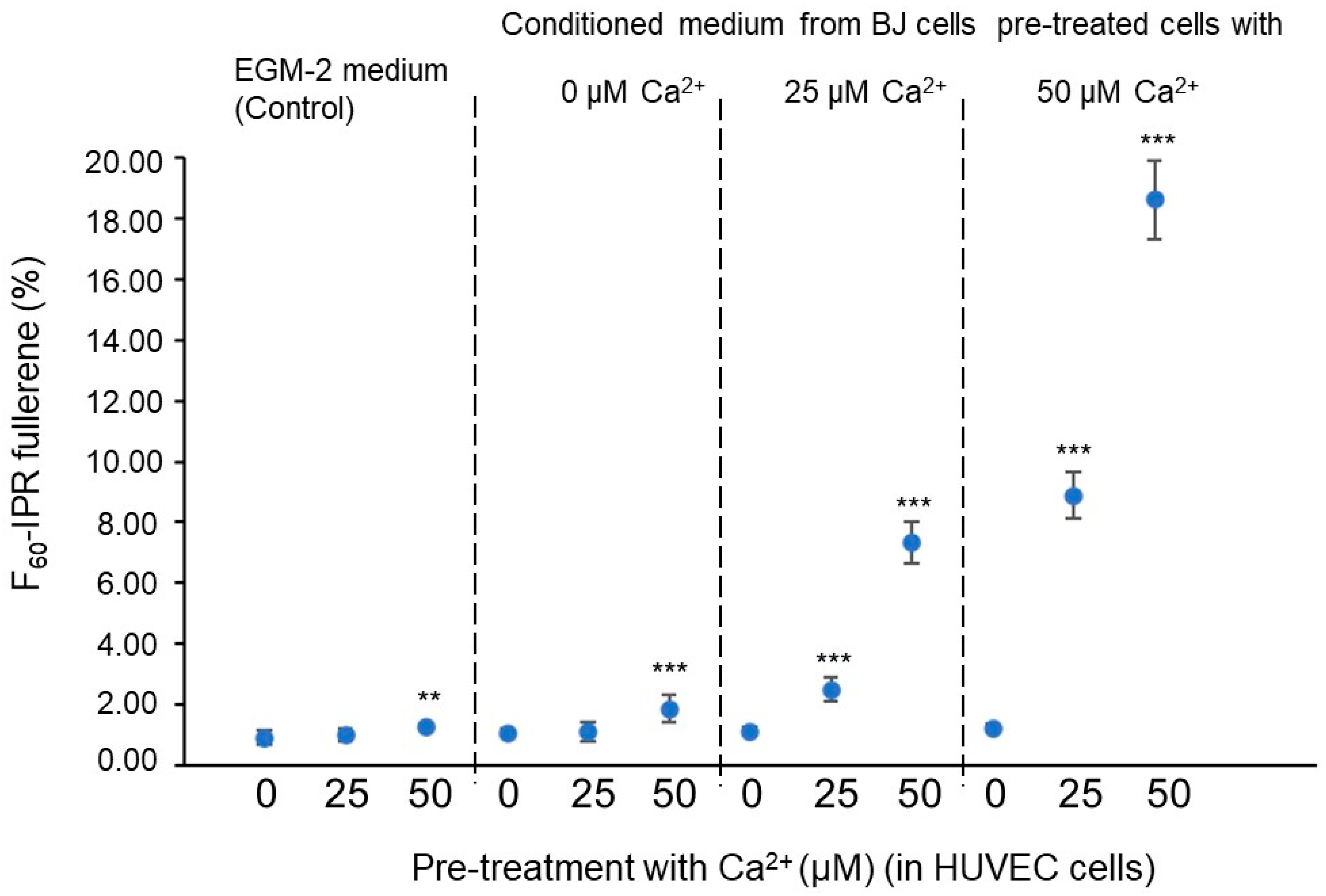
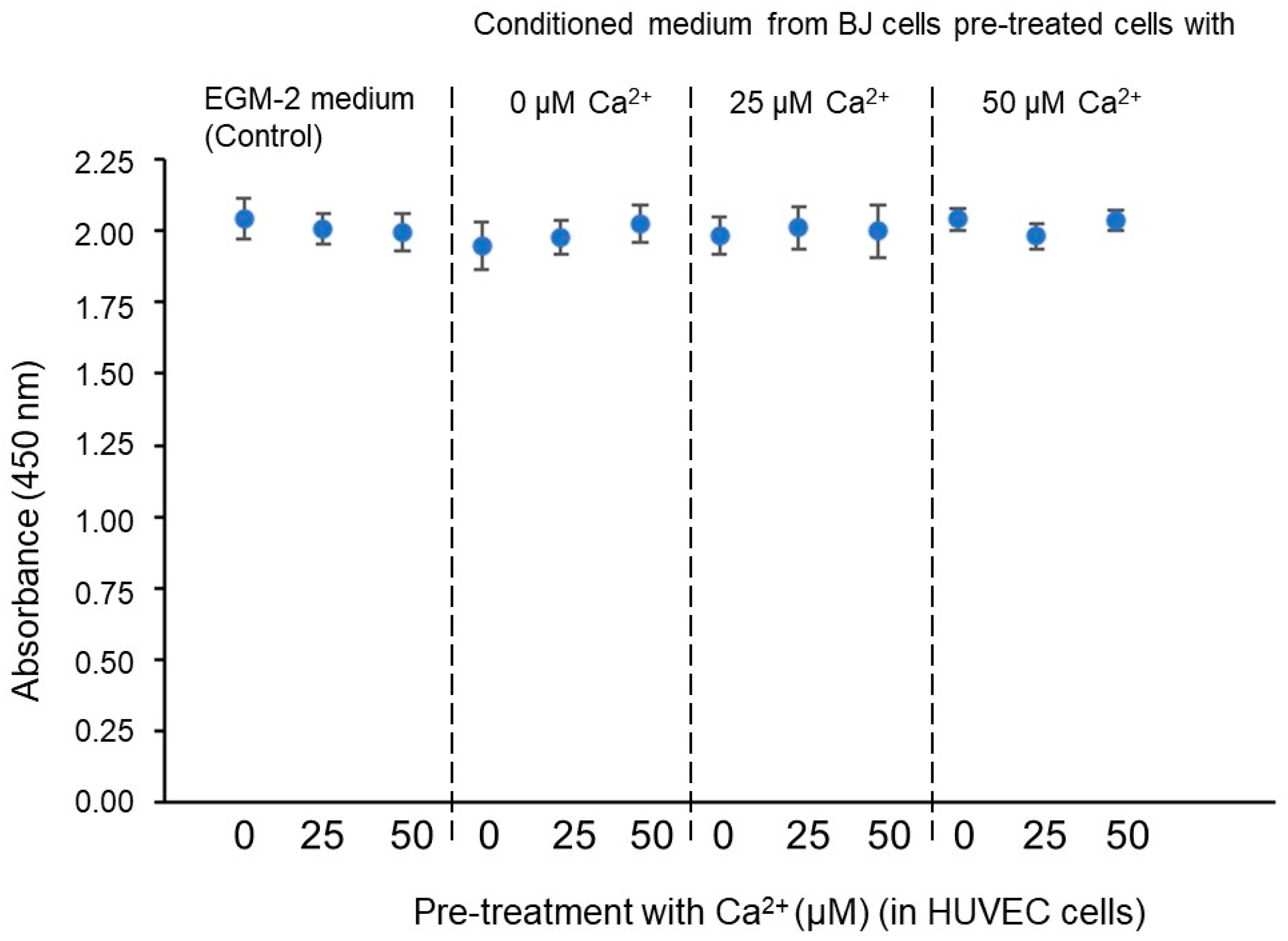
3. Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Folkmann, J. Tumor angiogenesis: Therapeutic implications. N. Engl. J. Med. 1971, 285, 1182–1186. [Google Scholar]
- Risau, W. Mechanism of angiogenesis. Nature 1997, 386, 671–674. [Google Scholar] [CrossRef] [PubMed]
- Masuda, H.; Asahara, T. Post-natal endothelial progenitor cells for neovascularization in tissue regeneration. Cardiovasc. Res. 2003, 58, 390–398. [Google Scholar] [CrossRef] [PubMed]
- Somers, G.R.; Bradbury, R.; Trute, L.; Conigrave, A.; Venter, D. Expression of the human P2Y6 nucleotide receptor in normal placenta and gestational thophoblastic disease. Lab. Investig. 1999, 79, 131–139. [Google Scholar]
- Tang, D.G.; Conti, C.J. Endothelial cell development, vasculogenesis, angiogenesis, and tumor neovascularization: An update. Semin. Thromb. Hemost. 2004, 30, 109–117. [Google Scholar] [PubMed]
- Mancuso, P.; Calleri, A.; Cassi, C.; Gobbi, A.; Capillo, M.; Pruneri, G.; Martinelli, G.; Bertolini, F. Circulating endothelial cells as a novel marker of angiogenesis. Adv. Exp. Med. Biol. 2003, 522, 83–97. [Google Scholar]
- Gómez-Gálvez, P.; Vicente-Munuera, P.; Tagua, A.; Forja, C.; Castro, A.M.; Letrán, M.; Valencia-Expósito, A.; Sotillos, S.; Martín-Bermudo, M.D.; Grima, C.; et al. Scutoids are a geometrical solution to three-dimensional packing of epithelia. Nat. Commun. 2018, 9, 2960–2974. [Google Scholar] [CrossRef]
- Serini, G.; Ambrosi, D.; Giraudo, E.; Gamba, A.; Preziosi, L.; Bussolino, F. Modeling the early stages of vascular network assembly. EMBO J. 2003, 22, 1771–1779. [Google Scholar] [CrossRef]
- Munaron, L. Intracellular calcium endothelial cells and angiogenesis. Recent Pat. Anticancer. Drug Discv. 2006, 1, 105–119. [Google Scholar] [CrossRef]
- Hanahan, D.; Folkman, J. Pattern and energy mechanisms of the angiogenesis switch during tumorogenesis. Cell 1996, 86, 353–364. [Google Scholar] [CrossRef]
- Rafii, S.; Avecilla, S.; Shmelkov, S.; Shido, K.; Tejada, R.; Moore, M.A.; Heissig, B.; Hattori, K. Angiogenic factors reconstitute hematopoiesis by recruiting stem cells from bone narrow microenvironment. Ann. N. Y. Acad. Sci. 2003, 996, 49–60. [Google Scholar] [CrossRef]
- Herskind, C.; Sitcht, C.; Sami, A.; Giordano, F.A.; Wenz, F. Gene expression profiles reveal extracellular matrix and inflammatory signaling in radiation-induced premature differentiation of human fibroblast in vitro. Frontiers in Cell Developmental Biology. 2021, 9, 539893. [Google Scholar] [CrossRef] [PubMed]
- Winkler, J.; Abisoye-Ogunniyan, A.; Metcalf, K.J.; Werb, Z. Concepts of extracellular matrix remodelling in tumour progression and metastasis. Nat. Commun. 2020, 11, 5120–5135. [Google Scholar] [CrossRef] [PubMed]
- Aisenbrey, E.A.; Murphy, W.L. Synthetic alternatives to Matrigel. Nat. Rev. Mater. 2020, 5, 539–551. [Google Scholar] [CrossRef] [PubMed]
- Karamanos, N.K.; Piperigkou, Z.; Passi, A.; Götte, M.; Rousselle, P.; Vlodavsky, I. Extracellular matrix-based cancer targeting. Trends Mol. Med. 2021, 27, 1000–1013. [Google Scholar] [CrossRef] [PubMed]
- Simons, M.; Gordon, E.; Claesson-Welsh, L. Mechanisms and regulation of endothelial VEGF receptor signaling. Nat. Rev. Mol. Cell Biol. 2016, 17, 611–625. [Google Scholar] [CrossRef] [PubMed]
- Hill, L.M.; Gavala, M.L.; Lenertz, L.Y.; Bertics, P.J. Extracellular ATP may contribute to tissue repair by rapidly stimulating purinergic receptor X7-dependent vascular endothelial growth factor release from primary human monocytes. J. Immunol. 2010, 185, 3028–3034. [Google Scholar] [CrossRef]
- Meldolesi, J.; Clementi, E.; Fusolato, C.; Zacchetti, D.; Pozzan, T. Ca2+ influx following receptor activation. Trends Pharmacol. Sci. 1991, 12, 289–292. [Google Scholar] [CrossRef]
- Clapham, D.E. Calcium signaling. Cell 2007, 131, 1047–1058. [Google Scholar] [CrossRef]
- West, A.E. Calcium regulation of neuronal gene expression. Proc. Natl. Acad. Sci. USA 2001, 98, 11024–11031. [Google Scholar] [CrossRef]
- Contreras, L. Mitochondria: The calcium connection. Biochim. Biophys. Acta 2010, 1797, 607–618. [Google Scholar] [CrossRef] [PubMed]
- Saidak, Z. The role of the calcium-sensing receptor in the development and progression of cancer. Endocrinol. Rev. 2009, 30, 1787–1795. [Google Scholar] [CrossRef] [PubMed]
- Nicholls, D.G.; Budd, S.L.; Castilho, R.F. Excitotoxicity and mitochondria. Biochem. Soc. Symp. 1999, 66, 55–67. [Google Scholar] [PubMed]
- Brookes, P.S.; Yoon, Y.; Sheu, S.S. Calcium, ATP, and ROS: A mitochondrial love-hate triangle. Am. J. Physiol. Cell Physiol. 2004, 287, C817–C833. [Google Scholar] [CrossRef] [PubMed]
- Moccia, F.; Berra-Rorneni, R.; Tanzi, F. Uptake on vascular endothelial Ca2+ signaling: A tale of ion channels, pumps and transporters. World J. Biol. Chem. 2012, 3, 127–158. [Google Scholar] [CrossRef] [PubMed]
- Moccia, F.; Tanzi, F.; Munaron, L. Endothelial remodeling and intracellular calcium machinery. Curr. Mol. Med. 2014, 14, 457–480. [Google Scholar] [CrossRef] [PubMed]
- Hill, J.M.; Zalos, G.; Halcox, J.P. Circulating endothelial progenitor cells, vascular function, and cardiovascular risk. N. Engl. J. Med. 2003, 348, 593–600. [Google Scholar] [CrossRef]
- Moccia, F.; Guerra, G. Ca2+ signalling in endothelial progenitor cells: Friends or foe? J. Cell. Physiol. 2016, 231, 314–327. [Google Scholar] [CrossRef]
- Kohn, E.C.; Alessandro, R.; Spoonster, J.; Wersto, R.P.; Liotta, L.A. Angiogenesis: Role of calcium-mediated signal transduction. Proc. Natl. Acad. Sci. USA 1995, 92, 1307–1311. [Google Scholar] [CrossRef]
- Weaire, D. The Kelvin Problem; Taylor & Francis: London, UK, 1994. [Google Scholar]
- Newkirk, E. Least-perimeter partitions of the sphere. Bachelor’s Thesis, William College, Williamstown, MA, USA, 2009. [Google Scholar]
- Spanier, E.H. Algebraic Topology; Springer: Berlin, Germany, 1995; p. 179. [Google Scholar]
- Pearse, B.M.F. Coated vesicles from pig brain: Purification and biochemical characterisation. J. Mol. Biol. 1975, 97, 93–98. [Google Scholar] [CrossRef]
- Andova, V.; Doslic, T.; Krnc, M.; Luzar, B.; Skrekovski, R. On the diameter and some related invariants of fullerene graphs. MATCH Commun. Math. Comput. Chem. 2012, 68, 109–130. [Google Scholar]
- Babic, D.; Klein, D.J.; Sah, C.H. Symmetry of fullerenes. Chem. Phys. Lett. 1993, 211, 235–241. [Google Scholar] [CrossRef]
- Bauer, A.L.; Jackson, T.L.; Jiang, Y. Topography of extracellular matrix mediates vascular morphogenesis and migration speeds in angiogenesis. PLoS Comput. Biol. 2009, 5, e100445. [Google Scholar] [CrossRef] [PubMed]
- Sporn, M.B. The war on cancer. Lancet 1996, 347, 1377–1381. [Google Scholar] [CrossRef] [PubMed]
- Chaffer, C.L.; Weinberg, R.A. A perspective on cancer cell metastasis. Science 2011, 331, 1559–1564. [Google Scholar] [CrossRef] [PubMed]
- Kanaeseki, T.; Kadota, K. The “vesicle in a basket.” A morphological study of the coated vesicle isolated from the nerve endings of the guinea pig brain, with special reference to the mechanism of membrane movements. J. Cell Biol. 1969, 42, 202–220. [Google Scholar]
- Crowther, R.A.; Finch, J.T.; Pearse, B.M.F. On the structure of coated vesicles. J. Mol. Biol. 1976, 103, 785–798. [Google Scholar] [CrossRef] [PubMed]
- Jones, D.E.H. Hollow molecules. New Sci. 1966, 32, 245. [Google Scholar]
- Osawa, E. Superaromaticity. Kagaku 1970, 25, 854–863. [Google Scholar]
- Kroto, H.W.; Heath, J.R.; O’Brien, S.C.; Curl, R.F.; Smalley, R.E. C60: Buckminster fullerene. Nature 1985, 316, 162–163. [Google Scholar] [CrossRef]
- Cox, S.J.; Graner, F. Large two-dimensional clusters of equal-area bubbles. Phil. Mag. 2003, 83, 2573–2584. [Google Scholar] [CrossRef]
- Cox, S.J. Calculations of the minimal perimeter for N deformable cells of equal area confined in a circle. Phil. Mag. Lett. 2006, 86, 569–578. [Google Scholar] [CrossRef]
- Andova, V.; Skrekovski, R. Diameter of full icosahedral-symmetry fullerene graphs. MATCH Commun. Math. Comput. Chem. 2013, 70, 205–220. [Google Scholar]
- Balaban, A.T. Topological indices based on topological distances in molecular graphs. Pure Appl. Chem. 1983, 55, 199–206. [Google Scholar] [CrossRef]
- Cvetkovic, D.; Doob, M.; Sachs, H. Spectra of Graphs—Theory and Application; Johann Ambrosius Barth Verlag: Heidelberg-Leipzig, Germany, 1995. [Google Scholar]
- Klug, H.; Alexander, L. X-ray Diffraction Procedure for Polycrystallite and Amorphous Materials, 2nd. ed.; John Wiley and Sons Press: New York, NY, USA, 1974. [Google Scholar]
- Landi, E.; Tampieri, A.; Celotti, G.; Sprio, S. Densification behaviour and mechanisms of synthetic hydroxyapatites. J. Eur. Ceram. Soc. 2000, 20, 2377–2387. [Google Scholar] [CrossRef]
- Ishida, T.; Kundu, R.K.; Yang, E.; Hirata, K.; Ho, Y.D.; Quertermous, T. Targeted disruption of endothelial cell-selective adhesion molecule inhibits angiogenic processes in vitro and in vivo. J. Biol. Chem. 2003, 278, 34598–34604. [Google Scholar] [CrossRef]
- Lloveras, B.; Edgerton, S. Evaluation of in vitro bromodeoxyuridine labeling of breast carcinomas with the use of a commercial kit. Am. J. Clin. Pathol. 1991, 95, 41–47. [Google Scholar] [CrossRef]
- Heymann, J.B.; Belnap, D.M. Bsoft: Image processing and molecular modeling for electron microscopy. J. Struct. Biol. 2007, 157, 3–18. [Google Scholar] [CrossRef]
- Sorzano, C.O.; Mazabini, R.; Velazquez-Muriel, J.; Bilbao-Castro, J.R.; Sheres, S.M.; Carazo, J.M.; Pascual-Montano, A. XMIPP: A new generation of an open-source image processing package for electron microscopy. J. Struct. Biol. 2004, 148, 194–204. [Google Scholar] [CrossRef]
- Frank, J.; Radarmacher, M.; Penczek, P.; Zhu, J.; Li, Y.; Ladjadj, M.; Leith, A. SPIDER and WEB: Processing and visualization of images in 3D electron microscopy and related fields. J. Struct. Biol. 1996, 116, 190–199. [Google Scholar] [CrossRef]
- Scheres, S.H.; Nunez-Ramirez, R.; Sorzano, C.O.; Carazo, J.M.; Marabini, R. Image processing for electron microscopy single-particle analysis using XMIPP. Nat. Protoc. 2008, 3, 977–990. [Google Scholar] [CrossRef]
- Neuman, S. The use of size-defined DNA-functionalized calcium phosphate nanoparticles to minimize intracellular calcium disturbance during transfection. Biomaterials 2009, 30, 6794–6802. [Google Scholar] [CrossRef] [PubMed]
- Rivas, M.; Turon, P.; Alemán, C.; Puiggalí, J.; del Valle, L.J. Incorporation of functionalized calcium phosphate nanoparticles in living cells. J. Clust. Sci. 2021, 5, 2781–2795. [Google Scholar] [CrossRef]
- Gamba, A.; Ambrosi, D.; Coniglio, A.; de Candia, A.; Di Talia, S.; Serini, G.; Preziosi, L.; Bussolino, F. Percolation, morphogenesis and burgers dynamics in blood vessels formation. Phys. Rev. Lett. 2003, 90, 118101. [Google Scholar] [CrossRef] [PubMed]
- Anderson, A.; Chaplain, M. Continuous and discrete mathematical-models of tumor-induced angiogenesis. Bull. Math. Biol. 1998, 60, 857–899. [Google Scholar] [CrossRef] [PubMed]
- Ambrosi, D.; Bussolino, F.; Preziosi, L. A review of vasculogenesis models. J. Theor. Med. 2005, 6, 145376. [Google Scholar] [CrossRef]
- Dai, X.; Cai, S.; Ye, Q.; Jiang, J.; Yan, X.; Xiong, X.; Jiang, Q.; Wang, A.C.-L.; Tan, Y. A novel in vitro angiogénesis: A model base on a microfluidic device. Chin. Sci. Bull. 2012, 56, 3301–3309. [Google Scholar] [CrossRef] [PubMed]
- Kroto, H.W. The stability of the fullerenes Cn, with n = 24, 28, 32, 36, 50, 60 and 70. Nature 1987, 329, 529–531. [Google Scholar] [CrossRef]
- Schein, S.; Sands-Kidner, M. A Geometric Principle May Guide Self-Assembly of Fullerene Cages from Clathrin Triskelia and from Carbon Atoms. Biophys. J. 2008, 94, 958–976. [Google Scholar] [CrossRef]
- Golderg, M. The isoperimetric problem for polyhedral. Tohoku Math. J. 1934, 40, 226–236. [Google Scholar]
- Klein, D.J.; Liu, X. Theorems for carbon cages. J. Math. Chem. 1992, 11, 199–205. [Google Scholar] [CrossRef]
- Kral, D.; Pangrac, O.; Sereni, J.S.; Skrekovski, R. Long cycles in fullerene graphs. J. Math. Chem. 2009, 45, 1021–1031. [Google Scholar] [CrossRef]
- Stone, A.J.; Wales, D.J. Theoretical studies of icosahedral C60 and some related species. Chem. Phys. Lett. 1986, 128, 501–503. [Google Scholar] [CrossRef]
- Goedgebeur, J.; Mckay, B.D. Recursive generation of IPR fullerenes. J. Math. Chem. 2015, 57, 1702–1724. [Google Scholar] [CrossRef]
- Fajtlowicz, S.; Larson, C.E. Graph-Theoretic Independence as a Predictor of Fullerene Stability. Chem. Phys. Lett. 2003, 377, 485–490. [Google Scholar] [CrossRef]
- Doslic, T. On some structural properties of fullerene graphs. J. Math. Chem. 2002, 31, 187–195. [Google Scholar] [CrossRef]
- Erman, R.; Kardos, F.; Miskuf, J. Long cycles in fullerene graphs. J. Math. Chem. 2009, 46, 1103–1111. [Google Scholar] [CrossRef]
- Fowler, P.W.; Manolopoulos, D.E.; Redmond, D.B.; Ryan, R.P. Possible symmetries of fullerene structures. Chem. Phys. Lett. 1993, 202, 371–378. [Google Scholar] [CrossRef]
- Petrie, R.J.; Doyle, A.D.; Yamada, K.M. Random versus directionally persistent cell migration. Nat. Rev. Mol. Cell Biol. 2009, 10, 538–549. [Google Scholar] [CrossRef]
- Arrieumerlou, C.; Meyer, T. A local coupling model and compass parameter for eukaryotic chemotaxis. Dev. Cell 2005, 8, 215–227. [Google Scholar] [CrossRef]
- Progogine, I.; Lefever, R.; Goldbeter, A.; Herschkowitz-Kaufman, M. Symmetry breaking instabilities in biological systems. Nature 1969, 223, 913–916. [Google Scholar] [CrossRef] [PubMed]
- Kondo, S.; Miura, T. Reaction-diffusion model as a framework for understanding biological pattern formation. Science 2010, 329, 1616–1620. [Google Scholar] [CrossRef] [PubMed]
- Dúzs, B.; Holló, G.; Kitahata, H.; Ginder, E.; Suematsu, N.J.; Lagzi, I.; Szalai, I. Appearance and suppression of Turing patterns under a periodically forced feed. Commun. Chem. 2023, 6, 3–9. [Google Scholar] [CrossRef] [PubMed]
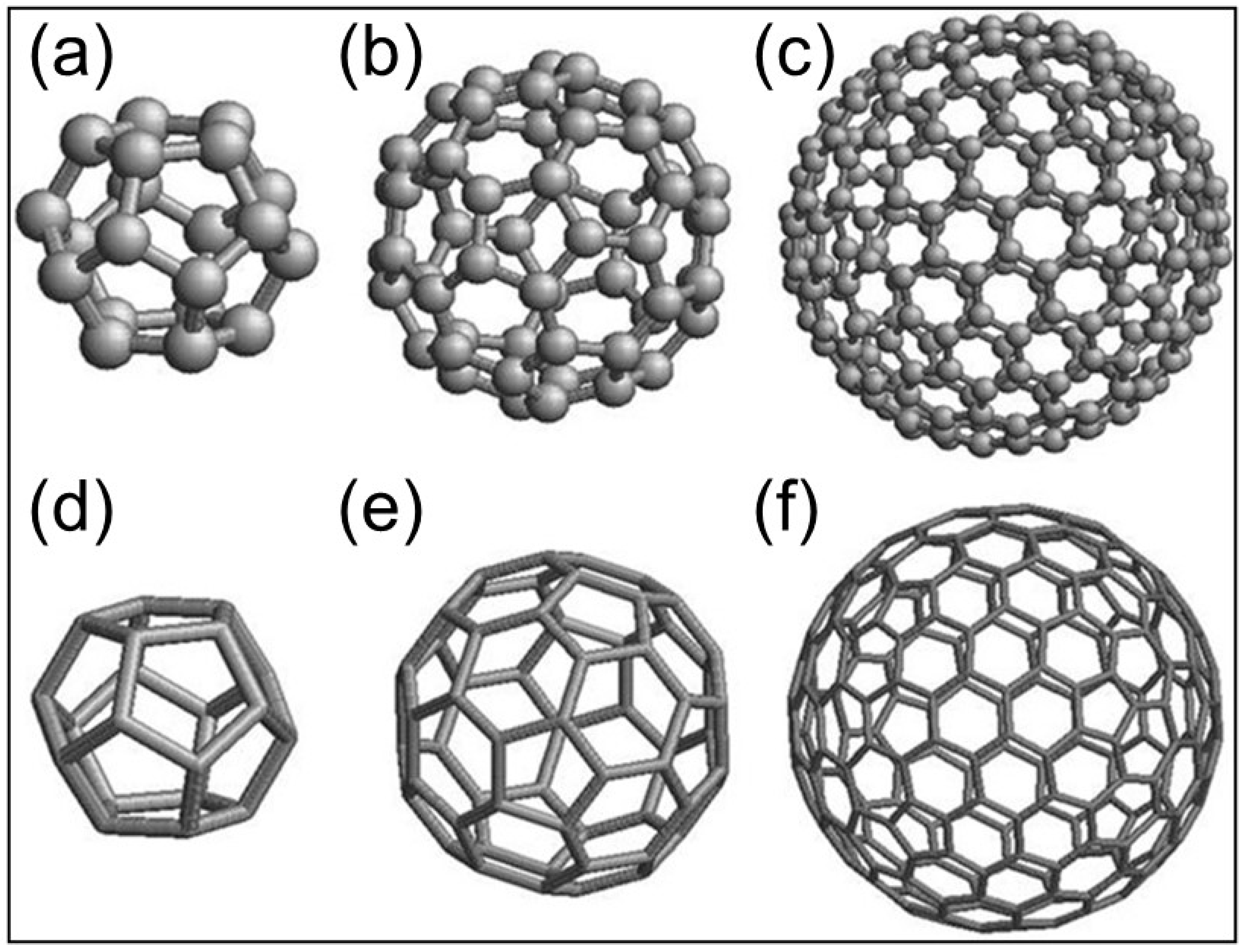

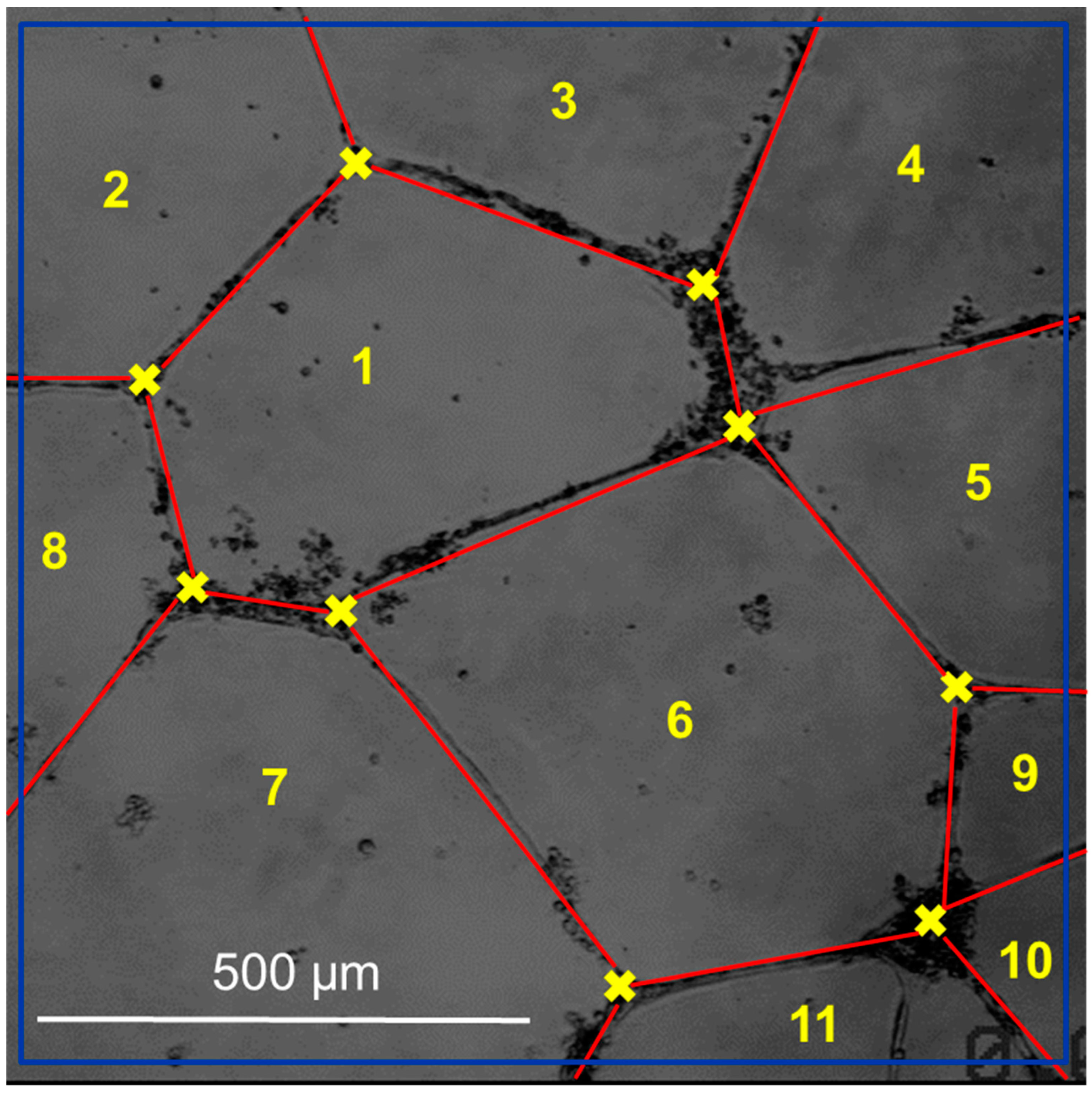
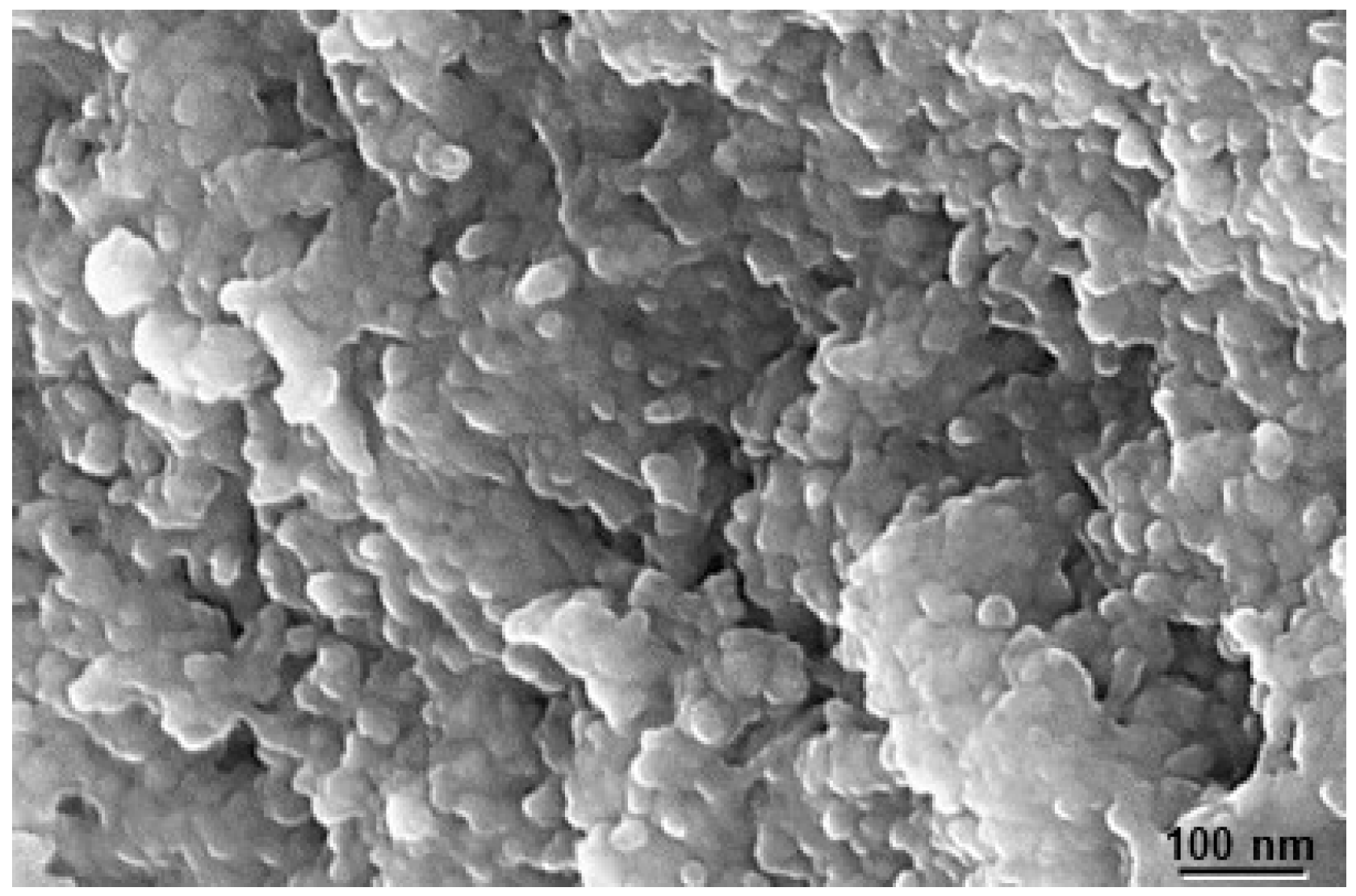


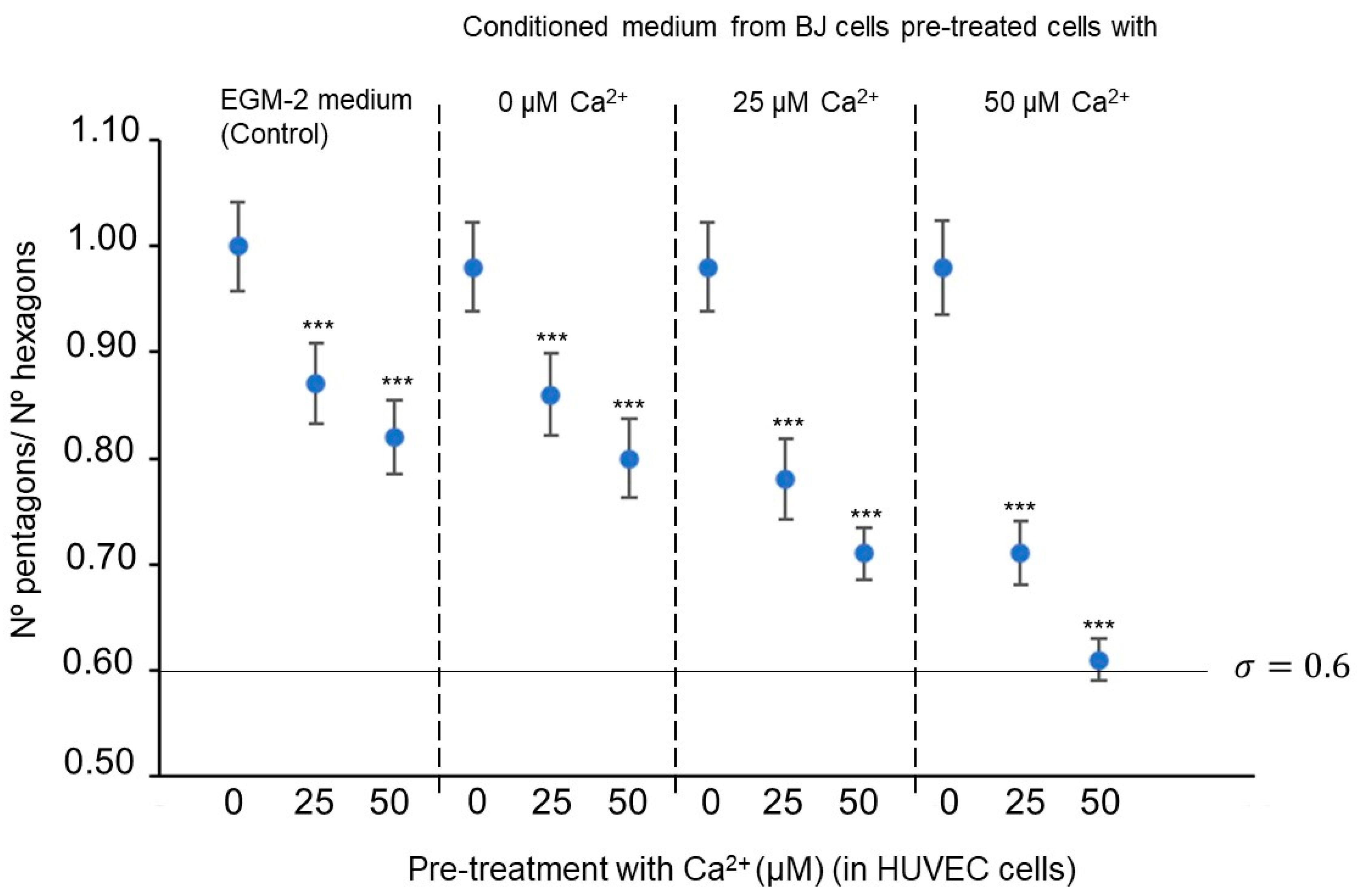
| Abbreviature | Composition |
|---|---|
| BJ-medium 1 | MEM basal medium + 1 % pen/strep + 2 mM L-glutamine + 10 %-v/v FBS |
| BJ-medium 2 | MEM basal medium (without calcium) + 1 % pen/strep + 2 mM L-glutamine + 10 %-v/v FBS |
| BJ-medium 3 | MEM basal medium (without calcium) + 1 % pen/strep + 2 mM L-glutamine + 10 %-v/v FBS. Then, calcium phosphate NPs, with diameter < 200 nm were mixed with the medium until final concentration of Ca2+ equivalent to 25 μM. |
| BJ-medium 4 | MEM basal medium (without calcium) + 1 % pen/strep + 2 mM L-glutamine + 10%-v/v FBS. Then, calcium phosphate NPs, with diameter < 200 nm were mixed with the medium until final concentration of Ca2+ equivalent to 50 μM. |
| BJ-medium 5 | MEM basal medium (without calcium), used as a washing culture medium. |
| BJ-medium 6 | Conditioned BJ-medium 2 obtained from 48 h exposition to sensitized BJ cell clone cultures and chelated with 0.5 mM EGTA. |
| BJ-medium 7 | Conditioned BJ-medium 3 obtained from 48 h exposition to sensitized BJ cell clone cultures and chelated with 0.5 mM EGTA. |
| BJ-medium 8 | Conditioned BJ-medium 4 obtained from 48 h exposition to sensitized BJ cell clone cultures and chelated with 0.5 mM EGTA. |
| Abbreviature | Composition |
|---|---|
| HUVEC-medium 1 | EGM-2 Bulletkit medium. |
| HUVEC-medium 2 | EGM-2 Bulletkit medium (without calcium). |
| HUVEC-medium 3 | EGM-2 Bulletkit medium (without calcium). Then, Ca2+ concentration in the culture media was adjusted to 25 μM adding calcium nanoparticles. |
| HUVEC-medium 4 | EGM-2 Bulletkit medium (without calcium). Then, Ca2+ concentration in the culture media was adjusted to 50 μM adding calcium nanoparticles. |
| HUVEC-medium 5 | EGM-2 Bulletkit medium (without calcium). Then, Ca2+ concentration in the culture media was adjusted to 100 μM adding calcium nanoparticles. |
| HUVEC-medium 6 | Endothelial Basal Medium (EBM) without Ca2+. |
| HUVEC-medium 7 | Endothelial Basal Medium (EBM) with Ca2+. |
| Abbreviature | Composition (v/v) |
|---|---|
| 1 | HUVEC-medium 2. |
| 2 | HUVEC-medium 3. |
| 3 | HUVEC-medium 4. |
| 4 | HUVEC-medium 2 + BJ-medium 6 (1/1). |
| 5 | HUVEC-medium 2 + BJ-medium 7 (1/1). |
| 6 | HUVEC-medium 2 + BJ-medium 8 (1/1). |
| 7 | HUVEC-medium 4 + BJ-medium 6 (1/1 to adjust the final Ca2+ concentration to 25 μM). |
| 8 | HUVEC-medium 4 + BJ-medium 7 (1/1 to adjust the final Ca2+ concentration to 25 μM). |
| 9 | HUVEC-medium 4 + BJ-medium 8 (1/1 to adjust the final Ca2+ concentration to 25 μM). |
| 10 | HUVEC-medium 5 + BJ-medium 6 (1/1 to adjust the final Ca2+ concentration to 50 μM). |
| 11 | HUVEC-medium 5 + BJ-medium 7 (1/1 to adjust the final Ca2+ concentration to 50 μM). |
| 12 | HUVEC-medium 5 + BJ-medium 8 (1/1 to adjust the final Ca2+ concentration to 50 μM). |
| ACP-NPs | |
|---|---|
| Zeta potential (mV) Average radius (R) and length (ℓ) of the observed nanostructures | −2.5 0.2 R 2.6 ± 0.2 nm |
| Cristallinity (see Equation (1)) | = 3 ± 1% |
| Crystallite size (see Equation (2)) | L = 6 ± 1 nm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rivas, M.; Reina, M. Effect of Calcium and Fullerene Symmetry Spatial Minimization on Angiogenesis. Symmetry 2024, 16, 55. https://doi.org/10.3390/sym16010055
Rivas M, Reina M. Effect of Calcium and Fullerene Symmetry Spatial Minimization on Angiogenesis. Symmetry. 2024; 16(1):55. https://doi.org/10.3390/sym16010055
Chicago/Turabian StyleRivas, Manuel, and Manuel Reina. 2024. "Effect of Calcium and Fullerene Symmetry Spatial Minimization on Angiogenesis" Symmetry 16, no. 1: 55. https://doi.org/10.3390/sym16010055
APA StyleRivas, M., & Reina, M. (2024). Effect of Calcium and Fullerene Symmetry Spatial Minimization on Angiogenesis. Symmetry, 16(1), 55. https://doi.org/10.3390/sym16010055








