On the Properties of the Modified λ-Bernstein-Stancu Operators
Abstract
:1. Introduction
2. Some Auxiliary Lemmas and Results
3. Approximating Properties of The Operators
4. Asymptotic Behavior of The Operators
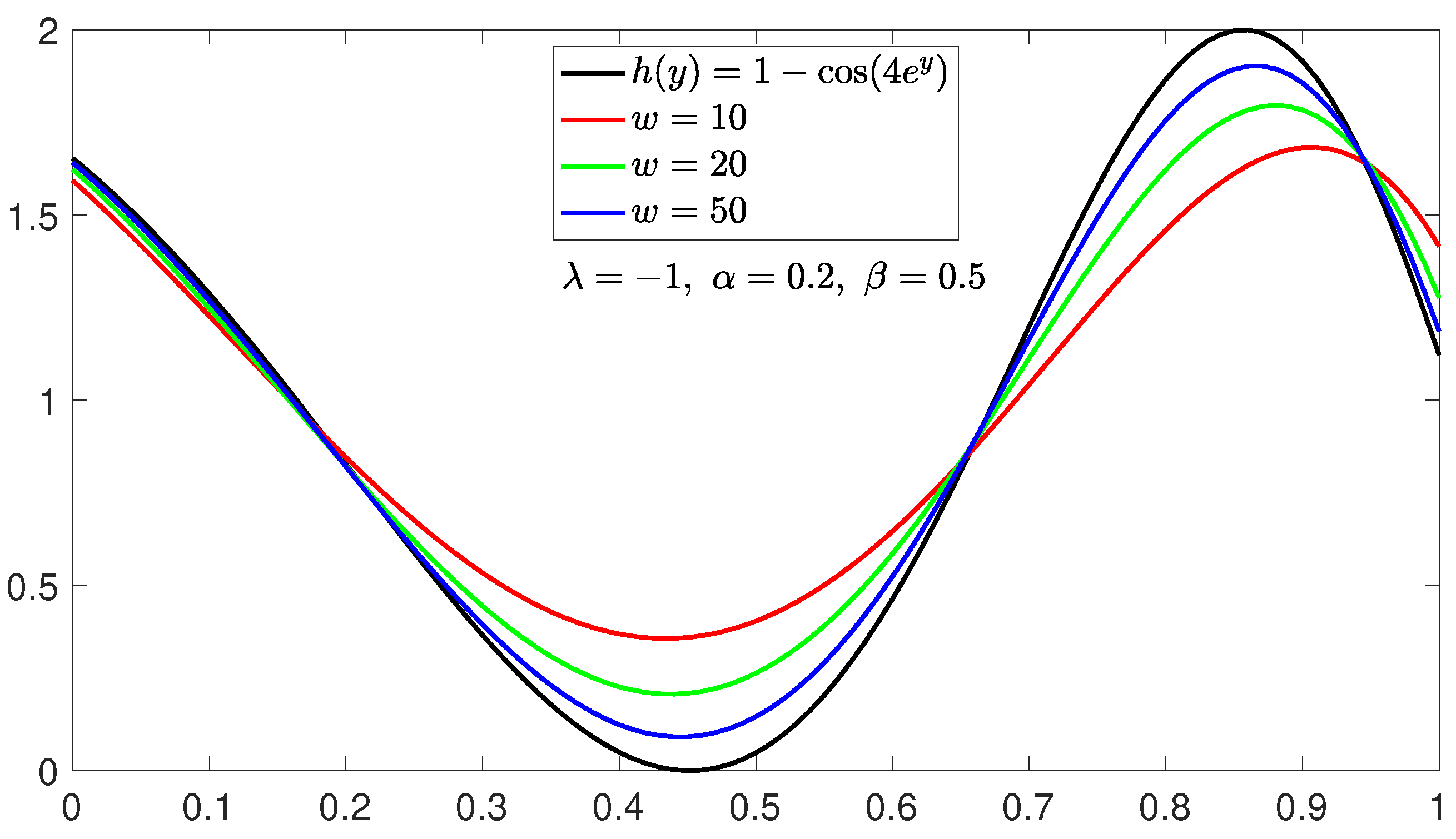
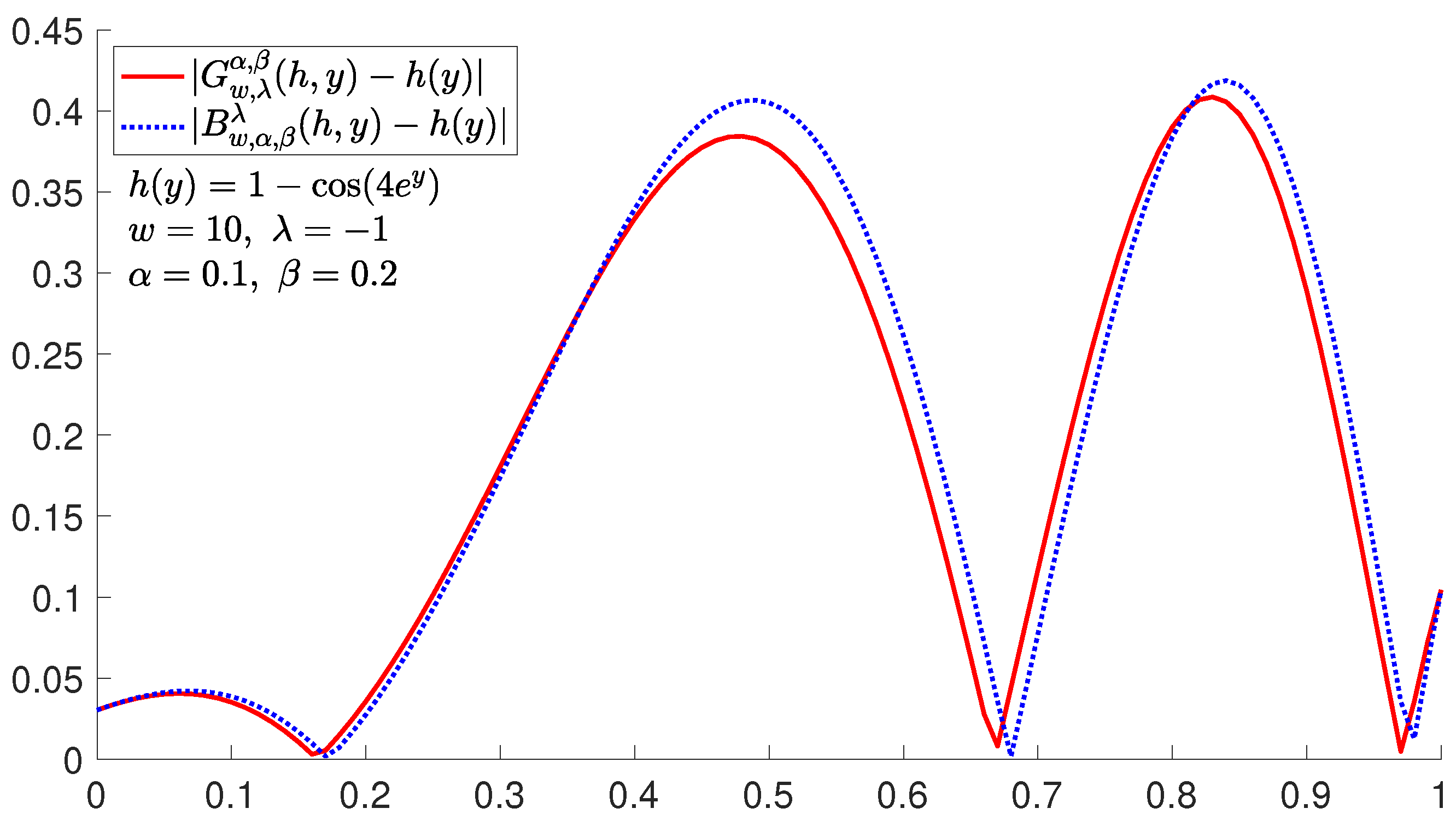
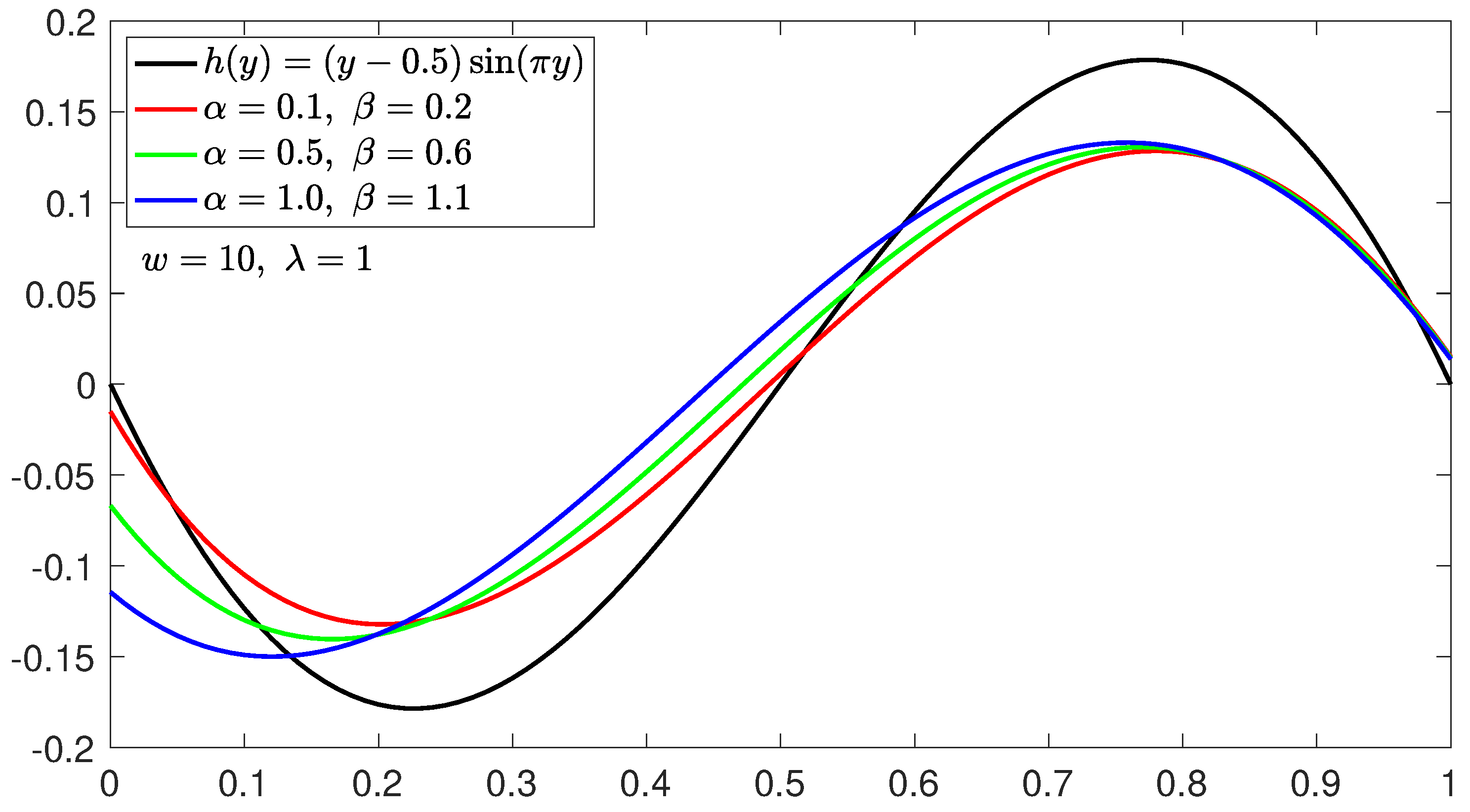
5. Some Numerical Examples and Graphs
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Weierstrass, K. Über die analytische Darstellbarkeit sogenannter willkürlicher Functionen einer reellen Veränderlichen. Sitzungsberichte der Königlich Preußischen Akad. Der Wiss. Berl. 1885, 2, 364. [Google Scholar]
- Bernšteın, S.N. Démonstration du théoreme de Weierstrass fondée sur le calcul des probabilities. Comm. Soc. Math. Kharkov 1912, 13, 1–2. [Google Scholar]
- Stancu, D.D. Approximation of function by a new class of polynomial operators. Rev. Roum. Mathématiques Pures Appliquées. Rom. J. Pure Appl. Math. Ed. Acad. Române 1968, 13, 1173–1194. [Google Scholar]
- Gadjiev, A.D.; Ghorbanalizadeh, A.M. Approximation properties of a new type Bernstein–Stancu polynomials of one and two variables. Appl. Math. Comput. 2010, 216, 890–901. [Google Scholar] [CrossRef]
- Uster, R.; İbikli, E. On The Rate of Convergence of The Stancu Type Bernstein Operators For Functions of Bounded Variation. Math. Sci. Appl. -Notes 2015, 3, 126–136. [Google Scholar] [CrossRef]
- Dong, L.X.; Yu, D.S. Pointwise approximation by a Durrmeyer variant of Bernstein-Stancu operators. J. Inequalities Appl. 2017, 2017, 1–13. [Google Scholar] [CrossRef] [PubMed]
- Çetin, N. On complex modified Bernstein-Stancu operators. Math. Found. Comput. 2023, 6, 63–77. [Google Scholar] [CrossRef]
- Cai, Q.B.; Lian, B.Y.; Zhou, G. Approximation properties of λ-Bernstein operators. J. Inequalities Appl. 2018, 2018, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Acu, A.M.; Manav, N.; Sofonea, D.F. Approximation properties of λ-Kantorovich operators. J. Inequalities Appl. 2018, 2018, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Srivastava, H.M.; Özger, F.; Mohiuddine, S.A. Construction of Stancu-type Bernstein operators based on Bézier bases with shape parameter λ. Symmetry 2019, 11, 316. [Google Scholar] [CrossRef]
- Kumar, A. Approximation properties of generalized λ-Bernstein–Kantorovich type operators. Rend. Del Circ. Mat. Palermo Ser. 2021, 70, 505–520. [Google Scholar] [CrossRef]
- Cai, Q.B.; Torun, G.; Dinlemez Kantar, Ü. Approximation Properties of Generalized λ-Bernstein–Stancu-Type Operators. J. Math. Hindawi 2021, 2021, 5590439. [Google Scholar] [CrossRef]
- Bodur, M.; Manav, N.; Tasdelen, F. Approximation properties of λ-Bernstein-kantorovich-stancu operators. Math. Slovaca 2022, 72, 141–152. [Google Scholar] [CrossRef]
- Cai, Q.B.; Khan, A.; Mansoori, M.S.; Iliyas, M.; Khan, K. Approximation by λ-Bernstein type operators on triangular domain. Filomat 2023, 37, 1941–1958. [Google Scholar] [CrossRef]
- Zhou, G.; Chen, S.; Zhao, G. Approximation properties of a new kind of λ-Bernstein Operators. 2024; in press. [Google Scholar]
- DeVore, R.A.; Lorentz, G.G. Constructive Approximation; Springer: Berlin/Heidelberg, Germany, 1993. [Google Scholar]
- Korovkin, P.P. On convergence of linear positive operators in the space of continuous functions (Russian). Dokl. Akad. Nauk. Sssr (NS) 1953, 90, 961–964. [Google Scholar]


| 0.055302823 | 0.028169281 | 0.018896155 | 0.011394022 | 0.005718237 | |
| 0.055214697 | 0.028144068 | 0.018884464 | 0.011389672 | 0.005717122 | |
| 0 | 0.055126571 | 0.028118854 | 0.018872772 | 0.011385321 | 0.005716008 |
| 0.055038445 | 0.028093641 | 0.018861081 | 0.011380971 | 0.005714894 | |
| 1 | 0.054950318 | 0.028068427 | 0.01884939 | 0.011376621 | 0.005713779 |
| 0.055029304 | 0.028087973 | 0.018858053 | 0.011379733 | 0.005714556 | |
| 0.055077937 | 0.028103414 | 0.018865413 | 0.011382527 | 0.005715282 | |
| 0 | 0.055126571 | 0.028118854 | 0.018872772 | 0.011385321 | 0.005716008 |
| 0.055175204 | 0.028134295 | 0.018880132 | 0.011388116 | 0.005716734 | |
| 1 | 0.055223838 | 0.028149736 | 0.018887492 | 0.01139091 | 0.00571746 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, Z.-P.; Torun, G.; Kangal, E.; Kantar, Ü.D.; Cai, Q.-B. On the Properties of the Modified λ-Bernstein-Stancu Operators. Symmetry 2024, 16, 1276. https://doi.org/10.3390/sym16101276
Lin Z-P, Torun G, Kangal E, Kantar ÜD, Cai Q-B. On the Properties of the Modified λ-Bernstein-Stancu Operators. Symmetry. 2024; 16(10):1276. https://doi.org/10.3390/sym16101276
Chicago/Turabian StyleLin, Zhi-Peng, Gülten Torun, Esma Kangal, Ülkü Dinlemez Kantar, and Qing-Bo Cai. 2024. "On the Properties of the Modified λ-Bernstein-Stancu Operators" Symmetry 16, no. 10: 1276. https://doi.org/10.3390/sym16101276
APA StyleLin, Z.-P., Torun, G., Kangal, E., Kantar, Ü. D., & Cai, Q.-B. (2024). On the Properties of the Modified λ-Bernstein-Stancu Operators. Symmetry, 16(10), 1276. https://doi.org/10.3390/sym16101276






