Assorted Spatial Optical Dynamics of a Generalized Fractional Quadruple Nematic Liquid Crystal System in Non-Local Media
Abstract
1. Introduction
2. Outline of the Methodology
- Step 1.
- We proceed with the wave transformation
- Step 2.
- The MSEM is performed by assuming the solution of Equation (6) as
- Step 3.
- We compute the positive integer N by implementing the homogeneous balance between terms containing the highest order derivative and nonlinearity in the completely integrated version of Equation (6).
- Step 4.
- We replace the assumed ansatz in Equation (7) with the value of N in the previous step and its essential derivatives in Equation (6). As a result, a polynomial of , , is obtained. We gather the terms of the same power of and make them vanish for each i, and we deduce a mixed algebraic–differential system. By solving this system, the values of and ’s are derived. To completely determine the exact solution of Equation (4), we place the results into Equation (5).
3. Mathematical Treatment
4. Application
- Case 1. The unknown function can be determined directly by solving the linear ordinary differential equations that appear in Equation (22), Equation (23), Equation (27), or Equation (28). With the first choice, we obtainandwhere , and are the constants of integration. For the parameters set in Equations (30) and (31), the closed-form spatial soliton solutions of our model will bewith
- Case 3. To expand the use of the MSEM, we assume that , with arbitrary constants and . We substitute them into the system of Equations (20)–(29) and solve for possible parameters, which givesprovided that . That is, applying the backward substitution through Equation (19), Equation (16), Equation (13), and Equations (8) and (9), the nematic wave profile and tilt angle of the reduced nth-quadruple systemshould be
- Case 4. In this case, the ansatz method is merged with the MSEM by assuming that . We substitute this into the system of Equations (20)–(29), collect the coefficients of , , and make them vanish, and the conducted algebraic system is solvable for possible parameters withprovided that . The nematic wave and tilt angle of our model should be
- Case 5. As in the previous case, with , two sets of existence and constraint parameters are found and listed as follows:
- Set 1.
- Set 2.
- Case 7. In the case of , we obtain
- Set 1.
- Set 2.
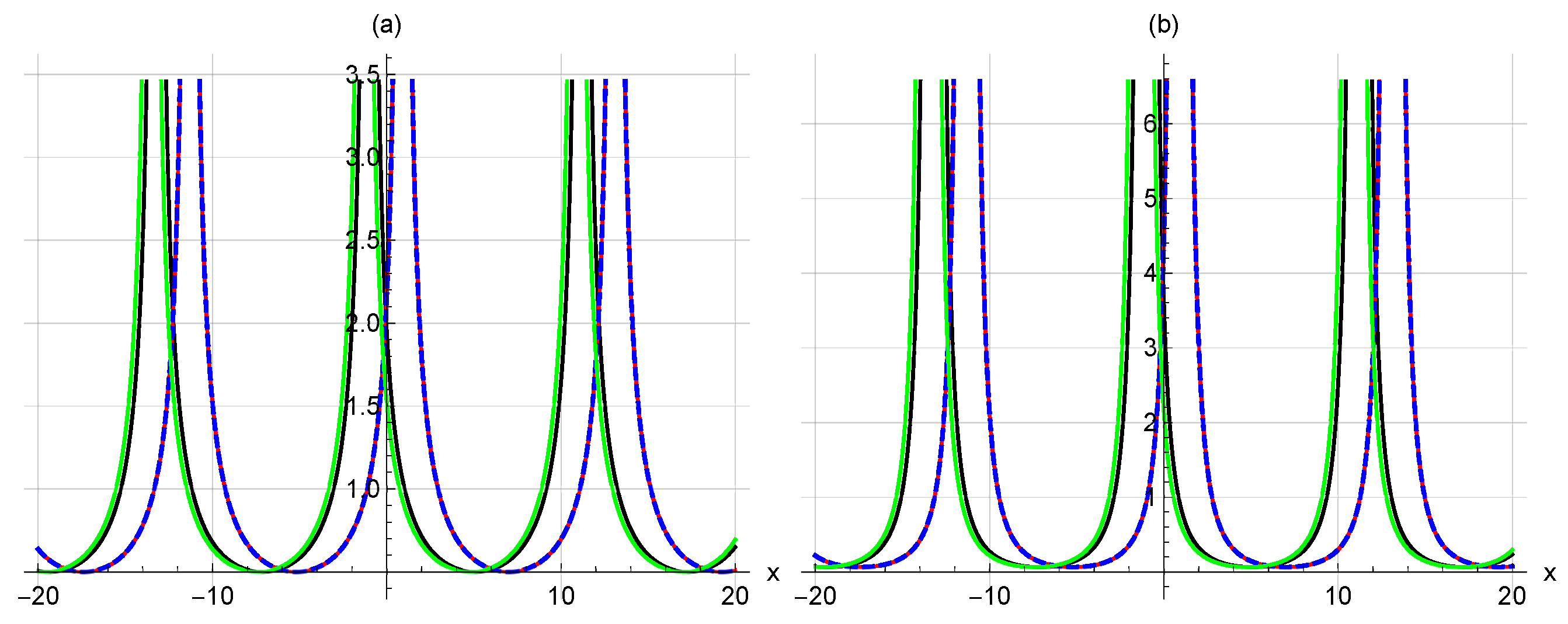
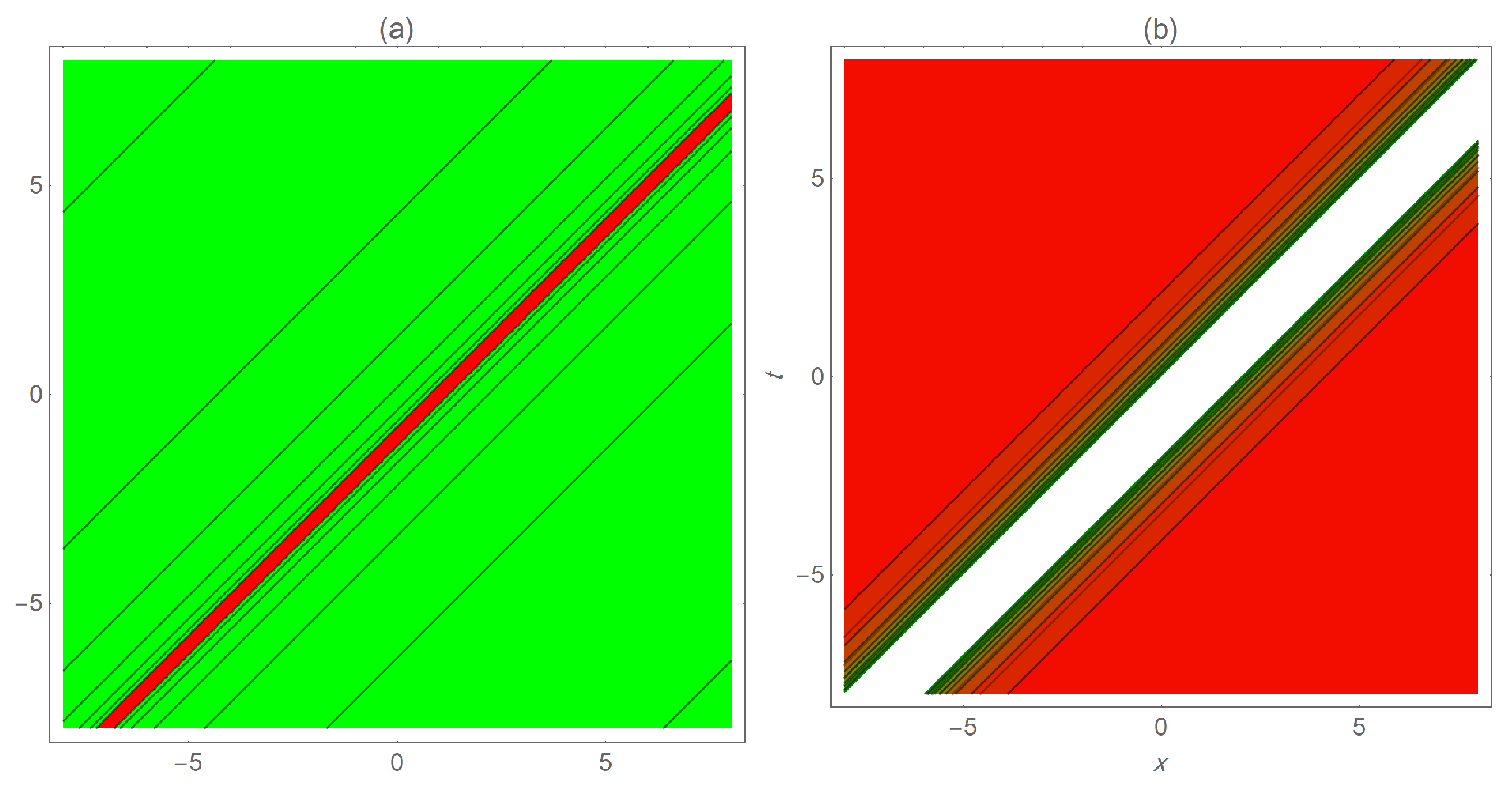
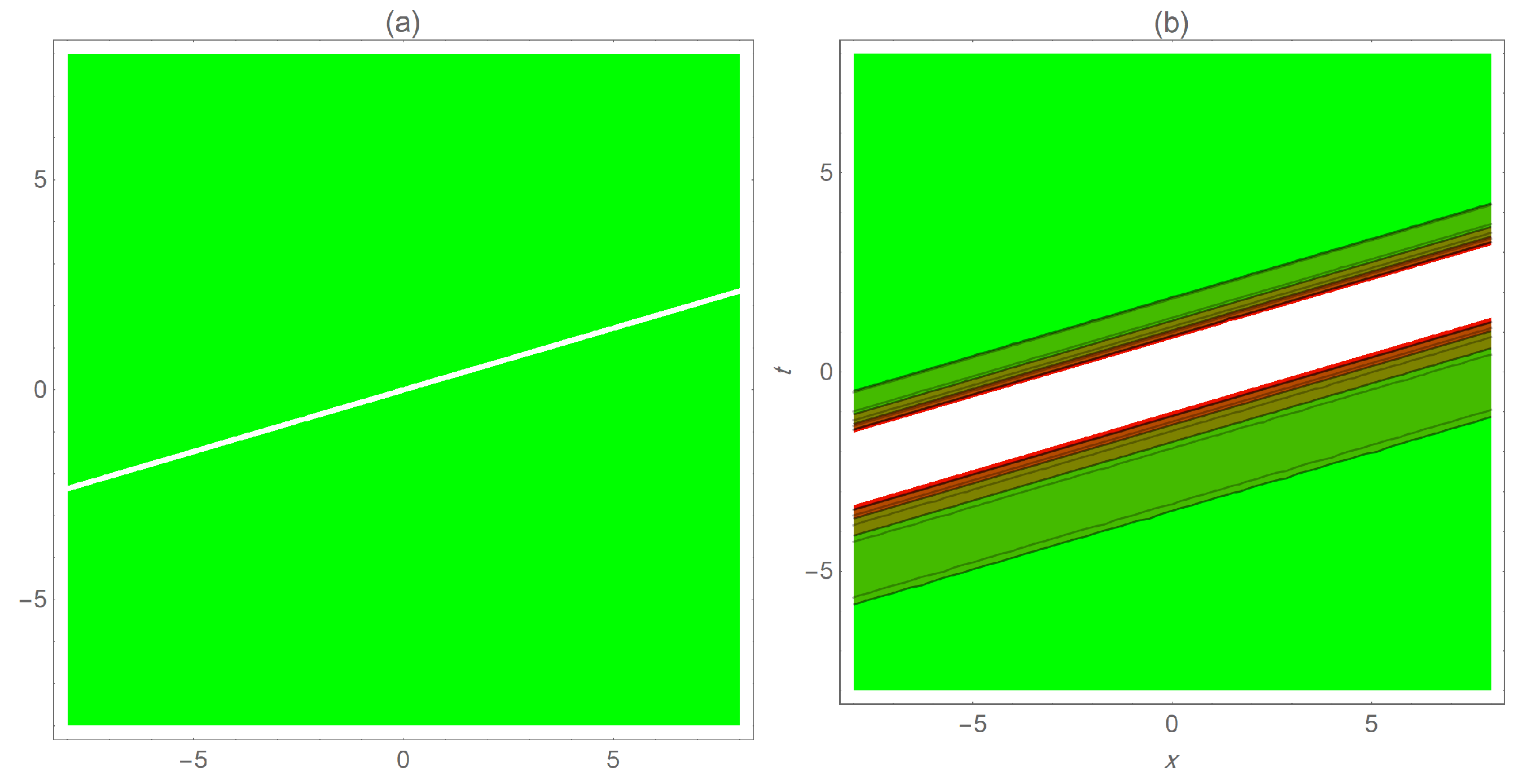
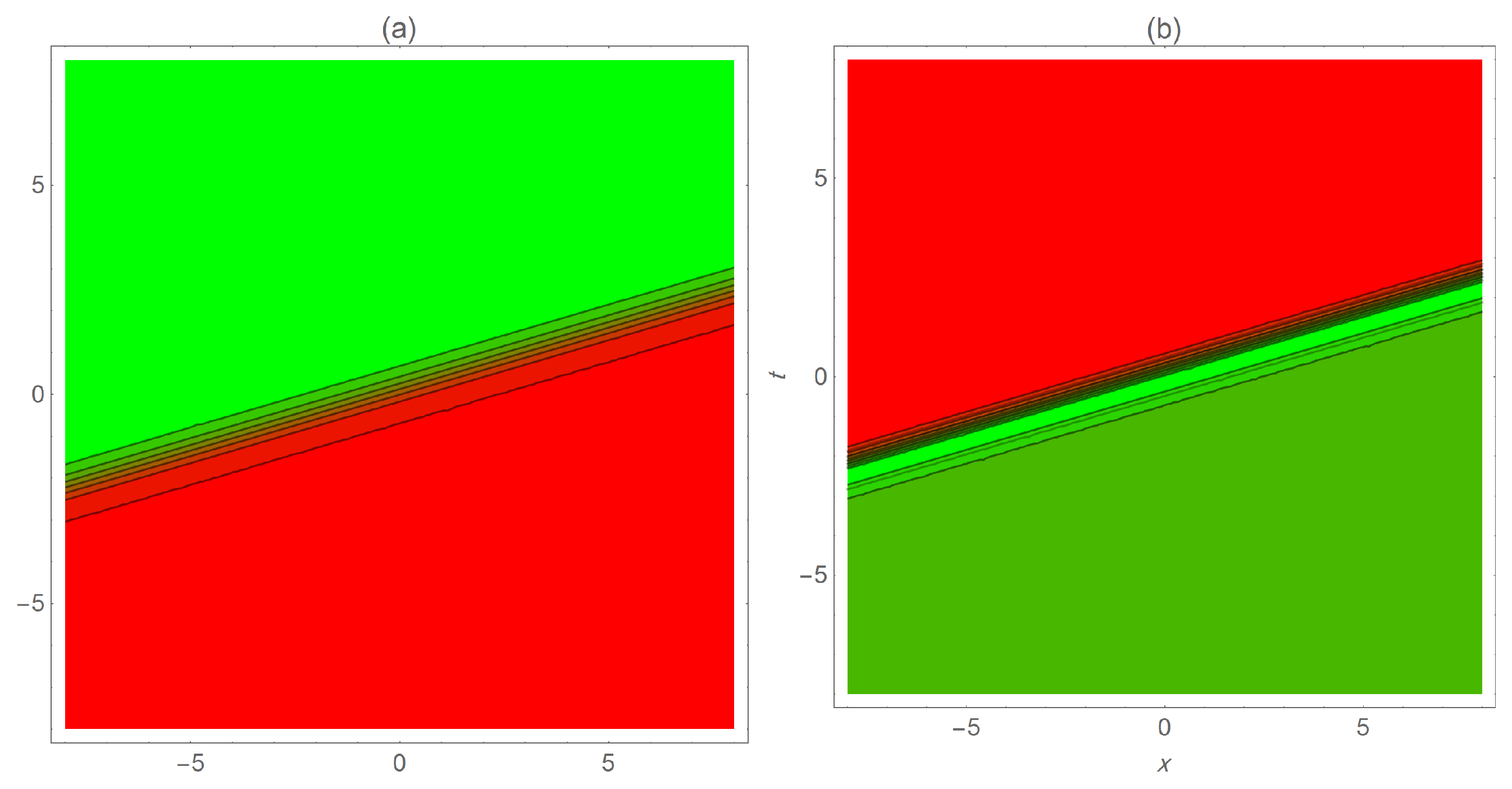
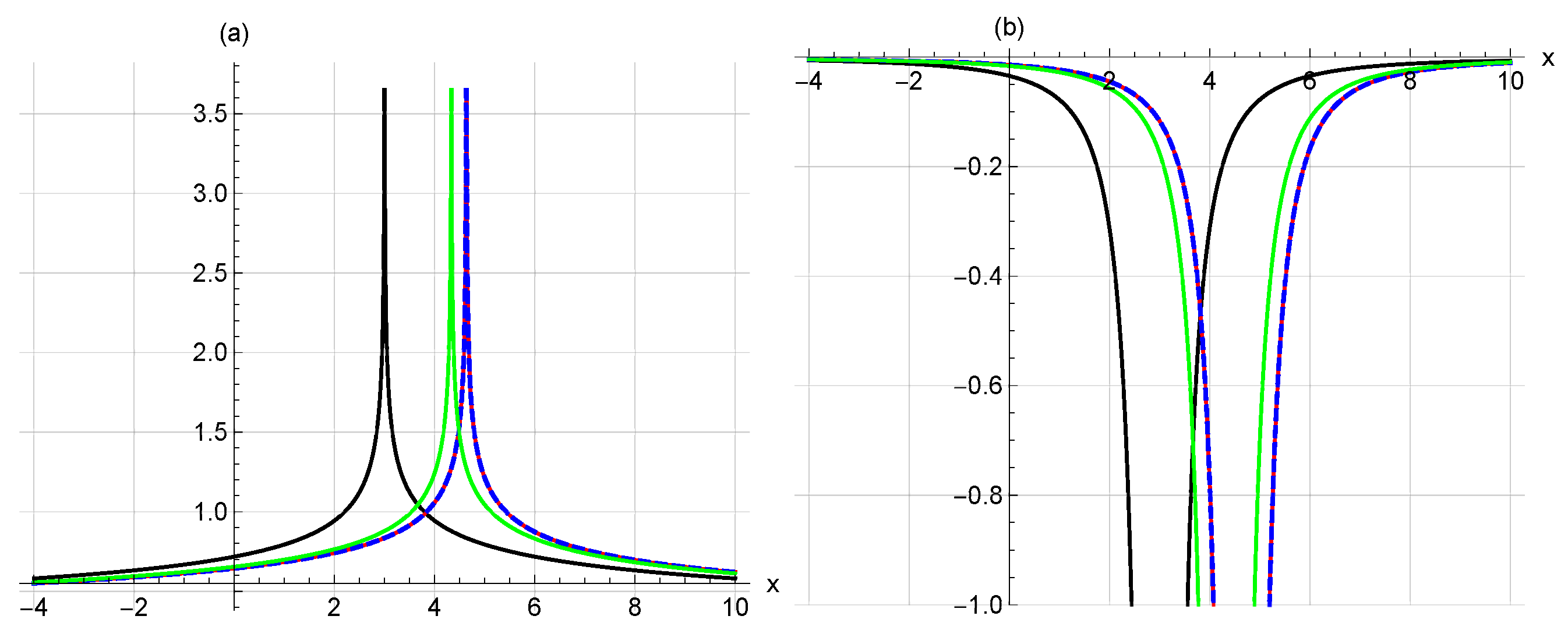
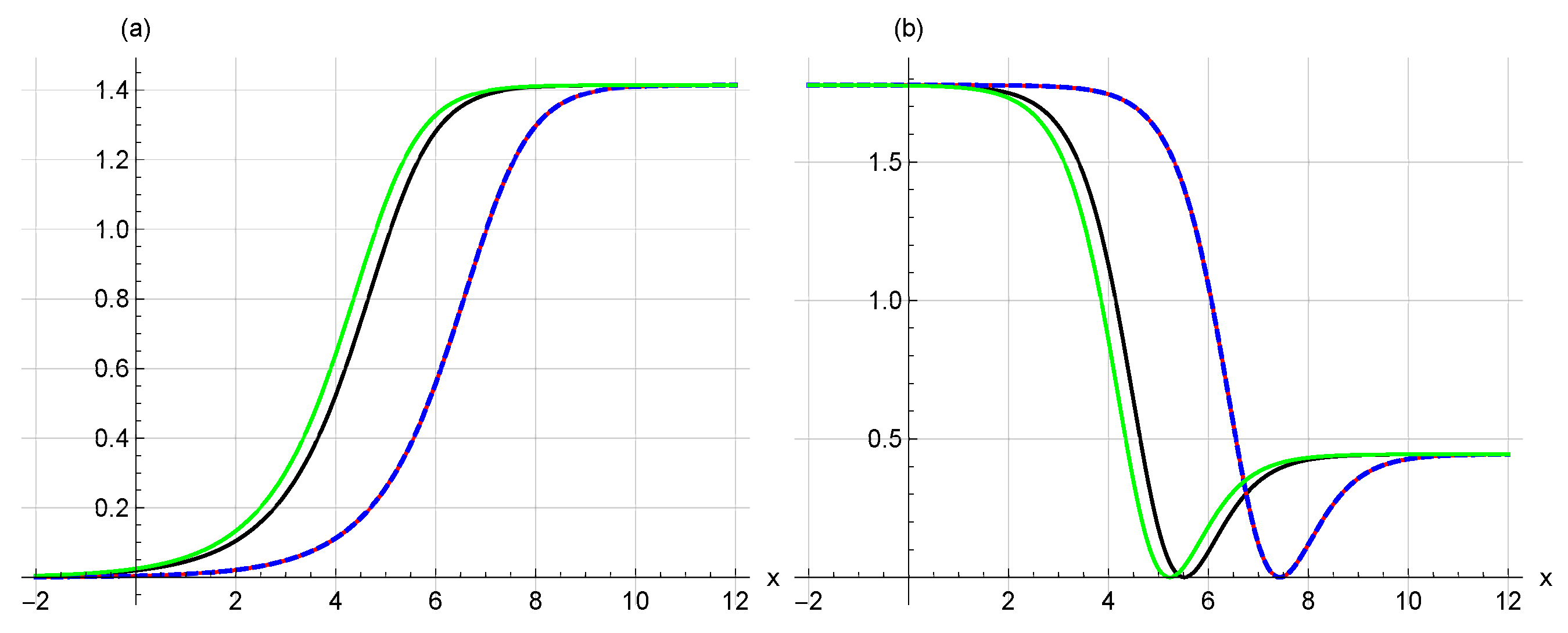
5. Graphical Representations
6. Fractional Impacts
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shi, S.; Han, D.; Cui, M. A multimodal hybrid parallel network intrusion detection model. Connect. Sci. 2023, 35, 2227780. [Google Scholar] [CrossRef]
- Zheng, Y.; Wang, Y.; Liu, J. Research on structure optimization and motion characteristics of wearable medical robotics based on Improved Particle Swarm Optimization Algorithm. Future Gener. Comput. Syst. 2022, 129, 187–198. [Google Scholar] [CrossRef]
- Faridi, W.A.; Bakar, M.A.; Riaz, M.B.; Myrzakulova, Z.; Myrzakulov, R.; Mostafa, M.A. Exploring the optical soliton solutions of Heisenberg ferromagnet-type of Akbota equation arising in surface geometry by explicit approach. Opt. Quantum Electron. 2024, 56, 1046. [Google Scholar] [CrossRef]
- Nadeem, M.; Iambor, L.F. Numerical Study of Time-Fractional Schrödinger Model in One-Dimensional Space Arising in Mathematical Physics. Fractal Fract. 2024, 8, 277. [Google Scholar] [CrossRef]
- Fei, R.; Guo, Y.; Li, J.; Hu, B.; Yang, L. An Improved BPNN Method Based on Probability Density for Indoor Location. IEICE Trans. Inf. Syst. 2023, 106, 773–785. [Google Scholar] [CrossRef]
- Az-Zo’bi, E. An approximate analytic solution for isentropic flow by an inviscid gas model. Arch. Mech. 2014, 66, 203–212. [Google Scholar]
- Sharma, N.; Alhawael, G.; Goswami, P.; Joshi, S. Variational iteration method for n-dimensional time-fractional Navier–Stokes equation. Appl. Math. Sci. Eng. 2024, 32, 2334387. [Google Scholar] [CrossRef]
- Moosavi Noori, S.R.; Taghizadeh, N. Study of Convergence of Reduced Differential Transform Method for Different Classes of Differential Equations. Int. J. Differ. Equ. 2021, 2021, 6696414. [Google Scholar] [CrossRef]
- Az-Zo’bi, E.A. Reliable analytic study for higher-dimensional telegraph equation. J. Math. Comput. Sci. 2018, 18, 423–429. [Google Scholar] [CrossRef]
- Kumar, S.; Malik, S.; Rezazadeh, H. The integrable Boussinesq equation and it’s breather, lump and soliton solutions. Nonlinear Dyn. 2022, 107, 2703–2716. [Google Scholar] [CrossRef]
- Ionescu, C.; Constantinescu, R. Optimal Choice of the Auxiliary Equation for Finding Symmetric Solutions of Reaction–Diffusion Equations. Symmetry 2024, 16, 335. [Google Scholar] [CrossRef]
- Rehman, H.U.; Seadawy, A.R.; Razzaq, S.; Rizvi, S.T. Optical fiber application of the Improved Generalized Riccati Equation Mapping method to the perturbed nonlinear Chen-Lee-Liu dynamical equation. Optik 2023, 290, 171309. [Google Scholar] [CrossRef]
- Fatema, K.; Islam, M.E.; Akhter, M.; Akbar, M.A.; Inc, M. Transcendental surface wave to the symmetric regularized long-wave equation. Phys. Lett. A 2022, 439, 128123. [Google Scholar] [CrossRef]
- Chen, H.; Zhu, Q.; Qi, J. Further results about the exact solutions of conformable space–time fractional Boussinesq equation (FBE) and breaking soliton (Calogero) equation. Results Phys. 2022, 37, 105428. [Google Scholar] [CrossRef]
- Liu, F.; Feng, Y. The modified generalized Kudryashov method for nonlinear space–time fractional partial differential equations of Schrödinger type. Results Phys. 2023, 53, 106914. [Google Scholar] [CrossRef]
- Alsharidi, A.K.; Bekir, A. Discovery of New Exact Wave Solutions to the M-Fractional Complex Three Coupled Maccari’s System by Sardar Sub-Equation Scheme. Symmetry 2023, 15, 1567. [Google Scholar] [CrossRef]
- Cao, Y.; Parvaneh, F.; Alamri, S.; Rajhi, A.A.; Anqi, A.E. Some exact wave solutions to a variety of the Schrödinger equation with two nonlinearity laws and conformable derivative. Results Phys. 2021, 31, 104929. [Google Scholar] [CrossRef]
- Bilal, M.; Ren, J. Dynamics of exact solitary wave solutions to the conformable time-space fractional model with reliable analytical approaches. Opt. Quant. Electron. 2022, 54, 40. [Google Scholar] [CrossRef]
- Assanto, G. Nematicons: Spatial Optical Solitons in Nematic Liquid Crystals; Wiley: Hoboken, NJ, USA, 2012. [Google Scholar]
- Peccianti, M.; Assanto, G. Nematicons. Phys. Rep. 2012, 516, 147–208. [Google Scholar] [CrossRef]
- Simoni, F. Nonlinear Optical Properties of Liquid Crystals; World Scientific Publishing: London, UK, 1997. [Google Scholar]
- Kumar, D.; Joardar, A.K.; Hoque, A.; Paul, G.C. Investigation of dynamics of nematicons in liquid crystals by extended sinh-Gordon equation expansion method. Opt. Quant. Electron. 2019, 51, 212. [Google Scholar] [CrossRef]
- Raza, N.; Afzal, U.; Butt, A.R.; Rezazadeh, H. Optical solitons in nematic liquid crystals with Kerr and parabolic law nonlinearities. Opt. Quant. Electron. 2019, 51, 107. [Google Scholar] [CrossRef]
- Ekici, M.; Mirzazadeh, M.; Sonmezoglu, A.; Ullah, M.Z.; Zhou, Q.; Moshokoa, S.P.; Biswas, A.; Belic, M. Nematicons in liquid crystals by extended trial equation method. J. Nonlinear. Opt. Phys. Mater. 2017, 26, 1750005. [Google Scholar] [CrossRef]
- Duran, S.; Karabulut, B. Nematicons in liquid crystals with Kerr Law by sub-equation method. Alex. Eng. J. 2022, 61, 1695–1700. [Google Scholar] [CrossRef]
- Ilhan, O.A.; Manafian, J.; Alizadeh, A.A.; Baskonus, H.M. New exact solutions for nematicons in liquid crystals by the tanϕ2- expansion method arising in fluid mechanics. Eur. Phys. J. Plus 2020, 135, 313. [Google Scholar] [CrossRef]
- Ismael, H.F.; Bulut, H.; Baskonus, H.M. W-shaped surfaces to the nematic liquid crystals with three nonlinearity laws. Soft Comput. 2021, 25, 4513–4524. [Google Scholar] [CrossRef]
- Kavitha, L.; Venkatesh, M.; Gopi, D. Shape changing nonlocal molecular deformations in a nematic liquid crystal system. J. Assoc. Arab. Univ. Basic Appl. Sci. 2015, 18, 29–45. [Google Scholar] [CrossRef]
- Savescu, M.; Johnson, S.; Sanchez, P.; Zhou, Q.; Mahmood, M.F.; Zerrad, E.; Biswas, A.; Belic, M. Nematicons in liquid crystals. J. Comput. Theor. Nanosci. 2015, 12, 4667–4673. [Google Scholar] [CrossRef]
- Marchant, T.R.; Smyth, N. Approximate techniques for dispersive shock waves in nonlinear media. J. Nonlinear Opt. Phys. Mater. 2012, 21, 1250035. [Google Scholar] [CrossRef]
- Altawallbeh, Z.; Az-Zo’bi, E.; Alleddawi, A.O.; Şenol, M.; Akinyemi, L. Novel liquid crystals model and its nematicons. Opt. Quant. Electron. 2022, 54, 861. [Google Scholar] [CrossRef]
- Az-Zo’bi, E.A.; Afef, K.; Ur Rahman, R.; Akinyemi, L.; Bekir, A.; Ahmad, H.; Tashtoush, M.A.; Mahariq, I. Novel topological, non-topological, and more solitons of the generalized cubic p-system describing isothermal flux. Opt. Quant. Electron. 2024, 56, 84. [Google Scholar] [CrossRef]
- Jawad, A.J.M.; Petković, M.D.; Biswas, A. Modified simple equation method for nonlinear evolution equations. Appl. Math. Comput. 2010, 217, 869–877. [Google Scholar]
- Zayed, E.M.E.; Ibrahim, S.A.H. Exact solutions of nonlinear evolution equations in mathematical physics using the modified simple equation method. Chin. Phys. Lett. 2012, 29, 060201. [Google Scholar] [CrossRef]
- Khan, K.; Akbar, M.A.; Ali, N.H. The Modified Simple Equation Method for Exact and Solitary Wave Solutions of Nonlinear Evolution Equation: The GZK-BBM Equation and Right-Handed Noncommutative Burgers Equations. Int. Sch. Res. Not. 2013, 2013, 146704. [Google Scholar] [CrossRef]
- Az-Zo’bi, E.A. New kink solutions for the van der Waals p-system. Math. Methods Appl. Sci. 2019, 42, 6216–6226. [Google Scholar] [CrossRef]
- Al-Amr, M.O.; El-Ganaini, S. New exact traveling wave solutions of the (4+1)-dimensional Fokas equation. Comput. Math. Appl. 2017, 74, 1274–1287. [Google Scholar] [CrossRef]
- Zhao, Y.-M.; He, Y.-H.; Long, Y. The simplest equation method and its application for solving the nonlinear NLSE, KGZ, GDS, DS, and GZ equations. J. Appl. Math. 2013, 7, 960798. [Google Scholar] [CrossRef]
- Az-Zo’bi, E.A.; AlZoubi, W.A.; Akinyemi, L.; Şenol, M.; Alsaraireh, I.W.; Mamat, M. Abundant closed-form solitons for time-fractional integro–differential equation in fluid dynamics. Opt. Quant. Electron. 2021, 53, 132. [Google Scholar] [CrossRef]
- Al-Amr, M.O.; Rezazadeh, H.; Ali, K.K.; Korkmazki, A. N1-soliton solution for Schrödinger equation with competing weakly nonlocal and parabolic law nonlinearities. Commun. Theor. Phys. 2020, 72, 065503. [Google Scholar] [CrossRef]
- Rasheed, N.M.; Al-Amr, M.O.; Az-Zo’bi, E.A.; Tashtoush, M.A.; Akinyemi, L. Stable Optical Solitons for the Higher-Order Non-Kerr NLSE via the Modified Simple Equation Method. Mathematics 2021, 9, 1986. [Google Scholar] [CrossRef]
- Bakıcıerler, G.; Alfaqeih, S.; Mısırlı, E. Application of the modified simple equation method for solving two nonlinear time-fractional long water wave equations. Revista Mexicana de Fısica 2021, 67, 060701. [Google Scholar]
- Bakıcıerler, G.; Mısırlı, E. Some New Traveling Wave Solutions of Nonlinear Fluid Models via the MSE Method. Fundam. J. Math. Appl. 2021, 4, 187–194. [Google Scholar]
- Az-Zo’bi, E.A. Peakon and solitary wave solutions for the modified Fornberg-Whitham equation using simplest equation method. Int. J. Math. Comput. Sci. 2019, 14, 635–645. [Google Scholar]
- Jumarie, G. Modified Riemann-Liouville derivative and fractional Taylor series of nondifferentiable functions further results. Comput. Math. Appl. 2006, 51, 1367–1376. [Google Scholar] [CrossRef]
- Atangana, A.; Baleanu, D.; Alsaedi, A. Analysis of time-fractional hunter-saxton equation: A model of neumatic liquid crystal. Open Phys. 2016, 14, 145–149. [Google Scholar] [CrossRef]
- da Sousa, J.V.C.; de Oliveira, E.C. A new truncated M-fractional derivative type unifying some fractional derivative types with classical properties. arXiv 2017, arXiv:1704.08187. [Google Scholar]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al Zubi, M.A.; Afef, K.; Az-Zo’bi, E.A. Assorted Spatial Optical Dynamics of a Generalized Fractional Quadruple Nematic Liquid Crystal System in Non-Local Media. Symmetry 2024, 16, 778. https://doi.org/10.3390/sym16060778
Al Zubi MA, Afef K, Az-Zo’bi EA. Assorted Spatial Optical Dynamics of a Generalized Fractional Quadruple Nematic Liquid Crystal System in Non-Local Media. Symmetry. 2024; 16(6):778. https://doi.org/10.3390/sym16060778
Chicago/Turabian StyleAl Zubi, Mohammad A., Kallekh Afef, and Emad A. Az-Zo’bi. 2024. "Assorted Spatial Optical Dynamics of a Generalized Fractional Quadruple Nematic Liquid Crystal System in Non-Local Media" Symmetry 16, no. 6: 778. https://doi.org/10.3390/sym16060778
APA StyleAl Zubi, M. A., Afef, K., & Az-Zo’bi, E. A. (2024). Assorted Spatial Optical Dynamics of a Generalized Fractional Quadruple Nematic Liquid Crystal System in Non-Local Media. Symmetry, 16(6), 778. https://doi.org/10.3390/sym16060778