Least Squares Estimation of Multifactor Uncertain Differential Equations with Applications to the Stock Market
Abstract
:1. Introduction
2. Least-Squares Estimation of Multifactor Uncertain Differential Equations
| Algorithm 1: Numerical algorithm for least squares estimation. |
Step 0: Input . Step 1: Determine the feasible regions of unknown parameters. Step 2: Set a precision and a step size . Step 3: For each , set . Step 4: Set , , and . Step 5: Set and . Step 6: Compute by
Step 7: If , then set and go to Step 8. Otherwise, go to Step 6. Step 8: If , . Otherwise, . Step 9: If , go to Step 5. Otherwise, go to Step 10. Step 10: and . Step 11: If , go to Step 12. Otherwise, go to Step 4. Step 12: Compute the objective function of the optimization problem (5) by
Step 13: Find such that reaches its minimum value. Step 14: Output . |
3. Numerical Examples
4. Application to Stock Market
4.1. Data Sources
4.2. Multifactor Uncertain Mean Reversion Stock Model
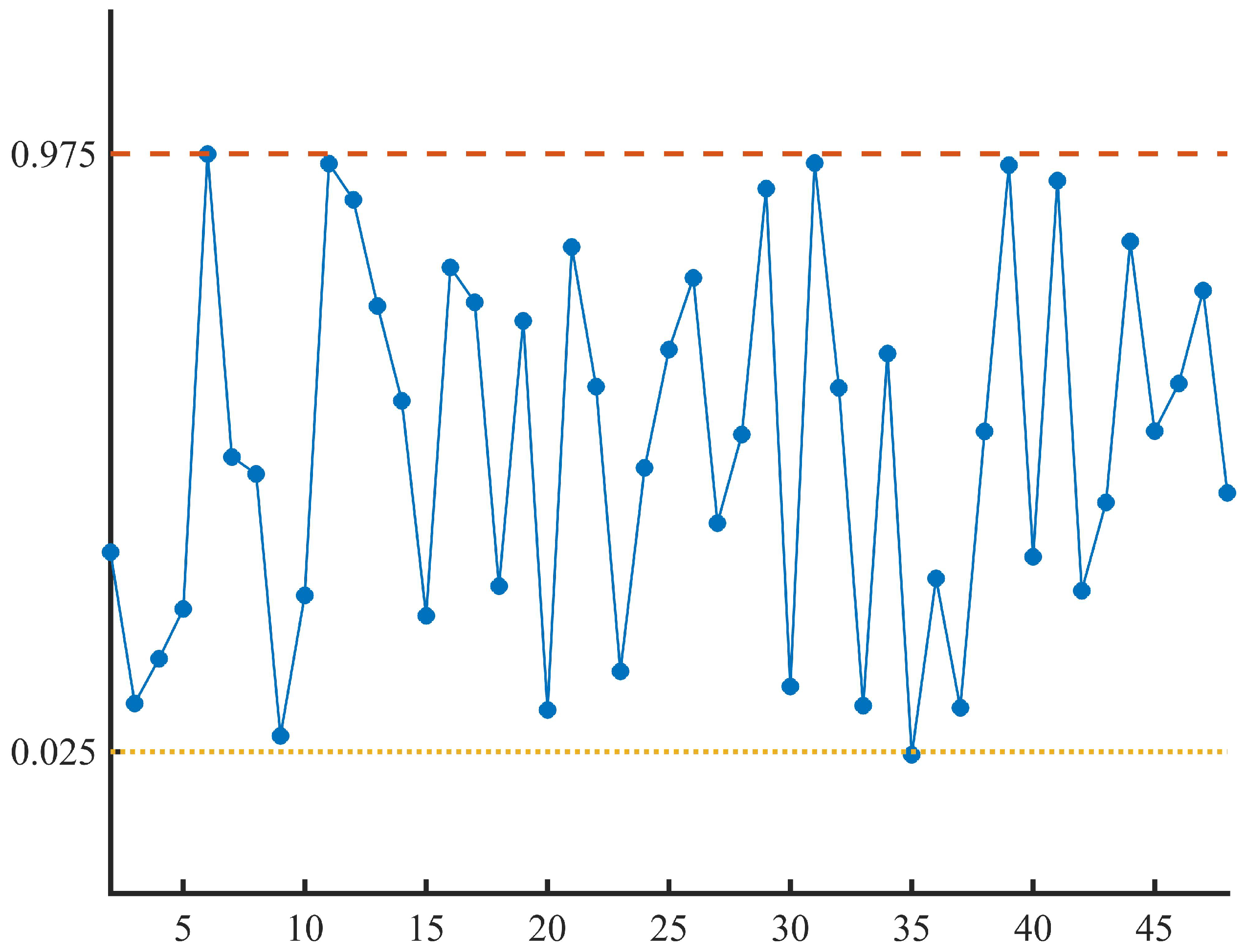
4.3. Uncertain Hypothesis Test
4.4. Stock Price Forecasting
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kolmogorov, A. Grundbegriffe der Wahrscheinlichkeitsrechnung; Springer: Berlin, Germany, 1933. [Google Scholar]
- Liu, B. Uncertainty Theory, 2nd ed.; Springer: Berlin, Germany, 2007. [Google Scholar]
- Yao, K. Uncertain Differential Equations; Springer: Berlin, Germany, 2016. [Google Scholar]
- Zhu, Y. Uncertain Optimal Control; Springer: Singapore, 2019. [Google Scholar]
- Yao, K. Uncertain Renewal Processes; Springer: Singapore, 2019. [Google Scholar]
- Zhang, B.; Peng, J. Uncertain Graph and Network Optimization; Springer: Singapore, 2022. [Google Scholar]
- Liu, B. Some research problems in uncertainty theory. J. Uncertain Syst. 2009, 3, 3–10. [Google Scholar]
- Liu, B. Fuzzy process, hybrid process and uncertain process. J. Uncertain Syst. 2008, 2, 3–16. [Google Scholar]
- Yang, L.; Liu, Y. Solution method and parameter estimation of uncertain partial differential equation with application to China’s population. Fuzzy Optim. Decis. Mak. 2024, 23, 155–177. [Google Scholar] [CrossRef]
- Ye, T.; Liu, B. Uncertain significance test for regression coefficients with application to regional economic analysis. Commun.-Stat.-Theory Methods 2023, 52, 7271–7288. [Google Scholar] [CrossRef]
- Jia, L.; Li, D.; Guo, F.; Zhang, B. Knock-in options of mean-reverting stock model with floating interest rate in uncertain environment. Int. J. Gen. Syst. 2024, 53, 331–351. [Google Scholar] [CrossRef]
- Liu, Z. Uncertain growth model for the cumulative number of COVID-19 infections in China. Fuzzy Optim. Decis. Mak. 2021, 20, 229–242. [Google Scholar] [CrossRef]
- Yang, L. Analysis of death toll from COVID-19 in China with uncertain time series and uncertain regression analysis. J. Uncertain Syst. 2022, 15, 2243007. [Google Scholar] [CrossRef]
- Ding, C.; Ye, T. Uncertain logistic growth model for confirmed COVID-19 cases in Brazil. J. Uncertain Syst. 2022, 15, 2243008. [Google Scholar] [CrossRef]
- Xie, J.; Lio, W. Uncertain nonlinear time series analysis with applications to motion analysis and epidemic spreading. Fuzzy Optim. Decis. Mak. 2024, 23, 279–294. [Google Scholar] [CrossRef]
- Yao, K.; Liu, B. Parameter estimation in uncertain differential equations. Fuzzy Optim. Decis. Mak. 2020, 19, 1–12. [Google Scholar] [CrossRef]
- Liu, Z. Generalized moment estimation for uncertain differential equations. Appl. Math. Comput. 2021, 392, 125724. [Google Scholar] [CrossRef]
- Sheng, Y.; Yao, K.; Chen, X. Least squares estimation in uncertain differential equations. IEEE Trans. Fuzzy Syst. 2020, 28, 2651–2655. [Google Scholar] [CrossRef]
- Yang, X.; Liu, Y.H.; Park, G. Parameter estimation of uncertain differential equation with application to financial market. Chaos Solitons Fract. 2020, 139, 110026. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, B. Estimating unknown parameters in uncertain differential equation by maximum likelihood estimation. Softw. Comput. 2022, 26, 2773–2780. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, B. Residual analysis and parameter estimation of uncertain differential equations. Fuzzy Optim. Decis. Mak. 2022, 21, 513–530. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, B. A modified uncertain maximum likelihood estimation with applications in uncertain statistics. Commun.-Stat.-Theory Methods 2023, 1–22. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, B. Estimation of uncertainty distribution function by the principle of least squares. Commun.-Stat.-Theory Methods 2023, 1–18. [Google Scholar] [CrossRef]
- Lio, W.; Liu, B. Initial value estimation of uncertain differential equations and zero-day of COVID-19 spread in China. Fuzzy Optim. Decis. Mak. 2021, 20, 177–188. [Google Scholar] [CrossRef]
- Mehrdoust, F.; Noorani, I.; Xu, W. Uncertain energy model for electricity and gas futures with application in spark-spread option price. Fuzzy Optim. Decis. Mak. 2023, 22, 123–148. [Google Scholar] [CrossRef]
- Yang, X.; Ke, H. Uncertain interest rate model for Shanghai interbank offered rate and pricing of American swaption. Fuzzy Optim. Decis. Mak. 2023, 22, 447–462. [Google Scholar] [CrossRef]
- Liu, Z.; Li, Y. Pricing and valuation of carbon swap in uncertain finance market. Fuzzy Optim. Decis. Mak. 2024, 1–18. [Google Scholar] [CrossRef]
- Ye, T.; Zheng, H. Analysis of birth rates in China with uncertain statistics. J. Intell. Fuzzy Syst. 2023, 44, 10621–10632. [Google Scholar] [CrossRef]
- Li, S.; Peng, J.; Zhang, B. Multifactor uncertain differential equation. J. Uncertain. Anal. Appl. 2015, 3, 7. [Google Scholar] [CrossRef]
- Ye, T.; Liu, B. Uncertain hypothesis test for uncertain differential equations. Fuzzy Optim. Decis. Mak. 2023, 22, 195–211. [Google Scholar] [CrossRef]
- Poterba, J.; Summers, L. Mean reversion in stock prices: Evidence and implications. J. Financ. Econ. 1988, 22, 27–59. [Google Scholar] [CrossRef]
- Peng, J.; Yao, K. A new option pricing model for stocks in uncertainty markets. Int. J. Oper. Res. 2011, 8, 18–26. [Google Scholar]
- Sun, Y.; Su, T. Mean-reverting stock model with floating interest rate in uncertain environment. Fuzzy Optim. Decis. Mak. 2017, 16, 235–255. [Google Scholar] [CrossRef]




| 0.08 | 0.15 | 0.34 | 0.44 | 0.54 | 0.64 | 0.89 | 0.98 | |
| 0.2000 | 0.3309 | 0.4117 | 0.3862 | 0.5283 | 0.4844 | 0.4766 | 0.6795 | |
| 1.03 | 1.25 | 1.27 | 1.31 | 1.52 | 1.68 | 1.87 | 2.09 | |
| 0.8896 | 1.0079 | 1.0060 | 1.0975 | 1.4860 | 1.5012 | 1.6221 | 1.7507 | |
| 2.30 | 2.54 | 2.69 | 2.76 | 2.96 | 3.07 | 3.13 | 3.36 | |
| 1.8903 | 1.8109 | 1.9047 | 1.8619 | 1.7348 | 1.7316 | 1.7694 | 1.5982 | |
| 3.39 | 3.57 | 3.62 | 3.66 | 3.83 | 3.99 | |||
| 1.5812 | 1.6741 | 1.6167 | 1.6351 | 1.8958 | 1.9915 |
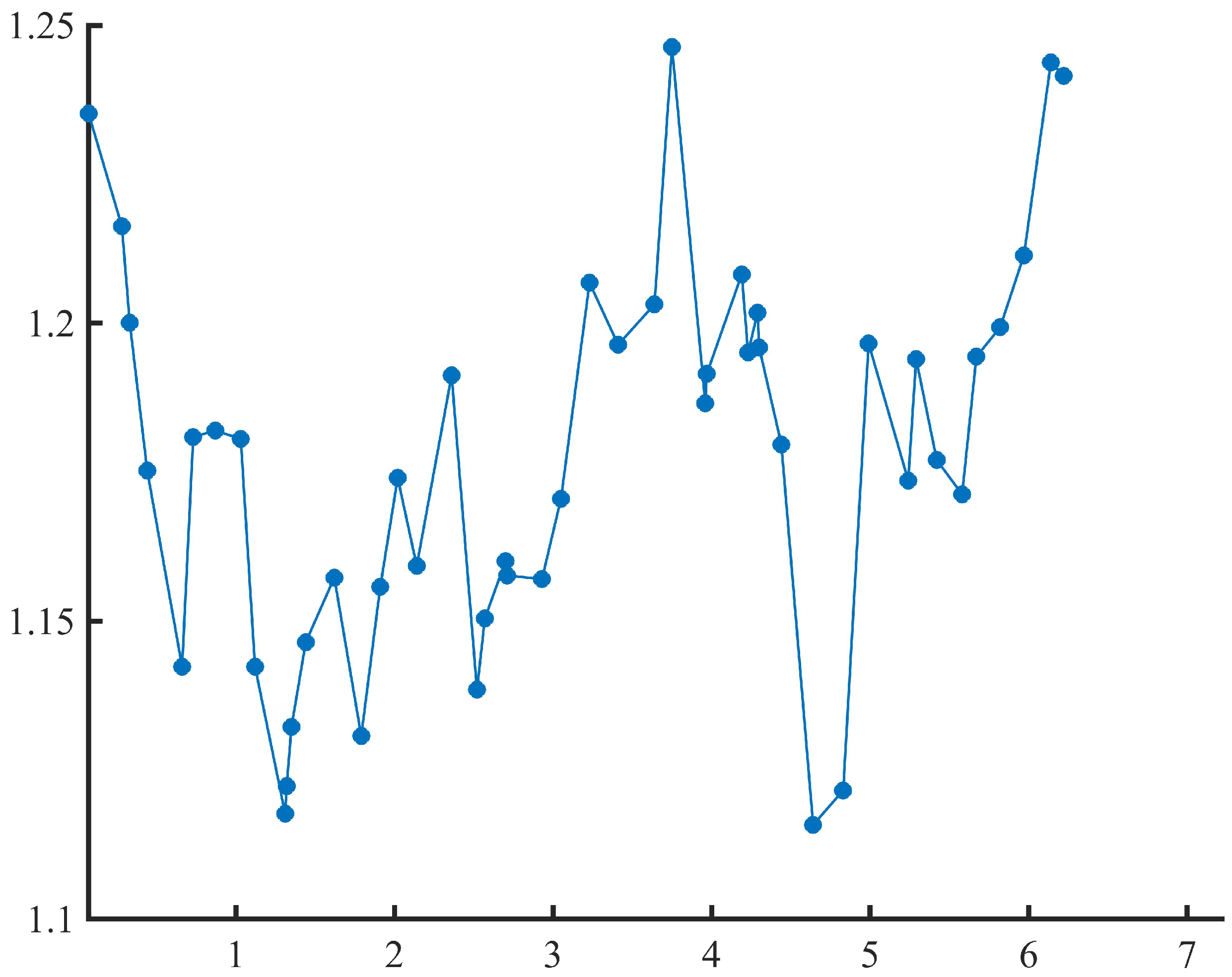
| 0.07 | 0.28 | 0.33 | 0.44 | 0.66 | 0.73 | 0.87 | 1.03 | |
| 1.2352 | 1.2163 | 1.2001 | 1.1753 | 1.1423 | 1.1809 | 1.1820 | 1.1806 | |
| 1.12 | 1.31 | 1.32 | 1.35 | 1.44 | 1.62 | 1.79 | 1.91 | |
| 1.1424 | 1.1177 | 1.1223 | 1.1322 | 1.1465 | 1.1573 | 1.1307 | 1.1558 | |
| 2.02 | 2.14 | 2.36 | 2.52 | 2.57 | 2.70 | 2.71 | 2.93 | |
| 1.1740 | 1.1593 | 1.1913 | 1.1385 | 1.1504 | 1.1601 | 1.1577 | 1.1571 | |
| 3.05 | 3.23 | 3.41 | 3.64 | 3.75 | 3.96 | 3.97 | 4.19 | |
| 1.1705 | 1.2068 | 1.1964 | 1.2032 | 1.2463 | 1.1866 | 1.1915 | 1.2082 | |
| 4.23 | 4.29 | 4.3 | 4.44 | 4.64 | 4.83 | 4.99 | 5.24 | |
| 1.1951 | 1.2018 | 1.1959 | 1.1796 | 1.1158 | 1.1216 | 1.1966 | 1.1736 | |
| 5.29 | 5.42 | 5.58 | 5.67 | 5.82 | 5.97 | 6.14 | 6.22 | |
| 1.1940 | 1.1770 | 1.1713 | 1.1944 | 1.1993 | 1.2114 | 1.2438 | 1.2415 |
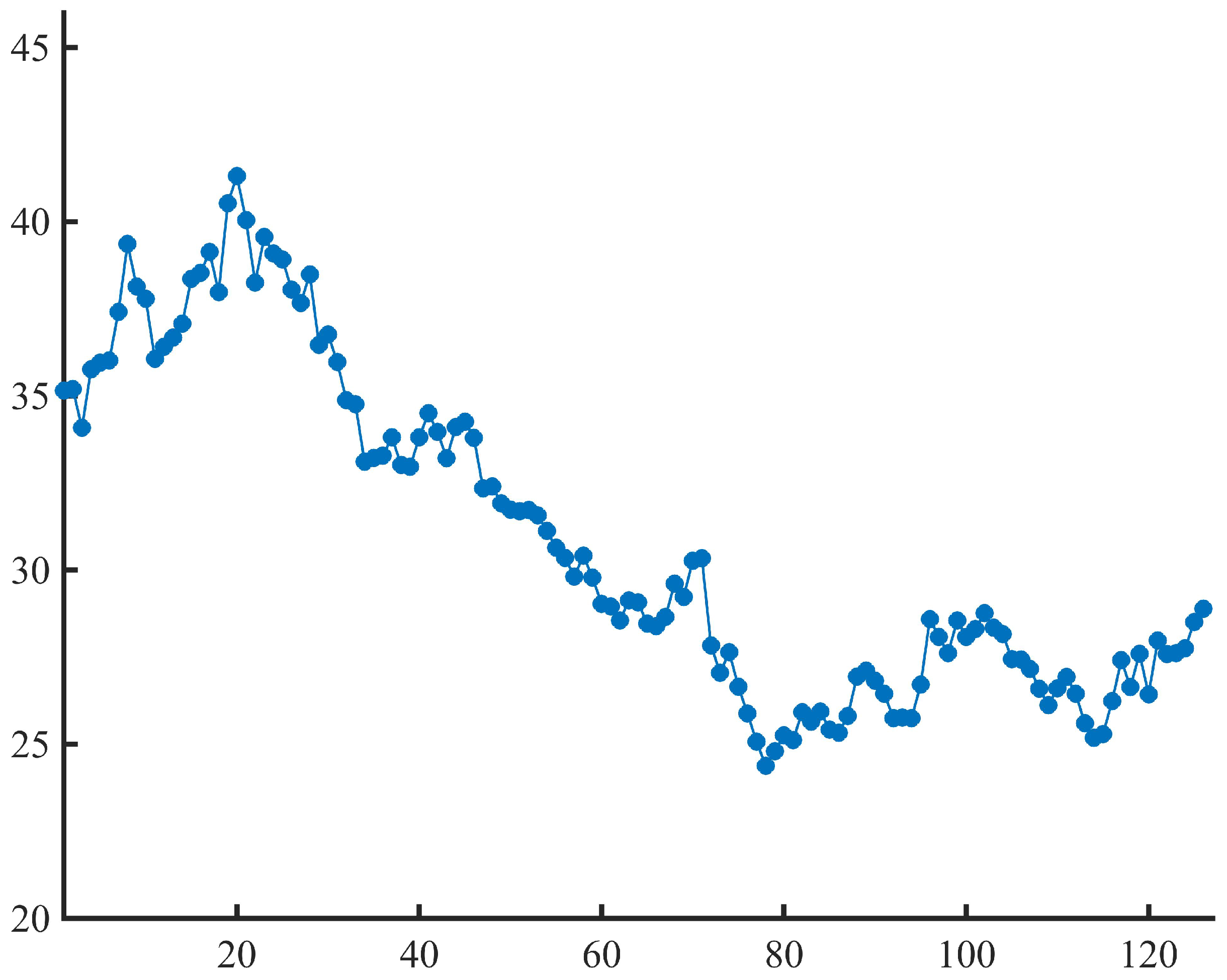
| 35.15 | 35.2 | 34.08 | 35.76 | 35.95 | 36.02 | 37.41 | 39.36 | 38.14 | 37.78 |
| 36.06 | 36.41 | 36.67 | 37.07 | 38.36 | 38.53 | 39.13 | 37.98 | 40.53 | 41.31 |
| 40.04 | 38.25 | 39.56 | 39.09 | 38.91 | 38.05 | 37.67 | 38.49 | 36.46 | 36.76 |
| 35.97 | 34.88 | 34.76 | 33.11 | 33.22 | 33.28 | 33.81 | 33.02 | 32.96 | 33.81 |
| 34.5 | 33.97 | 33.21 | 34.1 | 34.26 | 33.79 | 32.34 | 32.4 | 31.92 | 31.73 |
| 31.69 | 31.72 | 31.57 | 31.12 | 30.64 | 30.35 | 29.81 | 30.41 | 29.78 | 29.03 |
| 28.95 | 28.55 | 29.13 | 29.07 | 28.46 | 28.39 | 28.65 | 29.61 | 29.23 | 30.27 |
| 30.34 | 27.83 | 27.05 | 27.64 | 26.65 | 25.88 | 25.07 | 24.38 | 24.8 | 25.25 |
| 25.12 | 25.92 | 25.65 | 25.94 | 25.42 | 25.33 | 25.81 | 26.94 | 27.11 | 26.82 |
| 26.45 | 25.75 | 25.76 | 25.75 | 26.71 | 28.59 | 28.08 | 27.61 | 28.55 | 28.08 |
| 28.31 | 28.76 | 28.34 | 28.16 | 27.44 | 27.43 | 27.16 | 26.59 | 26.12 | 26.6 |
| 26.93 | 26.45 | 25.6 | 25.18 | 25.29 | 26.24 | 27.41 | 26.64 | 27.6 | 26.43 |
| 27.98 | 27.59 | 27.61 | 27.75 | 28.51 | 28.89 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, N.; Liu, Y. Least Squares Estimation of Multifactor Uncertain Differential Equations with Applications to the Stock Market. Symmetry 2024, 16, 904. https://doi.org/10.3390/sym16070904
Wu N, Liu Y. Least Squares Estimation of Multifactor Uncertain Differential Equations with Applications to the Stock Market. Symmetry. 2024; 16(7):904. https://doi.org/10.3390/sym16070904
Chicago/Turabian StyleWu, Nanxuan, and Yang Liu. 2024. "Least Squares Estimation of Multifactor Uncertain Differential Equations with Applications to the Stock Market" Symmetry 16, no. 7: 904. https://doi.org/10.3390/sym16070904





