Enumeration of n-Dimensional Hypercubes, Icosahedra, Rubik’s Cube Dice, Colorings, Chirality, and Encryptions Based on Their Symmetries
Abstract
:1. Introduction
2. Combinatorial and Group Theoretical Techniques
3. Results and Discussions
3.1. n-Cube Dice and Chirality
3.2. n-Cube Four-Color Problem: Dice Enumeration with Four Colors for the n-Cube and Their Chirality
3.3. Chessboard-Type Black and White Dice Enumerations in n-Dimension and Their Chirality
3.4. Rubik’s Cube Enumerations and Colorings
3.5. Dice of Different Shapes with Octahedral/Cubic Symmetries
3.6. Enumeration of Dice and Face Colorings of Different Shapes with Icosahedral Symmetries
4. Applications: Chirality, Zeolites, Mesoporous Materials, Nanomaterials, and Biological Networks
5. Applications to Cryptography
 in Wingding font. Note that with the increasing importance of artificial intelligence in the coming years, it is very clear that algorithms with machine learning and AI techniques can be developed to decrypt the messages sent through even such complex pockets of objects, for example, a grand cell-600 or a 12-cube. Indeed n-dimensional hypercubes and other combinatorially complex shapes considered here provide very compelling objects to generate packets of encrypted messages with face, edge, cell, tesseract, or other complex p-dimensional hyperplanes for complex encryption that cannot be easily decrypted without invoking very complex AI algorithms that can unravel puzzles in n-dimensional spaces. Consequently, the present investigation on combinatorics of dice of various shapes in n-dimensions indeed opens up such a plethora of applications in cryptography with potentials for defense and other applications.
in Wingding font. Note that with the increasing importance of artificial intelligence in the coming years, it is very clear that algorithms with machine learning and AI techniques can be developed to decrypt the messages sent through even such complex pockets of objects, for example, a grand cell-600 or a 12-cube. Indeed n-dimensional hypercubes and other combinatorially complex shapes considered here provide very compelling objects to generate packets of encrypted messages with face, edge, cell, tesseract, or other complex p-dimensional hyperplanes for complex encryption that cannot be easily decrypted without invoking very complex AI algorithms that can unravel puzzles in n-dimensional spaces. Consequently, the present investigation on combinatorics of dice of various shapes in n-dimensions indeed opens up such a plethora of applications in cryptography with potentials for defense and other applications.6. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Balasubramanian, K. Recursive Symmetries: Chemically Induced Combinatorics of Colorings of Hyperplanes of an 8-Cube for All Irreducible Representations. Symmetry 2023, 15, 1031. [Google Scholar] [CrossRef]
- Balasubramanian, K. Topological Indices, Graph Spectra, Entropies, Laplacians, and Matching Polynomials of n-Dimensional Hypercubes. Symmetry 2023, 15, 557. [Google Scholar] [CrossRef]
- Balasubramanian, K. Symmetry, Combinatorics, Artificial Intelligence, Music and Spectroscopy. Symmetry 2021, 13, 1850. [Google Scholar] [CrossRef]
- Carbó-Dorca, R. Boolean hypercubes and the Structure of Vector Spaces. J. Math. Sci. Model. 2018, 1, 1–14. [Google Scholar] [CrossRef]
- Carbó-Dorca, R. N-Dimensional Boolean hypercubes and the Goldbach conjecture. J. Math. Chem. 2016, 54, 1213–1220. [Google Scholar] [CrossRef]
- Carbó-Dorca, R. DNA unnatural base pairs and hypercubes. J. Math. Chem. 2018, 56, 1353–1536. [Google Scholar] [CrossRef]
- Carbó-Dorca, R.; Chakraborty, T. Quantum similarity description of a unique classical and quantum QSPR algorithm in molecular spaces: The connection with Boolean hypercubes, algorithmic intelligence, and Gödel’s incompleteness theorems. In Chemical Reactivity; Elsevier: Amsterdam, The Netherlands, 2023; pp. 505–572. [Google Scholar]
- Carbó-Dorca, R. Boolean Hypercubes as time representation holders. J. Math. Chem. 2018, 55, 1349–1352. [Google Scholar] [CrossRef]
- Carbó-Dorca, R.; Chakraborty, T. Divagations about the periodic table: Boolean hypercube and quantum similarity connections. J. Comput. Chem. 2019, 40, 2653–2663. [Google Scholar] [CrossRef]
- Kaatz, F.H.; Bultheel, A. Dimensionality of hypercube clusters. J. Math. Chem. 2016, 54, 33–43. [Google Scholar] [CrossRef]
- Gowen, A.A.; O’Donnella, C.P.; Cullenb, P.J.; Bell, S.J. Recent applications of chemical imaging to pharmaceutical process monitoring and quality control. Eur. J. Pharm. Biopharm. 2008, 69, 10–22. [Google Scholar] [CrossRef]
- Mezey, P.G. Similarity Analysis in two and three dimensions using lattice animals and ploycubes. J. Math. Chem. 1992, 11, 27–45. [Google Scholar] [CrossRef]
- Fralov, A.; Jako, E.; Mezey, P.G. Logical Models for Molecular Shapes and Families. J. Math. Chem. 2001, 30, 389–409. [Google Scholar] [CrossRef]
- Mezey, P.G. Some Dimension Problems in Molecular Databases. J. Math. Chem. 2009, 45, 1. [Google Scholar] [CrossRef]
- Mezey, P.G. Shape Similarity measures for Molecular Bodies: A Three-dimensional Topological Approach in Quantitative Shape-activity Relation. J. Chem. Inf. Comput. Sci. 1992, 32, 650. [Google Scholar] [CrossRef]
- Balasubramanian, K. Combinatorial Multinomial Generators for colorings of 4D-hypercubes and their applications. J. Math. Chem. 2018, 56, 2707–2723. [Google Scholar] [CrossRef]
- Balasubramanian, K. Nonrigid group theory, tunneling splittings, and nuclear spin statistics of water pentamer:(H2O)5. J. Phys. Chem. A 2004, 108, 5527–5536. [Google Scholar] [CrossRef]
- Balasubramanian, K. Group-Theory and Nuclear-Spin Statistics of Weakly-Bound (H2O)N, (NH3)N, (CH4)N, and NH4+(NH3)N. J. Chem. Phys. 1991, 95, 8273–8286. [Google Scholar] [CrossRef]
- Clifford, W.K. On the types of compound statement involving four classes. Proc. Manch. Lit. Philos. Soc. 1877, 16, 88–101. [Google Scholar]
- Clifford, W.K. Mathematical Papers; Tucker, R., Ed.; R.McMillan & Co.: London, UK, 1882. [Google Scholar]
- Harrison, M.A.; High, R.G. On the cycle index of a product of permutation group. J. Combin. Theory 1968, 4, 277–299. [Google Scholar] [CrossRef]
- Pólya, G.; Read, R.C. Combinatorial Enumeration of Groups, Graphs and Chemical Compounds; Springer: New York, NY, USA, 1987. [Google Scholar]
- Pólya, G. Sur les types des propositions composées. J. Symb. Log. 1940, 5, 98–103. [Google Scholar] [CrossRef]
- Banks, D.C.; Linton, S.A.; Stockmeyer, P.K. Counting Cases in Substitope Algorithms. IEEE Trans. Vis. Comput. Graph. 2004, 10, 371–384. [Google Scholar] [CrossRef] [PubMed]
- Bhaniramka, P.; Wenger, R.; Crawfis, R. Isosurfacing in higher Dimension. In Proceedings of the Visualization 2000, Salt Lake City, UT, USA, 8–13 October 2000; pp. 267–270. [Google Scholar]
- Aichholzer, O. Extremal Properties of 0/1-Polytopes of Dimension 5. In Polytopes—Combinatorics and Computation; Ziegler, G., Kalai, G., Eds.; Birkhäuser: Basel, Switzerland, 2000; pp. 11–130. [Google Scholar]
- Perez-Aguila, R. Enumerating the Configurations in the n-Dimensional Polytopes through Pólya’s counting and A Concise Representation. In Proceedings of the 2006 3rd International Conference on Electrical and Electronics Engineering, Veracruz, Mexico, 6–8 September 2006; IEEE: Piscataway, NJ, USA, 2006; pp. 1–4. [Google Scholar]
- Banks, D.C.; Stockmeyer, P.K. De Bruijn Counting for visualization Algorithms. In Mathematical Foundations of Scientific Visualization, Computer Graphics, and Massive Data Exploration; Springer: Berlin/Heidelberg, Germany, 2009; pp. 69–88. [Google Scholar]
- Perez-Aguila, R. Towards a New Approach for volume datasets based on orthogonal polytopes in four-dimensional color space. Eng. Lett. 2010, 18, 326. [Google Scholar]
- Chen, W.Y.C.; Guo, P.L. Equivalence Classes of Full-Dimensional 0/1-Polytopes with Many Vertices, January 2011. Available online: https://arxiv.org/pdf/1101.0410.pdf (accessed on 17 July 2024).
- Chen, W.Y.C. Induced cycle structures of the hyperoctahedral group. SIAM J. Disc. Math. 1993, 6, 353–362. [Google Scholar] [CrossRef]
- Ziegler, G.M. Lectures on 0/1-Polytopes. In Polytopes—Combinatorics and Computation; Kalai, G., Ziegler, G.M., Eds.; DMV Seminar; Birkhäuser: Basel, Switzerland, 2000; Volume 29. [Google Scholar] [CrossRef]
- Lemmis, P.W.H. Pólya Theory of hypercubes. Geom. Dedicata 1997, 64, 145–155. [Google Scholar] [CrossRef]
- Harary, F.; Palmer, E.M. Graphical Enumeration; Academic Press: New York, NY, USA, 1973. [Google Scholar]
- Liu, M.; Bassler, K.E. Finite size effects and symmetry breaking in the evolution of networks of competing Boolean nodes. J. Phys. A Math. Theor. 2010, 44, 045101. [Google Scholar] [CrossRef]
- Reichhardt, C.J.O.; Bassler, K.E. Canalization and symmetry in Boolean models for genetic regulatory networks. J. Phys. A Math. Theor. 2007, 40, 4339. [Google Scholar] [CrossRef]
- Balasubramanian, K. Applications of Combinatorics and Graph Theory to Quantum Chemistry and Spectroscopy. Chem. Rev. 1985, 85, 599–618. [Google Scholar] [CrossRef]
- Balasubramanian, K. Symmetry Groups of Nonrigid Molecules as Generalized Wreath-Products and Their Representations. J. Chem. Phys. 1980, 72, 665–677. [Google Scholar] [CrossRef]
- Balasubramanian, K. Nonrigid water octamer: Computations with the 8-cube. J. Comput. Chem. 2020, 41, 2469–2484. [Google Scholar] [CrossRef]
- Balasubramanian, K. Computations of Colorings 7D-Hypercube’s Hyperplanes for All Irreducible Representations. J. Comput. Chem. 2020, 41, 653–686. [Google Scholar] [CrossRef]
- Balasubramanian, K. Relativistic double group spinor representations of nonrigid molecules. J. Chem. Phys. 2004, 120, 5524–5535. [Google Scholar] [CrossRef] [PubMed]
- Balasubramanian, K. Generalization of De Bruijn’s Extension of Pólya’s Theorem to all characters. J. Math. Chem. 1993, 14, 113–120. [Google Scholar] [CrossRef]
- Balasubramanian, K. Generalization of the Harary-Palmer Power Group Theorem to all Irreducible Representations. J. Math. Chem. 2014, 52, 703–728. [Google Scholar] [CrossRef]
- Balasubramanian, K. Enumeration of Internal-Rotation Reactions and Their Reaction Graphs. Theor. Chim. Acta 1979, 53, 129–146. [Google Scholar] [CrossRef]
- Wallace, R. Spontaneous symmetry breaking in a non-rigid molecule approach to intrinsically disordered proteins. Mol. BioSystems 2012, 8, 374–377. [Google Scholar] [CrossRef]
- Wallace, R. Tools for the Future: Hidden Symmetries. In Computational Psychiatry; A Systems Biology Approach to the Epigenetics of Mental Disorders; Springer: Cham, Switzerland, 2017; pp. 153–165. [Google Scholar] [CrossRef]
- Darafsheh, M.R.; Farjami, Y.; Ashrafi, A.R. Computing the Full Non-Rigid Group of Tetranitrocubane and Octanitrocubane Using Wreath Product. MATCH Commun. Math. Comput. Chem 2005, 54, 53. [Google Scholar]
- Foote, R.; Mirchandani, G.; Rockmore, D. A two-dimensional Wreath Product Transforms. J. Symb. Comput. 2004, 37, 187–207. [Google Scholar] [CrossRef]
- Balasubramanian, K. A Generalized Wreath Product Method for the Enumeration of Stereo and Position Isomers of Polysubstituted Organic Compounds. Theor. Chim. Acta. 1979, 51, 37–51. [Google Scholar] [CrossRef]
- Balasubramanian, K. Symmetry Simplifications of Space Types in Configuration-Interaction Induced by Orbital Degeneracy. Int. J. Quantum Chem. 1981, 20, 1255–1271. [Google Scholar] [CrossRef]
- Balasubramanian, K. Nested wreath groups and their applications to phylogeny in biology and Cayley trees in chemistry and physics. J. Math. Chem. 2017, 55, 195–222. [Google Scholar] [CrossRef]
- Nandini, G.K.; Rajan, R.S.; Shantrinal, A.A.; Rajalaxmi, T.M.; Rajasingh, I.; Balasubramanian, K. Topological and Thermodynamic Entropy Measures for COVID-19 Pandemic through Graph Theory. Symmetry 2020, 12, 1992. [Google Scholar] [CrossRef]
- Rousseau, R. On Certain Subgroups of a Wreath Product. Match 1982, 13, 3–6. [Google Scholar]
- Florek, W.; Lulek, T.; Mucha, M. Hyperoctahedral groups, wreath products, and a general Weyl’s recipe. Z. Für Krist.-Cryst. Mater. 1988, 184, 31–48. [Google Scholar] [CrossRef]
- Balasubramanian, K. Generators of the Character Tables of Generalized Wreath Product Groups. Theor. Chim. Acta 1990, 78, 31–43. [Google Scholar] [CrossRef]
- Liu, X.Y.; Balasubramanian, K. Computer Generation of Character Tables of Generalized Wreath Product Groups. J. Comput. Chem. 1990, 11, 589–602. [Google Scholar] [CrossRef]
- Balasubramanian, K. A Method for Nuclear-Spin Statistics in Molecular Spectroscopy. J. Chem. Phys. 1981, 74, 6824–6829. [Google Scholar] [CrossRef]
- Balasubramanian, K. Operator and algebraic methods for NMR spectroscopy. I. Generation of NMR spin species. J. Chem. Phys. 1983, 78, 6358–6368. [Google Scholar] [CrossRef]
- King, R.B.; Rouvray, D.H. The enumeration of electron-rich and electron-poor polyhedral clusters. Theoret. Chim. Acta 1978, 48, 207–214. [Google Scholar] [CrossRef]
- King, R.B. Topological aspects of chemically significant polyhedra. J. Math. Chem. 1991, 7, 51–68. [Google Scholar] [CrossRef]
- Pande, S.; Gong, X.; Wang, L.-S.; Zeng, X.C. Au60–: The Smallest Gold Cluster with the High-Symmetry Icosahedral Core Au13. J. Phys. Chem. Lett. 2019, 10, 1820–1827. [Google Scholar] [CrossRef]
- Zhan, S.Z.; Liu, Y.L.; Cai, H.; Li, M.D.; Huang, Q.; Wang, X.D.; Li, M.; Dang, L.; Ng, S.W.; Lu, W.; et al. Icosidodecahedral Coordination-Saturated Cuprofullerene. Angew. Chem. 2023, 135, e202312698. [Google Scholar] [CrossRef]
- Karfunkel, H.R.; Dressler, T.; Hirsch, A. Heterofullerenes: Structure and property predictions, possible uses and synthesis proposals. J. Comput.-Aided Mol. Des. 1992, 6, 521–535. [Google Scholar] [CrossRef]
- Thilgen, C.; Diederich, F. Structural aspects of fullerene chemistry a journey through fullerene chirality. Chem. Rev. 2006, 106, 5049–5135. [Google Scholar] [CrossRef] [PubMed]
- Hummelen, J.C.; Keshavarz-K, M.; van Dongen, J.L.; Janssen, R.A.; Meijer, E.W.; Wudl, F. Resolution and circular dichroism of an asymmetrically cage-opened [60] fullerene derivative. Chem. Commun. 1998, 281–282. [Google Scholar] [CrossRef]
- Hashikawa, Y.; Okamoto, S.; Murata, Y. Synthesis of inter-[60] fullerene conjugates with inherent chirality. Nat. Commun. 2024, 15, 514. [Google Scholar] [CrossRef]
- Coxeter, H.S.M. Regular Polytopes; Dover Publications: New York, NY, USA, 1973. [Google Scholar]
- Ruen, T. By Self-Own Work, Free Public Domain Work Available to Anyone to Use for Any Purpose. Available online: https://commons.wikimedia.org/w/index.php?curid=11743942 (accessed on 17 July 2024).
- Bandelow, C. Inside Rubik’s Cube and Beyond; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- User: Imk3nnyma, CC BY-SA 4.0. Available online: https://commons.wikimedia.org/w/index.php?curid=79057596 (accessed on 17 July 2024).
- Buck, D.K.; Collins, A.A. POV-RAY. Public Domain, CC BY-SA 3.0. Available online: https://en.wikipedia.org/wiki/User:Cyp/Poly.pov (accessed on 17 July 2024).
- Balasubramanian, K. Double group of the icosahedral group (Ih) and its application to fullerenes. Chem. Phys. Lett. 1996, 260, 476–484. [Google Scholar] [CrossRef]
- Kroto, H.W.; Heath, J.R.; O’Brien, S.C.; Curl, R.F.; Smalley, R.E. C60: Buckminsterfullerene. Nature 1985, 318, 162–163. [Google Scholar] [CrossRef]
- Kroto, H.W.; Heath, J.R.; O’Brien, S.C.; Curl, R.F.; Smalley, R.E. Long Carbon Chain Molecules in Circumstellar Shells. Astrophys. J. 1987, 314, 352–355. [Google Scholar] [CrossRef]
- Balasubramanian, K.; Liu, X.Y. Spectra and Characteristic Polynomials of Polyhedral Clusters. Int. J. Quantum Chem. 1988, 22, 319–328. [Google Scholar] [CrossRef]
- Jacob, K.; Clement, J.; Micheal Arockiaraj, M.; Peter, P.; Balasubramanian, K. Distance-based topology and entropy analysis of tetragonal farneseite zeolites. 2024; submitted for Publication. [Google Scholar]

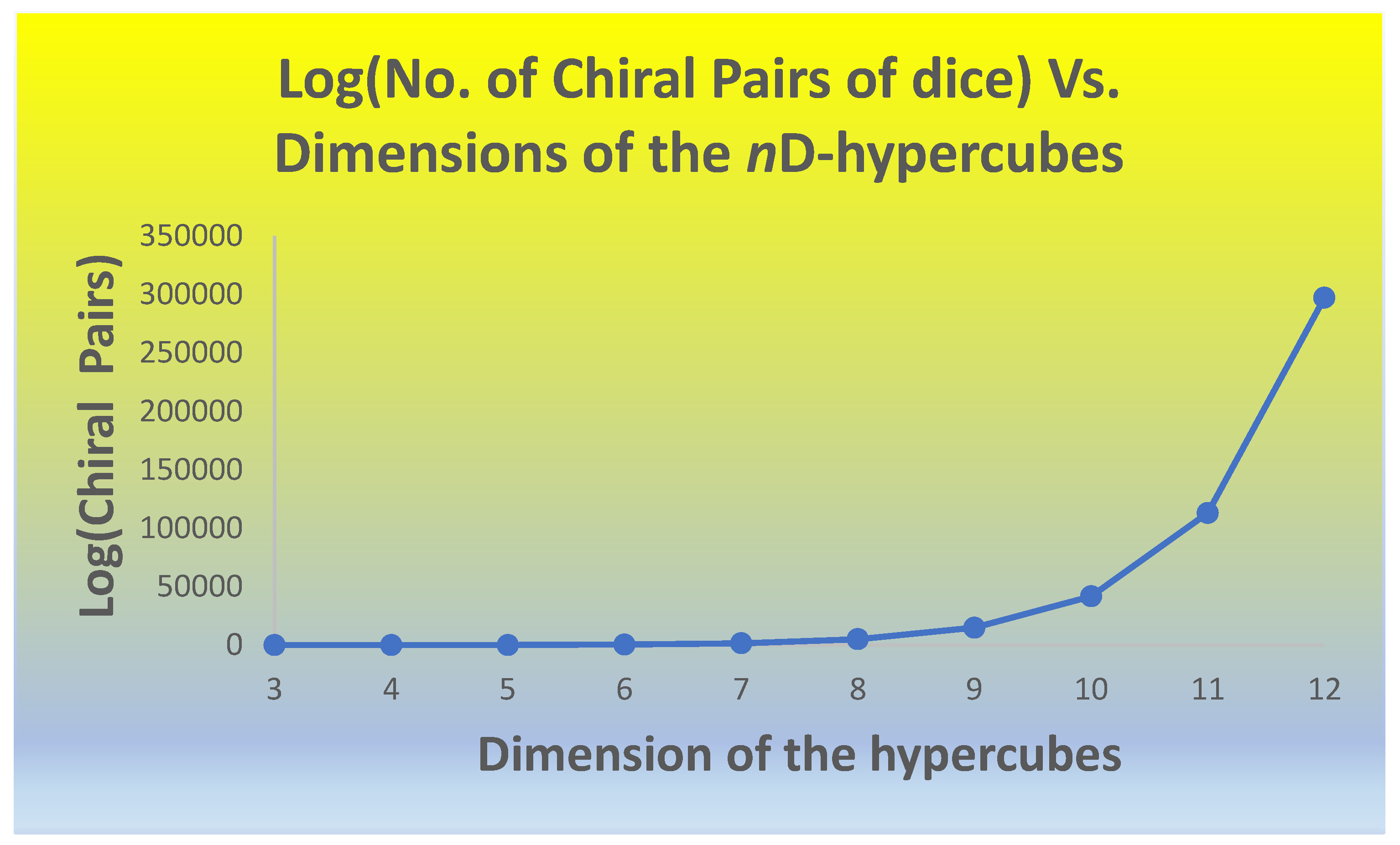

| n | Faces | No. of Dice | No. of Chiral Pairs of Dice |
|---|---|---|---|
| 3 | 6 | 30 | 15 |
| 4 | 24 | 3.23150209236062208 × 1021 | 1.61575104618031104 × 1021 |
| 5 | 80 | 3.7275758878262397028547673814159646 × 10115 | 1.8637879439131198514273836907079823 × 10115 |
| 6 | 240 | 1.7655752446384800870197812159231254 × 10464 | 8.827876223192400435098906079615627 × 10463 |
| 7 | 672 | 2.8214838544319294796427515741969896 × 101604 | 1.4107419272159647398213757870984948 × 101604 |
| 8 | 1792 | 1.7505019389666 × 105048 | 8.7525096948331 × 105047 |
| 9 | 4608 | 9.0935733829966 × 1014876 | 4.5467866914983 × 1014876 |
| 10 | 11,520 | 6.0441657269501 × 1041781 | 3.0220828634751 × 1041781 |
| 11 | 28,160 | 5.4587584881263 × 10113069 | 5.4587584881263 × 10113068 |
| 12 | 67,584 | 1.3084169531614 × 10297066 | 6.5420847658072 × 10297065 |
| Color Partition | No. of A1 Colorings a | No. of A3 Colorings a |
|---|---|---|
| 24 0 0 0 | 1 | 0 |
| 23 1 0 0 | 1 | 0 |
| 22 2 0 0 | 5 | 1 |
| 21 3 0 0 | 16 | 6 |
| 20 4 0 0 | 57 | 27 |
| 19 5 0 0 | 169 | 105 |
| 18 6 0 0 | 475 | 335 |
| 17 7 0 0 | 1099 | 866 |
| 16 8 0 0 | 2234 | 1849 |
| 15 9 0 0 | 3843 | 3307 |
| 14 10 0 0 | 5669 | 4967 |
| 13 11 0 0 | 7132 | 6336 |
| 12 12 0 0 | 7725 | 6871 |
| 22 1 1 0 | 5 | 1 |
| 21 2 1 0 | 32 | 13 |
| 20 3 1 0 | 158 | 97 |
| 19 4 1 0 | 688 | 503 |
| 18 5 1 0 | 2396 | 1973 |
| 17 6 1 0 | 6893 | 6025 |
| 16 7 1 0 | 16,303 | 14,810 |
| 15 8 1 0 | 32,156 | 29,818 |
| 14 9 1 0 | 53,118 | 49,918 |
| 13 10 1 0 | 74,020 | 70,054 |
| 12 11 1 0 | 87,278 | 82,892 |
| 20 2 2 0 | 244 | 145 |
| 19 3 2 0 | 1331 | 1021 |
| 18 4 2 0 | 5871 | 4986 |
| 17 5 2 0 | 20,208 | 18,285 |
| 16 6 2 0 | 56,090 | 52,312 |
| 15 7 2 0 | 126,548 | 120,340 |
| 14 8 2 0 | 235,721 | 226,411 |
| 13 9 2 0 | 365,096 | 353,006 |
| 12 10 2 0 | 473,741 | 459,377 |
| 11 11 2 0 | 516,370 | 501,374 |
| 18 3 3 0 | 7674 | 6682 |
| 17 4 3 0 | 33,276 | 30,591 |
| 16 5 3 0 | 110,825 | 105,097 |
| 15 6 3 0 | 292,629 | 281,871 |
| 14 7 3 0 | 623,256 | 606,096 |
| 13 8 3 0 | 1,087,220 | 1,062,760 |
| 12 9 3 0 | 1,567,505 | 1,536,897 |
| 11 10 3 0 | 1,879,494 | 1,845,178 |
| 16 4 4 0 | 138,453 | 131,493 |
| 15 5 4 0 | 437,694 | 423,510 |
| 14 6 4 0 | 1,087,993 | 1,062,443 |
| 13 7 4 0 | 2,168,246 | 2,129,446 |
| 12 8 4 0 | 3,517,231 | 3,464,261 |
| 11 9 4 0 | 4,684,708 | 4,622,058 |
| 10 10 4 0 | 5,152,084 | 5,085,022 |
| 14 5 5 0 | 1,303,756 | 1,275,752 |
| 13 6 5 0 | 3,031,508 | 2,983,480 |
| 12 7 5 0 | 5,618,270 | 5,548,102 |
| 11 8 5 0 | 8,418,614 | 8,328,046 |
| 10 9 5 0 | 10,284,396 | 10,182,022 |
| 12 6 6 0 | 6,553,122 | 6,474,210 |
| 11 7 6 0 | 11,217,872 | 11,108,824 |
| 10 8 6 0 | 15,415,306 | 15,281,078 |
| 9 9 6 0 | 17,123,720 | 16,981,820 |
| 10 7 7 0 | 17,611,556 | 17,467,588 |
| 9 8 7 0 | 22,006,070 | 21,840,340 |
| 8 8 8 0 | 24,753,462 | 24,573,093 |
| 21 1 1 1 | 51 | 22 |
| 20 2 1 1 | 425 | 281 |
| 19 3 1 1 | 2510 | 2015 |
| 18 4 1 1 | 11,325 | 9945 |
| 17 5 1 1 | 39,621 | 36,528 |
| 16 6 1 1 | 110,649 | 104,649 |
| 15 7 1 1 | 250,736 | 240,748 |
| 14 8 1 1 | 467,930 | 453,070 |
| 13 9 1 1 | 725,780 | 706,350 |
| 12 10 1 1 | 942,226 | 919,270 |
| 11 11 1 1 | 1,027,332 | 1,003,242 |
| 19 2 2 1 | 3756 | 3061 |
| 18 3 2 1 | 22,360 | 20,105 |
| 17 4 2 1 | 98,101 | 92,141 |
| 16 5 2 1 | 329,008 | 316,265 |
| 15 6 2 1 | 871,348 | 847,760 |
| 14 7 2 1 | 1,859,676 | 1,822,056 |
| 13 8 2 1 | 3,247,280 | 3,194,050 |
| 12 9 2 1 | 4,684,626 | 4,618,066 |
| 11 10 2 1 | 5,618,508 | 5,544,042 |
| 17 3 3 1 | 130,170 | 123,075 |
| 16 4 3 1 | 546,260 | 528,355 |
| 15 5 3 1 | 1,736,268 | 1,699,440 |
| 14 6 3 1 | 4,325,880 | 4,260,540 |
| 13 7 3 1 | 8,634,420 | 8,534,760 |
| 12 8 3 1 | 14,016,130 | 13,881,320 |
| 11 9 3 1 | 18,677,240 | 18,517,190 |
| 10 10 3 1 | 20,541,952 | 20,371,606 |
| 15 4 4 1 | 2,169,038 | 2,125,858 |
| 14 5 4 1 | 6,481,828 | 6,396,668 |
| 13 6 4 1 | 15,094,180 | 14,950,100 |
| 12 7 4 1 | 28,000,166 | 27,789,946 |
| 11 8 4 1 | 41,975,340 | 41,705,670 |
| 10 9 4 1 | 51,289,438 | 50,984,818 |
| 13 5 5 1 | 18,105,486 | 17,944,878 |
| 12 6 5 1 | 39,180,892 | 38,920,964 |
| 11 7 5 1 | 67,120,260 | 66,760,032 |
| 10 8 5 1 | 92,260,524 | 91,821,066 |
| 9 9 5 1 | 102,499,670 | 102,032,840 |
| 11 6 6 1 | 78,296,512 | 77,897,372 |
| 10 7 6 1 | 122,981,972 | 122,455,864 |
| 9 8 6 1 | 153,698,290 | 153,094,910 |
| 9 7 7 1 | 175,633,880 | 174,981,580 |
| 8 8 7 1 | 197,572,890 | 196,868,820 |
| 18 2 2 2 | 33,487 | 30,322 |
| 17 3 2 2 | 194,912 | 185,257 |
| 16 4 2 2 | 818,481 | 794,136 |
| 15 5 2 2 | 2,602,172 | 2,552,704 |
| 14 6 2 2 | 6,484,766 | 6,397,066 |
| 13 7 2 2 | 12,945,012 | 12,811,992 |
| 12 8 2 2 | 21,015,167 | 20,835,247 |
| 11 9 2 2 | 28,004,836 | 27,791,786 |
| 10 10 2 2 | 30,801,270 | 30,574,296 |
| 16 3 3 2 | 1,088,655 | 1,059,945 |
| 15 4 3 2 | 4,328,656 | 4,259,696 |
| 14 5 3 2 | 12,944,976 | 12,809,676 |
| 13 6 3 2 | 30,156,640 | 29,928,140 |
| 12 7 3 2 | 55,953,912 | 55,621,272 |
| 11 8 3 2 | 83,891,030 | 83,464,690 |
| 10 9 3 2 | 102,511,416 | 102,030,166 |
| 14 4 4 2 | 16,176,465 | 16,017,675 |
| 13 5 4 2 | 45,209,956 | 44,913,056 |
| 12 6 4 2 | 97,864,801 | 97,385,171 |
| 11 7 4 2 | 167,678,736 | 167,016,576 |
| 10 8 4 2 | 230,502,306 | 229,694,856 |
| 9 9 4 2 | 256,090,630 | 255,234,030 |
| 12 5 5 2 | 117,411,604 | 116,879,096 |
| 11 6 5 2 | 234,687,752 | 233,871,724 |
| 10 7 5 2 | 368,679,660 | 367,606,632 |
| 9 8 5 2 | 460,789,280 | 459,559,450 |
| 10 6 6 2 | 430,095,214 | 428,905,586 |
| 9 7 6 2 | 614,294,660 | 612,823,960 |
| 8 8 6 2 | 691,047,030 | 689,459,130 |
| 8 7 7 2 | 789,709,860 | 787,996,500 |
| 15 3 3 3 | 5,765,010 | 5,682,726 |
| 14 4 3 3 | 21,553,380 | 21,364,620 |
| 13 5 3 3 | 60,252,960 | 59,898,540 |
| 12 6 3 3 | 130,442,733 | 129,871,167 |
| 11 7 3 3 | 223,512,780 | 222,722,100 |
| 10 8 3 3 | 307,264,170 | 306,301,230 |
| 9 9 3 3 | 341,378,584 | 340,355,686 |
| 13 4 4 3 | 75,302,150 | 74,889,430 |
| 12 5 4 3 | 195,601,766 | 194,861,566 |
| 11 6 4 3 | 391,016,740 | 389,884,220 |
| 10 7 4 3 | 614,296,776 | 612,807,816 |
| 9 8 4 3 | 767,787,880 | 766,082,150 |
| 11 5 5 3 | 469,162,776 | 467,900,988 |
| 10 6 5 3 | 859,878,528 | 858,043,860 |
| 9 7 5 3 | 1,228,207,740 | 1,225,936,680 |
| 8 8 5 3 | 1,381,679,430 | 1,379,231,310 |
| 9 6 6 3 | 1,432,840,398 | 1,430,330,262 |
| 8 7 6 3 | 1,842,059,400 | 1,839,135,600 |
| 7 7 7 3 | 2,105,109,000 | 2,101,948,920 |
| 12 4 4 4 | 244,472,700 | 243,610,770 |
| 11 5 4 4 | 586,400,958 | 584,936,118 |
| 10 6 4 4 | 1,074,771,633 | 1,072,641,603 |
| 9 7 4 4 | 1,535,163,270 | 1,532,530,170 |
| 8 8 4 4 | 1,726,995,915 | 1,724,156,055 |
| 10 5 5 4 | 1,289,616,636 | 1,287,246,912 |
| 9 6 5 4 | 2,148,984,460 | 2,145,744,020 |
| 8 7 5 4 | 2,762,767,590 | 2,758,994,670 |
| 8 6 6 4 | 3,223,114,485 | 3,218,943,735 |
| 7 7 6 4 | 3,683,411,640 | 3,678,907,800 |
| 9 5 5 5 | 2,578,619,766 | 2,575,009,806 |
| 8 6 5 5 | 3,867,527,364 | 3,862,884,864 |
| 7 7 5 5 | 4,419,870,336 | 4,414,853,016 |
| 7 6 6 5 | 5,156,360,952 | 5,150,820,912 |
| 6 6 6 6 | 6,015,584,844 | 6,009,464,868 |
| nb | N(A1) |
|---|---|
| 0 | 1 |
| 1 | 1 |
| 2 | 11 |
| 3 | 139 |
| 4 | 4176 |
| 5 | 152635 |
| 6 | 5580266 |
| 7 | 182586993 |
| 8 | 5283117184 |
| 9 | 135891832431 |
| 10 | 3136801139463 |
| 11 | 65570741043751 |
| 12 | 1251192201334018 |
| 13 | 21943233858075034 |
| 14 | 355789263949855043 |
| 15 | 5360531557936453701 |
| 16 | 75382327861202736302 |
| 17 | 993272251366379046339 |
| 18 | 12305535660981459650639 |
| 19 | 143780450498062303705832 |
| 20 | 1588773890867771345864514 |
| 21 | 16644297515678879808798297 |
| 22 | 165686414507591622101552686 |
| 23 | 1570419052306115065132618723 |
| 24 | 14199205570066871577428871140 |
| 25 | 122681136017010754158309432703 |
| 26 | 1014478624347489084027884140426 |
| 27 | 8040682428518284564435181330429 |
| 28 | 61166619897340434689394786752894 |
| 29 | 447149083369066948751292268832796 |
| 30 | 3144948552967196406093283337735784 |
| 31 | 21304490197316629654279247580024508 |
| 32 | 139144951600574592746649080159671056 |
| 33 | 877034846450078628652128896100267264 |
| 34 | 5339594506322238375552079147434465280 |
| 35 | 31427327665763904958336467271605932032 |
| 36 | 178961171429990835258310915317808332800 |
| 37 | 986704837073310196335657862006172680192 |
| 38 | 5271081103312257287838648872443980546048 |
| 39 | 27301496996641809668910837171761665015808 |
| 40 | 137190022408121978237796878411228575170560 |
| 41 | 669219621503025866250112350741134231732224 |
| 42 | 3170826301883363428193286508672704248283136 |
| 43 | 14600549017974504078101835470726548970012672 |
| 44 | 65370639921385716211126737122035700519141376 |
| 45 | 284725453879813021523275240995846178515976192 |
| 46 | 1206988337099206608564703950062943154911313920 |
| 47 | 4982036965898851012606659737835556794489896960 |
| 48 | 20031940300384959298052361807805291335079952384 |
| 49 | 78492500768855341303876241965943810733935427584 |
| 50 | 299841352937027382765902773445578163675698561024 |
| 51 | 1117056020745788242590376169881100544777740353536 |
| 52 | 4060068998479884089705015623755515419238105874432 |
| 53 | 14401754183287135804246166953385408335748576313344 |
| 54 | 49872741338420266137322529195039577022891227611136 |
| 55 | 168660543435384899112410284701246690287995790032896 |
| 56 | 557182152420467968422421922547752056414106468483072 |
| 57 | 1798623088515194841712775021084035912510703835021312 |
| 58 | 5674965951694494062205410635512839112833661296181248 |
| 59 | 17505827173023693533563297554260934909613741294223360 |
| 60 | 52809245305288142132272869107153182169520534320054272 |
| 61 | 155830559917243698043653761141737793516691944388952064 |
| 62 | 449897906857848741029976049141268839915375321375834112 |
| 63 | 1271140117788842474479687792473475435071770769766744064 |
| 64 | 3515496888259767468163216556014840184143394537746726912 |
| 65 | 9518883882057216528608998367914143748380503439084355584 |
| 66 | 25239464838788074127879708050806830413286976339971145728 |
| 67 | 65547266894763058181097740456058221177846743153120378880 |
| 68 | 166759958423441309781250299599634334821117425640231927808 |
| 69 | 415691490562781235971341064888171821804599513259251335168 |
| 70 | 1015474926946222733578581339228367257143428701318056771584 |
| 71 | 2431418839167012178976836276667886688093053146703593472000 |
| 72 | 5707080330822570253408677742000332960960832242019794419712 |
| 73 | 13134102679153312363971846877730573818996158520436289699840 |
| 74 | 29640474965116258983498939796906579169557606545381706956800 |
| 75 | 65604251256123986550051347634863006077799034966669772455936 |
| 76 | 142430282332374444483519754796895120849199732824551463059456 |
| 77 | 303358003928693622016625857618977262242204533973550601601024 |
| 78 | 633940444107398210111331355025827016639517714599813255790592 |
| 79 | 1299979138549348228329063291451252319874543714414110345003008 |
| 80 | 2616208016330563309511496917583731993831663122674685994598400 |
| 81 | 5167818303862841105206809240136218974660614845481150429790208 |
| 82 | 10020525735538923606436025159797456261476584970542687191040000 |
| 83 | 19075217665242770238755496178613251023787049655038318777204736 |
| 84 | 35652490160037082470051758173077553030081498177735400332722176 |
| 85 | 65432805470185704297972943306063050464253178396461240116314112 |
| 86 | 117931219161381211234712613738006405558219054813035950984134656 |
| 87 | 208751813228192029082126716097532138898293814638576077423771648 |
| 88 | 362943493453561141472322840478800602327652562685480290771533824 |
| 89 | 619858550617317904536986031056340852095924421733185407357550592 |
| 90 | 1039984901591277817612034918976099698126465664356715106718973952 |
| 91 | 1714260826798809589470361996501267583138843705609748724712472576 |
| 92 | 2776357208619811182946532139254981696976848855712642666273439744 |
| 93 | 4418288891136903818022397130640910763680489058711583357765943296 |
| 94 | 6909451776565158098396675870686568083843531086171523326916165632 |
| 95 | 10618736414510664024904301130552081477721498205603021838987821056 |
| 96 | 16038716459417148787615793261697627570544461777441421000234237952 |
| 97 | 23810053300578035313573866471631103862233086767820943288313053184 |
| 98 | 34743241040639378059602569562006314113107754712353068755277840384 |
| 99 | 49833739674452441257207592441071504972429917631360631482888486912 |
| 100 | 70265572940977942172662549542368590308742746503025565569864695808 |
| 101 | 97397823878583286179928107075659666820641201605644990708709326848 |
| 102 | 132728407050226242931470451731079405476392486433062572665908232192 |
| 103 | 177830292941079820626630085054867470806112050016763218951307001856 |
| 104 | 234257212816614763710079762418248667308011746087552551552313982976 |
| 105 | 303418866124377217757817320211010493225990120075604352544075153408 |
| 106 | 386429687988593626389672704093941308509024324478124575046739427328 |
| 107 | 483939983088519120899215967419979205493870806825180030389467480064 |
| 108 | 595963127321972621107367481426572082532281766779222667980204670976 |
| 109 | 721716814738535651249288720668083207973311214195686476972126371840 |
| 110 | 859499115734074275578698042830041010298057135266173370496370868224 |
| 111 | 1006620585994861764191267638613753417255640297161311448121542180864 |
| 112 | 1159411210654796139113156147343784043908458958128856461986303574016 |
| 113 | 1313315353662069962889238509921566661821965008467180176968339423232 |
| 114 | 1463079385220025309534502263293078969347233893342480581579761516544 |
| 115 | 1603026109023679904359541354461619193561740429737992388679403831296 |
| 116 | 1727398824378965414180540034621696361312587920268799182661767659520 |
| 117 | 1830747471991382148362281571140508950236269928271495714066772525056 |
| 118 | 1908321517414745798716615408358714803655391352966327587613939073024 |
| 119 | 1956430463231924264230479584872704778184154074936145924234846142464 |
| 120 | 1972734050425523633099066888879877060171554196485690791403223777280 |
 Truncated octahedron: 604,800 chiral pairs of dice | 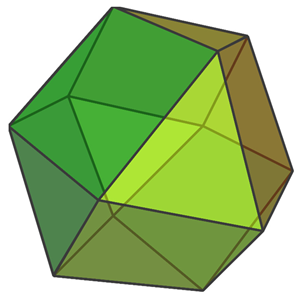 Cuboctahedron (isochiral with truncated octahedron): 604,800 chiral pairs of dice |
 Rhombicdodecahedron: 1,816,214,400 chiral pairs of dice | 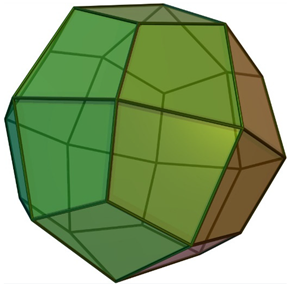 Deltoidal icositetrahedron: 133,382,785,536,000 chiral pairs of dice |
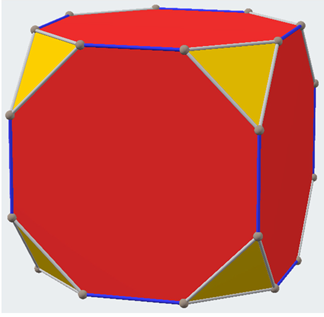 Truncated cube: 6!8!/48 604,800 chiral pairs of dice, isochiral with truncated octahedron |  Rhombic cuboctahedron: 6! 12! 8!/48 289,700,167,680,000 chiral pairs of dice |
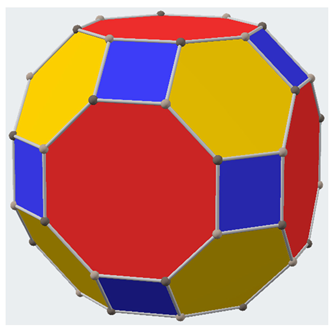 Truncated cuboctahedron: 289,700,167,680,000 chiral pairs of dice, isochiral with rhombic cuboctahedron | 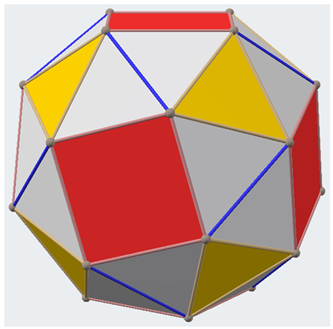 Sunb cube (chiral): 7.8939251080108059050165403648 × 1036 |
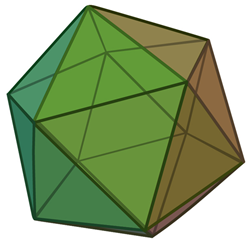 Icosahedron: 20,274,183,401,472,000 chiral pairs of dice | 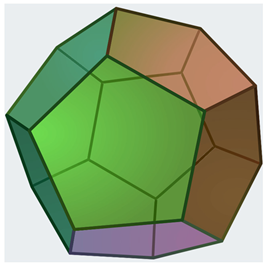 Dodecahedron: 3,991,680 chiral pairs of dice |
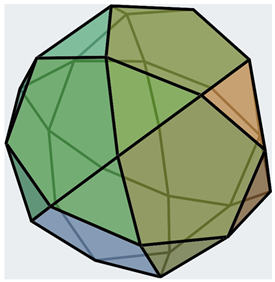 Icosododecahedron: 9.7113662879985303552 × 1024 chiral pairs of dice | 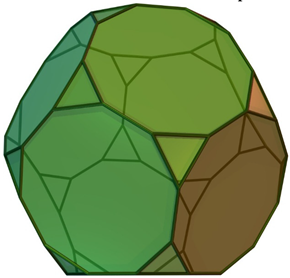 Truncated dodecahedron: 9.7113662879985303552 × 1024 chiral pairs of dice |
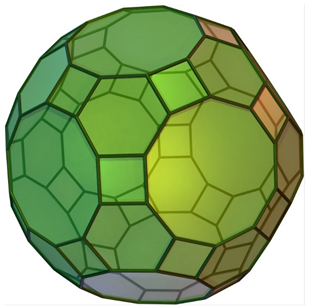 Truncated icosodedecahedron (archimedene: C120) 2.5759676805753124307695693098745908 × 1057 chiral pairs of dice |  Rhombicosidodecahedron: 2.5759676805753124307695693098745908 × 1057 chiral pairs of dice |
 Rhombic triacontahedron: 2.210440498434925488635904 × 1030 chiral pairs of dice | 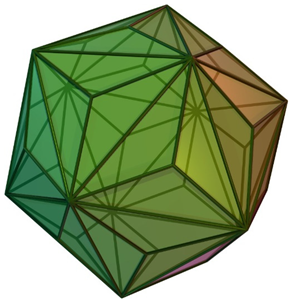 Triakis icosahedron: 6.93415592728449178689695098601947 × 1079 chiral pairs of dice |
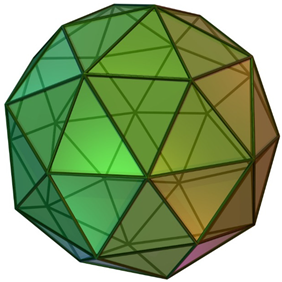 Pentakis dodecahedron: 6.93415592728449178689695098601947 × 1079 chiral pairs of dice | 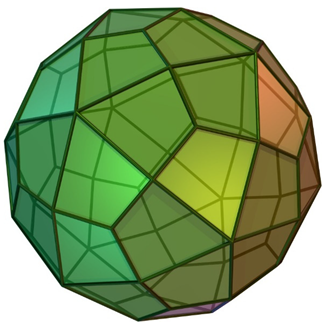 Deltoidal hexecontahedron: 6.93415592728449178689695098601947 × 1079 chiral pairs of dice |
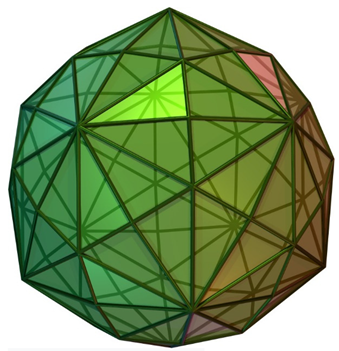 Disdyakis triacontahedron: 5.574585761207605881323431711741975 × 10196 chiral pairs of dice | 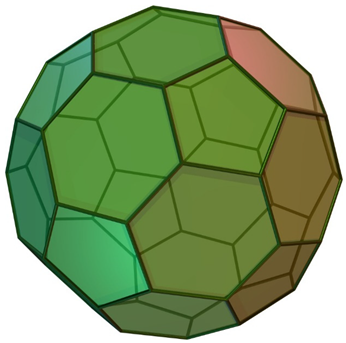 Truncated icosahedron: buckminsterfullerene (C60) 9.7113662879985303552 × 1024 chiral pairs of dice |
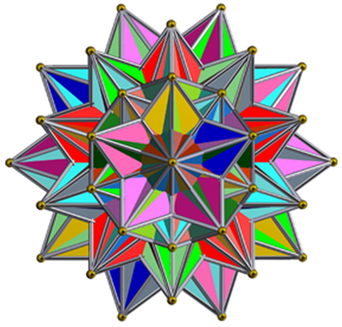 Grand 600-cell/grand polytetrahedron: 4.4102702121446021496460288562760144 × 103171 chiral pairs of dice |
| Hexagons | Pentagons | ||||
|---|---|---|---|---|---|
| Color Partition | Ag | Au | Color Partition | Ag | Au |
| 20 0 0 0 0 0 | 1 | 0 | 12 0 0 0 0 0 0 0 0 0 | 1 | 0 |
| 19 1 0 0 0 0 | 1 | 0 | 11 1 0 0 0 0 0 0 0 0 | 1 | 0 |
| 18 2 0 0 0 0 | 5 | 1 | 10 2 0 0 0 0 0 0 0 0 | 3 | 0 |
| 17 3 0 0 0 0 | 5 | 2 | 9 3 0 0 0 0 0 0 0 0 | 3 | 0 |
| 16 4 0 0 0 0 | 15 | 6 | 8 4 0 0 0 0 0 0 0 0 | 5 | 0 |
| 18 1 1 0 0 0 | 34 | 23 | 10 1 1 0 0 0 0 0 0 0 | 9 | 2 |
| 17 2 1 0 0 0 | 60 | 54 | 9 2 1 0 0 0 0 0 0 0 | 14 | 8 |
| 16 3 1 0 0 0 | 58 | 38 | 8 3 1 0 0 0 0 0 0 0 | 10 | 2 |
| 16 2 2 0 0 0 | 176 | 151 | 8 2 2 0 0 0 0 0 0 0 | 23 | 10 |
| 17 1 1 1 0 0 | 274 | 233 | 9 1 1 1 0 0 0 0 0 0 | 37 | 20 |
| 16 2 1 1 0 0 | 498 | 471 | 8 2 1 1 0 0 0 0 0 0 | 57 | 42 |
| 16 1 1 1 1 0 | 972 | 966 | 8 1 1 l 1 1 0 0 0 0 0 | 102 | 96 |
| 15 5 0 0 0 0 | 149 | 113 | 7 5 0 0 0 0 0 0 0 0 | 12 | 2 |
| 14 6 0 0 0 0 | 674 | 622 | 6 6 0 0 0 0 0 0 0 0 | 42 | 24 |
| 15 4 1 0 0 0 | 1337 | 1249 | 7 4 1 0 0 0 0 0 0 0 | 80 | 52 |
| 14 5 1 0 0 0 | 2610 | 2562 | 6 5 1 0 0 0 0 0 0 0 | 144 | 120 |
| 15 3 2 0 0 0 | 3928 | 3824 | 7 3 2 0 0 0 0 0 0 0 | 216 | 180 |
| 14 4 2 0 0 0 | 7776 | 7728 | 6 4 2 0 0 0 0 0 0 0 | 408 | 384 |
| 14 3 3 0 0 0 | 15,504 | 15,504 | 6 3 3 0 0 0 0 0 0 0 | 792 | 792 |
| 15 3 1 1 0 0 | 371 | 310 | 7 3 1 1 0 0 0 0 0 0 | 18 | 6 |
| 14 4 1 1 0 0 | 1984 | 1892 | 6 4 1 1 0 0 0 0 0 0 | 58 | 36 |
| 15 2 2 1 0 0 | 4984 | 4796 | 7 2 2 1 0 0 0 0 0 0 | 142 | 104 |
| 14 3 2 1 0 0 | 9744 | 9636 | 6 3 2 1 0 0 0 0 0 0 | 246 | 216 |
| 14 2 2 2 0 0 | 6557 | 6373 | 6 2 2 2 0 0 0 0 0 0 | 178 | 134 |
| 15 2 1 1 1 0 | 19,480 | 19,280 | 7 2 1 1 1 0 0 0 0 0 | 488 | 436 |
| 14 3 1 1 1 0 | 38,784 | 38,736 | 6 3 1 1 1 0 0 0 0 0 | 936 | 912 |
| 14 2 2 1 1 0 | 29,352 | 28,968 | 6 2 2 1 1 0 0 0 0 0 | 748 | 668 |
| 14 2 1 1 1 1 | 58,248 | 58,032 | 6 2 1 1 1 1 0 0 0 0 | 1416 | 1356 |
| 13 7 0 0 0 0 | 116,304 | 116,256 | 5 5 2 0 0 0 0 0 0 0 | 2784 | 2760 |
| 12 8 0 0 0 0 | 693 | 609 | 5 4 3 0 0 0 0 0 0 0 | 5544 | 5544 |
| 13 6 1 0 0 0 | 4597 | 4457 | 4 4 4 0 0 0 0 0 0 0 | 160 | 118 |
| 12 7 1 0 0 0 | 13,720 | 13,412 | 5 5 1 1 0 0 0 0 0 0 | 296 | 260 |
| 13 5 2 0 0 0 | 27,216 | 27,048 | 5 4 2 1 0 0 0 0 0 0 | 258 | 204 |
| 12 6 2 0 0 0 | 22,802 | 22,438 | 4 4 3 1 0 0 0 0 0 0 | 726 | 660 |
| 13 4 3 0 0 0 | 68,040 | 67,620 | 4 4 2 2 0 0 0 0 0 0 | 1404 | 1368 |
| 12 5 3 0 0 0 | 135,744 | 135,576 | 5 4 1 1 1 0 0 0 0 0 | 960 | 888 |
| 12 4 4 0 0 0 | 90,618 | 90,282 | 4 4 2 1 1 0 0 0 0 0 | 1440 | 1332 |
| 13 5 1 1 0 0 | 136,024 | 135,296 | 4 4 1 1 1 1 0 0 0 0 | 2808 | 2736 |
| 12 6 1 1 0 0 | 271,488 | 271,152 | 5 3 3 1 0 0 0 0 0 0 | 5544 | 5544 |
| 13 4 2 1 0 0 | 542,640 | 542,640 | 5 3 2 2 0 0 0 0 0 0 | 4224 | 4092 |
| 12 5 2 1 0 0 | 407,400 | 406,560 | 4 3 3 2 0 0 0 0 0 0 | 8352 | 8280 |
| 12 4 3 1 0 0 | 814,128 | 813,792 | 5 3 2 1 1 0 0 0 0 0 | 16,632 | 16,632 |
| 12 4 2 2 0 0 | 1135 | 1022 | 4 3 3 1 1 0 0 0 0 0 | 33,264 | 33,264 |
| 13 4 1 1 1 0 | 8501 | 8305 | 5 2 2 2 1 0 0 0 0 0 | 330 | 270 |
| 12 5 1 1 1 0 | 29,739 | 29,262 | 4 3 2 2 1 0 0 0 0 0 | 1194 | 1116 |
| 12 4 2 1 1 0 | 58,917 | 58,665 | 4 2 2 2 2 0 0 0 0 0 | 1818 | 1692 |
| 12 4 1 1 1 1 | 59,085 | 58,497 | 5 2 2 1 1 1 0 0 0 0 | 3510 | 3420 |
| 11 9 0 0 0 0 | 176,680 | 176,036 | 4 3 2 1 1 1 0 0 0 0 | 6948 | 6912 |
| 10 10 0 0 0 0 | 352,800 | 352,632 | 4 2 2 2 1 1 0 0 0 0 | 2376 | 2244 |
| 11 8 1 0 0 0 | 74,014 | 73,286 | 4 2 2 1 1 1 1 0 0 0 | 4656 | 4584 |
| 10 9 1 0 0 0 | 294,290 | 293,590 | 3 3 3 3 0 0 0 0 0 0 | 7008 | 6852 |
| 11 7 2 0 0 0 | 441,952 | 440,468 | 3 3 3 2 1 0 0 0 0 0 | 13,896 | 13,824 |
| 10 8 2 0 0 0 | 882,168 | 881,412 | 3 3 2 2 2 0 0 0 0 0 | 27,720 | 27,720 |
| 11 6 3 0 0 0 | 1,763,664 | 1,763,496 | 3 3 2 2 1 1 0 0 0 0 | 10,572 | 10,308 |
| 10 7 3 0 0 0 | 588,509 | 587,221 | 3 2 2 2 2 1 0 0 0 0 | 20,880 | 20,700 |
| 10 6 4 0 0 0 | 1,175,898 | 1,175,562 | 2 2 2 2 2 2 0 0 0 0 | 41,616 | 41,544 |
| 11 7 1 1 0 0 | 1,764,280 | 1,762,880 | 3 2 2 2 1 1 1 0 0 0 | 83,160 | 83,160 |
| 10 8 1 1 0 0 | 3,527,328 | 3,526,992 | 2 2 2 2 2 1 1 0 0 0 | 166,320 | 166,320 |
| 11 6 2 1 0 0 | 2,647,512 | 2,644,488 | 2 2 2 2 1 1 1 1 0 0 | 3156 | 3012 |
| 10 7 2 1 0 0 | 5,291,496 | 5,289,984 | 2 2 2 2 2 2 0 0 0 0 | 9312 | 9168 |
| 10 6 3 1 0 0 | 1466 | 1340 | 3 3 3 1 1 1 0 0 0 0 | 18,480 | 18,480 |
| 10 6 2 2 0 0 | 12,716 | 12,478 | 3 3 2 2 2 0 0 0 0 0 | 13,992 | 13,728 |
| 11 6 1 1 1 0 | 50,696 | 50,080 | 3 3 2 2 1 1 0 0 0 0 | 27,792 | 27,648 |
| 10 7 1 1 1 0 | 100,944 | 100,608 | 3 3 2 1 1 1 1 0 0 0 | 55,440 | 55,440 |
| 10 6 2 1 1 0 | 118,002 | 117,162 | 3 3 1 1 1 1 1 1 0 0 | 110,880 | 110,880 |
| 10 6 1 1 1 1 | 353,192 | 352,240 | 3 2 2 2 2 1 0 0 0 0 | 41,736 | 41,424 |
| 9 9 2 0 0 0 | 705,600 | 705,264 | 3 2 2 2 1 1 1 0 0 0 | 83,232 | 83,088 |
| 9 8 3 0 0 0 | 176,904 | 175,812 | 3 2 2 1 1 1 1 1 0 0 | 166,320 | 166,320 |
| 8 8 4 0 0 0 | 705,936 | 704,928 | 3 2 1 1 1 1 1 1 1 0 | 332,640 | 332,640 |
| 9 9 1 1 0 0 | 1,059,240 | 1,057,056 | 3 1 1 1 1 1 1 1 1 1 | 665,280 | 665,280 |
| 9 8 2 1 0 0 | 2,116,800 | 2,115,792 | 2 2 2 2 2 2 0 0 0 0 | 62,736 | 62,184 |
| 8 8 3 1 0 0 | 4,232,592 | 4,232,592 | 2 2 2 2 2 1 1 0 0 0 | 124,920 | 124,560 |
| 8 8 2 2 0 0 | 882,504 | 881,076 | 2 2 2 2 1 1 1 1 0 0 | 249,552 | 249,408 |
| 9 8 1 1 1 0 | 1,764,840 | 1,762,320 | 2 2 2 1 1 1 1 1 1 0 | 498,960 | 498,960 |
| 8 8 2 1 1 0 | 3,527,664 | 3,526,656 | 2 2 1 1 1 1 1 1 1 1 | 997,920 | 997,920 |
| 8 8 1 1 1 1 | 5,292,168 | 5,289,312 | |||
| 13 3 3 1 0 0 | 10,581,984 | 10,580,976 | |||
| 13 3 2 2 0 0 | 2,352,468 | 2,350,452 | |||
| 12 3 3 2 0 0 | 7,055,328 | 7,053,312 | |||
| 13 3 2 1 1 0 | 14,108,640 | 14,108,640 | |||
| 12 3 3 1 1 0 | 10,584,000 | 10,578,960 | |||
| 13 2 2 2 1 0 | 21,163,968 | 21,161,952 | |||
| 12 3 2 2 1 0 | 31,747,296 | 31,741,584 | |||
| 12 2 2 2 2 0 | 1648 | 1510 | |||
| 13 2 2 1 1 1 | 15,536 | 15,270 | |||
| 12 3 2 1 1 1 | 69,812 | 69,070 | |||
| 12 2 2 2 1 1 | 138,756 | 138,378 | |||
| 11 5 4 0 0 0 | 185,308 | 184,244 | |||
| 10 5 5 0 0 0 | 554,856 | 553,680 | |||
| 11 5 3 1 0 0 | 1,108,704 | 1,108,368 | |||
| 11 4 4 1 0 0 | 324,428 | 322,888 | |||
| 10 5 4 1 0 0 | 1,294,012 | 1,292,612 | |||
| 11 5 2 2 0 0 | 1,942,136 | 1,939,000 | |||
| 11 4 3 2 0 0 | 3,880,632 | 3,879,120 | |||
| 10 5 3 2 0 0 | 7,759,920 | 7,759,584 | |||
| 10 4 4 2 0 0 | 388,788 | 387,192 | |||
| 10 4 3 3 0 0 | 1,940,904 | 1,938,972 | |||
| 11 5 2 1 1 0 | 3,881,640 | 3,878,112 | |||
| 11 4 3 1 1 0 | 7,760,256 | 7,759,248 | |||
| 10 5 3 1 1 0 | 11,641,560 | 11,637,696 | |||
| 10 4 4 1 1 0 | 23,279,760 | 23,278,752 | |||
| 11 4 2 2 1 0 | 4,853,184 | 4,848,396 | |||
| 10 5 2 2 1 0 | 9,700,824 | 9,698,556 | |||
| 10 4 3 2 1 0 | 6,468,432 | 6,464,568 | |||
| 10 4 2 2 2 0 | 19,401,480 | 19,397,280 | |||
| 11 4 2 1 1 1 | 38,799,264 | 38,798,256 | |||
| 10 5 2 1 1 1 | 29,105,832 | 29,096,088 | |||
| 10 4 3 1 1 1 | 58,200,408 | 58,195,872 | |||
| 10 4 2 2 1 1 | 25,866,888 | 25,864,872 | |||
| 9 7 4 0 0 0 | 38,802,624 | 38,794,896 | |||
| 9 6 5 0 0 0 | 77,598,528 | 77,596,512 | |||
| 8 7 5 0 0 0 | 116,400,480 | 116,392,080 | |||
| 8 6 6 0 0 0 | 174,608,112 | 174,588,288 | |||
| 9 7 3 1 0 0 | 77,370 | 76,600 | |||
| 9 6 4 1 0 0 | 154,180 | 153,760 | |||
| 8 7 4 1 0 0 | 231,550 | 230,360 | |||
| 8 6 5 1 0 0 | 693,500 | 692,170 | |||
| 9 7 2 2 0 0 | 1,385,880 | 1,385,460 | |||
| 9 6 3 2 0 0 | 462,820 | 461,000 | |||
| 8 7 3 2 0 0 | 1,848,420 | 1,846,740 | |||
| 8 6 4 2 0 0 | 2,773,160 | 2,769,520 | |||
| 8 6 3 3 0 0 | 5,543,520 | 5,541,840 | |||
| 9 7 2 1 1 0 | 11,085,360 | 11,085,360 | |||
| 9 6 3 1 1 0 | 647,706 | 645,606 | |||
| 8 7 3 1 1 0 | 3,234,580 | 3,231,920 | |||
| 8 6 4 1 1 0 | 6,468,850 | 6,464,090 | |||
| 9 6 2 2 1 0 | 12,933,780 | 12,932,100 | |||
| 8 7 2 2 1 0 | 19,402,040 | 19,396,720 | |||
| 8 6 3 2 1 0 | 38,799,600 | 38,797,920 | |||
| 8 6 2 2 2 0 | 3,881,136 | 3,878,616 | |||
| 9 6 2 1 1 1 | 9,702,840 | 9,696,540 | |||
| 8 7 2 1 1 1 | 19,400,640 | 19,398,120 | |||
| 8 6 3 1 1 1 | 12,935,460 | 12,930,420 | |||
| 8 6 2 2 1 1 | 38,801,280 | 38,796,240 | |||
| 9 5 5 1 0 0 | 77,597,520 | 77,597,520 | |||
| 9 5 4 2 0 0 | 58,204,440 | 58,191,840 | |||
| 8 5 5 2 0 0 | 116,398,800 | 116,393,760 | |||
| 9 4 4 3 0 0 | 16,169,760 | 16,162,620 | |||
| 8 5 4 3 0 0 | 48,502,440 | 48,494,460 | |||
| 8 4 4 4 0 0 | 96,998,160 | 96,995,640 | |||
| 9 5 4 1 1 0 | 64,667,160 | 64,662,120 | |||
| 8 5 5 1 1 0 | 97,004,040 | 96,989,760 | |||
| 9 4 4 2 1 0 | 193,996,320 | 193,991,280 | |||
| 8 5 4 2 1 0 | 290,998,680 | 290,982,720 | |||
| 8 4 4 3 1 0 | 129,334,260 | 129,324,180 | |||
| 8 4 4 2 2 0 | 258,658,440 | 258,658,440 | |||
| 9 4 4 1 1 1 | 387,992,640 | 387,982,560 | |||
| 8 5 4 1 1 1 | 581,995,680 | 581,967,120 | |||
| 8 4 4 2 1 1 | 521,000 | 519,040 | |||
| 7 7 6 0 0 0 | 2,079,380 | 2,077,630 | |||
| 7 7 5 1 0 0 | 3,120,540 | 3,116,550 | |||
| 7 6 6 1 0 0 | 6,236,460 | 6,234,570 | |||
| 7 7 4 2 0 0 | 12,471,240 | 12,470,820 | |||
| 7 6 5 2 0 0 | 832,592 | 830,212 | |||
| 6 6 6 2 0 0 | 4,158,480 | 4,155,540 | |||
| 7 6 4 3 0 0 | 8,316,680 | 8,311,360 | |||
| 6 6 5 3 0 0 | 16,628,880 | 16,627,200 | |||
| 6 6 4 4 0 0 | 24,945,000 | 24,939,120 | |||
| 7 7 4 1 1 0 | 49,884,960 | 49,883,280 | |||
| 7 6 5 1 1 0 | 971,840 | 969,178 | |||
| 6 6 6 1 1 0 | 5,821,424 | 5,818,204 | |||
| 7 6 4 2 1 0 | 14,555,240 | 14,546,980 | |||
| 6 6 5 2 1 0 | 29,100,960 | 29,097,180 | |||
| 6 6 4 3 1 0 | 19,402,630 | 19,396,190 | |||
| 6 6 4 2 2 0 | 58,201,640 | 58,194,640 | |||
| 7 6 4 1 1 1 | 116,397,120 | 116,395,440 | |||
| 6 6 5 1 1 1 | 87,308,760 | 87,291,960 | |||
| 6 6 4 2 1 1 | 174,598,200 | 174,590,640 | |||
| 11 3 3 3 0 0 | 17,463,432 | 17,455,452 | |||
| 11 3 3 2 1 0 | 34,920,144 | 34,917,624 | |||
| 10 3 3 3 1 0 | 29,103,480 | 29,094,660 | |||
| 11 3 2 2 2 0 | 87,302,040 | 87,292,380 | |||
| 10 3 3 2 2 0 | 174,595,680 | 174,593,160 | |||
| 11 3 2 2 1 1 | 116,398,800 | 116,393,760 | |||
| 10 3 3 2 1 1 | 174,603,240 | 174,585,600 | |||
| 11 2 2 2 2 1 | 349,191,360 | 349,186,320 | |||
| 10 3 2 2 2 1 | 523,792,920 | 523,773,600 | |||
| 10 2 2 2 2 2 | 36,382,500 | 36,369,900 | |||
| 9 5 3 3 0 0 | 145,500,600 | 145,490,100 | |||
| 9 5 3 2 1 0 | 218,260,560 | 218,234,940 | |||
| 9 4 3 3 1 0 | 436,491,720 | 436,480,380 | |||
| 8 5 3 3 1 0 | 291,000,360 | 290,981,040 | |||
| 9 5 2 2 2 0 | 581,983,920 | 581,978,880 | |||
| 9 4 3 2 2 0 | 872,982,600 | 872,961,600 | |||
| 8 5 3 2 2 0 | 1,309,493,640 | 1,309,441,560 | |||
| 8 4 3 3 2 0 | 387,992,700 | 387,982,620 | |||
| 9 5 2 2 1 1 | 1,163,967,840 | 1,163,957,760 | |||
| 9 4 3 2 1 1 | 1,745,963,520 | 1,745,924,880 | |||
| 8 5 3 2 1 1 | 1,109,966 | 1,107,166 | |||
| 8 4 3 3 1 1 | 6,652,896 | 6,649,536 | |||
| 9 4 2 2 2 1 | 16,632,240 | 16,623,840 | |||
| 8 5 2 2 2 1 | 33,257,760 | 33,254,400 | |||
| 8 4 3 2 2 1 | 22,174,140 | 22,167,420 | |||
| 8 4 2 2 2 2 | 66,515,520 | 66,508,800 | |||
| 7 7 3 3 0 0 | 133,024,320 | 133,024,320 | |||
| 7 7 3 2 1 0 | 99,776,640 | 99,759,840 | |||
| 7 6 3 3 1 0 | 199,539,840 | 199,533,120 | |||
| 7 7 2 2 2 0 | 7,761,742 | 7,757,822 | |||
| 7 6 3 2 2 0 | 23,284,016 | 23,274,496 | |||
| 6 6 3 3 2 0 | 46,560,192 | 46,556,832 | |||
| 7 7 2 2 1 1 | 38,804,140 | 38,793,500 | |||
| 7 6 3 2 1 1 | 116,402,160 | 116,390,400 | |||
| 6 6 3 3 1 1 | 232,794,240 | 232,790,880 | |||
| 7 6 2 2 2 1 | 155,198,460 | 155,191,740 | |||
| 6 6 3 2 2 1 | 232,803,200 | 232,781,920 | |||
| 6 6 2 2 2 2 | 465,588,480 | 465,581,760 | |||
| 7 5 5 3 0 0 | 698,389,440 | 698,365,920 | |||
| 7 5 4 4 0 0 | 46,563,552 | 46,553,472 | |||
| 6 5 5 4 0 0 | 139,680,576 | 139,670,496 | |||
| 7 5 5 2 1 0 | 279,351,072 | 279,351,072 | |||
| 7 5 4 3 1 0 | 58,205,280 | 58,191,000 | |||
| 6 5 5 3 1 0 | 232,797,600 | 232,787,520 | |||
| 7 4 4 4 1 0 | 349,203,120 | 349,174,560 | |||
| 6 5 4 4 1 0 | 698,382,720 | 698,372,640 | |||
| 7 5 4 2 2 0 | 465,595,200 | 465,575,040 | |||
| 6 5 5 2 2 0 | 931,170,240 | 931,170,240 | |||
| 7 4 4 3 2 0 | 1,396,765,440 | 1,396,745,280 | |||
| 6 5 4 3 2 0 | 2,095,161,600 | 2,095,104,480 | |||
| 6 4 4 4 2 0 | 290,999,520 | 290,981,880 | |||
| 6 4 4 3 3 0 | 581,997,360 | 581,965,440 | |||
| 7 5 4 2 1 1 | 1,163,967,840 | 1,163,957,760 | |||
| 6 5 5 2 1 1 | 1,745,961,840 | 1,745,926,560 | |||
| 7 4 4 3 1 1 | 775,985,400 | 775,965,240 | |||
| 6 5 4 3 1 1 | 2,327,935,680 | 2,327,915,520 | |||
| 6 4 4 4 1 1 | 3,491,920,320 | 3,491,856,480 | |||
| 7 4 4 2 2 1 | 3,103,900,920 | 3,103,900,920 | |||
| 6 5 4 2 2 1 | 4,655,871,360 | 4,655,831,040 | |||
| 6 4 4 3 2 1 | 27,166,848 | 27,155,646 | |||
| 6 4 4 2 2 2 | 54,320,814 | 54,315,774 | |||
| 5 5 5 5 0 0 | 54,324,174 | 54,312,414 | |||
| 5 5 5 4 1 0 | 162,961,232 | 162,948,352 | |||
| 5 5 4 4 2 0 | 325,911,264 | 325,907,904 | |||
| 5 4 4 4 3 0 | 67,909,580 | 67,892,500 | |||
| 4 4 4 4 4 0 | 271,598,380 | 271,584,380 | |||
| 5 5 4 4 1 1 | 407,410,640 | 407,375,920 | |||
| 5 4 4 4 2 1 | 814,781,520 | 814,766,400 | |||
| 4 4 4 4 3 1 | 543,195,550 | 543,169,790 | |||
| 4 4 4 4 2 2 | 1,086,368,700 | 1,086,361,980 | |||
| 9 3 3 3 2 0 | 1,629,561,920 | 1,629,533,920 | |||
| 8 3 3 3 3 0 | 2,444,369,760 | 2,444,299,200 | |||
| 9 3 3 2 2 1 | 81,485,376 | 81,469,416 | |||
| 8 3 3 3 2 1 | 325,914,624 | 325,904,544 | |||
| 9 3 2 2 2 2 | 488,880,336 | 488,848,416 | |||
| 8 3 3 2 2 2 | 977,733,792 | 977,723,712 | |||
| 7 5 3 3 2 0 | 407,396,640 | 407,377,320 | |||
| 7 4 3 3 3 0 | 814,791,600 | 814,756,320 | |||
| 6 5 3 3 3 0 | 1,629,552,960 | 1,629,542,880 | |||
| 7 5 3 2 2 1 | 2,444,341,200 | 2,444,302,560 | |||
| 7 4 3 3 2 1 | 1,086,375,420 | 1,086,355,260 | |||
| 6 5 3 3 2 1 | 3,259,105,920 | 3,259,085,760 | |||
| 6 4 3 3 3 1 | 4,888,679,040 | 4,888,608,480 | |||
| 7 5 2 2 2 2 | 1,018,503,360 | 1,018,450,440 | |||
| 7 4 3 2 2 2 | 2,036,946,240 | 2,036,923,560 | |||
| 6 5 3 2 2 2 | 1,357,976,040 | 1,357,937,400 | |||
| 6 4 3 3 2 2 | 4,073,890,800 | 4,073,848,800 | |||
| 5 5 5 3 2 0 | 6,110,877,360 | 6,110,769,840 | |||
| 5 5 4 3 3 0 | 5,431,836,600 | 5,431,816,440 | |||
| 5 5 5 2 2 1 | 8,147,778,240 | 8,147,700,960 | |||
| 5 5 4 3 2 1 | 10,863,673,020 | 10,863,632,700 | |||
| 5 4 4 3 3 1 | 97,780,440 | 97,765,320 | |||
| 5 5 4 2 2 2 | 488,871,936 | 488,856,816 | |||
| 5 4 4 3 2 2 | 977,743,872 | 977,713,632 | |||
| 4 4 4 3 3 2 | 1,955,457,504 | 1,955,457,504 | |||
| 7 3 3 3 2 2 | 2,933,201,376 | 2,933,171,136 | |||
| 6 3 3 3 3 2 | 1,222,184,880 | 1,222,137,000 | |||
| 5 5 3 3 2 2 | 2,444,329,440 | 2,444,314,320 | |||
| 5 4 3 3 3 2 | 1,629,563,040 | 1,629,532,800 | |||
| 4 4 3 3 3 3 | 4,888,658,880 | 4,888,628,640 | |||
| 4 4 4 4 2 2 | 7,333,013,520 | 7,332,917,760 | |||
| 5 5 3 3 3 1 | 6,518,191,680 | 6,518,191,680 | |||
| 5 5 3 3 2 2 | 9,777,317,760 | 9,777,257,280 | |||
| 5 4 4 4 3 0 | 2,036,961,360 | 2,036,908,440 | |||
| 5 4 4 4 2 1 | 6,110,833,680 | 6,110,775,720 | |||
| 5 4 4 3 3 1 | 8,147,754,720 | 8,147,724,480 | |||
| 5 4 4 3 2 2 | 12,221,662,320 | 12,221,556,480 | |||
| 5 4 3 3 3 2 | 16,295,509,440 | 16,295,448,960 | |||
| 5 3 3 3 3 3 | 21,727,305,720 | 21,727,305,720 | |||
| 4 4 4 4 4 0 | 2,546,223,120 | 2,546,142,480 | |||
| 4 4 4 4 3 1 | 10,184,706,000 | 10,184,643,000 | |||
| 4 4 4 4 2 2 | 15,277,122,000 | 15,276,958,200 | |||
| 4 4 4 3 3 2 | 20,369,406,960 | 20,369,291,040 | |||
| 4 4 3 3 3 3 | 27,159,162,480 | 27,159,102,000 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Balasubramanian, K. Enumeration of n-Dimensional Hypercubes, Icosahedra, Rubik’s Cube Dice, Colorings, Chirality, and Encryptions Based on Their Symmetries. Symmetry 2024, 16, 1020. https://doi.org/10.3390/sym16081020
Balasubramanian K. Enumeration of n-Dimensional Hypercubes, Icosahedra, Rubik’s Cube Dice, Colorings, Chirality, and Encryptions Based on Their Symmetries. Symmetry. 2024; 16(8):1020. https://doi.org/10.3390/sym16081020
Chicago/Turabian StyleBalasubramanian, Krishnan. 2024. "Enumeration of n-Dimensional Hypercubes, Icosahedra, Rubik’s Cube Dice, Colorings, Chirality, and Encryptions Based on Their Symmetries" Symmetry 16, no. 8: 1020. https://doi.org/10.3390/sym16081020





