One-Cycle Control with Composite Function Embedded for Boost Converters
Abstract
1. Introduction
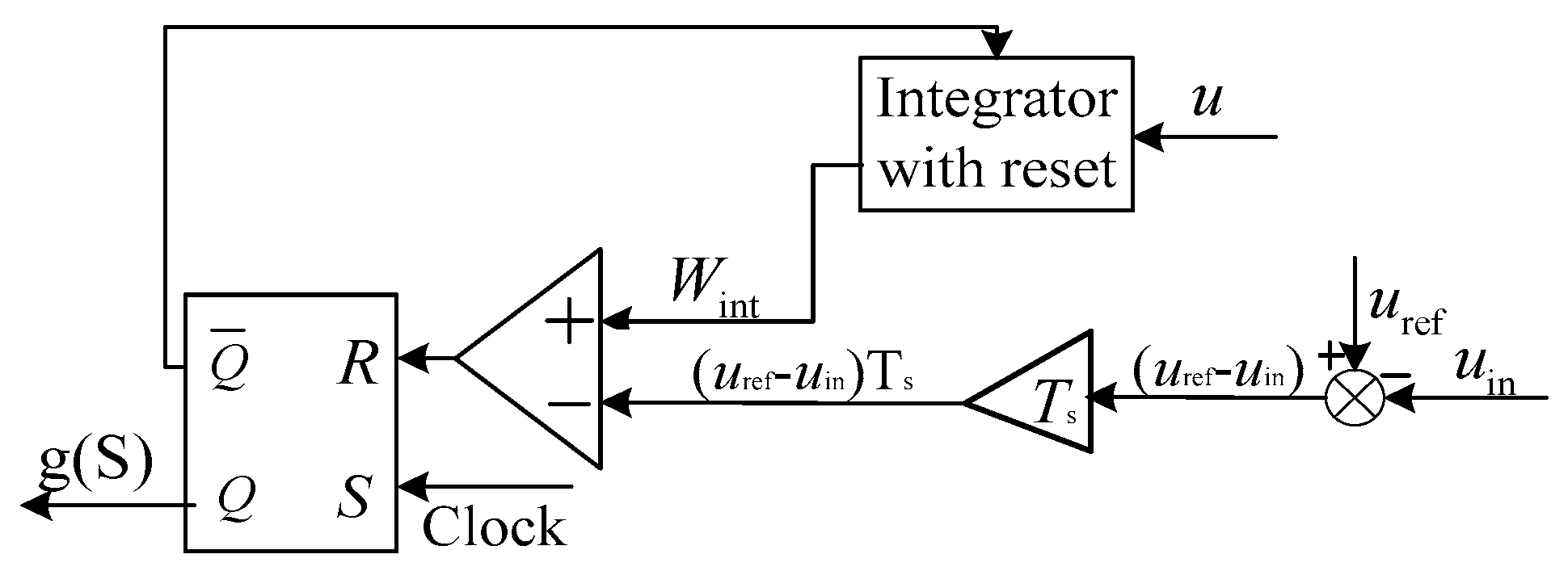
2. The Average Model of a Boost Converter and Stability Analysis of the Conventional OCC Boost Converter
2.1. The Average Model of a Boost Converter
2.2. The Stability Analysis of the OCC Boost Converter
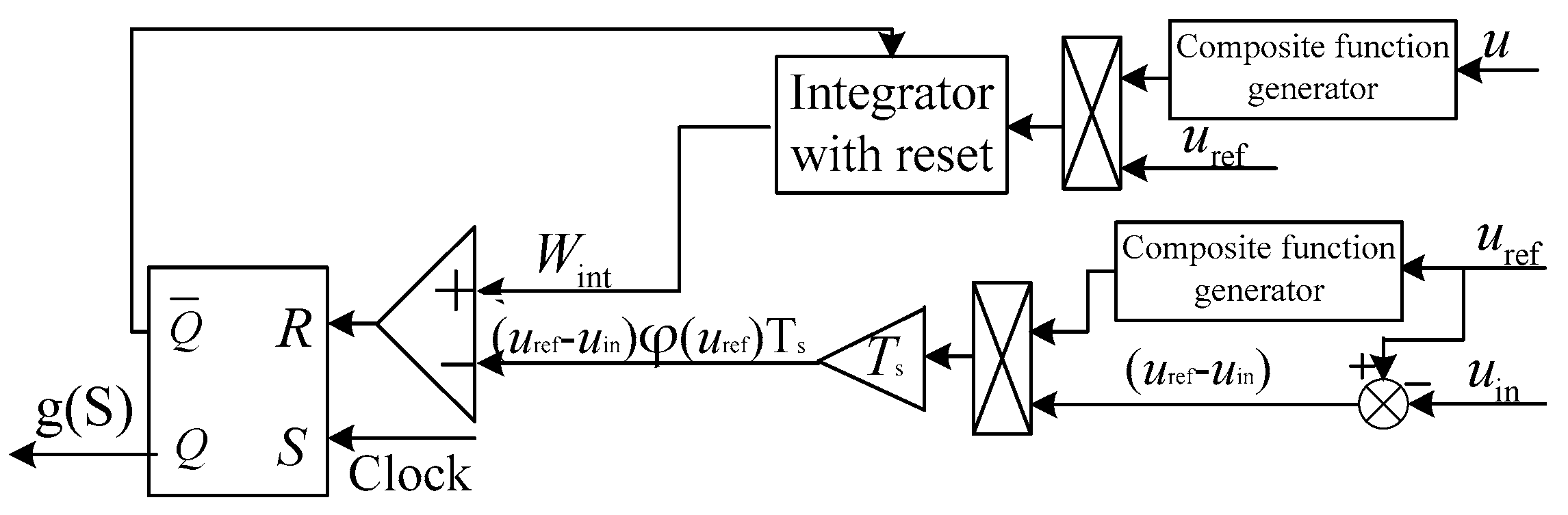
3. Stability Analysis of the Boost Converter Using the Proposed OCC with Composite Function Embedded
3.1. The Working Principle and Average Model of the Boost Converter Using the Proposed OCC with the Composite Function Embedded
3.2. The Stability Analysis of the Proposed OCC Boost Converter
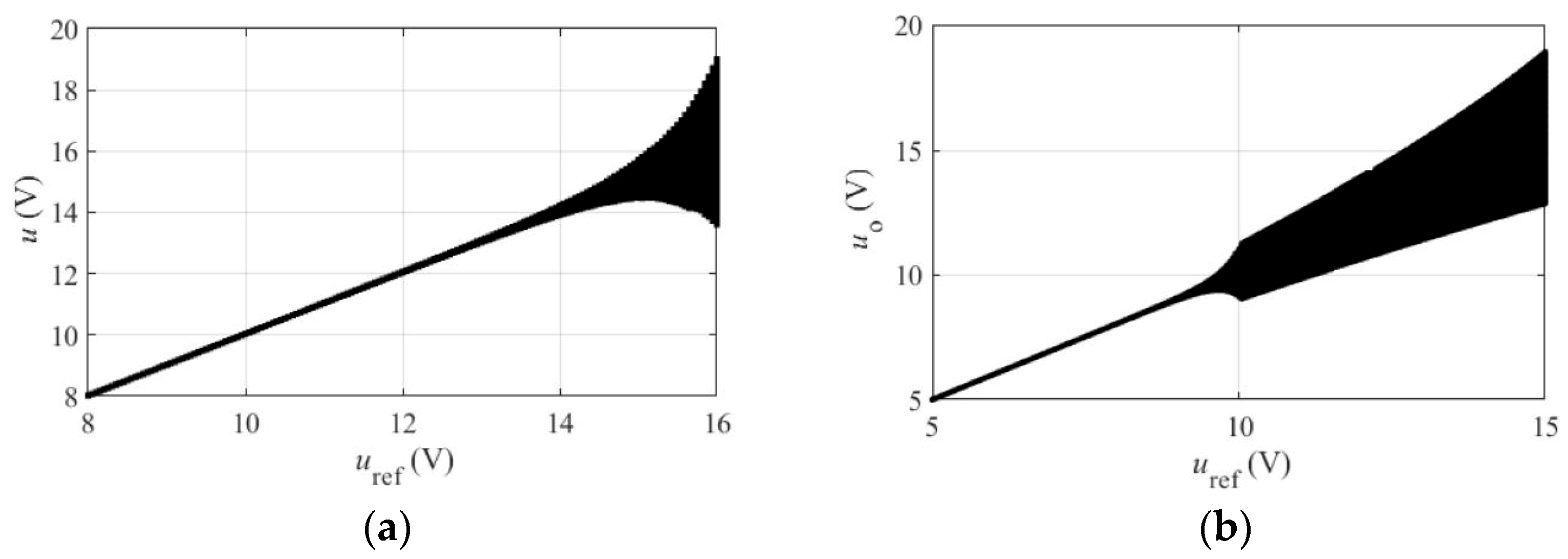
4. Calculation Verification
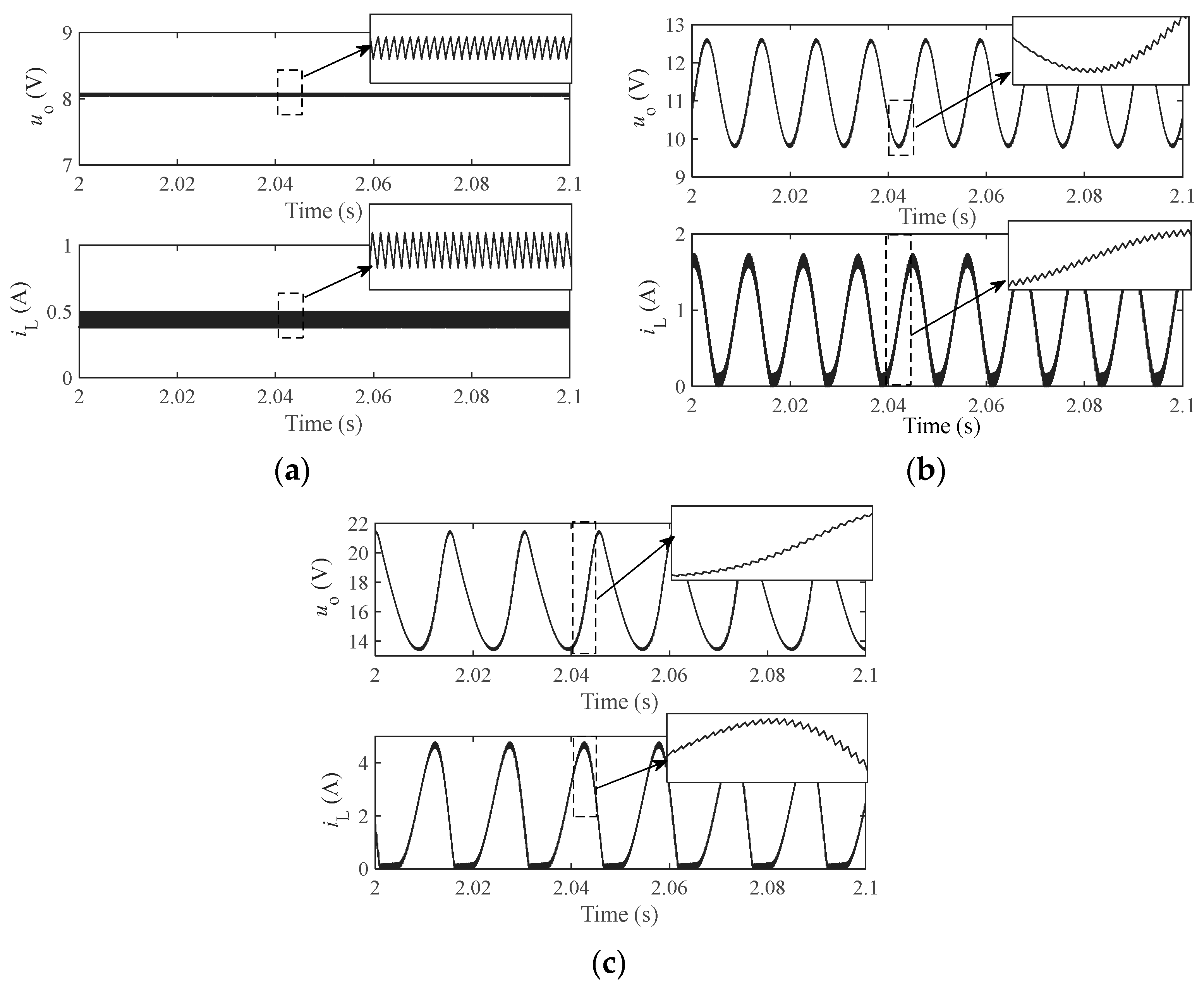
5. Simulation and Experimental Verification
5.1. The Simulation Results
5.2. The Experimental Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, Z.; Li, C.; Zheng, Z.D. A ZVS three-port DC-DC converter based on coupled inductor for fuel cell vehicle. IEEE Trans. Transp. Electrif. 2024, 10, 10314–10327. [Google Scholar] [CrossRef]
- Wang, F.L.; Zou, G.B.; Ding, H.X.; Xu, C.H. High voltage gain three-level DC-DC converter with switched-capacitor technique for DC wind farms. IEEE Trans. Ind. Electron. 2024, 71, 12267–12278. [Google Scholar] [CrossRef]
- Ertekin, D.A. High gain switched-inductor-capacitor DC-DC boost converter for photovoltaic-based micro-grid applications. CSEE J. Power Energy Syst. 2024, 10, 2398–2410. [Google Scholar]
- Trujillo, S.C.; Candelo-Becerra, J.E.; Hoyos, F.E. Numerical validation of a boost converter controlled by a quasi-sliding mode control technique with bifurcation diagrams. Symmetry 2022, 14, 694. [Google Scholar] [CrossRef]
- Jouili, K.; Charfeddine, M.; Alqarni, M. Adaptive feedback control of nonminimum phase boost converter with constant power load. Symmetry 2024, 16, 352. [Google Scholar] [CrossRef]
- Hu, W.; Zhang, B.; Yang, R.; Qiu, D.Y. Dynamic behaviours of constant on-time one-cycle controlled boost converter. IET Power Electron. 2018, 11, 160–167. [Google Scholar]
- Liu, Y.; Xiahou, K.; Wu, Q.H.; Pang, H.; Tang, G. Robust bang-bang control of disturbed nonlinear systems based on nonlinear observers. CSEE J. Power Energy Syst. 2020, 6, 193–202. [Google Scholar] [CrossRef]
- Chen, X.B.; Long, X.L.; Hu, W.; Xie, B.S. Bifurcation and chaos behaviors of Lyapunov function controlled PWM boost converter. Energy Rep. 2021, 7, 163–168. [Google Scholar] [CrossRef]
- Yu, X.H.; Yang, Y.M.; Xu, L.B.; Ke, D.L.; Zhang, Z.B.; Wang, F.X. Luenberger disturbance observer-based deadbeat predictive control for interleaved boost converter. Symmetry 2022, 14, 924. [Google Scholar] [CrossRef]
- Zheng, F.; Su, M.H.; Liu, B.J.; Liu, W.L. Adaptive virtual inertia control strategy for a grid-connected converter of DC microgrid based on an improved model prediction. Symmetry 2023, 15, 2072. [Google Scholar] [CrossRef]
- Zhang, L.; Zhang, Z.; Huang, L. Double Hopf bifurcation of time delayed feedback control for Maglev system. Nonlinear Dyn. 2012, 69, 961–967. [Google Scholar] [CrossRef]
- El Aroudi, A.; Orabi, M. Stabilizing technique for AC–DC boost PFC converter based on time delay feedback. IEEE Trans. Circuits Syst. II Exp. Briefs 2010, 57, 56–60. [Google Scholar] [CrossRef]
- Iu, H.H.C.; Robert, B. Control of chaos in a PWM current mode H-bridge inverter using time-delayed feedback. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 2003, 50, 1125–1129. [Google Scholar] [CrossRef]
- El Aroudi, A.; Haroun, R.; Cid-Pastor, A.; Martinez-Salamero, L. Suppression of line frequency instabilities in PFC AC-DC power supplies by feedback notch filtering the pre-regulator output voltage. IEEE Trans. Circuits Syst. I Reg. Pap. 2013, 60, 796–809. [Google Scholar]
- Liu, G.Y.; Caldognetto, T.; Mattavelli, P.; Magnone, P. Suppression of second-order harmonic current for droop-controlled distributed energy resource converters in DC microgrids. IEEE Trans. Ind. Electron. 2020, 67, 358–368. [Google Scholar] [CrossRef]
- Kavitha, A.; Uma, G. Resonant parametric perturbation method to control chaos in current mode controlled DC-DC buck-boost converter. J. Electr. Eng. Technol. 2010, 5, 171–178. [Google Scholar] [CrossRef]
- Wang, L.; Liu, Y.; Guo, Y.; Wu, Q.-H.; Tang, G. Stability analysis of DC-DC converters with energy balance control. CSEE J. Power Energy Syst. 2023, 9, 1765–1773. [Google Scholar]
- Lei, W.; Qinghua, W.; Wei, M.; Wenhu, T. Stability improvement for one cycle controlled boost converters based on energy balance principle. Chin. J. Electron. 2023, 32, 1293–1303. [Google Scholar] [CrossRef]
- Guo, Y.L.; Wu, Q.H.; Wang, L.; Tang, G.F. Stability enhancement of one-cycle controlled buck-boost converters with a composite function embedded. IEEE Trans. Circuits Syst. I Regul. Pap. 2020, 67, 3512–3520. [Google Scholar] [CrossRef]
- Ma, W.; Wang, M.Y.; Nie, H.L. Control of Hopf bifurcation in the one-cycle controlled boost converter and its experimental implementation. Acta Phys. Sin. 2011, 60, 100202. [Google Scholar] [CrossRef]

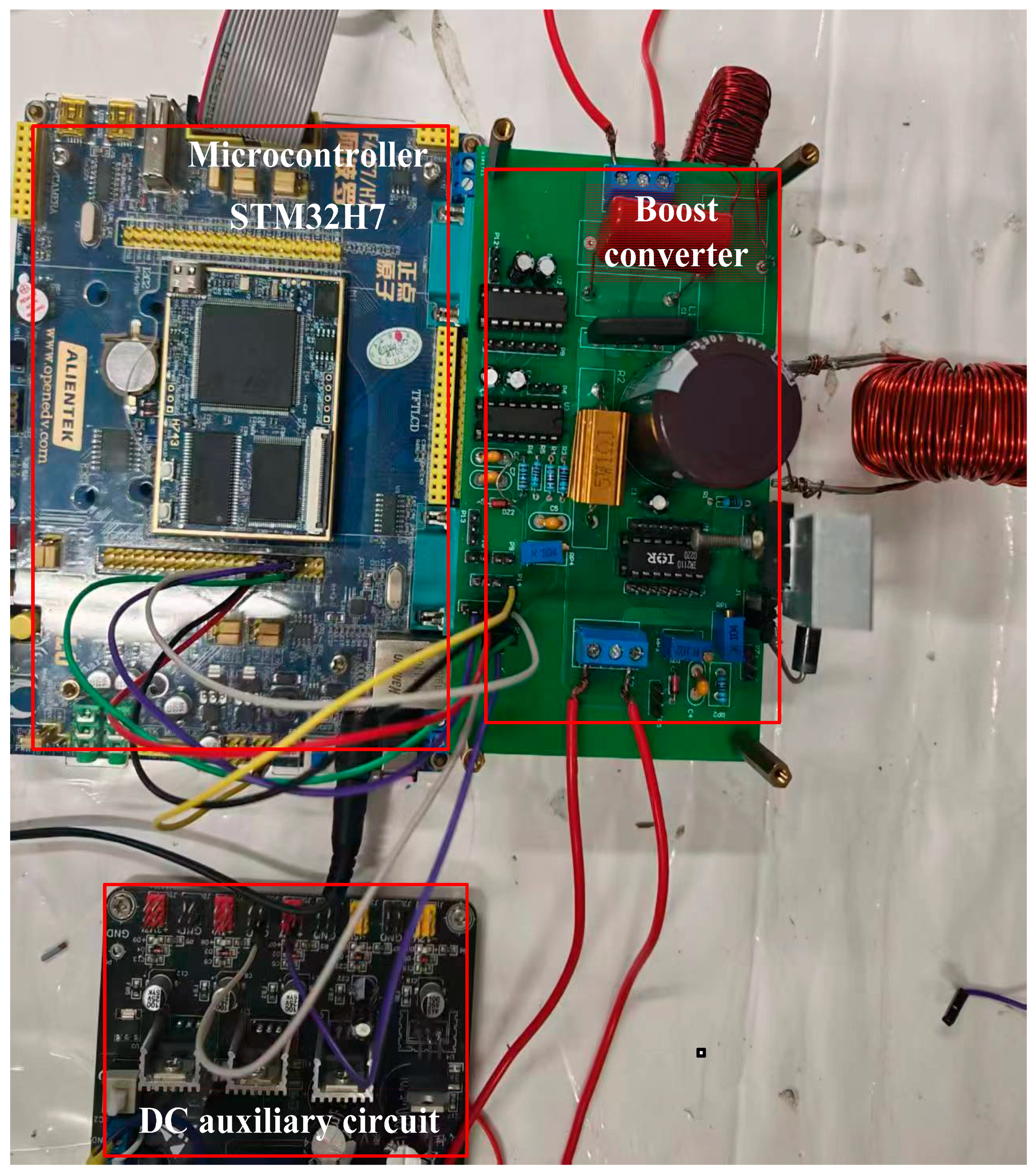
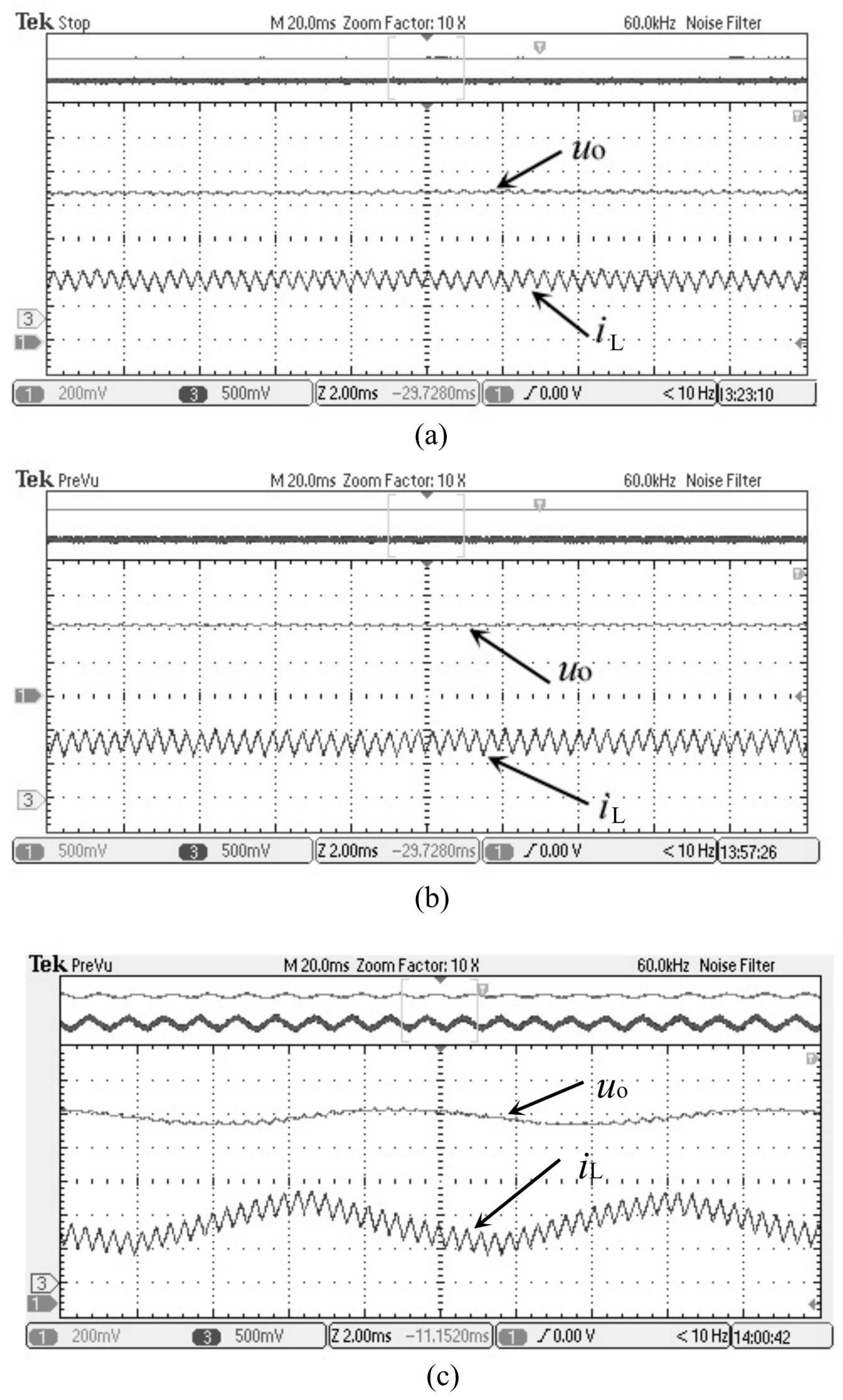
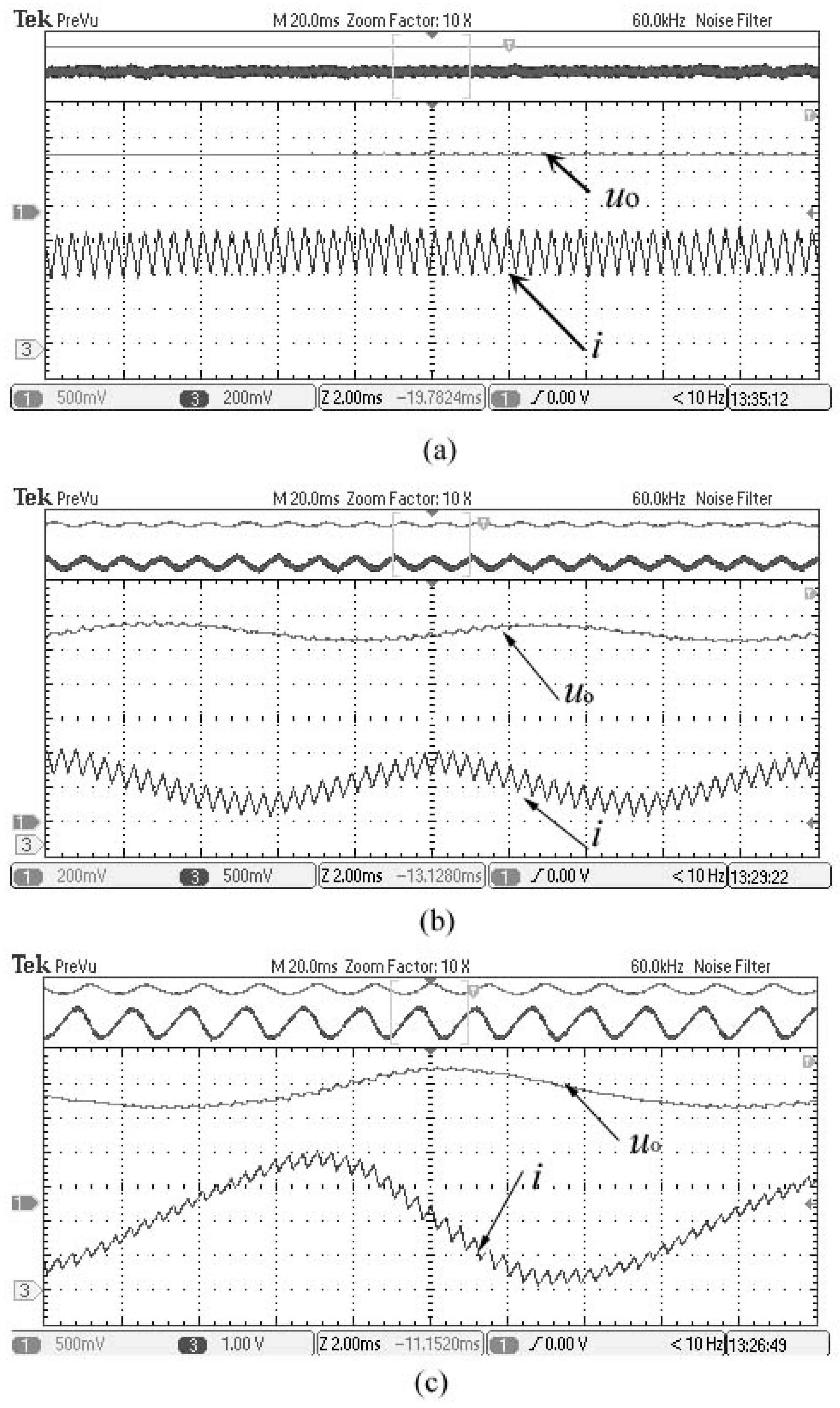
| (V) | L (µH) | C (µF) | R (Ω) | (Hz) |
|---|---|---|---|---|
| 5 | 3000 | 460 | 30 | 5000 |
| (V) | Theoretical | Simulation | Experiment | |||
|---|---|---|---|---|---|---|
| OCC | Embedded | OCC | Embedded | OCC | Embedded | |
| 8 | stable | stable | stable | stable | stable | stable |
| 11 | unstable | stable | unstable | stable | unstable | stable |
| 16 | unstable | unstable | unstable | unstable | unstable | unstable |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Chen, L.; Ma, W.; Li, J. One-Cycle Control with Composite Function Embedded for Boost Converters. Symmetry 2025, 17, 559. https://doi.org/10.3390/sym17040559
Wang L, Chen L, Ma W, Li J. One-Cycle Control with Composite Function Embedded for Boost Converters. Symmetry. 2025; 17(4):559. https://doi.org/10.3390/sym17040559
Chicago/Turabian StyleWang, Lei, Lidan Chen, Wei Ma, and Jubao Li. 2025. "One-Cycle Control with Composite Function Embedded for Boost Converters" Symmetry 17, no. 4: 559. https://doi.org/10.3390/sym17040559
APA StyleWang, L., Chen, L., Ma, W., & Li, J. (2025). One-Cycle Control with Composite Function Embedded for Boost Converters. Symmetry, 17(4), 559. https://doi.org/10.3390/sym17040559










