Diagnosis of the σ-, π- and (σ+π)-Aromaticity by the Shape of the NICS_zz-Scan Curves and Symmetry-Based Selection Rules

Abstract

The NICS_zz-scan curves of aromatic organic, inorganic and “all-metal” molecules in conjunction with symmetry-based selection rules provide efficient diagnostic tools of the σ-, π- and/or double (σ + π)-aromaticity. The NICS_zz-scan curves of σ-aromatic molecules are symmetric around the z-axis, having half-band widths approximately less than 3 Å with the induced diatropic ring current arising from T_x,y-allowed transitions involving exclusively σ-type molecular orbitals. Broad NICS_zz-scan curves (half-band width approximately higher than 3 Å) characterize double (σ + π)-aromaticity, the chief contribution to the induced diatropic ring current arising from T_x,y-allowed transitions involving both σ- and π-type molecular orbitals. NICS_zz-scan curves exhibiting two maxima at a certain distance above and below the molecular plane are typical for (σ + π)-aromatics where the π-diatropic ring current overwhelms the σ-type one. In the absence of any contribution from the σ-diatropic ring current, the NICS_zz(0) value is close to zero and the molecule exhibits pure π-aromaticity.

1. Introduction

The collection of recent reviews in the topic of aromaticity/antiaromaticity [1,2,3,4,5,6,7,8,9] shows a rather large consensus appear in the computation or experimental tests for the diagnosis of the aromaticity/antiaromaticity (structural, electronic, magnetic, thermodynamic and kinetic diagnostic tools) whereas the mechanisms themselves still remain open to the debate. Various criteria have been used to judge aromaticity/antiaromaticity, but no one is satisfactory, particularly in predicting the orbital type (s-, p-, d-, f-orbital) of aromaticity [10]. Among the usual diagnostic probes of aromaticity/antiaromaticity developed so far those based on the dynamic magnetic measurements, in particular the Nucleus Independent Chemical Shifts (NICS) was the most widely used as a quantitative measure for aromaticity [11,12,13]. The NICS index is the negative value of the isotropic magnetic shielding computed at chosen points in the vicinity of molecules and, hence, directly related to the shielding function. It is typically computed at ring centers, at points above, and even on grids [14] in and around the molecule. The NICS criterion continues to be enhanced and refined, with the out-of-plane zz-components of the shielding tensor elements NICS_zz(0) and NICS_zz(1) proposed to be a superior aromaticity descriptor [15,16]. Furthermore, the CMO-NICS criterion [17,18,19] of aromaticity dissects the total shieldings calculated at ring or cage centers, into individual contributions from each canonical molecular orbital (CMO).

It was also established that the shapes of the NICS-scan profiles provide a clear picture of the type of the ring current in aromatic and antiaromatic systems [20,21] and can be used to characterize unequivocally whether an inorganic system is aromatic, non-aromatic and antiaromatic [22,23]. The reliability of the NICS-scan scheme in assessing aromatic behavior of some planar six-membered heteroaromatic systems has been explored recently by Seal and Chakrabarti [24]. These authors using homodesmotic reactions, aromatic stabilization energy (ASE) and NICS-scan to assess aromaticity of several systems concluded that homodesmotic reactions and NICS-scan agree for all systems but ASE does not for some of them. Morao and Cossio [25] introduced a simple ring current model to characterize the in-plane σ- and out-of-plane π-aromaticity in pericyclic reactions. In-plane aromaticity exhibits a maximum diamagnetic shielding σ_zz^d at the ring center, while π-aromaticity exhibits two ring currents moving at a certain distance above and below the molecular plane. The σ-π separation in all metal aromatic and antiaromatic systems has critically been examined and computational strategies to unambiguously determine the aromaticity/antiaromaticity characteristics of such clusters have been presented by Pati et al. [26,27,28,29].

On the other hand, simple symmetry-based selection rules derived from group theory helped rationalize and predict ring currents to classify π systems [30,31,32,33]. Plotting of current-density maps was also suggested as a standard tool for the resolution of debates about aromaticity and for the interpretation of calculated chemical shift values [30,31,32,34,35,36]. Sundholm and coworkers [37,38,39] introduced the aromatic ring current shielding (ARCS) method to determine the strength of the induced ring current and consequently the relative degree of aromaticity in aromatic systems. In addition, they applied the gauge-including magnetically induced current (GIMIC) method to [c-Al₄]^2- and [c-Al₄]^4- clusters to elucidate the type of aromaticity in these aromatics [37,38,39]. Heine et al. [40,41] represented graphically with three-dimensional isosurfaces the induced magnetic field of cyclic molecules and showed that the appearance of the magnetic response characterizes aromatic, antiaromatic and non-aromatic molecules. They also showed that the z-component of the induced magnetic field is directly connected to NICS_zz. Finally, the recently developed Anisotropy of the Current Induced Density (ACID) mapping was suggested as a better probe of aromaticity [42,43].

Recently, we showed that the NICS_zz-scan curves in conjunction with symmetry-based selection rules for the lowest translationally- and rotationally-allowed transitions help rationalize and predict the orbital-type of aromaticity/antiaromaticity in magnetoresponsive three-membered rings of d- and f-block elements [44], and unequivocally probe the antiaromaticity in a wide range of antiaromatic organic and inorganic rings/cages [45]. Therefore, we thought it would be advisable to test the efficiency of the NICS_zz-scan profiles in conjunction with symmetry-based selection rules to provide a clear picture of the orbital type (s-, p-, d-, f-orbital) of aromaticity in a wide range of aromatic organic and inorganic molecules, proving its general use as a powerful diagnostic tool of the orbital type of aromaticity/antiaromaticity. To achieve this goal, we calculated the NICS_zz-scan profiles of a wide range of aromatic organic and inorganic molecules, whose magnetic-response properties have been exhaustively investigated and well-characterized in the framework of the aromaticity concept. The results obtained are thoroughly analyzed and presented herein.

2. Computational Details

In view of the good performance of density functional theory (DFT), we were inclined to use DFT calculations on the compounds we studied using the GAUSSIAN03 program suite [46]. The geometries of all species were fully optimized at the B3LYP level, using 6-311+G(d,p) basis set for all elements, except those of the fifth and sixth row elements for which the SDD basis set was used [47,48]. Full geometry optimization was performed for each structure using Schlegel’s analytical gradient method [49], and the attainment of the energy minimum was verified by calculating the vibrational frequencies that result in the absence of imaginary eigenvalues. All the stationary points were identified for minimum (number of imaginary frequencies NIMAG=0) or transition states (NIMAG=1). Magnetic shielding tensors were computed with the GIAO (gauge independent atomic orbitals) DFT method [50,51] as implemented in the GAUSSIAN03 series of programs [46] employing the B3LYP level of theory. Nucleus-Independent Chemical Shifts (NICS) were computed at the B3LYP level according to the procedure described by Schleyer et al. [11]. The computational protocol used is that applied in the previous studies of the aromaticity/antiaromaticity of most of the aromatic molecules discussed herein. The contribution to the induced ring current from the σ- and π-type diatropic and or paratropic translationally (T_x,y) and rotationally (R_z) allowed transitions was inferred from the estimated excitation energies based on symmetry and energy criteria. For a planar conjugated molecule in which the ring currents are induced by a magnetic field perpendicular to the molecular plane, three factors will determine the existence and strength of the contribution of an occupied-to-unoccupied orbital transition, ψ_n → ψ_p transition, namely symmetry, spatial distribution and energy. Symmetry determines whether a ψ_n → ψ_p transition contributes at all to the current density, spatial distribution of the orbitals determines whether an allowed transition moment will be large or small and the difference in orbital energies (excitation energies) affects the relative weight of a given transition moment in the total induced ring current. Thus, a ψ_n → ψ_p transition makes a conventionally diamagnetic (diatropic) contribution to current density if the direct product of representations Γ(ψ_n)× Γ(ψ_p)× Γ(T_x, T_y) contains a totally symmetric component, and a conventionally paramagnetic (paratropic) contribution to current density if the direct product Γ(ψ_n)× Γ(ψ_p)× Γ(R_z) contains a totally symmetric component. A mixed contribution results if both direct products have a totally symmetric component, while no contribution results if neither direct product has a totally symmetric component [30,31,32].

3. Results and Discussion

3.1. The NICS_zz-scan profiles of 2e- and 6e-σ-aromatics

Let us first examine the shape and most salient features of the NICS_zz-scan profiles of 2e- and 6e-σ-aromatics, the [c-H₃]⁺ and c-H₆ species being the prototypes [52,53,54,55]. It should be noted that the optimized structures of the [c-H₄]^2-, [c-H₅]^- and [c-H₆] clusters were obtained under symmetry constrains and correspond to third, second and first order saddle points, respectively. The optimized D_nh symmetric [c-H_x]^q aromatic rings are similar to the optimized structures reported previously [52,53,54,55]. The NICS_zz-scan profiles of selected 2e- and 6e-σ-aromatics are visualized in Figure 1, while the main features of the NICS_zz-scan curves along with the R_av values, e.g. the average distance of the ring current to the center of the ring, are compiled in

Table 1. The NICS values (in ppm) calculated at the ring center, NICS(0) and 1.0 Å above the ring center, NICS(1), the zz-component of the shielding tensor elements, NICS_zz(0) and NICS_zz(1) along with the half-band width of the NICS_zz-scan curves (in Å) and R_av (in Å) for selected σ-aromatic molecules computed at the B3LYP/6-311+G(d,p) level.

Compound	NICS(0)	NICS(1)	NICSzz(0)	NICSzz(1)	Half-band width	Rav
[c-H3]+ (D3h)	-33.5	-1.9	-36.9	-7.9	1.2	0.509
[c-H4]2- (D4h)	-16.0	-8.9	-66.8	-29.7	1.8	0.701
[c-H5]- (D5h)	-20.1	-9.4	-68.9	-30.5	1.8	0.843
[c-H6] (D6h)	-24.1	-10.0	-67.4	-31.1	1.9	0.992
[c-B3]- (D3h)	-73.6	-28.2	-75.6	-39.3	2.1	0.467
[c-B3]+ (D3h)	-65.4	-17.3	-65.7	-30.3	1.9	0.480
[c-B2C] (C2v)	-80.1	-22.6	-83.8	-31.6	1.6	0.438
[c-C3]2+ (D3h)	-95.0	-16.3	-100.3	-25.6	1.2	0.349
[c-B4] (D2h)	-35.7	-9.2	-36.8	4.7	0.9	0.647
[c-B4]2+ (D4h)	-28.8	-3.4	-29.9	1.5	1.0	0.698
[H2B(μ2-Η)2ΒΗ2] (D2h)	-26.0	-3.2	-20.8	-6.1	1.3	0.717
[c-B5]+ (D5h)	-36.2	-18.8	-56.6	-24.3	1.6	0.896
TS1a (D2h)	-27.8	-13.3	-64.4	-36.7	2.3	0.918
TS2 b (D3h)	-17.6	-10.2	-43.5	-28.6	2.8	1.382
[c-Li3]+ (D3h)	-11.1	-6.8	-8.7	-7.3	3.7	1.704
[c-C3H6] (D3h)	-42.7	-8.6	-29.8	-24.2	3.2	0.518

^a TS1 = transition state for the concerted transfer of two hydrogen atoms from ethane to ethylene (double group transfer reactions). ^b TS2 = transition state for the trimerization of acetylene to form benzene.

.

The half-band width, e.g., the width of the symmetric curve at half the NICS_zz value, of the NICS_zz-scan curves depends on the ring radius, R_av, evaluated as $R_{av}=\frac{1}{n}{\displaystyle \sum _{i=1}^n{R}_i}$, where n is the number of the nuclei of the ring, and R_i is the distance from the ring center to the nucleus i. For the rings where the 2p orbitals (radial 2p orbitals) participate also in the bonding, forming molecular orbitals contributing to the diatropic ring current of the σ-aromatics, R_av was evaluated as $R_{av}=\frac{1}{n}{\displaystyle \sum _{i=1}^n\left(R_i-\frac{4}{Z_i}{a}_0\right)}$, where Z_i is the atomic number of the i-atom, and a₀ is the Bohr radius. The term 4a₀/Z_i corresponds to the maximum of the probability of the radial function 4πr²R_n,l²(r) for a 2p atomic orbital.

It can be seen that the NICS_zz-scan curves are symmetric around the z-axis with the NICS_zz values decaying rapidly and monotically with respect to R. The half-band widths of the NICS_zz-scan curves for the σ-aromatics exhibiting s-orbital aromaticity range from 1.2 to 1.9 Å (R_av ranging from 0.509 to 0.992 Å) is characteristic of the in-plane pure σ-aromaticity due to cyclic delocalization of two 1s electrons over the three-membered ring in [c-H₃]⁺ and six 1s electrons over the four-, five- and six-membered rings in [c-H₄]^2-, [c-H₅]^- and [c-H₆]. Analogous symmetric sharp NICS_zz-scan curves with half-band widths in the range of 0.9 to 2.1 Å are obtained for the [c-B₃]^-, [c-B₃]⁺, [c-B₂C], [c-C₃]²⁺, [c-B₄], [c-B₄]²⁺, [c-B₅]⁺ (Figure 1b,c), as well as for the transition states TS1 and TS2 (Figure 1d) of the concerted transfer of two hydrogen atoms from ethane to ethylene (double group transfer reactions) and the trimerization of acetylene to form benzene respectively. shown in Scheme I. The latter two NICS_zz-scan curves have a slightly larger half-band width of 2.3 and 2.8 Å, respectively.

3.2. Magnetotropicity of the 2e- and 6e-σ-aromatics

In the [c-H₃]⁺ species with D_3h symmetry, the chief contribution to induced diatropic ring current arises from the T_x,y-allowed HOMO (a₁′) → LUMO (e′) transition with excitation energy 18.74 eV. In the [c-H₄]^2- (D_4h) cluster the chief contribution to induced diatropic ring current arises from the T_x,y-allowed HOMO,-1 (e_u) → LUMO (b_1g) and HOMO,-1 (e_u) → LUMO+1 (a_1g) transitions having excitation energies 6.33 and 6.77 eV respectively (notice that HOMO,-1 denotes HOMO and HOMO-1, LUMO+1,+2 denotes LUMO+1 and LUMO+2, etc.). In the [c-H₅]^- (D_5h) cluster the chief contribution to induced diatropic ring current arises from the T_x,y-allowed HOMO,-1 (e₁′) → LUMO+2 (a₁′) with excitation energy 11.02 eV. Finally, in the [c-H₆] (D_6h) cluster the chief contribution to induced diatropic ring current arises from the T_x,y-allowed HOMO,-1 (e_1u) → LUMO+2 (a_1g) with excitation energy 14.14 eV. The higher excitation energy of the T_x,y-allowed transition in the [c-H₃]⁺ cluster accounts well for the weaker aromatic character. It should be noted that all orbitals involved in the T_x,y-allowed transitions responsible for the induced diatropic ring current are σ-type MOs.

In the [c-B₃]^- and [c-C₃]²⁺ species with D_3h symmetry, the T_x,y-allowed HOMO (a₁′) → LUMO+1,+2 (e′) excitations with excitation energies 3.42 and 4.25 eV respectively are responsible for the induced diatropic ring current. On the other hand, for the [c-B₃]⁺ species the T_x,y-allowed HOMO,-1 (e′) → LUMO (a₁′) excitation with excitation energy 4.16 eV contributes primarily to the induced diatropic ring current. The lower magnetic aromaticity of the [c-B₃]⁺ species compared to that of the [c-B₃]^- 2e-σ-aromatic could be explained by the higher energy difference between the orbitals involved in the respective excitations (4.16 versus 3.42 eV). Both the e′ and a₁′ MOs participating in the T_x,y-allowed transitions are σ-type MOs and are primarily responsible for the σ-aromaticity of the [c-B₃]^-.and [c-B₃]⁺ species. The 1a₂″ bonding HOMO-1 in [c-B₃]^- and [c-C₃]²⁺ and HOMO in [c-B₃]⁺ constructed from the overlap of the 2p_z AOs of the ring atoms, being a pure π-bonding MO similar to the π-MO in the cyclopropenyl cation ([c-C₃H₃]⁺), is magnetically inactive and therefore cannot contribute to the induced diatropic ring current. The magnetic inactivity of the 1a₂″ π-MO is further confirmed from the NICS_zz-scan profile of the putative [c-B₃]³⁺ species where, due to the removal of two electrons, the 1a₂″ π-MO is vacant. The computed NICS_zz(0) and NICS_zz(1) values for the [c-B₃]³⁺ species are -50.7 and -6.3 ppm, respectively, while the half-band width is 1.0 Å. However, the higher NICS_zz(0) value of the [c-B₃]⁺ species by 15.0 ppm compared to that of the [c-B₃]³⁺ one is probably indicative for a small contribution of the 1a₂″ π-MO to the diatropic ring current as well, supported by the T_x,y-allowed transitions HOMO-1 (a₂″) → LUMO+5,+6 (e″) in [c-B₃]^-, HOMO (a₂″) → LUMO+3,+4 (e″) in [c-B₃]⁺ and HOMO-1 (a₂″) → LUMO+2,+3 (e″) in [c-C₃]²⁺ having relatively high excitation energies of 5.97, 8.77 and 11.20 eV, respectively. As the contribution of the 1a₂″ π-MO to the diatropic ring current giving rise to π-aromaticity is small, the NICS_zz-scan curve is still narrow with band width less than 3.0 Å. Boldyrev and co-workers [56,57,58] have identified [c-B₃]^- as 2+2 doubly aromatic system containing a π-type (1a₂″) HOMO-1 and radial σ-type (2a₁′) HOMO both molecular orbitals contributing to the induced diatropic ring current. The NICS_zz-scan curve of the [c-B₃]^- species in conjunction with symmetry-based selection rules are in support of the double aromaticity, but with the induced π-diatropic ring current much weaker than the dominant induced σ-diatropic ring current. In effect the canonical orbital contributions to the out-of-plane NICS tensor component (CMO-NICS_zz) for the [c-B₃]^- species estimated to be -16.1 ppm from the HOMO-4 (1a₁′), -36.0 ppm from the degenerate HOMO-2,-3 (1e′), -11.5 ppm from the HOMO-1 (1a₂″), the π-type MO and -11.7 ppm from the HOMO (2a₁′) clearly illustrate the much weaker induced π-diatropic ring current (-11.5 ppm) than the dominant σ-diatropic ring current (-63.8 ppm). Therefore, the [c-B₃]^- species could be considered practically as a σ-aromatic species. Similarly, the estimated CMO-NICS_zz values for the [c-B₃]⁺ species found to be -16.6 ppm from the HOMO-3 (1a₁′), -37.2 ppm from the degenerate HOMO-1,-2 (1e′) and only -12.3 ppm from the π-type HOMO (1a₂″). Compare again the much weaker induced π-diatropic ring current (-12.3 ppm) than the dominant σ-diatropic ring current (-53.8 ppm). Finally, the estimated CMO-NICS_zz values for the putative [c-B₃]³⁺ species found to be -18.1 ppm from the HOMO-1 (1a₁′) and -31.2 ppm from the degenerate HOMO,-1 (1e′) contribute exclusively to the induced σ-diatropic ring current. Notice that the 1a₁′ and 1e′ bonding and nonbonding MOs respectively are formed primarily from the overlap of the filled 2s AOs of the boron atoms. It can be concluded that the CMO-NICS_zz analysis data reinforce the efficiency of the NICS_zz-scan curves and symmetry-based rules model to diagnose the orbital type of aromaticity of the aforementioned 2e- and 6e-σ-aromatics.

In the [c-B₄] (D_2h) molecule the T_x,y-allowed transitions contributing to the induced diatropic ring current are the HOMO-2 (b_3u) → LUMO (a_g), HOMO-3 (b_2u) → LUMO (a_g) and HOMO (a_g) → LUMO+4 (b_2u) excitations with excitation energies 4.58, 6.93 and 6.96 eV respectively. In addition the R_z-allowed HOMO (a_g) → LUMO+3 (b_1g) transition with excitation energy 6.27 eV contributes to the long range induced paratropic ring current (Figure 1c). The same holds true for the [c-B₄]²⁺ (D_4h) dicationic species where the T_x,y-allowed transitions contributing to the induced diatropic ring current are the HOMO-2,-3 (e_u) → LUMO (a_1g) and HOMO (a_2u) → LUMO+2,+3 (e_u) excitations with excitation energies 6.26 and 6.36 eV, respectively, while the R_z-allowed HOMO-1 (b_2g) → LUMO+1 (b_1g) transition with excitation energy 5.25 eV contributes to the long range induced paratropic ring current. Finally, in the [c-B₅]⁺ (D_5h) molecule the T_x,y-allowed transitions contributing to the induced diatropic ring current are the HOMO-1 (a₂″) → LUMO,+1 (e₁″) and HOMO (a₁′) → LUMO+2,+3 (e₁′) excitations with excitation energies 4.86 and 4.96 eV, respectively.

It is important to note that the dominant contribution to aromaticity of the aforementioned σ-aromatics due to the in-plane σ-MOs constructed from the overlap of the 2s and/or 2p AOs of the ring atoms is nicely reflected on the excellent linear correlation of the NICS_zz(0) with the R_av of the rings (Figure 2).

In-plane aromaticity is a general feature of transition states associated with pericyclic reactions [25,56,57,58]. Cossio et al. [25] using a simple ring current model based on the NICS-scan profiles of the transition states was able to characterize the in-plane and π-aromaticity. For the transition states exhibiting in-plane aromaticity the NICS-scan curves showed a maximum at the molecular plane and decaying rapidly above and below of such plane. In contrast, in π-aromatic systems the NICS-scan curves showed a maximum at a certain distance above and below the molecular plane, which corresponds to the covalent radii of the involved atoms. The shapes of the NICS_zz-scan curves of the transition states TS1 and TS2 for the concerted transfer of two hydrogen atoms from ethane to ethylene (double group transfer reactions) [59] and the trimerization of acetylene to form benzene [60,61] respectively (Figure 1d), provide a clear evidence for their in-plane σ-aromatic character. Indeed the NICS_zz-scan curves are symmetric sharp curves with half-band widths less than 3 Å.

The predictive power of the NICS_zz-scan curves on the orbital type of aromaticity was further corroborated by exploring the orbital type of aromaticity of the [c-B₃H₇]^2- (C_s) [62] and [c-B₃(μ₂-P)₃]^2- (D_3h) σ-aromatics, both involving a three-membered B₃ ring. Indeed for both species the NICS_zz-scan curves are symmetric sharp curves with half-band widths of 1.6 and 1.8 Å, respectively. The computed NICS_zz(0), NICS_zz(1) values of the [c-B₃H₇]^2- and [c-B₃(μ₂-P)₃]^2- species are -46.1, -18.2 ppm and -38.9, -18.2, respectively. We also computed the NICS_zz-scan curve of diborane, B₂H₆, which showed all the characteristics of the NICS_zz-scan curves of pure σ-aromatics. It is a sharp symmetric curve with half-band width of 1.3 Å, with the NICS_zz(0) and NICS_zz(1) values being -20.8 and -6.1 ppm, respectively. On the basis of the linear relationship given in Figure 2 and using the R_av value of 0.717 Å, one obtains a NICS_zz(0) value of -20.4 ppm, which is in excellent agreement with the computed NICS_zz(0) value of -20.8 ppm.

In summary, all 2e- and 6e-σ-aromatics studied herein are characterized by sharp NICS_zz-scan curves symmetric around the z-axis (perpendicular to the ring plane) with the NICS_zz values decaying rapidly and monotically with respect to R, and having a half-band with approximately less than 3 Å.

3.3. The case of the [c-Li₃]⁺ and cyclopropane 2e-σ-aromatics

For the [c-Li₃]⁺ (D_3h) cation, another 2e-σ-aromatic prototype, the NICS_zz-scan curve, has also the features of the NICS_zz-scan curves of the pure σ-aromatic, but its half-band width is greater than 3 Å (3.7 Å). The broadening of the NICS_zz-scan curve of [c-Li₃]⁺ σ-aromatic is probably due to the bigger size of the three-membered Li₃ ring (R_av = 1.704 Å) that renders weaker the overlap of the 2s orbitals forming the 3c-2e bond. This is also mirrored on the estimated interaction energy between the Li₂ and Li⁺ fragments (-45.66 kcal/mol), which is much smaller than the interaction energy between the H₂ and H⁺ fragments to form the [c-H₃]⁺ species (-154.03 kcal/mol). Another plausible reason for the broadening of the NICS_zz-scan curve of [c-Li₃]⁺ σ-aromatic might be the contribution of the intrinsic local circulation of electrons around the nuclei to the diatropic NICS values. The pure σ-aromaticity in the [c-Li₃]⁺ cation was previously justified by Alexandrova and Boldyrev [63].

Considering the ring current model one would expect the ${\sigma }_{zz}^d/{\sigma }_{zz}^d(\max )$ versus z and the $NICS/NIC{S}_{\max }$ versus z curves to be similar:

$$\frac{{\sigma }_{zz}^d}{{\sigma }_{zz}^d(\max )}\approx \frac{NICS}{NIC{S}_{\max }^{}}$$

According to quantum theory for the ${\sigma }_{zz}^d(\max )$ occurring in the molecular plane (z = 0) for the $NIC{S}_{\max }^{}$ will have:

$$NIC{S}_{\max }^{}=-\kappa \frac{e^2{\mu }_0}{8\pi m_e}\frac{1}{R_{av}}$$

For two σ-aromatics exhibiting s-orbital aromaticity it can be easily written:

$$\frac{NIC{S}_{\max }^{}{}_1}{NIC{S}_{\max }^{}{}_2}=\frac{R_{av2}}{R_{av1}}$$

If we use as a reference σ-aromatic the prototype [c-H₃]⁺ cation we obtain the following relationship:

$$NIC{S}_{\max }^{}{}_2=-\frac{17.05}{R_{av2}}$$

On the basis of the above relationship we predict for the [c-Li₃]⁺ cation a NICS_max value of -10.0 ppm very close to the estimated NICS_zz(0) value of -11.2 ppm.

Cyclopropane being a saturated system was also found to be a strongly σ-aromatic molecule. Cyclopropane exhibits a ring current density induced by a perpendicular external magnetic field arising in the σ framework that is intense, diatropic and annular. The hallmarks of the σ-ring current in cyclopropane is reflected on the shape of the NICS_zz-scan curve, which corresponds to a symmetric curve with a maximum at z = 0 and a half-band width around 3.2 Å. Moreover, the main feature of the strong diatropic circulation in [c-C₃H₆], which reaches maximal intensity (j_max = 0.121 a.u.) outside the line of carbon centers [64,65], accounts well for the slight broadening of the NICS_zz-scan curve (half-band width > 3 Å). The calculation of the CMO-NICS_zz values for cyclopropane in D_3h symmetry showed that the Walsh orbitals HOMO,-1 (2e′), being σ-type MOs, have positive NICS_zz value of 22.2 ppm, the HOMO-2,-3 (1e″), being π-type MOs, have also positive NICS_zz value of 8.1 ppm and therefore induce σ- and π-type paratropic ring currents, respectively. On the other hand, the HOMO-5 (1a₂″), a π-type MO, has negative NICS_zz value of -8.7 ppm, which cancels the induced π-paratropic ring current from the 1e″ MOs. The remaining HOMO-4 (2a₁′), HOMO-6,-7 (1e′) and HOMO-8 (1a₁′) all being σ-type MOs, have negative NICS_zz values of -5.4, -18.8 and -21.3 ppm respectively. The CMO-NICS_zz results are nicely mirrored on the shape of the NICS_zz-scan curve of cyclopropane. It is interesting to notice that Pelloni et al. [65] demonstrated that both the large negative NICS value and anisotropies of cyclopropane are mainly determined by the in-plane components rather than the more relevant out-of-plane contribution. The existence of σ-aromaticity in cyclopropane has recently been challenged by ab initio valence bond (VBSCF/cc-PVTZ) computations which revealed directly that the σ-aromatic stabilization energy of cyclopropane is, at most, 3.5 kcal/mol relative to propane [66]. However, such small energy difference raised the question whether the cyclopropane is really the σ-aromatic paradigm.

3.4. The NICS_zz-scan curves of the M₃(μ₂-CO)₃(CO)₃ (M = Ni, Pd, Pt) molecules predict their σ-aromaticity

Let us now explore the efficiency of the NICS_zz-scan profiles to predict the pure σ-aromaticity characterizing the M₃(μ₂-CO)₃(CO)₃ (M = Ni, Pd, Pt) molecules. Recently, R. B. King [67] applied the σ-aromaticity model used for cyclopropane to Group 8 trinuclear metal-carbonyl complexes, [M₃(CO)₁₂] (M = Fe, Ru, Os). The σ-aromaticity of these molecules was further verified by a structural and NICS analysis reported recently [68]. According to this model the bonding in the M₃ triangles is composed by a core 3c-2e bond of Hückel topology formed by overlap of inward pointing radial hybrid orbitals of M and a surface (in-plane) 3c-4e bond of Möbius topology formed by tangential p-orbitals of M. Notice that for the M₃(μ₂-CO)₃(CO)₃ (M = Ni, Pd, Pt) molecules the presence of the μ₂-CO ligands precludes the 3c-4e Möbius surface bonding orbitals. The NICS_zz-scan curves of the Pt₃(μ₂-CO)₃(CO)₃ molecule along with the molecular orbital energy level diagram, symmetries, 3D molecular orbital pictures and lowest T_x,y-allowed transitions contributing to the diamagnetic ring current are visualized in Figure 3, while the most salient features of the NICS_zz-scan curves along with the half-band widths and R_av values of the rings of the M₃(μ₂-CO)₃(CO)₃ (M = Ni, Pd, Pt) molecules are compiled in

Table 2. The NICS values (ppm) calculated at the ring center, NICS(0) and 1.0 Å above the ring center, NICS(1), the zz-component of the shielding tensor element, NICS_zz(0) and NICS_zz(1) along with the half-band width (Å) and R_av (Å) for the M₃(μ₂-CO)₃(CO)₃ (M = Ni, Pd, Pt) molecules computed at the B3LYP/SDD(Pd,Pt)∪6-311+G(d,p) (C,O) levels.

Compound	NICS(0)	NICS(1)	NICSzz(0)	NICSzz(1)	Half-band width	Rav
Ni3(μ2-CO)3(CO)3 (C3v)	-34.2a	-21.0	-53.9a	-36.6	2.7	1.427
Pd3(μ2-CO)3(CO)3 (D3h)	-30.2	-14.0	-27.7	-23.6	4.6	1.602
Pt3(μ2-CO)3(CO)3 (D3h)	-39.3	-19.3	-37.9	-34.4	4.6	1.602

.

It can be seen that the NICS_zz-scan curves of the M₃ (M = Ni, Pd, Pt) clusters are sharp symmetric curves with half-band width less than 2 Å, illustrating their pure σ-aromatic character. The broadening of the NICS_zz-scan curves of the M₃(μ₂-CO)₃(CO)₃ (M = Ni, Pd, Pt) molecules (half-band width of 2.7-4.6 Å), could be attributed to the contribution of the diatropic NICS values of the strongly σ-aromatic c-M₂C rings to the diatropic NICS values of the M₃ core rings.

3.5. The NICS_zz-scan curves of triangular main group clusters exhibiting double (σ + π)-aromaticity

Let us further examine the NICS_zz-scan curves of three-membered rings of main group atoms, which have been extensively studied and characterized as double (σ + π)-aromatics. All these molecules showed NICS_zz-scan curves symmetric around the z-axis with the NICS_zz values decaying monotonically with respect to R, but with half-band width greater than 3 Å. The NICS_zz-scan profiles in the form of NICS_zz/NICS_zz^max versus R curves for selected triangular main group clusters exhibiting double (σ + π)-aromaticity are given in Figure 4, while the most salient features of the NICS_zz-scan curves along with the R_av values of the rings are compiled in

Table 3. The NICS values (in ppm) calculated at the ring center, NICS(0) and 1.0 Å above the ring center, NICS(1), the zz-component of the shielding tensor element, NICS_zz(0) and NICS_zz(1), the R_max (in Å) and the corresponding NICS_zz^max along with the R_av (in Å) for selected triangular (D_3h) main group double (σ + π)-aromatic clusters computed at the B3LYP/6-311+G(d,p) level.

Cluster	NICS(0)	NICS(1)	NICSzz(0)	NICSzz(1)	Rmax (Å)	NICSzzmax	ΔNICSa	Rav (Å)
[c-Be3]2- b	33.6	1.7	-44.1	-30.9	0.0	-44.1	0.0	1.245
[c-Be3]2- c	-65.0	-31.8	-42.8	-34.5	0.0	-42.8	0.0	1.200
[c-Mg3]2- b	-2.8	-4.0	-12.7	-12.2	0.4	-12.7	0.0	1.948
[c-Mg3]2- c	-36.5	-24.3	-13.6	-14.7	1.0	-14.7	1.1	1.859
[c-Al3]-	-35.6	-26.7	-34.4	-30.6	0.0	-34.4	0.0	1.465
[c-Al3]+	-26.4	-19.5	-30.4	-29.0	0.5	-31.1	0.7	1.486
[c-Ga3]-	-27.3	-22.6	-15.0	-25.1	1.1	-25.2	10.2	1.473
[c-Ga3]+	-14.4	-14.6	-11.8	-24.6	1.1	-24.8	13.0	1.529
[c-In3]-	-6.1	-10.6	-13.0	-13.8	1.0	-13.8	0.8	1.743
[c-Tl3]-	16.6	1.7	-5.4	-8.1	1.4	-8.6	3.2	1.824
[c-C3]2-	13.2	-10.2	-15.4	-11.6	0.0	-15.4	0.0	0.824
[c-Si3]2-	-3.1	-12.8	-26.4	-30.7	0.8	-31.0	4.6	1.355
[c-Ge3]2-	-4.2	-13.4	-21.2	-34.6	1.1	-34.6	13.4	1.444
[c-N3]+	-8.1	-20.5	-59.5	-35.3	0.3	-62.3	2.8	0.761
[c-P3]+	-12.9	-18.3	-40.3	-37.5	0.5	-42.6	2.3	1.221
[c-As3]+	-13.3	-18.4	-30.2	-39.4	0.8	-40.1	9.9	1.359
[c-Sb3]+	0.6	-7.3	-18.5	-22.4	1.0	-22.4	3.9	1.630
[c-Bi3]+	-11.8	-15.3	-19.2	-33.0	1.2	-33.4	14.2	1.686
c-S3	-42.3	-7.0	-32.4	-23.9	0.0	-32.4	0.0	1.226
c-Se3	-42.7	-9.8	-23.3	-25.6	0.8	-26.2	2.9	1.382
c-Te3	-23.8	-7.5	-13.1	-13.1	0.8	-13.2	0.1	1.669
c-Po3	-29.4	-9.5	-18.4	-16.7	0.0	-16.7	0.0	1.705

^a Δ_NICS = NICS_zz^max - NICS_zz(0). ^{b 1}A₁′(σ) ground state. ^{c 1}A₁′(π) electronic state.

.

3.5.1. Magnetotropicity of [c-Be₃]^2- and [c-Mg₃]^2- clusters

The ¹A₁′(σ) ground-state occupation in D_3h symmetry of 8 valence electrons for the [c-Be₃]^2- and [c-Mg₃]^2- clusters was found to be HOMO-3 (a₁′), HOMO-1,2 (e′), HOMO (a₁′). The lowest T_x,y-allowed transitions are the HOMO (a₁′) → LUMO+1,+2 (e′) and HOMO (a₁′) → LUMO+3,+4 (e′) with excitation energies of 1.22 and 1.76 eV for [c-Be₃]^2- cluster and 0.67 and 0.94 eV for [c-Mg₃]^2- cluster respectively. In these transitions a σ-type (a₁′) MO is involved in the excitation inducing a σ-diatropic ring current and therefore [c-Be₃]^2- and [c-Mg₃]^2- clusters in their ¹A₁′(σ) ground-states exhibit σ-aromaticity in line with the results concerning the tuning of aromaticity in trigonal alkaline earth metal clusters reported recently [69]. The NICS_zz-scan curves of the [c-Be₃]^2- and [c-Mg₃]^2- clusters in their ¹A₁′(σ) ground-states have the features of the NICS_zz-scan curves of pure σ-aromatics but their half-band width is greater than 3 Å (3.7 Å). The broadening of the NICS_zz-scan curves could be explained as in the case of the [c-Li₃]⁺ σ-aromatic.

We have also investigated the aromaticity/antiaromaticity of the ¹A₁′(π) electronic states of the [c-Be₃]^2- and [c-Mg₃]^2- clusters found as local minima at 4.6 and 5.3 kcal/mol higher in energy than the ¹A₁′(σ) ground states at the B3LYP/6-311+G(d) level in line with the results reported by Matito et al. [68]. The ¹A₁′(π) electronic state occupation in D_3h symmetry of 8 valence electrons for the [c-Be₃]^2- and [c-Mg₃]^2- clusters was found to be HOMO-3 (a₁′), HOMO-1,2 (e′), HOMO (a₂″). It can be seen that the lowest T_x,y-allowed transitions are the HOMO-1,2 (e′) → LUMO (a₁′) and HOMO (a₂″) → LUMO+5,6 (e″). In the first transition a σ-type (a₁′) MO is involved in the excitation inducing a σ- diatropic ring current, while in the second transition a π-type (a₂″) MO is involved inducing a π-diatropic ring current.

In the [c-Be₃]^2- cluster the σ-diatropic ring current overwhelms the π-diatropic ring current because of the lower excitation energy (1.59 eV) of the first transition relative to the second one (1.67 eV). This observation accounts well for the broadening of the NICS_zz-scan curve of the [c-Be₃]^2- cluster having its maximum at z = 0. On the other hand, in the [c-Mg₃]^2- cluster the π-diatropic ring current is much stronger than the σ-diatropic ring current because of the much lower excitation energy (0.94 eV) of the π-type transition relative to the σ-type one (2.19 eV). It is obvious then why the NICS_zz-scan curve of the ¹A₁′(π) [c- Mg₃]^2- exhibits two maxima at a certain distance above and below the molecular plane. Kuznetsov and Boldyrev showed that the unusual [c-Mg₃]^2- trigonal planar cluster exhibits an extraordinary type of aromaticity, where π-aromaticity occurs without initial formation of the σ-framework [70].

3.5.2. Magnetotropicity of the [c-E₃]^- (E = Al, Ga, In, Tl), [c-E₃]^2- (E = C, Si, Ge), [c-E₃]⁺ (E = N, P, As, Sb, Bi) and [c-E₃] (E = S, Se, Te, Po) rings

The NICS_zz-scan curves of the [c-Al₃]^-, [c-Ga₃]^-, [c-In₃]^- and [c-Tl₃]^- clusters looks like the mirror image of a typical scan of double (σ + π)-aromatic molecules (Figure 4b). The multiple aromaticity of the [c-X₃]^- (X = Al, and Ga) clusters was recently verified by Boldyrev et al. [56,62] through a molecular orbital analysis, and by Dixon et al. [71] by estimating the resonance energy using high-level ab initio quantum chemical calculations. The estimated excitation energies of the lowest T_x,y- and R_z-allowed transitions of the three-membered rings of main group atoms are compiled in

Table 4. Estimated excitation energies (in eV) of the lowest T_x,y- and R_z-allowed transitions of the three-membered rings of main group atoms.

Cluster	Excitations
	a1′ → e′ (σ-type)	a2″ → e″ (π-type)
[c-Al3]-	1.58	3.16
[c-Ga3]-	1.50	2.46
[c-In3]-	1.11	2.62
[c-Tl3]-	1.12	2.45
[c-C3]2-	5.18	7.39
[c-Si3]2-	3.41	3.61
[c-Ge3]2-	2.58	2.08
[c-N3]+	18.04	11.11
[c-P3]+	9.47	5.60
[c-As3]+	8.29	4.63
[c-Sb3]+	6.56	3.75
[c-Bi3]+	6.27	3.23
[c-S3]	9.29	8.00
[c-Se3]	7.98	7.29
[c-Te3]	6.13	7.30a
[c-Po3]	5.11	6.18a

^a e″ → a₂″ transition

.

The lowest T_x,y-allowed transitions of the three-membered rings of main group atoms are the a₁′ → e′ (σ-type) and a₂″ → e″ (π-type) excitations. The coexistence of both the σ- and π-type transitions accounts well for the double (σ + π)-aromatic character of the aforementioned rings. The extent of contribution of the two types of transitions to the induced diatropic ring current is determined by the excitation energies of the σ- and (π-type) transitions (Table 4). Furthermore, removal of two electrons from the [c-Al₃]^- and [c-Ga₃]^- clusters affords [c-Al₃]⁺ and [c-Ga₃]⁺ respectively, where the lowest σ-type transition disappears and therefore the π-diatropic ring current exceeds the σ-type one, a fact imprinted on the NICS_zz-scan curves of [c-Al₃]⁺ and [c-Ga₃]⁺ clusters which exhibit two maxima at a certain distance above and below the molecular plane (Table 3). The estimated excitation energies also illustrate that the induced π-diatropic ring current slightly overwhelms the σ-diatropic ring current in the [c-N₃]⁺ and [c-P₃]⁺ clusters, which unequivocally exhibit double (σ + π)-aromaticity. In effect, the NICS_zz-scan curves (Fig. 4d) for the [c-N₃]⁺ and [c-P₃]⁺ clusters are typical for double (σ + π)-aromatic molecules with slightly stronger π- than σ-aromatic character. According to the estimated excitation energies the [c-As₃]⁺, [c-Sb₃]⁺ and [c-Bi₃]⁺ clusters are doubly (σ + π)-aromatic molecules with slightly higher π- than σ-aromatic character. Finally, the NICS_zz-scan curves of the 18 valence electron [c-S₃], [c-Se₃], [c-Te₃] and [c-Po₃] clusters are also typical for double (σ + π)-aromatics with varying magnitude of π- and σ-aromatic character (Fig. 4e). For [c-S₃] and [c-Se₃] the π-component should be slightly stronger than the σ-one, while the opposite is true for the [c-Te₃] and [c-Po₃] clusters. This is compatible with the excitation energies of the lowest T_x,y-allowed transitions for the neutral triangular chalcogen clusters (Table 4). Computed GIAO-SCF NICS and localized (LMO) NICS analysis of the A_n, (A = O, S, Se; n = 3-6) clusters carried out by Schleyer et al. [72] indicated that the σ-ring electrons are chiefly responsible for the zigzac NICS(0) behavior of the A_n rings with the A₃ clusters being aromatic. It is important to notice that the double (σ + π)-aromaticity of all three-membered rings of main group atoms studied is reflected on the excellent linear correlation of the NICS_zz(0) values with the R_av values of the rings shown in Figure 5.

In summary all triangular main group clusters exhibiting double (σ + π)-aromaticity studied herein are characterized by broad NICS_zz-scan curves with half-band width >3 Å indicating double (σ + π)-aromaticity with comparable contribution to the induced total diatropic ring current from the σ- and π-diatropic ring currents.

3.6. The NICS_zz-scan curves of four- and five-membered rings of main group atoms exhibiting double (σ + π)-aromaticity

Next we examined the NICS_zz-scan curves of four- and five -membered rings of main group atoms, which have been extensively studied and characterized as double (σ + π)-aromatics. The NICS_zz-scan profiles in the form of NICS_zz/NICS_zz^max versus R curves of the [c-B₄]^2-, [c-Al₄]^2-, [c-Ga₄]^2-, [c-In₄]^2-, [c-Tl₄]^2-, [c-Si₄]²⁺ and [c-Mg₄]²⁺ clusters given in Figure 6 are symmetric around the z-axis with maximum at z = 0 and half-band width (except [c-B₄]^2-) greater than 3 Å.

The NICS_zz-scan curves of the [c-Al₄]^4- species as well as of the five-membered pnictogen [c-Pn₅]^- (Pn = N, P, As, Sb, Bi) clusters exhibit two maxima at a certain distance above and below the molecular plane (Figure 6), which are typical for double (σ + π)-aromatics where the π-diatropic ring current overwhelms the σ-type one. The most salient features of the NICS_zz-scan curves of all these clusters along with the R_av values of the rings are compiled in

Table 5. The NICS values (ppm) calculated at the ring center, NICS(0) and 1.0 Å above the ring center, NICS(1), the zz-component of the shielding tensor element, NICS_zz(0) and NICS_zz(1), the R_max (in Å) and the corresponding NICS_zz^max along with the R_av (in Å) for selected four- and five-membered σ-, π- and double (σ + π)-aromatic molecules.

Compound	NICS(0)	NICS(1)	NICSzz(0)	NICSzz(1)	Rmax (Å)	NICSzzmax	ΔNICSa	Rav
[c-B4]2- (D4h)	-44.8	-24.3	-83.4	-47.8	0.0	-83.4	0.0	0.727
[c-Al4]2- (D4h)	-34.4	-27.7	-66.2	-54.9	0.0	-66.2	0.0	1.832
[c-Ga4]2- (D4h)	-38.6	-29.7	-63.3	-57.6	0.0	-63.3	0.0	1.814
[c-In4]2- (D4h)	-22.6	-20.9	-54.3	-47.9	0.0	-54.3	0.0	2.094
[c-Tl4]2- (D4h)	-18.7	-18.7	-48.8	-43.8	0.0	-43.8	0.0	2.144
[c-Al4]4- (D2h)	-6.0	-12.1	-30.0	-32.2	0.9	-32.3	2.3	1.885
[c-Si4]2+ (D4h)	-42.0	-28.8	-78.1	-58.0	0.0	-78.1	0.0	1.633
[c-Mg4]2+ (D4h)	-10.7	-8.6	-31.0	-25.7	0.0	-31.0	0.0	2.213
c-Li2Mg2 (D2h)	4.0	-1.0	-26.6	-23.6	0.0	-26.6	0.0	2.066
[C5H5]- (D5h)	-12.6	-9.6	-16.2	-33.7	0.9	-34.0	17.8	1.204
[c-N5]- (D5h)	-16.5	-16.5	-41.2	-45.2	0.6	-51.9	10.7	1.128
[c-P5]- (D5h)	-16.7	-15.8	-31.8	-39.3	1.0	-39.3	7.5	1.808
[c-As5]- (D5h)	-15.3	-14.7	-22.3	-33.9	1.2	-34.6	12.3	1.993
[c-Sb5]- (D5h)	-9.0	-9.5	-14.9	-21.2	1.4	-22.5	7.6	2.347
[c-Bi5]- (D5h)	-9.3	-9.5	-3.2	-15.2	1.8	-20.8	17.7	2.464

^a Δ_NICS = NICS_zz^max - NICS_zz(0)

.

3.6.1. Magnetotropicity of the [c-E₄]^2- (E = B, Al, Ga, In, Tl), [c-Si₄]²⁺, [c-Mg₄]²⁺, [c-Li₂Mg₂], [c-C₅H₅]^-, and [c-E₅]^- (E = N, P, As, Sb, Bi) rings

Let us now analyze the shapes of the NICS_zz-scan curves of the four- and five-membered rings of main group atoms in relation with the relative ratio of the induced σ- and π-diatropic ring currents. The ground-state (¹A_1g) occupation in D_4h symmetry of 14 valence electrons for the [c-Al₄]^2-, [c-Ga₄]^2-, [c-In₄]^2- and [c-Tl₄]^2- clusters was found to be 1a_1g²1e_u⁴1b_1g²1b_2g²2a_1g²1a_2u². In [c-In₄]^2- cluster there is an inversion of the 1a_2u and 2a_1g. For the [c-B₄]^2- cluster the electron occupation follows a different order, e.g. 1a_1g²1e_u⁴1a_2u²1b_2g²2a_1g²1b_1g². The computed excitation energies of the main translational transitions of [c-Al₄]^2-, [c-Ga₄]^2-, [c-In₄]^2-, [c-Tl₄]^2- and [c-Si₄]²⁺ clusters are given in

Table 6. Estimated excitation energies (in eV) of the main translational σ- and π-type transitions of double (σ + π)-aromatic four-membered [c-Al₄]^2-, [c-Ga₄]^2-, [c-In₄]^2-, [c-Tl₄]^2- and [c-Si₄]²⁺ clusters in D_4h symmetry.

excitation	type	Excitation energy
		[c-Al4]2-	[c-Ga4]2-	[c-In4]2-	[c-Tl4]2-	[c-Si4]2+
a1g → eu	σ-	2.00	0.56	1.92	1.96	3.14
a2u → eg	π-	2.30	1.17	2.07	2.03	3.68
b2g → eu	σ-					4.20
b1g → eu	σ-					4.85

.

Based on the estimated excitation energies of the lowest T_x,y- allowed σ- and π-type 3a_g → 3e_u (1.42 eV) and 1a_2u → 1e_g (4.74 eV) transitions compiled in Table 6 we predict that the [c-B₄]^2- posses rather pure σ-aromatic character, which is also mirrored on the shape of the symmetric sharp (half-band width = 2.3 Å) NICS_zz-scan curve (Figure 6a). The same holds true for the [c-Mg₄]²⁺ and [c-Li₂Mg₂] clusters where the occupation of 6 valence electrons was found to be 1e_u⁴1a_1g² for [c-Mg₄]²⁺ (D_4h) and 1a_g²1b_1u²1b_2u² for [c-Li₂Mg₂] (D_2h). The estimated excitation energies of the lowest T_x,y-allowed transitions found to be 1.12 eV for the σ-type e_u → a_1g in [c-Mg₄]²⁺ (D_4h) and 2.06 eV for the σ-type b_2u → a_1g and 4.92 eV for the π-type a_g → b_3u in [c-Li₂Mg₂] (D_2h) accounts well for the shape of the NICS_zz-scan curves (Fig. 6a). As in the case of the [c-Li₃]⁺ (D_3h) cation the broadening of the NICS_zz-scan curves of [c-Mg₄]²⁺ and [c-Li₂Mg₂] clusters is probably due to the bigger size of the four-membered rings (R_av = 2.213 and 2.066 Å in c-Mg₄]²⁺ and [c-Li₂Mg₂] rings respectively) and/or to the contribution of the intrinsic local circulation of electrons around the nuclei to the diatropic NICS values. The pure σ-aromaticity in the [c-Mg₄]²⁺ and [c-Li₂Mg₂] clusters was previously justified by Alexandrova and Boldyrev [57]. In all these double (σ + π)-aromatic clusters the σ-aromatic character overwhelms the π-aromatic one enforcing the broadening of the NICS_zz-scan curves, which have half-band widths greater than 4 Å (Fig. 6). Of particular importance is the excellent linear correlation of the NICS_zz(0) values of the four-membered rings of 13 group atoms with the R_av values of the rings (Figure 7).

The double (σ + π)-aromaticity of the [c-Al₄]^2- cluster was first introduced in a seminal work published in Science by Boldyrev et al. [73] and subsequently justified by the use of different criteria of aromaticity, such as the induced magnetic field analysis [74], the aromatic ring current shielding (ARCS) [39,75], the ring current maps [76,77,78,79], NICS [80], resonance energy (RE) estimations [39,81], valence bond (VB) calculations [82], bifurcation analysis of electron localization function (ELF) [83], conceptual DFT descriptors [84], and quantum theory of atoms in molecules (QTAIM) calculations [85]. It should also be noticed that Sundholm et al. [75] based on ARCS calculations on the [c-Al₄]^2-, [c-Ga₄]^2-, [c-In₄]^2- and [c-Tl₄]^2- indicated that all these species are aromatic, the calculated ring-current susceptibilities and the (ring radius) found to be 16.2 nA/T (194 pm), 17.2 nA/T (190 pm), 19.3 nA/T (210 pm) and 18.4 nA/T (241 pm) respectively, at the 3-VE+sp2df HF level.

The NICS_zz-scan curve of the [c-Al₄]^4- cluster exhibits two maxima at a distance of 0.9 Å above and below the molecular plane (Fig. 6a), which is typical for double (σ + π)-aromatics where the π-type diatropic ring current exceeds slightly the σ-type one. In effect, this is compatible with the estimated excitation energies of the T_x,y-allowed σ-type a_g → b_2u (1.27 eV) and π-type b_3u → a_g (1.58 eV) and a_g → b_3u (2.05 eV) transitions. Notice the contribution of two T_x,y-allowed π-transitions to the π-diatropic ring current in [c-Al₄]^4- cluster.

The NICS_zz-scan curve of the [c-Si₄]²⁺ cluster, which is isoelectronic to [c-Al₄]^2- one, is typical for double (σ + π)-aromatics where the σ-type diatropic ring current overwhelms remarkably the π-type one. The curve is symmetric around the z-axis with a half-band width of 3.1 Å (Figure 6a). The stronger σ- than the π-aromatic character in [c-Si₄]²⁺ cluster is reflected on the estimated excitation energies (Table 6) for the a_1g → e_u, b_2g → e_u, b_1g → e_u σ-type transitions, compared to the a_2u → e_g π-type transition. The [c-Si₄]²⁺ has previously been characterized as triply aromatic, the triple aromaticity coming from the π_p-z-, σ_p-r- and σ_p-t-MOs (p-z, p-r and p-t are the vertical, radial and tangential 3p AOs of the Si atoms in the ring).

Finally, the NICS_zz-scan curves of the 26 valence electron five-membered pnictogen [c-Pn₅]^- (Pn = N, P, As, Sb, Bi) clusters in D_5h symmetry are typical for double (σ + π)-aromatics with the π-aromatic character exceeding at a different extent the σ-aromatic character (Figure 6b). There is an increase of the π-aromatic character as one goes down in the 15 group pnictogen atom five-membered rings. The NICS_zz-scan curve of the five-membered cyclopentadienyl [c-C₅H₅]^- ligand, a prototype of π-aromatic organic molecule, is also shown in Figure 6b.

The estimated excitation energies of the lowest T_x,y-allowed σ- and π-type transitions of the five-membered pnictogen [c-Pn₅]^- (Pn = N, P, As, Sb, Bi) rings in D_5h symmetry tabulated in

Table 7. Estimated excitation energies (in eV) of the main T_x,y-allowed σ- and π-type transitions of double (σ + π)-aromatic five-membered pnictogen [c-Pn₅]^- (Pn = N, P, As, Sb, Bi) rings and the [c-C₅H₅]^- ligand in D_5h symmetry.

excitation	Type	Excitation energy
		[c-N5]-	[c-P5]-	[c-As5]-	[c-Sb5]-	[c-Bi5]-	[c-C5H5]-
e1′ → a1′	σ-	9.24
e1′ → e2′	σ-	9.82
e2′ → e2′	σ-	9.84
e1″ → e2″	π-	8.20
e1′ → a1′	σ-		5.86
e1′ → e2′	σ-		5.99
e1″ → e2″	π-		4.28	3.58	2.95	2.05
e1′ → a1′	σ-			5.23		4.72
e1′ → e2′	σ-			5.10	5.10	3.84
a1′ → e1′	σ-				5.996	4.99
e1″ → e2″	π-						6.31
e1′ → a1′	σ-						8.88
e2′ → e1′	σ-						8.59

account well for the shape of the NICS_zz-scan curves, which are characteristic for the double (σ + π)-aromaticity of the rings.

It can be seen that all five-membered pnictogen [c-Pn₅]^- (Pn = N, P, As, Sb, Bi) rings are double (σ + π)-aromatic with stronger π- than σ-aromatic character. The relative strength of the π- and σ-aromatic character of the rings can be expressed qualitatively by the ratio of the excitation energies of the π- and σ-type T_x,y-allowed transitions which were found to be 1.13, 1.37, 1.42, 1.73 and 1.88 for the [c-N₅]^-, [c-P₅]^-, [c-As₅]^-, [c-Sb₅]^- and [c-Bi₅]^- respectively. It is interesting to notice that for the series of the five-membered pnictogen [c-Pn₅]^- clusters the NICS_zz(0) values are linearly correlated with the R_av values of the rings (Figure 8) following a similar pattern with that established for other series of aromatic clusters discussed herein.

The double (σ + π)-aromaticity of the pentagonal structures (D_5h symmetry) of the [c-Pn₅]^- clusters was justified by Boldyrev et al. [86] using molecular orbital and photoelectron spectra analysis and compared with the aromaticity of the isovalent cyclopentadiene, [c-C₅H₅]^- ligand. More recently, De Proft et al. [87] calculated the maps for the current density of the [c-P₅]^- and [c-As₅]^- clusters, which showed an apparently classic example of a diatropic ring current, but not solely π current. The current density at z = 1.0a₀ has approximately equal contribution from the four electrons in the 2e₁″ HOMO (π-MO) and the four electrons in the 6e₂′ HOMO-2 (σ-MO). The pattern of the current maps and orbital contributions of the [c-P₅]^- and [c-As₅]^- clusters are compatible with the NICS_zz-scan profiles.

In summary the four- and five-membered rings of main group clusters exhibiting double (σ + π)-aromaticity studied herein are characterized, as in the case of the three-membered rings, by broad NICS_zz-scan curves with half-band width approximately > 3 Å indicating double (σ + π)-aromaticity with comparable contribution to the induced total diatropic ring current from the σ- and π-diatropic ring currents.

3.7. The NICS_zz-scan curves versus the orbital-type of aromaticity in organic molecules

We next tested the predictive power of the NICS_zz-scan curves of the orbital-type of aromaticity for selected organic double (σ + π)- and pure π-aromatic molecules computed at the B3LYP/6-311+G(d,p) level. The NICS_zz-scan profiles in the form of NICS_zz/NICS_zz^max versus R curves of the selected organic molecules are depicted schematically in Figure 9, while the main features of the NICS_zz-scan curves of all molecules along with the R_av values of the rings are compiled in

Table 8. The NICS values (in ppm) calculated at the ring center, NICS(0) and 1.0 Å above the ring center, NICS(1), the zz-component of the shielding tensor element, NICS_zz(0) and NICS_zz(1), the R_max (in Å) and the corresponding NICS_zz^max (ppm) along with R_av (Å) for selected aromatic organic molecules computed at the B3LYP/6-311+G(d,p) level.

Compound	NICS(0)	NICS(1)	NICSzz(0)	NICSzz(1)	Rmax	NICSzzmax	ΔNICSa	Rav
C3H3+ (D3h)	-22.9	-14.7	-31.1	-28.8	0.5	-37.4	6.3	0.787
C6H6 (D6h)	-8.0	-10.2	-14.5	-29.2	1.0	-29.2	14.7	1.395
C6H64- (D6h)	-12.9	-14.1	-22.6	-37.9	1.0	-37.9	15.3	1.404
C6H66- (D6h)	-16.0	-16.9	-26.7	-41.5	1.0	-41.5	14.8	1.395
o-C6H4 (C2v) (S0)	-18.0	-12.7	-30.7	-32.9	0.7	-34.7	4.0	1.369
m-C6H4 (C2v) (S0)	-42.2	-16.3	-64.4	-39.9	0.0	-64.4	0.0	1.225
p-C6H4 (D2h) (S0)	-29.5	-18.7	-63.6	-47.3	0.0	-63.6	0.0	1.388
C6 (D3h)	-24.8	-12.0	-32.4	-28.3	0.4	-32.8	0.4	1.284
C64+ (D3h)	-61.4	-18.5	-69.0	-36.7	0.0	-69.0	0.0	1.356
C6H3+ (D6h)	-42.7	-19.9	-72.4	-51.9	0.0	-72.4	0.0	1.313
C5BH3 (C2v)	-34.5	-16.8	-58.3	-43.2	0.0	-58.3	0.0	1.336
Fulvalene2- (D2)	-12.1	-16.6	-9.9	-28.5	1.0	-28.5	18.6	1.224
Phenalenyl+ (D3h)	-3.6	-7.2	1.1	-17.3	1.3	-19.0	20.1	1.409
Oxazole (Cs)	-11.4	-9.7	-10.2	-27.9	0.9	-28.3	18.1	1.150
c-C7 (C2v)	-16.9	-14.2	-32.3	-33.7	0.7	-35.9	3.6	1.483
c-C8H242+ (D4h)	-16.1	-13.7	-43.8	-39.0	0.0	-43.8	0.0	3.072
c-C10 (D5h)	-29.9	-22.3	-73.0	-59.0	0.0	-73.0	0.0	2.064

^a Δ_NICS = NICS_zz^max - NICS_zz(0)

. The estimated excitation energies of the lowest T_x,y-allowed σ- and π-type transitions of the selected aromatic organic molecules are compiled in

Table 9. Estimated excitation energies (in eV) of the main T_x,y- and R_z-allowed σ- and π-type transitions of selected aromatic organic molecules.

excitation	type	Excitation energies
		C3H3+	C6H6	C6H64-	C6H66-	o-C6H4	m-C6H4	p-C6H4
a2″ → e″	π-	10.03
e′ → a1′	σ-	13.50
e′ → a1′	σ-	13.79
e1g → e2u	π-		6.59	6.32
e1g → e2u	π-		8.78	7.07
e2g → e2g	σ-		10.78	7.82	6.66
a1g → e1u	π-				2.80
e1u → a1g	π-				3.93
a2 → b2	π-					5.12
a1 → b2	π-					5.15
b2 → b2	σ-					8.71
a1 → a2	π-Rz					7.35
b1 → b2	π-Rz					5.11
a2 → b1	π-						5.41
a1 → b1	σ-						5.65
a1 → b2	σ-						7.37
a1 → a2	π-Rz						6.75
b1 → b2	π-Rz						8.46
b2g → au	π-							6.29
b3u → ag	π-							6.93
b2u → ag	σ-							8.00
b1g → ag	π-Rz							3.64
b1u → au	π-Rz							4.76

,

Table 10. Estimated excitation energies (in eV) of the lowest T_x,y- and R_z-allowed σ- and π-type transitions of selected aromatic organic molecules.

excitation	type	Excitation energies
		c-C6	[c-C6]4+	[c-C6H3]+	c-C5BH3	c-C7	c-C10
e′ → a1′	σ-	3.96
e″→ a2″	π-	9.89
a1′ → a2′	σ-Rz	9.14
a1g → e1u	σ-		4.66
e1g → e2u	π-		7.60
a1′ → e′	σ-			6.51
e″→ a2″	π-			10.59
a1 → b2	σ-				5.99
b1 → a1	π-				6.55
b1 → b2	π-Rz				5.98
a1 → b2	σ-					3.40
a1 → b1	π-					3.03
a1 → a2	σ-Rz					6.36
e2′ → e1′	σ-						6.53
e1′ → e2′	σ-						8.35
e1″ → e2″	π-						8.57

and

Table 11. Estimated excitation energies (in eV) of the lowest T_x,y- and R_z-allowed σ- and π-type transitions of selected aromatic organic molecules.

		Excitation energies
excitation	Type	Fulvalene2-	Phenalenyl+	Oxazole	[c-C8H24]2+
a → b2	π-	1.96
b2 → b1	π-	2.00
a → b3	π-	2.69
b2 → b3	π-Rz	2.10
e″ → a1″	π-		3.68
e″ → a2″	π-		6.51
a2″ → a1″	π-Rz		3.58
e1′ → a1′	σ-		10.51
a2′ → a1′	σ-Rz		11.75
a″ → a″	π-			6.64
a′ → a′	σ-			8.52
eu→ b1g	σ-				3.00
eu→ b2g	σ-				3.42

.

3.7.1. The NICS_zz-scan curves and magnetotropicity of the [C₃H₃]⁺, C₆H₆, [C₆H₆]^4-, [C₆H₆]^6-, o-C₆H₄, m-C₆H₄, and p-C₆H₄ molecules

For the [C₃H₃]⁺, C₆H₆, [C₆H₆]^4-, [C₆H₆]^6-, o-C₆H₄ (o-benzyne), m-C₆H₄ (m-benzyne), and p-C₆H₄ (p-benzyne) molecules the estimated excitation energies of the lowest T_x,y- and R_z-allowed σ- and π-type transitions are indicative of their double (σ + π)-aromatic character with varying σ-/π- ratio.

For the [C₃H₃]⁺, C₆H₆, [C₆H₆]^4- and [C₆H₆]^6- molecules the contribution to the ring current from π-delocalized electrons is significantly higher than from σ-delocalized electrons (compare the excitation energies of the σ- and π-type transitions given in Table 9), a fact that is clearly reflected on the shape of the NICS_zz-scan curves (Figure 9a). It is important to notice that the occupation of the antibonding MOs of benzene with four and six electrons affording the anionic [C₆H₆]^4- and [C₆H₆]^6- species, which correspond to local minima in the PES, increase the aromaticity with respect to benzene molecule, despite the bonding from the bonding MOs will be offset by the effect of the occupied antibonding counterparts. This observation illustrates that the dynamic magnetic response of the aromatic species expressed by the NICS values could not be outweighed from the occupation of the antibonding MOs, since the induced ring current from the occupied bonding MOs could not be equal and of opposite sign with that from the occupied antibonding counterparts. However, the anionic [C₆H₆]^4- and [C₆H₆]^6- species should be unstable with respect to electron autodetachment in the gas phase and the results for these metastable species should be approached with caution as it is the case of the “all-metal aromatic” [c-Al₄]^2- dianions [88].

For the singlet o- and m-benzyne isomers and the open-shell singlet p-benzyne the contribution to the ring current from π-delocalized electrons is also higher than from σ-delocalized electrons (compare the excitation energies of the σ- and π-type transitions given in Table 9). However, in the three isomers the induced paratropic current from π-delocalized electrons via the R_z-allowed transitions diminishes the induced π-diatropic ring current, thus rendering the contribution to the induced ring current from σ-delocalized electrons higher than from π-delocalized electrons, which is clearly reflected on the shape of the NICS_zz-scan curves (Figure 9a). It should be noticed that the relative aromaticities of the singlet o- and m-benzyne isomers and the open-shell singlet p-benzyne have previously been probed with a diverse aromaticity indicators [89]. Unlike benzene, the most negative NICS value occurs in the ring planes of the benzynes, which might indicate that the largest diamagnetic ring current is in the molecular plane. In effect, this is mirrored on the shapes of the NICS_zz-scan curves reported herein, which once again correctly predict the orbital-type of aromaticity in the isomeric benzyne molecules.

3.7.2. The NICS_zz-scan curves and magnetotropicity of the c-C₆, [c-C₆]⁴⁺, [c-C₆H₃]⁺, c-C₅BH₃, c-C₇ and c-C₁₀ molecules

The double (σ + π)-aromaticity is also present in the c-C₆, [c-C₆]⁴⁺, [c-C₆H₃]⁺, c-C₅BH₃, c-C₇ and c-C₁₀ molecules. The double (σ + π)-aromaticity of the c-C₆ cluster was first discussed by Schleyer et al. [16]. It was claimed that the c-C₆ cluster is also σ-antiaromatic due to cyclic delocalization of its four lone-pair electrons. In effect, the estimated excitation energies of the lowest T_x,y- and R_z-allowed transitions in the c-C₆ cluster (Table 10) indicate a strong induced σ-diatropic ring current, which is partly outweighed from the induced σ-paratropic ring current due to the σ-R_z transition but still the induced σ-diatropic ring current seems to be stronger than the π-diatropic ring current, which is compatible with the shape of the NICS_zz-scan curve (Figure 9b). The estimated excitation energies of the lowest T_x,y-allowed transitions in the cationic [c-C₆]⁴⁺ species clearly indicate that it is double (σ + π)-aromatic with σ-aromatic character much stronger than the π-aromatic one in line with the shape of the NICS_zz-scan curve (Figure 9b) having a half-band width of 2.1 Å, which is typical for completely σ-aromatic molecules.

The NICS_zz-scan curves of the [c-C₆H₃]⁺ and c-C₅BH₃ have the shape of the typical curves for double (σ + π)-aromatic molecules with σ-aromatic character much stronger than the π-aromatic one. Actually, the estimated excitation energies of the [c-C₆H₃]⁺ and c-C₅BH₃ molecules (Table 10) are indicative of the much stronger σ- than π-aromatic character. For the c-C₅BH₃ molecule the contribution to the diatropic ring current from π-delocalized electrons is further decreased from the induced paratropic ring current due to the π-R_z transition. Hofmann and Berndt [90] showed that the the NICS(1) values of the double aromatic isoelectronic [c-C₆H₃]⁺ and c-C₅BH₃ molecules are significantly larger than that of the benzene molecule due to the contribution of an additional aromatic system, namely the σ-aromatic component.

Akin to c-C₆ (D_3h) the c-C₁₀ (D_5h) cluster was also proved to be a double (σ + π)-aromatic molecule with enhanced aromaticity due to an additional diatropic subsystem (the diatropic in-plane radial system) [16]. This is consistent with the estimated excitation energies of the σ- and π-type transitions (Table 10), which indicate that the σ-aromatic component should be larger than the π-aromatic one and is mirrored on the shape of the NICS_zz-scan curve shown in Figure 9b. Schleyer et al. [16] showed that the c-C₇ (C_2v) cluster has mixed aromatic (π) and antiaromatic (radial) systems. According to our calculations for the c-C₇ (C_2v) cluster the lowest σ- and π-T_x,y-allowed transitions have much lower excitation energies than the σ-R_z-allowed ones (Table 10) and therefore it is expected to be a double(σ + π)-aromatic molecule involving stronger π- than σ-aromatic component, in line with the shape of the NICS_zz-scan curve (Figure 9b). The calculation of the CMO-NICS_zz values for the c-C₇ (C_2v) cluster showed that six σ-type MOs have positive NICS_zz values of totally 122.6 ppm and the remaining eighteen MOs have negative NICS_zz values of totally -154.9 ppm, thus rendering the c-C₇ (C_2v) cluster aromatic. The σ-type MOs (totally fifteen) contribute to diatropic CMO-NICS_zz values by -118.3 ppm and the π-type MOs (HOMO-3,-4,-6) contribute to diatropic CMO-NICS_zz values by -36.6 ppm. It is evident that the net effect would be a stronger π- than σ-aromatic component as it is reflected on the shape of the NICS_zz-scan curve of c-C₇ (C_2v) cluster. It should be noticed that the NICS_zz-scan curve of c-C₇ (C_2v) cluster is similar to that of the benzene molecule. This could be understood on the basis of the CMO-NICS_zz analysis data for benzene, which showed that five σ-type MOs have positive NICS_zz values of totally 85.9 ppm and the remaining sixteen MOs have negative NICS_zz values of totally -100.4 ppm. Among these MOs, nine σ-type MOs contribute to diatropic CMO-NICS_zz values by -39.0 ppm and seven π-type MOs contribute to diatropic CMO-NICS_zz values by -61.4 ppm. It is again evident that the net effect would be the dominant π-aromatic component for benzene.

3.7.3. The NICS_zz-scan curves and magnetotropicity of Fulvalene^2-, Phenalenyl⁺, Oxazole, and [c-C₈H₂₄]²⁺molecules

Very recently Mills and Benish [91] evaluated the aromaticity of the tetrabenzo[5.5]fulvalene dianion through magnetic criteria and found that all rings exhibit appreciable aromaticity. The NICS_zz-scan curve (Figure 9c) justifies the aromaticity of the tetrabenzo[5.5]fulvalene dianion, indicating that it is totally due to the π-aromatic system.

The lowest T_x,y-allowed transitions of the tetrabenzo[5.5]fulvalene dianions are only of the π-type (Table 11). The same holds also true for the 12π electron phenalenyl cation which supports diatropic perimeter ring current. The NICS_zz-scan curve (Figure 9c) of the phenalenyl cation is typical for aromatic molecules exhibiting pure π-aromatic character. Very recently, Fowler et al. [92] using the ipsocentric approach predicted the diatropicity of the phenalenyl cation which arises mainly from the contribution of the doubly degenerate 2e HOMO-2 pair.

Morao and Cosio [25] applied a simple ring current model for describing the in-plane aromaticity in pericyclic reactions to oxazole, a five-membered π-aromatic heterocycle containing two heteroatoms. They found that this kind of aromatic compound can be described using two ring currents. The NICS_zz-scan curve (Figure 9c) of oxazole in conjunction with the estimated excitation energies for the lowest T_x,y-allowed σ- and π-type transitions (Table 11) clearly indicate the double (σ + π)-aromatic character of oxazole involving stronger π- than σ-aromatic component.

Finally, both the shape of the NICS_zz-scan curve (Figure 9c) and the estimated excitation energies for the T_x,y-allowed transitions (Table 11) for the hypothetical [c-C₈H₂₄]²⁺ species with delocalized electrons in a cyclic array of p orbitals arranged tangentially in σ-bonding fashion are in support of the pure σ-aromatic character in line with the ring-current maps calculated by Fowler et al. [93] It should be noted that the [c-C₈H₂₄]²⁺ species correspond to a sixth order saddle point in the PES. The broadening of the NICS_zz-scan curve is what it is expected for an eigth-membered ring with R_av = 3.072 Å.

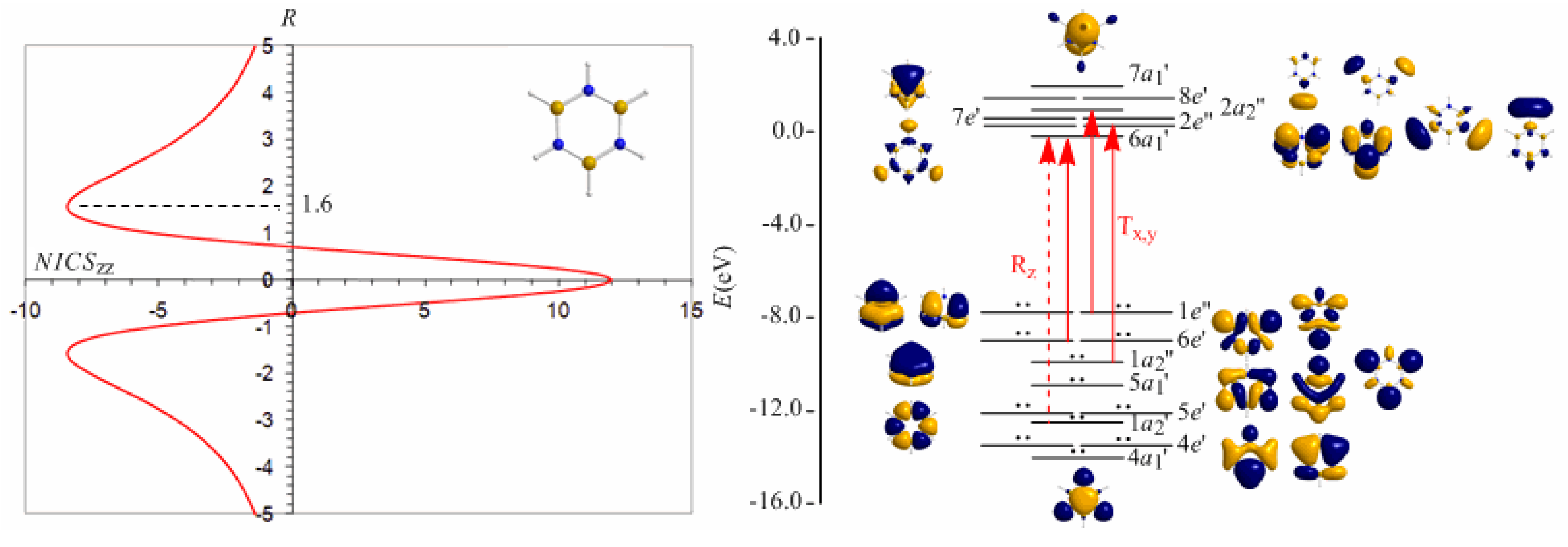
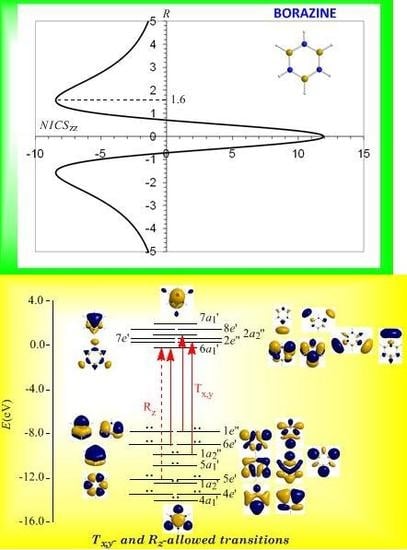
3.8. Predicting the peculiar aromaticity of borazine

Borazine, c-B₃N₃H₆ - the boron-nitrogen six-membered ring compound often referred to as “inorganic benzene - has been subject of controversial discussions with respect to the aromaticity of this molecule. Very recently Islas et al. [94] in their publication entitled Borazine: to be or not to be aromatic based on a detailed analysis of two molecular fields, the induced magnetic field (B^ind) and the electron localization function (ELF) indicated that borazine can be described as a π-aromatic compound but it is not a globally aromatic species, since the delocalized system is not as homogeneous as that of benzene. The induced magnetic field in borazine is weaker than that of benzene but nevertheless pronounced and long-ranged. The NICS_zz-scan curve of borazine shown in Figure 10 clearly demonstrates the long-range π-aromaticity and the in-plane σ-antiaromaticity of borazine. It shows a maximum negative (diatropic) NICS_zz value of -8.4 ppm at z = 1.6 Å above and below the ring and a maximum positive (paratropic) NICS_zz value of 12.0 ppm at z = 0.

The estimated excitation energies of the lowest T_x,y-allowed transitions are for the π-type e″ → a₂″ and a₂″ → e″ excitations 8.57 eV and 10.19 eV respectively and for the σ-type e′ → a₁′ excitation 9.05 eV. The latter is outweighed by the T_x,y-allowed a₂′ → a₁′ transition with excitation energy 12.04 eV. The relatively high excitation energies lead to weak induced π-diatropic ring currents, which nicely explain the substantial decrease of the π-electron delocalization relative to benzene (compare the NICS_zz^max values of -8.4 and -29.2 ppm, for the borazine and benzene molecules, respectively). It is interesting to notice that the local contributions of the σ electrons in borazine according to the calculations carried out by Islas et al. [94] generate a short-range response and a paratropic (antiaromatic) region in the center of the ring, while the π-orbital contribution to B^ind shows the typical response of an aromatic system. This is exactly the topology of the total ring currents represented by the NICS_zz-scan curve of borazine (Figure 10).

3.9. Applying the magnetic NICS_zz-scan criterion to diagnose aromaticity/antiaromaticity in the non-planar corannulene, corannulene dianion and sumarene molecules

The bowl-shaped corannulene, C₂₀H₁₀, corannulene dianion, [C₂₀H₁₀]^2- and sumarene, C₂₁H₁₂, polycyclic hydrocarbons, which share a fullerene substructure, have been studied extensively in recent years [95,96]. Such studies have emphasized the description of their aromaticity and electron interactions on curved surfaces from both the experimental and theoretical perspectives.

The counter-rotating ring currents in coronene and corannulene have been investigated by Jenneskens et al. [97] Very recently Monaco and Zanasi [98] calculated the π current of aromatic polycyclic hydrocarbons placed in a uniform magnetic field and found substantial localization on subunits in some cases. Moreover, Zanasi et al. [99] in their recent publication entitled Magnetic Euripi in Corannulene computed ab initio current densities for corannulene dianion, dication and tetraanion and found large π-ring currents with respect to benzene which undergo remarkable changes in response to variation in the oxidation state. The three corannulene ions along with the neutral species constitute a full set that spans all of the possible patterns of rim and hub circulations. Thus, for the neutral corannulene the magnetotropicity corresponds to paratropic/hub-diatropic/rim pattern, while for the corannulene dianion to diatropic/hub-paratropic/rim pattern.

The NICS_zz-scan curves of corannulene, corannulene dianion and sumanene computed for both the inner (hub) and outer (rim) rings are shown in Figure 11.

The NICS_zz-scan curve of the inner (hub) five-membered ring of corannulene clearly demonstrates the antiaromatic character of the ring (maximum NICS_zz(0.2) value of 54.5 ppm) in line with the computed current-density maps for the σ, π, and total (σ + π) electrons at 1 a₀ above the molecular planes showing a paramagnetic counter-circulation on the inner hub [97,98,99]. The NICS_zz-scan curve of the inner (hub) five-membered ring of corannulene is similar to the NICS_zz-scan curve of the highly antiaromatic cross-conjugated annelated five-membered ring system, acepentalene, [45] corresponding to relatively narrow curve with half-band width of 1.3 Å. On the other hand, the NICS_zz-scan curve of the outer (rim) six-membered rings of corannulene exhibiting two maxima at a certain distance above and below the molecular plane and having NICS_zz(0) value close to zero indicates their pure π-type aromatic character (minimum NICS_zz(1.1) and NICS_zz(-1.3) values of -24.1 and -17.1 ppm respectively), which is also consistent with the computed current-density maps showing a clear diamagnetic circulation around the outer rim [97,98,99]. It is important to be noticed that both the central paramagnetic (paratropic) and the outer diamagnetic (diatropic) induced ring currents are enhanced inside the bowl but depleted on the outside as a result of ring current superpositions.

The NICS_zz-scan curve of the inner (hub) five-membered ring exhibiting two maxima at a certain distance above and below the molecular plane and having NICS_zz(0) value close to zero clearly demonstrate the high π-aromaticity (diatropicity) of the hub (minimum NICS_zz(0.4) value of -293.8 ppm). The NICS_zz-scan curve of the outer (rim) six-membered rings of corannulene dianion is typical of highly in-plane antiaromatic systems [45] (maximum NICS_zz(0.2) value of 216.6 ppm). Notice again that the inner (hub) diamagnetic (diatropic) induced ring current is strongly enhanced inside the bowl but depleted on the outside. It is important to be noted that the NICS_zz-scan curves of corannulene and corannulene dianion correctly predict the paratropic/hub-diatropic/rim and diatropic/hub-paratropic/rim patterns respectively obtained by the ab initio computed probability current densities [99].

The NICS_zz-scan curve of the inner six-membered ring of sumanene indicates paratropicity at the ring center with a maximum NICS_zz(-0.2) value of 15.3 ppm and a long range diatropicity with minimum NICS_zz(1.7) and NICS_zz(-1.9) values of -14.5 and -8.2 ppm respectively. Therefore the inner six-membered ring of sumanene can be considered practically as non aromatic. Notice again the enhancement of the induced diatropic ring currents inside the bowl. The NICS_zz-scan curve of the outer five-membered ring of sumanene (a typical curve for in-plane antiaromatic systems) [45] clearly illustrates the antiaromatic character of the ring, while the NICS_zz-scan curve of the outer six-membered ring of sumanene exhibiting two maxima at a certain distance above and below the molecular plane and having NICS_zz(0) value close to 0 is characteristic of the pure π-aromaticity enhanced inside the bowl (NICS_zz(1.1) = -29.8 ppm), but depleted on the outside (NICS_zz(-1.4) = -16.2 ppm).

The 3D molecular orbital pictures of the MOs participating in the lowest T_x,y- and R_z-allowed transitions in corannulene, corannulene dianion and sumanene are shown in Figure 12.

In the corannulene molecule with C_5v symmetry, the chief contribution to induced diatropic ring current arises from the T_x,y-allowed HOMO,-1 (e₁) → LUMO+2 (a₁) and HOMO,-1 (e₁) → LUMO+3 (a₂) transitions with excitation energies 5.75 and 6.23 eV respectively. On the other hand, the chief contribution to induced paratropic ring current arises from the R_z-allowed HOMO,-1 (e₁) → LUMO,+1 (e₁) transition with excitation energy 4.35 eV. For the corannulene dianion with C_s symmetry, the chief mixed contribution to induced paratropic and diatropic ring currents arises from the lowest R_z- and T_x,y-allowed HOMO (a″) → LUMO (a′), HOMO (a″) → LUMO+1 (a″) and HOMO (a″) → LUMO+2 (a′) transitions with excitation energies 0.83, 0.96 and 1.07 eV respectively.

In the sumanene molecule with C_3v symmetry, the chief contribution to induced diatropic ring current arises from the lowest T_x,y-allowed HOMO,-1 (e) → LUMO+2 (a₁) transition with excitation energy 4.66 eV. Furthermore, the chief contribution to induced paratropic ring current arises from the lowest R_z-allowed HOMO,-1 (e) → LUMO,+1 (e) and HOMO-2 (a₂) → LUMO+2 (a₁) transitions with excitation energies 4.65 and 5.36 eV, respectively.

The estimated excitation energies of the lowest T_x,y- and R_z-allowed transitions of the corannulene, corannulene dianion and sumanene molecules in conjunction with the 3D molecular orbital pictures of the relevant MOs account well for the magnetotropicity patterns derived from the shapes of the NICS_zz-scan curves.

4. Conclusions

To summarize, in the present computational study we showed that the NICS_zz-scan curves computed at the GIAO/B3LYP level in conjunction with symmetry-based selection rules provide efficient diagnostic tools of the orbital-type, σ-, π- and double (σ + π)- of aromaticity in a wide range of aromatic organic and main group inorganic rings.

Thus, the NICS_zz-scan curves of any aromatic molecule exhibiting pure σ-aromatic character are symmetric around the z-axis (perpendicular to the ring plane) with the NICS_zz values decaying rapidly and monotically with respect to R and having a half-band width approximately less than 3 Å. This is the case of σ-aromatics exhibiting s-orbital aromaticity due to cyclic delocalization of two 1s electrons over the three-membered ring in [c-H₃]⁺ and six 1s electrons over the four-, five- and six-membered rings in [c-H₄]^2-, [c-H₅]^- and [c-H₆] species, the [c-B₃]^-, [c-B₃]⁺, [c-B₂C], [c-C₃]²⁺, [c-B₄], [c-B₄]²⁺, [c-B₅]⁺ aromatics with in-plane σ-MOs of Hückel topology constructed from the overlap of the 2p AOs of the ring atoms, the M₃(μ₂-CO)₃(CO)₃ (M = Ni, Pd, Pt) molecules, as well as for the transition states of the concerted transfer of two hydrogen atoms from ethane to ethylene (double group transfer reactions) and the trimerization of acetylene to form benzene, respectively. In all cases, the chief contribution to induced diatropic ring current arises from the lowest T_x,y-allowed occupied-to-unoccupied molecular orbital transitions involving exclusively σ-type molecular orbitals.; the estimated excitation energies accounting for the relative strength of their aromatic character.

A broad NICS_zz-scan curve with half-band width approximately > 3 Å is typical for double (σ + π)-aromatic molecules with comparable contribution to the induced total diatropic ring current from the σ- and π-diatropic ring currents. This is the case of a number of three-membered rings of main group atoms including the [c-Be₃]^2-, [c-Mg₃]^2-, [c-E₃]^- (E = Al, Ga, In, Tl), [c-E₃]^2- (E = C, Si, Ge), [c-E₃]⁺ (E = N, P, As, Sb, Bi) and [c-E₃] (E = S, Se, Te, Po), four-membered rings of main group atoms including the [c-E₄]^2- (E = B, Al, Ga, In, Tl), [c-Si₄]²⁺, [c-Mg₄]²⁺ and [c-Li₂Mg₂] and five-membered rings of main group atoms including the [c-E₅]^- (E = N, P, As, Sb, Bi) and [c-C₅H₅]^-. The magnitude and type (σ-, π-) of the induced diatropic and paratropic ring currents are determined by the excitation energies of the lowest T_x,y- and R_z-allowed occupied → unoccupied molecular orbital transitions respectively with the coexistence of both the σ- and π-type transitions accounting well for the double (σ + π)-aromatic character of all these clusters.

The NICS_zz-scan curves exhibiting two maxima at a certain distance above and below the molecular plane are typical for double (σ + π)-aromatics where the π-diatropic ring current overwhelms the σ-type one. In the absence of any contribution from the σ-diatropic ring current the NICS_zz(0) value is close to zero and the molecule is a pure π-aromatic molecule. This is the case of a vast number of organic aromatic molecules. Again the estimated excitation energies of the lowest T_x,y- and R_z-allowed occupied → unoccupied molecular orbital transitions are indicative of the double (σ + π)-aromatic character of the organic aromatics accounting well for the observed varying σ-/π- ratio.

Finally, the NICS_zz-scan curves correctly predict the peculiar aromaticity of borazine and the magnetotropicity (diatropicity/paratropicity) patterns of the non-planar corannulene, corannulene dianion and sumanene.