Heterogeneous Nucleation and Growth of CaCO3 on Calcite (104) and Aragonite (110) Surfaces: Implications for the Formation of Abiogenic Carbonate Cements in the Ocean
Abstract
1. Introduction
2. Materials and Methods
2.1. Sample Preparation
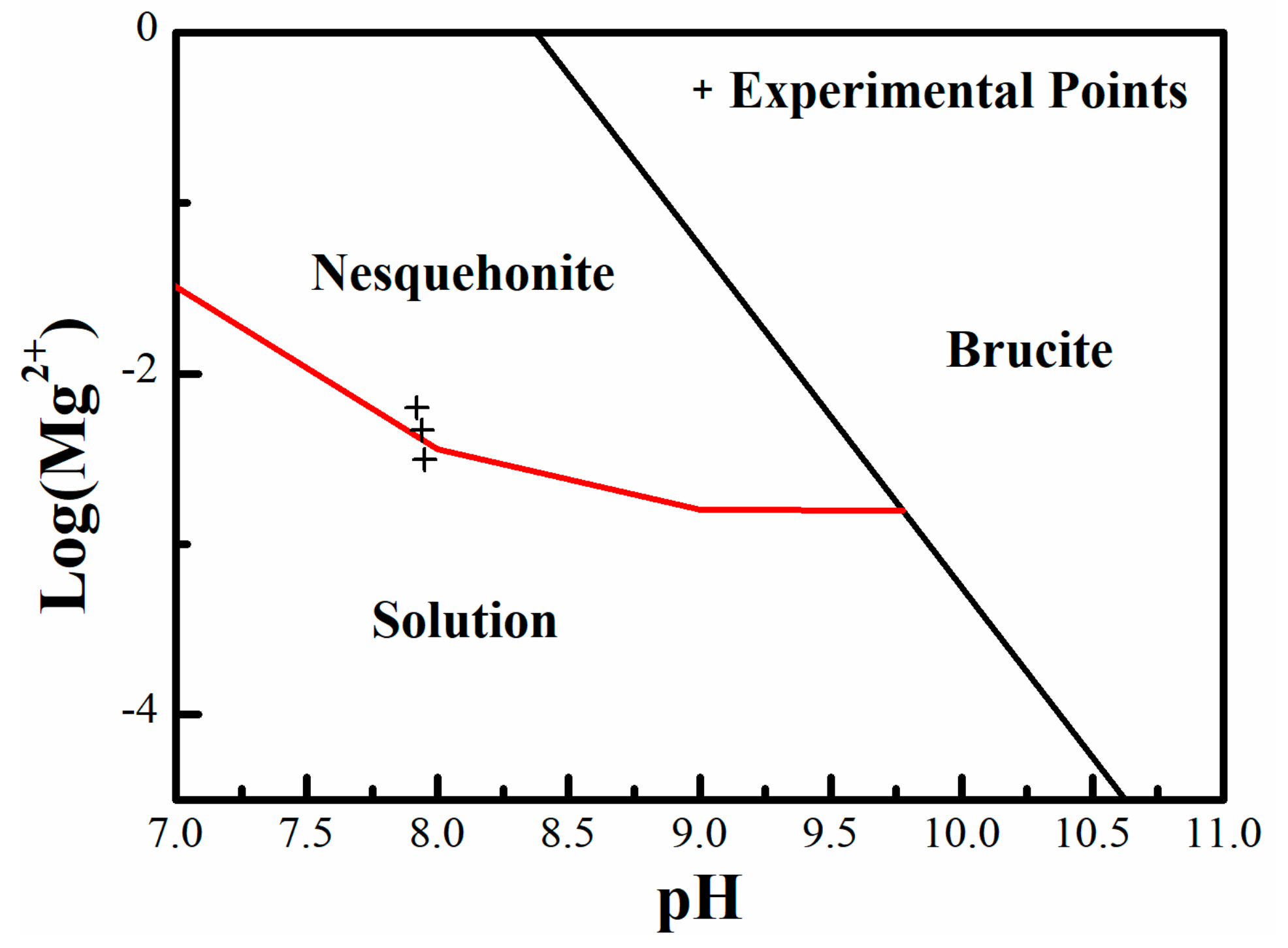
2.2. Solution Preparation
2.3. Growth Experiments Measured by In Situ AFM
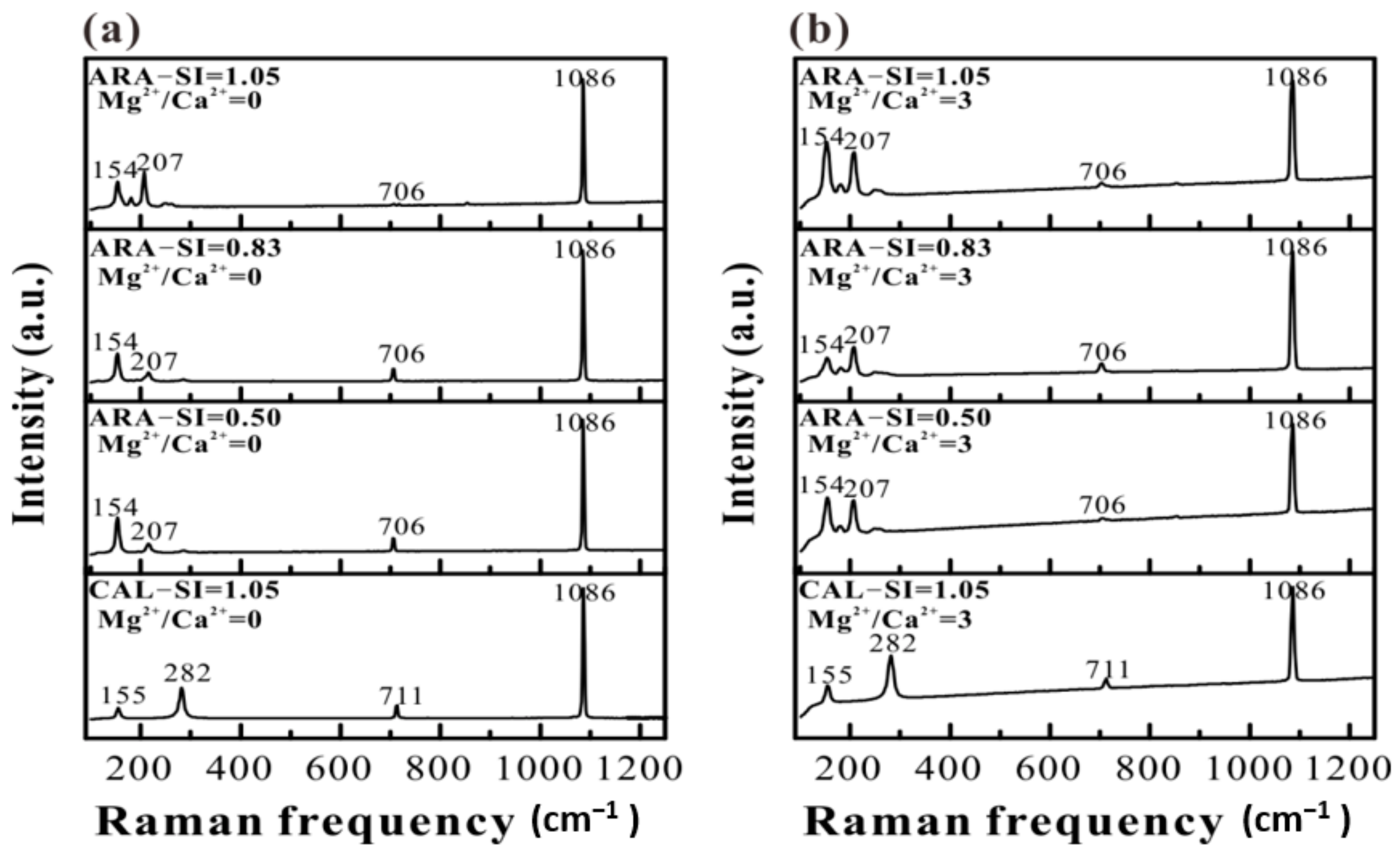
2.4. SEM, XRD and Raman Spectroscopy Analysis
3. Results
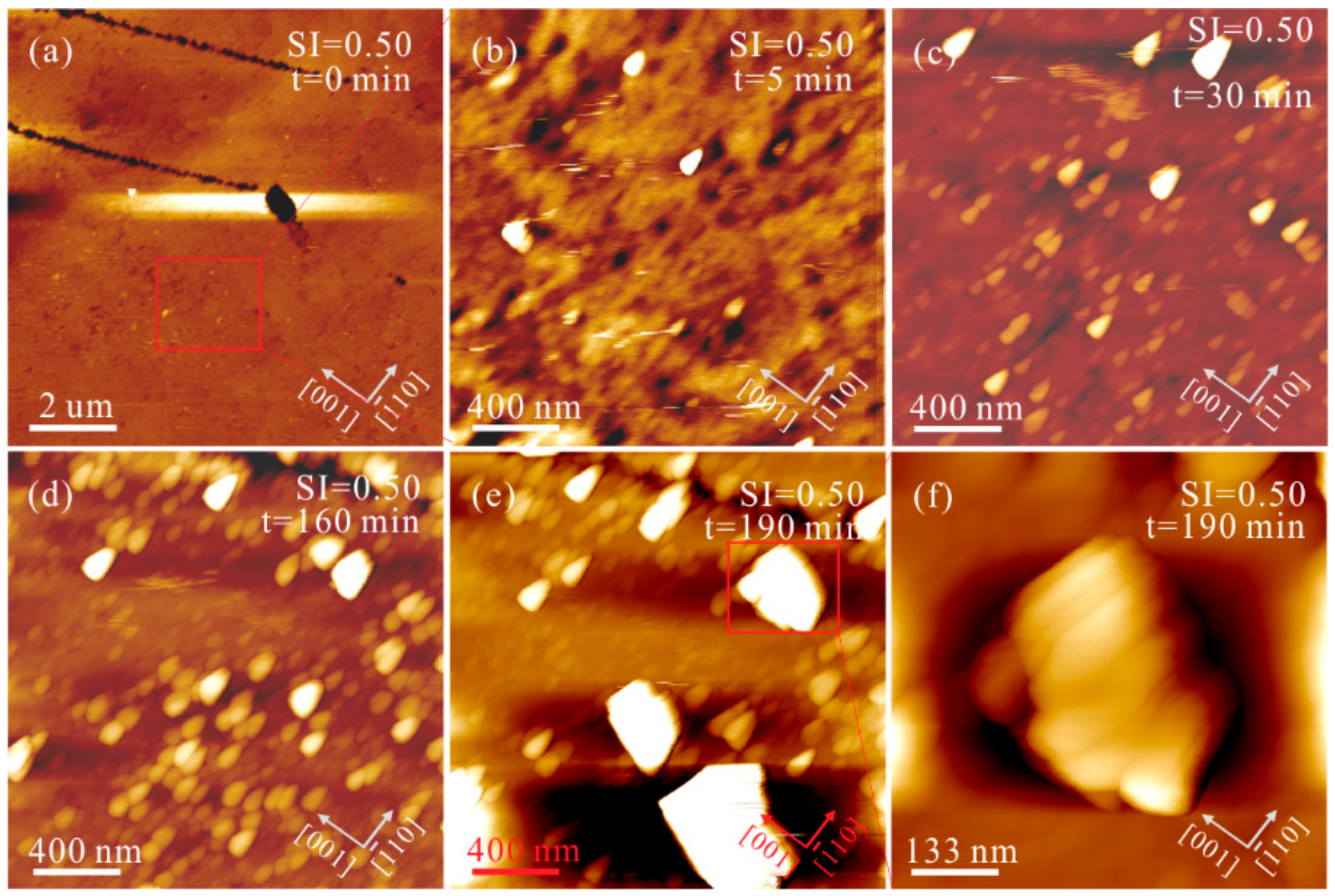
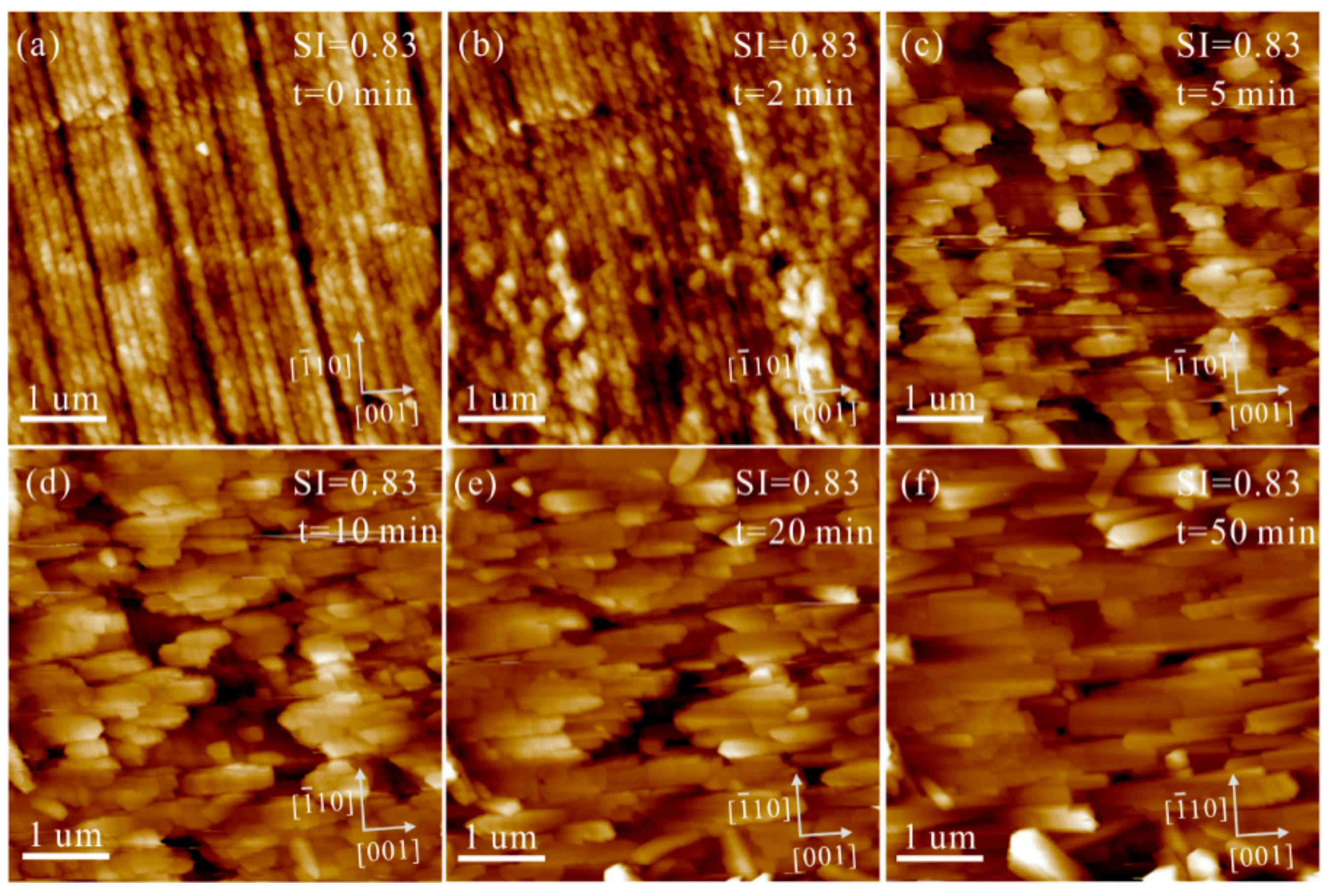
3.1. The Heterogeneous Nucleation and Growth of CaCO3 on Calcite (104) Surfaces
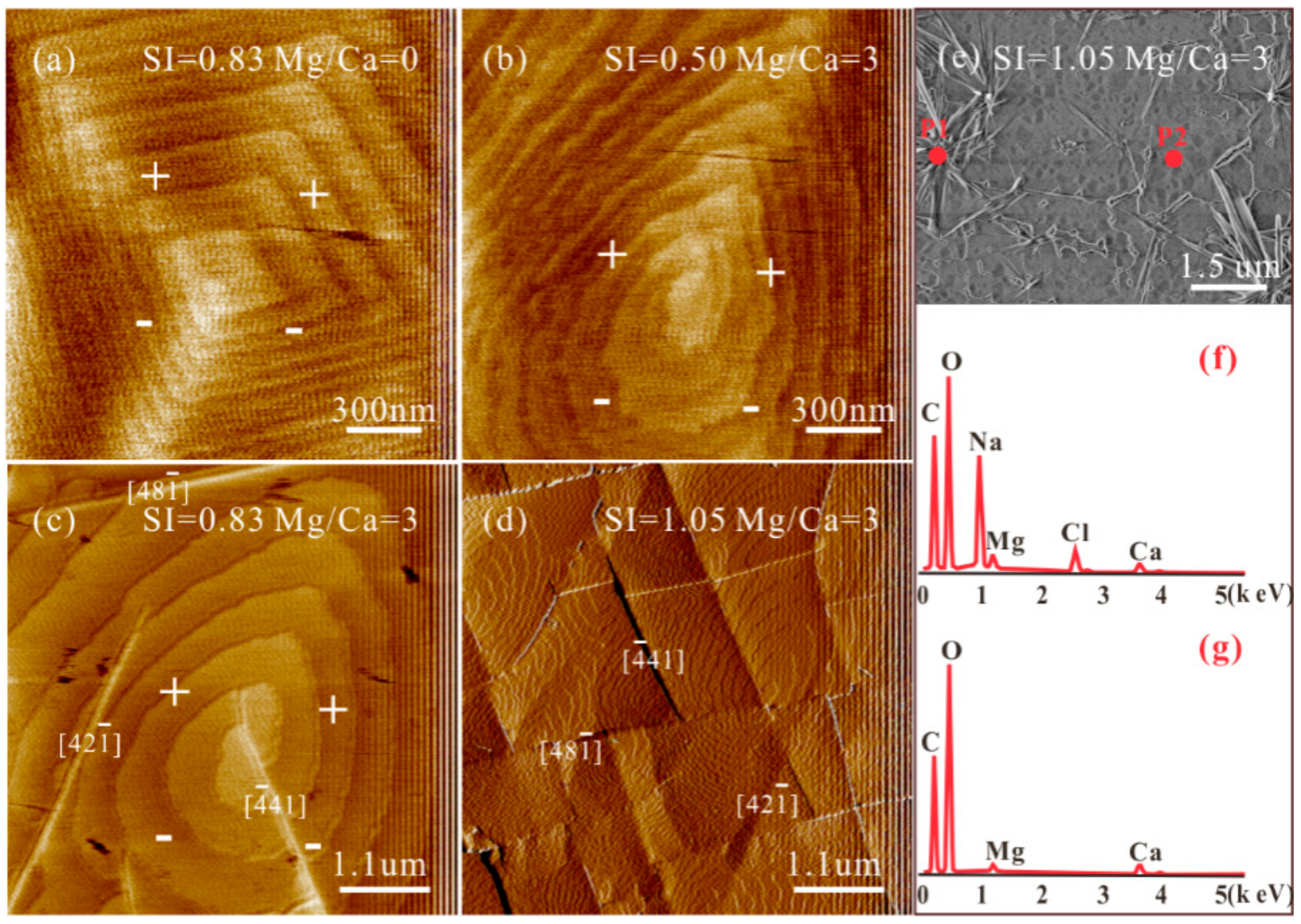
3.2. The Heterogeneous Nucleation and Growth of CaCO3 on Aragonite (110) Surfaces
3.3. Mineral Phases of CaCO3 Dependent on Calcite (104) and Aragonite (110) Substrates
4. Discussion
4.1. Different Crystallization Pathways of Heterogeneous Nucleation and Growth of CaCO3 on Calcite (104) and Aragonite (110) Surfaces
4.2. Effects of Mg2+ and Saturation States on the Heterogeneous Nucleation and Growth of CaCO3 on Calcite (104) and Aragonite (110) Surfaces
4.3. Comparison with Previous Studies
4.3.1. Calcite Precipitation
4.3.2. Aragonite Precipitation
4.4. Implications for the Formation of Abiogenic Carbonate Cements
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Morse, J.W.; Arvidson, R.S.; Lüttge, A. Calcium Carbonate Formation and Dissolution. Chem. Rev. 2007, 107, 342–381. [Google Scholar] [CrossRef]
- Lasaga, A.C. Kinetic Theory in the Earth Sciences; Princeton University Press: Princeton, NJ, USA, 1998; Available online: https://www.jstor.org/stable/j.ctt7zvgxm (accessed on 23 March 2019).
- Teng, H.H.; Dove, P.M.; De Yoreo, J.J. Kinetics of calcite growth: Surface processes and relationships to macroscopic rate laws. Geochim. Cosmochim. Acta 2000, 64, 2255–2266. [Google Scholar] [CrossRef]
- Davies, G.R. Former magnesian calcite and aragonite submarine cements in upper Paleozoic reefs of the Canadian Arctic: A summary. Geology 1977, 5, 11–15. [Google Scholar] [CrossRef]
- Kendall, A.C. Fascicular-optic calcite; a replacement of bundled acicular carbonate cements. J. Sediment. Res. 1977, 47, 1056–1062. [Google Scholar] [CrossRef]
- Reid, R.P.; Macintyre, I.G. Carbonate recrystallization in shallow marine environments: A widespread diagenetic process forming micritized grains. J. Sediment. Res. 1998, 68, 928–946. [Google Scholar] [CrossRef]
- Walter, L.M. Relative efficiency of carbonate dissolution and precipitation during diagenesis: A progress report on the role of solution chemistry. Soc. Econ. Paleontol. Mineral. Spec. Publ. 1986, 38, 1–12. [Google Scholar]
- Burton, E.A.; Walter, L.M. The role of pH in phosphate inhibition of calcite and aragonite precipitation rates in seawater. Geochim. Cosmochim. Acta 1990, 54, 797–808. [Google Scholar] [CrossRef]
- Renard, F.; Montes-Hernandez, G.; Ruiz-Agudo, E.; Putnis, C.V. Selenium incorporation into calcite and its effect on crystal growth: An atomic force microscopy study. Chem. Geol. 2013, 340, 151–161. [Google Scholar] [CrossRef]
- Xu, M.; Riechers, S.L.; Ilton, E.S.; Du, Y.; Kovarik, L.; Varga, T.; Arey, B.W.; Qafoku, O.; Kerisit, S. Manganese-calcium intermixing facilitates heteroepitaxial growth at the (101¯4) calcite-water interface. Chem. Geol. 2017, 470, 152–163. [Google Scholar] [CrossRef]
- Dobberschutz, S.; Nielsen, M.R.; Sand, K.K.; Civioc, R.; Bovet, N.; Stipp, S.L.S.; Andersson, M.P. The mechanisms of crystal growth inhibition by organic and inorganic inhibitors. Nat. Commun. 2018, 9, 1–6. [Google Scholar] [CrossRef]
- Schott, J.; Pokrovsky, O.S.; Oelkers, E.H. The Link between Mineral Dissolution/Precipitation Kinetics and Solution Chemistry. Rev. Mineral. Geochem. 2009, 70, 207–258. [Google Scholar] [CrossRef]
- Burton, E.A. Controls on marine carbonate cement mineralogy: Review and reassessment. Chem. Geol. 1993, 105, 163–179. [Google Scholar] [CrossRef]
- Sunagawa, I. Crystals: Growth, Morphology and Perfection; Cambridge University Press: Cambridge, UK, 2005; pp. 1–308. [Google Scholar]
- Stack, A.G.; Grantham, M.C. Growth rate of calcite steps as a function of aqueous calcium-to-carbonate ratio: Independent attachment and detachment of calcium and carbonate ions. Cryst. Growth Des. 2010, 10, 1409–1413. [Google Scholar] [CrossRef]
- Larsen, K.; Bechgaard, K.; Stipp, S.L.S. The effect of the Ca2+ to CO32− activity ratio on spiral growth at the calcite (101¯4) surface. Geochim. Cosmochim. Acta 2010, 74, 2099–2109. [Google Scholar] [CrossRef]
- Sand, K.K.; Tobler, D.J.; Larsen, K.K.; Makovicky, E.; Andersson, M.P.; Stipp, S.L.S. Calcite growth kinetics: Dependence on saturation index, Ca2+:CO32−activity ratio, and surface atomic structure. Cryst. Growth Des. 2016, 16, 3602–3612. [Google Scholar] [CrossRef]
- Gutjahr, A.; Dabringhaus, H.; Lacmann, R. Studies of the growth and dissolution kinetics of the CaCO3 polymorphs calcite and aragonite II. The influence of divalent cation additives on the growth and dissolution rates. J. Cryst. Growth 1996, 158, 310–315. [Google Scholar] [CrossRef]
- Dove, P.M.; De Yoreo, J.J.; Weiner, S. Biomineralization: Reviews in Mineralogy and Geochemistry; Mineralogical Society of America; Geochemical Society: Chantilly, VA, USA; Washington, DC, USA, 2003; pp. 1–377. [Google Scholar]
- Xu, J.; Wang, J.; Hong, M.; Teng, H.H. Solution-chemistry control of Mg2+-calcite interaction mechanisms: Implication for biomineralization. Am. Mineral. 2016, 101, 1104–1112. [Google Scholar] [CrossRef]
- Mavromatis, V.; Goetschl, K.E.; Grengg, C.; Konrad, F.; Purgstaller, B.; Dietzel, M. Barium partitioning in calcite and aragonite as a function of growth rate. Geochim. Cosmochim. Acta 2018, 237, 65–78. [Google Scholar] [CrossRef]
- Berner, R.; Westrich, J.; Graber, R.; Smith, J.; Martens, C. Inhibition of Aragonite precipitation from Supersaturated Seawater: A Laboratory and Field Study. Am. J. Sci. 1978, 278, 816–837. [Google Scholar] [CrossRef]
- Morse, J.W.; Mucci, A. Composition of carbonate overgrowths produced on Iceland spar calcite crystals buried in Bahamian carbonate-rich sediments. Sediment. Geol. 1984, 40, 287–291. [Google Scholar] [CrossRef]
- Lioliou, M.G.; Paraskeva, C.A.; Koutsoukos, P.G.; Payatakes, A.C. Heterogeneous nucleation and growth of calcium carbonate on calcite and quartz. J. Colloid Interface Sci. 2007, 308, 421–428. [Google Scholar] [CrossRef] [PubMed]
- Ren, D.; Feng, Q.; Bourrat, X. Effects of additives and templates on calcium carbonate mineralization in vitro. Micron 2011, 42, 228–245. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; Fernandez-Martinez, A.; Lee, B.; Waychunas, G.A.; Jun, Y.S. Interfacial energies for heterogeneous nucleation of calcium carbonate on mica and quartz. Environ. Sci. Technol. 2014, 48, 5745–5753. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; Jun, Y.S. The apparent activation energy and pre-exponential kinetic factor for heterogeneous calcium carbonate nucleation on quartz. Commun. Chem. 2018, 1, 1–9. [Google Scholar] [CrossRef]
- Lin, Y.; Hu, Q.; Chen, J.; Ji, J.; Teng, H.H. Formation of metastable CaCO3 polymorphs in the presence of oxides and silicates. Cryst. Growth Des. 2009, 9, 4634–4641. [Google Scholar] [CrossRef]
- Xu, H.; Zhou, M.; Fang, Y.; Teng, H.H. Effect of mica and hematite (001) surfaces on the precipitation of calcite. Minerals 2018, 8, 17. [Google Scholar] [CrossRef]
- Sun, W.; Jayaraman, S.; Chen, W.; Persson, K.A.; Ceder, G. Nucleation of metastable aragonite CaCO3 in seawater. Proc. Natl. Acad. Sci. USA 2015, 112, 3199–3204. [Google Scholar] [CrossRef]
- Li, L.; Fijneman, A.J.; Kaandorp, J.A.; Aizenberg, J.; Noorduin, W.L. Directed nucleation and growth by balancing local supersaturation and substrate/nucleus lattice mismatch. Proc. Natl. Acad. Sci. USA 2018, 115, 3575–3580. [Google Scholar] [CrossRef]
- Bathurst, R.G. Diagenetically enhanced bedding in argillaceous platform limestones: Stratified cementation and selective compaction. Sedimentology 1987, 34, 749–778. [Google Scholar] [CrossRef]
- Berner, R.A. The role of magnesium in the crystal growth of calcite and aragonite from sea water. Geochim. Cosmochim. Acta 1975, 39, 489–504. [Google Scholar] [CrossRef]
- Mucci, A.; Morse, J.W. The incorporation of Mg2+ and Sr2+ into calcite overgrowths: Influences of growth rate and solution composition. Geochim. Cosmochim. Acta 1983, 47, 217–233. [Google Scholar] [CrossRef]
- Zhong, S.; Mucci, A. Calcite and aragonite precipitation from seawater solutions of various salinities: Precipitation rates and overgrowth compositions. Chem. Geol. 1989, 78, 283–299. [Google Scholar] [CrossRef]
- Teng, H.H.; Dove, P.M.; Orme, C.A.; Yoreo, J.J.D. Thermodynamics of Calcite Growth: Baseline for Understanding Biomineral Formation. Science 1998, 282, 724–727. [Google Scholar] [CrossRef] [PubMed]
- Hong, M.; Xu, J.; Teng, H.H. Evolution of calcite growth morphology in the presence of magnesium: Implications for the dolomite problem. Geochim. Cosmochim. Acta 2016, 172, 55–64. [Google Scholar] [CrossRef]
- Sinha, S.; Rez, P. Distortions of the calcite and aragonite atomic structures from interstitial water. Mater. Chem. Phys. 2015, 157, 56–62. [Google Scholar] [CrossRef]
- Pytkowicz, R.M. Rates of inorganic calcium carbonate nucleation. J. Geol. 1965, 73, 196–199. [Google Scholar] [CrossRef]
- Lippmann, F. Sedimentary Carbonate Minerals; Springer: Berlin/Heidelberg, Germany, 1973; pp. 1–233. [Google Scholar]
- Gustafsson, J.P. Visual MINTEQ 3.0 User Guide; KTH, Department of Land and Water Recources: Stockholm, Sweden, 2011; Available online: http://www2.lwr.kth.se/English/OurSoftware/vminteq/contact.html (accessed on 20 March 2012).
- Tang, H.; Xian, H.; He, H.; Wei, J.; Liu, H.; Zhu, J.; Zhu, R. Kinetics and mechanisms of the interaction between the calcite (10.4) surface and Cu2+-bearing solutions. Sci. Total Environ. 2019, 668, 602–616. [Google Scholar] [CrossRef]
- Van Driessche, A.E.; Kellermeier, M.; Benning, L.G.; Gebauer, D. New Perspectives on Mineral Nucleation and Growth: From Solution Precursors to Solid Materials; Springer: Cham, Switzerland, 2016; pp. 1–380. [Google Scholar]
- De Yoreo, J.J.; Gilbert, P.U.P.A.; Sommerdijk, N.A.J.M.; Penn, R.L.; Whitelam, S.; Joester, D.; Zhang, H.; Rimer, J.D.; Navrotsky, A.; Banfield, J.F.; et al. Crystallization by particle attachment in synthetic, biogenic, and geologic environments. Science 2015, 349, 498–507. [Google Scholar] [CrossRef]
- Gránásy, L.; Pusztai, T.; Tegze, G.; Warren, J.A.; Douglas, J.F. Growth and form of spherulites. Phys. Rev. E 2005, 72, 11605. [Google Scholar] [CrossRef]
- Cubillas, P.; Köhler, S.; Prieto, M.; Causserand, C.; Oelkers, E.H. How do mineral coatings affect dissolution rates? An experimental study of coupled CaCO3 dissolution—CdCO3 precipitation. Geochim. Cosmochim. Acta 2005, 69, 5459–5476. [Google Scholar] [CrossRef]
- Zhou, G.T.; Yao, Q.Z.; Ni, J.; Jin, G. Formation of aragonite mesocrystals and implication for biomineralization. Am. Mineral. 2009, 94, 293–302. [Google Scholar] [CrossRef]
- Nielsen, M.H.; Aloni, S.; De Yoreo, J.J. In situ TEM imaging of CaCO3 nucleation reveals coexistence of direct and indirect pathways. Science 2014, 345, 1158–1162. [Google Scholar] [CrossRef] [PubMed]
- Walker, J.M.; Marzec, B.; Nudelman, F. Solid-State Transformation of Amorphous Calcium Carbonate to Aragonite Captured by CryoTEM. Angew. Chem. Int. Edit. 2017, 56, 11740–11743. [Google Scholar] [CrossRef] [PubMed]
- Alberstein, R.G.; Tezcan, F.A. Observations of the birth of crystals. Nature 2018, 556, 41–42. [Google Scholar] [CrossRef] [PubMed]
- Sethmann, I.; Wang, J.; Becker, U.; Putnis, A. Strain-induced segmentation of magnesian calcite thin films growing on a calcite substrate. Cryst. Growth Des. 2010, 10, 4319–4326. [Google Scholar] [CrossRef]
- Shannon, R.D. Revised effective ionic-radii and systematic studies of interatomic distances in halides and chalcogenides. Acta Crystallogr. Sect. A. 1976, 32, 751–767. [Google Scholar] [CrossRef]
- Elhadj, S.; De Yoreo, J.J.; Hoyer, J.R.; Dove, P.M. Role of molecular charge and hydrophilicity in regulating the kinetics of crystal growth. Proc. Natl. Acad. Sci. USA 2006, 103, 19237–19242. [Google Scholar] [CrossRef]
- Nemeth, P.; Mugnaioli, E.; Gemmi, M.; Czuppon, G.; Demeny, A.; Spoetl, C. A nanocrystalline monoclinic CaCO3 precursor of metastable aragonite. Sci. Adv. 2018, 4, eaau6178. [Google Scholar] [CrossRef]
- Wasylenki, L.E.; Dove, P.M.; Wilson, D.S.; De Yoreo, J.J. Nanoscale effects of strontium on calcite growth: An in situ AFM study in the absence of vital effects. Geochim. Cosmochim. Acta 2005, 69, 3017–3027. [Google Scholar] [CrossRef]
- Teng, H.H.; Dove, P.M.; DeYoreo, J.J. Reversed calcite morphologies induced by microscopic growth kinetics: Insight into biomineralization. Geochim. Cosmochim. Acta 1999, 63, 2507–2512. [Google Scholar] [CrossRef]
- De Yoreo, J.J.; Vekilov, P.G. Principles of crystal nucleation and growth. In Biomineralization: Reviews in Mineralogy and Geochemistry; Mineralogical Society of America; Geochemical Society: Chantilly, VA, USA; Washington, DC, USA, 2003; Volume 54, pp. 57–93. [Google Scholar]
- Davis, K.J.; Dove, P.M.; De Yoreo, J.J. The role of Mg2+ as an impurity in calcite growth. Science 2000, 290, 1134–1137. [Google Scholar] [CrossRef] [PubMed]
- Astilleros, J.; Fernández-Díaz, L.; Putnis, A. The role of magnesium in the growth of calcite: An AFM study. Chem. Geol. 2010, 271, 52–58. [Google Scholar] [CrossRef]
- Mass, T.; Giuffre, A.J.; Sun, C.Y.; Stifler, C.A.; Frazier, M.J.; Neder, M.; Tamura, N.; Stan, C.V.; Marcus, M.A.; Gilbert, P.U. Amorphous calcium carbonate particles form coral skeletons. Proc. Natl. Acad. Sci. USA 2017, 114, 7670–7678. [Google Scholar] [CrossRef]
- Luo, C.; Xie, L.; Wang, X.X. In vitro growth of aragonite crystal on nacre surface. Key Eng. Mat. 2007, 330, 1335–1338. [Google Scholar] [CrossRef]
- Gal, A.; Wirth, R.; Kopka, J.; Fratzl, P.; Faivre, D.; Scheffel, A. Macromolecular recognition directs calcium ions to coccolith mineralization sites. Science 2016, 353, 590–593. [Google Scholar] [CrossRef] [PubMed]
- Branson, O.; Bonnin, E.A.; Perea, D.E.; Spero, H.J.; Zhu, Z.; Winters, M.; Hönisch, B.; Russell, A.D.; Fehrenbacher, J.S.; Gagnon, A.C. Nanometer-scale chemistry of a calcite biomineralization template: Implications for skeletal composition and nucleation. Proc. Natl. Acad. Sci. USA 2016, 113, 12934–12939. [Google Scholar] [CrossRef]
- Gal, A.; Wirth, R.; Barkay, Z.; Eliaz, N.; Scheffel, A.; Faivre, D. Templated and self-limiting calcite formation directed by coccolith organic macromolecules. Chem. Commun. 2017, 53, 7740–7743. [Google Scholar] [CrossRef]






| SIcal | Mg2+/Ca2+ | MgCl2 (mM) | CaCl2 (mM) | NaHCO3 (mM) | NaCl (mM) | pH | IS (M) |
|---|---|---|---|---|---|---|---|
| 0.50 | 0 | 0 | 1.00 | 10.00 | 90.00 | 7.98 | 0.10 |
| 3 | 3.15 | 1.05 | 10.50 | 85.00 | 7.95 | 0.10 | |
| 0.83 | 0 | 0 | 1.50 | 15.00 | 89.00 | 7.97 | 0.11 |
| 3 | 4.65 | 1.55 | 15.50 | 70.00 | 7.94 | 0.10 | |
| 1.05 | 0 | 0 | 2.00 | 20.00 | 85.00 | 7.96 | 0.11 |
| 3 | 6.30 | 2.10 | 21.00 | 60.00 | 7.92 | 0.10 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, H.; Wu, X.; Xian, H.; Zhu, J.; Wei, J.; Liu, H.; He, H. Heterogeneous Nucleation and Growth of CaCO3 on Calcite (104) and Aragonite (110) Surfaces: Implications for the Formation of Abiogenic Carbonate Cements in the Ocean. Minerals 2020, 10, 294. https://doi.org/10.3390/min10040294
Tang H, Wu X, Xian H, Zhu J, Wei J, Liu H, He H. Heterogeneous Nucleation and Growth of CaCO3 on Calcite (104) and Aragonite (110) Surfaces: Implications for the Formation of Abiogenic Carbonate Cements in the Ocean. Minerals. 2020; 10(4):294. https://doi.org/10.3390/min10040294
Chicago/Turabian StyleTang, Hongmei, Xiao Wu, Haiyang Xian, Jianxi Zhu, Jingming Wei, Hongmei Liu, and Hongping He. 2020. "Heterogeneous Nucleation and Growth of CaCO3 on Calcite (104) and Aragonite (110) Surfaces: Implications for the Formation of Abiogenic Carbonate Cements in the Ocean" Minerals 10, no. 4: 294. https://doi.org/10.3390/min10040294
APA StyleTang, H., Wu, X., Xian, H., Zhu, J., Wei, J., Liu, H., & He, H. (2020). Heterogeneous Nucleation and Growth of CaCO3 on Calcite (104) and Aragonite (110) Surfaces: Implications for the Formation of Abiogenic Carbonate Cements in the Ocean. Minerals, 10(4), 294. https://doi.org/10.3390/min10040294






