Gas Migration Patterns with Different Borehole Sizes in Underground Coal Seams: Numerical Simulations and Field Observations
Abstract
:1. Introduction
2. Geological Settings
3. Numerical Modelling
3.1. Basic Theory and Model Settings
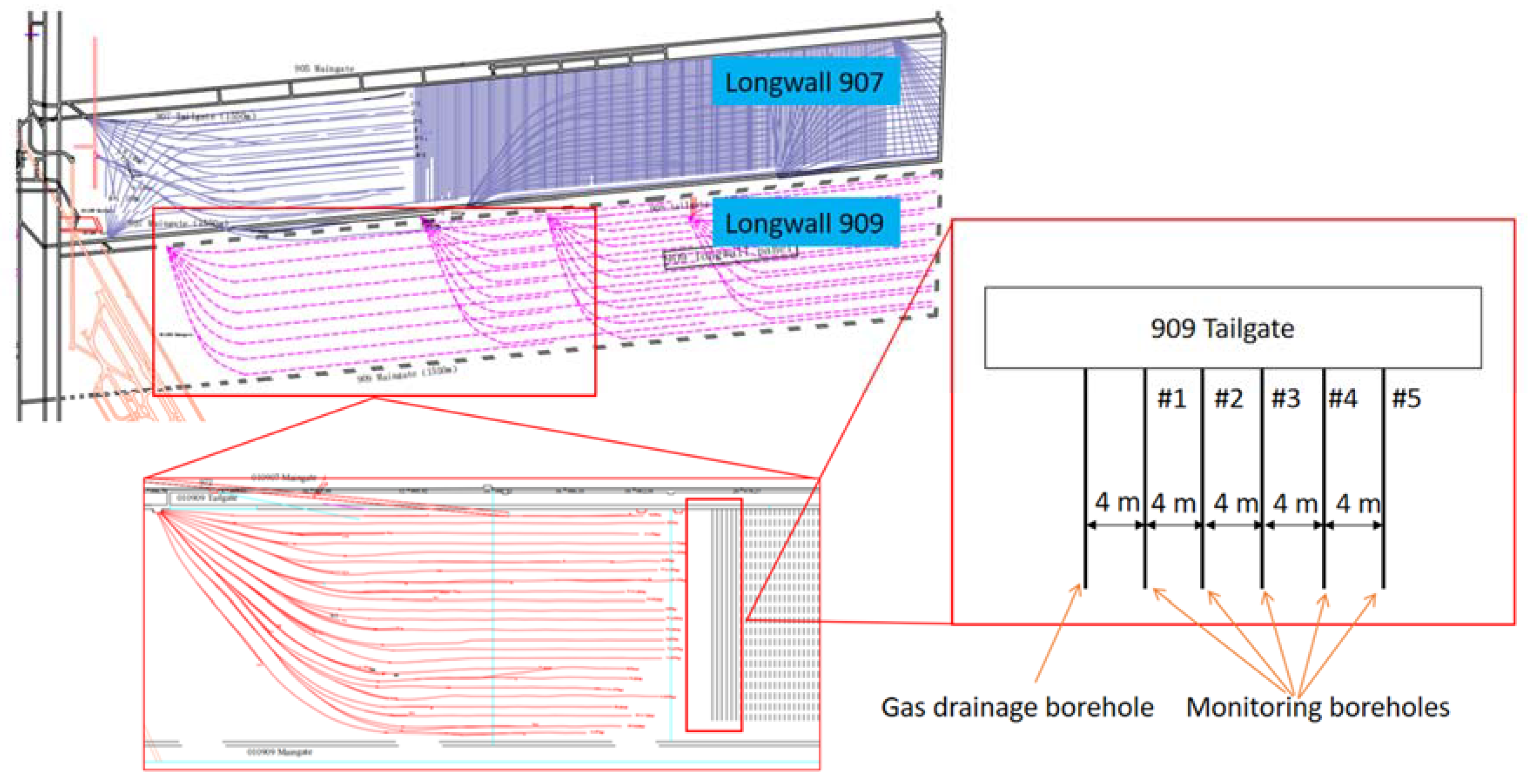
3.2. Field Data Collection
3.3. Illustration of Borehole Layout
3.4. Model Description and Selection of Parameters
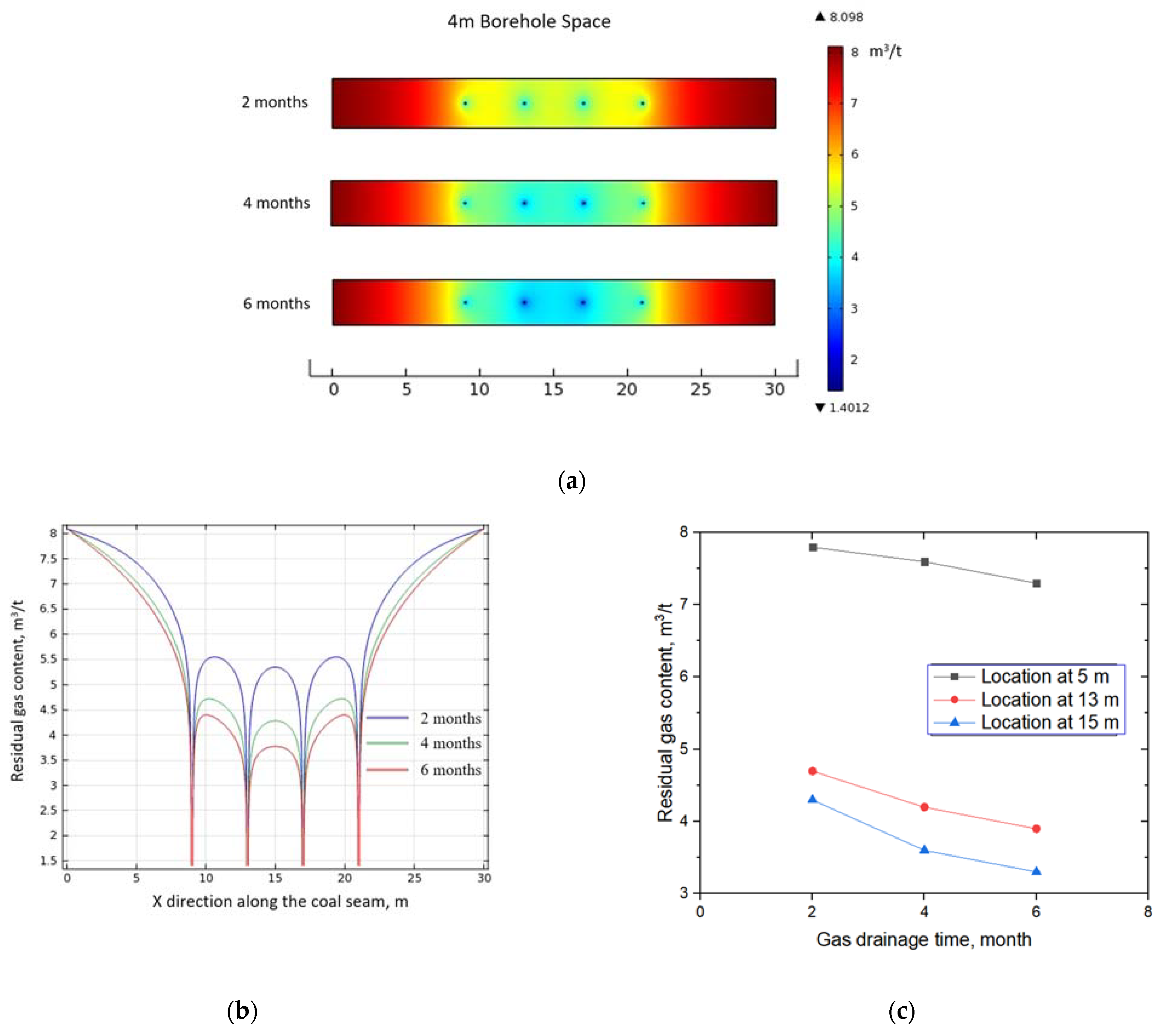
3.5. Results
3.5.1. Directional Borehole Simulations
3.5.2. Normal Parallel Borehole Simulations
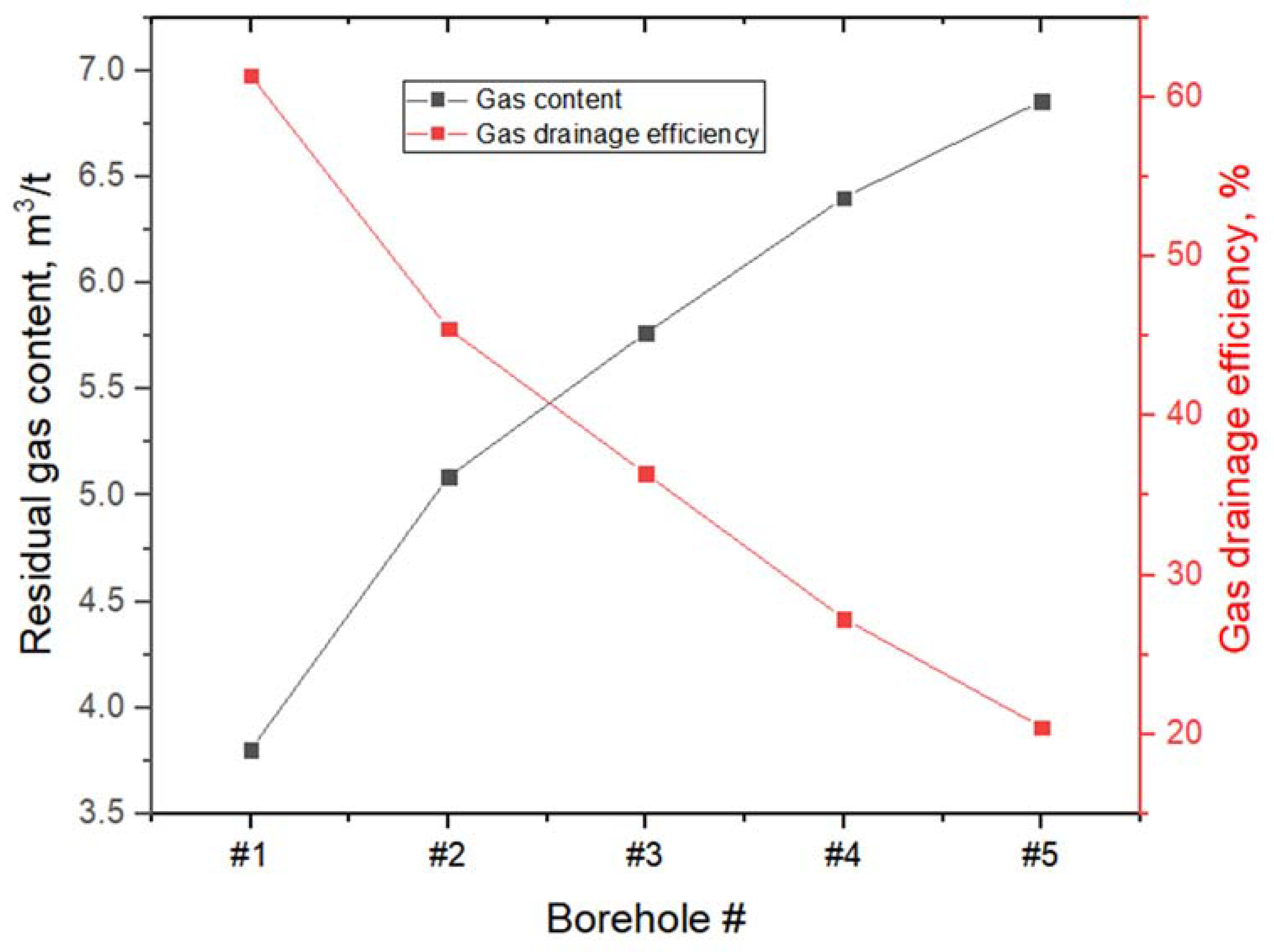
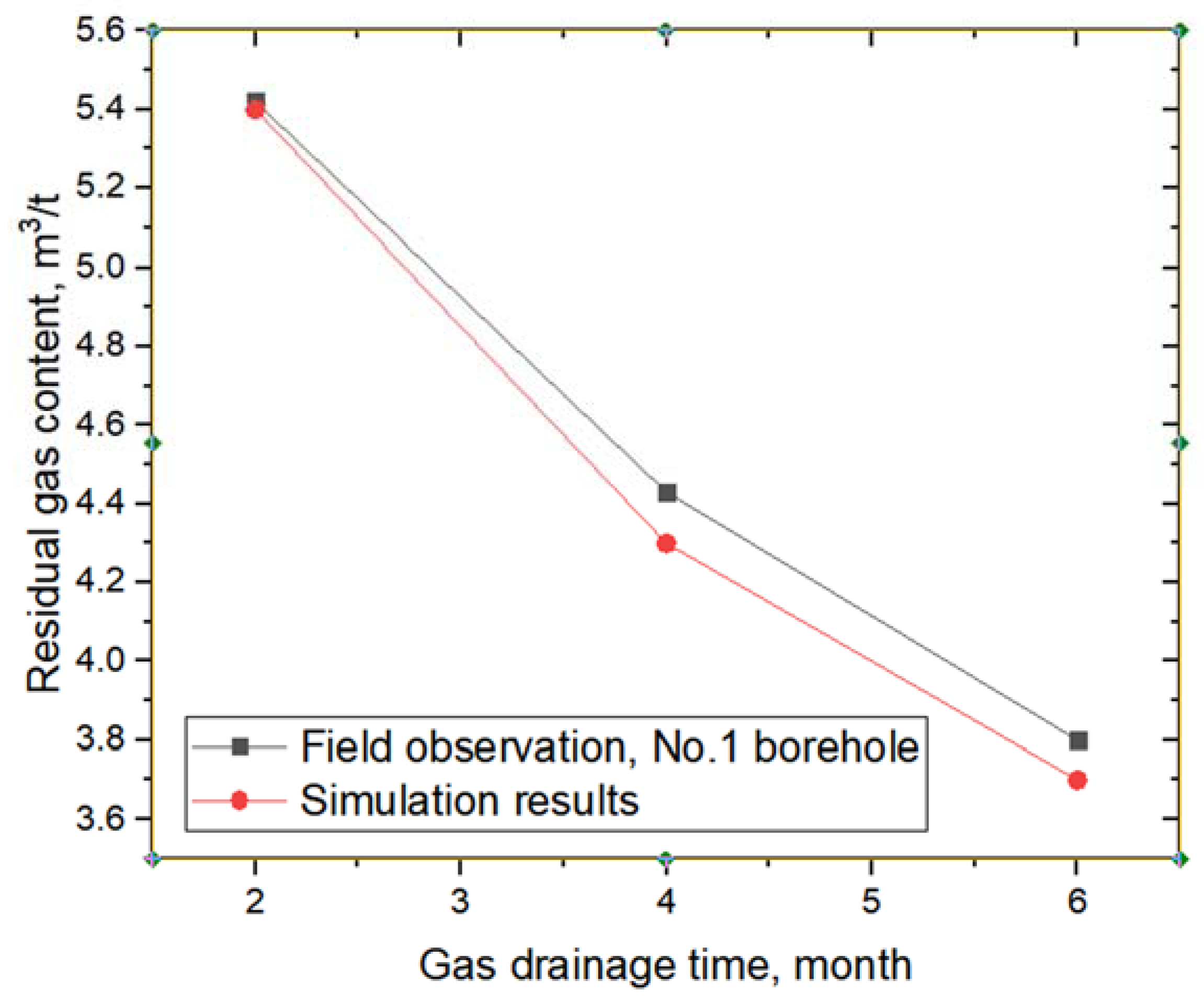
4. Field Observations and Discussions
5. Future Studies for CO2 Geo-Sequestration in Coal Seams
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hamawand, I.; Yusaf, T.; Hamawand, S.G. Coal seam gas and associated water: A review paper. Renew. Sustain. Energy Rev. 2013, 22, 550–560. [Google Scholar] [CrossRef]
- Fleming, D.A.; Measham, T.G. Local economic impacts of an unconventional energy boom: The coal seam gas industry in Australia. Aust. J. Agric. Resour. Econ. 2015, 59, 78–94. [Google Scholar] [CrossRef] [Green Version]
- Yang, W.; Lu, C.; Si, G.; Lin, B.; Jiao, X. Coal and gas outburst control using uniform hydraulic fracturing by destress blasting and water-driven gas release. J. Nat. Gas Sci. Eng. 2020, 79, 103360. [Google Scholar] [CrossRef]
- Fan, C.; Li, S.; Luo, M.; Du, W.; Yang, Z. Coal and gas outburst dynamic system. Int. J. Min. Sci. Technol. 2017, 27, 49–55. [Google Scholar] [CrossRef]
- Zhang, G.; Ranjith, P.G.; Wu, B.; Perera, M.S.A.; Haque, A.; Li, D. Synchrotron X-ray tomographic characterization of microstructural evolution in coal due to supercritical CO2 injection at in-situ conditions. Fuel 2019, 255, 115696. [Google Scholar] [CrossRef]
- Zhang, G.; Ranjith, P.G.; Fu, X.; Li, X. Pore-fracture alteration of different rank coals: Implications for CO2 sequestration in coal. Fuel 2021, 289, 119801. [Google Scholar] [CrossRef]
- Si, G.; Durucan, S.; Jamnikar, S.; Lazar, J.; Abraham, K.; Korre, A.; Shi, J.-Q.; Zavšek, S.; Mutke, G.; Lurka, A. Seismic monitoring and analysis of excessive gas emissions in heterogeneous coal seams. Int. J. Coal Geol. 2015, 149, 41–54. [Google Scholar] [CrossRef]
- Salmachi, A.; Rajabi, M.; Wainman, C.; Mackie, S.; McCabe, P.; Camac, B.; Clarkson, C. History, Geology, In Situ Stress Pattern, Gas Content and Permeability of Coal Seam Gas Basins in Australia: A Review. Energies 2021, 14, 2651. [Google Scholar] [CrossRef]
- Viney, N.R.; Post, D.A.; Crosbie, R.S.; Peeters, L.J.M. Modelling the impacts of future coal mining and coal seam gas extraction on river flows: A methodological framework. J. Hydrol. 2021, 596, 126–144. [Google Scholar] [CrossRef]
- Zhang, Q.; Zheng, J.; Zhang, B.; Linga, P. Coal mine gas separation of methane via clathrate hydrate process aided by tetrahydrofuran and amino acids. Appl. Energy 2021, 287, 116576. [Google Scholar] [CrossRef]
- Zhao, P.; Zhuo, R.; Li, S.; Lin, H.; Shu, C.-M.; Laiwang, B.; Jia, Y.; Suo, L. Fractal characteristics of gas migration channels at different mining heights. Fuel 2020, 271, 117479. [Google Scholar] [CrossRef]
- Zhao, P.; Liu, H.; Ho, C.-H.; Li, S.; Liu, Y.; Lin, H.; Yan, M. Evaluating Methane Adsorption Characteristics of Coal-Like. Materials 2020, 13, 751. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhao, P.; Zhuo, R.; Li, S.; Shu, C.-M.; Jia, Y.; Lin, H.; Chang, Z.; Ho, C.-H.; Laiwang, B.; Xiao, P. Fractal characteristics of methane migration channels in inclined coal seams. Energy 2021, 225, 120127. [Google Scholar] [CrossRef]
- Qin, L.; Wang, P.; Li, S.; Lin, H.; Wang, R.; Wang, P.; Ma, C. Gas adsorption capacity changes in coals of different ranks after liquid nitrogen freezing. Fuel 2021, 292, 120404. [Google Scholar] [CrossRef]
- Xie, W.; Wang, M.; Duan, H. Adsorption Characteristics and Controlling Factors of CH4 on Coal-Measure Shale, Hedong Coalfield. Minerals 2021, 11, 63. [Google Scholar] [CrossRef]
- Keles, C.; Tang, X.; Schlosser, C.; Louk, A.K.; Ripepi, N.S. Sensitivity and history match analysis of a carbon dioxide “huff-and-puff” injection test in a horizontal shale gas well in Tennessee. J. Nat. Gas Sci. Eng. 2020, 77, 103226. [Google Scholar] [CrossRef]
- Zhang, Z.; Liu, G.; Cao, Y.; Lin, J.; Jin, Y.; Xian, B.; Lv, R.; Zhang, Z. Experimental Investigation of CS2 Extraction to Enhance the Permeability of Coal. Transp. Porous Media 2021, 136, 899–922. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, F.; Bai, Q. Underground space utilization of coalmines in China: A review of underground water reservoir construction. Tunn. Undergr. Space Technol. 2021, 107, 103657. [Google Scholar] [CrossRef]
- Cao, W.; Shi, J.-Q.; Durucan, S.; Si, G.; Korre, A. Gas-driven rapid fracture propagation under unloading conditions in coal and gas outbursts. Int. J. Rock Mech. Min. Sci. 2020, 130, 104325. [Google Scholar] [CrossRef]
- Lin, J.; Ren, T.; Wang, G.; Nemcik, J. Simulation investigation of N2-injection enhanced gas drainage: Model development and identification of critical parameters. J. Nat. Gas Sci. Eng. 2018, 55, 30–41. [Google Scholar] [CrossRef]
- Huo, B.; Jing, X.; Fan, C.; Han, Y. Numerical investigation of flue gas injection enhanced underground coal seam gas drainage. Energy Sci. Eng. 2019, 7, 3204–3219. [Google Scholar] [CrossRef]
- Dong, J.; Cheng, Y.; Pan, Z. Comparison of transient and pseudo-steady diffusion of methane in coal and implications for coalbed methane control. J. Pet. Sci. Eng. 2020, 184, 106543. [Google Scholar] [CrossRef]
- Xia, T.; Zhou, F.; Gao, F.; Kang, J.; Liu, J.; Wang, J. Simulation of coal self-heating processes in underground methane-rich coal seams. Int. J. Coal Geol. 2015, 141–142, 1–12. [Google Scholar] [CrossRef]
- Zhang, Y.; Underschultz, J.; Langhi, L.; Mallants, D.; Strand, J. Numerical modelling of coal seam depressurization during coal seam gas production and its effect on the geomechanical stability of faults and coal beds. Int. J. Coal Geol. 2018, 195, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Zhang, C.; Yu, L.; Feng, R.; Zhang, Y.; Zhang, G. A Numerical Study of Stress Distribution and Fracture Development above a Protective Coal Seam in Longwall Mining. Processes 2018, 6, 146. [Google Scholar] [CrossRef] [Green Version]
- Lin, J.; Ren, T.; Cheng, Y.; Nemcik, J.; Wang, G. Cyclic N2 injection for enhanced coal seam gas recovery: A laboratory study. Energy 2019, 188, 116115. [Google Scholar] [CrossRef]
- Lin, J.; Ren, T.; Wang, G.; Booth, P.; Nemcik, J. Experimental investigation of N2 injection to enhance gas drainage in CO2-rich low permeable seam. Fuel 2018, 215, 665–674. [Google Scholar] [CrossRef]
- Bai, G.; Su, J.; Zhang, Z.; Lan, A.; Zhou, X.; Gao, F.; Zhou, J. Effect of CO2 injection on CH4 desorption rate in poor permeability coal seams: An experimental study. Energy 2022, 238, 121674. [Google Scholar] [CrossRef]
- Awan, F.U.R.; Keshavarz, A.; Akhondzadeh, H.; Al-Anssari, S.; Al-Yaseri, A.; Nosrati, A.; Ali, M.; Iglauer, S. Stable Dispersion of Coal Fines during Hydraulic Fracturing Flowback in Coal Seam Gas Reservoirs—An Experimental Study. Energy Fuels 2020, 34, 5566–5577. [Google Scholar] [CrossRef]
- Sampath, K.H.S.M.; Perera, M.S.A.; Elsworth, D.; Ranjith, P.G.; Matthai, S.K.; Rathnaweera, T.; Zhang, G. Effect of coal maturity on CO2-based hydraulic fracturing process in coal seam gas reservoirs. Fuel 2019, 236, 179–189. [Google Scholar] [CrossRef]
- Zhao, P.; Liu, H.; Li, S.; Lin, H.; Jia, Y.; Yan, M.; Yuan, M.; Lin, J. Experimental Investigation of the Adsorption Characteristics of Mixed Coal and Variations of Specific Surface Areas before and after CH4 Adsorption. Appl. Sci. 2019, 9, 524. [Google Scholar] [CrossRef] [Green Version]
- Saghafi, A. Potential for ECBM and CO2 storage in mixed gas Australian coals. Int. J. Coal Geol. 2010, 82, 240–251. [Google Scholar] [CrossRef]
- Suchowerska, A.M.; Merifield, R.S.; Carter, J.P. Vertical stress changes in multi-seam mining under supercritical longwall panels. Int. J. Rock Mech. Min. Sci. 2013, 61, 306–320. [Google Scholar] [CrossRef]
- Du, F.; Wang, K. Unstable failure of gas-bearing coal-rock combination bodies: Insights from physical experiments and numerical simulations. Process Saf. Environ. Prot. 2019, 129, 264–279. [Google Scholar] [CrossRef]
- Kiyama, T.; Nishimoto, S.; Fujioka, M.; Xue, Z.; Ishijima, Y.; Pan, Z.; Connell, L.D. Coal swelling strain and permeability change with injecting liquid/supercritical CO2 and N2 at stress-constrained conditions. Int. J. Coal Geol. 2011, 85, 56–64. [Google Scholar] [CrossRef]
- Sarda, S.; Jeannin, L.; Bourbiaux, B. Hydraulic characterization of fractured reservoirs: Simulation on discrete fracture models. In Proceedings of the SPE Reservoir Simulation Symposium, Houston, TX, USA, 11–14 February 2001. [Google Scholar]
- Fan, C.; Elsworth, D.; Li, S.; Zhou, L.; Yang, Z.; Song, Y. Thermo-hydro-mechanical-chemical couplings controlling CH4 production and CO2 sequestration in enhanced coalbed methane recovery. Energy 2019, 173, 1054–1077. [Google Scholar] [CrossRef]
- Fan, C.; Li, S.; Elsworth, D.; Han, J.; Yang, Z. Experimental investigation on dynamic strength and energy dissipation characteristics of gas outburst—Prone coal. Energy Sci. Eng. 2020, 8, 1015–1028. [Google Scholar] [CrossRef]
- Birkedal, K.A.; Hauge, L.P.; Graue, A.; Ersland, G. Transport mechanisms for CO2-CH4 exchange and safe CO2 storage in hydrate-bearing sandstone. Energies 2015, 8, 4073–4095. [Google Scholar] [CrossRef] [Green Version]
- Tambach, T.J.; Mathews, J.P.; van Bergen, F. Molecular Exchange of CH4 and CO2 in Coal: Enhanced Coalbed Methane on a Nanoscale. Energy Fuels 2009, 23, 4845–4847. [Google Scholar] [CrossRef]
- Liu, Q.; Chu, P.; Zhu, J.; Cheng, Y.; Wang, D.; Lu, Y.; Liu, Y.; Xia, L.; Wang, L. Numerical assessment of the critical factors in determining coal seam permeability based on the field data. J. Nat. Gas Sci. Eng. 2020, 74, 103098. [Google Scholar] [CrossRef]
- Yang, X.; Wang, G.; Du, F.; Jin, L.; Gong, H. N2 injection to enhance coal seam gas drainage (N2-ECGD): Insights from underground field trial investigation. Energy 2022, 239, 122247. [Google Scholar] [CrossRef]
- Saghafi, A.; Faiz, M.; Roberts, D. CO2 storage and gas diffusivity properties of coals from Sydney Basin, Australia. Int. J. Coal Geol. 2007, 70, 240–254. [Google Scholar] [CrossRef]
- Guo, H.; Ni, X.; Wang, Y.; Du, X.; Yu, T.; Feng, R. Experimental Study of CO2-Water-Mineral Interactions and Their Influence on the Permeability of Coking Coal and Implications for CO2-ECBM. Minerals 2018, 8, 117. [Google Scholar] [CrossRef] [Green Version]
- Lin, J.; Ren, T.; Cheng, Y.; Nemcik, J. Laboratory quantification of coal permeability reduction effect during carbon dioxide injection process. Process Saf. Environ. Prot. 2021, 148, 638–649. [Google Scholar] [CrossRef]
- Sander, R.; Connell, L.D.; Camilleri, M.; Pan, Z. CH4, CO2, N2 diffusion in Bowen Basin (Australia) coal: Relationship between sorption kinetics of coal core and crushed coal particles. J. Nat. Gas Sci. Eng. 2020, 81, 103468. [Google Scholar] [CrossRef]
- Sander, R.; Connell, L.D.; Pan, Z.; Camilleri, M.; Heryanto, D.; Lupton, N. Core flooding experiments of CO2 enhanced coalbed methane recovery. Int. J. Coal Geol. 2014, 131, 113–125. [Google Scholar] [CrossRef]
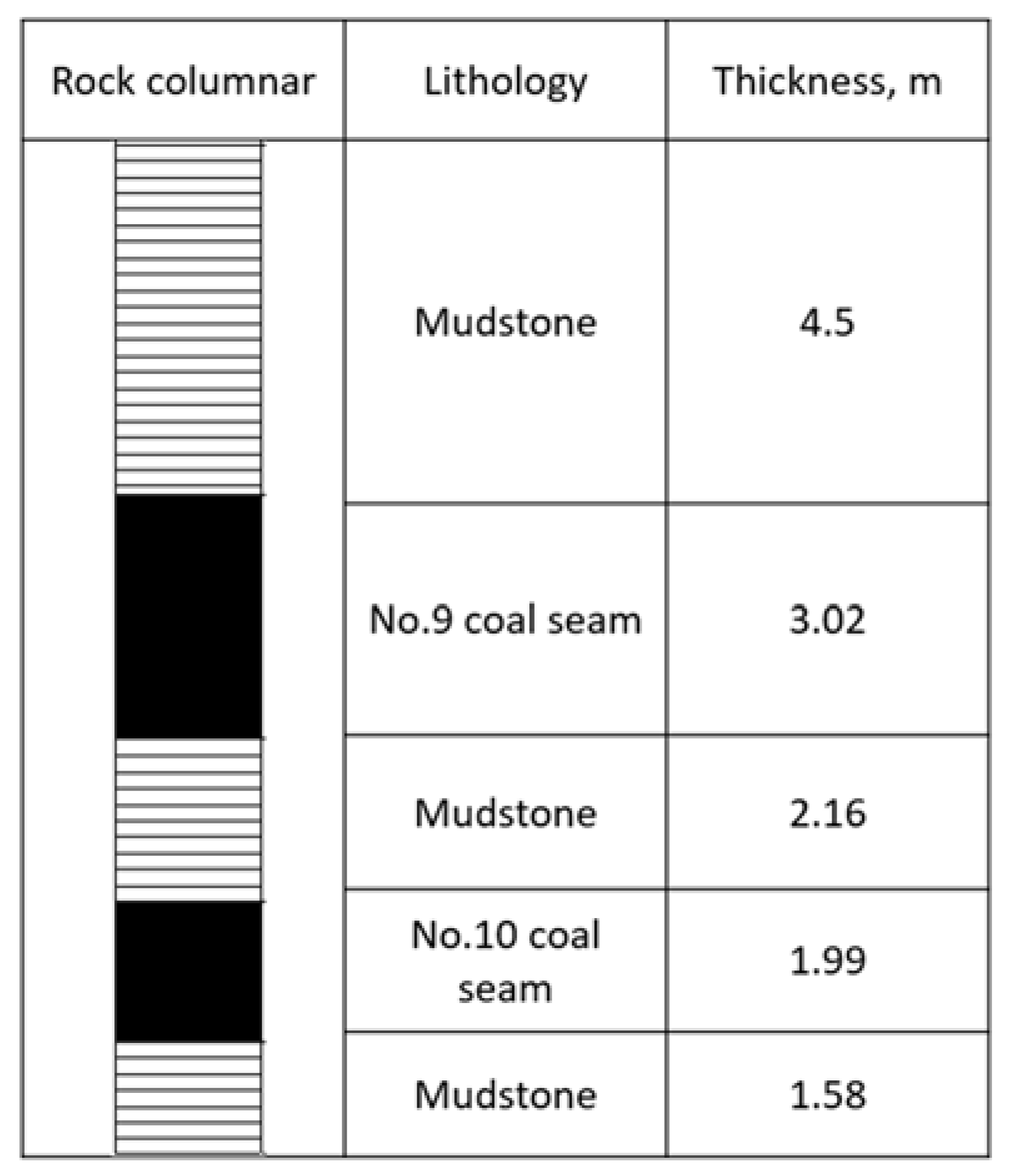
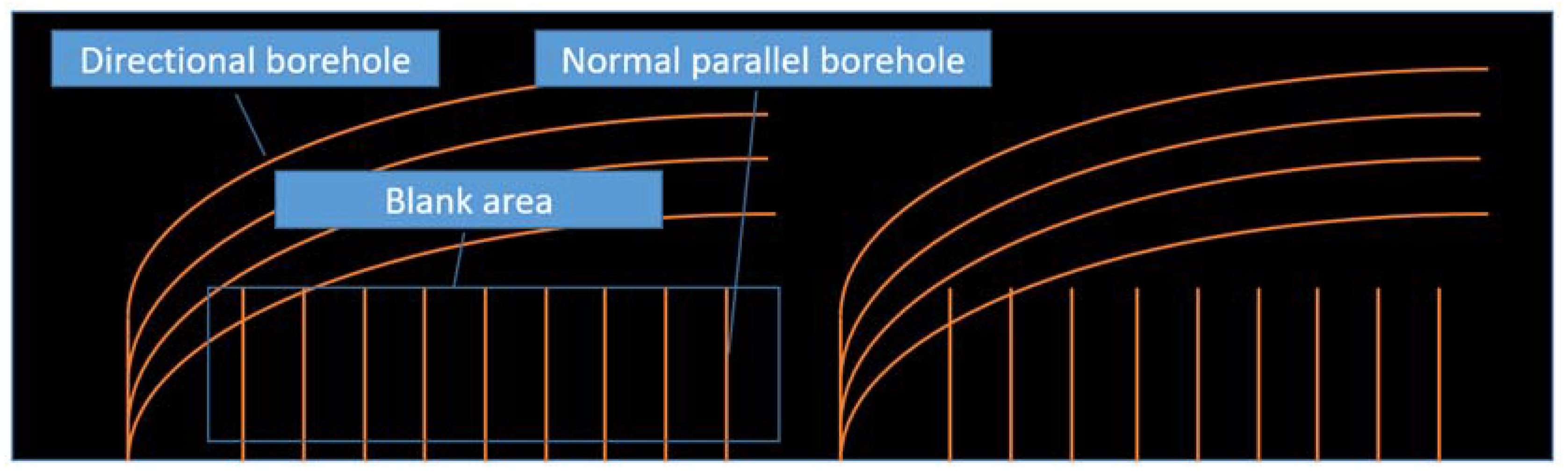


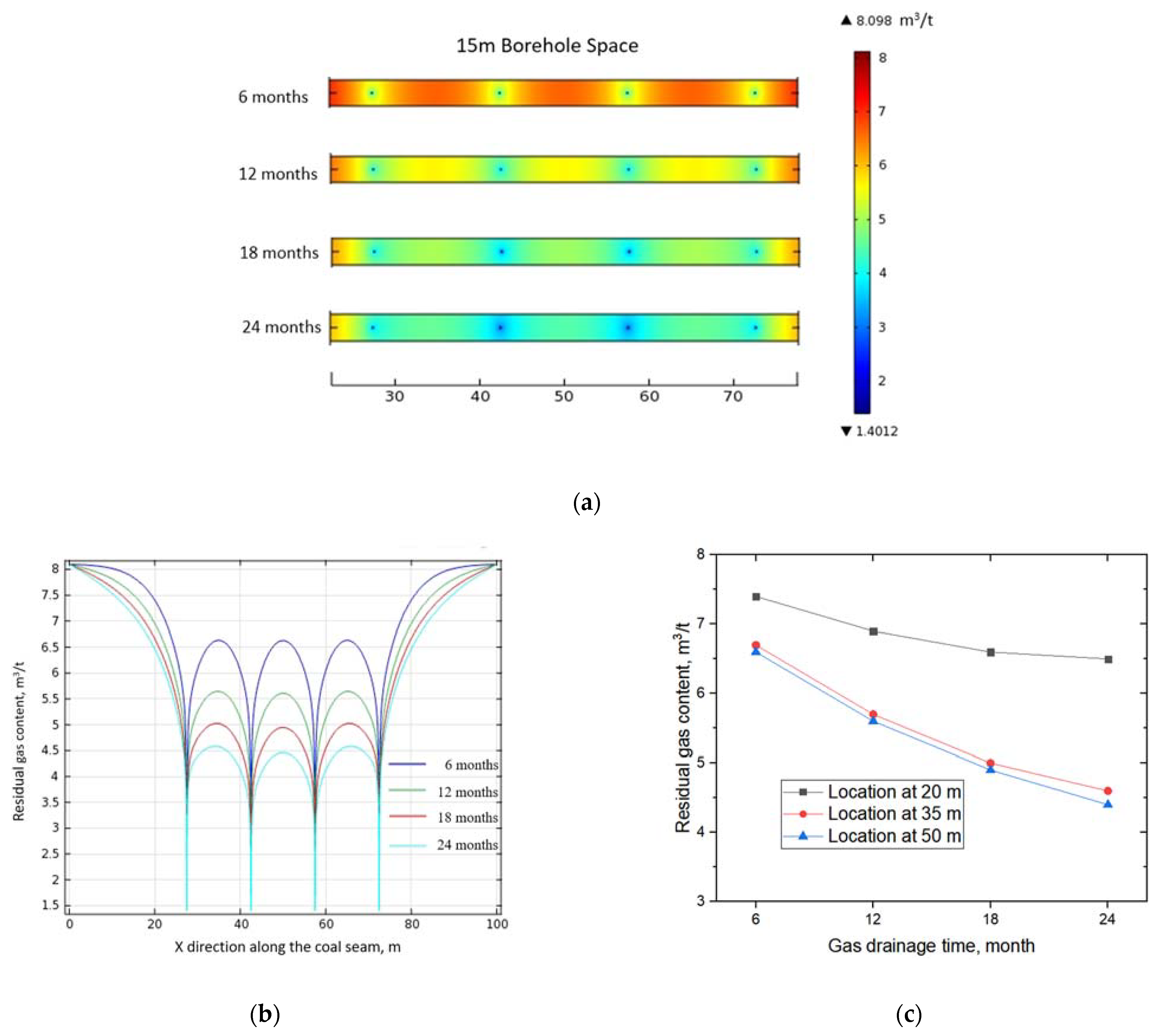
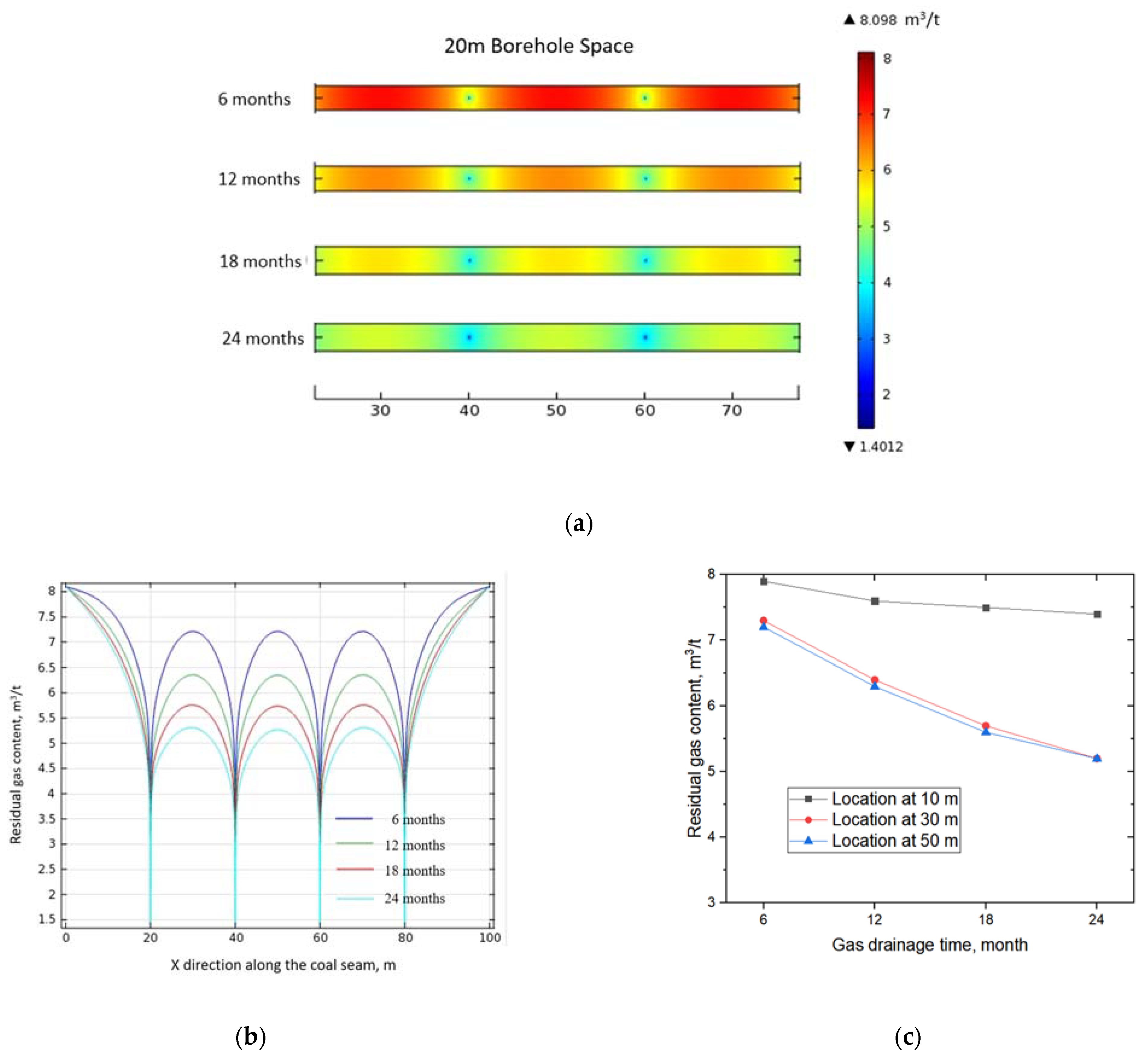



| Location | Langmuir Adsorption Constant | Moisture Mad (%) | Ash Ad (%) | Volatile Matter Vdaf (%) | Fixed Carbon (%) | Density (t/m3) | Porosity (%) | |
|---|---|---|---|---|---|---|---|---|
| VL (m3/t) | PL (MPa) | |||||||
| Excavation heading No. 906 roadway | 28.41 | 1.11 | 0.35 | 10.15 | 26.45 | 63.05 | 1.34 | 3.6 |
| Sample No. | Location | Coal Seam Thickness, m | Depth of Cover, m | Total Gas Content, m3/t |
|---|---|---|---|---|
| 1 | No. 906 roadway, Borehole 1 | 2.91 | 209.20 | 7.90 |
| 2 | No. 906 roadway, Borehole 2 | 2.91 | 207.50 | 7.78 |
| 3 | No. 905 return airway, Borehole 1 | 2.87 | 207.80 | 7.89 |
| 4 | No. 905 return airway, Borehole 2 | 2.81 | 208.00 | 8.06 |
| 5 | No. 905 return airway, Borehole 3 | 3.02 | 201.20 | 7.34 |
| 6 | Main return airway of No. 9 coal seam, Borehole 1 | 2.97 | 201.20 | 7.45 |
| 7 | Main return airway of No. 9 coal seam, Borehole 2 | 2.89 | 201.00 | 7.82 |
| 8 | Main return airway of No. 9 coal seam, Borehole 3 | 2.88 | 200.98 | 7.76 |
| 9 | Excavation heading No. 907 roadway, Borehole 1 | 3.03 | 201.30 | 7.93 |
| 10 | Excavation heading No. 907 roadway, Borehole 2 | 3.01 | 201.30 | 8.10 |
| 11 | Excavation heading No. 907 roadway, Borehole 3 | 3.16 | 202.08 | 7.50 |
| 12 | Maingate of No. 9 coal seam, Borehole 1 | 3.15 | 202.08 | 7.60 |
| 13 | Maingate of No. 9 coal seam, Borehole 2 | 3.30 | 203.10 | 7.78 |
| 14 | Maingate of No. 9 coal seam, Borehole 3 | 3.30 | 203.10 | 7.95 |
| 15 | No. 904 return airway, Borehole 1 | 2.91 | 209.10 | 7.89 |
| 16 | No. 904 return airway, Borehole 2 | 2.90 | 207.50 | 7.76 |
| 17 | No. 904 return airway, Borehole 3 | 2.86 | 207.80 | 7.88 |
| 18 | No. 903 return airway, Borehole 1 | 3.08 | 200.20 | 7.94 |
| 19 | No. 903 return airway, Borehole 2 | 2.83 | 208.00 | 8.04 |
| 20 | No. 903 return airway, Borehole 3 | 2.86 | 201.20 | 8.12 |
| 21 | No. 902 return airway, Borehole 1 | 2.90 | 207.80 | 8.02 |
| 22 | No. 902 return airway, Borehole 2 | 2.86 | 203.80 | 8.05 |
| 23 | No. 902 return airway, Borehole 3 | 3.08 | 205.30 | 8.22 |
| 24 | No. 901 return airway | 3.08 | 210.00 | 8.78 |
| No. | Borehole Type | Space (at the End of the Borehole) |
|---|---|---|
| 1 | Directional borehole | 10 m |
| 2 | Directional borehole | 15 m |
| 3 | Directional borehole | 20 m |
| 4 | Normal parallel borehole | 2 m |
| 5 | Normal parallel borehole | 4 m |
| 6 | Normal parallel borehole | 6 m |
| Parameter | Value |
|---|---|
| Initial gas content | 8.9 m3/t |
| Initial gas pressure | 0.51 MPa |
| Coal seam permeability | 0.85 mD |
| Coal porosity | 3.6% |
| Temperature | 298 K |
| Langmuir volume | 28.41 m3/t |
| Langmuir pressure | 1.11 MPa |
| Coal density | 1.34 t/m3 |
| Borehole diameter | 113 mm (directional borehole)/96 mm (normal borehole) |
| Borehole drainage negative pressure | 30 KPa |
| Borehole Size, m | 10 | 15 | 20 | |
|---|---|---|---|---|
| Drainage Time, Month | ||||
| 6 | × | × | × | |
| 12 | √ | × | × | |
| 18 | √ | √ | × | |
| 24 | √ | √ | √ | |
| Borehole Size, m | 2 | 4 | 6 | |
|---|---|---|---|---|
| Drainage Time, Month | ||||
| 2 | √ | × | × | |
| 4 | √ | √ | × | |
| 6 | √ | √ | √ | |
| Borehole Size, m | 2 | 4 | 6 | |
|---|---|---|---|---|
| Drainage Time, Month | ||||
| 2 | 11.5–18.5 m | - | - | |
| 4 | 11.3–18.7 m | 9.5–20.5 m | - | |
| 6 | 11–19 m | 9.3–20.7 m | 5.4–24.6 m | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, H.; Shu, Z.; Shi, Y.; Wang, X.; Xiao, X.; Lin, J. Gas Migration Patterns with Different Borehole Sizes in Underground Coal Seams: Numerical Simulations and Field Observations. Minerals 2021, 11, 1254. https://doi.org/10.3390/min11111254
Liu H, Shu Z, Shi Y, Wang X, Xiao X, Lin J. Gas Migration Patterns with Different Borehole Sizes in Underground Coal Seams: Numerical Simulations and Field Observations. Minerals. 2021; 11(11):1254. https://doi.org/10.3390/min11111254
Chicago/Turabian StyleLiu, Haibo, Zhihang Shu, Yinbin Shi, Xuebing Wang, Xucheng Xiao, and Jia Lin. 2021. "Gas Migration Patterns with Different Borehole Sizes in Underground Coal Seams: Numerical Simulations and Field Observations" Minerals 11, no. 11: 1254. https://doi.org/10.3390/min11111254
APA StyleLiu, H., Shu, Z., Shi, Y., Wang, X., Xiao, X., & Lin, J. (2021). Gas Migration Patterns with Different Borehole Sizes in Underground Coal Seams: Numerical Simulations and Field Observations. Minerals, 11(11), 1254. https://doi.org/10.3390/min11111254







