Morphometric Parameters of Krumbein Grain Shape Charts—A Critical Approach in Light of the Automatic Grain Shape Image Analysis
Abstract
:1. Introduction
2. Materials and Methods
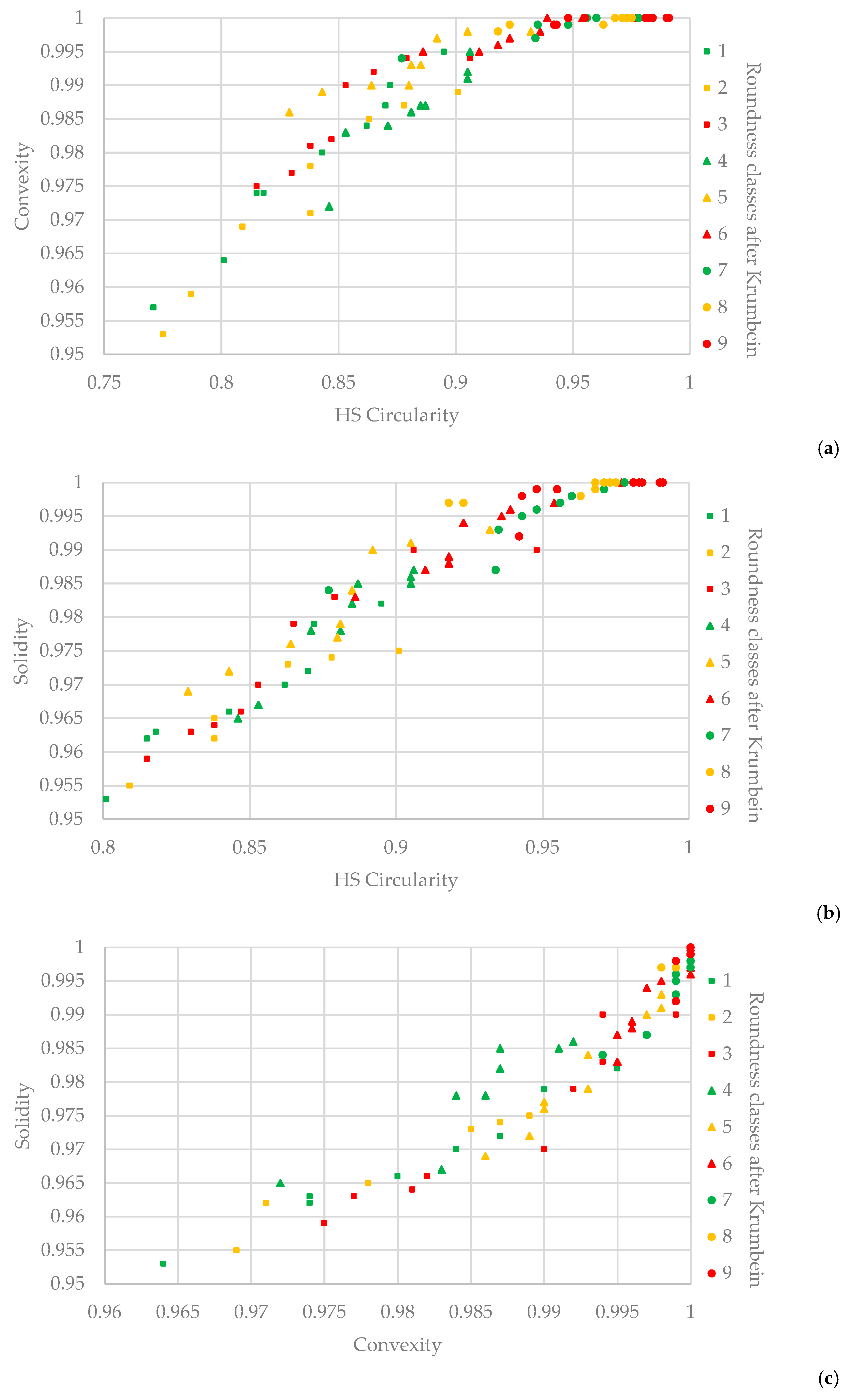
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Wentworth, C.R. A scale of grade and class terms for clastic sediments. J. Geol. 1922, 30, 377–392. [Google Scholar] [CrossRef]
- Pentland, A. A method of measuring the angularity of sands. Proc. Trans. R. Soc. Can. 1927, 21, 43. [Google Scholar]
- Mitchell, J.K.; Soga, K. Fundamentals of Soil Behavior; John Wiley & Sons, Ltd.: New York, NY, USA, 2005. [Google Scholar]
- Krumbein, W.C. Measurement and geological significance of shape and roundness of sedimentary particles. J. Sediment. Petrol. 1941, 11, 64–72. [Google Scholar] [CrossRef]
- Powers, M.C. A new roundness scale for sedimentary particles. J. Sediment. Petrol. 1953, 23, 117–119. [Google Scholar] [CrossRef]
- Cailleux, A. Les actions éoliennes périglaciaires en Europe. Mem. Soc. Geol. Fr. 1942, 46, 1–176. [Google Scholar]
- Cailleux, A. Application à la Géographie des Méthodes D’étude des Sables et des Galets; Universidade do Brasil, Faculdade Nacional de Filosofia, Centro de Pesquisas de Geografia do Brasil: Rio de Janeiro, Brazil, 1961.
- Vallejo, L.E.; Zhou, Y. The Relationship Between the Fractal Dimension and Krumbein’s Roundness Number. Soils Found. 1995, 35, 163–167. [Google Scholar] [CrossRef] [Green Version]
- Els, B.G. Pebble morphology of an ancient conglomerate; the Middelvlei gold placer, Witwatersrand, South Africa. J. Sediment. Res. 1988, 58, 894–901. [Google Scholar]
- Pan, T.; Tutumluer, E. Evaluation of visual based aggregate shape classifications using the University of Illinois Aggregate Image Analyzer (UIAIA). In Proceedings of the Pavement Mechanics and Performance, Shanghai, China, 6–8 June 2006; pp. 126–133. [Google Scholar]
- Roussillon, T.; Piégay, H.; Sivignon, I.; Tougne, L.; Lavigne, F. Automatic computation of pebble roundness using digital imagery and discrete geometry. Comput. Geosci. 2009, 35, 1992–2000. [Google Scholar] [CrossRef]
- Maroof, M.A.; Mahboubi, A.; Noorzad, A.; Safi, Y. A new approach to particle shape classification of granular materials. Transp. Geotech. 2020, 22, 100296. [Google Scholar] [CrossRef]
- Selley, R.C. Applied Sedimentology; Academic Press: San Diego, CA, USA, 2000. [Google Scholar]
- Tunwal, M.; Mulchrone, K.F.; Meere, P.A. Quantitative characterization of grain shape: Implications for textural maturity analysis and discrimination between depositional environments. Sedimentology 2018, 65, 1761–1776. [Google Scholar] [CrossRef]
- Tunwal, M.; Mulchrone, K.F.; Meere, P.A. A new approach to particle shape quantification using the curvature plot. Powder Technol. 2020, 374, 377–388. [Google Scholar] [CrossRef]
- Tunwal, M.; Mulchrone, K.F.; Meere, P.A. Image based Particle Shape Analysis Toolbox (IPSAT). Comput. Geosci. 2020, 135, 104391. [Google Scholar] [CrossRef]
- Dill, H.G.; Buzatu, A.; Balaban, S.J.; Ufer, K.; Techmer, A.; Schedlinsky, W.; Füssl, M. The transition of very coarse-grained meandering to straight fluvial drainage systems in a tectonized foreland-basement landscape during the Holocene (SE Germany) –A joint geomorphological-geological study. Geomorphology 2020, 370, 107364. [Google Scholar] [CrossRef]
- Folk, R.L. Stages of textural maturity in sedimentary rocks. J. Sediment. Res. 1951, 21, 127–130. [Google Scholar] [CrossRef]
- Mycielska-Dowgiałło, E.; Woronko, B. Rounding and frosting analysis of quartz grains of sand fraction, and its interpretative value. Przegląd Geol. 1998, 46, 1275–1281. [Google Scholar]
- Dill, H.G.; Buzatu, A.; Balaban, S.-I.; Ufer, K.; Gómez Tapias, J.; Bîrgăoanu, D.; Cramer, T. The “badland trilogy” of the Desierto de la Tatacoa, upper Magdalena Valley, Colombia, a result of geodynamics and climate: With a review of badland landscapes. Catena 2020, 194, 104696. [Google Scholar] [CrossRef]
- Kasse, C.; Vandenberghe, D.; de Corte, F.; van den Haute, P. Late Weichselian fluvio-aeolian sands and coversands of the type locality Grubbenvorst (southern Netherlands): Sedimentary environments, climate record and age. J. Quat. Sci. 2007, 22, 695–708. [Google Scholar] [CrossRef]
- Ulusoy, U.; Kursun, I. Comparison of different 2D image analysis measurement techniques for the shape of talc particles produced by different media milling. Miner. Eng. 2011, 24, 91–97. [Google Scholar] [CrossRef]
- Schneider, C.A.; Rasband, W.S.; Eliceiri, K.W. NIH Image to ImageJ: 25 years of image analysis. Nat. Methods 2012, 9, 671–675. [Google Scholar] [CrossRef]
- Heilbronner, R.; Barrett, S. Image Analysis in Earth Sciences: Microstructures and Textures of Earth Materials; Springer: Berlin, Germany, 2014. [Google Scholar]
- Chávez, G.M.; Castillo-Rivera, F.; Montenegro-Ríos, J.A.; Borselli, L.; Rodríguez-Sedano, L.A.; Sarocchi, D. Fourier Shape Analysis, FSA: Freeware for quantitative study of particle morphology. J. Volcanol. Geotherm. Res. 2020, 404, 107008. [Google Scholar] [CrossRef]
- Olson, E. Particle Shape Factors and Their Use in Image Analysis—Part 1: Theory. J. GXP Compliance 2011, 15, 85–96. [Google Scholar]
- Morphologi G3. User manual. Man0410; Malvern Instruments Ltd.: Worcestershire, UK, 2008.
- Campaña, I.; Benito-Calvo, A.; Pérez-González, A.; Bermúdez de Castro, J.M.; Carbonell, E. Assessing automated image analysis of sand grain shape to identify sedimentary facies, Gran Dolina archaeological site (Burgos, Spain). Sediment. Geol. 2016, 346, 72–83. [Google Scholar] [CrossRef]
- Joo, Y.J.; Soreghan, A.M.; Madden, M.E.E.; Soreghan, G.S. Quantification of particle shape by an automated image analysis system: A case study in natural sediment samples from extreme climates. Geosci. J. 2018, 22, 525–532. [Google Scholar] [CrossRef]
- Kapui, Z.; Kereszturi, A.; Kiss, K.; Szalai, Z.; Újvári, G.; Hickman-Lewis, K.; Foucher, F.; Westall, F. Fluvial or aeolian grains? Separation of transport agents on Mars using earth analogue observations. Planet. Space Sci. 2018, 163, 56–76. [Google Scholar] [CrossRef]
- Resentini, A.; AndÒ, S.; Garzanti, E. Quantifying Roundness of Detrital Minerals By Image Analysis: Sediment Transport, Shape Effects, and Provenance Implications. J. Sediment. Res. 2018, 88, 276–289. [Google Scholar] [CrossRef]
- Roettig, C.-B.; Varga, G.; Sauer, D.; Kolb, T.; Wolf, D.; Makowski, V.; Espejo, J.M.R.; Zöller, L.; Faust, D. Characteristics, nature, and formation of palaeosurfaces within dunes on Fuerteventura. Quat. Res. 2019, 91, 4–23. [Google Scholar] [CrossRef]
- Varga, G.; Kovács, J.; Szalai, Z.; Cserháti, C.; Újvári, G. Granulometric characterization of paleosols in loess series by automated static image analysis. Sediment. Geol. 2018, 370, 1–14. [Google Scholar] [CrossRef]
- Sochan, A.; Zieliński, P.; Bieganowski, A. Selection of shape parameters that differentiate sand grains, based on the automatic analysis of two-dimensional images. Sediment. Geol. 2015, 327, 14–20. [Google Scholar] [CrossRef]
- Mycielska-Dowgiałło, E.; Woronko, B. The degree of aeolization of Quaternary deposits in Poland as a tool for stratigraphic interpretation. Sediment. Geol. 2004, 168, 149–163. [Google Scholar] [CrossRef]
- Szmańda, J.; Witkowski, K.; Chmielowska, D.; Smolska, E.; Szwarczewski, P. Analiza graniformametryczna obtoczenia ziaren kwarcu w świetle parametrów uzyskiwanych aparatem Morphologi G3. In Geneza, Litologia i Stratygrafia Utworów Czwartorzędowych. T.7; Kostrzewski, A., Stach, A., Majewski, M., Eds.; Instytut Geoekologii i Geoinformacji UAM: Poznań, Poland, 2018; pp. 191–195. [Google Scholar]
- Blott, S.; Pye, K. Particle shape: A review and new methods of characterization and classification. Sedimentology 2008, 55, 31–63. [Google Scholar] [CrossRef]
- Suzuki, K.; Fujiwara, H.; Ohta, T. The evaluation of macroscopic and microscopic textures of sand grains using elliptic Fourier and principal component analysis: Implications for the discrimination of sedimentary environments. Sedimentology 2015, 62, 1184–1197. [Google Scholar] [CrossRef]
- Cox, E.P. A Method of Assigning Numerical and Percentage Values to the Degree of Roundness of Sand Grains. J. Paleontol. 1927, 1, 179–183. [Google Scholar]
- Riley, N.A. Projection Sphericity. J. Sediment. Res. 1941, 11, 94–97. [Google Scholar]
- Zingg, T. Beitrag zur Schotteranalyse; ETH: Zürich, Switzerland, 1935. [Google Scholar]
- Barret, P.J. The shape of rock particles, a critical review. Sedimentology 1980, 27, 291–303. [Google Scholar] [CrossRef]
- Tarriño, A. A New Methodology and Classification System for Describing Three-Dimensional Particle Formats: Application to Clastic Lithic Products of Archaeological and Geological Origin. Archaeometry 2015, 57, 928–948. [Google Scholar] [CrossRef]
- Domokos, G.; Jerolmack, D.J.; Sipos, A.Á.; Török, Á. How River Rocks Round: Resolving the Shape-Size Paradox. PLoS ONE 2014, 9, e88657. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vangla, P.; Roy, N.; Gali, M.L. Image based shape characterization of granular materials and its effect on kinematics of particle motion. Granul. Matter 2017, 20, 6. [Google Scholar] [CrossRef]
- Boggs, S. Principles of Sedimentology and Stratigraphy, 4th ed.; Pearson Prentice Hall: Upper Saddle River, NJ, USA, 2006. [Google Scholar]
- Becker, L.W.M.; Hjelstuen, B.O.; Støren, E.W.N.; Sejrup, H.P. Automated counting of sand-sized particles in marine records. Sedimentology 2018, 65, 842–850. [Google Scholar] [CrossRef]
- Røthe, T.O.; Bakke, J.; Støren, E.W.N.; Bradley, R.S. Reconstructing Holocene Glacier and Climate Fluctuations From Lake Sediments in Vårfluesjøen, Northern Spitsbergen. Front. Earth Sci. 2018, 6, 91. [Google Scholar] [CrossRef] [Green Version]
- Li, C.; Holden, J.; Grayson, R. Effects of rainfall, overland flow and their interactions on peatland interrill erosion processes. Earth Surf. Process. Landforms 2018, 43, 1451–1464. [Google Scholar] [CrossRef]
- Varga, G. Changing nature of Saharan dust deposition in the Carpathian Basin (Central Europe): 40 years of identified North African dust events (1979–2018). Environ. Int. 2020, 139, 105712. [Google Scholar] [CrossRef] [PubMed]
- Kozakiewicz, J. Image analysis algorithm for detection and measurement of Martian sand grains. Earth Sci. Inform. 2018, 11, 257–272. [Google Scholar] [CrossRef] [Green Version]
- Varga, G.; Újvári, G.; Kovács, J.; Jakab, G.; Kiss, K.; Szalai, Z. Granulometric profiling of aeolian dust deposits by automated image analysis. In Proceedings of the EGU General Assembly Conference, Vienna, Austria, 17–22 April 2016; p. EPSC2016-5512. [Google Scholar]



| Class. No. | Shape Pattern | HS Circularity | Convexity | Solidity | Aspect Ratio |
|---|---|---|---|---|---|
| 1.1 |  | 0.895 A+ | 0.995 A+ | 0.979 | 0.818 |
| 1.2 |  | 0.801 | 0.98 | 0.953 A− | 0.896 |
| 1.3 |  | 0.87 | 0.99 | 0.97 A+ | 0.965 |
| 1.4 |  | 0.818 | 0.974 | 0.963 | 0.749 |
| 1.5 |  | 0.862 | 0.974 | 0.972 | 0.818 |
| 1.6 |  | 0.843 | 0.964 − | 0.962 | 0.887 |
| 1.7 |  | 0.771 A− | 0.957 A− | 0.948 A− | 0.985 |
| 1.8 |  | 0.815 | 0.984 | 0.966 | 0.822 |
| 1.9 |  | 0.872 | 0.987 | 0.982 | 0.783 |
| AVG | 0.855 | 0.978 | 0.966 | 0.858 | |
| SD | 0.040 | 0.012 | 0.011 | 0.081 | |
| 2.1 |  | 0.775 A− | 0.943 A− | 0.949 | 0.795 |
| 2.2 |  | 0.838 | 0.969 | 0.955 | 0.886 |
| 2.3 |  | 0.809 | 0.989 A+ | 0.965 | 0.889 |
| 2.4 |  | 0.838 | 0.959 | 0.974 | 0.896 |
| 2.5 |  | 0.787 | 0.971 | 0.936 A− | 0.883 |
| 2.6 |  | 0.863 | 0.985 | 0.962 | 0.982 |
| 2.7 |  | 0.901 A+ | 0.987 | 0.975 A+ | 0.925 |
| 2.8 |  | 0.878 A+ | 0.978 | 0.973 | 0.856 |
| 2.9 |  | 0.755 A− | 0.953 A− | 0.938 A− | 0.836 |
| AVG | 0.827 | 0.970 | 0.959 | 0.883 | |
| SD | 0.049 | 0.016 | 0.015 | 0.053 | |
| 3.1 |  | 0.847 | 0.982 | 0.959 A− | 0.832 |
| 3.2 |  | 0.815 A− | 0.975 A− | 0.966 | 0.791 |
| 3.3 |  | 0.879 | 0.981 | 0.97 | 0.854 |
| 3.4 |  | 0.83 | 0.992 | 0.964 | 0.907 |
| 3.5 |  | 0.853 | 0.994 | 0.983 | 0.65 |
| 3.6 |  | 0.865 | 0.994 | 0.979 | 0.704 |
| 3.7 |  | 0.838 | 0.977 A− | 0.963 | 0.746 |
| 3.8 |  | 0.948 A+ | 0.999 A+ | 0.990 A+ | 0.993 |
| 3.9 |  | 0.906 | 0.99 | 0.990 A+ | 0.976 |
| AVG | 0.865 | 0.987 | 0.974 | 0.828 | |
| SD | 0.041 | 0.009 | 0.012 | 0.650 | |
| 4.1 |  | 0.881 | 0.987 | 0.985 | 0.809 |
| 4.2 |  | 0.905 A+ | 0.995 A+ | 0.985 | 0.844 |
| 4.3 |  | 0.887 | 0.992 | 0.978 | 0.799 |
| 4.4 |  | 0.846 A− | 0.972 A− | 0.965 A− | 0.905 |
| 4.5 |  | 0.906 A+ | 0.986 | 0.987 | 0.807 |
| 4.6 |  | 0.853 A+ | 0.983 | 0.967 A− | 0.815 |
| 4.7 |  | 0.885 | 0.991 | 0.986 | 0.807 |
| 4.8 |  | 0.905 A+ | 0.984 | 0.982 | 0.906 |
| 4.9 |  | 0.871 | 0.987 | 0.978 | 0.855 |
| AVG | 0.882 | 0.986 | 0.979 | 0.839 | |
| SD | 0.022 | 0.007 | 0.008 | 0.042 | |
| 5.1 |  | 0.829 A− | 0.986 A− | 0.993 A+ | 0.57 |
| 5.2 |  | 0.932 A+ | 0.998 A+ | 0.984 | 0.941 |
| 5.3 |  | 0.881 | 0.993 | 0.977 | 0.746 |
| 5.4 |  | 0.892 | 0.997 | 0.99 | 0.643 |
| 5.5 |  | 0.885 | 0.99 | 0.969 A− | 0.787 |
| 5.6 |  | 0.843 A− | 0.99 | 0.972 A− | 0.69 |
| 5.7 |  | 0.864 | 0.993 | 0.979 | 0.769 |
| 5.8 |  | 0.88 | 0.989 | 0.976 | 0.738 |
| 5.9 |  | 0.905 | 0.998 | 0.991 A+ | 0.997 |
| AVG | 0.879 | 0.993 | 0.981 | 0.765 | |
| SD | 0.031 | 0.004 | 0.009 | 0.135 | |
| 6.1 |  | 0.936 | 0.998 | 0.996 | 0.755 |
| 6.2 |  | 0.918 | 1 | 0.995 | 0.804 |
| 6.3 |  | 0.91 | 0.995 | 0.983 A− | 0.981 |
| 6.4 |  | 0.939 | 0.996 | 0.988 | 0.908 |
| 6.5 |  | 0.886 A− | 0.995 | 0.987 | 0.84 |
| 6.6 |  | 0.918 | 0.997 | 0.989 | 0.752 |
| 6.7 |  | 0.954 | 0.996 | 0.994 | 0.907 |
| 6.8 |  | 0.923 | 1 | 0.997 | 0.744 |
| 6.9 |  | 0.977 A+ | 1 | 1 A+ | 0.969 |
| AVG | 0.929 | 0.997 | 0.992 | 0.851 | |
| SD | 0.026 | 0.002 | 0.006 | 0.094 | |
| 7.1 |  | 0.935 | 1 | 0.997 | 0.781 |
| 7.2 |  | 0.956 | 1 | 0.993 | 0.782 |
| 7.3 |  | 0.934 | 0.994 A− | 0.987 A− | 0.88 |
| 7.4 |  | 0.978 A+ | 0.999 | 0.999 | 0.989 |
| 7.5 |  | 0.96 | 0.999 | 0.996 | 0.827 |
| 7.6 |  | 0.943 | 0.999 | 0.995 | 0.794 |
| 7.7 |  | 0.971 | 1 | 1 | 0.82 |
| 7.8 |  | 0.948 | 1 | 0.998 | 0.89 |
| 7.9 |  | 0.877 A− | 0.997 | 0.984 A− | 0.628 |
| AVG | 0.945 | 0.999 | 0.994 | 0.821 | |
| SD | 0.030 | 0.002 | 0.005 | 0.098 | |
| 8.1 |  | 0.984 | 1 | 1 | 0.936 |
| 8.2 |  | 0.973 | 0.998 A− | 0.997 A− | 0.962 |
| 8.3 |  | 0.963 | 1 | 1 | 0.809 |
| 8.4 |  | 0.975 | 1 | 0.999 | 0.852 |
| 8.5 |  | 0.968 | 1 | 1 | 0.762 |
| 8.6 |  | 0.918 A− | 0.999 | 0.997 A− | 0.657 |
| 8.7 |  | 0.968 | 0.999 | 1 | 0.799 |
| 8.8 |  | 0.971 | 1 | 1 | 0.765 |
| 8.9 |  | 0.923 A− | 1 | 0.998 | 0.671 |
| AVG | 0.960 | 1.000 | 0.999 | 0.801 | |
| SD | 0.023 | 0.001 | 0.001 | 0.104 | |
| 9.1 |  | 0.984 | 1 | 1 | 0.934 |
| 9.2 |  | 0.942 A− | 1 | 1 | 0.709 |
| 9.3 |  | 0.983 | 0.999 | 0.999 | 0.962 |
| 9.4 |  | 0.955 | 1 | 0.998 | 0.758 |
| 9.5 |  | 0.943 A− | 1 | 0.992 A− | 0.739 |
| 9.6 |  | 0.981 | 1 | 1 | 0.847 |
| 9.7 |  | 0.991 | 1 | 1 | 0.972 |
| 9.8 |  | 0.948 | 0.999 | 0.999 | 0.734 |
| 9.9 |  | 0.99 | 1 | 1 | 0.899 |
| AVG | 0.969 | 1.000 | 0.999 | 0.839 | |
| SD | 0.021 | 0.000 | 0.003 | 0.106 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Szmańda, J.B.; Witkowski, K. Morphometric Parameters of Krumbein Grain Shape Charts—A Critical Approach in Light of the Automatic Grain Shape Image Analysis. Minerals 2021, 11, 937. https://doi.org/10.3390/min11090937
Szmańda JB, Witkowski K. Morphometric Parameters of Krumbein Grain Shape Charts—A Critical Approach in Light of the Automatic Grain Shape Image Analysis. Minerals. 2021; 11(9):937. https://doi.org/10.3390/min11090937
Chicago/Turabian StyleSzmańda, Jacek Bogusław, and Karol Witkowski. 2021. "Morphometric Parameters of Krumbein Grain Shape Charts—A Critical Approach in Light of the Automatic Grain Shape Image Analysis" Minerals 11, no. 9: 937. https://doi.org/10.3390/min11090937
APA StyleSzmańda, J. B., & Witkowski, K. (2021). Morphometric Parameters of Krumbein Grain Shape Charts—A Critical Approach in Light of the Automatic Grain Shape Image Analysis. Minerals, 11(9), 937. https://doi.org/10.3390/min11090937







