Surrounding Rock Stability in Unsupported Roof Area and Rapid Heading Technique for Deep Arch Coal Roadways under Goaf
Abstract
:1. Introduction
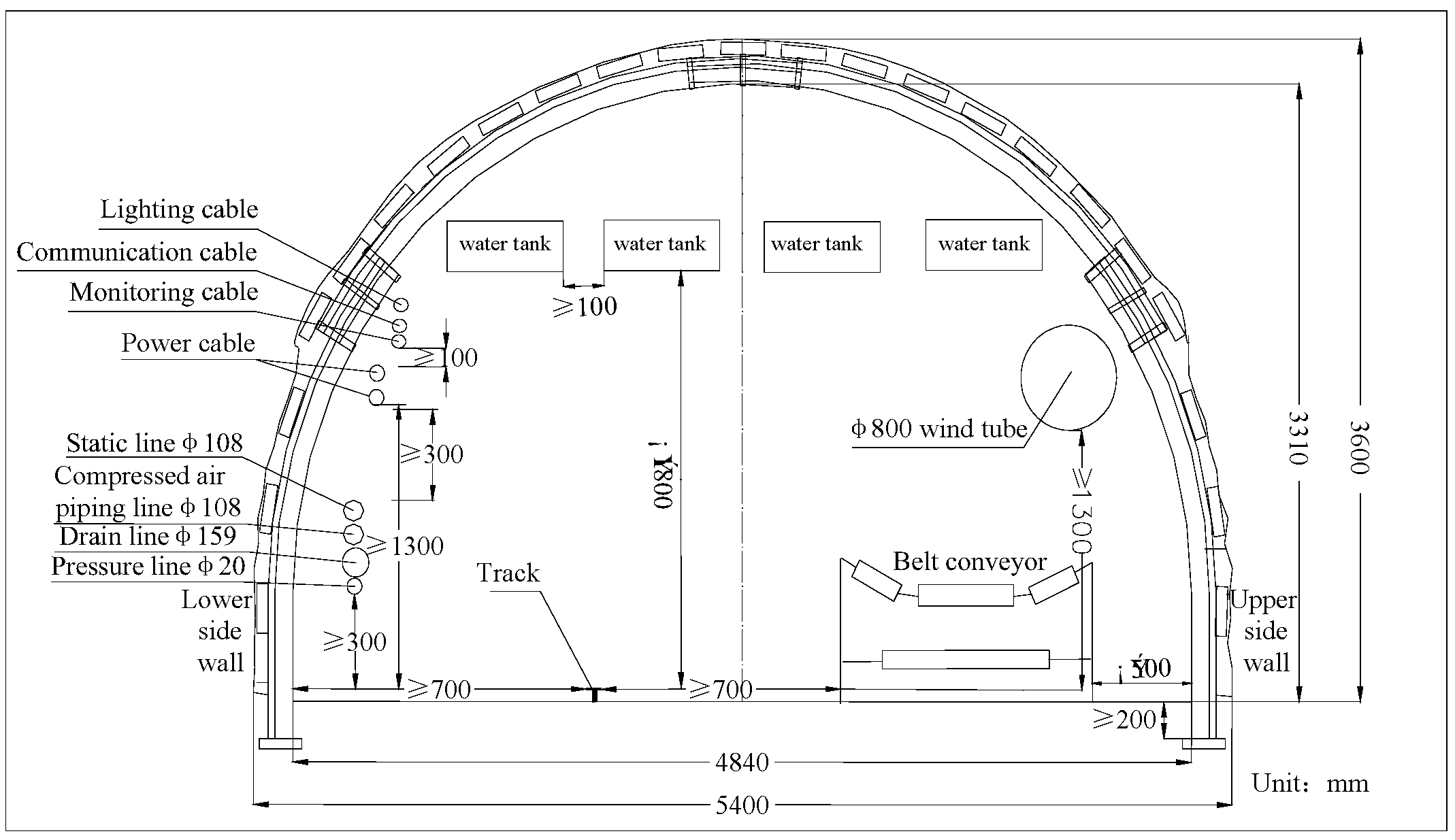
2. Project Overview
3. Mechanical Behavior of Top Cover Cylindrical Shell Model of Unsupported Roof Area in Arch Roadway
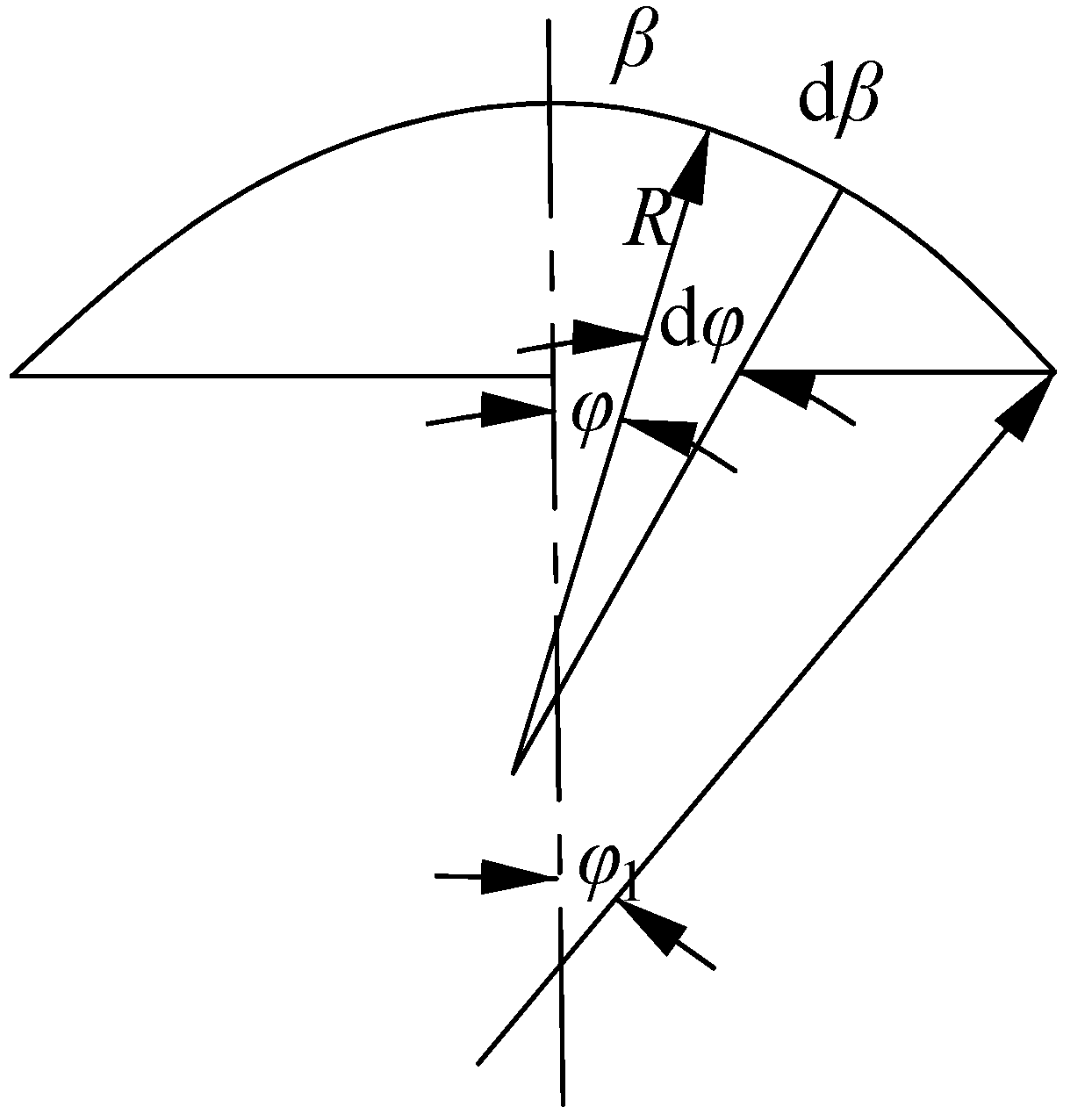
3.1. Moment Free Theory of Cylindrical Shell
3.2. Moment Free Calculation of Top Cover Cylindrical Shell
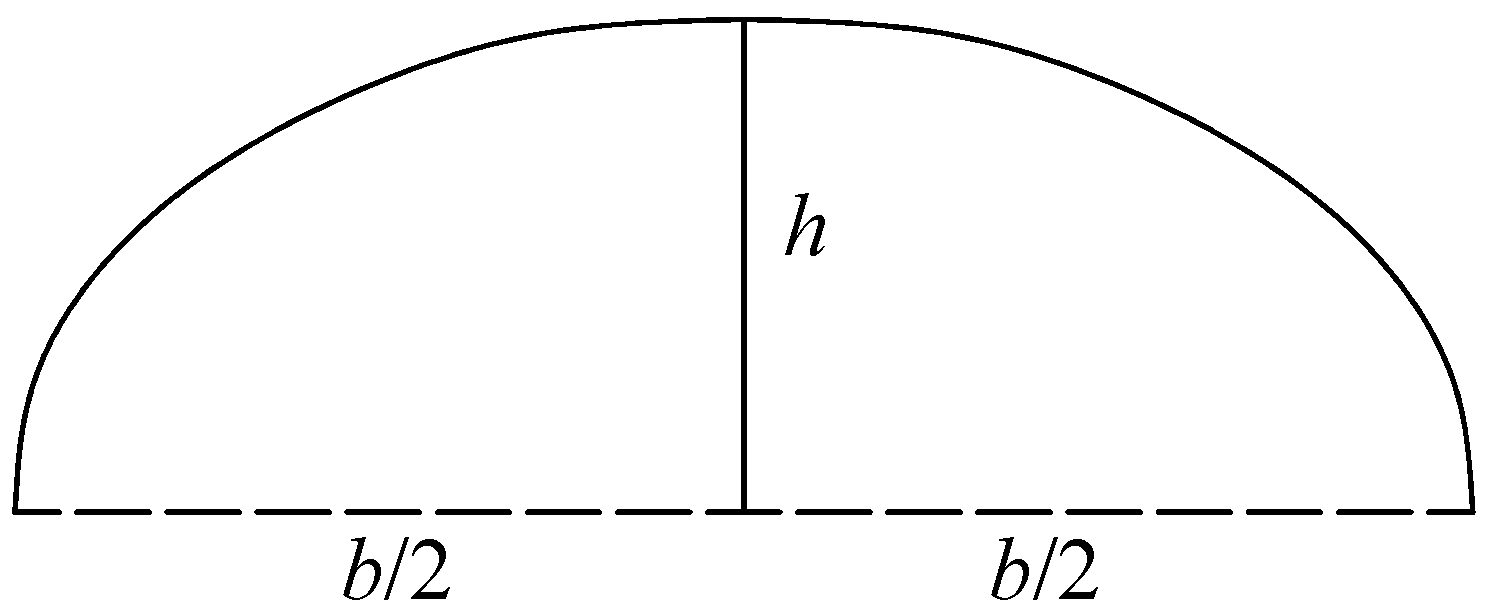
3.3. URD Calculation for Semicircular Arch Roadway
- (1)
- The maximum URD for heading arch roadways is closely related to the roof rock tensile strength, roadway arch height, roadway width, and roof load, each of which has roughly the same degree of influence, suggesting that an arch roadway is well stable and highly adaptable to varying geological conditions.
- (2)
- The URD of arch roadways is insignificantly sensitive to roadway width. Therefore, an arch section is more beneficial to roadways with a large section.
- (3)
- Arch height has a great influence on the URD of arch roadways, so a large arch height would be recommendable in practical engineering design. Tensile strength also has a great influence on the URD of arch roadways, so in cases of degraded roof tensile strength, a small URD would be more preferable.
4. Numerical Simulation of Arch Roadway under Goaf
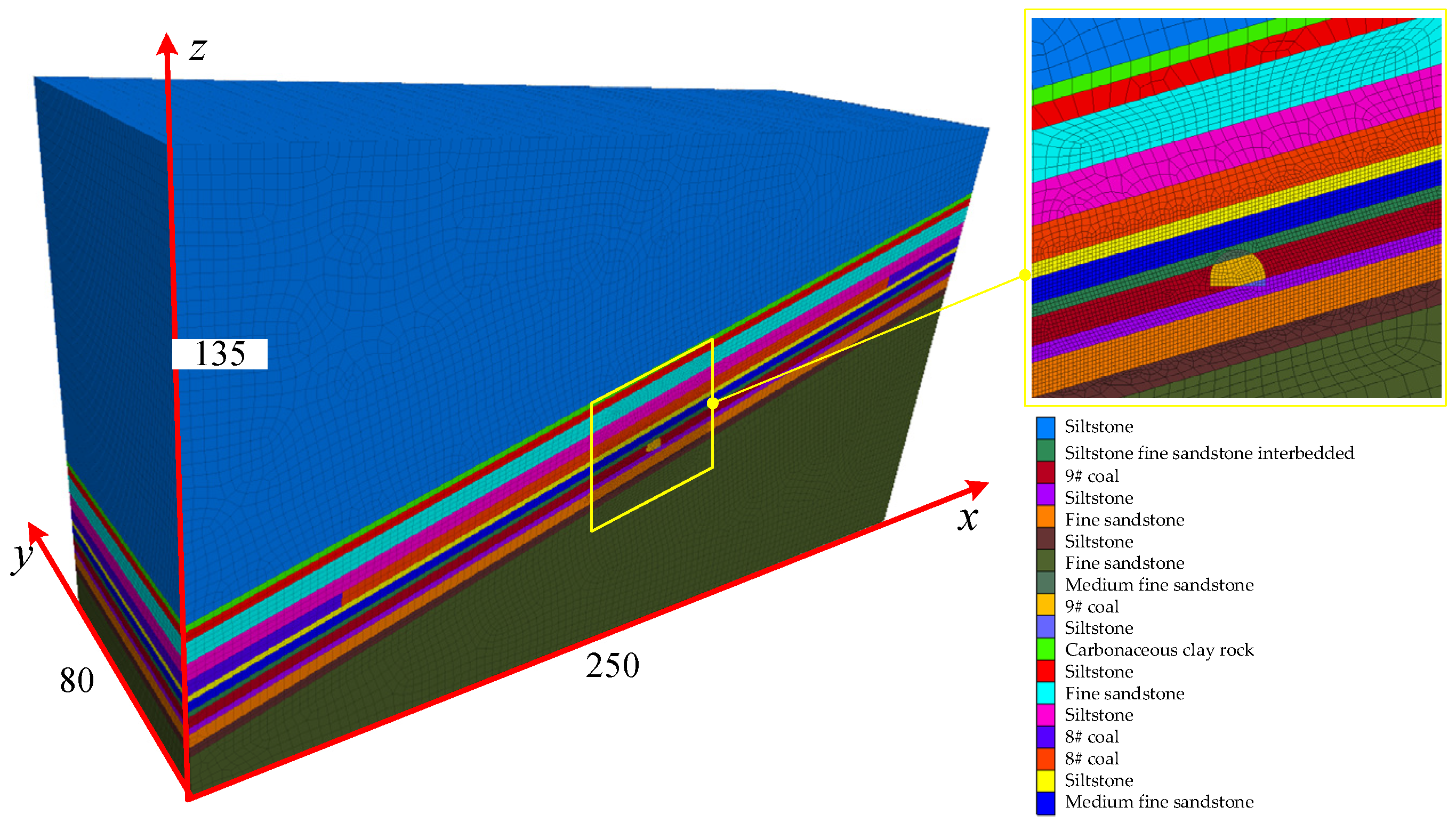
4.1. Model Building
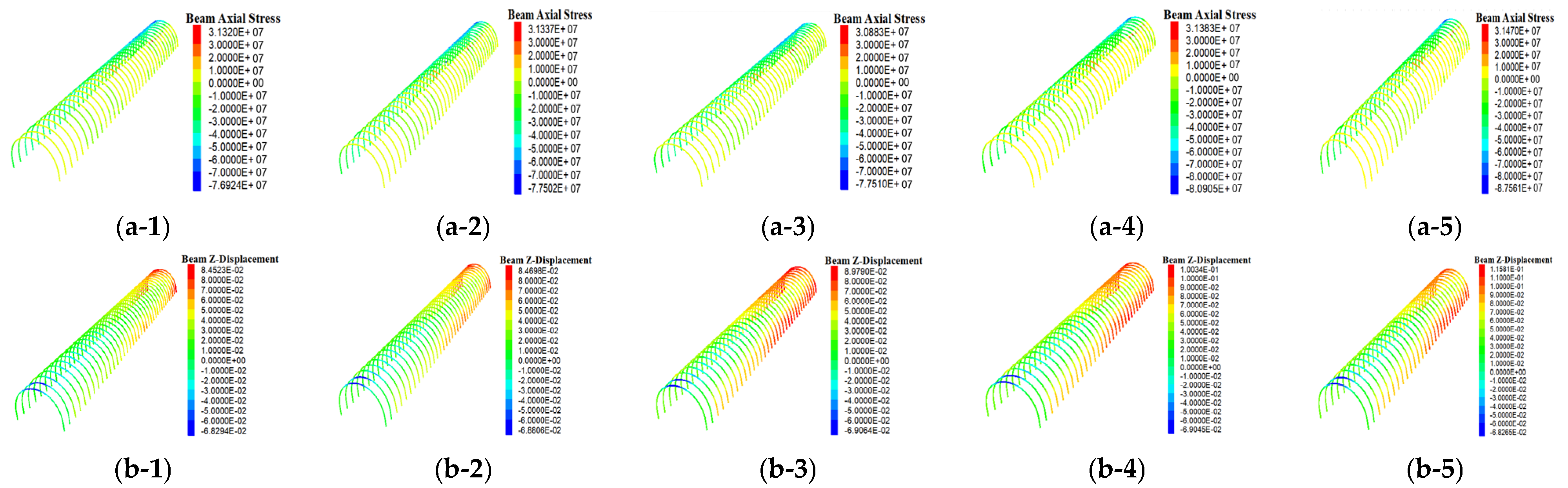
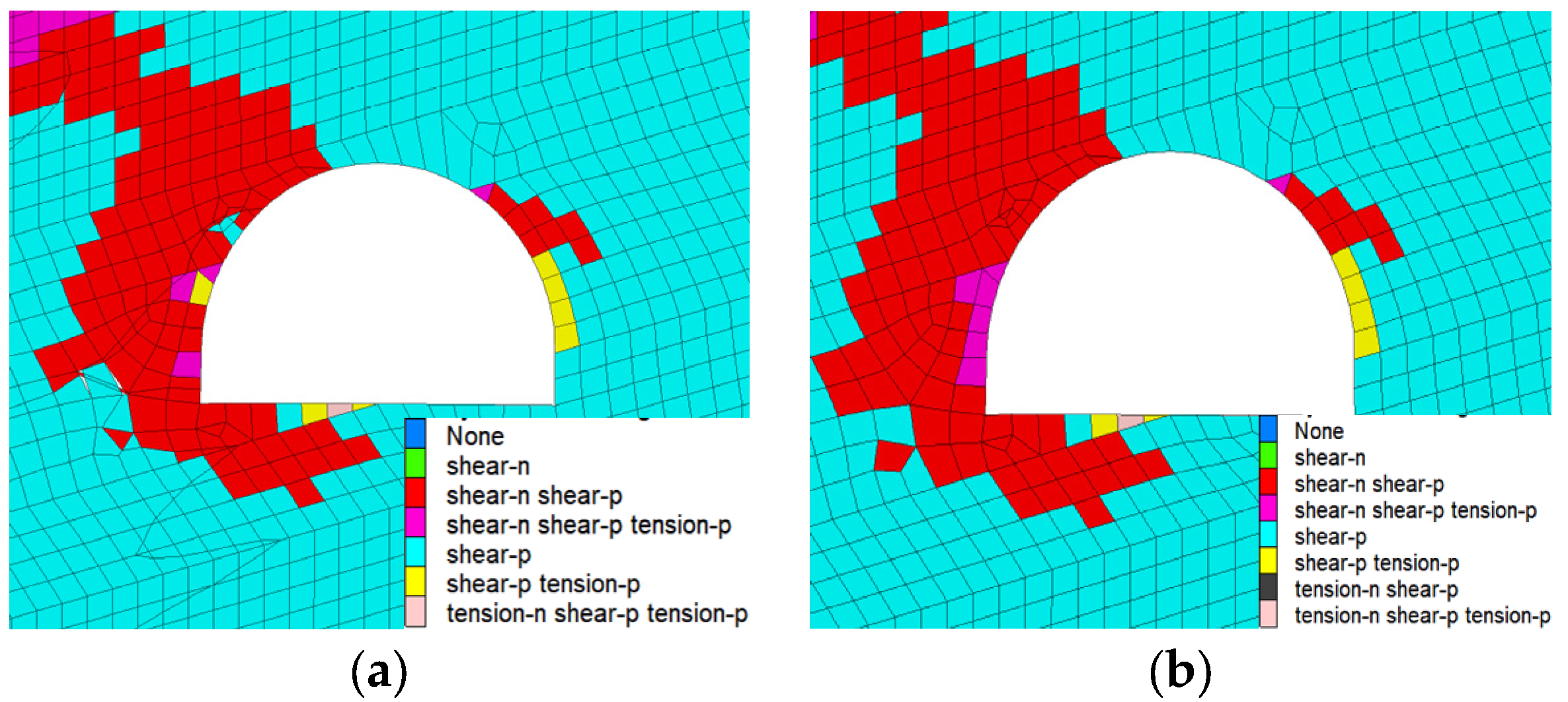
4.2. Influence of URD on Roadway Surrounding Rock Stability in Unsupported Roof Area
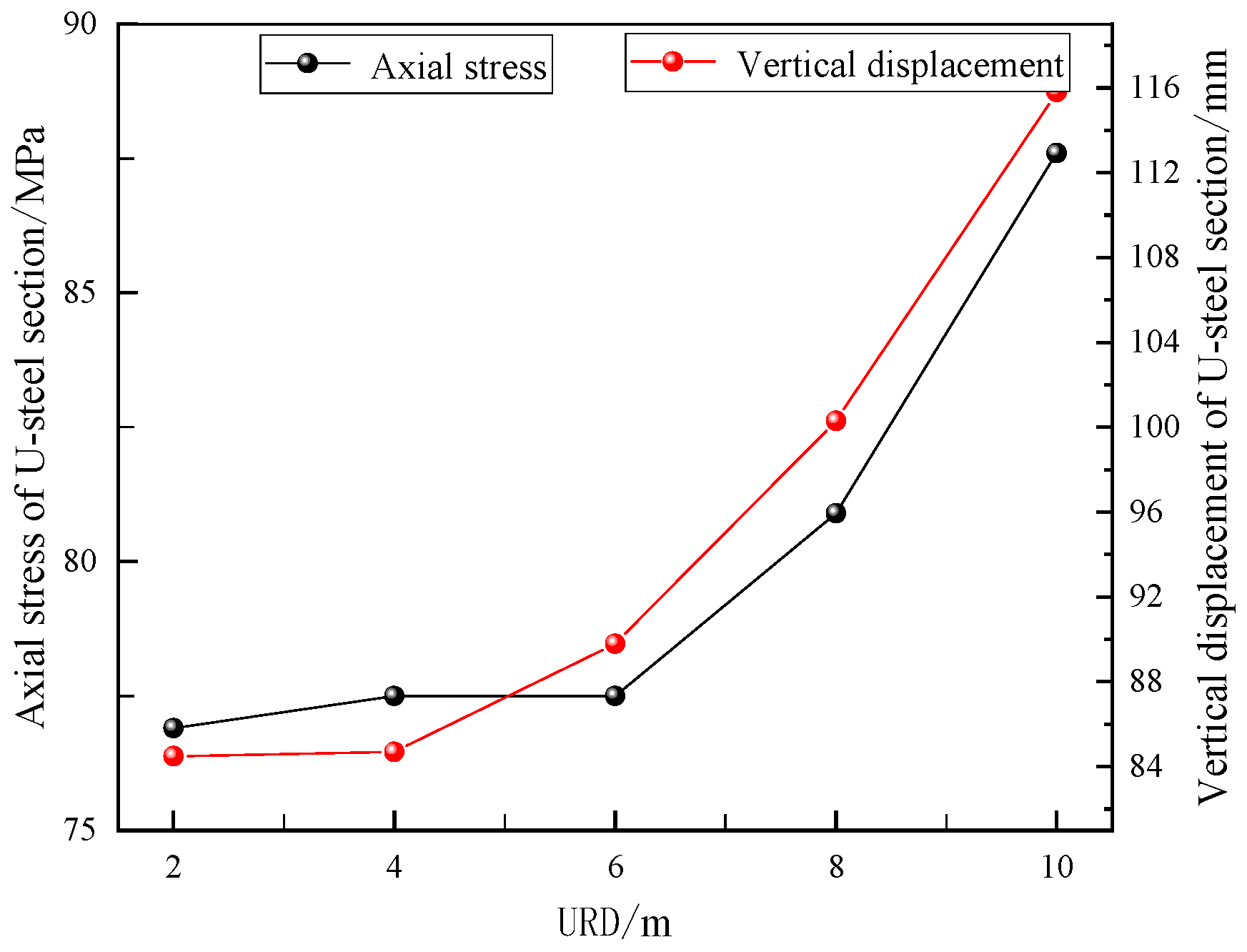
4.3. Influence of URD on U-Steel Shed Support
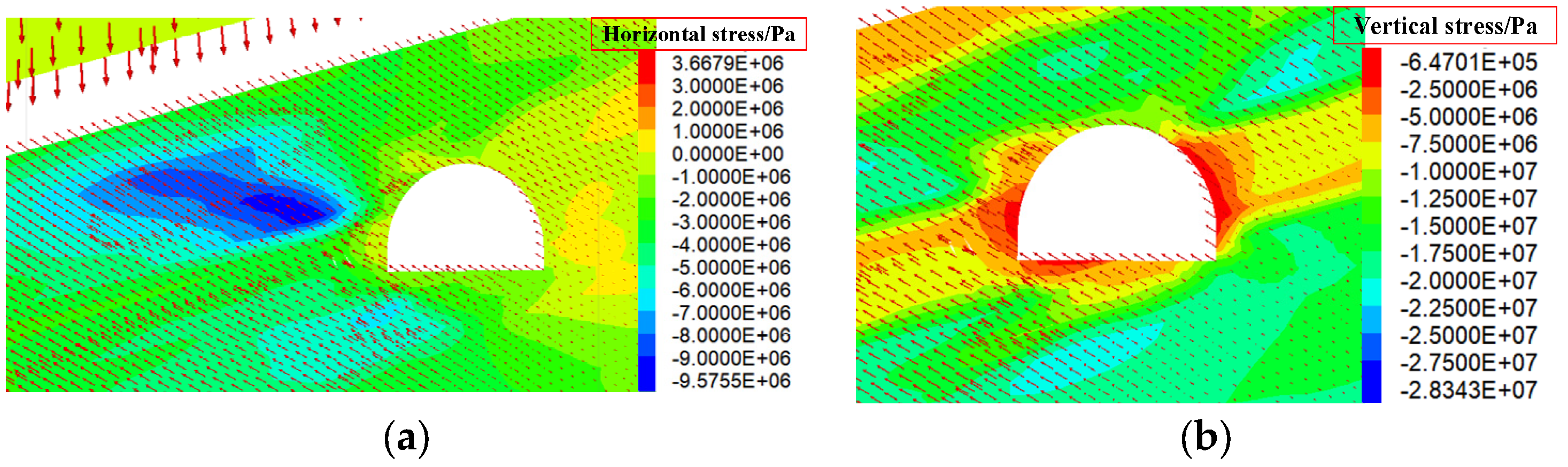
4.4. Influence of Maximum Horizontal Stress on Roadway Surrounding Rock Stability in Unsupported Roof Area
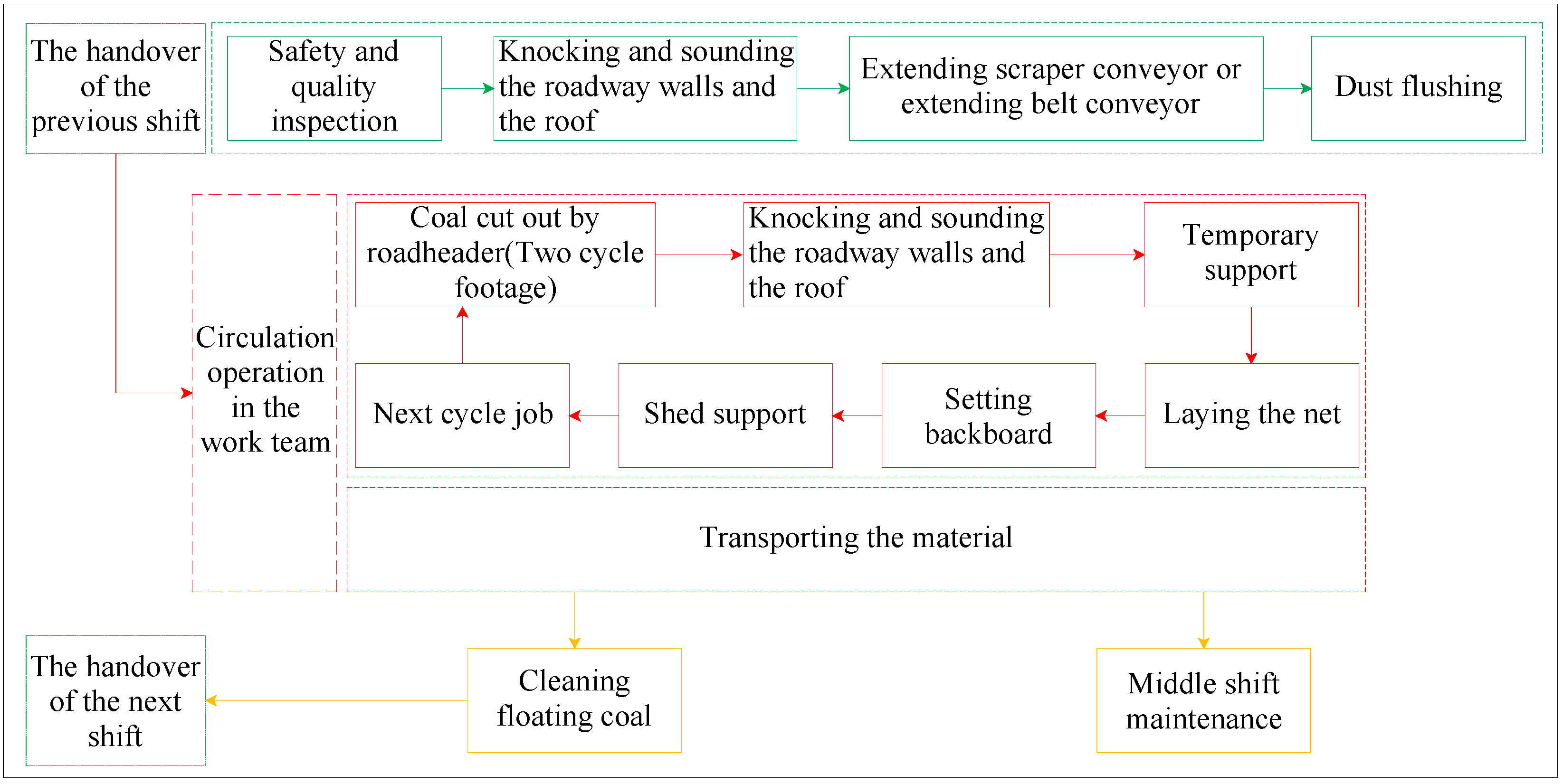
5. Design and Performance of Modified Rapid Heading Equipment
- (1)
- The roadway is supported by shed support, and it has a large section. U-steel support is both large and heavy. When installing shed support, temporary scaffolds have to be put up, and a back plate has to be inserted in advance. This requires a lot of labor and time. Manual shed installation is time and labor consuming. The safety factor and cycle efficiency are both low.
- (2)
- The temporary support devices for traditional roadheaders are generally suitable for flat-roofed and immediately supported roadways or those permanently supported by rock bolts. For shed roadways, as effective temporary support is not available when installing shed support, the workers usually have to operate under an exposed roof, and the safety factor is very low. To ensure safety, the maximum URD of the roadway has to be limited to a small value. Therefore, the heading efficiency is very low.
5.1. Combined Onboard Standing Platform + Onboard Beam Lift Device
5.2. New Onboard Temporary Support Device for Long Excavation
6. Field Application and Effect
7. Conclusions
- (1)
- Based on the thin shell theory, a top cover cylindrical shell model for roadways with an arch section was built. The formula for calculating the maximum URD of an arch roof was derived. The influences of roadway width, tensile strength, buried depth, and arch height on, and their contributions to, the maximum URD were analyzed. The results showed that arch roadways are highly adaptive to varying geological conditions, and the theoretical maximum URD for the 3098 heading face is 2.4 m.
- (2)
- Numerical simulation revealed that when the URD is around 2 m, the roadway surrounding rock is well stable and the roadway is free of tensile failure. When the URD increases beyond 4 m, tensile failure occurs on the roadway sides and the roadway becomes less stable. Therefore, a maximum URD of 2–3 was used for numerical calculation. New failure occurs on the arch roof with the increase in URD, suggesting that an arch roof has the best stability. Properly increasing URD helps a U-steel to gain support strength quickly. In the shallow part of the roadway surrounding rock under goaf, where failures are prominently concentrated, as further failure will occur at points where horizontal stresses are concentrated, failures are primarily determined by horizontal stresses.
- (3)
- A combined onboard standing platform + onboard beam lift device and a new onboard temporary support device for long excavation were developed as a solution to the low shed and temporary support efficiency for roadways with a URD of 2.4 m. When these devices are used on-site in conjunction with the optimized process flow, the average footage per month is over 550 m with a peak of 846.4 m; the footage per shift is 16 supports totaling 14.1 m. The forming quality of a shed-supported roadway is good enough to accommodate normal mining of the working face, consequently the rapid heading of the roadway.
Author Contributions
Funding
Conflicts of Interest
References
- Shi, Y.; Ye, Y.; Hu, N.; Jiao, Y.; Wang, X. Physical Simulation Test on Surrounding Rock Deformation of Roof Rockburst in Continuous Tunneling Roadway. Minerals 2021, 11, 1335. [Google Scholar] [CrossRef]
- Wang, X.R.; Wang, E.Y.; Liu, X.F. Micromechanisms of coal fracture: Insights from quantitative AE technique. Theor. Appl. Fract. Mech. 2021, 114, 103000. [Google Scholar] [CrossRef]
- Wang, G.; Guo, Y.; Wang, P. A new experimental apparatus for sudden unloading of gas-bearing coal. Bull. Eng. Geol. Environ. 2020, 79, 857–868. [Google Scholar] [CrossRef]
- Wang, K.; Guo, Y.; Xu, H. Deformation and permeability evolution of coal during axial stress cyclic loading and unloading: An experimental study. Geomech. Eng. 2021, 24, 519–529. [Google Scholar]
- Wang, H.; Wang, L.J.; Zhang, X.F. Theory and technology of efficient excavation with the integration of excavation and anchor. J. China Coal Soc. 2020, 45, 2021–2030. [Google Scholar]
- Cheng, H.; Tang, B.; Tang, Y.Z.; Yao, Z.S.; Wang, C.B.; Rong, C.X. Full face Hard Rock Roadheader for deep roadway and its key technology for rapid construction. J. China Coal Soc. 2020, 45, 3314–3324. [Google Scholar]
- Du, Q.J.; Zhao, Q.F.; Yang, Z.; Guo, J.Z. Research and Practice on rapid tunneling of large section coal roadway under complex geological conditions. Coal Eng. 2013, 45, 76–79. [Google Scholar]
- Yang, R.S.; Wang, X. Study on rapid tunneling construction technology of large section semi coal rock roadway. China Min. Mag. 2012, 21, 87–88. [Google Scholar]
- Wang, X.F.; Zhang, D.S.; Shao, P.; Zhang, W. Rapid tunneling technology of deep soft rock bolt shotcrete support roadway. J. Min. Saf. Eng. 2011, 28, 415–419. [Google Scholar]
- Rostami, J. Development of a Force Estimation Model for Rock Fragmentation with Disc Cutters through Theoretical Modeling and Physical Measurement of Crushed Zone Pressure; Colorado School of Mines: Golden, CO, USA, 1997; Volume 38, pp. 56–64. [Google Scholar]
- Yi, H.; Ouyang, Z.; Zhou, X.; Li, Z.; Chen, J.; Li, K.; Liu, K. Study on the Modification of Confining Rock for Protecting Coal Roadways against Impact Loads from a Roof Stratum. Minerals 2021, 11, 1331. [Google Scholar] [CrossRef]
- Ahmad, M.; Hu, J.L.; Hadzima Nyarko, M.; Ahmad, F.; Tang, X.W.; Rahman, Z.U.; Nawaz, A.; Abrar, M. Rockburst Hazard Prediction in Underground Projects Using Two Intelligent Classification Techniques: A Comparative Study. Symmetry 2021, 13, 632. [Google Scholar] [CrossRef]
- Aslannezhad, M.; Keshavarz, A.; Kalantariasl, A.; Aslannezhad, M.; Keshavarz, A.; Kalantariasl, A. Evaluation of mechanical, chemical, and thermal effects on wellbore stability using different rock failure criteria. J. Nat. Gas Sci. Eng. 2020, 78, 103278. [Google Scholar] [CrossRef]
- Hu, G.J.; Yang, T.H.; Zhou, J.R.; Yu, Q.L.; Xie, L.K.; Liu, H.L.; Zhao, Y.; Taheri, A. Mechanism of Surrounding Rock Failure and Crack Evolution Rules in Branched Pillar Recovery. Minerals 2017, 7, 96. [Google Scholar] [CrossRef] [Green Version]
- Justo, J.; Castro, J.; Cicero, S.; Sanchez, C.M.; Husillos, R. Notch effect on the fracture of several rocks: Application of the Theory of Critical Distances. Theor. Appl. Fract. Mech. 2017, 90, 251–258. [Google Scholar] [CrossRef] [Green Version]
- Girard, J.; Silber, R.E.; Mohiuddin, A.; Chen, H.Y.; Karato, S. Development of a Stress Sensor for In-Situ High-Pressure Deformation Experiments Using Radial X-ray Diffraction. Minerals 2020, 10, 166. [Google Scholar] [CrossRef] [Green Version]
- Yu, Y.; Wang, X.; Bai, J.; Zhang, L.; Xia, H. Deformation Mechanism and Stability Control of Roadway Surrounding Rock with Compound Roof: Research and Applications. Energies 2020, 13, 1350. [Google Scholar] [CrossRef]
- Zhang, N.; Han, C.L.; Xie, Z.Z. Theory and efficient support technology of continuous beam roof control in coal roadway. J. Min. Form. Control. Eng. 2019, 1, 48–55. [Google Scholar]
- Ma, H.W.; Wang, S.B.; Mao, Q.H.; Shi, Z.W.; Zhang, X.H.; Yang, Z.; Cao, X.G.; Xue, X.S.; Xia, J.; Wang, C.W. Key common technologies for intelligent excavation of coal mine roadways. J. China Coal Soc. 2021, 46, 310–320. [Google Scholar]
- Ma, H.W.; Wang, P.; Wang, S.B.; Mao, Q.H.; Shi, Z.W.; Xia, J.; Yang, Z.; Xue, X.S.; Wang, C.W. Intelligent parallel collaborative control method for coal mine excavation robot system. J. China Coal Soc. 2021, 46, 2057–2067. [Google Scholar]
- Zhang, Z.Q.; Chen, F.F.; Li, N.; He, M.M.; Moayedi, H. Influence of Fault on the Surrounding Rock Stability for a Mining Tunnel: Distance and Tectonic Stress. Adv. Civ. Eng. 2019, 2019, 2054938. [Google Scholar] [CrossRef]



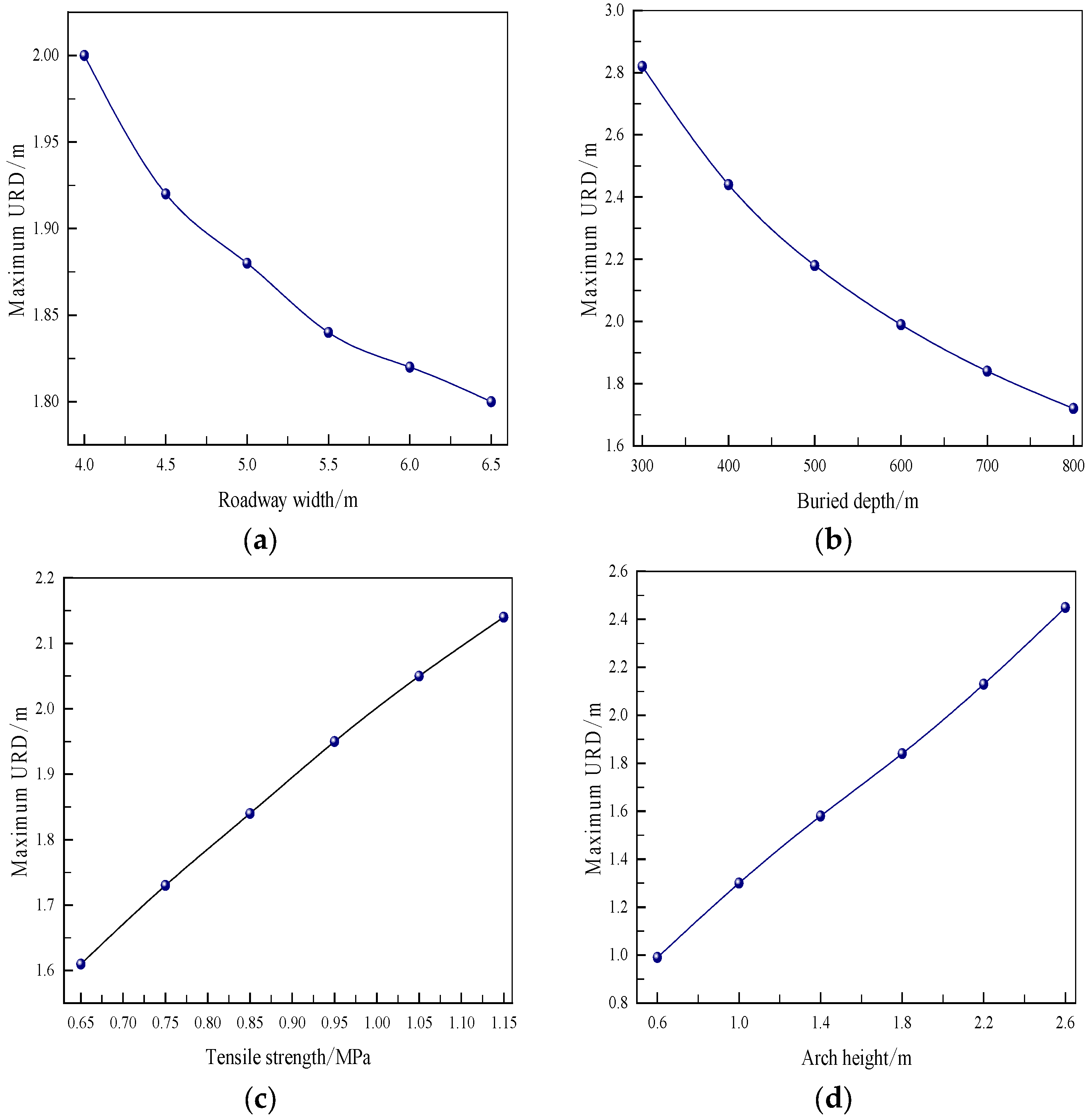
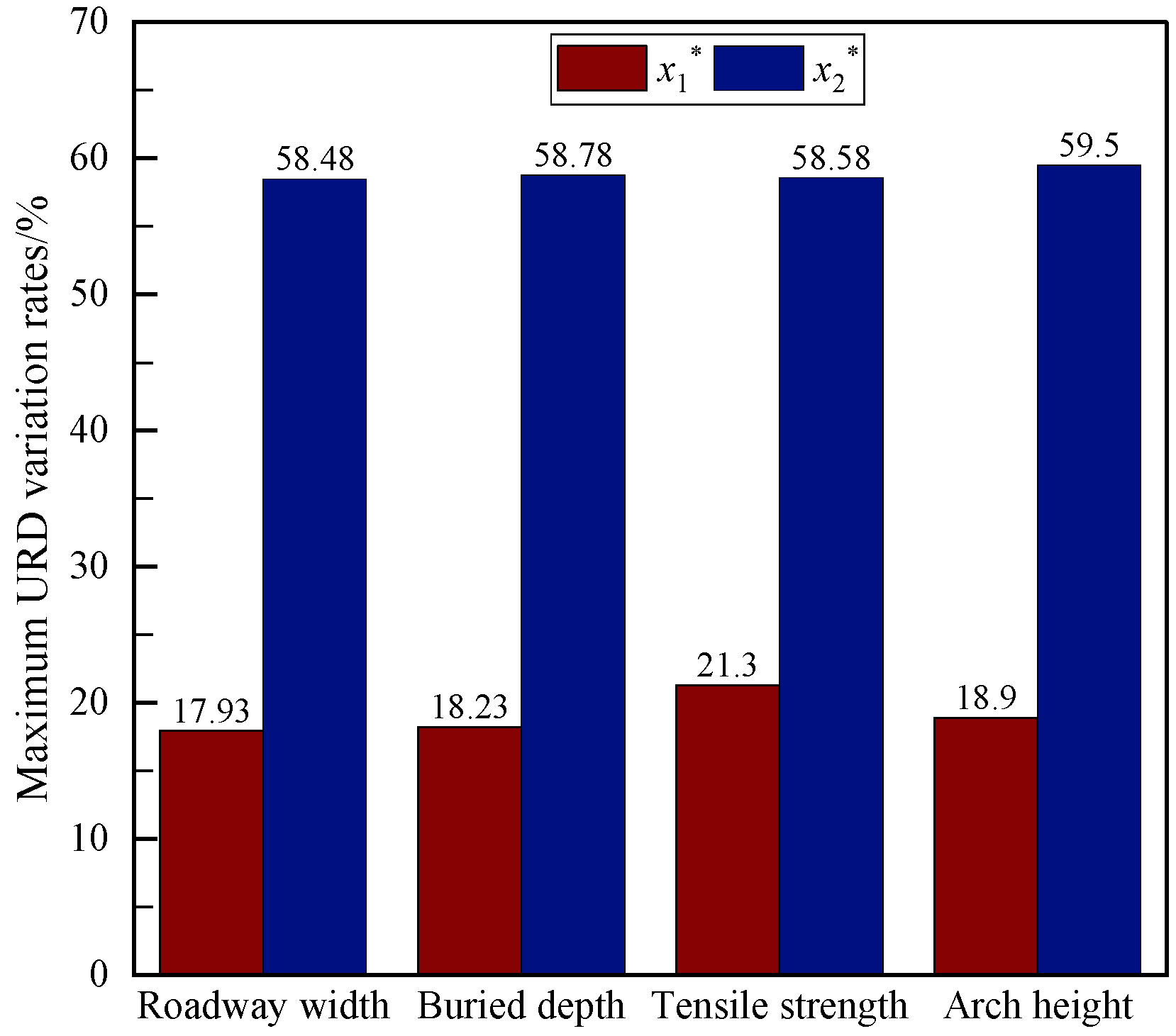





| Roadway Width/m | 4.0 | 4.5 | 5.0 | 5.5 | 6.0 | 6.5 |
|---|---|---|---|---|---|---|
| Theoretical max URD/m | 2.0 | 1.92 | 1.88 | 1.84 | 1.82 | 1.8 |
| Buried depth/m | 300 | 400 | 500 | 600 | 700 | 800 |
| Theoretical max URD/m | 2.82 | 2.44 | 2.18 | 1.99 | 1.84 | 1.72 |
| Tensile strength/MPa | 0.65 | 0.75 | 0.85 | 0.95 | 1.05 | 1.15 |
| Theoretical max URD/m | 1.61 | 1.73 | 1.84 | 1.95 | 2.05 | 2.14 |
| Arch height/m | 0.6 | 1.0 | 1.4 | 1.8 | 2.2 | 2.6 |
| Theoretical max URD/m | 0.99 | 1.3 | 1.58 | 1.84 | 2.13 | 2.45 |
| Rock Stratum | Thickness (m) | Unit Weight (kg·m−3) | Bulk Modulus (GPa) | Shear Modulus (GPa) | Internal Angle of Friction (°) | Tensile Strength (MPa) | Cohesion (MPa) |
|---|---|---|---|---|---|---|---|
| Siltstone | 13.4 | 2·560 | 2.1 | 1.6 | 38 | 1.935 | 2.1 |
| Carbonaceous clay rock | 1.7 | 2·530 | 2.0 | 1.5 | 36 | 1.175 | 1.9 |
| Siltstone | 2.3 | 2·650 | 3.34 | 2.8 | 28 | 1.36 | 1.68 |
| Fine sandstone | 5.1 | 2·700 | 2.58 | 1.88 | 42 | 2.61 | 2.4 |
| Siltstone | 4.2 | 2·560 | 2.1 | 1.6 | 38 | 1.935 | 2.1 |
| 8# coal | 3.6 | 1·380 | 1.8 | 1.43 | 28 | 0.85 | 1.1 |
| Siltstone | 1.5 | 2·560 | 2.1 | 1.6 | 38 | 1.935 | 2.1 |
| Medium fine sandstone | 2.5 | 2·600 | 2.3 | 1.8 | 40 | 2.15 | 2.2 |
| Siltstone fine sandstone interbedded | 1.3 | 2·710 | 2.69 | 1.98 | 42 | 2.675 | 2.5 |
| 9# coal | 2.8 | 1·380 | 1.8 | 1.43 | 28 | 0.85 | 1.1 |
| Siltstone | 1.6 | 2·560 | 2.1 | 1.6 | 38 | 1.935 | 2.1 |
| Fine sandstone | 3.4 | 2·700 | 2.58 | 1.88 | 42 | 2.61 | 2.4 |
| Siltstone | 2.5 | 2·560 | 2.1 | 1.6 | 38 | 1.935 | 2.1 |
| Fine sandstone | 5.8 | 2·700 | 2.58 | 1.88 | 42 | 2.61 | 2.4 |
| Series | Input and Use Time | Features |
|---|---|---|
| 1st generation | 2009.8–2016.4 | One single manipulator, hydraulic powered, serves as a mechanical beam lift only. |
| 2nd generation | 2016.5–2017.4 | A standing platform added to the 1st generation to allow the worker to insert the back plate for the upper roof support safely and provide the needed standing space. |
| 3rd generation | 2017.5–present | A 90° rotating beam fixing function added to the 2nd generation so that when the roof is broken, the operator can fix the beam from the side instead of the front of the heading face and then rotate the beam to the heading face prior to the next step. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bi, Y.; Wang, M.; Wu, C.; Huang, Y. Surrounding Rock Stability in Unsupported Roof Area and Rapid Heading Technique for Deep Arch Coal Roadways under Goaf. Minerals 2022, 12, 1329. https://doi.org/10.3390/min12101329
Bi Y, Wang M, Wu C, Huang Y. Surrounding Rock Stability in Unsupported Roof Area and Rapid Heading Technique for Deep Arch Coal Roadways under Goaf. Minerals. 2022; 12(10):1329. https://doi.org/10.3390/min12101329
Chicago/Turabian StyleBi, Yewu, Mingxing Wang, Chao Wu, and Yucheng Huang. 2022. "Surrounding Rock Stability in Unsupported Roof Area and Rapid Heading Technique for Deep Arch Coal Roadways under Goaf" Minerals 12, no. 10: 1329. https://doi.org/10.3390/min12101329
APA StyleBi, Y., Wang, M., Wu, C., & Huang, Y. (2022). Surrounding Rock Stability in Unsupported Roof Area and Rapid Heading Technique for Deep Arch Coal Roadways under Goaf. Minerals, 12(10), 1329. https://doi.org/10.3390/min12101329





