A Chemical Separation and Measuring Technique for Titanium Isotopes for Titanium Ores and Iron-Rich Minerals
Abstract
:1. Introduction
2. Materials and Methods
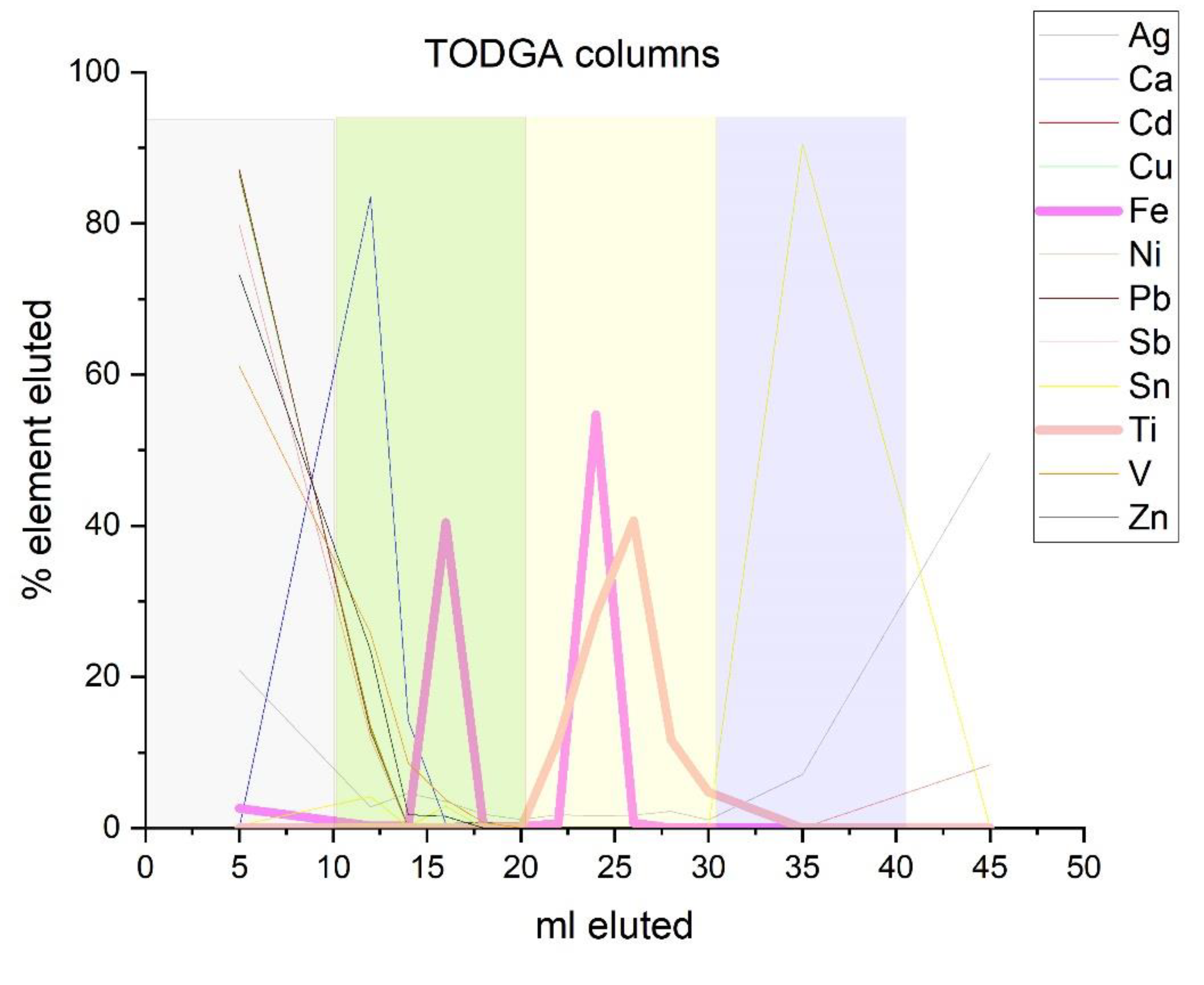
2.1. Sample Preparation and Ion-Exchange Chromatography
2.2. Mass Spectrometry
3. Discussion
3.1. Rationale to Use 46Ti, 47Ti, and 49Ti, Mass Dependence, and the Bracketing Approach
- (1)
- The concentrations of Ti in the solutions are high (dissolution of materials that have Ti in 1000 of ppm to % level ranges), which demands copious amounts of spike or an extraordinarily low amount of sample. This is why multiple scientists analyze transition metal isotopes, such as Ti, in higher-concentration materials (for instance, Zn, Mo, Sb, and Sn [20,23,24,25,26,27,28,29,30,31,32,33,34]) and correct for mass bias with the purification and bracketing techniques presented here.
- (2)
- (3)
- Although mass bias corrections with bracketing provide significantly higher errors, as demonstrated here, the full procedural error of approximately 0.1‰ is adequate to differentiate the variations observed in 2 mineral and 4 solution standards, which span over 5 per mil. Given the significant isotopic ranges observed in these materials and the known Ti-isotope values in the published works, the higher error limits the discrimination of differences among the materials. Equally important, Zhu et al. [2] presented the results using this technique for Ti-isotope compositions in rocks, which possess a more complicated chemical matrix associated with the dissolution of rocks that possess multiple-mineral species
3.2. Error Analysis and the Importance of Dry Plasma in Measurements
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Niederer, F.; Papanastassiou, D.; Wasserburg, G. The Isotopic Composition of Titanium in the Allende and Leoville Meteorites. Geochim. Cosmochim. Acta 1981, 45, 1017–1031. [Google Scholar] [CrossRef]
- Zhu, X.K. High Precision Measurement of Titanium Isotope Ratios by Plasma Source Mass Spectrometry. Int. J. Mass Spectrom. 2002, 220, 21–29. [Google Scholar] [CrossRef]
- Zhang, J. A New Method for MC-ICPMS Measurement of Titanium Isotopic Composition: Identification of Correlated Isotope Anomalies in Meteorites. J. Anal. At. Spectrom. 2011, 26, 2197–2205. [Google Scholar] [CrossRef]
- Torrano, Z.A. Titanium Isotope Signatures of Calcium-Aluminum-Rich Inclusions from CV and CK Chondrites: Implications for Early Solar System Reservoirs and Mixing. Geochim. Cosmochim. Acta 2019, 263, 13–30. [Google Scholar] [CrossRef] [PubMed]
- Makishima, A. Separation of Titanium from Silicates for Isotopic Ratio Determination Using Multiple Collector ICP-MS. J. Anal. At. Spectrom. 2002, 17, 1290–1294. [Google Scholar] [CrossRef]
- Johnson, A.C. Titanium Isotopic Fractionation in Kilauea Iki Lava Lake Driven by Oxide Crystallization. Geochim. Cosmochim. Acta 2019, 264, 180–190. [Google Scholar] [CrossRef]
- Hinton, R.W.; Davis, A.M.; Scatena-Wachel, D.E. Large Negative Ti-50 Anomalies in Refractory Inclusions from the Murchison Carbonaceous Chondrite-Evidence for Incomplete Mixing of Neutron-Rich Supernova Ejecta into the Solar System. Astrophys. J. 1987, 313, 420–428. [Google Scholar] [CrossRef]
- Fahey, A. 26Al, 244Pu, 50Ti, REE, and trace element abundances in hibonite grains from CM and CV meteorites. Geochim. Cosmochim. Acta 1987, 51, 329–350. [Google Scholar] [CrossRef]
- Zhang, J. Calcium and Titanium Isotopic Fractionations during Evaporation. Geochim. Cosmochim. Acta 2014, 140, 365–380. [Google Scholar] [CrossRef]
- Millet, M.-A. Titanium Stable Isotope Investigation of Magmatic Processes on the Earth and Moon. Earth Planet. Sci. Lett. 2016, 449, 197–205. [Google Scholar] [CrossRef] [Green Version]
- Wang, W. Equilibrium Inter-Mineral Titanium Isotope Fractionation: Implication for High-Temperature Titanium Isotope Geochemistry. Geochim. Cosmochim. Acta 2020, 269, 540–553. [Google Scholar] [CrossRef]
- Williams, N.H. Titanium Isotope Fractionation in Solar System Materials. Chem. Geol. 2021, 568, 120009. [Google Scholar] [CrossRef]
- Greber, N.D. Titanium Isotopic Evidence for Felsic Crust and Plate Tectonics 3.5 Billion Years Ago. Science 2017, 357, 1271–1274. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Deng, Z. Titanium Isotopes as a Tracer for the Plume or Island Arc Affinity of Felsic Rocks. Proc. Natl. Acad. Sci. USA 2019, 116, 1132–1135. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhao, X. Titanium Isotopic Fractionation during Magmatic Differentiation. Contrib. Mineral. Petrol. 2020, 175, 1–16. [Google Scholar] [CrossRef]
- Hoare, L. Melt Chemistry and Redox Conditions Control Titanium Isotope Fractionation during Magmatic Differentiation. Geochim. Cosmochim. Acta 2020, 282, 38–54. [Google Scholar] [CrossRef]
- Millet, M.-A.; Dauphas, N. Ultra-Precise Titanium Stable Isotope Measurements by Double-Spike High Resolution MC-ICP-MS. J. Anal. At. Spectrom. 2014, 29, 1444–1458. [Google Scholar] [CrossRef]
- Marechal, C.N.; Telouk, P.; Albarede, F. Precise Analysis of Copper and Zinc Isotopic Compositions by Plasma-Source Mass Spectrometry. Chem. Geol. 1999, 156, 251–273. [Google Scholar] [CrossRef]
- Mathur, R. Cu Isotopic Fractionation in the Supergene Environment with and without Bacteria. Geochim. Cosmochim. Acta 2005, 69, 5233–5246. [Google Scholar] [CrossRef]
- Mason, T.F.D. Zn and Cu Isotopic Variability in the Alexandrinka Volcanic-Hosted Massive Sulphide (VHMS) Ore Deposit, Urals, Russia. Chem. Geol. 2005, 221, 170–187. [Google Scholar] [CrossRef]
- Rudge, J.F.; Reynolds, B.C.; Bourdon, B. The Double Spike Toolbox. Chem. Geol. 2009, 265, 420–431. [Google Scholar] [CrossRef]
- John, S.G. Optimizing Sample and Spike Concentrations for Isotopic Analysis by Double-Spike ICPMS. J. Anal. At. Spectrom. 2012, 27, 2123–2131. [Google Scholar] [CrossRef]
- Weiss, D.J. Controls of Cu and Zn Isotope Variability within a VHMS Deposit. Abstracts with Programs; Geological Society of America: Boulder, CO, USA, 2004; Volume 36. [Google Scholar]
- John, S.G. Zinc Stable Isotopes in Seafloor Hydrothermal Vent Fluids and Chimneys. Earth Planet. Sci. Lett. 2008, 269, 17–28. [Google Scholar] [CrossRef]
- Zeng, Z. Iron, Copper, and Zinc Isotopic Fractionation in Seafloor Basalts and Hydrothermal Sulfides. Mar. Geol. 2021, 436, 106491. [Google Scholar] [CrossRef]
- Barling, J.; Arnold, G.L.; Anbar, A.D. Natural Mass-Dependent Variations in the Isotopic Composition of Molybdenum. Earth Planet. Sci. Lett. 2001, 193, 447–457. [Google Scholar] [CrossRef]
- Hannah, J.L. Molybdenum Isotope Variations in Molybdenite: Vapor Transport and Rayleigh Fractionation of Mo. Geology 2007, 35, 703–706. [Google Scholar] [CrossRef]
- Mathur, R. Variation of Mo Isotopes from Molybdenite in High-Temperature Hydrothermal Ore Deposits. Miner. Depos. 2010, 45, 43–50. [Google Scholar] [CrossRef]
- Zhai, D. Antimony Isotope Fractionation in Hydrothermal Systems. Geochim. Cosmochim. Acta 2021, 306, 84–97. [Google Scholar] [CrossRef]
- Yamazaki, E. Feasibility Studies of Sn Isotope Composition for Provenancing Ancient Bronzes. J. Archaeol. Sci. 2014, 52, 458–467. [Google Scholar] [CrossRef]
- Brügmann, G.; Berger, D.; Pernicka, E. Determination of the Tin Stable Isotopic Composition in Tin-Bearing Metals and Minerals by MC-ICP-MS. Geostand. Geoanal. Res. 2017, 41, 437–448. [Google Scholar] [CrossRef]
- Badullovich, N. Tin Isotopic Fractionation during Igneous Differentiation and Earth’s Mantle Composition. Geochem. Perspect. Lett. 2017, 5, 24–28. [Google Scholar] [CrossRef] [Green Version]
- Mason, A. Provenance of Tin in the Late Bronze Age Balkans Based on Probabilistic and Spatial Analysis of Sn Isotopes. J. Archaeol. Sci. 2020, 122, 105181. [Google Scholar] [CrossRef]
- She, J.-X. Sn Isotope Fractionation during Volatilization of Sn (IV) Chloride: Laboratory Experiments and Quantum Mechanical Calculations. Geochim. Cosmochim. Acta 2020, 269, 184–202. [Google Scholar] [CrossRef]
- Klaver, M.; Coath, C.D. Obtaining Accurate Isotopic Compositions with the Double Spike Technique: Practical Considerations. Geostand. Geoanal. Res. 2019, 43, 5–22. [Google Scholar] [CrossRef] [Green Version]
- Shirey, S.B.; Walker, R.J. Carius Tube Digestion for Low-Blank Rhenium-Osmium Analysis. Anal. Chem. 1995, 67, 2136–2141. [Google Scholar] [CrossRef]
- Kurzweil, F. Accurate Stable Tungsten Isotope Measurements of Natural Samples Using a 180 W–183 W Double-Spike. Chem. Geol. 2018, 476, 407–417. [Google Scholar] [CrossRef]
- Blichert-Toft, J.; Chauvel, C.; Albarède, F. Separation of Hf and Lu for High-Precision Isotope Analysis of Rock Samples by Magnetic Sector-Multiple Collector ICP-MS. Contrib. Mineral. Petrol. 1997, 127, 248–260. [Google Scholar] [CrossRef]
- Albarede, F. Precise and accurate isotopic measurements using multiple-collector ICPMS. Geochim. Cosmochim. Acta 2004, 68, 2725–2744. [Google Scholar] [CrossRef]
- Horn, I. In Situ Iron Isotope Ratio Determination Using UV-Femtosecond Laser Ablation with Application to Hydrothermal Ore Formation Processes. Geochim. Cosmochim. Acta 2006, 70, 3677–3688. [Google Scholar] [CrossRef]
- Kuhn, H.-R.; Pearson, N.; Jackson, S. The Influence of the Laser Ablation Process on Isotopic Fractionation of Copper in LA-MC-ICP-MS. J. Anal. At. Spectrom. 2007, 22, 547–552. [Google Scholar] [CrossRef]
- Greber, N.D. Titanium Isotopic Compositions of Bulk Rocks and Mineral Separates from the Kos Magmatic Suite: Insights into Fractional Crystallization and Magma Mixing Processes. Chem. Geol. 2021, 578, 120303. [Google Scholar] [CrossRef]

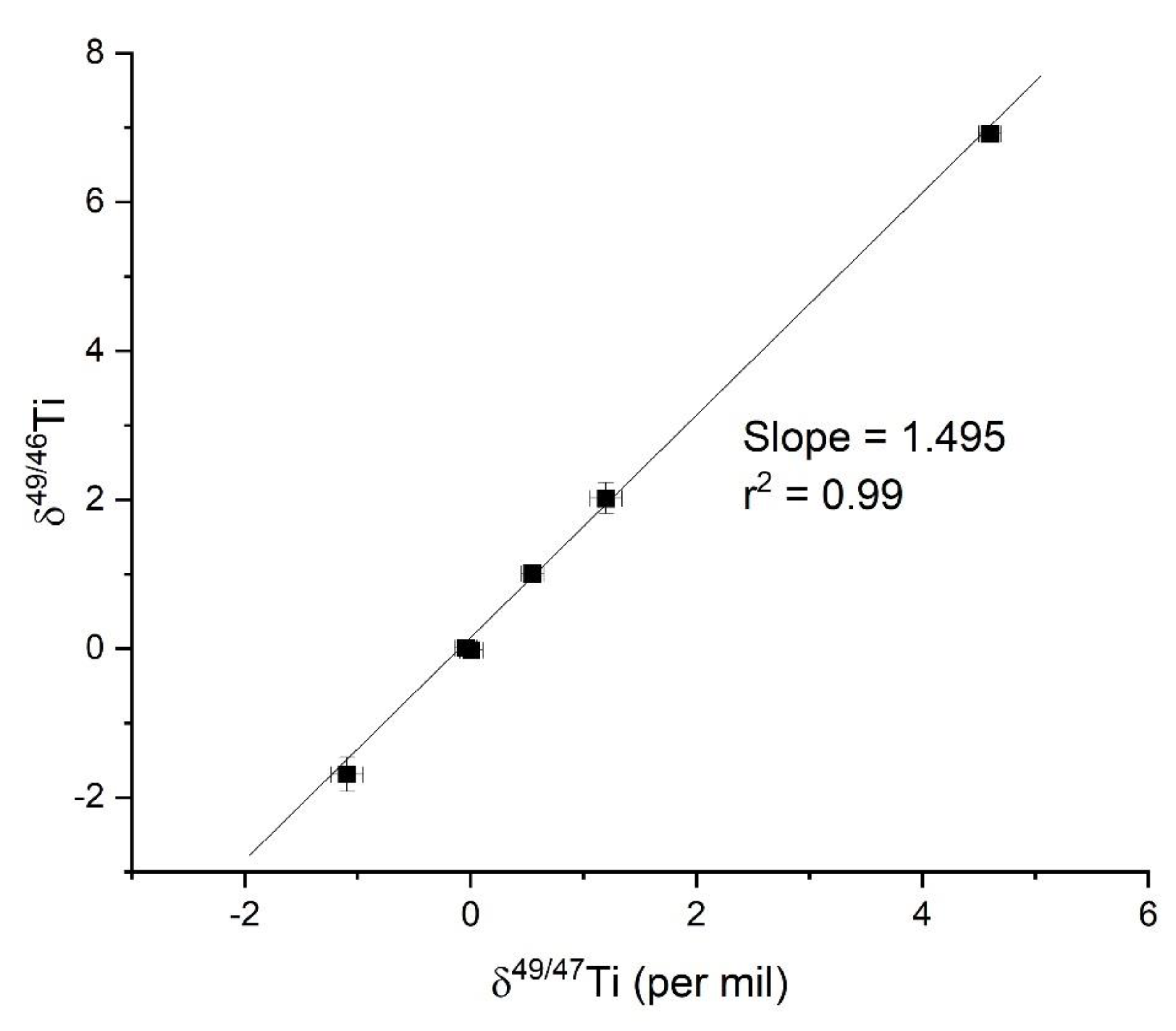
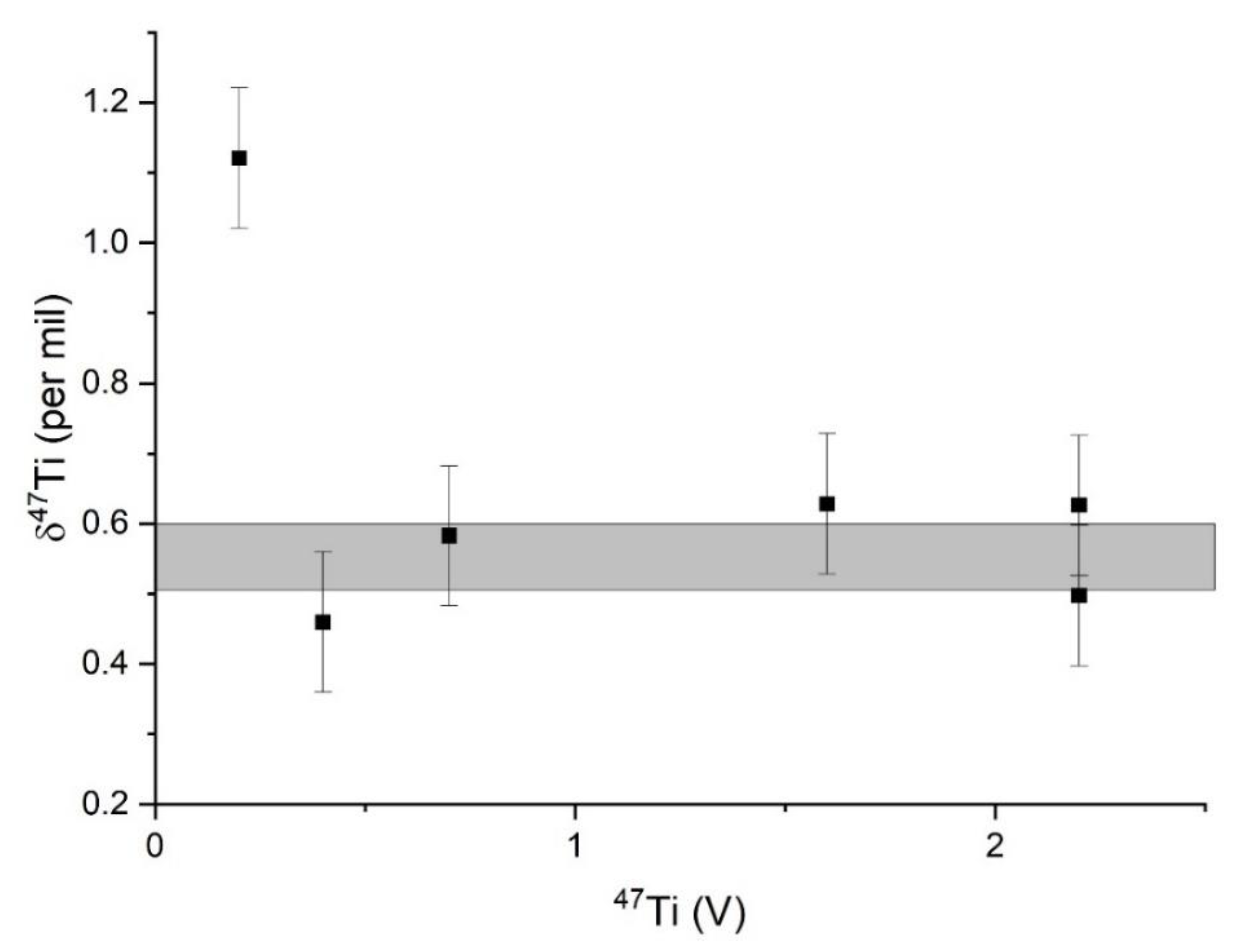
| Sample | Analyte | δ49/47Ti | 1σ | δ49/46Ti | 1σ | A.S. | n | Location | I.C. |
|---|---|---|---|---|---|---|---|---|---|
| LC 05 82.6 | Magnetite | 1.21 | 0.11 | 2.02 | 0.14 | 8 | 8 | Rutgers | Yes |
| LC 05 82.6 1 | Magnetite | 1.07 | 0.08 | 1.92 | 0.09 | 1 | 2 | WSU | Yes |
| CM3218 | Rutile | −0.04 | 0.06 | 0.01 | 0.06 | 2 | 4 | Rutgers | Yes |
| CM3218 | Rutile | −0.12 | 0.06 | −0.09 | 0.06 | 1 | 2 | Rutgers | No |
| Alfa Star | Standard sol | −0.04 | 0.04 | 0.01 | 0.04 | 2 | 4 | Rutgers | Yes |
| VWR | Fe–Ti oxide | −1.64 | 0.06 | −2.24 | 0.1 | 1 | 9 | Rutgers | Yes |
| Sample | δ49/47Ti1 | 1σ | n | δ49/47Ti2 | 1σ | n | δ49/47Ti3 | 1σ | n |
|---|---|---|---|---|---|---|---|---|---|
| Wet plasma (WSU) | 1.5 | 0.25 | 12 | 5.1 | 0.3 | 8 | 0.25 | 0.31 | 13 |
| Dry plasma (WSU) | 0.55 | 0.05 | 14 | 4.6 | 0.1 | 10 | 0.06 | 0.03 | 12 |
| Dry plasma (Rutgers) | n.a. | n.a. | n.a. | 4.6 | 0.07 | 6 | −0.09 | 0.05 | 7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mathur, R.; Emproto, C.; Simon, A.C.; Godfrey, L.; Knaack, C.; Vervoort, J.D. A Chemical Separation and Measuring Technique for Titanium Isotopes for Titanium Ores and Iron-Rich Minerals. Minerals 2022, 12, 644. https://doi.org/10.3390/min12050644
Mathur R, Emproto C, Simon AC, Godfrey L, Knaack C, Vervoort JD. A Chemical Separation and Measuring Technique for Titanium Isotopes for Titanium Ores and Iron-Rich Minerals. Minerals. 2022; 12(5):644. https://doi.org/10.3390/min12050644
Chicago/Turabian StyleMathur, Ryan, Christopher Emproto, Adam C. Simon, Linda Godfrey, Charles Knaack, and Jeffery D. Vervoort. 2022. "A Chemical Separation and Measuring Technique for Titanium Isotopes for Titanium Ores and Iron-Rich Minerals" Minerals 12, no. 5: 644. https://doi.org/10.3390/min12050644
APA StyleMathur, R., Emproto, C., Simon, A. C., Godfrey, L., Knaack, C., & Vervoort, J. D. (2022). A Chemical Separation and Measuring Technique for Titanium Isotopes for Titanium Ores and Iron-Rich Minerals. Minerals, 12(5), 644. https://doi.org/10.3390/min12050644






