Long- and Short-Term Strategies for Estimation of Hydraulic Fracturing Cost Using Fuzzy Logic

Abstract

Over two decades, block caving mining has developed the application of hydraulic fracturing as a preconditioning method. This study aims to estimate hydraulic fracturing costs in block caving operations and suggests the base case of specified costs based on the U.S. Energy Information Administration (EIA) report. Furthermore, it applies cavability factors to develop the long- and short-term strategies through the fuzzy inference system. In the long-term strategy, we suggest three possible scenarios for reducing the long-term strategy’s uncertainty by considering the association for the advancement of cost engineering (AACE)’s contingency rate. Moreover, each fuzzy membership function of the three possible redeveloped scenarios was analysed through arithmetic operations over independent/dependent fuzzy numbers for comparing each scenario. The outcome of flexible cost estimation suggested deciding on the scale of infrastructure and ore production by facilitating undercut propagation and controlling block height of block caving operation including additional fragmentation processes. The result of this study also illustrated that systematic fuzzy cost engineering could help estimate the initial stage of budgeting. In addition, through solving the uncertainty of fuzzy calculation values, the project schedule identification is presented by recognising the dependence on each scenario’s common characteristic of the cavability parameter and cost contingency rate.

1. Introduction

Block caving is a mass underground mining method that suitably applies for massive cavable orebodies. Due to the capability of large extraction in massive orebodies, the block caving method has the superiority of operating cost by economies of scale over any other underground mining method. Furthermore, including the facilitation of block caving mechanism helps obtain small fragmentation and reduce the entire cost of the comminution process. Even though block caving could induce a low-cost mining method through an automatic flow system, it is limited in application to uncavable hard rock masses that highly rely on the influence of gravitational force. Thus, the application of an effective hard rock-preconditioning method is vitally required to achieve the desired depth, production rate, and caving performance, such as cave initiation, cave propagation, and draw rates.

Preconditioning is a prerequisite and comprehensive process applied to perturb a rock mass property before cave initiation by altering/modifying its in situ geomechanical properties through destressing and fracturing [1]. These processes comprise treating the rock mass either by drilling a single hole and pressurising it with fluid, called Hydraulic Fracturing (HF) or by drilling up holes into the rock mass and packing them with explosives to break the in situ rock masses. The extensive use of preconditioning methods nowadays suggests that it may become a fundamental part of block caving.

Over the past two decades, applying the preconditioning technique used in the caving method has evolved to extract massive, deeper, and low-grade ore bodies. Various engineering and academic studies have been conducted considering the extraction of ore from larger and deeper mines, including Ridgeway Deeps [2,3], Northparkes mine [4,5,6], Cadia East mine [7], Salvador mine [8], and the El Teniente mine [9] which has a product range from 6 to 35 Mtpa with plans to increase production in future.

HF, before its application in the mining industry, has been widely used in the petroleum industry to recover trapped oil from low-porosity and low-permeability rock. It was used for the first time in mining operations at Northparkes mine (Australia) in 1997 [4]. The potential of its practical use in the full-scale mining industry is to mitigate the risks associated with underground mass mining, such as rockburst, by inducing micro-seismic events by injecting a fluid to cause a fault slippage [10]. For controlling the outburst in the coal mine, the massive HF method is recently used through surface HF in underground coal rock [11]. In addition, the contribution of the natural fracture is identified for flow channels when the HF technique is applied [12].

In the HF application, new fractures are created by inducing tensile stresses along the borehole boundary by applying pressure in an isolated section of the hole. Water or a water-based cross-linked polymer gel is injected in an isolated section between the two straddle packers. The fracture initiates along the borehole boundary, mostly below the borehole collar, after the pressure exceeds the rock mass tensile strength and propagates the fracture length by increasing the injection pressure. This results in a reduction of connection time between the two fractures during the caving process. The HF method could enhance the cavability, draw rate, and fragmentation size in block caving operations by connecting existing natural fractures and new fractures.

Even though there is a growth in demand for applying such preconditioning methods, there are difficulties estimating the economic cost/benefit. Since preconditioning techniques have just been applied to block caving operations, there are uncertainties in analysing costs in its practical application. In addition, a lack of knowledge for estimating the cost of preconditioning techniques causes hardship to the decision-makers. Thus, decision-makers should often rely on experts’ knowledge. Since the conventional approach of the mining industry flows with decision trees, it often has caused trouble with the imprecision of prediction because of the deterministic way of decision. To avoid these uncertainties, which in an operating situation always incur major problems in the mining industry, this study applied the fuzzy inference system (FIS) to develop a pragmatic decision-making support tool that enables a more smooth and practical approximation in a probabilistic way.

This study addresses the short-and long-term strategies of the HF cost estimation in block caving, where the proposed approach is not only reducing the uncertainty in the economic assessment of HF by using fuzzy modelling, but also addressing how to identify the dependence on cost measurement due to engineering variables and order the scenario between several cases. For fuzzy modelling in the short-term and long-term based on the characteristics of block caving, the input parameters would be selected from the cavability’s natural factors and induced factors, respectively. In a short-term strategy, the cost of HF was measured for one borehole by using the natural factor of rock mass, such as uniaxial compressive stress, in situ stress, water, and discontinuity properties. In a long-term strategy, it was modelled for a year-based project by induced factors that indicate the character of block caving, such as block height, draw rate, fragmentation, and propagation of caving. Through induced factors, we make different scenarios and differentiate both correlated cases and uncorrelated cases by considering the variable’s dependency on how to decide the contingency rate.

The rest of the paper is organised as follows. Section 2 describes the related case studies for fuzzy cost estimation. Section 3 provides a brief explanation based on the fuzzy set theory and discusses HF cost and cavability index. In Section 4, the results of fuzzy modelling in short-term strategy and long-term strategy suggest the application of contingency rate on the results of the defuzzified long-term strategy’s result. Finally, some conclusions are provided in Section 5.

2. Fuzzy Logic Applications into the Mining and Cost Estimation

The application of fuzzy logic in the mining industry is well explored. For handling the complex mine design and implementing the techniques, the trends of using fuzzy logic suggest a new approach to solving the issues related to traditional economic activity in mineral sectors [13]. Bandopadhyay [14] analysed the roof and floor conditions for mining method selection with fuzzy aggregation procedures. Den Hartog MH [15] developed a performance prediction of a rock-cutting trencher with a knowledge-based fuzzy model. In this paper, the developed model uses six input variables (rock strength, joint spacing of three joint sets, joint orientation, and trench dimensions) for estimating the performance of a trencher (bit consumption and production rates). Jiang, Y.M. [16] applied the fuzzy set theory in the evaluation of roof categories in longwall mining. Cabseoy, T. [17] and Bascetin, A. [18] used fuzzy set theory for the selection of optimum equipment combinations in surface mines. Yao, Y., Li, X., Yuan, Z. [19] developed fuzzy logic and a neural network approach by utilising data obtained from cutting tests. Wei, X., Wang, C.Y., Zhoub, Z.H. [20] suggested the fuzzy ranking system for evaluating the sawability of granite and for selecting a suitable tool and determining the optimum sawing parameters. Li, W. et al. [21] applied the fuzzy probability measures to the analysis on actual cases of excavation, mining, ground surface movement, and subsidence. M. Iphar [22] applied fuzzy sets to the diggability index (DI) rating method for surface mine equipment selection. They selected the inputs as weathering degree, rock strength (UCS), joint spacing, bedding spacing and put them into a fuzzy inference system for DI rating. Edrahim Ghaesei and Mohammad Ataei [23] proposed the roof fall rate, which was applied to fuzzy logic by using the input parameters as CMRR (coal mine roof rating), PRSUP (primary roof support), intersection span, and depth of cover. Dong, L.J. et al. [24] developed the evaluation index of a man–machine–environment system and established it for the clean and safe production grade of a phosphorous mine by using the grid-based fuzzy Borda method (GFB).

The fuzzy logic for the case of cost estimation analysis has had a few applications: Muñoz, M., & Miranda, E. [25] introduced a FIS for estimating the premium cost of an options exchange. It is applied in the derivative market in Mexico, and its results are compared with the Black–Sholes theoretical model. Kasie, F.M. et al. [26] proposed a decision support system that stabilises the flow of fixtures in manufacturing systems. This paper justifies the cost-effectiveness of the decision support system and strengthens the decision proposed using a similarity measure. Fayek, A.R., & Rodriguez Flores, J.R. [27] developed a fuzzy model to assess the quality of infrastructure projects at the conceptual cost estimating stage. In this paper, the fuzzy expert system provides a systematic approach for assessing the quality and cost of components to perform a fitness. Alshibani, A., & Hassanain, M.A. [28] applied the fuzzy set theory for estimating the maintenance cost of constructed facilities. Tony Chen [29] proposed an entropy-consensus agent-based fuzzy collaborative intelligence (FCI) for estimating the minimum unit cost of a semiconductor product, which has critical weakness due to insufficient consensus. Anthony, K. et al. [30] illustrated the construction of cost estimating relationships (CERs) using fuzzy sets and fuzzy inferencing procedures. Petley, G.J., & Edwards, D.W. [31] developed fuzzy matching to use in capital cost estimation about the plant materials of constructions. Zima & Krzysztof [32] suggested fuzzy case-based reasoning (CBR) for estimating costs in the early phase of the construction project. Shaheen, A. A. et al. [33] addressed the fuzzy range estimating model that utilises the major cost packages identified by experts. Through this method, the paper would like to overcome the shortcomings of Monte Carlo Simulations. Plebankiewicz, E. et al. [34] performed the analysis for the validity of the model structure assumptions by comparing the selected initially fuzzy approach and probabilistic approach to calculate life cycle cost (LCC). Cocodia, E. [35] illustrated fuzzy analysis for the development of cost estimation reasoning (CER)s risk for use in conceptual cost estimates for futures Floating Production, Storage and Off (PRSO) developments.

Moreover, fuzzy engineering economic analysis is researched by many authors. Kahraman, C. et al. [36] developed engineering economy techniques under fuzziness which are the new extensions of the fuzzy set such as intuitionistic, hesitant and type-2 fuzzy sets to be applied to environmental problems. Kaino, T et al. [37] described fuzzy sensitivity analysis and its application. Çoban, V., & Onar, S.Ç. [38] applied the Pythagorean fuzzy sets for dealing with uncertainty and imprecision inherent in production levels and energy price for life-cycle cost (LCC) and levelled cost of energy (LCOE) methods. Dimitrovski, A.D. & Matos, M.A. [39] applied the fuzzy sets approach in utility economic analysis. In this paper, they considered the dependence that may exist between the fuzzy variables and the influence of this dependence on the results. They also considered mathematical operations over independent fuzzy numbers.

In this paper, a new systematic strategy with the FIS of HF method is developed with fundamental fuzzy rules based on the engineering index of cavability. Furthermore, we propose a method that considers contingency costs to reduce risk and uncertainty for long term HF cost estimation.

3. Fuzzy Inference System Development

3.1. Fuzzy Set

Fuzzy set theory is designed for representing the formalised tools to deal with, hardly representing the probabilistic model. Fuzzy set theories could provide better rational decision-making tools with specified membership functions than probabilistic methods. However, no answer is always correct when rules or facts are based on experience and past data which are often ambiguous. The fuzzy set theory also can be used in uncertain economic decisions to deal with vagueness. The methodology of the paper, for dealing with the mathematical expression of cavability index (CI), which is a classified rating system, utilises fuzzy set theory and gives the rating system the quantitative number through a membership function of fuzzy numbers. The fuzzy modelling method does not exclude statistical methods but becomes a tool when other approaches for HF cost optimisation are not pertinent.

3.1.1. Fuzzy Arithmetic Operation

In general, a financial calculation is difficult to convert into a probability model due to uncertainty. However, the fuzzy financial calculation can address this uncertainty because it can show the decision-maker what can happen. For this calculation, arithmetic calculations of fuzzy numbers can be considered, synthesised in the equation for generic operation, and described by the extension principles [40]. It is noteworthy that if the computation of fuzzy numbers is the same number of arithmetic calculations, the calculation can be solved when both variables are independent, such as the normal number of arithmetic calculations. Still, when both variables are dependent, the arithmetic calculations cannot be applied, and the extension principle should be applied. In other words, an extension principle is a method of extending an ordinary number of operations to fuzzy concepts.

When applying the extension principle, it is necessary to consider dependency and independency in probabilistic calculations. In most cases, however, these dependent features tend to be neglected without proper consideration. For example, although the tendency of rock characteristics in mines is heterogeneous, it does not mean all variables of cavability are independent of each other characteristic of the rock mass. This could reveal the correlation between the two variables expressed as fuzzy numbers, as it implies that the variables will behave in the same way. In other words, if one of the variables takes a certain value from its support set, the other variables could share precedent values in proportion to the support set. Thus, the more information with common characteristics, the more uncertainty can be remained by considering dependence. In this situation, the dependence between variables of cavability also works in cost estimation because some uncertain values directly or indirectly affect the elements of HF cost estimation through the motivated use of common uncertain parameters. Therefore, this paper excludes the tendency to exacerbate the uncertainty of long-term operation. That can occur from the induced cavability factor, expressed by the fuzzy set in block caving from a long-term perspective. It has modelled three scenarios for consideration of the dependence.

3.1.2. Fuzzy Inferences System

Fuzzy Inferences System (FIS), based on fuzzy propositions and fuzzy sets, is commonly referred to fuzzy reasoning as the computing framework. FIS is constituted of a fuzzifier, rule-based structure, and defuzzifier. For using FIS between cavability index and HF cost estimation, it is fuzzified through the Mamdani fuzzy algorithm in this paper. Through a fuzzified algorithm, a rule-based structure drives reasonable conclusions from soft computing between obscure information and other information when the defuzzification processes ended.

3.2. Cost Optimisation in Mining

Cost optimisation is a continuous process aiming to minimise operating costs whilst improving business values without significantly impacting other associated factors. Cost optimisation has become a vital part of the success of mining projects. However, there are several parameters associated with the uncertainty which can affect the overall cost optimisation process. Therefore, the selection of parameters is key to the success of the cost optimisation process.

Before considering the Hydraulic Fracturing (HF) costs, it is required to review and analyse the newest creative techniques of HF in block cave mining. Firstly, the problem of using either a cased borehole or an open borehole is questionable. Usually, in the oil and gas industry, the cased borehole is applied to the HF operations to retrieve the fluids and initiate the HF process by making perforations link up a single axial HF. After the perforation process, the HF may initiate from its preferred propagation plane outside the cased borehole. However, in cave mining, for the HF initiation process, directional HF could be introduced for inducing lower breakdown pressure and promoting HF transverse initiation [4,7]. Unlike the cased borehole case, the initiation of the HF process in the open borehole is replaced through the cutting machine, which creates an initial notch around an open borehole as an artificial weakness for the hydraulic fractures to initiate [41].

Next, using proppant material for HF in cave mining is questionable. According to recent research, HF for preconditioning in cave mining is suggested for using proppants to enhance the induced stress shadow effect. Usually, in the oil and gas industry, proppant is injected into HF to induce greater length and width of the fracture. The formed fractures are prevented from being closed due to in situ stress and are kept semi-permanent by injecting the proppant. In the geotechnical view, fractures caused by HF can be re-orientation in the preferred direction due to the effects of stress shadow. Because HF causes stress in the rock due to the liquid injected into the rock, the stress shadow effect induces deformation of the rock and forms the stress reversal region. In other words, since the injection rate exceeds the flow capacity of the rock formation, the stress created here is called the stress shadow effect, which deforms the existing state of stress due to the superimposition of in situ stress. The stress shadow effect depends on whether a stress reversal region occurs around an existing fracture or between existing fractures and the extent of this stress reversal region [41]. Because the preconditioning of HF used in block caving is to make the rock mass more blocky, it is preferred that the formed fractures become networks or connect with existing cracks. Therefore, it becomes more important to form a joint network by maintaining the crack and how cracks occur easily. However, HF, usually used in conventional block-caving, does not use proppant (mainly using sand), but it is suggested to induce stress shadow effects to each crack by keeping the gap between the cracks by injecting proppant into the cracks, which are caused by HF.

Lastly, the cost estimation of HF to be applied in block caving mines is relatively unprecedented compared to that of the oil and gas industry. Thus, this study refers to a report by the U.S Energy Information Administration (EIA) that performed an analysis of upstream drilling and production costs conducted by IHS Global Inc. [42]. However, the cost of HF used in the oil industry cannot be directly applied to block cave mining as the above-mentioned paper discussed. This is because of the differences in HF techniques between the oil and gas industry and the block cave mining, which were summarised in table 1 in Q. He et al. [41].

According to Bunger et al. [43] and Adams and Rowe [44], the most significant difference between the gas & oil industry and the mining industry is the fracture size.

Table 1. Differences between hydraulic fracturing in the cave mining industry and hydraulic fracturing in the shale gas industry, revised by Bunger et al. [43] and Adams and Rowe [44].

Application	HF Size	$\mathbf{Injection}\mathbf{Volume}\left({\mathbf{m}}^3\right)$	Injection Rate (L/s)	Addictive	Proppant	Distances between Fractures (m)	${\mathsf{\sigma }}_3$ Orientation
Cave mining industry	About 30 m in radius	8–20	5–10	No	Some	1.25	Mostly vertical in Australia
Shale gas industry	Hundreds of meters in half-length	135–1000	75–250	Yes	Yes	About 100	Mostly horizontal

shows that the amount of injection rates used in the oil industry differs by a factor of 25 from those used in the block cave mining industry and, in terms of injection volumes, they differ by a factor 12.5 to 50 from those used in the block cave mining industry. Above all, the reason that caused the quantitative difference between the two industries in HF is the size of the fracture, and similarly, distances between fractures create the difference in the quantitative factors for HF.

3.3. Cost Variables of Hydraulic Fracturing Costs

Many factors may also contribute to the fluctuation of HF costs. For example, HF cost is influenced by drilling cost (rig rates and drilling), fluid cost, proppant cost, pump cost, and equipment cost heavily impacted by more significant market conditions and the time required for drilling the total well depth. In addition, HF costs depend on rock mass conditions and the cost of minerals. Furthermore, HF costs are influenced by geological properties for geo-steering, which is required to optimise the preconditioning efficiency by orienting the wellbore perpendicular to the minimum in situ stress orientation and inducing the interaction between hydraulic fractures and natural fractures for the growth of hydraulic fractures. Therefore, HF costs might need a comprehensive function of budgeting estimation.

Based on the listed reasons above, the elements of the HF cost estimation used for block caving should include the following: the HF uses an open borehole rather than a cased borehole, and by using proppant to form stress reversal regions and to utilise the effect of stress shadow effect, the use of proppant to expand the width of the fracture and to proceed in the preferred direction. In addition, the study rectifies the EIA’s HF cost estimation for the difference in HF size and HF distances between fractures. Furthermore, HF cost factors are discussed that may contribute to formulating an estimation. As a result, the values are rectified and supplemented for all the illustrated cost aspects described in

Table 2. P10, P50, and P90 of one borehole cost estimation with 500 m length for HF in block cave mining.

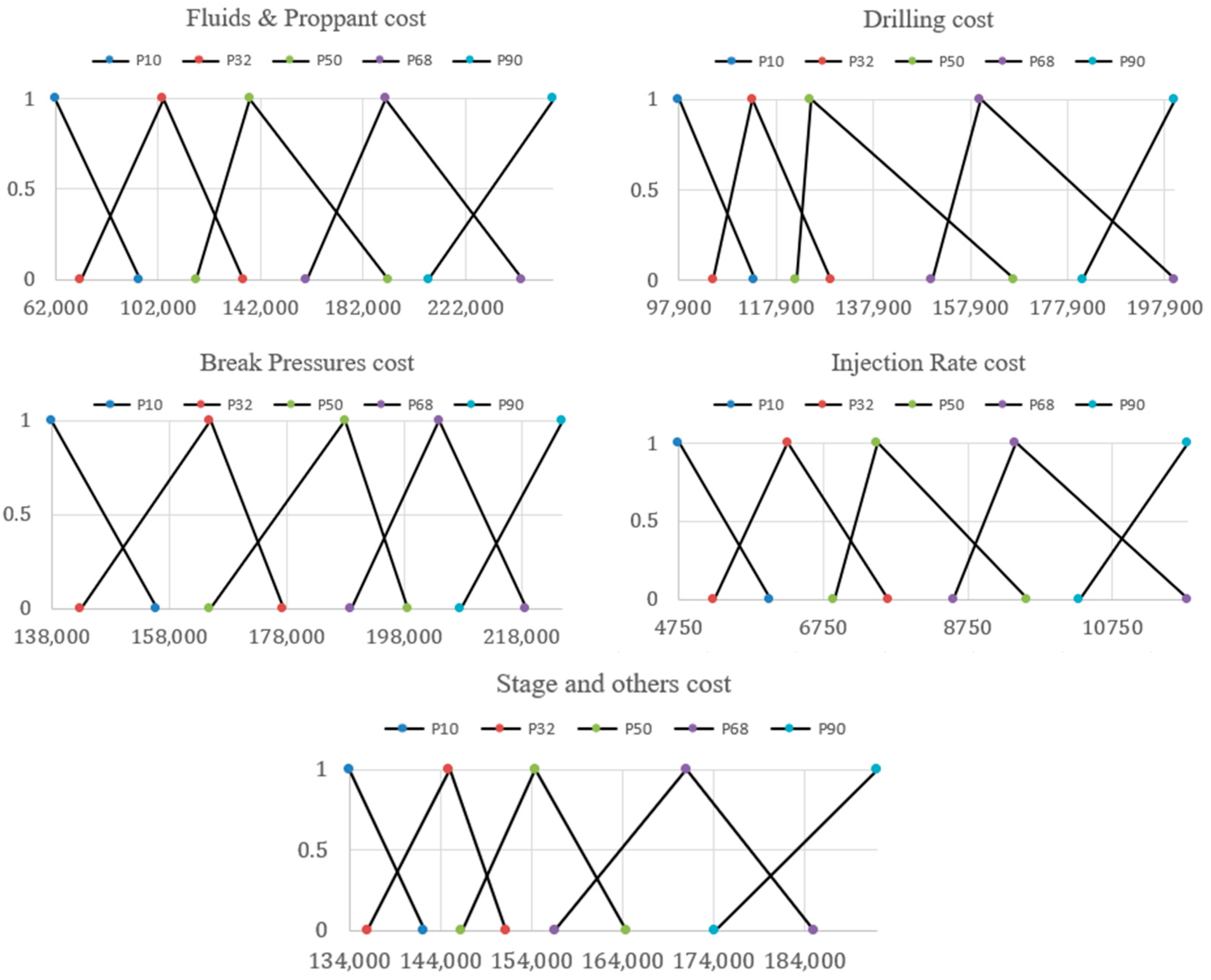
HF Cost Component	Items	P10	P50	P90
Frack cost	Fluid cost	$31,250	$68,750	$125,000
Proppant cost	Water and sand cost	$31,250	$68,750	$131,250
Drilling cost		$97,500	$125,000	$200,000
Frack pumping cost	Stage cost	$15,000	$16,250	$16,875
	Break pressures cost	$137,500	$187,500	$225,000
	Injection rate cost	$4750	$7500	$11,750
Others		$118,750	$137,500	$175,000

. In Table 2, the P10, P50, and P90 stand for percentiles such as P denotes the percentiles in the distribution, P10 denotes the lower 10% of the observations and P50 denotes a value close to the mean value.

3.3.1. Drilling Related Costs

Drilling related costs make up roughly 20% to 25% of the total cost of HF in block caving. This cost is associated with borehole depth, drilling rates, drilling penetration, and drilling efficiency. Drilling costs had many parameters which are likely linked to quantifiable costs, such as drilling crews’ wages and drilling equipment. It also includes intangible costs such as the drill bits consumption, equipment rental fees, drilling costs, and other service costs.

3.3.2. Frack Pumping Costs

The frack pumping cost is a highly volatile variable. It consists of 20% to 25% of total HF costs. Between the frack pumping variables, only the injection rate is selected for considering the difference of application between block caving and the oil-gas industry. Furthermore, the break pressure, which must be higher than the intact rock strength, would also consist in fracking pumping costs. Moreover, in a block caving HF operation, there is no need to take an option for several stages. Thus, this study just assumed the operation would be set as a single stage.

3.3.3. Fluid and Proppant Costs

The fluid and proppant costs make up 15% to 30% of the total HF borehole cost. As discussed above, the purpose of the fluid and proppant in HF is to initiate and propagate fractures. Several substances could make up the proppant, such as artificial proppant and coated proppant, etc. However, this study only considers the fluid and proppant as water and sand, respectively. Even though water has a low viscosity and can be injected at a high or low rate, it could initiate micro-fractures and propagate in the target area. It is also available in abundance and cheaper than any other fluid used for HF.

3.4. Cavability

Cavability is the ability to unravel the in situ rock mass when developing the undercut process and consider all three stages of caving: initiation, propagation, and continuous caving, which is the vital feature of the caving process [45]. In addition, the cavability of rock mass will have an essential role in controlling the mine design at the initial stage of economic issues in a given geological environment. On the other hand, the preconditioning task has the ultimate goal of weakening the rock mass or developing discontinuities. Preconditioning has been used for enhancing the cave initiation and propagation to achieve the desired draw rates. Because of the effects that can be achieved by preconditioning are the same as the purpose of recognising the rock mass’s cavability in cost-effective utility, the study assumed preconditioning and cavability share direct intermediary characteristics. In other words, if cavability ultimately represents the degree of capability for caving in situ rock mass in the orebody, then preconditioning is intended to proceed with this process for improving the ability into the formed rock to be caved. Since the natural factors of cavability can directly or indirectly affect boreholes required for preconditioning, this value is presented through fuzzy logic at a short-term cost estimation. The induced factors of cavability propose a strategy to estimate costs for long-term planning of preconditioning. For instance, if obtained from the rock with weak geology of the orebody, preconditioning can maximise its effectiveness by reducing the strength of the pressure that can be seen as an effect, and later transferred to a part made of strong rock that would be estimated through cost estimation fuzzy logic in the long-term plan.

3.4.1. Cavability Index

The Cavability index (CI) is referred to the degree of cavability in the caving mining method. CI is an essential geomechanics factor for conducting caving by forming an ellipsoid shape in the draw column using the gravitational separation process. In addition to this importance, CI helps address uncertainty in the design and planning process phases of the caving operation because the engineer’s decision is incomplete with information and data on the rock mass. Therefore, CI is often obtained through structural analysis, numerical modelling, and an empirical chart estimated using rock mass rating (RMR) and IRMR [46,47]. In addition, CI obtained from these empirical formulas ultimately helps infer the dimension of the undercut which is the most important component of block caving design, and to set up the hydraulic radius (HR). HR is necessary to ensure the direction of caving propagation for the unsupported area of the back of the cave. That is, the determination of HR is used by CI to decide the required dimension for caving and sustain mining operations.

CI of the orebody is influenced by the rock mass of its natural properties. It is also influenced by induced factors resulting from the mining process [48,49]. These properties have been demonstrated by a review of RMR classification [45].

Natural Factor

The natural factors in cavability generally use the physical properties of rock mass classification. Such a natural factor includes uniaxial compressive strength (UCS), in situ stress, water, and rock mass discontinuity properties. UCS shows the characteristics of rock material strength. In addition, in situ stress serves as an important factor in the natural factor of cavability, as it informs the magnitude and orientation of regional stress in the caving mine [48]. Water reduces the effective stress of discontinuous joints in the rock mass, weakening their shear strength. Water can increase the capability of cavability by reducing the friction between the joints. Lastly, the natural factors also include discontinuity properties. This factor also distinguishes six ways of spacing, orientation, aperture, persistence, roughness, and filling of joints as used in representative rock mass classifications (RMR, IRMR, Q systems).

Induced Factor

The induced factor represents the structural characteristics required by the caving process. These induced factors are made up by engineering decision-makers and are regarded as a way for mining the orebody. Induced factors are included in an undercut, HR, block height, and fragmentation. For identifying the uncertainty of mine, cavability is specified by each of the criteria.

First, undercut is favoured to choose the advanced undercut method, which is the development of undercut ahead of the partially developing extraction level. This method helps ramp up the production material and destress the extraction level for reducing the abutment stress, which could damage the extraction level. HR is the area divided by the perimeter. The effects of HR are included as the induced factor of cavability, as they play a significant role in the design of caved material channels and in determining the overall development. Block height is one of the critical parts of induced factors in cavability. After cave initiation, cave material falls off from the caprock as an ellipsoid shape. The vertical heights of the ellipsoid as the height of draw (HOD) influence secondary fragmentation. Since as long as HOD applies, secondary fragmentation is a much finer fragmentation during the separate gravitational work and facilitates the transition of primary fragmentation to secondary fragmentation. In this sense, block height is also included in induced factors of cavability. Lastly, fragmentation of ore in block caving influences the overall operation process due to its impact reaching the comminution process, equipment selection, production rates, determination of drawbell plan and size, and continuous production schedule. Since the fragmentation of a deposit could change the entire mine life, diagnosing the result of the preconditioning method makes the right decision more important.

4. Cost Estimation with a Strategic View

4.1. Long- and Short-Term Strategy for Estimation of HF Cost Using Fuzzy Logic

The study not only plans to model the cost estimation of HF using fuzzy functions but also proposes correlated and uncorrelated cost estimation to avoid ignoring the effect of the interdependence of certain variables on the cost estimation. To consider these features, this paper referred to the work of Dimitrovski, A.D., & Matos, M.A [50]. Even though that study had an analysis of recognising and designing dependent differences as cash flows parameter which usually consists of interest rates and time values, this study turns our attention to the engineering parameters’ degree of the dependent and independent relationship since inadequate values of engineering variables can also lead to additional uncertainties in cost estimation. It can occur mainly in the industries on which upstream operations (oil and mining sector) are based because the physical properties of the underground are invisible and the exact estimate of the material being produced is almost impossible. To overcome those uncertainties, the study proposes a model that considers the extent to which variables are caused by their dependence, as well as probabilistic estimation methods.

Before investigating in the light of the strategic approach using fuzzy logic, the study we discuss the basic definition of the strategy. A term of periodic strategy in mining operations ultimately exists for the optimisation of the cost. In other words, this is to make the utilisation of the budget to induce the best efficiency through maximised cash flow with low capital cost and low operating costs. Thus, the project’s duration can be divided into short-term, medium-term, and long-term. The short-term can be defined as day-to-day, but in this paper, the concept of the shift-to-shift is addressed, and the long-term defines each other period within a year’s range if the operation of HF lasts just about three years. However, the medium-term was not discussed because the HF fracturing project had a relatively smaller magnitude of size than other projects.

Furthermore, the short-term strategy is modelled to estimate the HF cost with the natural factors of cavability as input parameters. The output of this model is modelled based on “the fracture cost”, “proppant cost”, “drilling cost”, “fracking pumping cost”, and “others” (such as labour costs and other related operating costs). The short-term strategy will propose that those assigned orientations determine the timing of expansion (adjusting the rate of undercut process and initial production), information on the scale of infrastructure, and direction of preconditioning operation. Although the short-term strategy proposed in this paper may not address the complexity of all phenomena occurring in mining operations, it is assumed that the criterion of the cavability parameter would provide an optimal approach with sufficient logical information due to the cost per borehole of HF preconditioning. In addition, it will induce not only to be practical but also provide the opportunity to create periodic updates and action plans for real mine sites through achievable scenario analysis. It will also help decide the equipment selection and borehole spacing of the HF and drilling diameter of the borehole.

The long-term strategy of mining planning would generally propose to solve the problem of large-scale optimisation, which is aimed at finding block extraction sequences. Since the sequence problem is directly the order to derive the maximum NPV, it performs the optimal strategy, incorporating physical and economic constraints and future changes in mineral prices. However, this paper will set the induced factors of cavability in optimising HF cost estimation as constraints of the long-term strategy. In this case, it suggests three possible scenarios for HF cost. Thus, according to those assumptions, the analysis was made to changes in three scenarios, as the measurement of HF costs from a long-term perspective can vary. Those modelled scenarios are integral to the optimisation of HF operation; these inform the development of long-term planning parameters and contain the consideration of contingency planning for practical operations. To sum up, all these processes through HF preconditioning aim to maximise rock mass’s cavability assessment, the efficiency of capital costs, and the scheduling problem. The outcome of maximised cost estimation would help decide on the scale of infrastructure and ore production by facilitating undercut propagation and controlling block heights of block caving operation with inducing additional fragmentation.

4.2. Short-Term Strategy for HF Operation

In this study, the Mamdani FIS was applied to construct short-term HF costs. The essential component of this model is made up of the input-output sets and if-then rules. The fuzzy set of input-output sets is developed by triangular and trapezoidal membership functions, which can minimise their uncertainty. After setting the input-output sets, all the consequent fuzzy sets will be integrated into a new fuzzy set by using the maximum operator as the if-then rules construction process. Once the construction of if-then rules is finalised, it requires a procedure of defuzzification that converts the form of output-fuzzy sets into a crisp numerical value. The defuzzification method is processed by the method of COA defuzzification, and it would perform to get the crispy value of each HF cost variable.

For the short-term strategy, it is necessary to restrict the range of the scale to one borehole operation. This model is assigned to the 9 input variables (UCS, in situ stress, joint spacing, joint orientation, water, joint aperture, joint persistence, joint roughness, joint filling), and five output variables (stage and others costs, fluid and proppant costs, Drilling costs, break pressures, injection rates). Those fuzzified sets are illustrated in Figure 1. Before specifically describing this model, there is a questionable relationship between input variables (cavability) and “stage and other costs”, which is the subset of HF cost estimation. Due to the property of cavability, it seems not to relate to the stage cost and other costs associated to the operation’s maintenance and labour cost. However, the lower the CI, the longer the time to shift the operation of HF, so the other and stage costs were set to output variables together because the time, cost, and labour cost would also increase due to the chain of actions.

Specifically, input and output variables are illustrated with trapezoidal and triangular membership functions in Figure 1 and Figure 2. The membership function of input variables is classified into five classes. The fuzzy input sets were designed to intersect each class based on the membership degree of 0.5, respectively. The output variable is also classified into five classes. However, it was determined that the three classes (P10, P50, P90) that were originally calculated alone would not be more detailed to calculate the value but would bring up the result of the broad and wide value. Thus, this research goes a little further and classifies it into five classes by adding more P32 and P68 cases. This helps to flexibly cope with the estimation of output variables by applying one standard deviation. The fuzzy output sets, categorised into five classes, are designed to intersect each class based on the membership degree of 0.4 (Figure 2).

The next stage of fuzzy modelling is the construction of the if-then rules. As discussed before, the CI is classified into the five parameters. Thus, the number of if-then rules will have the 5 powers of 9 rules. However, among them, the if-then rule is applied as itself, the number of rules would be too large. Thus, the generality of the rule is given in consideration of the weighted value of the CI of Rafiee [44]. Moreover, the natural characteristic of rock will ignore some impossible rules. When constructing the if-then rules, the logical rules set that the higher the CI, the lower the HF cost, contrary to the lower the CI, the higher the HF cost. As the last process of this methodology, the COA method is employed for the defuzzification process due to the calculation simplicity. The fuzzy set output was translated into a crisp numerical value through the COA defuzzification method, which would lead to the final HF cost estimation. Following the determination of the cost estimation, its membership degree is obtained by using the fuzzy sets, which represent the output variables.

4.3. Long-Term Strategy for HF Estimation

In this section, the study tries to prioritise the uncertainties encountered when executing the HF long-term planning in the block caving mine. First of all, mines in block caving are exposed to environments where the characteristics of rocks can be distributed variously. Therefore, long-term strategies for HF should be considered heterogeneous characteristics of rock for mining planning and constructing essential infrastructure rather than a homogenous characteristic of rock. Thus, the study makes a choice to measure the cavability of the induced factors for dealing with the heterogeneous features of the block caving sector as constraints of the long-term strategy. Then, according to the nature of rock characteristics, three scenarios in the block caving sector have been suggested. Those cases are illustrated in

Table 3. Three scenarios are illustrated for long-term strategies for hydraulic fracturing costs.

Rating	0	1	2	3	4
Scenario A
Hydraulic radius		18.5 m
Fragmentation		512
Block height		125 m
Undercut direction			Fair
Scenario B
Hydraulic radius				50 m
Fragmentation					0.2
Block height			180 m
Undercut direction			Fair
Scenario C
Hydraulic radius		25
Fragmentation			10
Block height				225 m
Undercut direction			Fair

.

Secondly, those scenarios are modelled with FIS in the same way as the short-term strategy. Fuzzy modelling for long-term strategy is constructed based on four induced factors of cavability to extract defuzified long-term HF cost estimation with the range of 1 year. For further explanation, induced factors are made of HR, fragmentation size, block height, and undercut direction as the input variables in fuzzy sets. Finally, those defuzzified results are compared and analysed by presenting the correlated and uncorrelated cases of the differentiated long-term budget, according to the composition of scenarios A, B, and C. For comparison of analysis, the rectified extension principle has been used rather than the applied arithmetical operation mentioned above.

Those modelled scenarios are integral to the optimisation of HF operation, and these are not only informing the development of long-term planning parameters but also containing the consideration of contingency planning for practical operations. Measuring the amount of uncertainty would help make a decision maker construct the scenario’s consecutive sequence in the mining operation. To sum up, all these processes through HF preconditioning aim to maximise rock mass’s cavability assessment, the efficiency of capital costs, and the scheduling problem. The outcome of flexible cost estimation would help decide on the scale of infrastructure, ore production by facilitating undercut propagation, and controlling block heights of block caving operation by inducing an additional fragmentation process.

After assuming that the induced cavability factor is an input variable, it is compounded to each output result for recalculation of fuzzy correlation. Furthermore, this strategy utilises the value of contingency cost, which is referred to as association for the advancement of cost engineering (AACE)’s contingency table. Through this way, the long-term strategy for HF operations could conclude to validate “cost estimation” through the fuzzy economic analysis.

The scenarios assumed in Table 3 imply that each of them has a different rock property in one massive ore body. However, this scenario also implies uncertainty in scheduling decisions or budgeting about which part of the rock mass to approach first. Thus, to design the long-term strategy, we followed the way we used in the short-term strategy method with fuzzy input and output variables. After the following results are obtained from the FIS, each of which is defuzzified by the elements of the COA shown in

Table 4. After defuzzified results of long-term cost estimation of hydraulic fracturing.

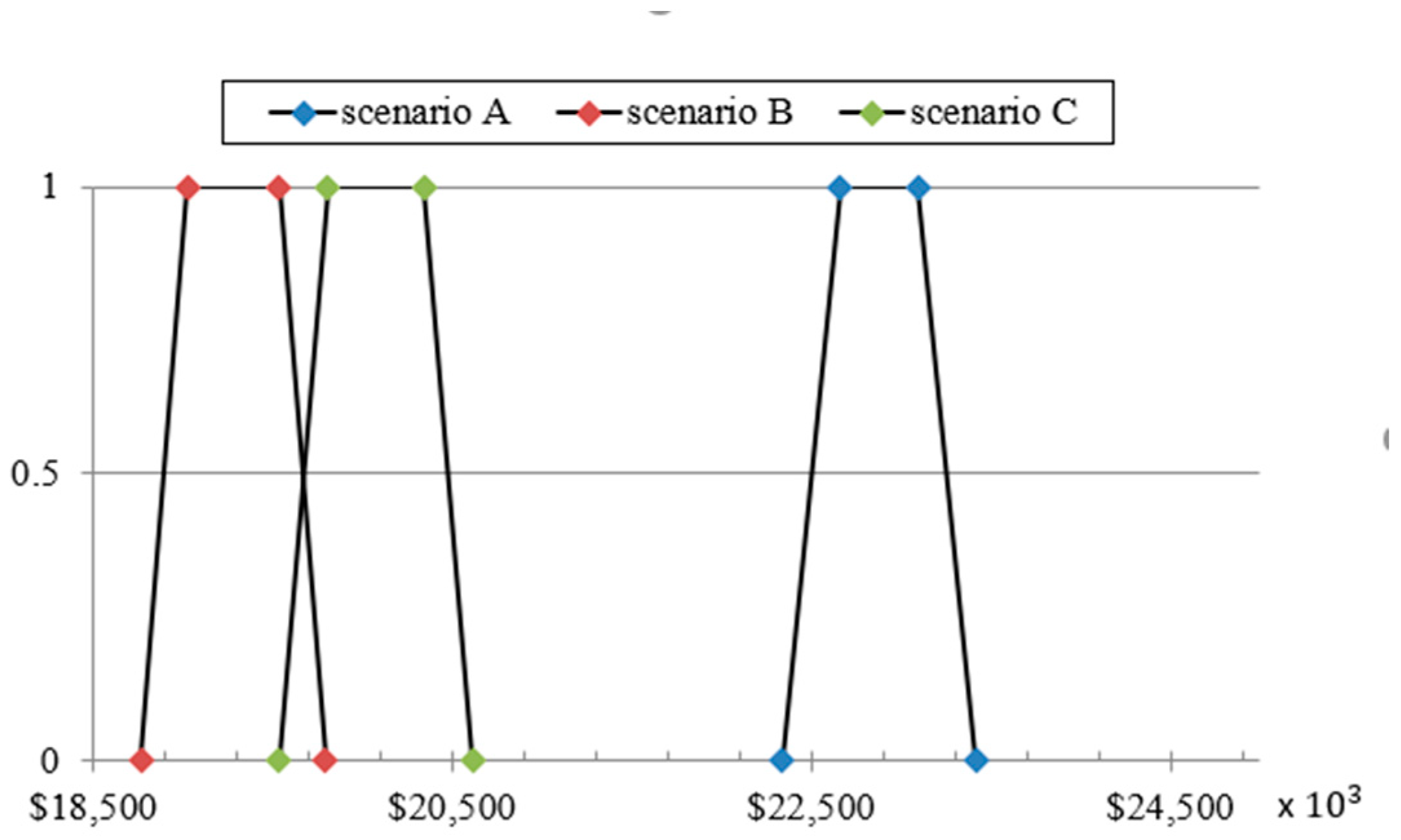
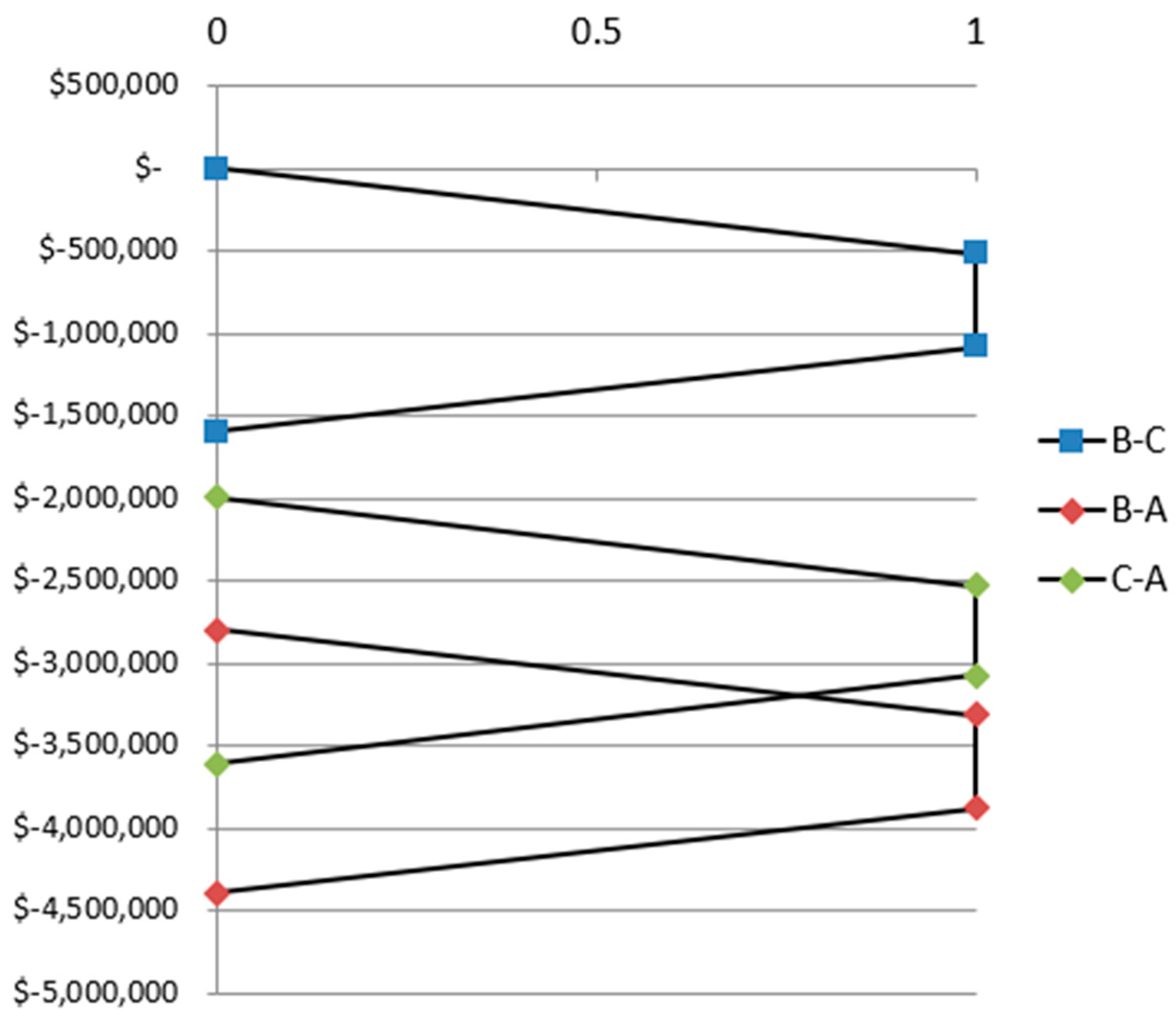
Scenarios/Cost	Others and Stage Cost	Fluid and Proppants Costs	Drilling Costs Costs	Break Pressures Costs	Injection Rate Costs
scenario A	$4,950,000	$5,780,000	$4,770,000	$5,800,000	$280,000
scenario B	$4,380,000	$3,900,000	$3,810,000	$4,840,000	$208,000
scenario C	$4,460,000	$4,290,000	$3,870,000	$5,160,000	$225,000

. The basic logical configuration is the same the lower the score of cavability, the higher the cost of HF.

The defuzified values were available according to the assigned fuzzy rules shown in Table 4. However, although the values have tentatively been obtained through long-term cost estimation for each scenario through an intuitive and uncertainty-tackling FIS by considering engineering parameters, it is still too vague to apply the estimated cost. Here, the study is going to focus a little more on the nature of estimation. Universally, cost estimation should not represent individual numbers but the range of risks and possible costs. Although the study used the data based on the cost estimation for HF in the oil industry through the EIA HF report, there are limitations to applying the environmental constraints of the underground mining industry and unstable operation, so cost estimation must be extended into those uncertainties.

If the values are concluded only with the individual values shown above in Table 4, one will not be able to respond appropriately within the range sanctioned in the project when risk events occur because it is easy to become a time range of cost estimation. Therefore, if possible, it appropriate cost estimation is considered to derive the optimal condition cost through risk analysis before the funding decision.

Before taking the concept of contingency cost, too much or too little range of cost should be considered as it may cause problems. Since the initial starting point of a project may tend to schedule HF projects quickly and safely, it derives from overestimating the budget calculated for the project. In this case, the overrun cost estimation will lock up the capital, which may adversely affect the budget for the next schedule. On the other hand, the more detailed and strategic the project is, the more pressure it may create for lower cost estimation, which increases the likelihood that the outcome of the project will be disruptive. In general, to quantify the risk, detailed distribution of the contingency could be decisive, but it is beyond the scope of this paper to discuss how to quantify the risk.

There are two representative methods for quantifying the risk, the parametric estimating method and the expected value, which are introduced in RP 42R-08 and RP 44R-08 on AACE’s website, respectively. However, parametric estimating is chosen for this study because a method is used to quantify systemic risk because it helps analyse the impact of risk through empirical data. Before using this quantification method, it is necessary to classify the project definition of HF application in block cave operation by referring to AACE’s class estimate. For this reason, it is summarised with estimate criteria in

Table 5. AACE estimate Classes, the Maturity level of project definition deliverables and the Expected accuracy range [51].

Estimate Class	Primary Characteristic	Secondary Characteristic
	Maturity level of project definition deliverables	Expected Accuracy range
Class5	Key deliverables and target status: block flow diagram by key stakeholders	P10: −20% to −50% P90: +30% to +100%
Class 4	Key deliverables and target status: process flow diagrams (PFDS) issued for design.	P10: −15% to −30% P90: +20% to +50%
Class 3	Key deliverables and target status: piping and instrumentation diagrams (P& IDs) issued for design.	P10: −10% to −20% P90: 10% to +30%
Class 2	Key deliverables and target status: All specifications and datasheet complete including for instrumentation.	P10: −5% to −15% P90: +5% to +20%
Class 1	Key deliverables and target status: All deliverables in the maturity matrix complete.	P10: −3% to −10% P90: +3% to +15%

for deciding the scope of the project range, which describes the AACE’s estimate class of deliverable status and target status by referring to RP 18R-97 [51].

Through Table 5, the application of HF in the block cave mine was assumed to be the initial control estimate against which all costs were assigned. Therefore, as this criterion, the study considers that the HF operation could belong to Class 3.

However, before taking the parametric estimation in this paper, the systemic contingency allowance table, proposed by Hollmann, J.K. [52], is applied to the fuzzy model for CPI research (which is publicly available on AACE’s website) since the in-depth analysis of parametric estimating in this study is beyond the scope of this paper. This table is introduced in

Table 6. Typical systemic contingency allowances based on project attributes by Hollmann, J.K. [52].

Systemic Contingency as a Percentage of the Unexpended Base Estimate
Scope Definition		Class 3			Class 4			Class 5
Complexity		Low	Medium	High	Low	Medium	High	Low	Medium	High
Tech.	Low	3%	8%	12%	10%	15%	20%	19%	24%	29%
	Medium	6%	11%	15%	13%	18%	23%	22%	27%	32%
	High	15%	20%	25%	22%	27%	32%	32%	47%	42%

. As followed by research, the contingency percentage was determined by the technology rating with the complexity of the project for each class in the systemic contingency allowance table.

Accordingly, the study considered the cavability to represent the complex operation and assumed the distribution of contingency cost. For example, the higher the cavability, the more likely it is that accidental risk events in the underground mine could be created, so a high percentage of contingency cost was allocated. Conversely, if the cavability is lowered, the HF cost will be higher, but the accidental events in the underground mine will be less. As those assumptions followed, it is assumed that the complexity of the contingency rate is proportional to the degree of cavability, and the HF technology criterion is tentatively set at the medium level.

Systemic contingency cost would be set up with the crispy costs, which would take a task of fuzzified parameters as HF cost contingency. According to those criteria, the contingency cost of scenarios a, b and c will be assigned to the systemic contingency percentage of 6%, 12.5%, and 11.5%, respectively. After this contingency cost is allocated to each scenario, the crispy values are assumed as the median values of the corresponding fuzzy number for the fuzzification modelling. Then, it is redistributed to the trapezoidal fuzzy model through each value setting with 0-cut intervals and 1-cut intervals of the fuzzy numbers.

As follows from

Table 7. Contingency rate considered with fuzzy sets.

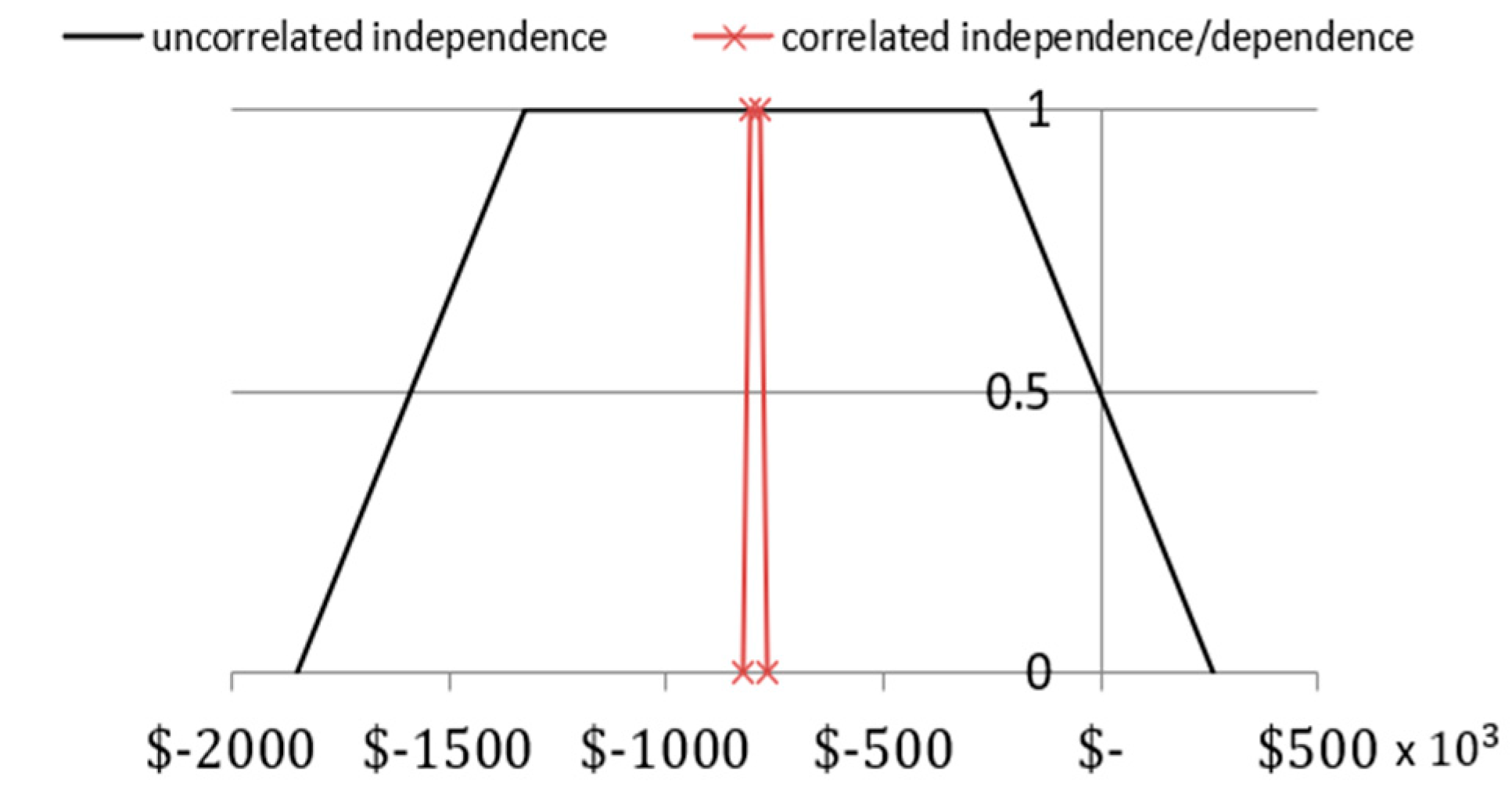
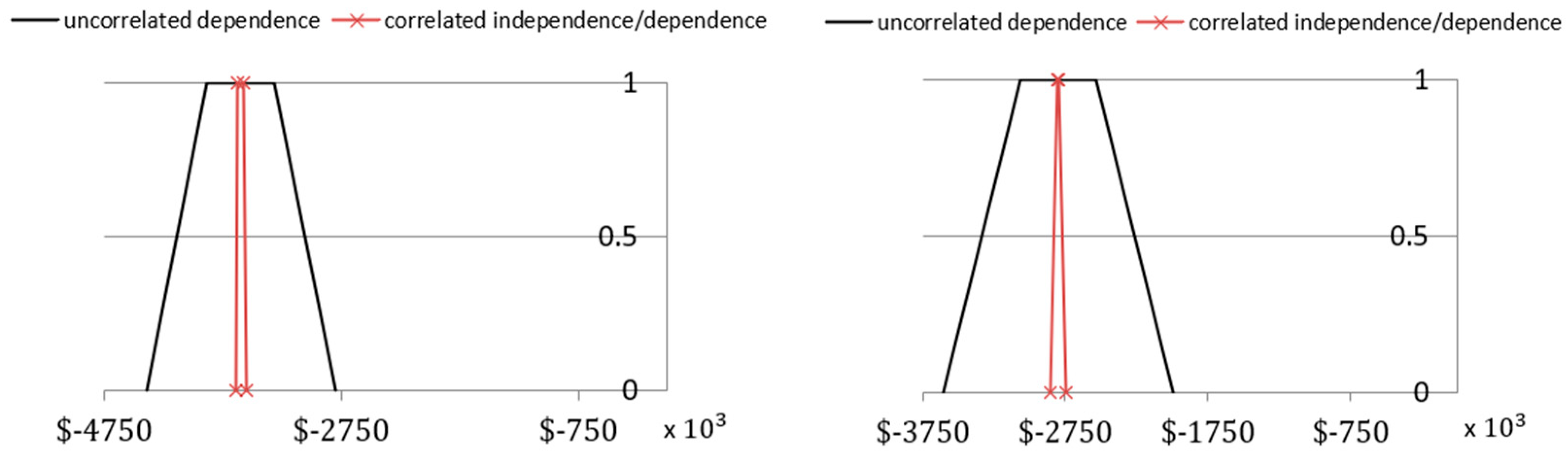
Contingency Rate/α Cut Intervals	α = 0	α = 1	α = 1	α = 0
Scenario A	3.5%	5%	7%	8.5%
Scenario B	9.5%	11%	14%	15.5%
Scenario C	8.5%	10%	13%	14.5%

, the contingency costs are associated with each scenario, with the contingency cost rate, which contains the uncertainty in this model. Finally, the corresponding trapezoidal fuzzy intervals are multiplied with Table 4 (defuzzified model) and the result of this calculation is shown in

Table 8. Fuzzified cost estimation included in the range of contingency costs.

Scenarios/Cost Estimation as per Contingency Rate	α = 0	α = 1	α = 1	α = 0
A	3.5%	5%	7%	8.5%
	$22,335,300	$22,659,000	$23,090,600	$23,414,300
B	9.5%	11%	14%	15.5%
	$18,766,110	$19,023,180	$19,537,320	$19,794,390
C	8.5%	10%	13%	14.5%
	$19,535,425	$19,805,500	$20,345,650	$20,615,725

. Thus, Figure 3 could illustrate the considered form of both trapezoidal shapes of graphs. It can be regarded as a final representation of the long-term cost estimation of HF for each scenario, including the contingency rate.

For comparing the cost estimation of HF from the strategic perspective, however, the uncertainty of the fuzzy equation should consider the relationship between the engineering parameter and the cost contingency rate. Firstly, as illustrated in Figure 3, it is evident that scenario A is the last option to consider if ordered in terms of cost. However, scenarios B and C cannot be confident about which one is clearly the better option. Because scenarios B and c overlap each other in the fuzzy trapezoidal function, the right extreme of scenario B and the left extreme of scenario C are overlapping. Thus, it is impossible to find a definite answer as to which scenario should be worked on first. To address this, the calculation is done through the arithmetic operation of the fuzzy number introduced earlier in Section 4. The difference between scenarios B and C in values through the calculation method using Zadeh’s extension principle is presented in Figure 4. However, in the case of calculation, the value of the assumption that the variables of cost estimation included in scenarios b and c are independent of each other is implied.

More specifically, the subtraction between independent fuzzy numbers located at both extremes naturally implicates the best estimate, which tends to simplify the various variables used to derive the calculation. However, the simplification of the calculation may induce results that ignore both recognition of cavability variables commonly used for cost estimation and contingency rate newly introduced for long-term strategy. In other words, if the dependence between variables is not considered, the result of fuzzy arithmetic operation causes a result of exaggerated uncertainty. As a result, the fuzzy arithmetic operation by the extension principle is applied. In the light of the characteristic of fuzzy arithmetic operation, the use of fuzzy logic models allows the computation of variable dependencies. For optimisation of decision making through these considerations, calculations that consider dependencies for each scenario are presented in Figure 5 and Figure 6.

As presented in Figure 4, according to this perspective, it noted that the difference between scenarios b and c has a positive value at the right extreme when independent of each other. In this case, it implies that there is a possibility that scenario C will be better. However, if the two variables are dependent on each other, the right extreme value of the difference between the two scenarios appears as approximate 0. It implies that the value of scenario B has a lower cost than choosing scenario C in any case.

In this way, the recognised differences of the dependence for each case are illustrated in the left figure of Figure 6 for scenarios B and A and the right figure for scenarios C and A.

Figure 7 is a summary graph so that the graphs shown in Figure 5 and Figure 6 can be compared collectively. Through the comparison between newly formed fuzzy graphs, it is possible to find out at what probability the cost can be made in determining the priority of each scenario.

5. Conclusions

The cost estimation of hydraulic fracturing in block caving is presented with short-term and long-term strategies by using fuzzy modelling. A rigorous literature review was performed in the oil and gas industry and in block caving for base cost estimation by using the U.S. Energy Information Administration (EIA) data. The result of hydraulic fracturing base cost estimation is presented in this paper. This modelling used the natural and induced factors of the cavability index to parameterise engineering variables for cost estimation purposes.

The short-term strategy is modelled with 9 natural factors of cavability as the input variable and 5 cost variables as the output variable in the fuzzy inference system. The long-term strategy is modelled with 4 induced factors of cavability as the input variable and 5 cost variables as the output variables in the fuzzy inference system (FIS). In the long-term strategy, the result of the defuzzifed values is reconstructed through the fuzzifying process for considering the inherent uncertainty of cost estimation. After this, the paper presents the correlated case and uncorrelated case of the differentiated long-term budget, it identifies to prioritise the project schedule by recognising the dependence on each scenario’s common characteristic of the cavability parameter and cost contingency rate which is suggested by the association for the advancement cost engineering (AACE). The outcome of flexible cost estimation would help to decide on the scale of infrastructure, ore production by facilitating undercut propagation, and controlling block height of block caving operation including an additional fragmentation process.

Through this study, it is noteworthy that it is possible to present the inferential process for cost estimation of a project without comparative historical data in the project’s industry area. Thus, it would be another method for an initial cost estimate.