Applying Grey Relational Analysis in the Evaluation of the Balance of Children with Intellectual Disability

Abstract

In addition to intellectual performance, children with intellectual disability also seem to have lower performance than children without intellectual disability in terms of balance. Therefore, they often experience walking instability or fall due to imbalance, causing injuries. With regard to balance training courses provided by medical or special education personnel for children with intellectual disability, although there are subjective observation scales that describe their balance in a qualitative way, there are still few direct measurement methods that can provide personnel with the ability to evaluate the training results of an intervention program. The purpose of this study was to provide a method for evaluating the balance of children with intellectual disability to facilitate a general inspection or evaluation of balance before and after the implementation of various intervention programs that help movement development. In recent years, the force platform system has been widely used in the research of the elderly balance, yet the research on balance assessment tools applied to children is rare. This study used the objective, fast, and accurate characteristics of the force platform system to analyze the key points of the sit-to-stand movement and the movement balance parameters of children with intellectual disability and children without intellectual disability. Using the grey relational analysis (GRA) method, the time factors and weight factors from the average performance of children without intellectual disabilities was used as the analysis data. After analyzing the relevance between each participant and the target, a norm for evaluating the balance of children with intellectual disability was established. Hence, this valuable result can provide researchers, special education teachers, and related professionals with an effective and time-saving evaluation of the balance of children with intellectual disability.

1. Introduction

Balance is an important basis for human movement development [1]. From the birth of a baby, balance is manifested in learning to lie, sit, crawl, walk, run, etc. Most basic movements require good balance. With good balance, one can maintain the stability and smoothness of body movements when performing activities. Conversely, with poor balance, it is easy to experience falls, causing injuries. For this reason, balance plays an important role in the development stage of children’s body control [2]. However, children with intellectual disability typically have significantly lower intelligence levels than average. They can not only experience problems with communication and learning but also have difficulties in cognition, motor coordination, and balance.

In the daily life of people with intellectual disabilities, it is common to see cases of injuries caused by falls. Enkelaar et al. [3] suggested that this can be attributed to poor balance, and the condition of falling is often used as an indicator for incomplete movement development. Moreover, Giagazoglou et al. [4] regarded it as likely that people with intellectual disability would exhibit poor balance and motor impairment. This deficiency may lead to the loss of basic functioning, or it may restrict the autonomy of the individual in daily life. Cox et al. [5] pointed out that people with intellectual disability have a higher chance of falling than people without intellectual disability, and the risk of related injuries due to falls is also extremely high. For example, Srikanth et al. [6] examined osteoporosis in people with intellectual disability, the symptoms and related issues of which greatly increase the risk of fracture injury due to falls. Furthermore, Sherrard et al. [7] also pointed out that the proportion of people with intellectual disability who are hospitalized due to fall injuries is twice that of the population without intellectual disability, and that this increased proportion should not be underestimated. Since balance plays a role in providing greater stability of various movements in daily activities, improving the balance of children with intellectual disability could reduce the incidence of fall accidents [8]. For this reason, methods for strengthening the balance of people with intellectual disability is very important, and many intervention programs and evaluations have emerged to date [9].

If children with intellectual disability are not trained through intervention programs to improve their mobility, especially with respect to the development of motor skills, they can find it particularly difficult [10]. Early training of the sense of balance can help improve balance, as well as the ability to perform various sports skills. It can also prevent danger to children with intellectual disability during physical exercise and prevent injuries or accidents caused by sports activities. This training includes toe-to-heel walking, straight walking, side walking, backward walking, Z-walking, standing on one foot, standing on both feet, tandem standing, and other training [11]. Although the above activities are popular rehabilitation exercises, Giagazoglou et al. [4] noted that, in view of the cognitive and mental characteristics of children with intellectual disability, the planning of intervention programs should be made more interesting to arouse the interest of children. A preliminary study of trampoline exercise intervention showed that the balance and movement performance of children with intellectual disability did indeed make significant progress following the implementation of the program. This research also provided options for new intervention programs to improve the balance of children with intellectual disability.

Regarding general inspections or the evaluation of balance before and after the implementation of various intervention programs, the methods commonly used in clinical practice include the Berg Balance Scale (BBS) [12,13], the Performance-Oriented Mobility Assessment (POMA) [14], Single-Leg Stance (SLS) [14,15], the Time Up and Go Test (TUGT) [16], and the Three-Minute Walk test (3MWT) [17]. However, these methods or scales for assessing balance are mostly implemented by young people for the elderly. More research is required to verify whether they are applicable to children without intellectual disability. It is necessary to further explore the differences in the balance of children with intellectual disability before and after participating in intervention programs [18]. The Pediatric Balance Scale (PBS), as an example, is dedicated to children (revised from the Berg Balance Scale, BBS), and it is often used to assess movement deficit and balance in children without intellectual disability. Although the PBS obtained results empirically and with consistent reliability in a study of children without intellectual disability [19], there are many cognitive tests related to understanding, which may result in underestimating or overestimating the balance of children with intellectual disability. The evaluators may also subjectively judge the starting point of the action to be too early, concluding that children with intellectual disability have poor movement ability on the basis of this assessment [20]. In addition, evaluators may have different cognitive standards for the indicators in the checklist, and children with intellectual disability may have differing levels of ability to respond to the instructions. If there are quantitative indicators of equipment, there is an opportunity to eliminate the subjective influence of the evaluators.

In recent years, the sit-to-stand movement has been investigated using observational performance tests to assess the risk of falling or performance measurements [21,22]. The force platform system has been widely used in sit-to-stand research regarding elderly ability [23], yet the research on balance assessment tools applied in children is rare. This study uses the objective, fast, and accurate characteristics of the force platform system to analyze the key points of the sit-to-stand movement and the movement balance parameters of children with intellectual disability and children without intellectual disability. Regardless of the level of cognitive ability, a norm suitable for the assessment of the balance of children with intellectual disability was established, so as to facilitate their general examination or the assessment of balance before and after the implementation of various interventions programs that help movement development. Hence, this valuable result can provide researchers, special education teachers, and related professionals with an effective and time-saving evaluation of the balance of children with intellectual disability.

2. Grey Relational Analysis

Grey relational analysis (GRA) is used to carry out relational relevant analysis, model establishment, prediction, and decision making of a system under conditions of an uncertain research system model, incomplete information, and unclear operation status [24]. Its analysis is applicable to various fields, such as environmental engineering, agriculture, transportation, economics, medical treatment, sports, and education, with considerable research results [25,26,27,28,29,30,31,32,33,34]. This measurement method is used for the analysis of the relational degree of discrete sequences between various factors, in addition to identifying the irregularities of existing data. GRA generation methods are applied to reduce the randomness of data. The main objective of this data transformation is to discover hidden regularity. A closer development trend indicates a better relationship between factors, which is suitable for the calculation of measures between discrete sequences [35]. The basic analysis steps are mathematically described below.

In a GRA space,$\{P(X);\mathrm{\Gamma }\}$, sequences $x_i(x_i(1),x_i(2),x_i(3)\cdots ,x_i(k))\in X$ exist, where $i=0,1,2,\cdots ,m,k=1,2,3,\cdots ,n\in N$.

$$\begin{array}{c}{x}_0=(x_0(1),x_0(2),x_0(3),\cdots ,x_0(k))\\ x_1=(x_1(1),x_1(2),x_1(3),\cdots ,x_1(k))\\ x_2=(x_2(1),x_2(2),x_2(3),\cdots x_2(k))\\ ⋮=⋮\\ x_m=(x_m(1),x_m(2),x_m(3),\cdots ,x_m(k))\end{array}$$

(1)

According to the grey system theory, sequence$x_0(k)$is taken as the reference sequence, and the other sequences $x_i(k)$ are taken as comparison sequences, denoted as the localization GRA grade. The paper uses Nagai’s GRA grade, shown in Equation (2).

$${\mathrm{\Gamma }}_{0i}=\mathrm{\Gamma }(x_0(k),x_i(k))=\frac{{\overline{\Delta }}_{\max .}-{\overline{\Delta }}_{0i}}{{\overline{\Delta }}_{\max .}-{\overline{\Delta }}_{\min .}},{\overline{\Delta }}_{0i}=\sqrt{{\displaystyle \sum _{k=1}^n{\left[{\Delta }_{0i}(k)\right]}^2}},$$

(2)

where $i=1,2,3,\cdots ,m,k=1,2,3,\cdots ,n,j\in I$.

I.

$x_0$is a standard sequence, whereas $x_i$are comparison sequences.

II.

${\Delta }_{0i}=\left|\right|x_0(k)-x_i(k)\left|\right|$ is the norm between$x_0$and$x_i$, whereas ${\overline{\Delta }}_{0i}$ is the mean of ${\Delta }_{0i}$.

III.

${\Delta }_{\min .}=\underset{j\in i}{\overset{\min .\min .}{\forall }}\forall k\left|\right|x_0(k)-x_j(k)\left|\right|$ ${\Delta }_{\max .}=\underset{j\in i}{\overset{\max .\max .}{\forall }}\forall k\left|\right|x_0(k)-x_j(k)\left|\right|$.

3. Method

In this study, due to the difficulty in recruiting children with intellectual disability and the limited types and numbers of balance factors to be selected, grey system theory was used to establish a model and to make decisions in the context of system model uncertainty and system relational analysis. The study data were normalized to the best score with the aim of targeting the performance of children without intellectual disability using various balance parameters of the force platform system. To understand the relationship between the measurable time and weight factors describing the performance of children with intellectual disability and children without intellectual disability during the sit-to-stand movement on the force platform system, a comparative study was carried out as described below.

3.1. Participants

Intellectual disability is divided into four levels according to one’s IQ score: mild, moderate, severe, and extremely severe. Generally, there are huge differences in various abilities among those with intellectual disabilities. The IQ scores of those with mild intellectual disability (80% of the population) range from 55 to 70 points, which is about two to three standard deviations lower than average intelligence. The IQ scores of those with moderate intellectual disability (12% of the population) range from 40 to 55 points, which is about three to four standard deviations lower than average intelligence. Those with severe and extremely severe intellectual disability account for about 7% and 1% of the population, respectively. Typically, individuals in these groups are unable to completely care for themselves, and their balance is known to be poor; thus, these groups were not in the scope of this study.

This study recruited 13 children without intellectual disability (age: M = 9.6 years, SD = 1.4) with standard BMI and standard intelligence, 12 children with mild intellectual disability (age: M = 9.8 years, SD = 0.9), and 8 children with moderate intellectual disability (age: M = 8.9 years, SD = 0.8). The IQ of the children without intellectual disability was within one standard deviation of average intelligence, the IQ of the children with mild intellectual disability was between 55 and 70 points, and the IQ of the children with moderate intellectual disability was between 40 and 55 points. All children with intellectual disability required a disability card to verify their identity. In addition, all children had to meet six conditions: (1) age of 7–12 years old; (2) ability to stand from a chair without any help; (3) no serious cardiopulmonary abnormalities; (4) no medicine usage for 3 months which could affect or improve balance; (5) no previous orthopedic treatment involving lower back, pelvis, or limbs; (6) no acute diseases such as coronary heart disease, heart failure, or lung infection within the last 3 months.

In addition, all children and parents were informed of the experimental needs and signed the informed consent form, reviewed and approved by the research ethics review committee (NTU-REC, 201906ES015).

Table 1. Basic information of the participants.

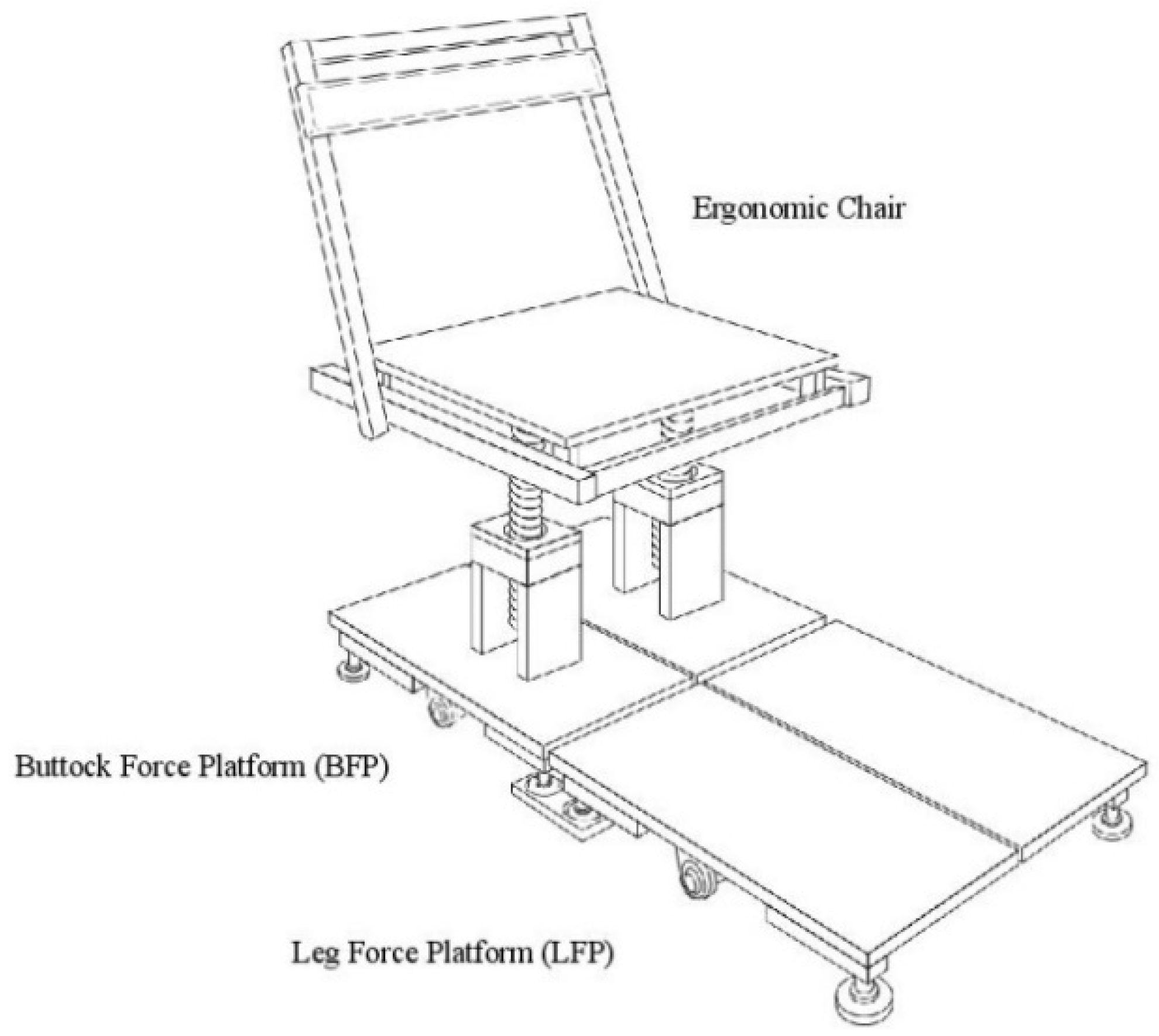
Variable	Children Without Intellectual Disability (n = 13)		Children with Mild Intellectual Disability (n = 12)		Children with Moderate Intellectual Disability (n = 8)
	M	SD	M	SD	M	SD
Age (years)	9.6	1.4	9.8	0.9	8.9	0.8
Weight (kg)	33.1	6.4	37.7	12.6	25.9	8.5
Hight (cm)	139.2	10.0	138.4	10.8	130.2	10.2
BMI	16.9	1.6	19.3	4.5	15.3	3.8

shows the basic information of the participants.

3.2. Apparatus

The key points underlying the performance of participants were recorded as a function of the force and time changes during the sit-to-stand movement on the force platform system. In this study, the ground reaction force was defined as the force applied to the ground or chair by the feet or buttocks, respectively. Vertical ground reaction forces were recorded from two separate force platforms (size, 500 mm × 500 mm; accuracy, 0.20 N) with each platform composed of four load cells. The distance between the force platforms was 10 mm (see the experimental apparatus in Figure 1). An experimental ergonomic chair was designed according to the specifications of the American National Standards Institute (ANSI) and the National Standards of the People’s Republic of China (GB). According to the ANSI, the angle of the seat plane and that between the seat plane and back were set to 4° and 100°, respectively. The depth and width of the seat were set to 40 and 45 cm, respectively. The height of the seat plane was adjustable between 32 and 46 cm (GB). A personal computer simultaneously saved the two sets of ground reaction force data at 1000 Hz using a 16 bit analog-to-digital converter acquisition board (NI PCI-6220; National Instruments) and dynamic amplifiers. Figure 1 shows the force platforms and ergonomic chair without armrests.

3.3. Procedure

The participants, with their arms folded across their chest, sat on an armless chair of popliteal height on a buttock force platform. To make sure that the participants’ trunks were leaning back in a standard position, a back support on the chair was used, while their bare feet were positioned on the leg force platform. There were no other restrictions applied to the initial position. Each participant performed the task in a comfortable and natural manner and at a self-selected speed. A registered nurse was present during all trials for safety.

3.4. Measurements

The force changes during the movement of the participant were measured using the force platform system, as shown in Figure 2. The horizontal axis shows the time course, while the vertical axis shows the ratio of the positive force measured by the force platform system to the weight of the participants (% body weight, BW). The two curves (Curve B, force on the hips; Curve L, force on the legs) obtained from the force platform system describe the ground reaction force (GRF) as a function of time, and Curve T is the sum of the force on the buttocks and legs (B + L). The profiles of Curves B (B_s), L (L_s), and T (T_s) were identified immediately after the action cue when the difference between the GRA at that timepoint and the previous value was not equal to zero. T_p and L_p occurred when the ground reaction force for Curves T and L, respectively, were maximal. Seat off was defined as the time at which the thighs lost contact with the chair, when the instant of Curve B was at zero (B₀). The GRF oscillated following seat off, and T_min represents the minimum body force during the ascending phase [36]. T_pw and L_pw are the maximum forces of Curves T and L, respectively.

In this study, the measurement results at key points of the sit-to-stand movement were obtained through the force platform system, yielding 12 factors in total (seven time factors and five weight factors) as shown in

Table 2. Explanation of Time Factors.

Time Factors (Time)
No.	Factors	Explanation	No.	Factors	Explanation
01	Bs–Tp	duration between Bs and Tp	05	Tp–Lp	duration between Tp and Lp
02	Bs–Lp	duration between Bs and Lp	06	Tp–seat off	duration between Tp and seat off
03	Bs–seat off	duration between Bs and seat off	07	Lp–seat off	duration between Lp and seatoff
04	Bs–end	duration between Bs and end

and

Table 3. Explanation of Weight Factors.

Weight Factors (Weight)
No.	Factors	Explanation
01	Tpw	The maximum ground reaction force of Curve T
02	Lpw	The maximum ground reaction force of Curve L
03	Tminw	The minimum body force during the ascending phase
04	Tpw–Tminw	The force difference between Tpw and Tminw
05	Lpw–Tminw	The force difference between Lpw and Tminw

.

Table 4. Raw data describing the balance factors of children without intellectual disability.

	Time Factors (ms)							Weight Factors
	Bs–Tp	Bs–Lp	Bs–Seat off	Bs–End	Tp–Lp	Tp–Seat off	Lp–Seat off	Tpw	Lpw	Tminw	Tpw–Tminw	Lpw–Tminw
1	433	499	632	1256	66	199	133	1.30	1.20	0.77	0.52	0.43
2	726	781	900	1739	55	174	119	1.47	1.31	0.70	0.78	0.61
3	725	745	821	2034	20	96	76	1.43	1.41	0.88	0.55	0.52
4	1074	1173	1245	2135	99	171	72	1.27	1.12	0.86	0.40	0.26
5	1043	1077	1233	2204	34	190	156	1.34	1.30	0.82	0.51	0.48
6	732	794	927	1675	62	195	133	1.31	1.25	0.84	0.47	0.41
7	753	767	811	1691	14	58	44	1.52	1.50	0.76	0.76	0.74
8	703	717	752	1375	14	49	35	1.44	1.42	0.66	0.78	0.76
9	484	629	717	1481	145	233	88	1.29	1.21	0.97	0.32	0.24
10	904	999	1183	1621	95	279	184	1.34	1.25	0.74	0.60	0.50
11	744	777	927	1784	33	183	150	1.52	1.41	0.93	0.59	0.48
12	623	699	798	1213	76	175	99	1.28	1.20	0.78	0.50	0.42
13	820	874	896	1607	54	76	22	1.23	1.21	0.77	0.46	0.44

,

Table 5. Raw data describing the balance factors of children with mild intellectual disability.

	Time Factors (ms)							Weight Factors
	Bs–Tp	Bs–Lp	Bs–Seat off	Bs–End	Tp–Lp	Tp–Seat off	Lp–Seat off	Tpw	Lpw	Tminw	Tpw–Tminw	Lpw–Tminw
14	1196	1359	1495	2656	163	299	136	1.19	1.13	0.88	0.31	0.25
15	918	980	1070	2189	62	152	90	1.23	1.21	0.89	0.34	0.32
16	1255	1315	1448	2388	60	193	133	1.20	1.16	0.93	0.27	0.23
17	885	903	1046	1921	18	161	143	1.33	1.30	0.72	0.61	0.58
18	1057	1089	1193	2421	32	136	104	1.18	1.15	0.81	0.36	0.33
19	697	817	879	1485	120	182	62	1.24	1.19	0.74	0.50	0.45
20	841	873	952	1638	32	111	79	1.10	1.08	0.89	0.21	0.19
21	1033	1096	1210	2289	63	177	114	1.17	1.11	0.83	0.35	0.29
22	885	929	1045	1914	44	160	116	1.26	1.23	0.83	0.43	0.40
23	946	1127	1217	1713	181	271	90	1.22	1.13	0.93	0.28	0.20
24	861	885	977	2189	24	116	92	1.20	1.19	0.96	0.24	0.23
25	902	978	1081	1948	76	179	103	1.28	1.16	0.95	0.34	0.22

and

Table 6. Raw data describing the balance factors of children with moderate intellectual disability.

	Time Factors (ms)							Weight Factors
	Bs–Tp	Bs–Lp	Bs–Seat off	Bs–End	Tp–Lp	Tp–Seat off	Lp–Seat off	Tpw	Lpw	Tminw	Tpw–Tminw	Lpw–Tminw
26	497	777	814	3085	280	317	37	1.06	1.03	0.90	0.16	0.13
27	1041	1124	1269	2754	83	228	145	1.12	1.09	0.94	0.18	0.14
28	972	1039	1160	1439	67	188	121	1.22	1.16	0.65	0.58	0.51
29	574	803	1079	1775	229	505	276	1.06	0.94	0.91	0.16	0.04
30	1851	2017	2218	3301	166	367	201	1.10	0.90	0.84	0.26	0.06
31	1154	1290	1375	3095	136	221	85	1.11	1.04	0.81	0.30	0.23
32	577	604	763	1082	27	186	159	1.21	1.19	0.56	0.65	0.63
33	1517	1602	1747	2666	85	230	145	1.15	1.10	0.84	0.30	0.26

show the raw data describing the balance of children without intellectual disability, children with mild intellectual disability, and children with moderate intellectual disability.

4. Calculation Results

The GRA calculation results of the time factors and weight factors obtained by the participants on the force platform system through the sit-to-stand performance are described below.

4.1. Time Factors

(1) Setting the standard sequence

The average of the seven factors of the transposition performance of children without intellectual disabilities on the force platform system was taken as the reference series.

$$x_0(k)=\frac{{\displaystyle \sum _{i=1}^N{x}_i(k)}}{N},$$

(3)

where N is the number of children without intellectual disability.

From Table 3, the data of 13 children without intellectual disability were substituted into Equation (3) to obtain the following standard sequence:

$$x_0=(751.0769,810.0769,910.9231,1678.0769,59,159.8462,100.8462).$$

(2) Setting the comparison sequences

Factors $x_1$ to $x_{13}$ for children without intellectual disability (Table 3), factors $x_{14}$ to $x_{25}$ for children with mild intellectual disability (Table 4), and factors $x_{26}$ to $x_{33}$ for children with moderate intellectual disability (Table 5) yielded the following comparison sequences:

$$x_1=(1.2964,1.1992,0.7724,0.524,0.4268),$$

$$x_2=(1.4731,1.3107,0.6981,0.7751,0.6126),$$

$$x_3=(1.4310,1.4056,0.8815,0.5495,0.5241),$$

$$x_{14}=(841,873,952,1638,32,111,79),$$

$$x_{15}=(1033,1096,1210,2289,63,177,114),$$

$$x_{16}=(885,929,1045,1914,44,160,1163),$$

$$x_{31}=(1154,1290,1375,3095,136,221,85),$$

$$x_{32}=(577,604,763,1082,27,186,159),$$

$$x_{33}=(1517,1602,1747,2666,85,230,145).$$

(3) Determining the difference sequences

$$\begin{array}{l}{\Delta }_{01}=(318.0769,311.0769,278.9231,422.077,7,39.1538,32.1538),\\ {\overline{\Delta }}_{01}=201.2088.\end{array}$$

$$\begin{array}{l}{\Delta }_{02}=(25.0769,29.0769,10.9231,60.923,4,14.1538,18.1538),\\ {\overline{\Delta }}_{02}=23.1868.\end{array}$$

$$\begin{array}{l}{\Delta }_{03}=(26.0769,65.0769,89.9231,355.923,39,63.8462,24.8462),\\ {\overline{\Delta }}_{03}=94.956.\end{array}$$

$$\begin{array}{l}{\Delta }_{14}=(89.9231,62.9231,41.0769,40.077,27,48.8462,21.8462),\\ {\overline{\Delta }}_{14}=47.3846.\end{array}$$

$$\begin{array}{l}{\Delta }_{15}=(281.9231,285.9231,299.0769,610.923,4,17.1538,13.1538),\\ {\overline{\Delta }}_{15}=216.0220.\end{array}$$

$$\begin{array}{l}{\Delta }_{16}=(133.9231,118.9231,134.0769,235.923,15,0.1538,15.1538,),\\ {\overline{\Delta }}_{16}=93.3077.\end{array}$$

$$\begin{array}{l}{\Delta }_{31}=(402.9231,479.9231,464.0769,1416.923,77,61.1538,15.8462),\\ {\overline{\Delta }}_{31}=416.8352.\end{array}$$

$$\begin{array}{l}{\Delta }_{32}=(174.0769,206.0769,147.9231,596.077,32,26.1538,58.1538),\\ {\overline{\Delta }}_{32}=177.2088.\end{array}$$

$$\begin{array}{l}{\Delta }_{33}=(765.9231,791.9231,836.0769,987.923,26,70.1538,44.1538),\\ {\overline{\Delta }}_{33}=503.1648.\end{array}$$

Δ_max = 1622.923, Δ_min = 01538.

(4) Calculating the GRA grade

The results were substituted into Equation (2) to calculate the GRA grade, as shown in

Table 7. GRA grade for time factors of balance.

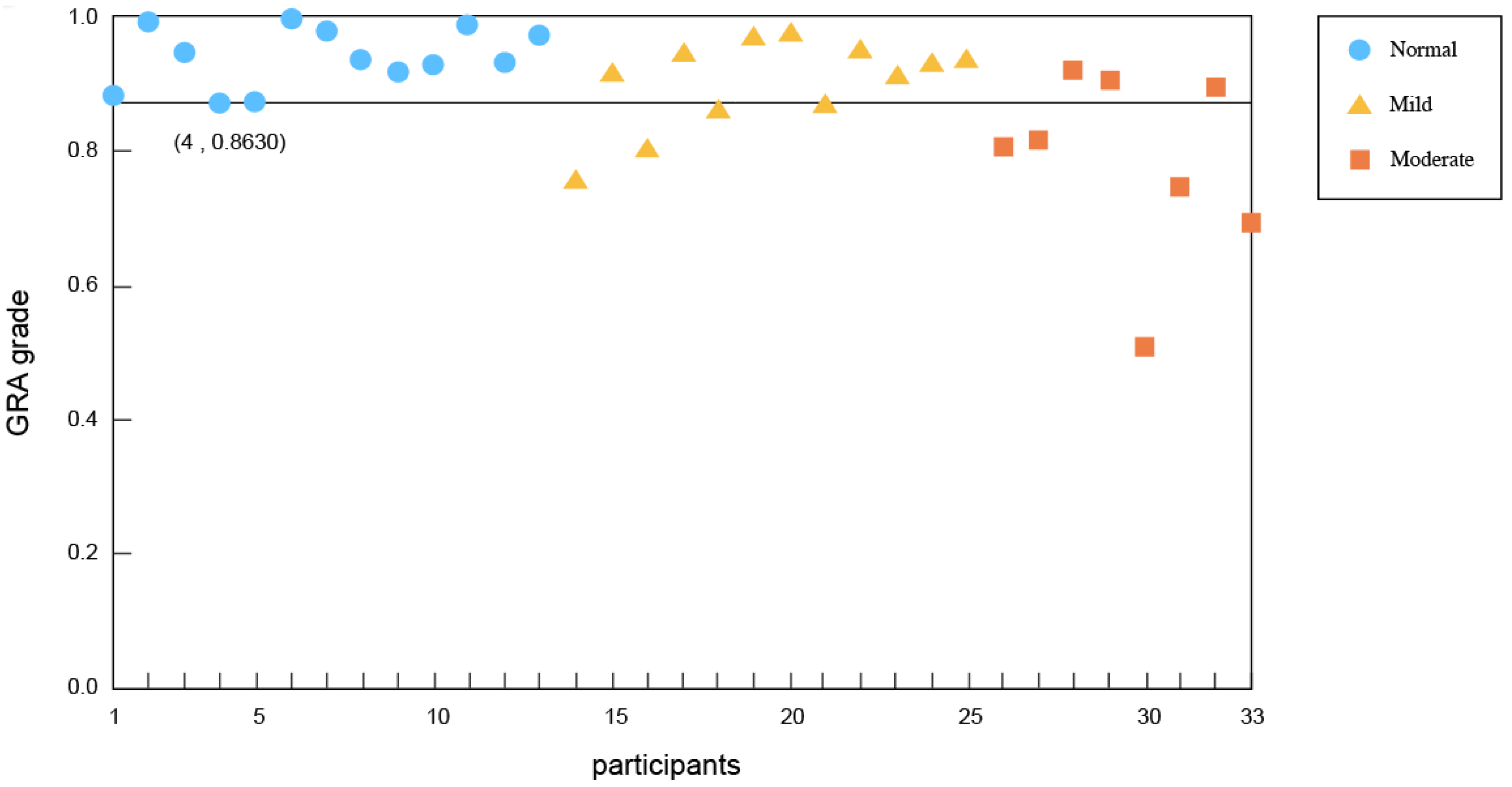
No.	Grey Relational Grade	No.	Grey Relational Grade	No.	Grey Relational Grade
x1	0.876104	x15	0.909550	x29	0.899785
x2	0.985806	x16	0.795669	x30	0.502607
x3	0.941580	x17	0.939427	x31	0.743228
x4	0.863041	x18	0.853622	x32	0.890893
x5	0.866535	x19	0.964177	x33	0.690029
x6	0.989125	x20	0.970895
x7	0.968267	x21	0.866975
x8	0.927481	x22	0.942596
x9	0.911080	x23	0.903536
x10	0.920046	x24	0.925307
x11	0.977166	x25	0.929919
x12	0.925165	x26	0.803517
x13	0.966067	x27	0.808698
x14	0.750596	x28	0.912563

.

4.2. Weight Factors

(1) Setting the standard sequence

Similarly, using Equation (3), the average performance of the five factors of the transposition performance of children without intellectual disabilities on the force platform system was regarded as the target value of reference series, and the standard sequence was obtained.

From Table 3, the data of 21 children without intellectual disability were substituted into Equation (3) to obtain the following standard sequence:

$$x_0=(1.3649,1.2911,0.8073,0.5576,0.4838).$$

(2) Setting the comparison sequences

Factors $x_1$ to $x_{13}$ for children without intellectual disability (Table 3), factors $x_{14}$ to $x_{25}$ for children with mild intellectual disability (Table 4), and factors $x_{26}$ to $x_{33}$ for children with moderate intellectual disability (Table 5) yielded the following comparison sequences:

$$x_1=(1.2964,1.1992,0.7724,0.524,0.4268),$$

$$x_2=(1.4731,1.3107,0.6981,0.7751,0.6126),$$

$$x_3=(1.4310,1.4056,0.8815,0.5495,0.5241),$$

$$x_{14}=(1.1009,1.0818,0.8906,0.2103,0.1912),$$

$$x_{15}=(1.1704,1.113,0.8252,0.3452,0.2878),$$

$$x_{16}=(1.2589,1.2279,0.829,0.4299,0.3989),$$

$$x_{31}=(1.1081,1.041,0.811,0.2971,0.23),$$

$$x_{32}=(1.2124,1.1924,0.5578,0.6546,0.6346),$$

$$x_{33}=(1.145,1.1009,0.8413,0.3038,0.2597).$$

(3) Determining the difference sequences

$${\Delta }_{01}=(0.0685,0.0919,0.349,0.0336,0.057),{\overline{\Delta }}_{01}=0.0572.$$

$${\Delta }_{02}=(0.1082,0.0196,0.1092,0.2175,0.1288),{\overline{\Delta }}_{02}=0.1167.$$

$${\Delta }_{03}=(0.0661,0.1145,0.0742,0.0081,0.0403),{\overline{\Delta }}_{03}=0.0606.$$

$${\Delta }_{14}=(0.2640,0.2093,0.0833,0.3473,0.2926),{\overline{\Delta }}_{14}=0.2393.$$

$${\Delta }_{15}=(0.1945,0.1781,0.0179,0.2124,0.1960),{\overline{\Delta }}_{15}=0.1598.$$

$${\Delta }_{16}=(0.106,0.0632,0.0217,0.1277,0.0849),{\overline{\Delta }}_{16}=0.0807.$$

$${\Delta }_{31}=(0.2568,0.2501,0.0037,0.2605,0.2538),{\overline{\Delta }}_{31}=0.2050.$$

$${\Delta }_{32}=(0.1525,0.0987,0.2495,0.097,0.1508),{\overline{\Delta }}_{32}=0.1497.$$

$${\Delta }_{33}=(0.2199,0.1902,0.034,0.2539,0.2241),{\overline{\Delta }}_{33}=0.1844.$$

Δ_max = 0.4479, Δ_min = 0.0015.

(4) Calculating the GRA grade

The results were substituted into Equation (2) to calculate the GRA grade, as shown in

Table 8. GRA grade for weight factors of balance.

No.	Grey Relational Grade	No.	Grey Relational Grade	No.	Grey Relational Grade
x1	0.8753	x15	0.6995	x29	0.2879
x2	0.7420	x16	0.5694	x30	0.3733
x3	0.8676	x17	0.8785	x31	0.5442
x4	0.6888	x18	0.6964	x32	0.6681
x5	0.9560	x19	0.8309	x33	0.5903
x6	0.8778	x20	0.4673
x7	0.6153	x21	0.6455
x8	0.6258	x22	0.8226
x9	0.6493	x23	0.5610
x10	0.9163	x24	0.5637
x11	0.8071	x25	0.6274
x12	0.8537	x26	0.3680
x13	0.8235	x27	0.4199
x14	0.6051	x28	0.7889

.

5. Discussion

Using the force platform system, taking into account the GRA grade for the time factors and the weight factors with respect to the lower limit of children without intellectual disability, the sit-to-stand movement was measured to evaluate the balance of children with mild intellectual disability and moderate intellectual disability. The results revealed that the lower limit of the GRA grade for the time factor of children without intellectual disability was 0.8630, and the proportions of children with mild and moderate intellectual disability under this limit were 25% and 62.5%, respectively. The lower limit of the GRA grade for the weight factor of children without intellectual disability was 0.6153, and the proportions of the children with mild and moderate intellectual disability under this limit were 46.9% and 75%, respectively. The results show that, in terms of both the time factor and the weight factor, the proportion of children with intellectual disability who had lower performance than children without intellectual disability in the sit-to-stand movements tended to increase with the severity of intellectual disability. Figure 3 and Figure 4 show the GRA grade distribution diagrams of the time factor and weight factor of the participants.

In order to establish a norm for evaluating the balance of children with intellectual disability, the time factors and weight factors obtained in Figure 3 and Figure 4 were used as the x-axis and y-axis to plot the distribution areas of the GRA. Using the GRA grades 0.8630 for the time factor and 0.6153 for the weight factor as the boundaries, four regions were distinguished, as shown in Figure 5: Area I, GRA grade of the time factor ≥0.8630 and GRA grade of the weight factor ≥0.6153; Area II, GRA grade of the time factor <0.8630 and GRA grade of the weight factor ≥0.6153; Area III, GRA grade of the time factor <0.8630 and GRA grade of the weight factor <0.6153; Area IV, GRA grade of the time factor ≥0.8630 and GRA grade of the weight factor <0.6153. The population of each group in the region is shown in

Table 9. The population of each group in the GRA grade distribution areas.

	Children Without Intellectual Disability	Children with Mild Intellectual Disability	Children with Moderate Intellectual Disability
I	100.0%	50.0%	25.0%
II	0%	8.3%	0%
III	0%	16.7%	62.5%
IV	0%	25.0%	12.5%

.

The composition of the time and weight factor parameters in this study reflects the time and force differences of the participants during the sit-to-stand movement as recorded by the force platform system. Although the influence of each factor on the balance of different intellectual groups is unknown and the operation status is unclear, through GRA grade analysis, it can be clearly seen that the translocation performance of children with different levels of intellectual disability was different from the trend seen in children without intellectual disability.

The participants in Area I were characterized by a fast transposition movement speed and high physical endurance; as the performance of children without intellectual disability was taken as the boundary, all children without intellectual disability were located in this area. Half of the children with mild intellectual disability (50.0%) and a small number of children with moderate intellectual disability (25.0%) were also located in Area I. Typically, the intelligence level of children with intellectual disability is lower to that of children without intellectual disability, but the performance of transposition or balance in daily life is still the same, which is consistent with the observation.

The participants in Area II exhibited a slower transposition movement speed but high physical endurance. In this study, only a few children with mild intellectual disability (8.3%) were located in this area, suggesting a slow motion speed to achieve a standing position, while using less force.

The characteristics of the participants in Area III were a slow transposition movement speed and low physical endurance. It is noticeable that a high percentage (62.5%) of children with moderate intellectual disability were located in this area, whereas children with mild intellectual disability accounted for 16.7%. Participants in this area adopted the strategy of slowing down and reducing the impact of force to complete the transposition. The reason may be that most children with moderate intellectual disability have poor physical strength; alternatively, this may have been due to more falling experiences in the past. The formation of self-protection is very similar to the performance seen in the frail and elderly in transposition.

In contrast to Area II, participants in Area IV were characterized by a fast transposition speed but low physical endurance. This seems to be the most efficient strategy with the lowest physical burden, but only a small number of children with mild intellectual disability (25.0%) and moderate intellectual disability (12.5%) were in this range. This may be related to the transposition strategy of less intelligent participants who exhibit higher balance skills due to psychological factors and relatively strong lower-limb muscle strength.

6. Conclusions

The clinical assessment of balance is mostly carried out using a slightly subjective assessment tool commonly used in the assessment of children’s balance. Although there are empirical studies on the reliability of children without intellectual disability, when applied to children with intellectual disability, there may be limitations due to their cognition, thus increasing the risk of misjudgment. This study provided an assessment method for the balance of children with intellectual disability, using the objective, fast, and accurate force platform system. The key parameters of the sit-to-stand movements of children without intellectual disabilities were derived using GRA, and the lower limits of the GRA grade for time factors and weight factors were used as the boundaries to plot the GRA distribution areas. After analyzing the differences between each participant and the target, a norm for evaluating the balance of children with intellectual disability was established.

The balance assessment of children with intellectual disability showed that the majority of children with mild intellectual disability and a small number of children with moderate intellectual disability fell into Area I. Obviously, although these children have lower intelligence than children without intellectual disability, their daily activities and balance are equivalent. Moreover, a few children with mild intellectual disability and moderate intellectual disability who fell into Area II and Area IV had lower motor efficiency and tolerance than children without intellectual disability. Rehabilitation can be carried out through intervention programs such as relevant balance training courses to achieve the level of balance typically seen in children without intellectual disability. In this study, several children with moderate intellectual disability (62.5%) fell into Area III. It is obvious that low daily activity and balance are indeed related to intelligence. It is recommended that people who fall into this category should be more actively involved in rehabilitation intervention programs. Following their implementation, the balance of children with intellectual disability can be improved, and fall injuries can be reduced, allowing them to be more independent in their daily activities and enhancing their quality of life.