Computational Experiments with the Roots of Fibonacci-like Polynomials as a Window to Mathematics Research
Abstract
:1. Introduction
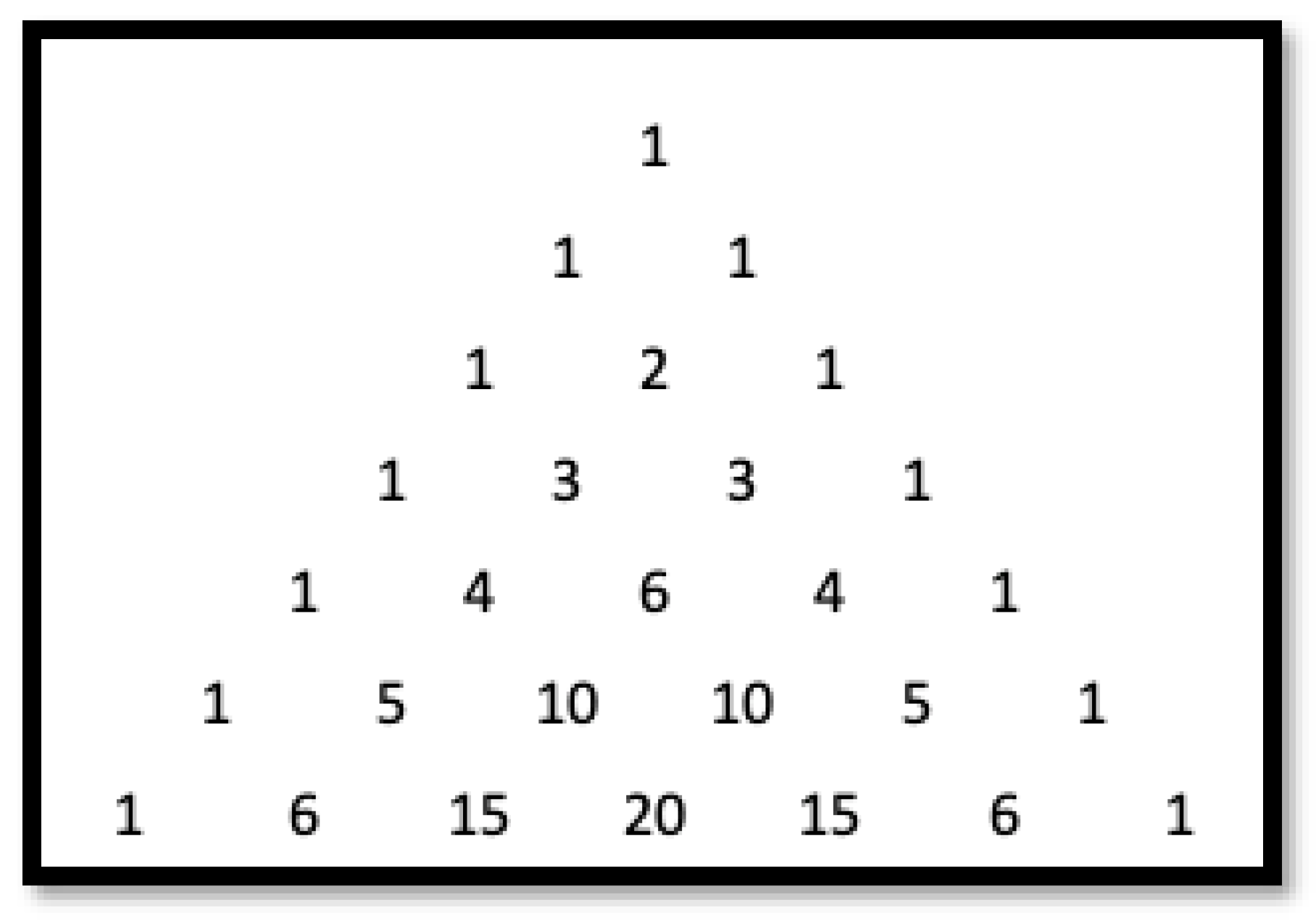
2. Connecting Fibonacci-like Polynomials to Cycles Formed by Generalized Golden Ratios
3. Ordinality Assignments of the Roots of Fibonacci-like Polynomials
4. Exploring the Case of 2kp-Cycles (Period-Doubling) in Fibonacci-like Polynomials
4.1. The Case of the Largest Root of the Polynomial
- (1)
- The largest root of a fourth degree Fibonacci-like polynomial that forms a trivial 10-cycle from two identical 5-cycles coincides with ;
- (2)
- The second root from the right on the ordered list of the roots of a ninth degree Fibonacci-like polynomial that forms a trivial 20-cycle from four identical 5-cycles coincides with ;
- (3)
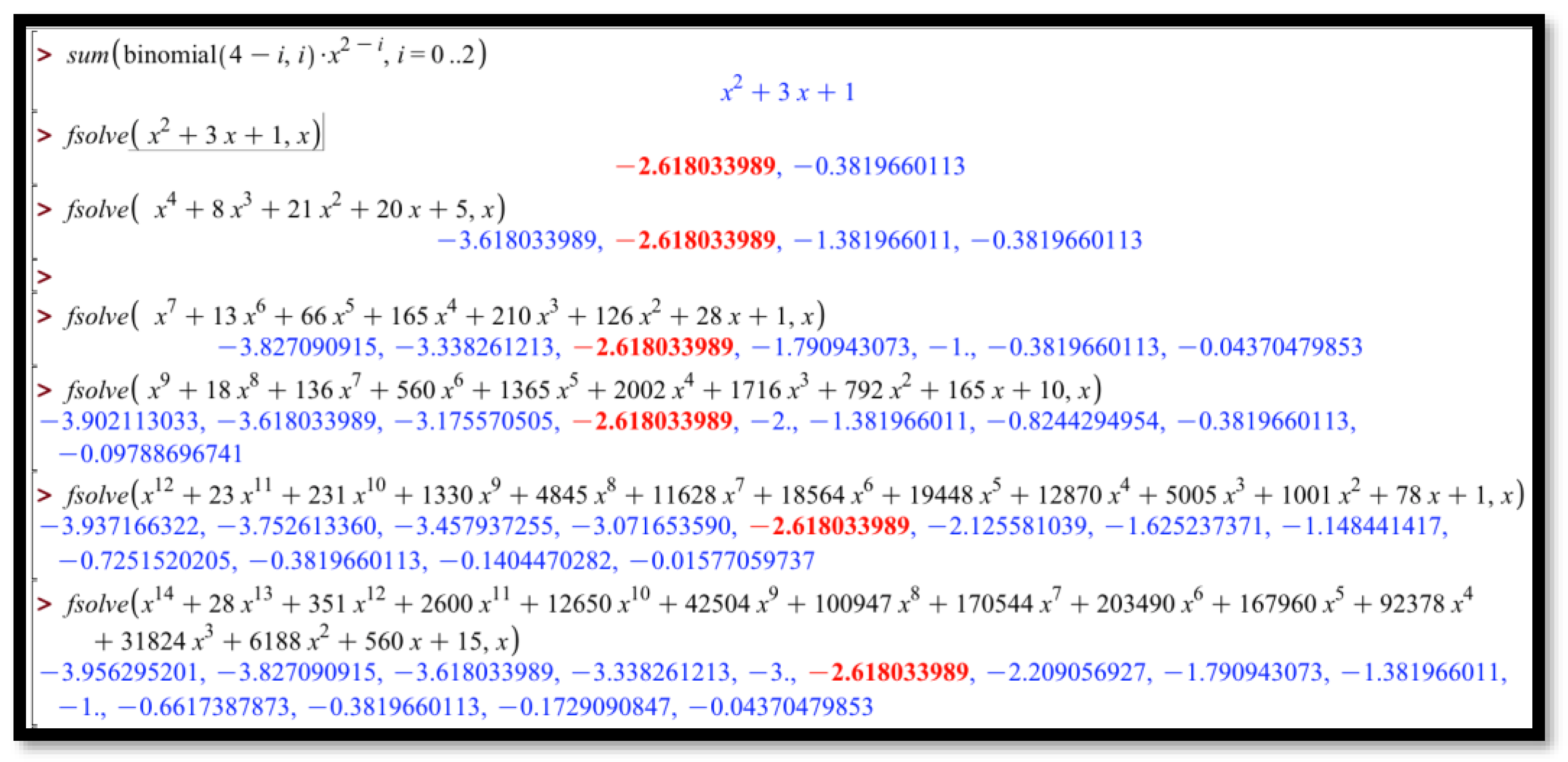
- The fourth root from the right on the ordered list of the roots of a 19th degree Fibonacci-like polynomial that forms a trivial 40-cycle from eight identical 5-cycles coincides with (Figure 5);
- (4)
- The eighth root from the right on the ordered list of the roots of a 39th degree Fibonacci-like polynomial that forms a trivial 80-cycle from 16 identical 5-cycles coincides with , and so on.
4.2. The Case of the Largest Root of the Polynomial
- (1)
- It is the first root from the right on the ordered list of the roots of a 6th degree Fibonacci-like polynomial that is responsible for a trivial cycle of period 14;
- (2)
- It is the second root from the right on the ordered list of the roots of a 13th degree Fibonacci-like polynomial that is responsible for a trivial cycle of period 28;
- (3)
- It is the fourth root from the right on the ordered list of the roots of a 27th degree Fibonacci-like polynomial that is responsible for a trivial cycle of period 56 (see Figure 6);
- (4)
- It is the eighth root from the right on the ordered list of the roots of a 55th degree Fibonacci-like polynomial that is responsible for a trivial cycle of period 112, and so on. Noting that the following inductive generalization can be made.
- (1)
- It is the second root both from the right and the left on the ordered list of the roots of a third degree Fibonacci-like that is responsible for a trivial 8-cycle;
- (2)
- It is the fourth root both from the right and the left on the ordered list of the roots of a seventh degree polynomial that is responsible for a trivial 16-cycle;
- (3)
- It is the eighth root both from the right and the left on the ordered list of the roots a 15th degree polynomial that is responsible for a trivial 32-cycle, and so on.
4.3. The Case of the Smallest Root of the Polynomial
- (1)
- The second root from the left on the ordered list of the roots of a fourth degree Fibonacci-like polynomial that forms a trivial 10-cycle from two identical 5-cycles;
- (2)
- The fourth root from the left on the ordered list of the roots of a ninth degree Fibonacci-like polynomial that forms a trivial 20-cycle from four identical 5-cycles;
- (3)
- The eighth root from the left on the ordered list of the roots of a 19th degree Fibonacci-like polynomial that forms a trivial 40-cycle from eight identical 5-cycles (see Figure 5);
- (4)
- The 16th root from the left on the ordered list of the roots of a 39th degree Fibonacci-like polynomial that forms a trivial 80-cycle from 16 identical 5-cycles, and so on.
- (1)
- The second root from the left on the ordered list of the roots of a sixth degree Fibonacci-like polynomial responsible for a trivial cycle of period 14 constructed out of two 7-cycles;
- (2)
- The fourth root from the left on the ordered list of the roots of a 13th degree Fibonacci-like polynomial responsible for a trivial cycle of period 28 constructed out of four 7-cycles;
- (3)
- The eighth root from the left on the ordered list of the roots of a 27th degree Fibonacci-like polynomial responsible for a trivial cycle of period 56 constructed out of eight 7-cycles (see Figure 6);
- (4)
- The 16th root from the left on the ordered list of the roots of a 55th degree Fibonacci-like polynomial responsible for a trivial cycle of period 112 constructed out of 16 7-cycles, and so on.
5. Generalization in the Case of Period Doubling Cycles
6. The Case of kp-Cycles (Period-Multiplying) in Fibonacci-like Polynomials
- (1)
- When n = 0, the number −1 is the first from the right and the first from the left;
- (2)
- When n = 1, the number −1 is the first from the right and the second from the left;
- (3)
- When n = 2, the number −1 is the second from the right and the third from the left;
- (4)
- When n = 3, the number −1 is the second from the right and the fourth from the left;
- (5)
- When n = 4, the number −1 is the third from the right and the fifth from the left;
- (6)
- When n = 5, the number −1 is the third from the right and the sixth from the left.
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Abramovich, S.; Leonov, G.A. Revisiting Fibonacci Numbers through a Computational Experiment; Nova Science Publishers: New York, NY, USA, 2019. [Google Scholar]
- Kline, M. Mathematics for the Non-Mathematician; Dover: New York, NY, USA, 1985. [Google Scholar]
- Borwein, J.M.; Bailey, D.H. Mathematics by Experiment: Plausible Reasoning in the 21st Century; A. K. Peters: Natick, MA, USA, 2008. [Google Scholar]
- Webb, W.; Parberry, E. Divisibility properties of Fibonacci polynomials. Fibonacci Q. 1969, 7, 457–463. [Google Scholar]
- Falcon, S.; Plaza, A. The k-Fibonacci sequence and the Pascal 2-triangle. Chaos Solut. Fractals 2007, 33, 38–49. [Google Scholar] [CrossRef]
- Abramovich, S.; Leonov, G.A. Fibonacci numbers revisited: Technology-motivated inquiry into a two-parametric difference equation. Int. J. Math. Educ. Sci. Technol. 2008, 39, 749–766. [Google Scholar] [CrossRef]
- Leonov, G.A.; Shumafov, M.M. Stabilization of Linear Systems; Cambridge Scientific Publishers: Cambridge, UK, 2012. [Google Scholar]








| F-L pol. | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Pol. deg. | 2 | 4 | 7 | 9 | 12 | 14 | 17 | 19 | 22 |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | |
| 2 | 3 | 5 | 6 | 8 | 9 | 11 | 12 | 14 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abramovich, S.; Kuznetsov, N.V.; Leonov, G.A. Computational Experiments with the Roots of Fibonacci-like Polynomials as a Window to Mathematics Research. Axioms 2022, 11, 48. https://doi.org/10.3390/axioms11020048
Abramovich S, Kuznetsov NV, Leonov GA. Computational Experiments with the Roots of Fibonacci-like Polynomials as a Window to Mathematics Research. Axioms. 2022; 11(2):48. https://doi.org/10.3390/axioms11020048
Chicago/Turabian StyleAbramovich, Sergei, Nikolay V. Kuznetsov, and Gennady A. Leonov. 2022. "Computational Experiments with the Roots of Fibonacci-like Polynomials as a Window to Mathematics Research" Axioms 11, no. 2: 48. https://doi.org/10.3390/axioms11020048
APA StyleAbramovich, S., Kuznetsov, N. V., & Leonov, G. A. (2022). Computational Experiments with the Roots of Fibonacci-like Polynomials as a Window to Mathematics Research. Axioms, 11(2), 48. https://doi.org/10.3390/axioms11020048








