Randomly Stopped Sums, Minima and Maxima for Heavy-Tailed and Light-Tailed Distributions
Abstract
:1. Introduction
2. Heavy-Tailed and Light-Tailed Distributions
- (i)
- F is heavy-tailed,
- (ii)
- for any ,
- (iii)
- .
- (i’)
- F is light-tailed,
- (ii’)
- for some ,
- (iii’)
- .
- (i)
- If andthen .
- (ii)
- If , and for some and large x (), then .
3. Main Results
- (i)
- for any , and ;
- (ii)
- for some , and ;
- (iii)
- for some , and for all ;
- (iv)
- for some and .
- (v)
- , , for all and
- (vi)
- for some , and .
- (i)
- If for some and for all , then ;
- (ii)
- If for some , then ;
- (iii)
- Distribution belongs to the class if , for all , and
- (i)
- If andfor , then and
- (ii)
- If for , then .
- (i)
- If and for , then and
- (ii)
- If , then for any r.v. ν.
- (i)
- for all and ;
- (ii)
- for some and ;
- (iii)
- ;
- (iv)
- for some in the case of infinite or for some in the case of finite .
- (v)
- for some and .
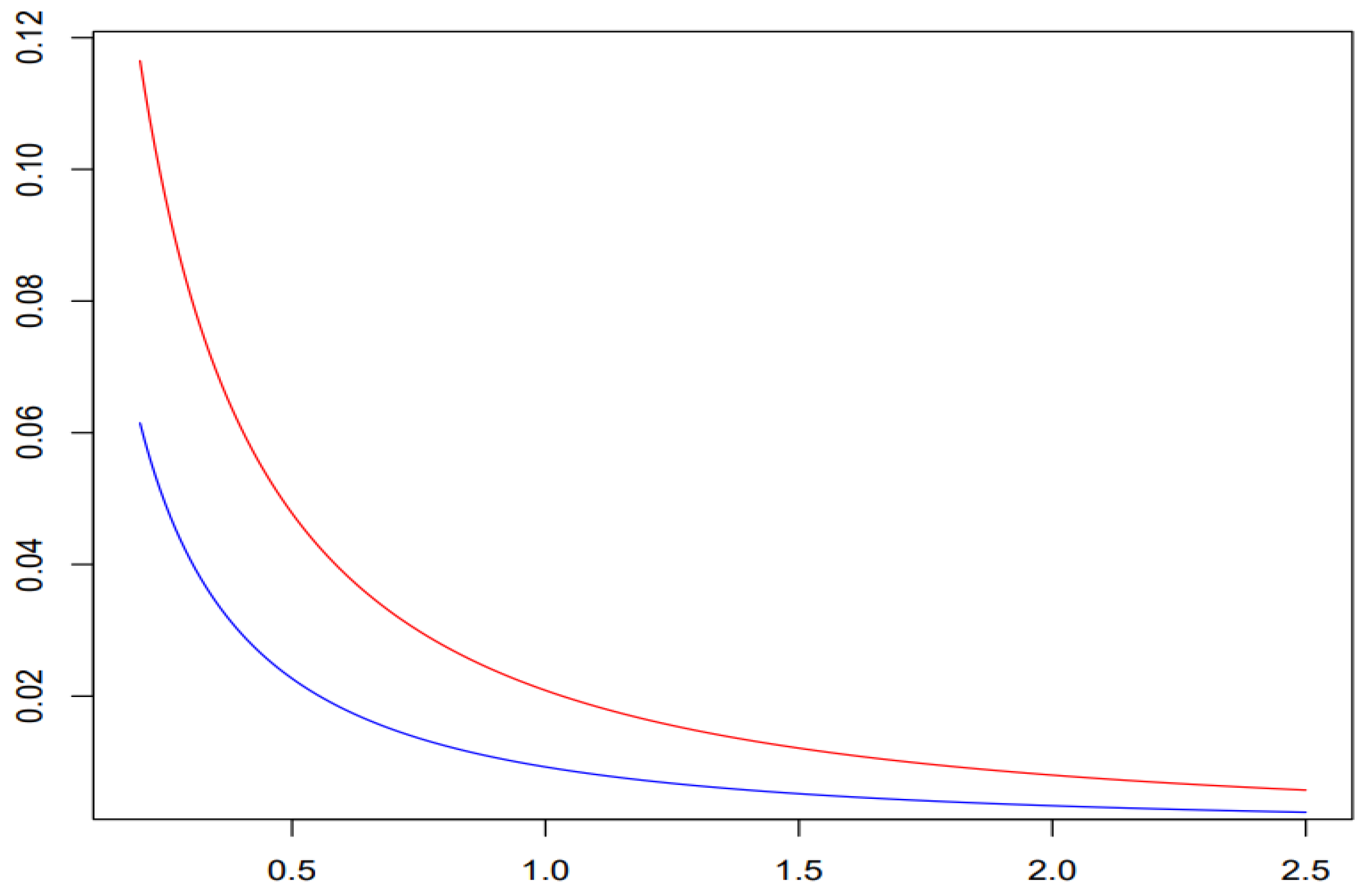
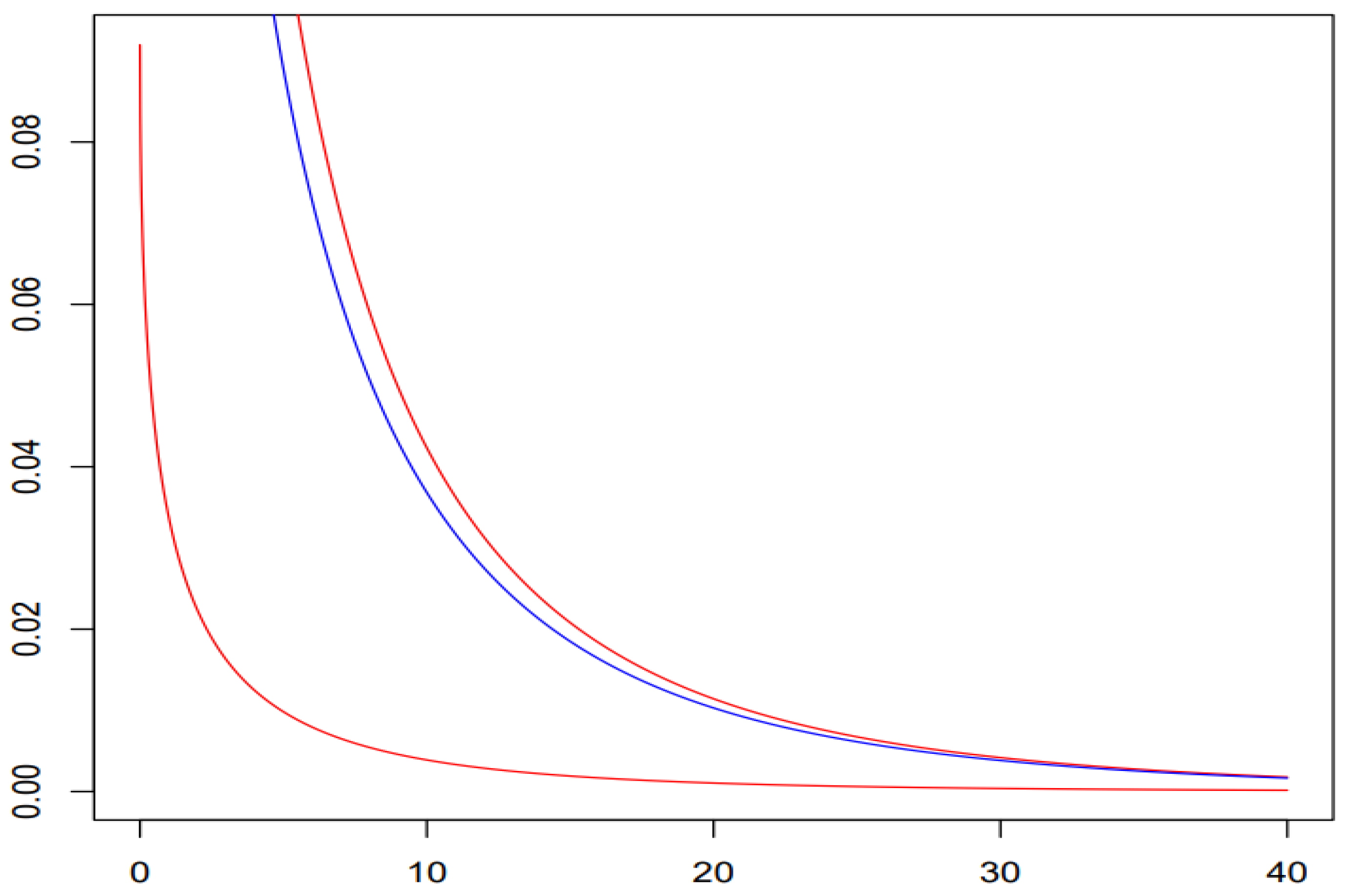
4. Examples
5. Proofs of the Main Results
5.1. Proof of Proposition 1
5.2. Proof of Proposition 2
5.3. Proof of Proposition 3
5.4. Proof of Proposition 4
5.5. Proof of Proposition 5
6. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Albin, J.M.P. A note on the closure of covolution power mixtures (random sums) of exponential distributions. J. Aust. Math. Soc. 2008, 84, 1–7. [Google Scholar] [CrossRef]
- Andrulytė, I.M.; Manstavičius, M.; Šiaulys, J. Randomly stopped maximum and maximum of sums with consistently varying distributions. Mod. Stoch. Theory Appl. 2017, 4, 65–78. [Google Scholar] [CrossRef]
- Cheng, D.; Ni, F.; Pakes, A.G.; Wang, Y. Some properties of the exponential distribution class with application to risk theory. J. Korean Stat. Soc. 2012, 41, 515–527. [Google Scholar] [CrossRef]
- Cline, D.B.H. Convolutions of the distributions with exponential tails. J. Aust. Math. Soc. 1987, 43, 347–365. [Google Scholar] [CrossRef]
- Danilenko, S.; Šiaulys, J. Random convolution of O-exponential distributions. Nonlinear Anal. Model. 2015, 20, 447–454. [Google Scholar] [CrossRef]
- Danilenko, S.; Šiaulys, J. Randomly stopped sums of not identically distributed heavy tailed random variables. Stat. Probab. Lett. 2016, 113, 84–93. [Google Scholar] [CrossRef]
- Danilenko, S.; Markevičiūtė, J.; Šiaulys, J. Randomly stopped sums with exponential-type distributions. Nonlinear Anal. Model. 2017, 22, 793–807. [Google Scholar] [CrossRef]
- Danilenko, S.; Paškauskaitė, S.; Šiaulys, J. Random convolution of inhomogeneous distributions with O-exponential tail. Mod. Stoch. Theory Appl. 2016, 3, 79–94. [Google Scholar] [CrossRef]
- Danilenko, S.; Šiaulys, J.; Stepanauskas, G. Closure properties of O-exponential distributions. Stat. Probab. Lett. 2018, 140, 63–70. [Google Scholar] [CrossRef]
- Dirma, M.; Nakliuda, N.; Šiaulys, J. Generalized moments of sums with heavy-tailed random summands. Lith. Math. J. 2023, 63, 254–271. [Google Scholar] [CrossRef]
- Embrechts, P.; Goldie, C.M. On the closure and factorization properties of subexponential and related distributions. J. Aust. Math. Soc. Ser. A 1980, 29, 243–256. [Google Scholar] [CrossRef]
- Geng, B.; Liu, Z.; Wang, S. A Kesten-type inequality for randomly weighted sums of dependent subexponential random variables with applications to risk theory. Lith. Math. J. 2023, 63, 81–91. [Google Scholar] [CrossRef]
- Karasevičienė, J.; Šiaulys, J. Randomly stopped sums with generalized subexponential distribution. Axioms 2023, 12, 641. [Google Scholar] [CrossRef]
- Karasevičienė, J.; Šiaulys, J. Randomly stopped minimum, maximum, minimum of sums and maximum of sums with generalized subexponential distributions. Axioms 2024, 13, 85. [Google Scholar] [CrossRef]
- Kizinevič, E.; Sprindys, J.; Šiaulys, J. Randomly stopped sums with consistently varying distributions. Mod. Stoch. Theory Appl. 2016, 3, 165–179. [Google Scholar] [CrossRef]
- Konstantinides, D.; Leipus, R.; Šiaulys, J. A note on product-convolution for generalized subexponential distributions. Nonlinear Anal. Model. 2022, 27, 11054–11067. [Google Scholar] [CrossRef]
- Konstantinides, D.; Leipus, R.; Šiaulys, J. On the non-closure under convolution for strong subexponential distributions. Nonlinear Anal. Model. 2023, 28, 97–115. [Google Scholar] [CrossRef]
- Leipus, R.; Šiaulys, J. Closure of some heavy-tailed distribution classes under random convolution. Lith. Math. J. 2012, 52, 249–258. [Google Scholar] [CrossRef]
- Leipus, R.; Šiaulys, J. On the random max-closure for heavy-tailed random variables. Lith. Math. J. 2017, 57, 208–221. [Google Scholar] [CrossRef]
- Lin, J.; Wang, Y. New examples of heavy-tailed O-subexponenial distributions and related closure properties. Stat. Probab. Lett. 2012, 82, 427–432. [Google Scholar] [CrossRef]
- Ragulina, O.; Šiaulys, J. Randomly stopped minima and maxima with exponential-type distributions. Nonlinear Anal. Model. 2019, 24, 297–313. [Google Scholar] [CrossRef]
- Shimura, T.; Watanabe, T. Infinite divisibility and generalized subexponentiality. Bernoulli 2005, 11, 445–469. [Google Scholar] [CrossRef]
- Sprindys, J.; Šiaulys, J. Regularly distributed randomly stopped sum, minimum and maximum. Nonlinear Anal. Model. 2020, 25, 509–522. [Google Scholar] [CrossRef]
- Teicher, H. Moments of randomly stopped sums revisited. J. Theor. Probab. 1995, 8, 779–793. [Google Scholar] [CrossRef]
- Tesemnikov, P.I. On the distribution tail of the sum of the maxima of two randomly sums in the presence of heavy tails. Sib. Elektron. Mat. Izv. 2019, 16, 1785–1794. [Google Scholar] [CrossRef]
- Watanabe, T. Convolution equivalence and distributions of random sums. Probab. Theory Relat. Fields 2008, 142, 367–397. [Google Scholar] [CrossRef]
- Watanabe, T. The Wiener condition and the conjectures of Embrechts and Goldie. Ann. Probab. 2019, 47, 1221–1239. [Google Scholar] [CrossRef]
- Watanabe, T.; Yamamuro, K. Ratio of the tail of an infinity divisible distribution on the line to that of its Lévy measure. Electron. J. Probab. 2010, 15, 44–74. [Google Scholar] [CrossRef]
- Xu, H.; Foss, S.; Wang, Y. Convolution and convolution-root properties of long-tailed distributions. Extremes 2015, 18, 605–628. [Google Scholar] [CrossRef]
- Xu, H.; Wang, Y.; Cheng, D.; Yu, C. On the closure under infinitely divisible distribution roots. Lith. Math. J. 2022, 62, 258–287. [Google Scholar] [CrossRef]
- Markovich, N.M.; Rodionov, I.V. Maxima and sums of non-stationary random length sequences. Extremes 2020, 23, 451–464. [Google Scholar] [CrossRef]
- Leipus, R.; Šiaulys, J.; Konstantinides, D. Minimum of heavy-tailed random variables is not heavy-tailed. AIMS Math. 2023, 8, 13066–13072. [Google Scholar] [CrossRef]
- Andersen, E.S. On the collective theory of Risk in case of contagion between claims. Bull. Inst. Math. Appl. 1957, 12, 275–279. [Google Scholar]
- Asmussen, S.; Albrecher, H. Ruin Probabilities, 2nd ed.; Word Scientific: Singapore, 2010. [Google Scholar]
- Denisov, D.; Foss, S.; Korshunov, D. Tail asymptotics for the supremum of random walk when the mean is not finite. Queuing Syst. 2004, 46, 15–33. [Google Scholar] [CrossRef]
- Embrechts, P.; Klüppelberg, C.; Mikosch, T. Modelling Extremal Events for Insurance and Finance; Springer: New York, NY, USA, 1997. [Google Scholar]
- Embrechts, P.; Veraverbeke, N. Estimates for the probability of ruin with special emphasis and the possibility of large claims. Insur. Math. Econ. 1982, 1, 55–72. [Google Scholar] [CrossRef]
- Klüppelberg, C. Subexponential distributions and integrated tails. J. Appl. Probab. 1988, 25, 132–141. [Google Scholar] [CrossRef]
- Bingham, N.H.; Goldie, C.M.; Teugels, J.L. Regular Variation; Cambridge University Press: Cambridge, UK, 1987. [Google Scholar]
- Borovkov, A.A.; Borovkov, K.A. Asymptotic Analysis of Random Walks: Heavy-Tailed Distributions; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Foss, S.; Korshunov, D.; Zachary, S. An Introduction to Heavy-Tailed and Subexponential Distributions, 2nd ed.; Springer: New York, NY, USA, 2013. [Google Scholar]
- Konstantinides, D.G. Risk Theory: A Heavy Tail Approach; World Scientific: Hackensack, NJ, USA, 2018. [Google Scholar]
- Leipus, R.; Šiaulys, J.; Konstantinides, D. Closure Properties for Heavy-Tailed and Related Distributions: An Overview; Springer: Cham, Switzerland, 2023. [Google Scholar]
- Nair, J.; Wierman, A.; Zwart, B. The Fundamentals of Heavy Tails: Properties, Emergence, and Estimation; Cambridge University Press: Cambridge, UK, 2022. [Google Scholar]
- Liu, Y. A general treatement of alternative expectation formulae. Stat. Probab. Lett. 2020, 166, 108863. [Google Scholar] [CrossRef]
- Hall, P. The distribution of means for samples of sizes N drawn from a population in which the variate takes values between 0 and 1, all such values being equally probable. Biometrika 1927, 19, 240–245. [Google Scholar] [CrossRef]
- Irwin, J.O. On the frequency distribution of the means of samples from a population having any law of frequency with finite moments, with special reference to Pearson’s type II. Biometrika 1927, 19, 225–239. [Google Scholar] [CrossRef]
- Johnson, N.L.; Kotz, S.; Balakrishnan, N. Continuous Univariate Distributions, 2nd ed.; Wiley: New York, NY, USA, 1995; Volume 2. [Google Scholar]
- Tadikamalla; Pandu, R. A look at the Burr and related distributions. Int. Stat. Rev. 1980, 48, 337–344. [Google Scholar] [CrossRef]
- Chen, H.; Fan, K. Tail Value-at-Risk based profiles for extreme risks and their application in distributionally robust portfolio selections. Mathematics 2023, 11, 91. [Google Scholar] [CrossRef]
- Mehta, M.J.; Yang, F. Portfolio optimization for extreme risks with maximum diversification: An empirical analysis. Risks 2022, 10, 101. [Google Scholar] [CrossRef]
- Sepanski, J.H.; Wang, X. New classes of distortion risk measures and their estimation. Risks 2023, 11, 194. [Google Scholar] [CrossRef]
- Mahdavi, A.; Kharazm, O.; Contreras-Reyes, J.E. On the contaminated weighted exponential distribution: Applications to modelling insurance claim data. J. Risk Financ. Manag. 2022, 15, 500. [Google Scholar] [CrossRef]
- Olmos, N.M.; Gómez-Déniz, F.; Venegas, O. The heavy-tailed Gleser model: Properties, estimation, and applications. Mathematics 2022, 10, 4577. [Google Scholar] [CrossRef]
- Grün, B.; Miljkovic, T. Extending composite loss models using a general framework of advanced computational tools. Scand. Actuar. J. 2019, 2019, 642–660. [Google Scholar] [CrossRef]
- Marambakuyana, W.A.; Shongwe, S.C. Composite and mixture distributions for heavy-tailed data—An application to insurance claims. Mathematics 2024, 12, 335. [Google Scholar] [CrossRef]
- Klebanov, L.B.; Kuvaeva-Gudoshnikova, Y.V.; Rachev, S.T. Heavy-tailed probability distributions: Some examples of their appearance. Mathematics 2023, 11, 3094. [Google Scholar] [CrossRef]
- Santoro, K.I.; Gallardo, D.I.; Venegas, O.; Cortés, I.E.; Gómes, H.W. A heavy-tailed distribution based on the Lomax-Reyleigh distribution with application to medical data. Mathematics 2023, 11, 4626. [Google Scholar] [CrossRef]
- Markovich, N.; Vaičiulis, M. Extreme value statistics for envolving random networks. Mathematics 2023, 11, 2171. [Google Scholar] [CrossRef]
- Rusev, V.; Skorikov, A. The asymptotics of moments for the remaining time of heavy-tail distributions. Comput. Sci. Math. Forum 2023, 7, 52. [Google Scholar] [CrossRef]
- Sousa-Vieira, M.E.; Fernández-Veiga, M. Study of coded ALOHA with multi-user detection under heavy-tailed and correlated arivals. Future Internet 2023, 15, 132. [Google Scholar] [CrossRef]
- Klüppelberg, C.; Mikosch, T. Large deviations of heavy-tailed random sums with applications in insurance and finance. J. Appl. Probab. 1997, 34, 293–308. [Google Scholar] [CrossRef]
- Aleškevičienė, A.; Leipus, R.; Šiaulys, J. Tail behavior of random sums under consistent variation with applications to the compound renewal risk model. Extremes 2008, 11, 261–279. [Google Scholar] [CrossRef]
- Olvera-Cravioto, M. Asymptotics for weighted random sums. Adv. Appl. Probab. 2012, 44, 1142–1172. [Google Scholar] [CrossRef]
- Volkovich, Y.; Litvak, N. Asymptotic analysis for personalized Web search. Adv. Appl. Probab. 2010, 42, 577–604. [Google Scholar] [CrossRef]
- Faÿ, G.; González-Arévalo, B.; Mikosch, T.; Samorodnitsky, G. Modeling teletraffic arrivals by a Poisson cluster process. Queueing Syst. 2006, 54, 121–140. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leipus, R.; Šiaulys, J.; Danilenko, S.; Karasevičienė, J. Randomly Stopped Sums, Minima and Maxima for Heavy-Tailed and Light-Tailed Distributions. Axioms 2024, 13, 355. https://doi.org/10.3390/axioms13060355
Leipus R, Šiaulys J, Danilenko S, Karasevičienė J. Randomly Stopped Sums, Minima and Maxima for Heavy-Tailed and Light-Tailed Distributions. Axioms. 2024; 13(6):355. https://doi.org/10.3390/axioms13060355
Chicago/Turabian StyleLeipus, Remigijus, Jonas Šiaulys, Svetlana Danilenko, and Jūratė Karasevičienė. 2024. "Randomly Stopped Sums, Minima and Maxima for Heavy-Tailed and Light-Tailed Distributions" Axioms 13, no. 6: 355. https://doi.org/10.3390/axioms13060355
APA StyleLeipus, R., Šiaulys, J., Danilenko, S., & Karasevičienė, J. (2024). Randomly Stopped Sums, Minima and Maxima for Heavy-Tailed and Light-Tailed Distributions. Axioms, 13(6), 355. https://doi.org/10.3390/axioms13060355





