The Split Equality Fixed-Point Problem and Its Applications
Abstract
1. Introduction
2. Preliminaries
- a.
- b.
- is demiclosed at zero only if is demiclosed at zero.
- c.
- is Lipschitz with
- d.
- is quasi-nonexpansive only if is quasi-pseudocontractive.
- a.
- , exist;
- b.
- For any weak cluster point of then
- a.
- b.
- then,
3. Main Results
- (A1)
- are two quasi-pseudocontractive operators with in addition, suppose is L-Lipschitz.
- (A2)
- are linear and bounded operators with their adjoints and respectively.
- (A3)
- are demiclosed at origin.
- (A4)
- Let and be defined below:where
- (A5)
- Algorithm:Let ( be defined bywhere are chosen arbitrarily, and where and , respectively,
- (i)
- exist, for all
- (ii)
- belong to
4. The SEFPP without Prior Knowledge of Operator Norms
- (i)
- (ii)
- such that and
5. Applications
5.1. Application to the SFP
5.2. Application to the Split Variational Inequality Problem (SVIP)
- (B1)
- (B2)
- and as in Theorem 1.
- (B3)
- and are demiclosed at zero.
- (B4)
- Let and be defined as follows:where
- (B5)
- Algorithm:Let be defined bywhere are chosen arbitrarily, and with and respectively.
5.3. Application to the Split Convex Minimization Problem (SCMP)
- (C1)
- and be defined as above;
- (C2)
- and as in Theorem 1;
- (C3)
- and are demiclosed at zero;
- (C4)
- Let and be defined aswhere
- (C5)
- Algorithm: Let be defined aswhere and are chosen arbitrarily, and where and respectively.
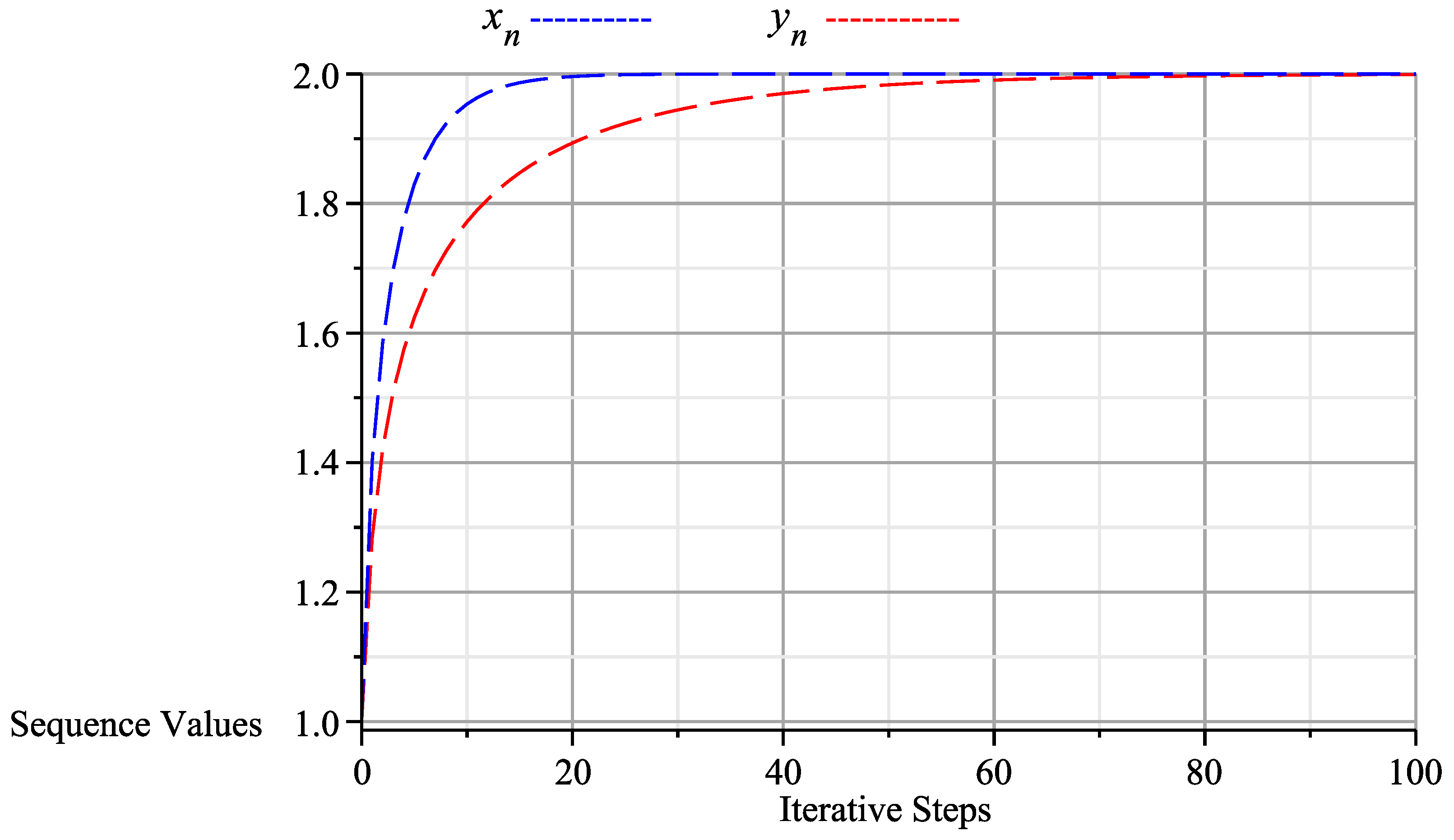
6. Numerical Examples
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chang, S.-S.; Yao, J.-C.; Wen, C.-F.; Zhao, L.-C. On the split equality fixed point problem of quasi-pseudo-contractive mappings without a priori knowledge of operator norms with applications. J. Optim. Theory Appl. 2020, 185, 343–360. [Google Scholar] [CrossRef]
- Censor, Y.; Elfving, T. A multiprojection algorithm using Bregman projections in a product space. Numer. Algorithms 1994, 8, 221–239. [Google Scholar] [CrossRef]
- Censor, Y.; Bortfeld, T.; Martin, B.; Trofimov, A. A unified approach for inversion problems in intensity-modulated radiation therapy. Phys. Med. Biol. 2006, 51, 2353–2365. [Google Scholar] [CrossRef] [PubMed]
- Censor, Y.; Elfving, T.; Kopf, N.; Bortfeld, T. The multiple-sets split feasibility problem and its applications for inverse problems. Inverse Probl. 2005, 21, 2071–2084. [Google Scholar] [CrossRef]
- Censor, Y.; Motova, A.; Segal, A. Perturbed projections and subgradient projections for the multiple-sets split feasibility problem. J. Math. Anal. Appl. 2007, 327, 1244–1256. [Google Scholar] [CrossRef]
- Byrne, C. Iterative oblique projection onto convex subsets and the split feasibility problem. Inverse Probl. 2002, 18, 441–453. [Google Scholar] [CrossRef]
- Moudafi, A.; Al-Shemas, E. Simultaneous iterative methods for split equality problem. Trans. Math. Program. Appl. 2013, 1, 1–10. [Google Scholar]
- Moudafi, A. Alternating CQ-algorithm for convex feasibility and split fixed-point problems. J. Nonlinear Convex Anal. 2014, 15, 809–818. [Google Scholar]
- Mohammed, L.B.; Kılıçman, A. Strong Convergence for the Split Common Fixed-Point Problem for Total Quasi-Asymptotically Nonexpansive Mappings in Hilbert Space. Abstr. Appl. Anal. 2015, 2015, 1–7. [Google Scholar] [CrossRef]
- Mohammed, L.; Kılıçman, A.; Saje, A.U. On split equality fixed-point problems. Alex. Eng. J. 2023, 66, 43–51. [Google Scholar] [CrossRef]
- Khan, A.R. Iterative Methods for Nonexpansive Type Mappings. In Fixed Point Theory and Graph Theory; Academic Press: Cambridge, MA, USA, 2016; pp. 231–285. [Google Scholar]
- Antón-Sancho, Á. Fixed points of automorphisms of the vector bundle moduli space over a compact Riemann surface. Mediterr. J. Math. 2024, 21, 1–20. [Google Scholar] [CrossRef]
- Che, H.; Li, M. A simultaneous iterative method for split equality problems of two finite families of strictly pseudononspreading mappings without prior knowledge of operator norms. Fixed Point Theory Appl. 2015, 2015, 1. [Google Scholar] [CrossRef]
- Chang, S.-S.; Wang, L.; Qin, L.-J. Split equality fixed point problem for quasi-pseudo-contractive mappings with applications. Fixed Point Theory Appl. 2015, 2015, 208. [Google Scholar] [CrossRef]
- Opial, Z. Weak convergence of the sequence of successive approximations for nonexpansive mappings. Bull. Am. Math. Soc. 1967, 73, 591–597. [Google Scholar] [CrossRef]
- Xu, H.-K. Iterative Algorithms for Nonlinear Operators. J. Lond. Math. Soc. 2002, 66, 240–256. [Google Scholar] [CrossRef]
- Censor, Y.; Gibali, A.; Reich, S. Algorithms for the Split Variational Inequality Problem. Numer. Algorithms 2012, 59, 301–323. [Google Scholar] [CrossRef]
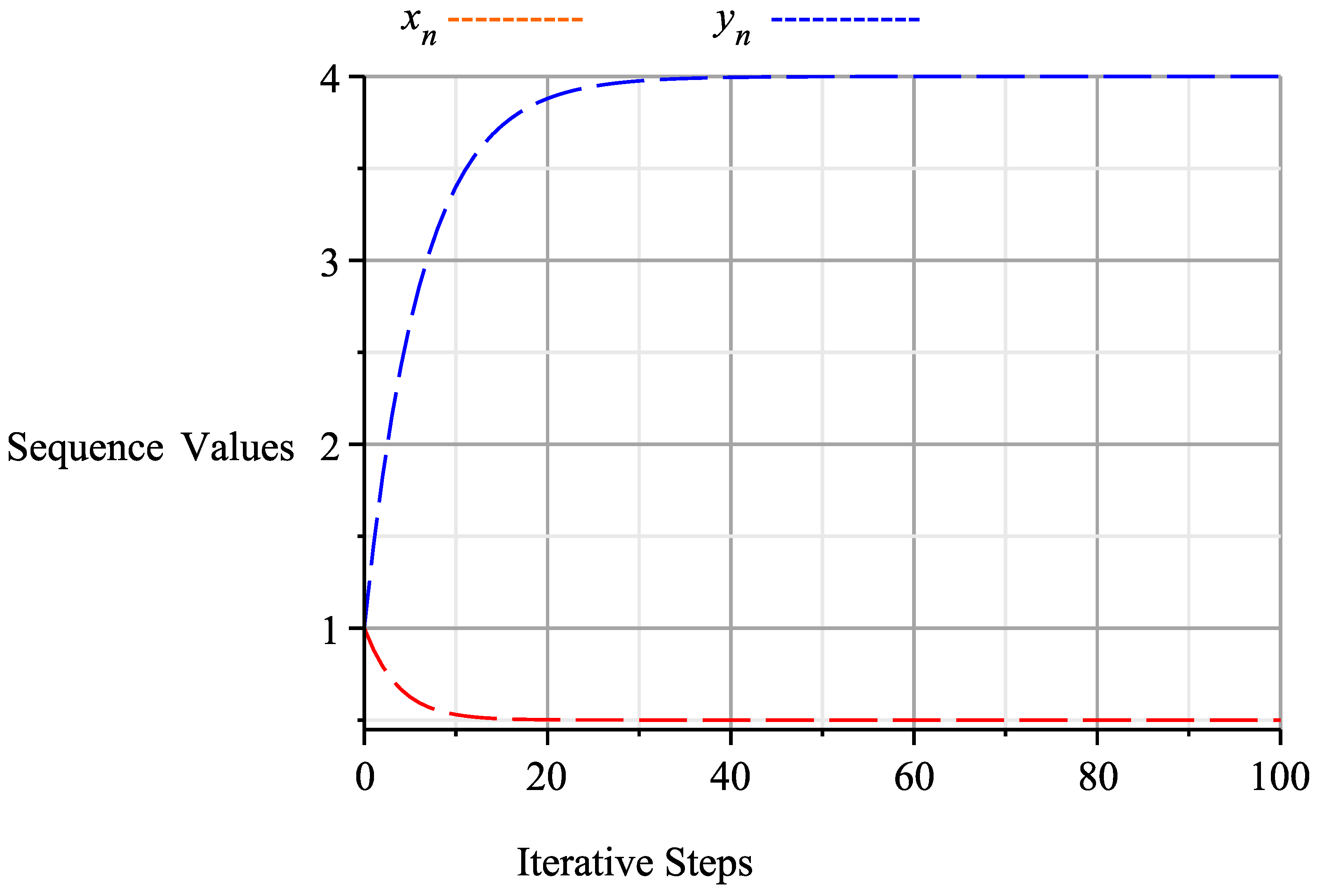
| n | ||
|---|---|---|
| 1 | 1.000000000 | 1.000000000 |
| 2 | 1.446428571 | 0.8831268924 |
| 3 | 1.826424319 | 0.7916797163 |
| . | . | . |
| . | . | . |
| 73 | . | 0.5000000002 |
| 74 | . | 0.5000000000 |
| . | . | . |
| 98 | 3.999999582 | 0.5000000000 |
| 99 | 3.999999644 | 0.5000000000 |
| 100 | 3.999999697 | 0.5000000000 |
| n | ||
|---|---|---|
| 1 | 1.000000000 | 1.000000000 |
| 2 | 1.402141502 | 1.283490816 |
| 3 | 1.584779961 | 1.420942750 |
| . | . | . |
| . | . | . |
| . | . | . |
| 80 | 1.999999998 | . |
| 81 | 1.999999999 | . |
| . | . | . |
| 98 | 1.999999999 | 1.998915702 |
| 99 | 1.999999999 | 1.998975310 |
| 100 | 1.999999999 | 1.999031634 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohammed, L.B.; Kilicman, A. The Split Equality Fixed-Point Problem and Its Applications. Axioms 2024, 13, 460. https://doi.org/10.3390/axioms13070460
Mohammed LB, Kilicman A. The Split Equality Fixed-Point Problem and Its Applications. Axioms. 2024; 13(7):460. https://doi.org/10.3390/axioms13070460
Chicago/Turabian StyleMohammed, Lawan Bulama, and Adem Kilicman. 2024. "The Split Equality Fixed-Point Problem and Its Applications" Axioms 13, no. 7: 460. https://doi.org/10.3390/axioms13070460
APA StyleMohammed, L. B., & Kilicman, A. (2024). The Split Equality Fixed-Point Problem and Its Applications. Axioms, 13(7), 460. https://doi.org/10.3390/axioms13070460







