Effect of Blade Thickness on Internal Flow and Performance of a Plastic Centrifugal Pump
Abstract
:1. Introduction
2. Geometry and Parameters
3. Numerical Methods
3.1. Governing Equations
3.2. Numerical Setup and Mesh Generation
4. Test Setup
5. Results and Discussions
5.1. Flow Field Analysis of CTB
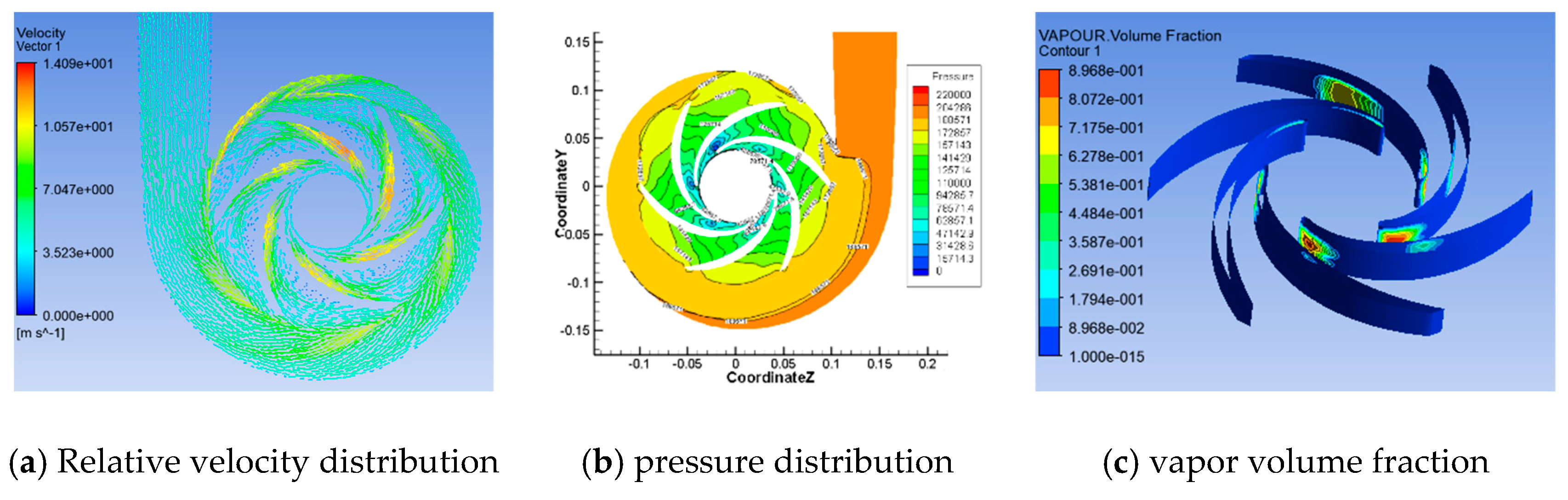
5.1.1. Discussion of the CTB’s Pressure Distribution
5.1.2. Discussion of the CTB’s Velocity Distribution
5.1.3. Discussion of the CTB’s Vapor Volume Fraction
5.1.4. Effects of CTB on Pump Performance
5.2. Discussion of the Minimum Blade Thickness
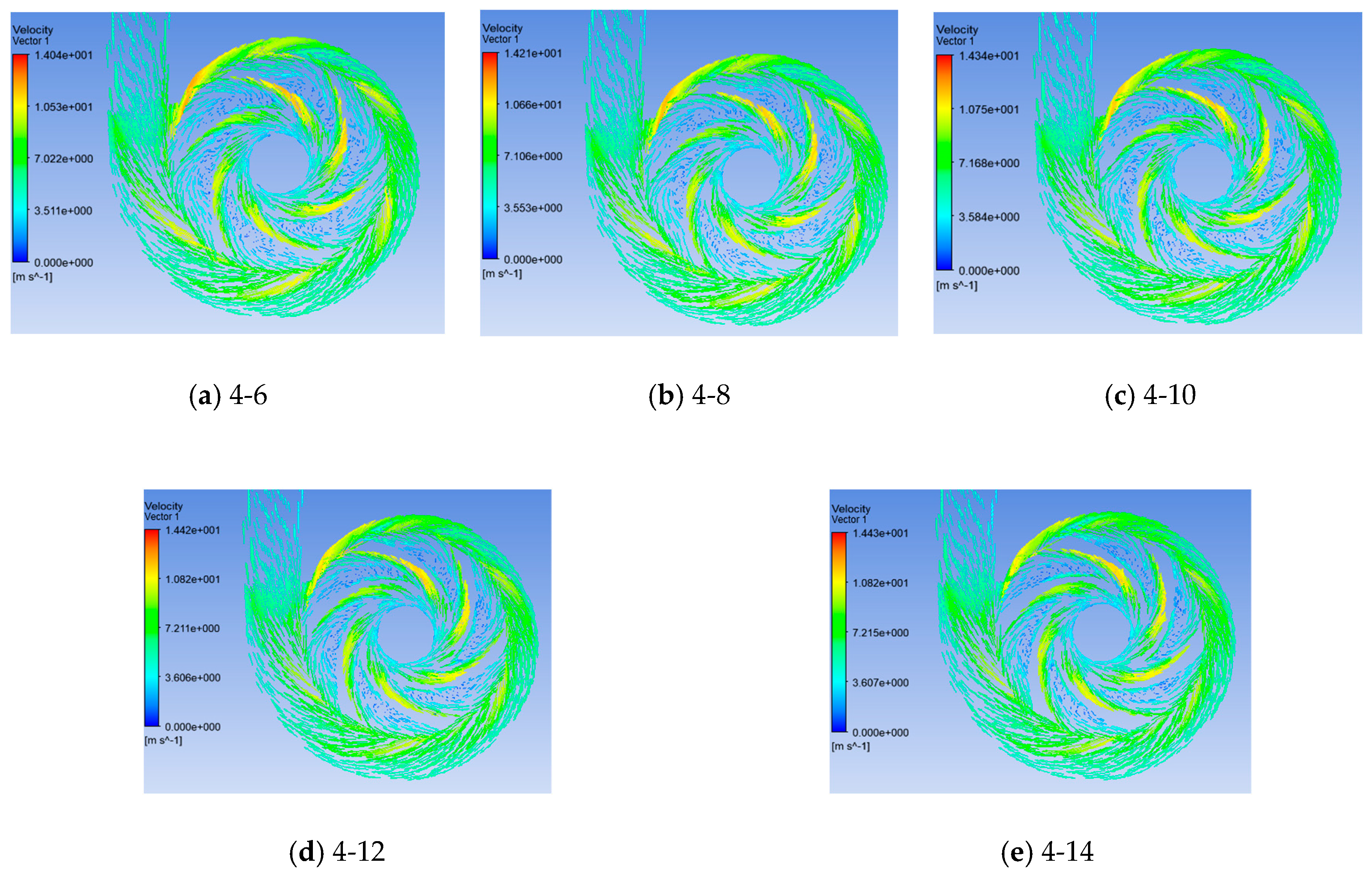
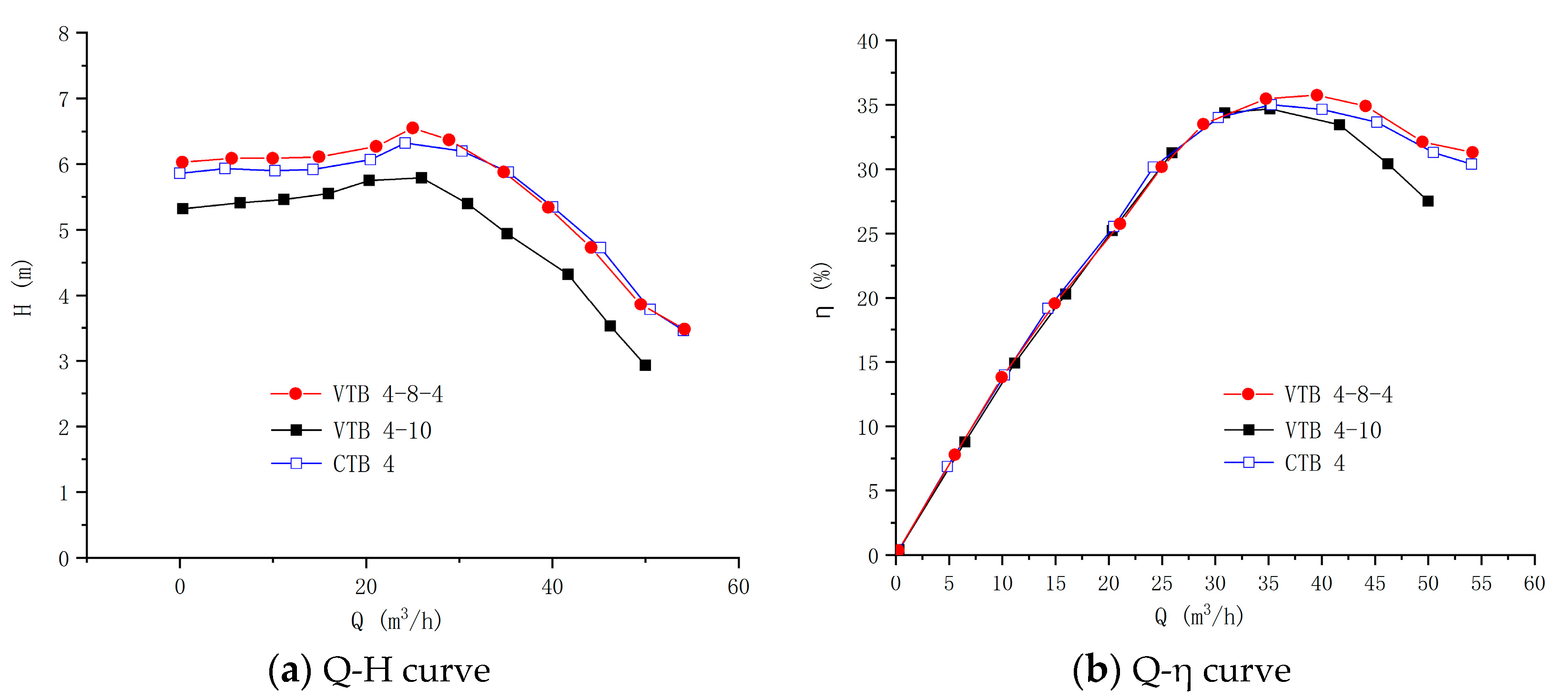
5.3. Effects of VTB on Pump Performance
5.4. Optimization of the Blade Profile
5.5. Test
6. Conclusions
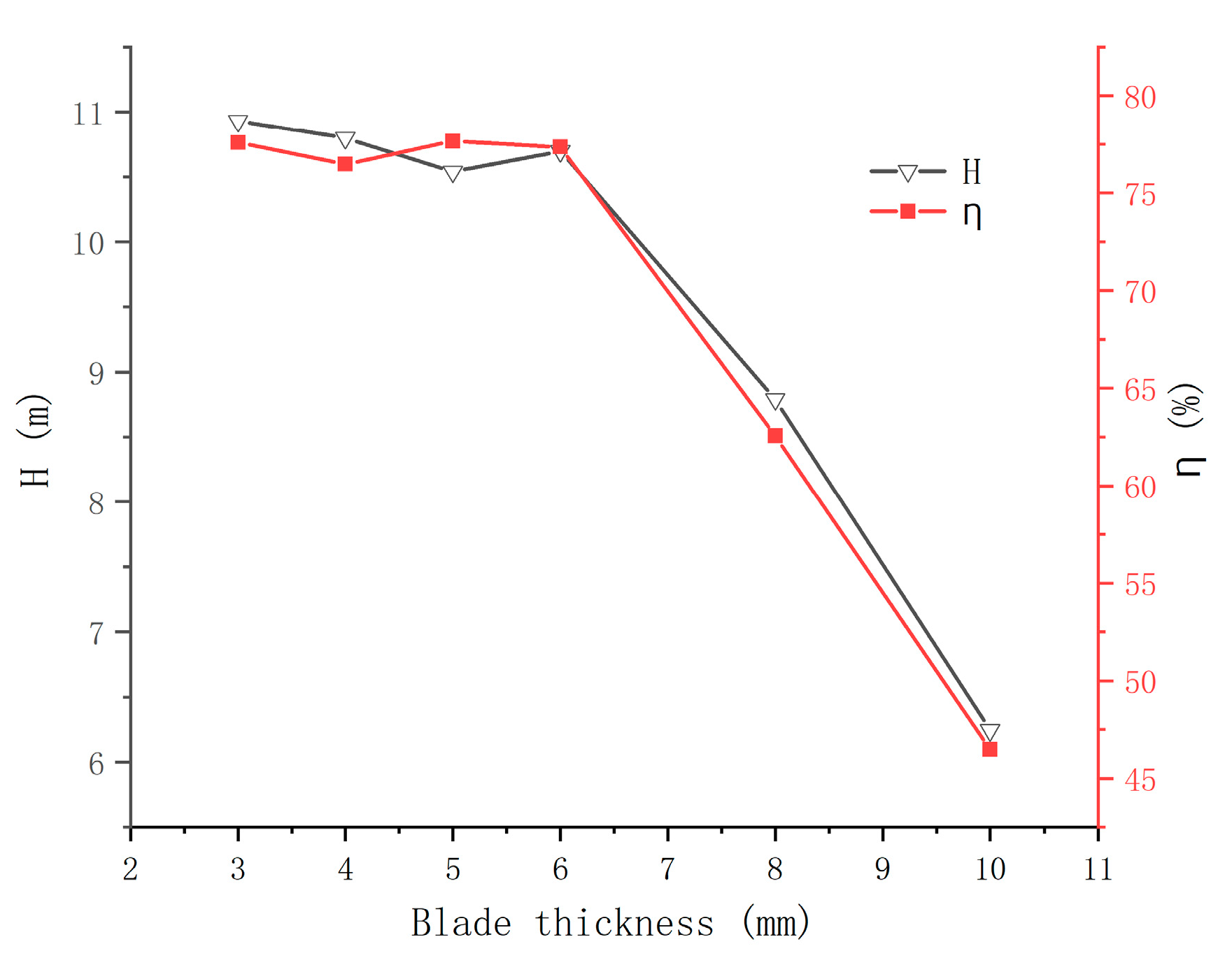
- The blade thickness had a significant impact on the performance of the plastic pump. For CTB, the pump performance changed a little when the thickness was 3 mm to 5 mm. When the blade thickness exceeded 6mm, the performance parameters of the pump decreased rapidly. The head and efficiency CTB 10 decreased by 42.2% and 30%, respectively, compared with CTB 4.
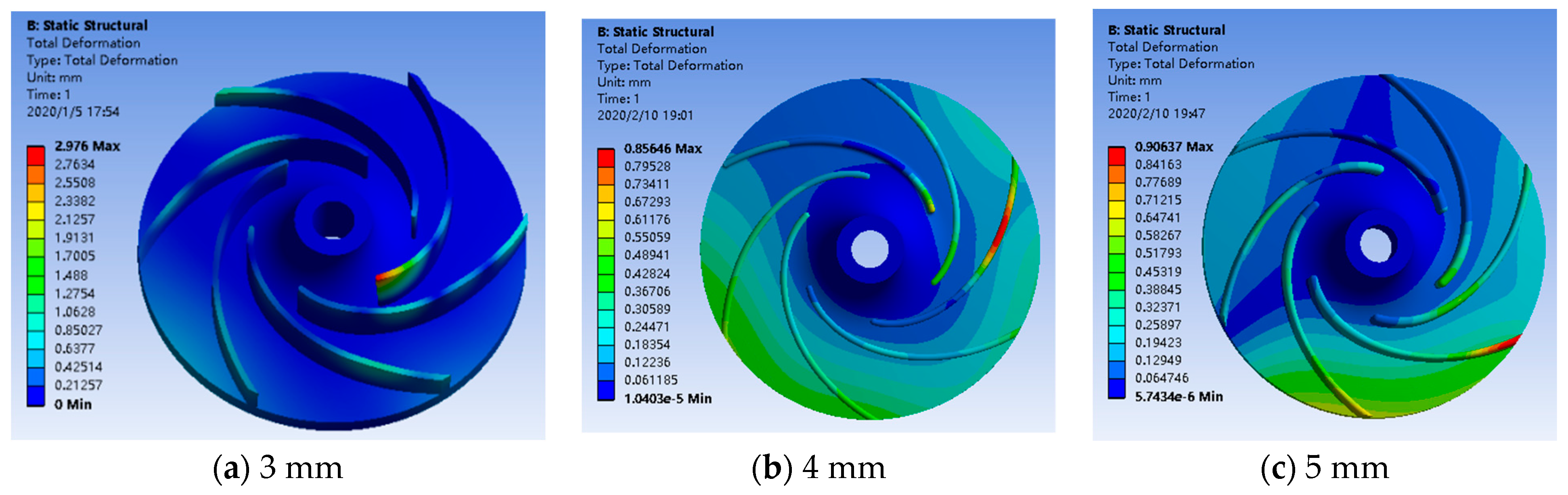
- Based on the finite element analysis, the minimum blade thickness of the researched pump should be 4 mm in order to meet the requirement of blade strength.
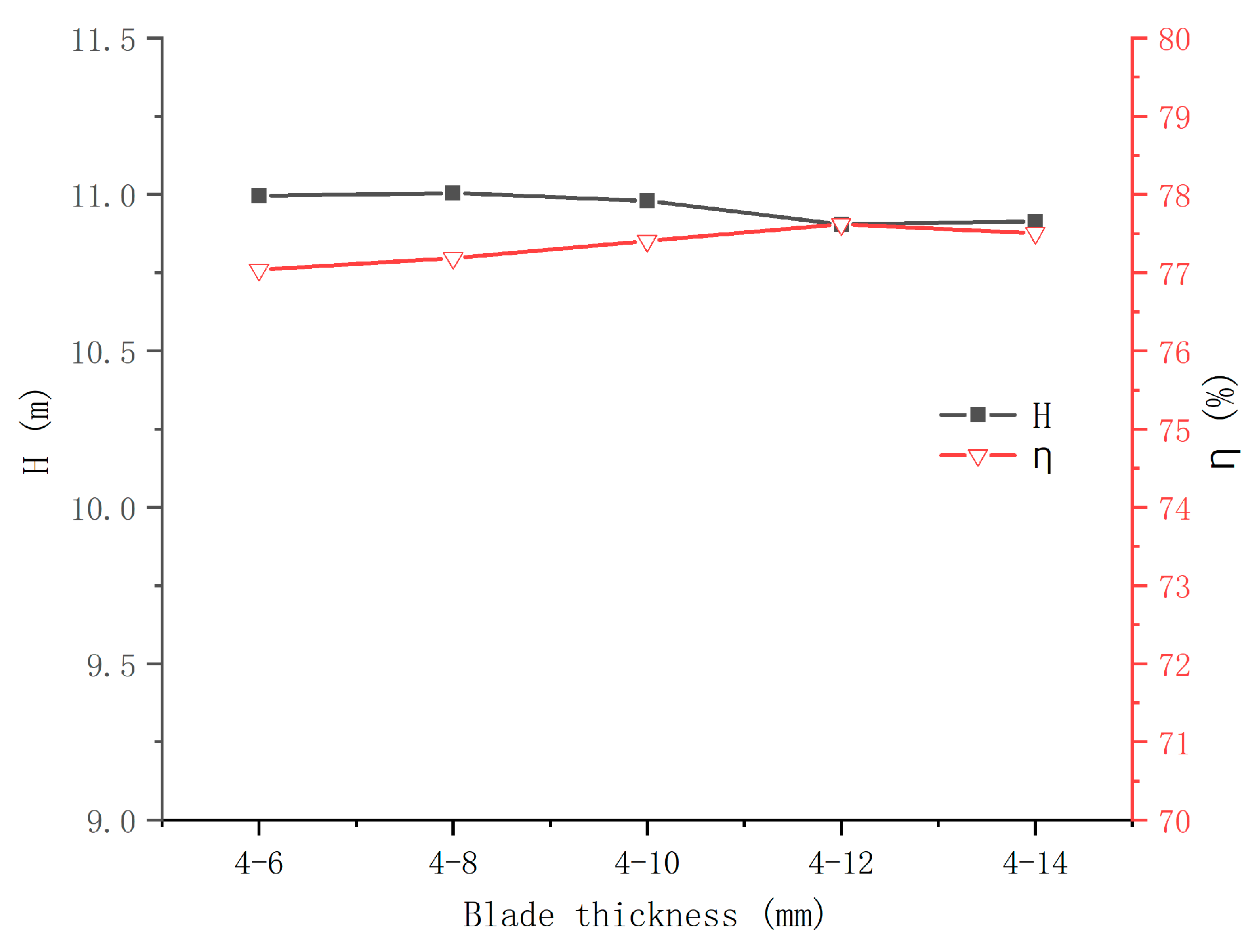
- The head and efficiency of the pump did not change significantly, when the trailing edge thickness of the VTB changed within a certain range (6 mm to 14 mm).
- The thickness distribution of VTB was optimized, and the maximum thickness was changed from the trailing edge to 60% chord length. The efficiency of the optimized impeller VTB 4-8-4 was 1.67% higher than that of CTB 4.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, J.; Tang, L.; Zhang, Y. The Influence of Blade Angle on the Performance of Plastic Centrifugal Pump. Adv. Mater. Sci. Eng. 2020, 6, 1–20. [Google Scholar] [CrossRef]
- Tang, L.; Liu, M.; Ma, F. Thermosetting Coupling Analysis and Parameter Optimization of the Plastic Lining Pump Structure. Adv. Mater. Sci. Eng. 2019, 6, 1–15. [Google Scholar] [CrossRef] [Green Version]
- Guan, X. Modern Pumps Theory and Design; Astronautic Publishing House: Beijing, China, 2011. [Google Scholar]
- Luo, X.; Zhang, Y.; Peng, J.; Xu, H.; Yu, W. Impeller inlet geometry effect on performance improvement for centrifugal pumps. J. Mech. Sci. Technol. 2008, 22, 1971–1976. [Google Scholar] [CrossRef]
- Tan, L.; Zhu, B.; Cao, S.; Bing, H.; Wang, Y. Influence of Blade Wrap Angle on Centrifugal Pump Performance by Numerical and Experimental Study. Chin. J. Mech. Eng. 2014, 27, 171–177. [Google Scholar] [CrossRef]
- Zhang, H.; Tang, L.; Zhao, Y. Influence of Blade Profiles on Plastic Centrifugal Pump Performance. Adv. Mater. Sci. Eng. 2020, 2020, 6665520. [Google Scholar] [CrossRef]
- Suh, J.W.; Kim, J.W.; Choi, Y.S.; Kim, J.H.; Joo, W.G.; Lee, K.Y. Multi-Objective Optimization of the Hydrodynamic Performance of the Second Stage of a Multi-Phase Pump. Energies 2017, 10, 1334. [Google Scholar] [CrossRef] [Green Version]
- Wang, W.; Pei, J.; Yuan, S.; Zhang, J.; Yuan, J.; Xu, C. Application of different surrogate models on the optimization of centrifugal pump. J. Mech. Sci. Technol. 2016, 30, 567–574. [Google Scholar] [CrossRef]
- Liu, H.; Kai, W.; Yuan, S.; Tan, M.; Liang, D. Multicondition Optimization and Experimental Measurements of a Double-Blade Centrifugal Pump Impeller. J. Fluids Eng. 2013, 135, 111031. [Google Scholar] [CrossRef] [Green Version]
- Kumar, P.M.; Seo, J.; Seok, W.; Rhee, S.H.; Samad, A. Multi-fidelity optimization of blade thickness parameters for a horizontal axis tidal stream turbine. Renew. Energy 2019, 135, 277–287. [Google Scholar] [CrossRef]
- Sjka, B.; Ysca, B.; Yong, C.C.; Jwc, C.; Jhka, B. Effect of blade thickness on the hydraulic performance of a Francis hydro turbine model. Renew. Energy 2019, 134, 807–817. [Google Scholar]
- Yang, S.S.; Wang, C.; Chen, K.; Yuan, X. Research on Blade Thickness Influencing Pump as Turbine. Adv. Mech. Eng. 2014, 6, 1–8. [Google Scholar] [CrossRef]
- Qiu, N.; Zhou, W.; Che, B.; Wu, D.; Zhu, H. Effects of microvortex generators on cavitation erosion by changing periodic shedding into new structures. Phys. Fluids. 2020, 32, 104108. [Google Scholar] [CrossRef]
- Chang, H.; Li, W.; Shi, W.; Liu, J. Effect of blade profile with different thickness distribution on the pressure characteristics of novel self-priming pump. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 518. [Google Scholar] [CrossRef]
- Kim, Y.I.; Kim, S.; Yang, H.; Lee, K.Y.; Choi, Y.S. Analysis of internal flow and cavitation characteristics for a mixed-flow pump with various blade thickness effects. J. Mech. Sci. Technol. 2019, 33, 3333–3344. [Google Scholar] [CrossRef]
- Nazeryan, M.; Lakzian, E. Detailed entropy generation analysis of a Wells turbine using the variation of the blade thickness. Energy 2017, 143, 385–405. [Google Scholar] [CrossRef]
- Ma, C.; Li, W.; Wang, H.; Chen, H. Influence of Thickness Distribution of Diffuser Vane on Performance of Centrifugal Compressor. J. Eng. Therm. Energy Power 2018, 33, 52–57. [Google Scholar]
- Wang, C.; Shi, W.; Zhou, L.; Lu, W. Effect Analysis of Geometric Parameters on Stainless Steel Stamping Multistage Pump by Experimental Test and Numerical Calculation. Adv. Mech. Eng. 2015, 5, 1–8. [Google Scholar] [CrossRef]
- Ha, Y.; Hou, L. Effects of blade thickness on performance of axial flow pump and analysis of internal flow field. Trans. Chin. Soc. Agric. Eng. 2012, 28, 75–81. [Google Scholar]
- Yang, M.G.; Sheng, L.U.; Gao, B.; Wang, D.; Wang, J. Influence of Blade Thickness on Energy Performance of Mixed Flow Nuclear Main Pump. Fluid Mach. 2015, 43, 28–32. [Google Scholar]
- Zhao, W.; Liu, M.; Liu, Y.; Sheng, J.; Song, Q. Effects of blade thickness on performance of low-specific-speed centrifugal pump. J. Drain. Irrig. Mach. Eng. 2015, 33, 1033–1037. [Google Scholar]
- Qian, B.; Wu, P.; Huang, B.; Zhang, K.; Wu, D. Optimization of a Centrifugal Impeller on Blade Thickness Distribution to Reduce Hydro-induced Vibration. J. Fluids Eng. 2019, 142, 021202. [Google Scholar] [CrossRef]
- Tao, Y.; Yuan, S.; Liu, J.; Zhang, F.; Tao, J. The influence of the blade thickness on the pressure pulsations in a ceramic centrifugal slurry pump with annular volute. Proc. Inst. Mech. Eng. Part A J. Power Energy 2017, 231, 415–431. [Google Scholar] [CrossRef]
- Tao, Y.; Bai, Y.; Wu, Y. Influence of Blade Thickness on Solid–Liquid Two-Phase Flow and Impeller Wear in a Ceramic Centrifugal Slurry Pump. Processes 2021, 9, 1259. [Google Scholar] [CrossRef]
- Tao, Y.; Yuan, S.; Liu, J.; Zhang, F.; Tao, J. Influence of Blade Thickness on Transient Flow Characteristics of Centrifugal Slurry Pump with Semi-open Impeller. Chin. J. Mech. Eng. 2016, 29, 1209–1217. [Google Scholar] [CrossRef]
- Xu, Z.; Kong, F.; Zhang, H.; Zhang, K.; Wang, J.; Qiu, N. Research on Visualization of Inducer Cavitation of High-Speed Centrifugal Pump in Low Flow Conditions. J. Mar. Sci. Eng. 2021, 9, 1240. [Google Scholar] [CrossRef]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics; Addison-Wesley-Longman: Boston, MA, USA, 1995. [Google Scholar]
- Zhang, D.; Shi, W.; Pan, D.; Dubuisson, M. Numerical and experimental investigation of tip leakage vortex cavitation patterns and mechanisms in an axial flow pump. J. Fluids Eng. 2015, 137, 121103. [Google Scholar] [CrossRef]
- Al-Obaidi, A.R. Investigation of the influence of various numbers of impeller blades on internal flow field analysis and the pressure pulsation of an axial pump based on transient flow behavior. Heat Transfer. 2020, 49, 2000–2024. [Google Scholar] [CrossRef]
- Strait, D.S.; Qian, W.; Dechow, P.C.; Ross, C.F.; Richmond, B.G.; Spencer, M.A. Modeling elastic properties in finite-element analysis: How much precision is needed to produce an accurate model? Anat. Rec. Part A Discov. Mol. Cell. Evol. Biol. 2010, 283A, 275–287. [Google Scholar] [CrossRef]
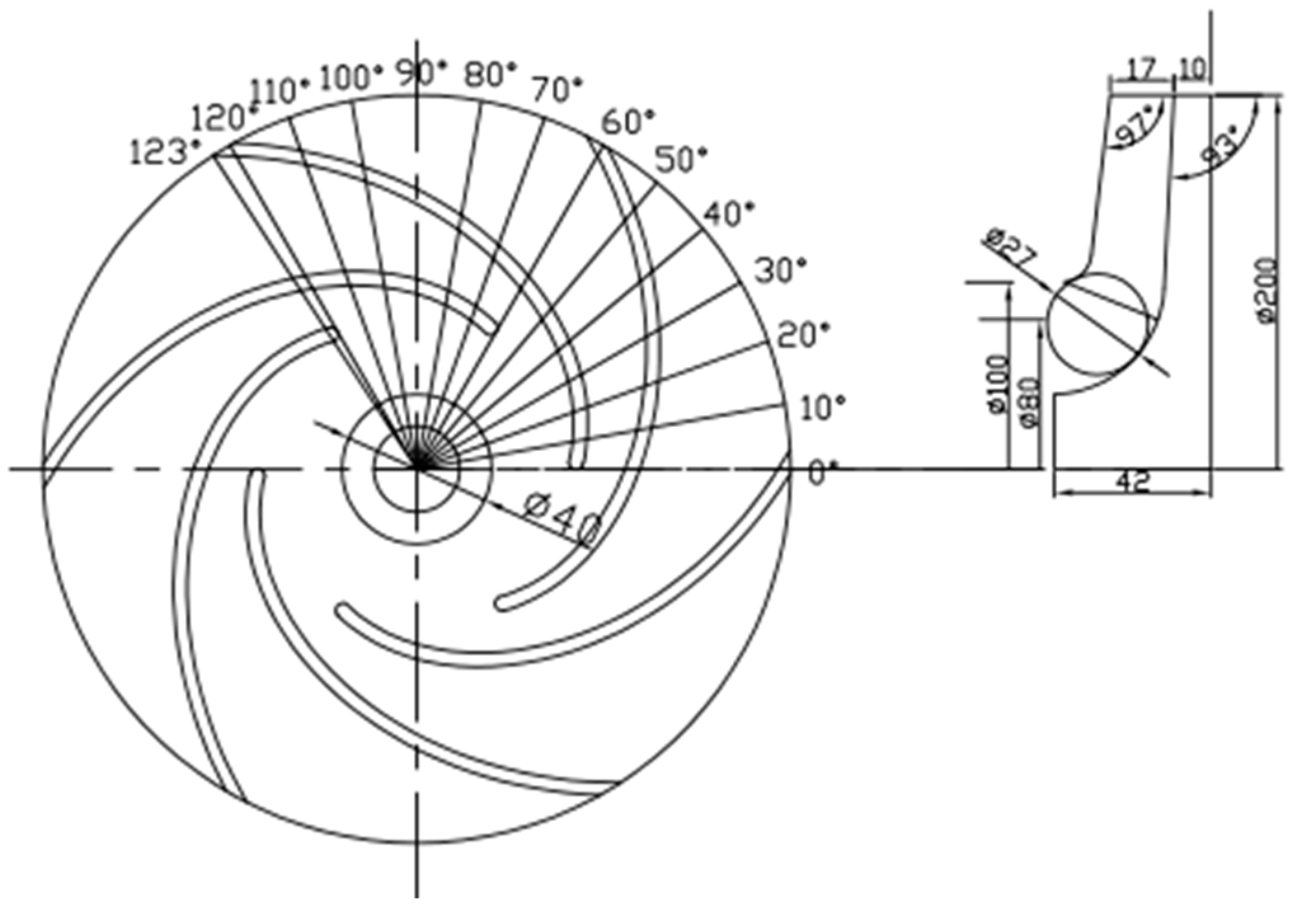
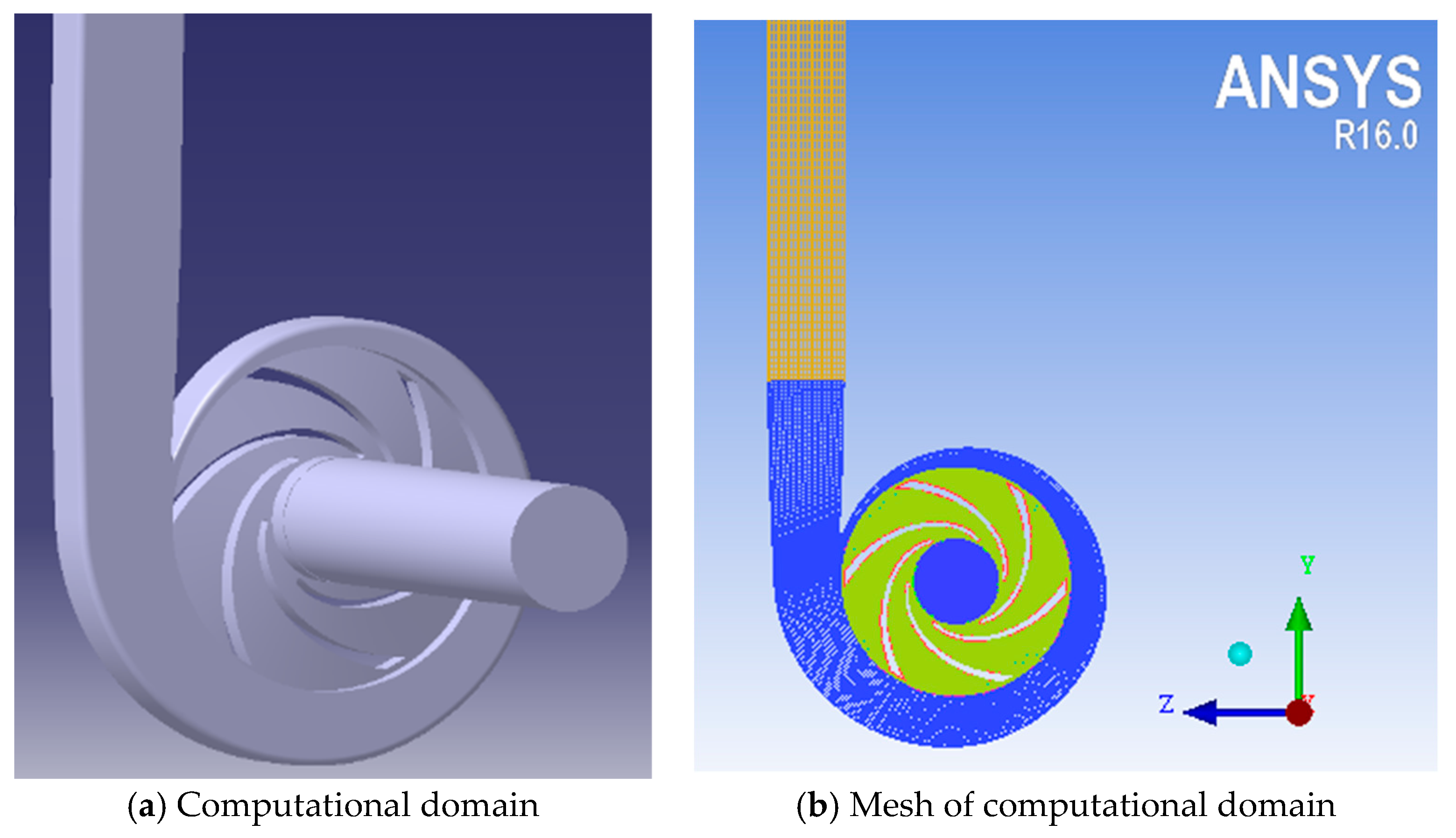

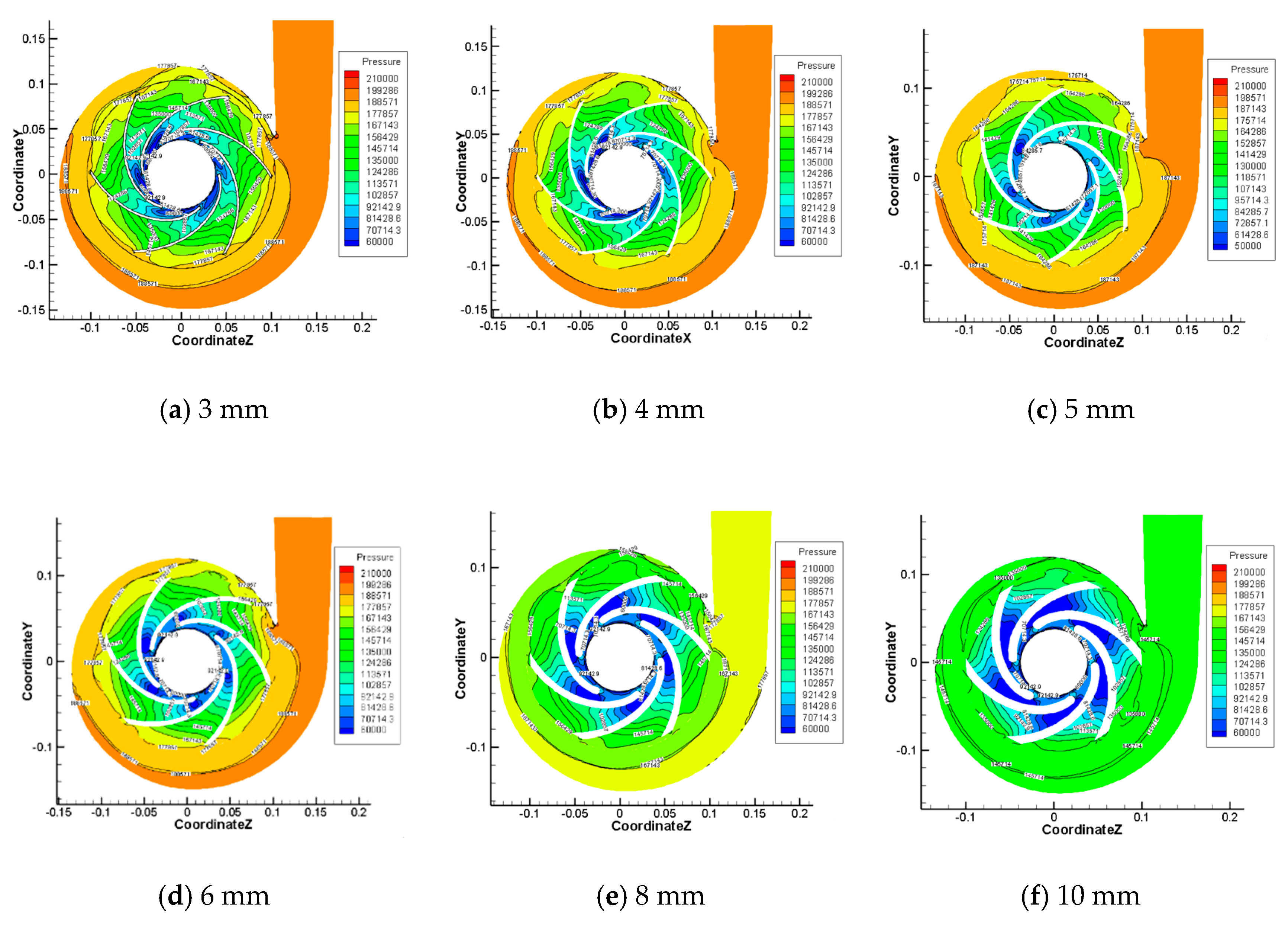
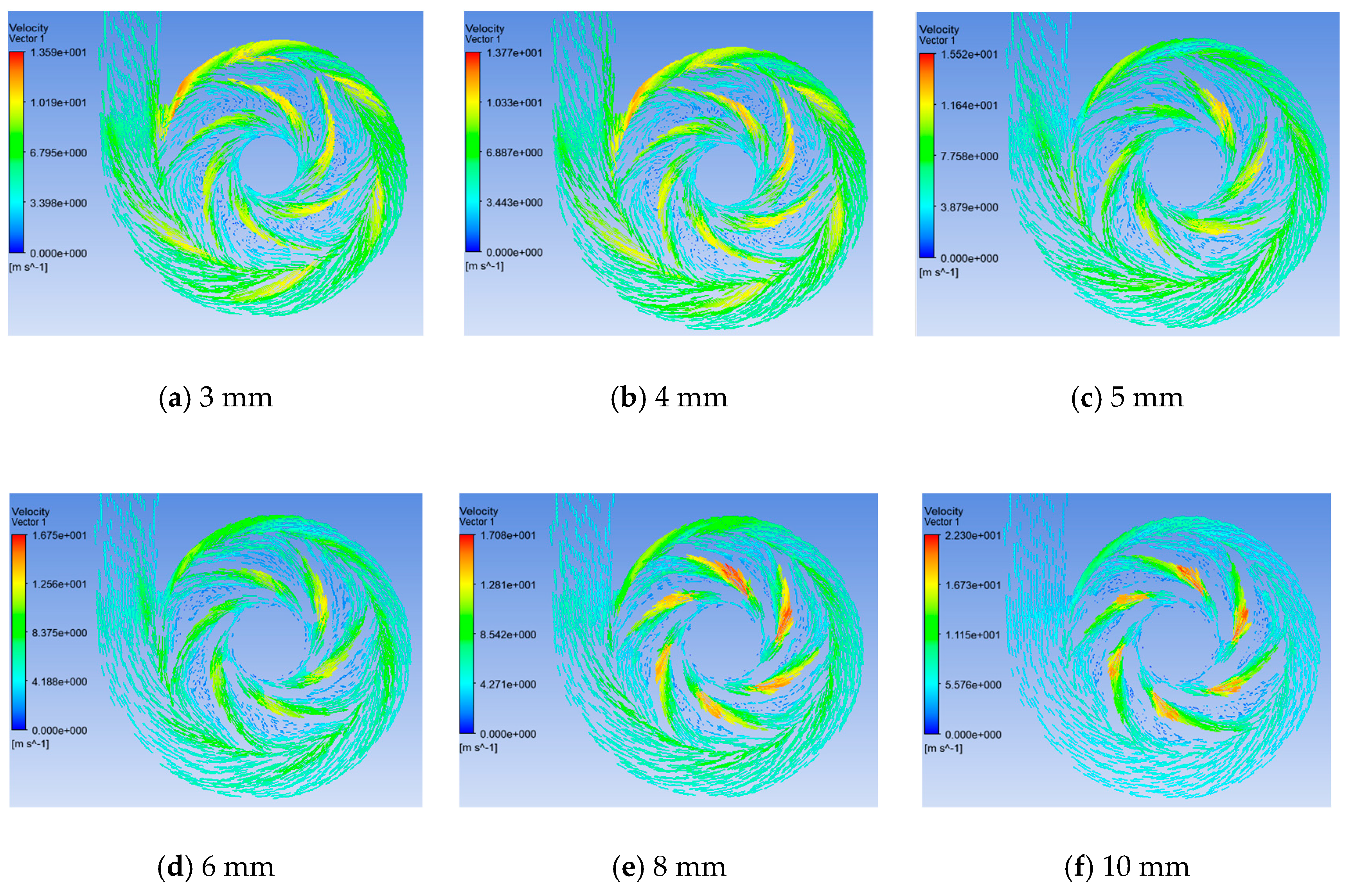





| Variables | Value |
|---|---|
| Inlet diameter (mm) | 80 |
| Outlet diameter (mm) | 200 |
| Inlet width (mm) | 27 |
| Outlet width (mm) | 17 |
| Inlet angle (°) | 19 |
| Outlet angle (°) | 33 |
| Wrap angle (°) | 123 |
| Number of blades | 6 |
| Scheme | No. of Mesh Cells (×104) | Head (m) | Deviation of Head (%) |
|---|---|---|---|
| 1 | 61 | 9.87 | 8 |
| 2 | 75 | 10.42 | 2.3 |
| 3 | 89 | 10.68 | 0.2 |
| 4 | 114 | 10.69 | 0.3 |
| 5 | 142 | 10.66 | - |
| Inlet Section | Impeller | Volute | Outlet Section | |
|---|---|---|---|---|
| No. of cells | 67922 | 498486 | 278164 | 51302 |
| Orthogonality | 0.77–1 | 0.271–1 | 0.251–1 | 0.758–1 |
| Skewness | 0.63–1 | 0.214–1 | 0.236–1 | 0.589–1 |
| Aspect ratio | 0.038–0.989 | 0.015–0.98 | 0.015–0.998 | 0.018–1 |
| Min angle | 52.48 | 18.22 | 19.94 | 50.52 |
| Quality | 0.732 | 0.341 | 0.284 | 0.742 |
| Instruments | Unit | Accuracy | Uncertainty Value |
|---|---|---|---|
| Electromagnetic flowmeter | m3/h | ±0.5% | ±0.05 |
| Pressure transducer (inlet) | MPa | ±0.5% | ±0.005 |
| Pressure transducer (outlet) | MPa | ±0.5% | ±0.0005 |
| Tachometer | rpm | ±0.1% | ±3 |
| Scheme | Thickness of Leading Edge (mm) | Thickness of Trailing Edge (mm) |
|---|---|---|
| VTB 4–6 | 4 | 6 |
| VTB 4–8 | 4 | 8 |
| VTB 4–10 | 4 | 10 |
| VTB 4–12 | 4 | 12 |
| VTB 4–14 | 4 | 14 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, Z.; Kong, F.; Tang, L.; Liu, M.; Wang, J.; Qiu, N. Effect of Blade Thickness on Internal Flow and Performance of a Plastic Centrifugal Pump. Machines 2022, 10, 61. https://doi.org/10.3390/machines10010061
Xu Z, Kong F, Tang L, Liu M, Wang J, Qiu N. Effect of Blade Thickness on Internal Flow and Performance of a Plastic Centrifugal Pump. Machines. 2022; 10(1):61. https://doi.org/10.3390/machines10010061
Chicago/Turabian StyleXu, Zhenfa, Fanyu Kong, Lingfeng Tang, Mingwei Liu, Jiaqiong Wang, and Ning Qiu. 2022. "Effect of Blade Thickness on Internal Flow and Performance of a Plastic Centrifugal Pump" Machines 10, no. 1: 61. https://doi.org/10.3390/machines10010061
APA StyleXu, Z., Kong, F., Tang, L., Liu, M., Wang, J., & Qiu, N. (2022). Effect of Blade Thickness on Internal Flow and Performance of a Plastic Centrifugal Pump. Machines, 10(1), 61. https://doi.org/10.3390/machines10010061







